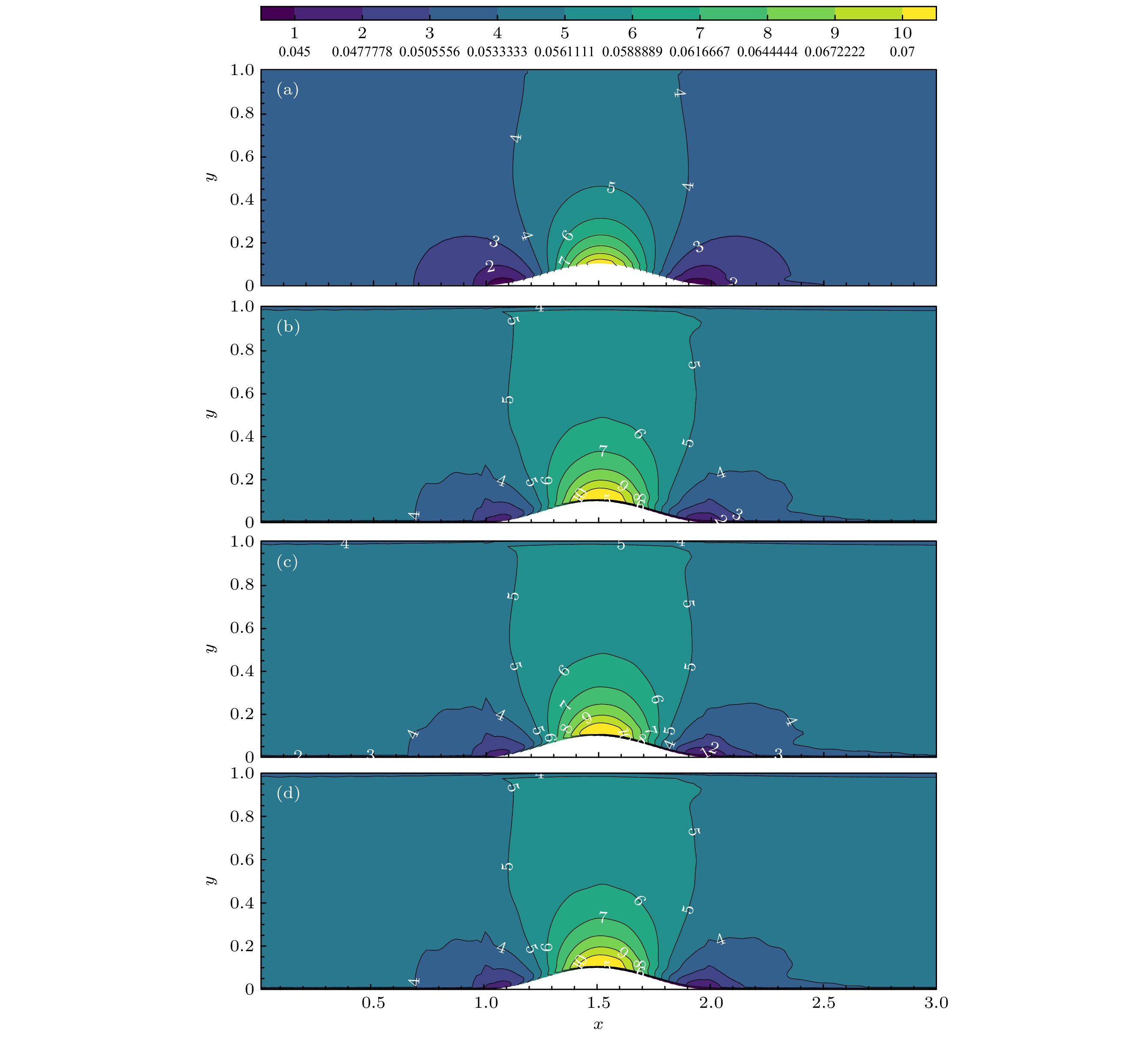
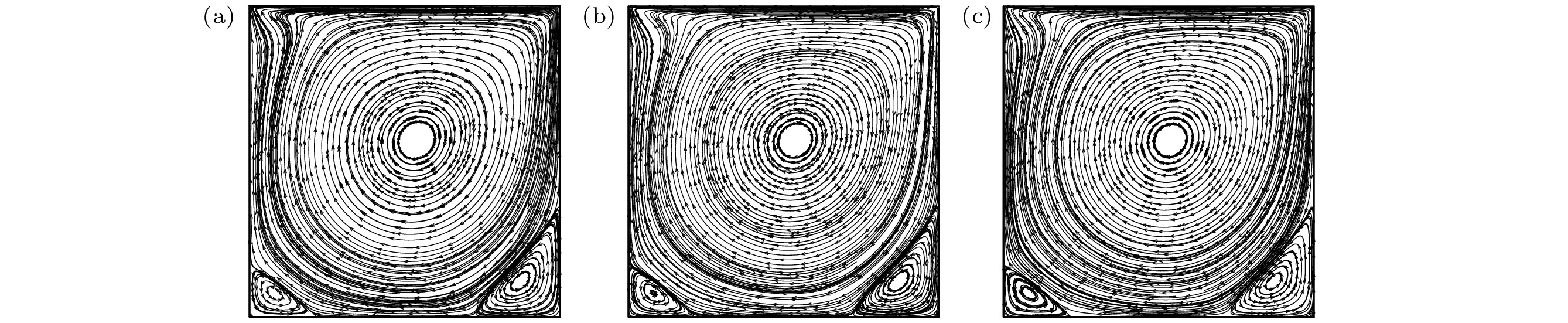
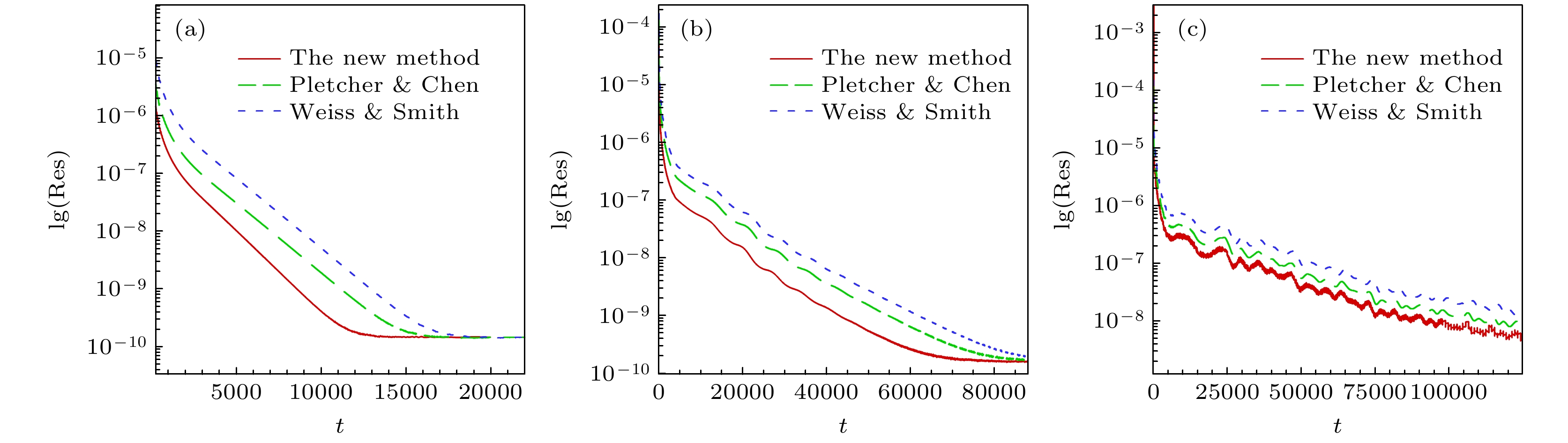
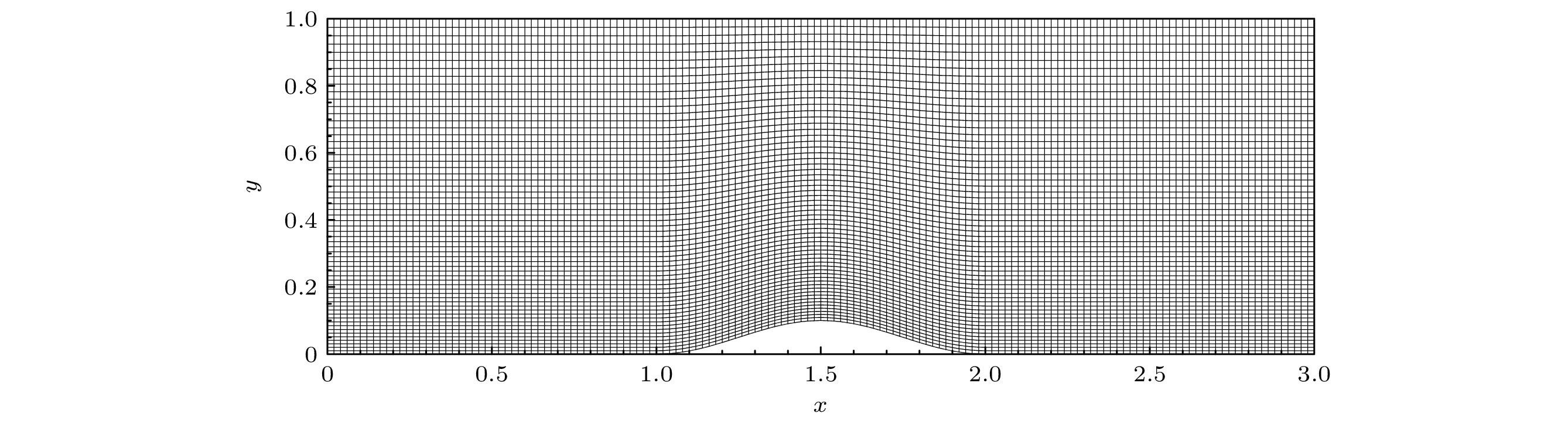
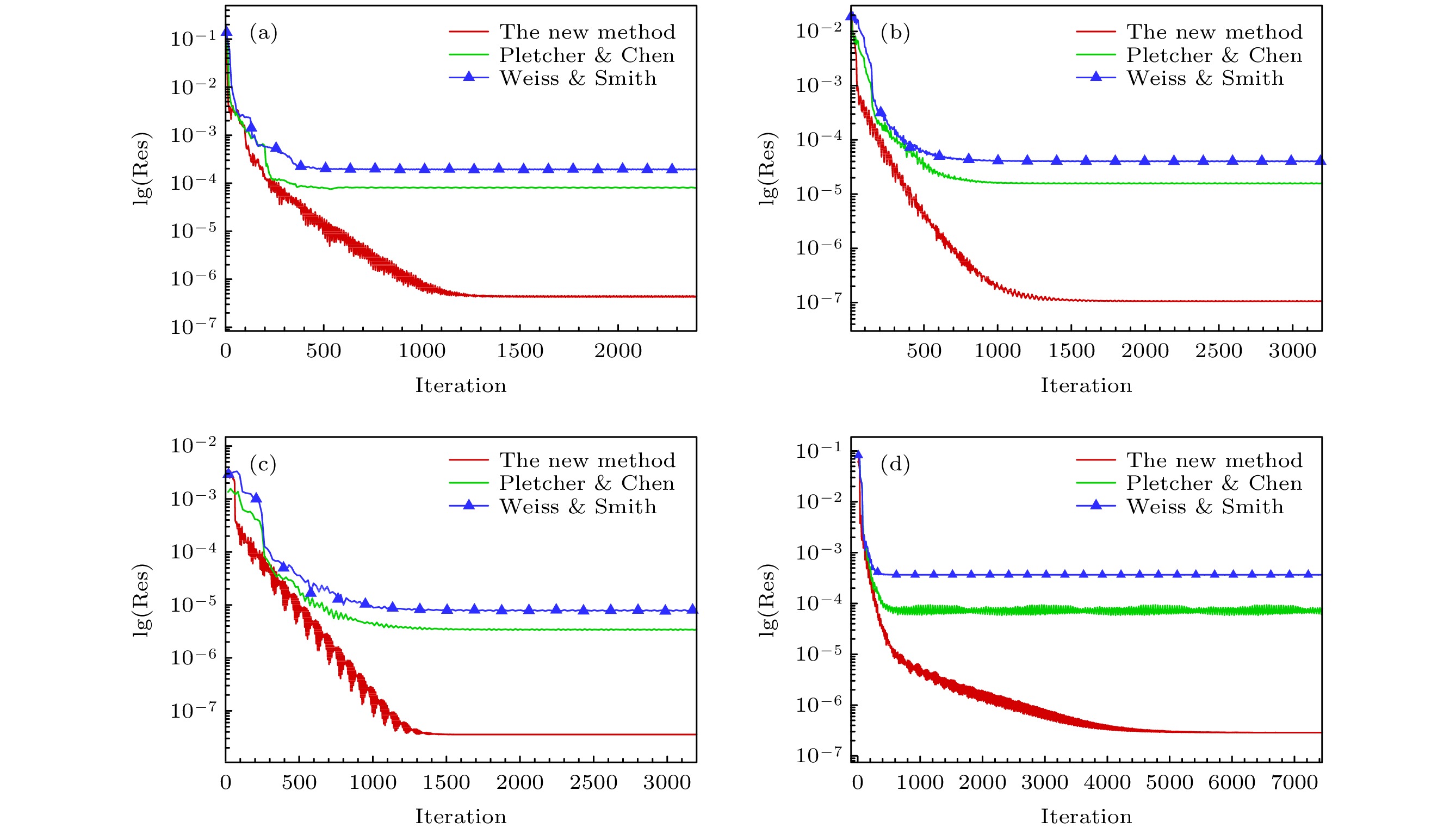
-
Low velocity flows often exhibit incompressible properties, and one of the most prohibitive aspects of these problems is a large number of computer resources required, including both CPU time and memory. Various numerical schemes used to calculate incompressible flow are constantly updated to accelerate convergence and reduce resource occupation, but incompressible flow is an ideal model for studying theoretical problems after all. In addition, it is a common phenomenon that high-speed and low-speed flow regions exist in the same system, and the influence of heat and volume force cannot be ignored in some cases. The artificial compressibility method is based on the idea that the numerical algorithms for compressible flows are used to solve incompressible flow. The system of compressible flow governing equations at very low Mach numbers is stiff due to the large disparity in acoustic wave speed, u + c, and the waves convecting at fluid speed, u. The preconditioning algorithm is effective to change the eigenvalues of the compressible flow equations system so as to remove the large disparity in wave speed, and the essence is to multiply the time derivatives with a suitable matrix. A function in low growth rate with Mach number as a variable is used to construct another new preconditioning matrix. Compared with other matrices of Dailey, Weiss, Choi and Pletcher, the new matrix can well improve the stiffness of the governing equations and the smoothness of eigenvalues in all-speed domain. A one-dimensional numerical example shows that the preconditioning matrix has ability to improve the efficiency of solving low-speed flow problems. These preconditioning matrices are extended to two-dimensional problems to simulate inviscid flow passing through a pipe with bulge and viscous flows passing through a flat and cavity. The results indicate that the new matrix has not only better accuracy but also higher efficiency than Weiss’s and Pletcher’s.
-
Keywords:
- preconditioning /
- compressible flow /
- astringency /
- numerical simulation
[1] Yin P, Liandrat J, Shen W 2021 J. Comput. Phys. 434 110207
Google Scholar
[2] Fernandez Fidalgo J, Nogueira X, Ramirez L, Colominas I 2018 Comput. Methods Appl. Mech. Eng. 335 91
Google Scholar
[3] Ahmed T, Rehman A, Ali A, Qamar S 2021 Results Phys. 23 104078
Google Scholar
[4] Zhang Y, Liu Y, Zhang Y, Wang W R, Han Y F 2021 Int. Commun. Heat. Mass Transfer 129 105688
Google Scholar
[5] Xie W J, Tian Z Y, Zhang Y, Yu H 2022 Comput. Fluids 233 105215
Google Scholar
[6] Ma Chao, Wu J, Yu H, Yang L 2022 Comput. Math. Appl. 105 13
Google Scholar
[7] Lehel F, Hona J 2021 Chin. J. Phys. 73 360
Google Scholar
[8] 张庆海, 李阳 2021 70 130201
Google Scholar
Zhang Q H, Li Y 2021 Acta Phys. Sin. 70 130201
Google Scholar
[9] Xie W, Luo Z B, Zhou Y, Gao T X, Wu Y, Wang Q 2021 Acta. Astronaut. 188 416
Google Scholar
[10] Barhoumi B, Bessrour J 2017 J. Comput. Phys. 21 86
Google Scholar
[11] Bykerk T, Verstraete D, Steelant J 2020 Aerosp. Sci. Technol. 103 105883
Google Scholar
[12] Huber S E, Trummer M R 2016 Comput. Math. Appl. 71 319
Google Scholar
[13] 胡嘉懿, 张文欢, 柴振华, 施保昌, 汪一航 2019 68 234701
Google Scholar
Hu J Y, Zhang W H, Chai Z H, Shi B C, Wang Y H 2019 Acta Phys. Sin. 68 234701
Google Scholar
[14] 辛建建, 陈振雷, 石凡, 石伏龙 2020 69 044702
Google Scholar
Xin J J, Chen Z L, Shi F, Shi F L 2020 Acta Phys. Sin. 69 044702
Google Scholar
[15] Imran M A, Shaheen A, Sherif E M, Rahimi-Gorji M, Seikh A H 2020 Chin. J. Phys. 66 60
Google Scholar
[16] 尹纪富, 尤云祥, 李巍, 胡天群 2014 63 044701
Google Scholar
Yin J F, You Y X, Li W, Hu T Q 2014 Acta Phys. Sin. 63 044701
Google Scholar
[17] 牛海波, 易仕和, 刘小林, 霍俊杰, 冈敦殿 2021 70 134701
Google Scholar
Niu H B, Yi S H, Liu X L, Huo J J, Gang D D 2021 Acta Phys. Sin. 70 134701
Google Scholar
[18] 李高华, 王福新 2018 67 054701
Google Scholar
Li G H, Wang F X 2018 Acta Phys. Sin. 67 054701
Google Scholar
[19] Fasel M 1976 J. Fluid. Mech. 78 355
Google Scholar
[20] Aziz K 1967 Phys. Fluids 10 314
Google Scholar
[21] Harlow F H, Welch J E 1965 Phys. Fluids 8 2182
Google Scholar
[22] Hirt C W, Nichols B D, Romero N C 1974 SOLA: A Numerical Solution Algorithm for Transient Fluid Flows (Los Alamos: Los Alamos Scientific Laboratory) Report5852
[23] Patankar S V, Spalding D B 1972 Int. J. Heat. Mass Transfer 15 1787
Google Scholar
[24] Patankar S V 1981 Numer. Heat Transfer 4 409
Google Scholar
[25] Van Doormaal J P, Raithby G D 1984 Numer. Heat Transfer 7 147
Google Scholar
[26] Minkowycz W J, Sparrow E M, Pletcher R H, Schneider G E 1988 Handbook of Numerical Heat Transfer (New York: John Wiley & Sons) pp241–289
[27] Issa I R, Gosman A D, Watkins A P 1986 J. Comput. Phys. 62 66
Google Scholar
[28] Davis G D V 1983 Int. J. Numer. Methods Fluids 3 249
Google Scholar
[29] Chorin A J 1967 J. Comput. Phys. 2 12
Google Scholar
[30] Turkel E 1993 Appl. Numer. Math. 12 257
Google Scholar
[31] Choi D, Merkel C L 1987 AIAA J. 23 581
[32] Viviand H 1985 Numerical Methods for the Euler Equations of Fluid Dynamics (Philadelphia: SIAM) p519
[33] Choi Y H, Merkle C L 1993 J. Comput. Phys. 105 205
[34] Buelow P E O, Venkateswaran S, Charles L 1993 AIAA J. 32 2401
Google Scholar
[35] Godfrey A G, Walters R W, Leer B V 1993 31st Aerospace Sciences Meeting Reno, NV, USA, January 11–14, 1993 p535
[36] Weiss J M, Smith W A 1994 Fluid Dynamics Conference Colorado Springs, CO, USA, June 20–23, 1994 p2209
[37] Pletcher R H, Chen K H 1993 11th Computational Fluid Dynamics Conference Orlando, FL, USA, July 6–9, 1993 p3368
[38] Dailey L D, Pletcher R H 1996 Comput. Fluids 25 791
Google Scholar
[39] 成小磊 2007 硕士学位论文 (南京: 南京航空航天大学)
Cheng X L 2007 M. S. Thesis (Nanjing: Nanjing University of Aeronautics and Astronautics) (in Chinese)
[40] 谭勤学, 任静, 蒋洪德 2015 清华大学学报(自然科学版) 55 134
Google Scholar
Tan Q X, Ren J, Jiang H D 2015 J. Tsinghua Univ. (Science and Technology) 55 134
Google Scholar
[41] Buelow P E G, Schwer D A, Feng F J, Merkle C L 1997 13th Computational Fluid Dynamics Conference Snowmass Village, CO, USA, June 29–July 2, 1997 p120
[42] Roe P 1981 J. Comput. Phys. 43 357
Google Scholar
[43] Jiang G S, Shu C W 1996 J. Comput. Phys. 126 202
Google Scholar
[44] Sun Z S, Yu H U, Luo L, Yang Z W 2014 Sci. Chin. Phys. Mech. 57 971
Google Scholar
[45] Shu C W, Osher S 1988 J. Comput. Phys. 77 439
Google Scholar
[46] 胡丽燕 2011 硕士学位论文 (南京: 南京航空航天大学)
Hu L Y 2011 M. S. Thesis (Nanjing: Nanjing University of Aeronautics and Astronautics) (in Chinese)
[47] Ghia U, Ghia K N, Shin C T 1982 J. Comput. Phys. 48 387
Google Scholar
[48] Delery J 1981 14th Fluid and Plasma Dynamics Conference Palo Alto, CA, USA, June 23–25, 1981 p1245
-
图 1 经不同预处理方法后的特征系统(对于每种预处理后的速度有, U' = (λ3+λ4)/2, C' = |λ3–λ4|/2, 均为无量纲化速) (a) 条件数CN; (b) 伪速度U'; (c) 伪声速C'; (d) B函数; (e) 本文预处理法得到的特征根
Figure 1. Characteristic system of the governing equations obtained after different preconditioning: (a) Condition number; (b) pseudo-speed; (c) pseudo-sound speed; (d) function B; (e) the eigenvalues obtained after preconditioning in this paper
图 2 数值方法得到的解曲线与精确解曲线 (a) k = 0.5; (b) k = 0.04; (c) k = 0.01; (d) k = 5×10–3; (e) k = 1×10–3; (f) k = 2×10–4
Figure 2. Solution curves obtained by numerical solution and exact solution curves: (a) k = 0.5; (b) k = 0.04; (c) k = 0.01; (d) k = 5×10–3; (e) k = 1×10–3; (f) k = 2×10–4
图 3 Ma = 0.1时边界层流动的速度剖面
Figure 3. Velocity profile of the boundary layer flow when Ma = 0.1.
图 4 边界层流动的收敛历程
Figure 4. Convergence histories of the boundary layer flow when Ma = 0.1.
图 5 Re = 100时的空腔流线图 (a) 本文方法; (b) Weiss & Smith方法; (c) Pletcher & Chen方法
Figure 5. Velocity streamline diagram of cavity, Re = 100: (a) The new method; (b) method of Weiss & Smith; (c) method of Pletcher & Chen.
图 6 Re = 1000时的空腔流线图 (a) 本文方法; (b) Weiss & Smith方法; (c) Pletcher & Chen方法
Figure 6. Velocity streamline diagram of cavity, Re = 1000: (a) The new method; (b) method of Weiss & Smith; (c) method of Pletcher & Chen.
图 7 Re = 10000时的空腔流线图 (a) 本文方法; (b) Weiss & Smith方法; (c) Pletcher & Chen方法
Figure 7. Velocity streamline diagram of cavity, Re = 10000: (a) The new method; (b) method of Weiss & Smith; (c) method of Pletcher & Chen.
图 8 本文预处理后的中心线速度分布 (a)水平中心线上的速度v; (b)垂直中心线上的速度u
Figure 8. Velocity along lines through geometric center caculated by the new method: (a) v-velocity along horizontal center line; (b) v-velocity along horizontal center line.
图 9 方腔流动的收敛历程对比 (a) Re = 100; (b) Re = 1000; (c) Re = 10000
Figure 9. Convergence histories of the the laminar flow in a square cavity: (a) Re = 100; (b) Re = 1000; (c) Re = 10000.
图 10 二维鼓包流动问题的计算网格
Figure 10. Computational grid for the two-dimensional bump flow problem.
图 11 在不同Mach数下计算二维凸包管道问题的收敛历程 (a) Ma0 = 0.01; (b) Ma0 = 0.05; (c) Ma0 = 0.1; (d) Ma0 = 0.2
Figure 11. Convergence histories of the two-dimensional bump flow problem under different Mach numbers: (a) Ma0 = 0.01; (b) Ma0 = 0.05; (c) Ma0 = 0.1; (d) Ma0 = 0.2.
图 12 Ma0 = 0.05时流场的等Mach线 (a) 精确解; (b) Weiss & Smith; (c) Pletcher & Chen; (d) 本文方法
Figure 12. Mach contour of the two-dimensional bump flow problem, Ma0 = 0.05: (a) Exact solution; (b) Weiss & Smith; (c) Pletcher & Chen; (d) the new method in this paper.
表 1 数值解与精确解之间的误差
Table 1. Errors between numerical solutions and exact solutions.
k t x = 0.2 x = 0.4 x = 0.6 x = 0.8 x = 1.0 0.5 exact solution 0.89253 1.0425 1.1925 1.3050 1.4925 errors of no
preconditioning100 3.9029×10–5 1.5695×10–5 2.5871×10–5 3.4728×10–5 4.2505×10–5 150 1.1399×10–5 9.3383×10–6 1.3694×10–5 1.8359×10–5 2.2459×10–5 200 6.7822×10–6 4.6281×10–6 7.3170×10–6 5.8126×10–6 6.0973×10–6 errors of
preconditioning100 3.0175×10–6 9.8264×10–7 2.2705×10–6 1.8797×10–6 2.8140×10–6 0.04 exact solution 0.52790 0.53622 0.54454 0.55286 0.56118 errors of no preconditioning 100 9.3060×10–5 9.3411×10–5 9.3782×10–5 9.4174×10–5 9.4586×10–5 150 5.5757×10–5 5.5289×10–5 5.4841×10–5 5.4415×10–5 5.4010×10–5 200 4.5265×10–5 4.4483×10–5 4.3722×10–5 4.2983×10–5 4.2267×10–5 errors of
preconditioning100 3.9460×10–6 3.1288×10–6 5.5530×10–7 2.5512×10–6 3.1288×10–6 0.01 exact solution 0.50692 0.50894 0.51096 0.51298 0.51500 errors of no
preconditioning100 4.0176×10–4 4.0335×10–4 4.0494×10–4 4.0652×10–4 4.0811×10–4 150 2.6008×10–4 2.6108×10–4 2.6208×10–4 2.6308×10–4 2.6407×10–4 200 1.9108×10–4 1.9178×10–4 1.9249×10–4 1.9319×10–4 1.9389×10–4 100 3.3569×10–6 2.2214×10–6 7.5880×10–6 4.8149×10–6 6.3518×10–6 
DownLoad: CSV
-
[1] Yin P, Liandrat J, Shen W 2021 J. Comput. Phys. 434 110207
Google Scholar
[2] Fernandez Fidalgo J, Nogueira X, Ramirez L, Colominas I 2018 Comput. Methods Appl. Mech. Eng. 335 91
Google Scholar
[3] Ahmed T, Rehman A, Ali A, Qamar S 2021 Results Phys. 23 104078
Google Scholar
[4] Zhang Y, Liu Y, Zhang Y, Wang W R, Han Y F 2021 Int. Commun. Heat. Mass Transfer 129 105688
Google Scholar
[5] Xie W J, Tian Z Y, Zhang Y, Yu H 2022 Comput. Fluids 233 105215
Google Scholar
[6] Ma Chao, Wu J, Yu H, Yang L 2022 Comput. Math. Appl. 105 13
Google Scholar
[7] Lehel F, Hona J 2021 Chin. J. Phys. 73 360
Google Scholar
[8] 张庆海, 李阳 2021 70 130201
Google Scholar
Zhang Q H, Li Y 2021 Acta Phys. Sin. 70 130201
Google Scholar
[9] Xie W, Luo Z B, Zhou Y, Gao T X, Wu Y, Wang Q 2021 Acta. Astronaut. 188 416
Google Scholar
[10] Barhoumi B, Bessrour J 2017 J. Comput. Phys. 21 86
Google Scholar
[11] Bykerk T, Verstraete D, Steelant J 2020 Aerosp. Sci. Technol. 103 105883
Google Scholar
[12] Huber S E, Trummer M R 2016 Comput. Math. Appl. 71 319
Google Scholar
[13] 胡嘉懿, 张文欢, 柴振华, 施保昌, 汪一航 2019 68 234701
Google Scholar
Hu J Y, Zhang W H, Chai Z H, Shi B C, Wang Y H 2019 Acta Phys. Sin. 68 234701
Google Scholar
[14] 辛建建, 陈振雷, 石凡, 石伏龙 2020 69 044702
Google Scholar
Xin J J, Chen Z L, Shi F, Shi F L 2020 Acta Phys. Sin. 69 044702
Google Scholar
[15] Imran M A, Shaheen A, Sherif E M, Rahimi-Gorji M, Seikh A H 2020 Chin. J. Phys. 66 60
Google Scholar
[16] 尹纪富, 尤云祥, 李巍, 胡天群 2014 63 044701
Google Scholar
Yin J F, You Y X, Li W, Hu T Q 2014 Acta Phys. Sin. 63 044701
Google Scholar
[17] 牛海波, 易仕和, 刘小林, 霍俊杰, 冈敦殿 2021 70 134701
Google Scholar
Niu H B, Yi S H, Liu X L, Huo J J, Gang D D 2021 Acta Phys. Sin. 70 134701
Google Scholar
[18] 李高华, 王福新 2018 67 054701
Google Scholar
Li G H, Wang F X 2018 Acta Phys. Sin. 67 054701
Google Scholar
[19] Fasel M 1976 J. Fluid. Mech. 78 355
Google Scholar
[20] Aziz K 1967 Phys. Fluids 10 314
Google Scholar
[21] Harlow F H, Welch J E 1965 Phys. Fluids 8 2182
Google Scholar
[22] Hirt C W, Nichols B D, Romero N C 1974 SOLA: A Numerical Solution Algorithm for Transient Fluid Flows (Los Alamos: Los Alamos Scientific Laboratory) Report5852
[23] Patankar S V, Spalding D B 1972 Int. J. Heat. Mass Transfer 15 1787
Google Scholar
[24] Patankar S V 1981 Numer. Heat Transfer 4 409
Google Scholar
[25] Van Doormaal J P, Raithby G D 1984 Numer. Heat Transfer 7 147
Google Scholar
[26] Minkowycz W J, Sparrow E M, Pletcher R H, Schneider G E 1988 Handbook of Numerical Heat Transfer (New York: John Wiley & Sons) pp241–289
[27] Issa I R, Gosman A D, Watkins A P 1986 J. Comput. Phys. 62 66
Google Scholar
[28] Davis G D V 1983 Int. J. Numer. Methods Fluids 3 249
Google Scholar
[29] Chorin A J 1967 J. Comput. Phys. 2 12
Google Scholar
[30] Turkel E 1993 Appl. Numer. Math. 12 257
Google Scholar
[31] Choi D, Merkel C L 1987 AIAA J. 23 581
[32] Viviand H 1985 Numerical Methods for the Euler Equations of Fluid Dynamics (Philadelphia: SIAM) p519
[33] Choi Y H, Merkle C L 1993 J. Comput. Phys. 105 205
[34] Buelow P E O, Venkateswaran S, Charles L 1993 AIAA J. 32 2401
Google Scholar
[35] Godfrey A G, Walters R W, Leer B V 1993 31st Aerospace Sciences Meeting Reno, NV, USA, January 11–14, 1993 p535
[36] Weiss J M, Smith W A 1994 Fluid Dynamics Conference Colorado Springs, CO, USA, June 20–23, 1994 p2209
[37] Pletcher R H, Chen K H 1993 11th Computational Fluid Dynamics Conference Orlando, FL, USA, July 6–9, 1993 p3368
[38] Dailey L D, Pletcher R H 1996 Comput. Fluids 25 791
Google Scholar
[39] 成小磊 2007 硕士学位论文 (南京: 南京航空航天大学)
Cheng X L 2007 M. S. Thesis (Nanjing: Nanjing University of Aeronautics and Astronautics) (in Chinese)
[40] 谭勤学, 任静, 蒋洪德 2015 清华大学学报(自然科学版) 55 134
Google Scholar
Tan Q X, Ren J, Jiang H D 2015 J. Tsinghua Univ. (Science and Technology) 55 134
Google Scholar
[41] Buelow P E G, Schwer D A, Feng F J, Merkle C L 1997 13th Computational Fluid Dynamics Conference Snowmass Village, CO, USA, June 29–July 2, 1997 p120
[42] Roe P 1981 J. Comput. Phys. 43 357
Google Scholar
[43] Jiang G S, Shu C W 1996 J. Comput. Phys. 126 202
Google Scholar
[44] Sun Z S, Yu H U, Luo L, Yang Z W 2014 Sci. Chin. Phys. Mech. 57 971
Google Scholar
[45] Shu C W, Osher S 1988 J. Comput. Phys. 77 439
Google Scholar
[46] 胡丽燕 2011 硕士学位论文 (南京: 南京航空航天大学)
Hu L Y 2011 M. S. Thesis (Nanjing: Nanjing University of Aeronautics and Astronautics) (in Chinese)
[47] Ghia U, Ghia K N, Shin C T 1982 J. Comput. Phys. 48 387
Google Scholar
[48] Delery J 1981 14th Fluid and Plasma Dynamics Conference Palo Alto, CA, USA, June 23–25, 1981 p1245
DownLoad:
Catalog
Metrics
- Abstract views: 4620
- PDF Downloads: 74
- Cited By: 0
