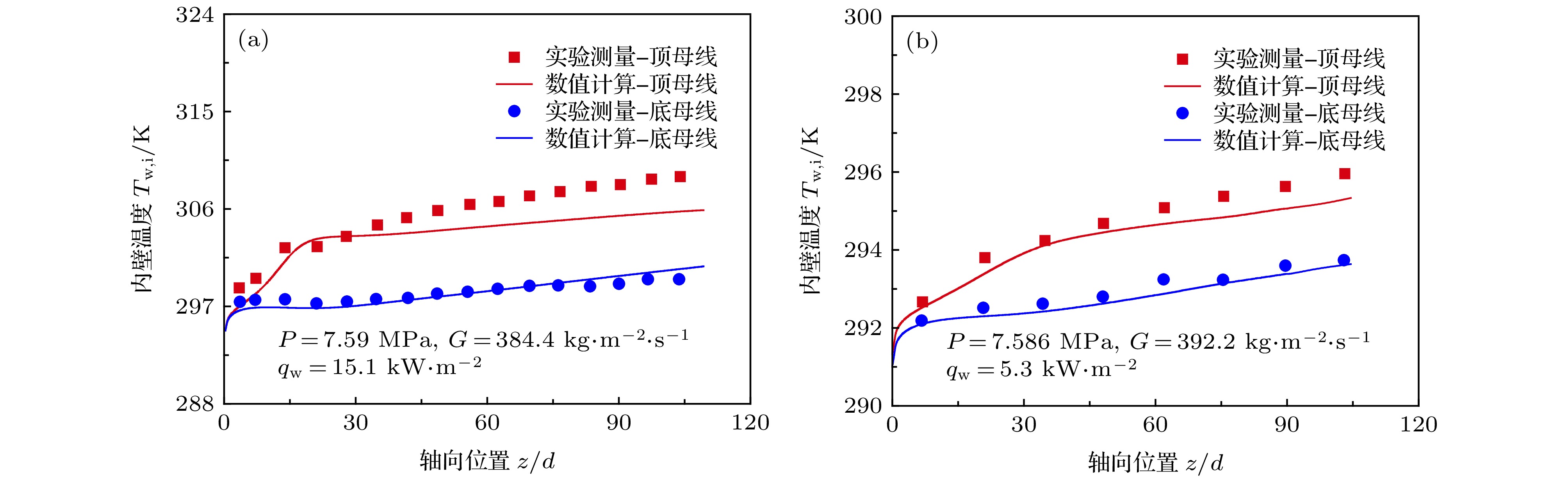
-
采用SST k-ω低雷诺数湍流模型对加热条件下超临界压力CO2在内径di = 22.14 mm, 加热长度Lh = 2440 mm水平圆管内三维稳态流动与传热特性进行了数值计算. 通过超临界CO2在水平圆管内的流动传热实验数据验证了数值模型的可靠性和准确性. 首先, 研究了超临界压力CO2在水平圆管内的流动传热特点, 基于超临界CO2在类临界温度Tpc处发生类液-类气“相变”的假设, 揭示了水平圆管顶母线和底母线区域不同的流动传热行为. 然后, 分析了热流密度qw和质量流速G对水平圆管内超临界压力CO2流动换热的影响, 通过获取流体域内的物性分布、速度分布和湍流分布等详细信息, 重点解释了不同热流密度qw和质量流速G下顶母线内壁温度Tw,i分布产生差异的传热机理, 分析结果确定了类气膜厚度δ、类气膜性质、轴向速度u和湍动能k是影响顶母线壁温分布差异的主要因素. 研究结果可以为超临界压力CO2换热装置的优化设计和安全运行提供理论指导.In the present study, the three-dimensional steady-state numerical simulation has been performed by using ANSYS Fluent15.0 with SST k-ω low Reynolds turbulence model to study flow and heat transfer characteristics for supercritical CO2 in the horizontal straight tube with inner diameter di = 22.14 mm and heating length Lh = 2440 mm under heating condition. The reliability and accuracy of the numerical model was verified by the experimental data of flow and heat transfer of supercritical CO2 in horizontal tube. Firstly, flow and heat transfer characteristics of supercritical CO2 was studied in horizontal tube. Based on the assumption that the supercritical CO2 will undergoes “phase transition” between liquid-like and vapor-like at pseudocritical temperature Tpc, the differences between top generatrix and bottom generatrix of horizontal tube at flow and heat transfer behaviors were revealed. The results show flow and heat transfer characteristics of supercritical CO2 in horizontal tube are similar to those under subcritical pressure. Then, the influences of heat flux qw and mass flux G on flow and heat transfer of supercritical CO2 were analyzed. The higher heat flux qw is or the smaller mass flux G is, the higher inner wall temperature Tw,i at top generatrix is. The reasons for difference in the distribution of inner wall temperature Tw,i at top generatrix under different heat flux qw and mass flux G were explained by capturing detailed information about thermophysical properties distribution including specific heat at constant pressure cp and thermal conductivity λ, axial velocity distribution and turbulent kinetic energy distribution in the fluid domain. It is observed that vapor-like film thickness δ, vapor-like film property characterized by specific heat at constant pressure cp and thermal conductivity λ, axial velocity u and turbulent kinetic energy k are the main factors affecting the difference in inner wall temperature distribution at top generatrix. The present work can provide a theoretical guidance for design and safe operation of heat exchanger for supercritical CO2 Brayton cycle.
-
Keywords:
- supercritical CO2 /
- horizontal flow /
- heat transfer /
- numerical simulation
[1] Dostal V 2004 Ph.D. Dissertation (Massachusetts: Massachusetts Institute of Technology
[2] Al-Sulaiman F A, Atif M 2015 Energy 82 61
Google Scholar
[3] Li M J, Zhu H H, Guo J Q, Wang K, Tao W Q 2017 Appl. Therm. Eng. 126 255
Google Scholar
[4] Xu J L, Sun E H, Li M J, Liu H, Zhu B G 2018 Energy 157 227
Google Scholar
[5] Kim H Y, Kim H R, Kang D J, Song J H, Bae Y Y 2008 Nucl. Eng. Technol. 40 155
Google Scholar
[6] Kim D E, Kim M H 2010 Nucl. Eng. Des. 240 3336
Google Scholar
[7] Li Z H, Jiang P X, Zhao C R, Zhang Y 2010 Exp. Therm. Fluid Sci. 34 1162
Google Scholar
[8] Kim D E, Kim M H 2011 Int. J. Heat Fluid Flow 32 176
Google Scholar
[9] Jiang P X, Zhang Y, Xu Y J, Shi R F 2008 Int. J. Therm. Sci. 47 998
Google Scholar
[10] Liu S H, Huang Y P, Wang J F, Leung L K H 2018 Int. J. Heat Mass Transfer 117 595
Google Scholar
[11] Zhu B G, Xu J L, Wu X M, Xie J, Li M J 2019 Int. J. Therm. Sci. 136 254
Google Scholar
[12] Adebiyi G A, Hall W B 1976 Int. J. Heat Mass Transfer 19 715
Google Scholar
[13] Pidaparti S R, McFarland J A, Mikhaeil M M, Anderson M H, Ranjan D 2015 Nucl. Eng. Radiat. Sci. 1 031001
Google Scholar
[14] Chu X, Laurien E 2016 J. Supercrit. Fluids 116 172
Google Scholar
[15] Wang J H, Guo P C, Yan J G, Zhu F L, Luo X Q 2019 Adv. Mech. Eng. 11 1687814019830804
[16] Tanimizu K, Sadr R 2016 Heat Mass Transfer 52 713
Google Scholar
[17] Kim T H, Kwon J G, Kim M H, Park H S 2018 Exp. Therm. Fluid Sci. 92 222
Google Scholar
[18] Wang K Z, Xu X X, Wu Y Y, Liu C, Dang C B 2015 J. Supercrit. Fluids 99 112
Google Scholar
[19] Menter F R 1994 AIAA J. 32 1598
Google Scholar
[20] Holman J P, Rea S N, Howard C E 1965 Int. J. Heat Mass Transfer 8 1095
Google Scholar
[21] Banuti D T 2015 J. Supercrit. Fluids 98 12
Google Scholar
[22] 韩布兴 2010 超临界流体科学与技术(北京: 中国石化出版社) 第4页
Han B X 2010 Supercritical Fluid Science and Technology (Beijing: China Petrochemical Press) p4 (in Chinese)
[23] Yang Z, Peng X F, Ye P 2008 Int. J. Heat Mass Transfer 51 1003
Google Scholar
[24] Da R E, Del C D 2012 J. Heat Transfer 134 051019
Google Scholar
[25] 徐济鋆 2001 沸腾传热和气液两相流(北京: 原子能出版社) 第16—19页
Xu J J 2001 Boiling Heat Transfer and Gas-liquid Two-phase Flow (Beijing: Atomic Energy Press) pp16−19 (in Chinese)
[26] Yang C Y, Xu J L, Wang X D, Zhang W 2013 Int. J. Heat Mass Transfer 64 212
Google Scholar
[27] Wang X C, Xiang M J, Huo H J, Liu Q 2018 Appl. Therm. Eng. 141 775
Google Scholar
[28] 闫晨帅, 朱兵国, 尹少军, 孙恩慧, 徐进良, 刘欢 2019 中国科学: 技术科学
Google Scholar
Yan C S, Z hu, B G, Yin S J, Sun E H, Xu J L, Liu H 2019 Sci. Sin. Tech.
Google Scholar
-
图 3 不同压力下, S-CO2热物性曲线 (a) 定压比热cp; (b) 密度ρ; (c) 导热系数λ; (d) 黏度μ
Fig. 3. Thermophysical properties of S-CO2 under different pressures: (a) Specific heat at constant pressure cp; (b) density ρ; (c) thermal conductivity λ; (d) viscosity μ.
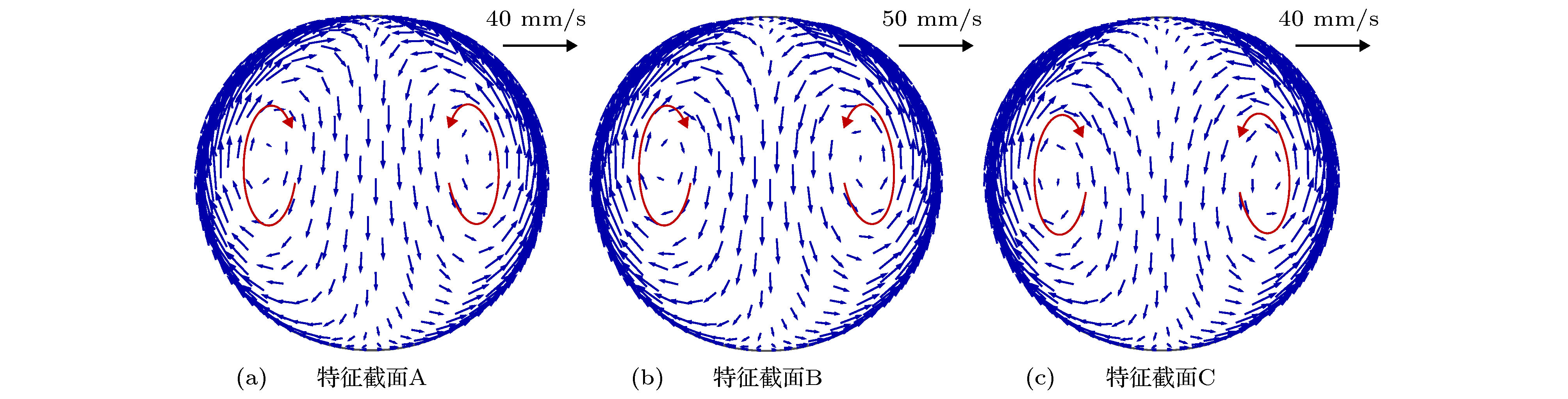
图 5 水平圆管内S-CO2传热特性典型曲线 (a) 顶母线与底母线内壁温Tw,i和传热系数h轴向分布; (b) 类气膜厚度δ周向分布
Fig. 5. Typical curves of heat transfer characteristics for S-CO2 in horizontal tube: (a) Axial distribution of inner wall temperature and heat transfer coefficient at top generatrix and bottom generatrix; (b) circumferential distribution of vapor-like film thickness.
图 6 特征横截面内矢量速度分布
Fig. 6. Vector velocity distribution in the characteristic cross-sections.
图 8 水平圆管内S-CO2轴向速度沿径向分布
Fig. 8. Radial distribution of axial velocity for S-CO2 in horizontal tube.
图 9 热流密度qw对壁温和二次流强度的影响
Fig. 9. Influences of heat flux on wall temperature and second flow intensity.
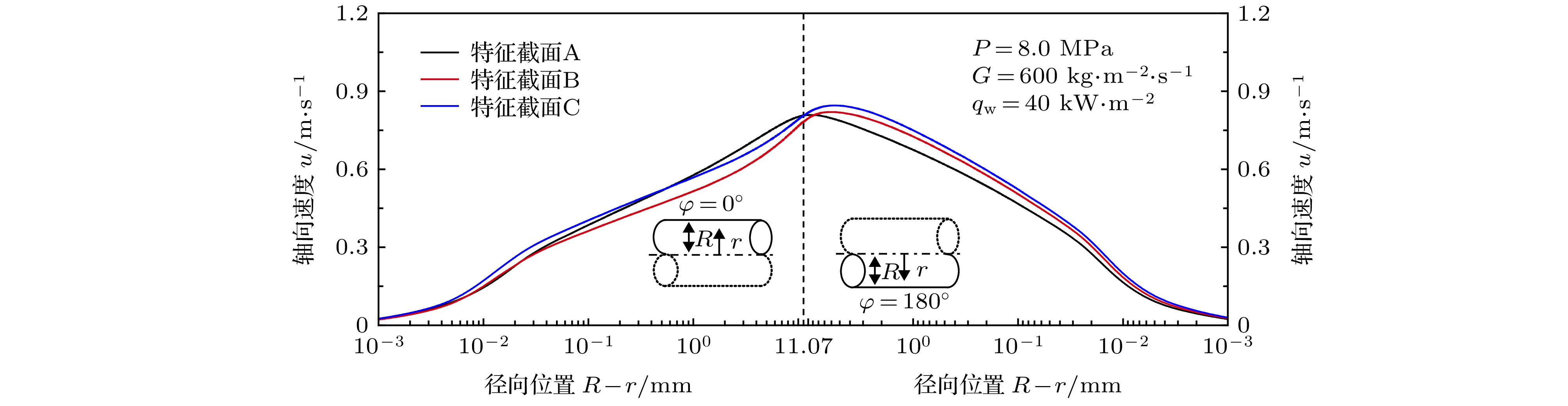
图 10 不同热流密度时, 水平圆管特征截面内热物性、轴向速度和湍动能沿径向分布曲线 (a) 定压比热cp; (b) 导热系数λ; (c) 轴向速度u; (d) 湍动能k
Fig. 10. Radial distribution curves of thermophyical properties in the characteristic cross-sections under different heat flux: (a) Specific heat at constant pressure cp; (b) thermal conductivity λ; (c) axial velocity u; (d) turbulent kinetic energy k.
图 11 质量流速G对壁温和二次流强度的影响
Fig. 11. Effects of mass flux on wall temperature and second flow intensity.
图 12 不同质量流速下, 水平圆管特征截面内热物性、轴向速度和湍动能沿径向分布曲线 (a) 定压比热cp; (b) 导热系数λ; (c) 轴向速度u; (d) 湍动能k
Fig. 12. Radial distribution curves of thermophyical properties in the characteristic cross-sections under different mass flux: (a) Specific heat at constant pressure cp; (b) thermal conductivity λ; (c) axial velocity u; (d) turbulent kinetic energy k.
-
[1] Dostal V 2004 Ph.D. Dissertation (Massachusetts: Massachusetts Institute of Technology
[2] Al-Sulaiman F A, Atif M 2015 Energy 82 61
Google Scholar
[3] Li M J, Zhu H H, Guo J Q, Wang K, Tao W Q 2017 Appl. Therm. Eng. 126 255
Google Scholar
[4] Xu J L, Sun E H, Li M J, Liu H, Zhu B G 2018 Energy 157 227
Google Scholar
[5] Kim H Y, Kim H R, Kang D J, Song J H, Bae Y Y 2008 Nucl. Eng. Technol. 40 155
Google Scholar
[6] Kim D E, Kim M H 2010 Nucl. Eng. Des. 240 3336
Google Scholar
[7] Li Z H, Jiang P X, Zhao C R, Zhang Y 2010 Exp. Therm. Fluid Sci. 34 1162
Google Scholar
[8] Kim D E, Kim M H 2011 Int. J. Heat Fluid Flow 32 176
Google Scholar
[9] Jiang P X, Zhang Y, Xu Y J, Shi R F 2008 Int. J. Therm. Sci. 47 998
Google Scholar
[10] Liu S H, Huang Y P, Wang J F, Leung L K H 2018 Int. J. Heat Mass Transfer 117 595
Google Scholar
[11] Zhu B G, Xu J L, Wu X M, Xie J, Li M J 2019 Int. J. Therm. Sci. 136 254
Google Scholar
[12] Adebiyi G A, Hall W B 1976 Int. J. Heat Mass Transfer 19 715
Google Scholar
[13] Pidaparti S R, McFarland J A, Mikhaeil M M, Anderson M H, Ranjan D 2015 Nucl. Eng. Radiat. Sci. 1 031001
Google Scholar
[14] Chu X, Laurien E 2016 J. Supercrit. Fluids 116 172
Google Scholar
[15] Wang J H, Guo P C, Yan J G, Zhu F L, Luo X Q 2019 Adv. Mech. Eng. 11 1687814019830804
[16] Tanimizu K, Sadr R 2016 Heat Mass Transfer 52 713
Google Scholar
[17] Kim T H, Kwon J G, Kim M H, Park H S 2018 Exp. Therm. Fluid Sci. 92 222
Google Scholar
[18] Wang K Z, Xu X X, Wu Y Y, Liu C, Dang C B 2015 J. Supercrit. Fluids 99 112
Google Scholar
[19] Menter F R 1994 AIAA J. 32 1598
Google Scholar
[20] Holman J P, Rea S N, Howard C E 1965 Int. J. Heat Mass Transfer 8 1095
Google Scholar
[21] Banuti D T 2015 J. Supercrit. Fluids 98 12
Google Scholar
[22] 韩布兴 2010 超临界流体科学与技术(北京: 中国石化出版社) 第4页
Han B X 2010 Supercritical Fluid Science and Technology (Beijing: China Petrochemical Press) p4 (in Chinese)
[23] Yang Z, Peng X F, Ye P 2008 Int. J. Heat Mass Transfer 51 1003
Google Scholar
[24] Da R E, Del C D 2012 J. Heat Transfer 134 051019
Google Scholar
[25] 徐济鋆 2001 沸腾传热和气液两相流(北京: 原子能出版社) 第16—19页
Xu J J 2001 Boiling Heat Transfer and Gas-liquid Two-phase Flow (Beijing: Atomic Energy Press) pp16−19 (in Chinese)
[26] Yang C Y, Xu J L, Wang X D, Zhang W 2013 Int. J. Heat Mass Transfer 64 212
Google Scholar
[27] Wang X C, Xiang M J, Huo H J, Liu Q 2018 Appl. Therm. Eng. 141 775
Google Scholar
[28] 闫晨帅, 朱兵国, 尹少军, 孙恩慧, 徐进良, 刘欢 2019 中国科学: 技术科学
Google Scholar
Yan C S, Z hu, B G, Yin S J, Sun E H, Xu J L, Liu H 2019 Sci. Sin. Tech.
Google Scholar
下载:
计量
- 文章访问数: 10378
- PDF下载量: 176
- 被引次数: 0
