-
为构造一类扰动Kadomtsev-Petviashvili (KP)方程的级数解, 利用同伦近似对称法求出三种情形下具有通式形式的相似解以及相应的相似方程. 而且, 对于第三种情形下的前几个相似方程, 雅可比椭圆函数解亦遵循共同的表达式, 这可以产生形式紧凑的级数解, 从而为收敛性的探讨提供便利: 首先, 对于扰动KP方程的微扰项, 给定
$u$ 关于变量$y$ 的导数阶数$n$ , 若$n\leqslant 1$ ($n\geqslant 3$ ), 则减小(增大)$|a/b|$ 致使收敛性改善; 其次, 减小$\varepsilon$ ,$|\theta-1|$ 以及$|c|$ 均有助于改进收敛性. 在更一般情形下, 仅当微扰项的导数阶数为偶数时, 扰动KP方程才存在雅可比椭圆函数解.-
关键词:
- 同伦近似对称法 /
- 扰动Kadomtsev-Petviashvili方程 /
- 级数解 /
- 收敛性
This paper is devoted to constructing series solutions to one kind of perturbed Kadomtsev-Petviashvili (KP) equations, of which the perturbation terms are of all six-order derivatives of space variable$x$ and$y$ . First, by making the series solutions expansion with respect to the homotopy parameter$q$ , the homotopy model of the perturbed KP equations can be decomposed into infinite number of approximate equations of the general form. Second, Lie symmetry method is applied to these approximate equations to achieve similarity solutions and the related similarity equations with common formulae in three cases. Third, for the first few similarity equations in the third case, Jacobi elliptic function solutions are constructed through a step-by-step procedure and are also subject to common formulae for each equation of the whole kind of perturbed KP equations. Finally, one kind of compact series solutions for the original perturbed KP equations is obtained from these Jacobi elliptic function solutions. The convergence of these series solution is dependent on perturbation parameter$\epsilon$ , auxiliary parameter$\theta$ and arbitrary constants$\{a, b, c\}$ , among which the most prominent is decreasing arbitrary constant$c$ or perturbation parameter$\varepsilon$ . For the perturbation term in perturbed KP equations, given the derivative order$n$ of$u$ with respect to$y$ , smaller (greater)$|a/b|$ causes the improved convergence provided$n\leqslant 1$ ($n\geqslant 3$ ). Nonetheless, the decrease of arbitrary constant$|c|$ or$|a/b|$ leads to the enlargement of period in a certain direction and thus should be specified appropriately. This paper also considers the perturbed KP equations with more general perturbation terms. Only if the derivative order of the perturbation term is an even number, do Jacobi elliptic function series solutions exist for perturbed KP equations. The existence of series solutions can serve as a criterion of solvability for perturbed equations.-
Keywords:
- approximate homotopy symmetry method /
- perturbed Kadomtsev-Petviashvili equation /
- series solutions /
- convergence
[1] Cole J D 1968 Perturbation Methods in Applied Mathematics (Waltham: Blaisdell)
[2] Van Dyke M 1975 Perturbation Methods in Fluid Mechanics (Stanford: Parabolic Press)
[3] Bluman G W, Kumei S 1989 Symmetries and Differential Equations (Berlin: Springer)
[4] Olver P J 1993 Applications of Lie Group to Differential Equations (2nd ed.) (New York: Springer)
[5] Bluman G W, Cole J D 1969 J. Math. Mech. 18 1025
[6] Clarkson P A, Kruskal M D 1989 J. Math. Phys. 30 2201
Google Scholar
[7] Lou S Y, Ma H C 2005 J. Phys. A, Math. Gen. 38 L129
Google Scholar
[8] Lou S Y, Jia M, Tand X Y, Huang F 2007 Phys. Rev. E 75 056318
Google Scholar
[9] Lou S Y, Hu X R, Chen Y 2012 J. Phys. A: Math. Theor. 45 155209
Google Scholar
[10] Cheng X P, Lou S Y, Chen C L, Tang X Y 2014 Phys. Rev. E 89 043202
Google Scholar
[11] Chen J C, Xin X P, Chen Y 2014 J. Math. Phys. 55 053508
Google Scholar
[12] Baikov V A, Gazizov R K, Ibragimov N H 1988 Mat. Sb. 136 435(English Transl. in 1989 Math. USSR Sb. 64 427)
[13] Fushchich W I, Shtelen W M 1989 J. Phys. A: Math. Gen. 22 L887
Google Scholar
[14] Pakdemirli M, Yurusoy M, Dolapci I T 2004 Acta Appl. Math. 80 243
Google Scholar
[15] Wiltshire R 2006 J. Comput. Appl. Math. 197 287
Google Scholar
[16] Jiao X Y, Yao R X, Lou S Y 2008 J. Math. Phys. 49 093505
Google Scholar
[17] Jia M, Wang J Y, Lou S Y 2009 Chin. Phys. Lett. 26 020201
Google Scholar
[18] Jiao X Y 2018 Chin. Phys. B 27 100202
Google Scholar
[19] Liao S J 1999 Int. J. Non-Linear Mech. 34 759
Google Scholar
[20] Liao S J 1999 Int. J. Non-linear Dynam. 19 93
Google Scholar
[21] Liao S J 2002 J. Fluid Mech. 453 411
Google Scholar
[22] Lyapunov A M 1992 General Problem on Stability of Motion (English translation) (London: Taylor and Francis) p29
[23] Karmishin A V, Zhukov A I, Kolosov V G 1990 Methods of Dynamics Calculation and Testing for Thin Walled Structures (Moscow: Mashinostroyenie) (in Russian) p52
[24] Adomian G 1994 Solving Frontier Problems of Physics: The Decomposition Method (Boston and London: Kluwer Academic Publishers)
[25] 焦小玉, 高原, 楼森岳 2009 中国科学 G辑: 物理学 力学 天文学 39 964
Jiao X Y, Gao Y, Lou S Y 2009 Sci. China Ser. G. Phys. Mech. Astron. 39 964
[26] Jiao X Y, Yao R X, Lou S Y 2009 Chin. Phys. Lett. 26 040202
Google Scholar
[27] Kadomtsev B B, Petviashvili V I 1970 Sov. Phys.: Dokl. 15 539
[28] Lou S Y 2015 Stud. Appl. Math. 134 372
Google Scholar
-
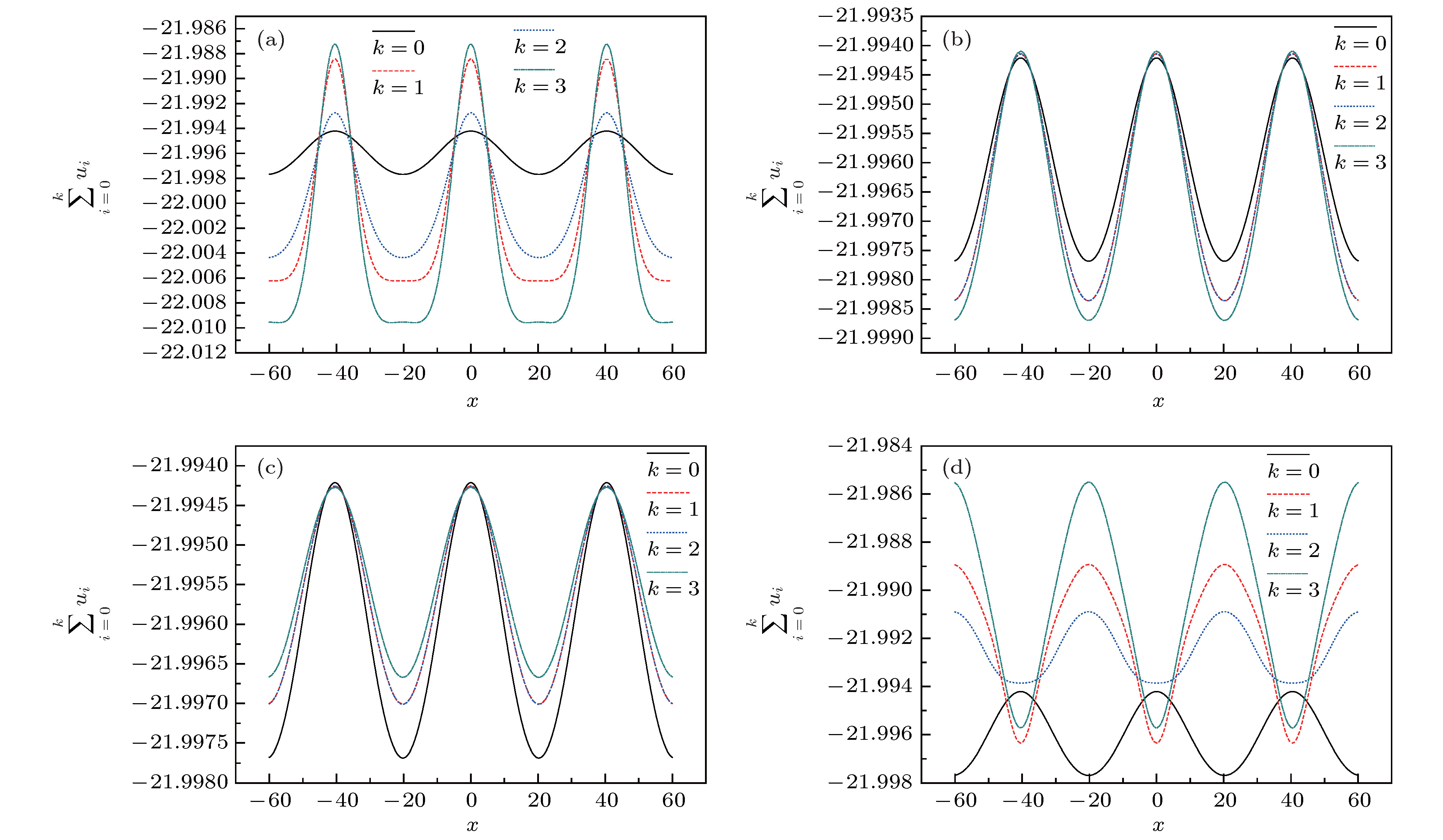
图 1 当n = 1, α = 1, h(t) = t, m = 0.5, c = 1, ε = 0.1, a = 2b并且t = 0, y = 0时, 截断级数解
$\sum\nolimits_{i = 0}^k {{u_k}\left( {k = 0,1,2,3} \right)} $ 的截线图Fig. 1. Transversals of truncated series solution
$\sum\nolimits_{i = 0}^k {{u_k}} $ for$\left( {k = 0,1,2,3} \right)$ when n = 1, α = 1, h(t) = t, m = 0.5, c = 1, ε = 0.1, a = 2b and t = 0, y = 0
图 4 当n = 3, α = 1, h(t) = t, m = 0.5, c = 1, ε = 0.1, a = b/2并且t = 0, y = 0时, 截断级数解
$\sum\nolimits_{i = 0}^k {{u_k}\left( {k = 0,1,2,3} \right)}$ 的截线图Fig. 4. Transversals of truncated series solution
$\sum\nolimits_{i = 0}^k {{u_k}}$ for$\left( {k = 0,1,2,3} \right)$ when n = 3, α = 1, h(t) = t, m = 0.5, c = 1, ε = 0.1, a = b/2 and t = 0, y = 0
图 2 当n = 1, α = 1, h(t) = t, m = 0.5, c = 1, ε = 0.1, a = b/2并且t = 0, y = 0时, 截断级数解
$\sum\nolimits_{i = 0}^k {{u_k}\left( {k = 0,1,2,3} \right)} $ 的截线图Fig. 2. Transversals of truncated series solution
$\sum\nolimits_{i = 0}^k {{u_k}}$ for$\left( {k = 0,1,2,3} \right)$ when n = 1, α = 1, h(t) = t, m = 0.5, c = 1, ε = 0.1, a = b/2 and t = 0, y = 0
图 3 当n = 3, α = 1, h(t) = t, m = 0.5, c = 1, ε = 0.1, a = 2b并且t = 0, y = 0时, 截断级数解
$\sum\nolimits_{i = 0}^k {{u_k}\left( {k = 0,1,2,3} \right)}$ 的截线图Fig. 3. Transversals of truncated series solution
$\sum\nolimits_{i = 0}^k {{u_k}}$ for$\left( {k = 0,1,2,3} \right)$ when n = 3, α = 1, h(t) = t, m = 0.5, c = 1, ε = 0.1, a = 2b and t = 0, y = 0 -
[1] Cole J D 1968 Perturbation Methods in Applied Mathematics (Waltham: Blaisdell)
[2] Van Dyke M 1975 Perturbation Methods in Fluid Mechanics (Stanford: Parabolic Press)
[3] Bluman G W, Kumei S 1989 Symmetries and Differential Equations (Berlin: Springer)
[4] Olver P J 1993 Applications of Lie Group to Differential Equations (2nd ed.) (New York: Springer)
[5] Bluman G W, Cole J D 1969 J. Math. Mech. 18 1025
[6] Clarkson P A, Kruskal M D 1989 J. Math. Phys. 30 2201
Google Scholar
[7] Lou S Y, Ma H C 2005 J. Phys. A, Math. Gen. 38 L129
Google Scholar
[8] Lou S Y, Jia M, Tand X Y, Huang F 2007 Phys. Rev. E 75 056318
Google Scholar
[9] Lou S Y, Hu X R, Chen Y 2012 J. Phys. A: Math. Theor. 45 155209
Google Scholar
[10] Cheng X P, Lou S Y, Chen C L, Tang X Y 2014 Phys. Rev. E 89 043202
Google Scholar
[11] Chen J C, Xin X P, Chen Y 2014 J. Math. Phys. 55 053508
Google Scholar
[12] Baikov V A, Gazizov R K, Ibragimov N H 1988 Mat. Sb. 136 435(English Transl. in 1989 Math. USSR Sb. 64 427)
[13] Fushchich W I, Shtelen W M 1989 J. Phys. A: Math. Gen. 22 L887
Google Scholar
[14] Pakdemirli M, Yurusoy M, Dolapci I T 2004 Acta Appl. Math. 80 243
Google Scholar
[15] Wiltshire R 2006 J. Comput. Appl. Math. 197 287
Google Scholar
[16] Jiao X Y, Yao R X, Lou S Y 2008 J. Math. Phys. 49 093505
Google Scholar
[17] Jia M, Wang J Y, Lou S Y 2009 Chin. Phys. Lett. 26 020201
Google Scholar
[18] Jiao X Y 2018 Chin. Phys. B 27 100202
Google Scholar
[19] Liao S J 1999 Int. J. Non-Linear Mech. 34 759
Google Scholar
[20] Liao S J 1999 Int. J. Non-linear Dynam. 19 93
Google Scholar
[21] Liao S J 2002 J. Fluid Mech. 453 411
Google Scholar
[22] Lyapunov A M 1992 General Problem on Stability of Motion (English translation) (London: Taylor and Francis) p29
[23] Karmishin A V, Zhukov A I, Kolosov V G 1990 Methods of Dynamics Calculation and Testing for Thin Walled Structures (Moscow: Mashinostroyenie) (in Russian) p52
[24] Adomian G 1994 Solving Frontier Problems of Physics: The Decomposition Method (Boston and London: Kluwer Academic Publishers)
[25] 焦小玉, 高原, 楼森岳 2009 中国科学 G辑: 物理学 力学 天文学 39 964
Jiao X Y, Gao Y, Lou S Y 2009 Sci. China Ser. G. Phys. Mech. Astron. 39 964
[26] Jiao X Y, Yao R X, Lou S Y 2009 Chin. Phys. Lett. 26 040202
Google Scholar
[27] Kadomtsev B B, Petviashvili V I 1970 Sov. Phys.: Dokl. 15 539
[28] Lou S Y 2015 Stud. Appl. Math. 134 372
Google Scholar
下载:
计量
- 文章访问数: 6375
- PDF下载量: 42
- 被引次数: 0
