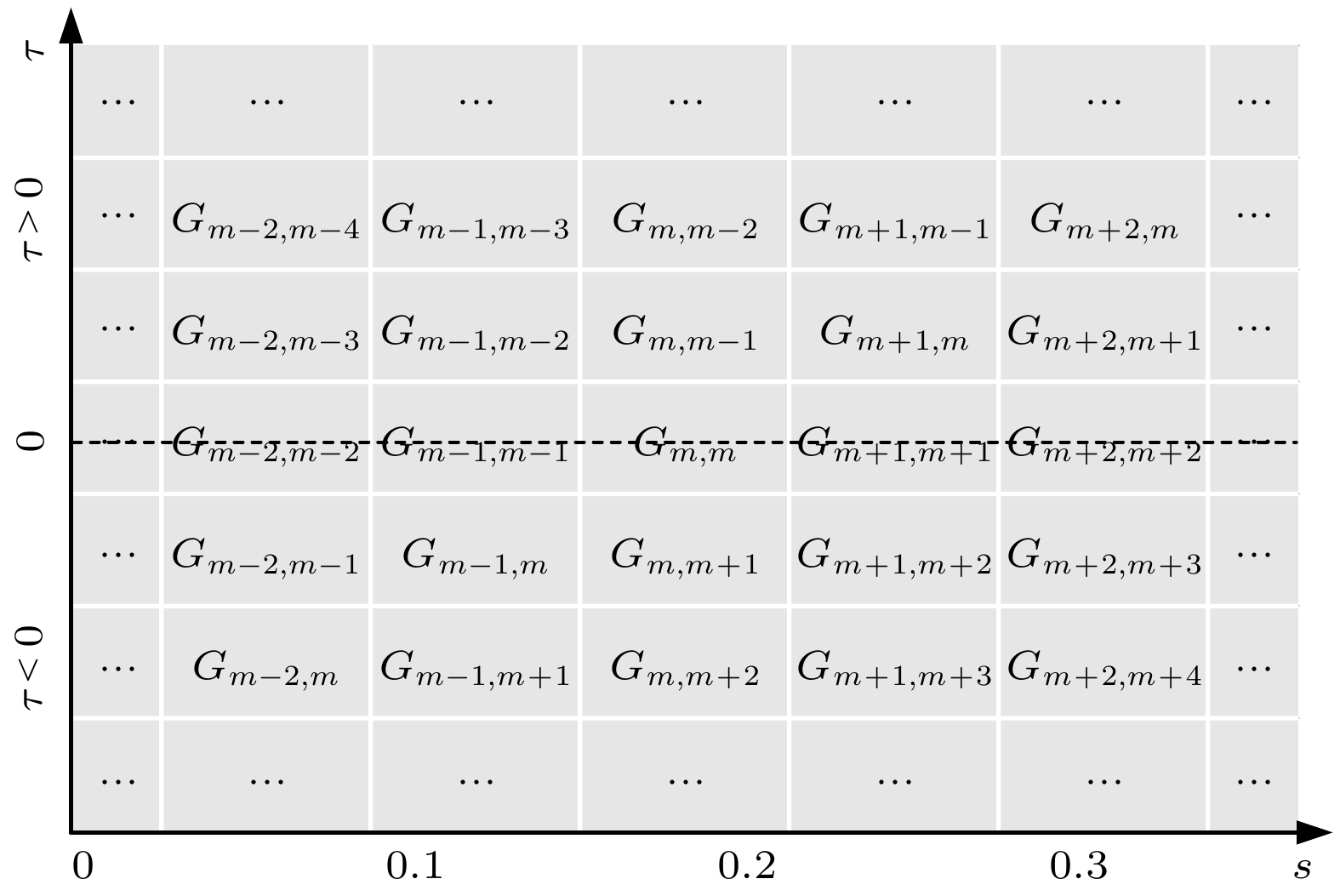
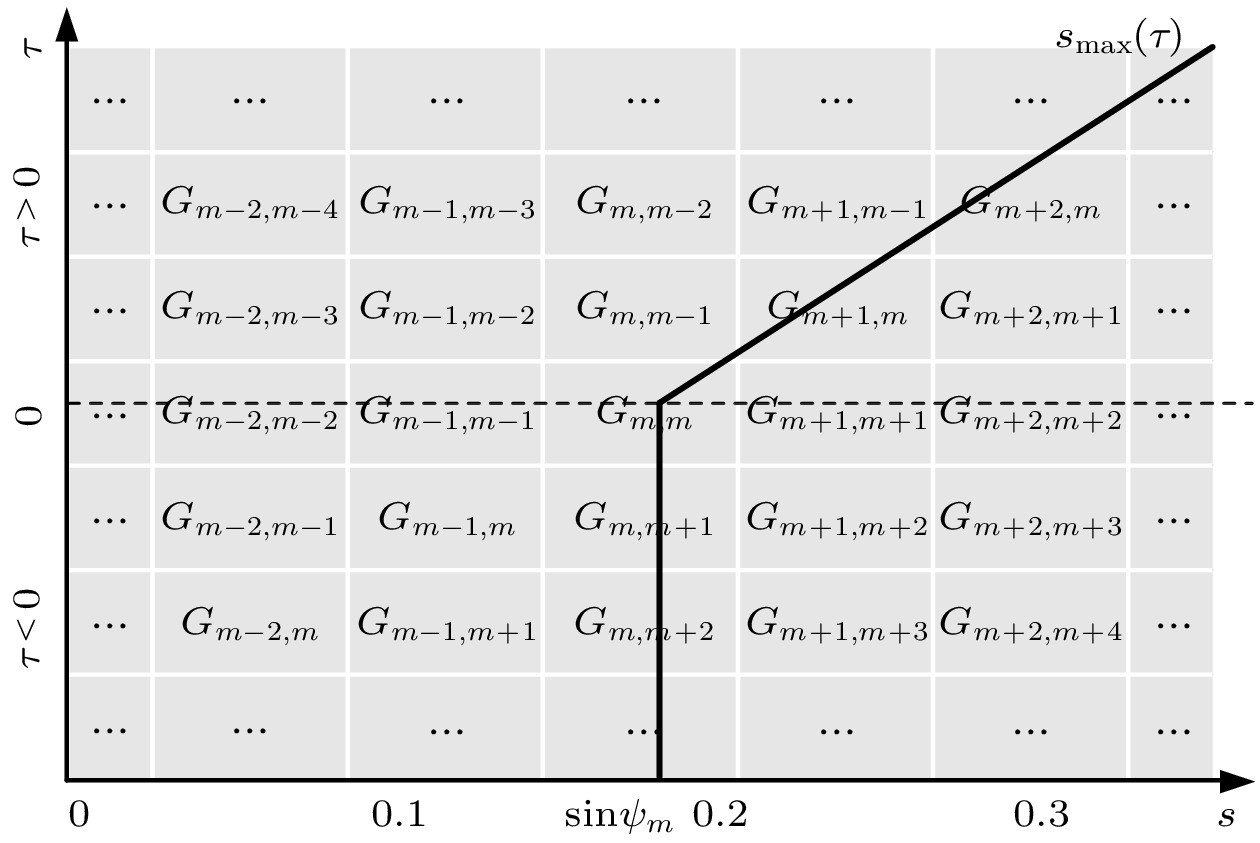
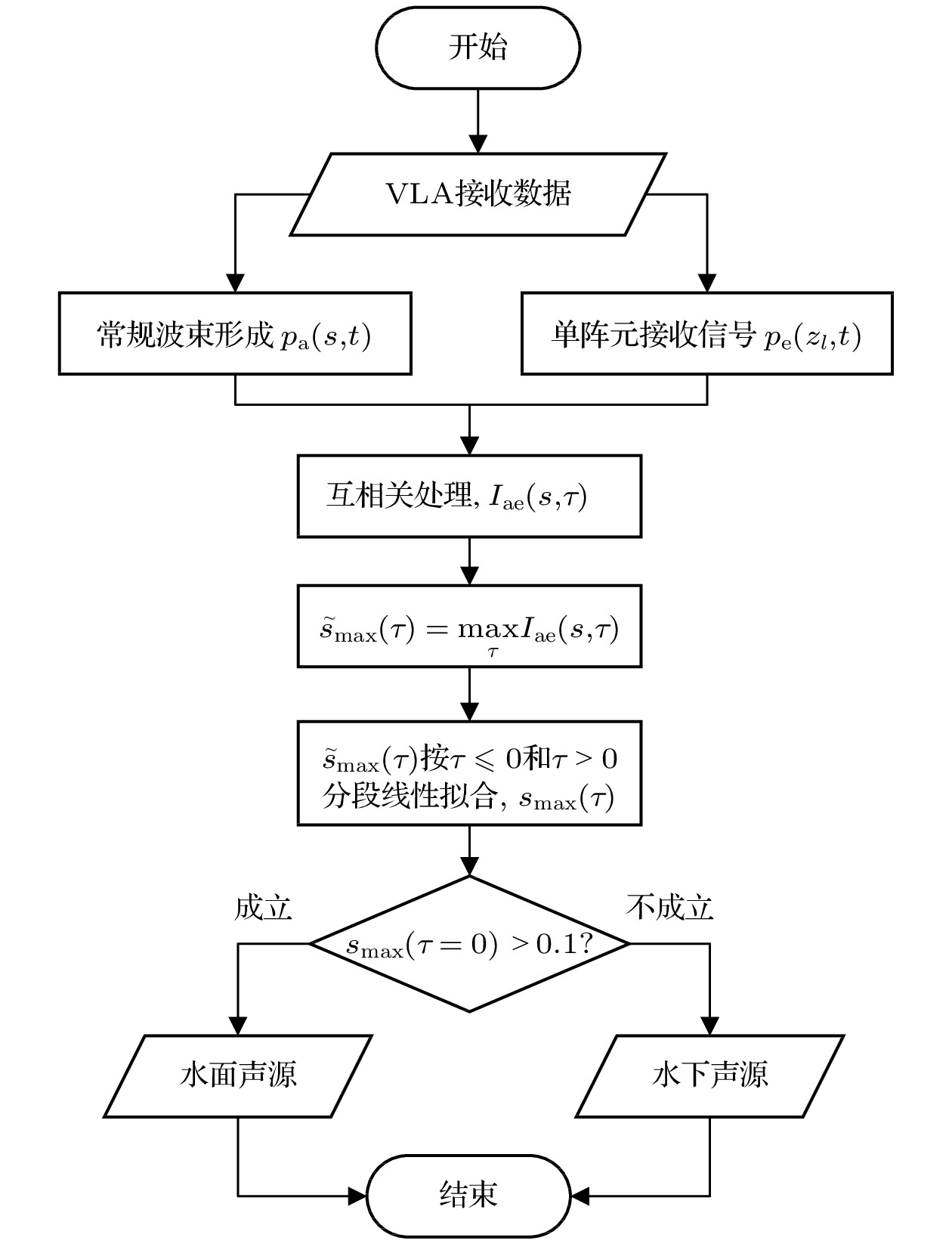
-
In this paper, the peak migration line of cross-correlation output is utilized to discriminate the depth of an underwater acoustic source in shallow water environment with a negative thermocline through studying the modal arrival structure of the source at different depths. The discrimination can be done according to the position of the migration line when the cross-correlation delay is zero since the type of dominant normal mode is determined by the depth of the source. Firstly, using the beam output of a vertical linear array and the received signal of a single array element to do cross-correlation processing, a two-dimensional intensity image of cross-correlation delay versus elevation angle is obtained. Then, the peaks in the angle domain at different delays can be extracted from the image. The cross-correlation peak migration line can be achieved by piecewise linear fitting which is divided into two parts corresponding to the delay being less than or equal to zero and the delay being more than zero, respectively. Finally, the source depth is determined by the position of the curve where the cross-correlation time delay is zero. Theoretical analysis shows that the migration line is little influenced by the sound velocity profile (SVP) varying with depth, the discrimination threshold is determined by the lower-order normal modes, the identification region is determined by the thickness and depth of the thermocline and the higher strength of the thermocline is instrumental in distinguishing. The numerical results and the experimental results prove the method's effectiveness which can be implemented without SVP details or mode separation, or source movement.
-
Keywords:
- negative thermocline in shallow water /
- peak migration line /
- source depth discrimination /
- normal mode
[1] Touzé G L, Nicolas B, Lacoume J L, Mars J, Fattaccioli D 2005 IEEE OCEANS Brest, France, June 20–23, 2005 p725
[2] Nicolas B, Mars J, Lacoume J-L 2006 EURASIP J. Adv. Signal Process. 2006 1
Google Scholar
[3] Courtois F L, Bonnel J 2015 J. Acoust. Soc. Am. 138 575
Google Scholar
[4] Lopatka M, Touzé G L, Nicolas B, Cristol X, Mars J, Fattaccioli D 2010 EURASIP J. Adv. Signal Process. 2010 1
Google Scholar
[5] Shang E C 1985 J. Acoust. Soc. Am. 77 1413
Google Scholar
[6] Neilsen T B, Westwood E K 2002 J. Acoust. Soc. Am. 111 748
Google Scholar
[7] Reeder B D 2014 J. Acoust. Soc. Am. 135 EL1
Google Scholar
[8] Yang T C 2015 J. Acoust. Soc. Am. 138 1678
Google Scholar
[9] Turgut A, Fialkowski L T, Schindall J A 2016 J. Acoust. Soc. Am. 139 EL184
Google Scholar
[10] 刘志韬, 郭良浩, 闫超 2019 声学学报 5 925
Google Scholar
Liu Z T, Guo L H, Yan C 2019 Acta Acust. 5 925
Google Scholar
[11] Jensen F B, Kuperman W A, Porter M B, Schmidt H 2011 Computational Ocean Acoustics (New York: Springer) pp739– 740
[12] Lee S, Makris N C 2006 J. Acoust. Soc. Am. 119 336
Google Scholar
[13] Gong Z, Ratilal P, Makris N C 2015 J. Acoust. Soc. Am. 138 2649
Google Scholar
[14] Ewing W M, Jardetzky W S, Press F 1957 Elastic Waves in Layered Media (McGraw-Hill: New York) pp341–367
[15] Premus V E, Helfrick M N 2013 J. Acoust. Soc. Am. 133 4019
Google Scholar
[16] Urick R J 1983 Principles of Underwater Sound (Vol. 3) (McGraw-Hill: New York) pp120–121
[17] 张仁和 1979 声学学报 2 102
Google Scholar
Zhang R H 1979 Acta Acust. 2 102
Google Scholar
[18] 高大治 2012 博士学位论文(青岛: 中国海洋大学)
Gao D Z 2012 Ph. D. Dissertation (Qingdao: Ocean University of China) (in Chinese)
[19] Weston D E. 1960 J. Acoust. Soc. Am. 32 647
Google Scholar
[20] Marine Physical Lab http://swellex96.ucsd.edu/index.htm [2021-10-19]
-
图 1 VLA、声源位置和负跃层声速剖面示意图
Figure 1. VLA, the position of the source and SVP in shallow water with a negative thermocline.
图 2 互相关输出示意图
Figure 2. The cross-correlation output.
图 3 TM和NTM在不同接收深度上的俯仰角示意图
Figure 3. The elevation angles of TM and NTM at different depths.
图 4 互相关输出峰值迁移曲线示意图
Figure 4. The peak migration line of the cross-correlation output.
图 5 判别算法流程图
Figure 5. The flow diagrams of the source depth discrimination.
图 6 负跃层波导环境及其相关参数
Figure 6. The shallow water waveguide with a negative thermocline and its environmental parameters.
图 7 各阶模态函数
Figure 7. The mode functions.
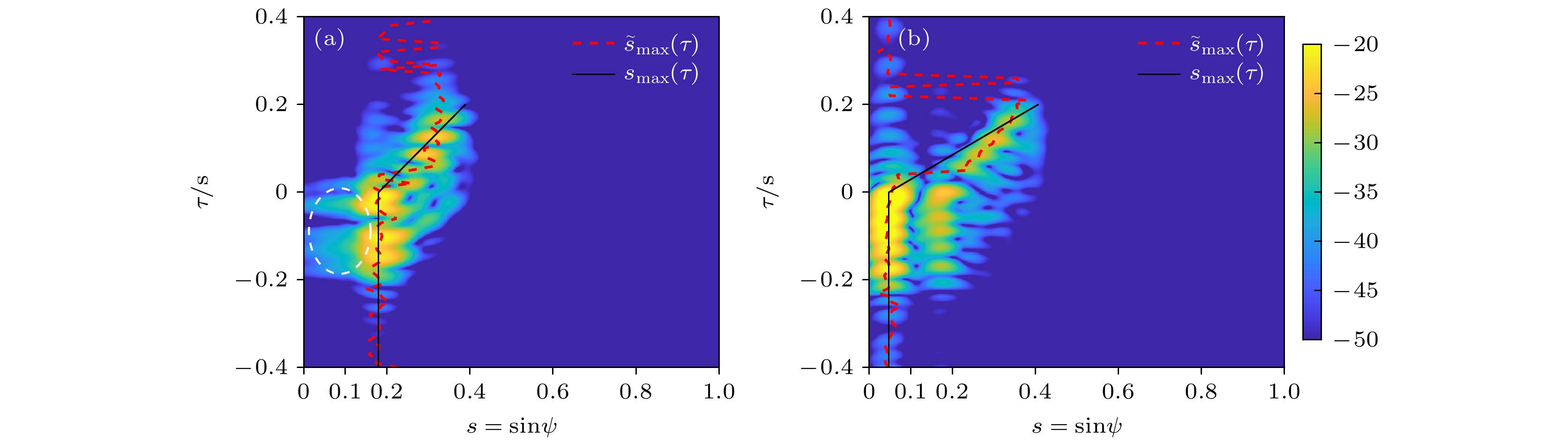
图 8 不同声源深度的
${I_{{\text{ae}}}}(s, \tau )$ ,${\tilde s_{\max }}(\tau )$ 和${s_{\max }}(\tau )$ (a)声源深度10 m, 白色虚线圈标注了峰裂分现象; (b)声源深度50 mFigure 8.
${I_{{\text{ae}}}}(s, \tau )$ ,${\tilde s_{\max }}(\tau )$ and${s_{\max }}(\tau )$ of sources at different depths: (a) Source at a depth of 10 m, with the peak splitting indicated by white dashed circle; (b) source at a depth of 50 m.
图 9 不同声速差时的各阶模态函数 (a)
$\Delta c$ = 0 m/s; (b)$\Delta c$ = 40 m/sFigure 9. The mode functions with different
$\Delta c$ : (a)$\Delta c$ = 0 m/s; (b)$\Delta c$ = 40 m/s.
图 10 不同声速差时不同声源深度的
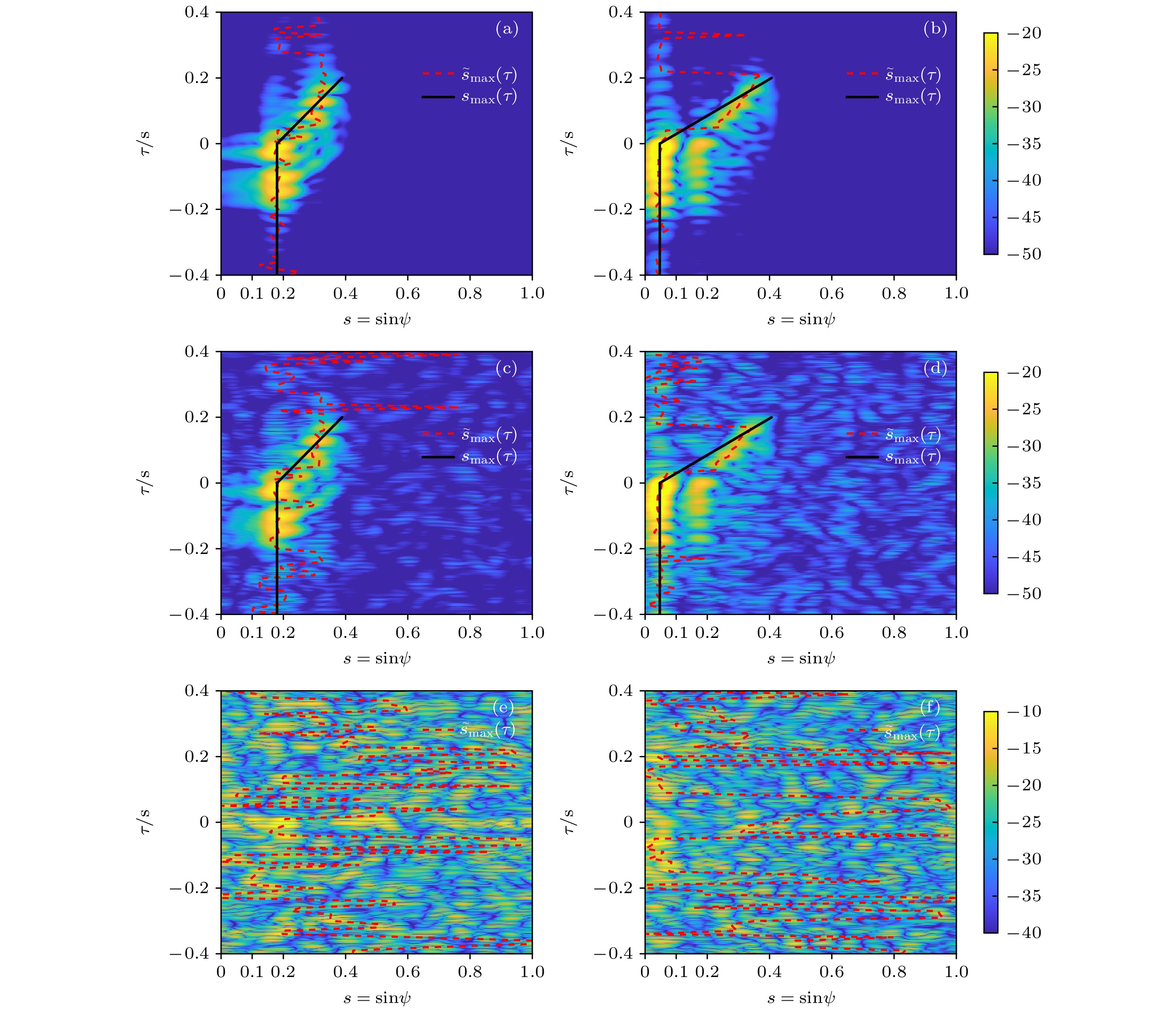
${I_{{\text{ae}}}}(s, \tau )$ ,${\tilde s_{\max }}(\tau )$ 和${s_{\max }}(\tau )$ (a)$\Delta c$ = 0 m/s, 声源深度10 m; (b)$\Delta c$ = 0 m/s, 声源深度50 m; (c)$\Delta c$ = 40 m/s, 声源深度10 m; (d)$\Delta c$ = 40 m/s, 声源深度50 mFigure 10.
${I_{{\text{ae}}}}(s, \tau )$ ,${\tilde s_{\max }}(\tau )$ and${s_{\max }}(\tau )$ of sources at different depths with different$\Delta c$ : (a)$\Delta c$ = 0 m/s, source at a depth of 10 m; (b)$\Delta c$ = 0 m/s, source at a depth of 50 m; (c)$\Delta c$ = 40 m/s, source at a depth of 10 m; (d)$\Delta c$ = 40 m/s, source at a depth of 50 m.
图 11 不同SNR条件下, 不同声源深度的
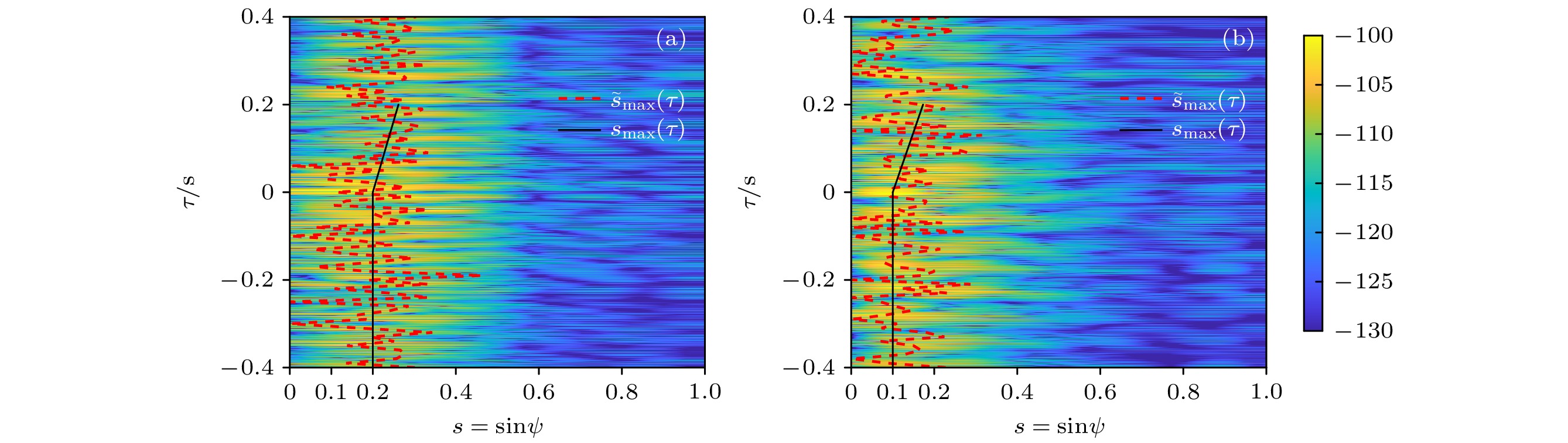
$ {I_{{\text{ae}}}}\left( {s, \tau } \right) $ ,${\tilde s_{\max }}(\tau )$ 和${s_{\max }}(\tau )$ (a) SNR = –10 dB, 声源深度10 m; (b) SNR = –10 dB, 声源深度50 m; (c) SNR = –20 dB, 声源深度10 m; (d) SNR = –20 dB, 声源深度50 m; (e) SNR = –25 dB, 声源深度10 m; (f) SNR = –25 dB, 声源深度50 mFigure 11.
$ {I_{{\text{ae}}}}\left( {s, \tau } \right) $ ,${\tilde s_{\max }}(\tau )$ and${s_{\max }}(\tau )$ of sources at different depths with different SNR: (a) SNR = –10 dB, source at a depth of 10 m; (b) SNR = –10 dB, source at a depth of 50 m; (c) SNR = –20 dB, source at a depth of 10 m; (d) SNR = –20 dB, source at a depth of 50 m; (e) SNR = –25 dB, source at a depth of 10 m; (f) SNR = –25 dB, source at a depth of 50 m.
图 12 S5试验情况 (a) 试验场环境与发射船轨迹; (b) 声速剖面
Figure 12. Event S5: (a) The environment and the track of the source ship; (b) SVP.
图 13 S5试验波导下频率135 Hz的各阶模态函数
Figure 13. The mode functions in S5 waveguide with f = 135 Hz.
图 14 J-13和J-15声源的
${I_{{\text{ae}}}}(s, \tau )$ ,${\tilde s_{\max }}(\tau )$ 和${s_{\max }}(\tau )$ (a)J-13, 9 m; (b)J-15, 54 mFigure 14.
$ {I_{{\text{ae}}}}(s, \tau ) $ ,${\tilde s_{\max }}(\tau )$ and${s_{\max }}(\tau )$ of different sources: (a) J-13, 9 m; (b) J-15, 54 m.表 1 不同声源的频率
Table 1. Frequencies transmitted by different sources.
声源类型 频率/Hz J-13(9 m) 109 127 145 163 198 232 280 335 385 J-15(54 m) 49 64 79 94 112 130 148 166 201 235 283 338 388 
DownLoad: CSV
-
[1] Touzé G L, Nicolas B, Lacoume J L, Mars J, Fattaccioli D 2005 IEEE OCEANS Brest, France, June 20–23, 2005 p725
[2] Nicolas B, Mars J, Lacoume J-L 2006 EURASIP J. Adv. Signal Process. 2006 1
Google Scholar
[3] Courtois F L, Bonnel J 2015 J. Acoust. Soc. Am. 138 575
Google Scholar
[4] Lopatka M, Touzé G L, Nicolas B, Cristol X, Mars J, Fattaccioli D 2010 EURASIP J. Adv. Signal Process. 2010 1
Google Scholar
[5] Shang E C 1985 J. Acoust. Soc. Am. 77 1413
Google Scholar
[6] Neilsen T B, Westwood E K 2002 J. Acoust. Soc. Am. 111 748
Google Scholar
[7] Reeder B D 2014 J. Acoust. Soc. Am. 135 EL1
Google Scholar
[8] Yang T C 2015 J. Acoust. Soc. Am. 138 1678
Google Scholar
[9] Turgut A, Fialkowski L T, Schindall J A 2016 J. Acoust. Soc. Am. 139 EL184
Google Scholar
[10] 刘志韬, 郭良浩, 闫超 2019 声学学报 5 925
Google Scholar
Liu Z T, Guo L H, Yan C 2019 Acta Acust. 5 925
Google Scholar
[11] Jensen F B, Kuperman W A, Porter M B, Schmidt H 2011 Computational Ocean Acoustics (New York: Springer) pp739– 740
[12] Lee S, Makris N C 2006 J. Acoust. Soc. Am. 119 336
Google Scholar
[13] Gong Z, Ratilal P, Makris N C 2015 J. Acoust. Soc. Am. 138 2649
Google Scholar
[14] Ewing W M, Jardetzky W S, Press F 1957 Elastic Waves in Layered Media (McGraw-Hill: New York) pp341–367
[15] Premus V E, Helfrick M N 2013 J. Acoust. Soc. Am. 133 4019
Google Scholar
[16] Urick R J 1983 Principles of Underwater Sound (Vol. 3) (McGraw-Hill: New York) pp120–121
[17] 张仁和 1979 声学学报 2 102
Google Scholar
Zhang R H 1979 Acta Acust. 2 102
Google Scholar
[18] 高大治 2012 博士学位论文(青岛: 中国海洋大学)
Gao D Z 2012 Ph. D. Dissertation (Qingdao: Ocean University of China) (in Chinese)
[19] Weston D E. 1960 J. Acoust. Soc. Am. 32 647
Google Scholar
[20] Marine Physical Lab http://swellex96.ucsd.edu/index.htm [2021-10-19]
DownLoad:
Catalog
Metrics
- Abstract views: 4340
- PDF Downloads: 72
- Cited By: 0
