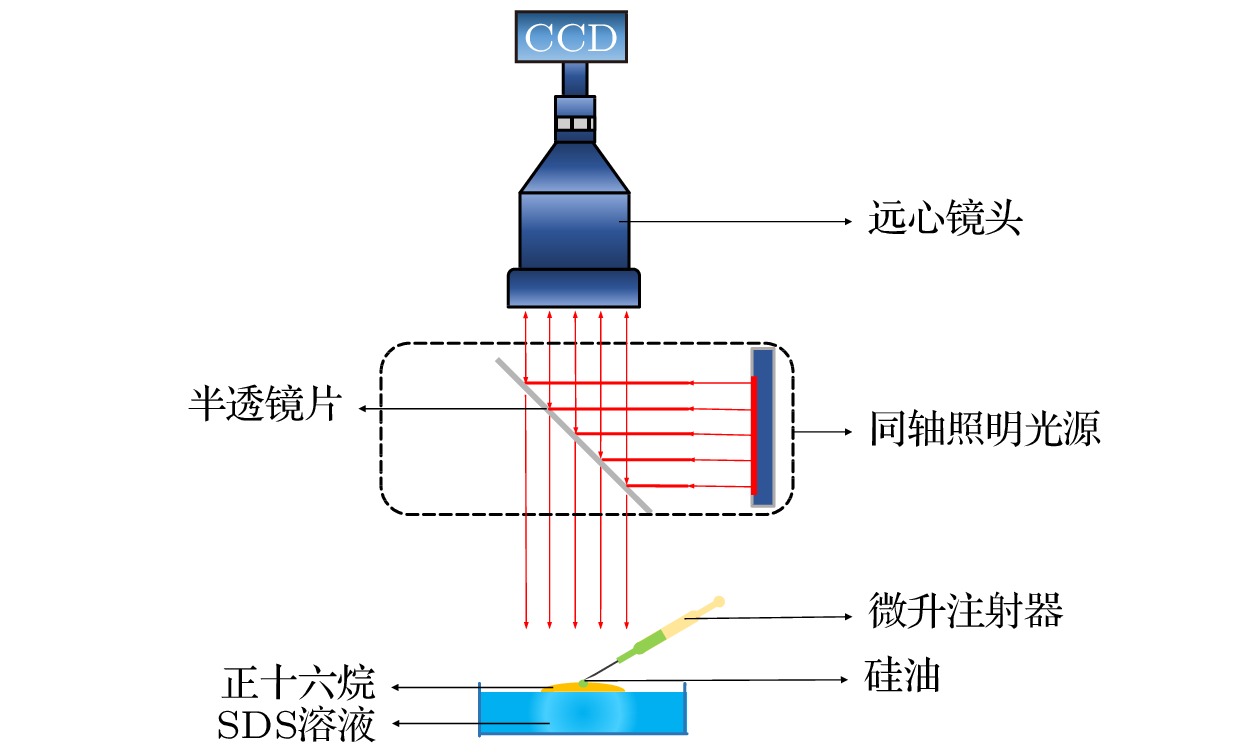
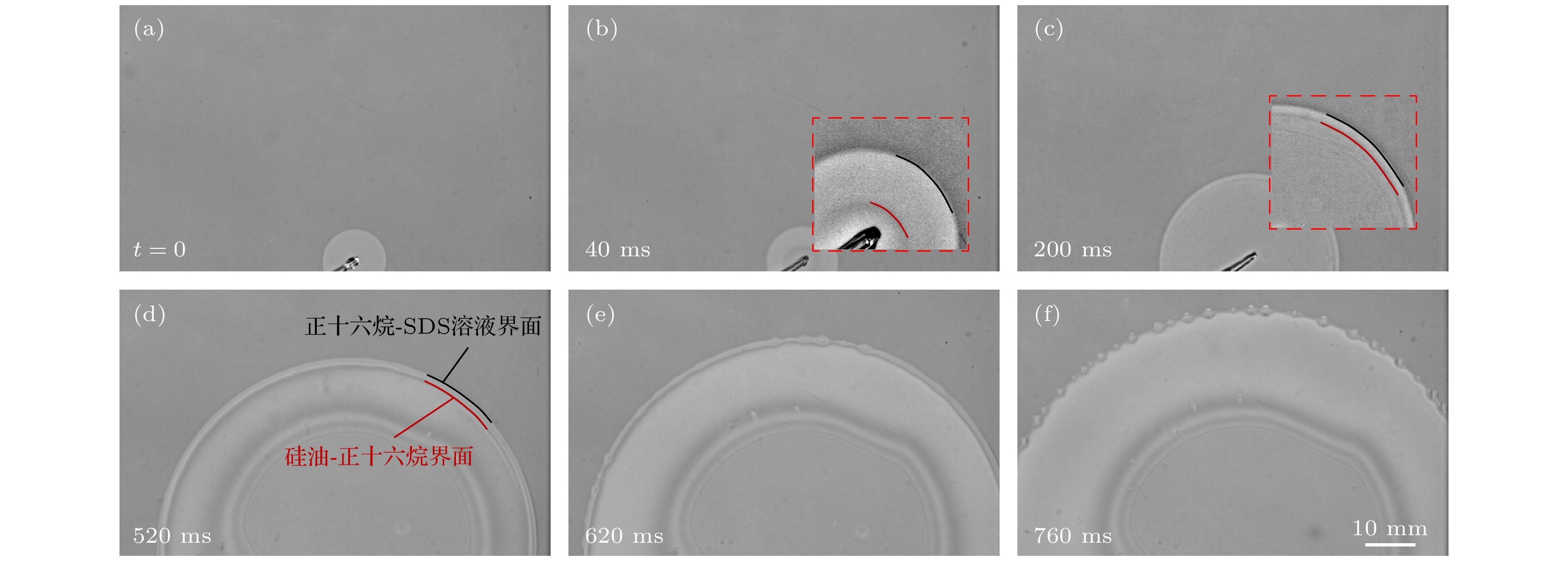
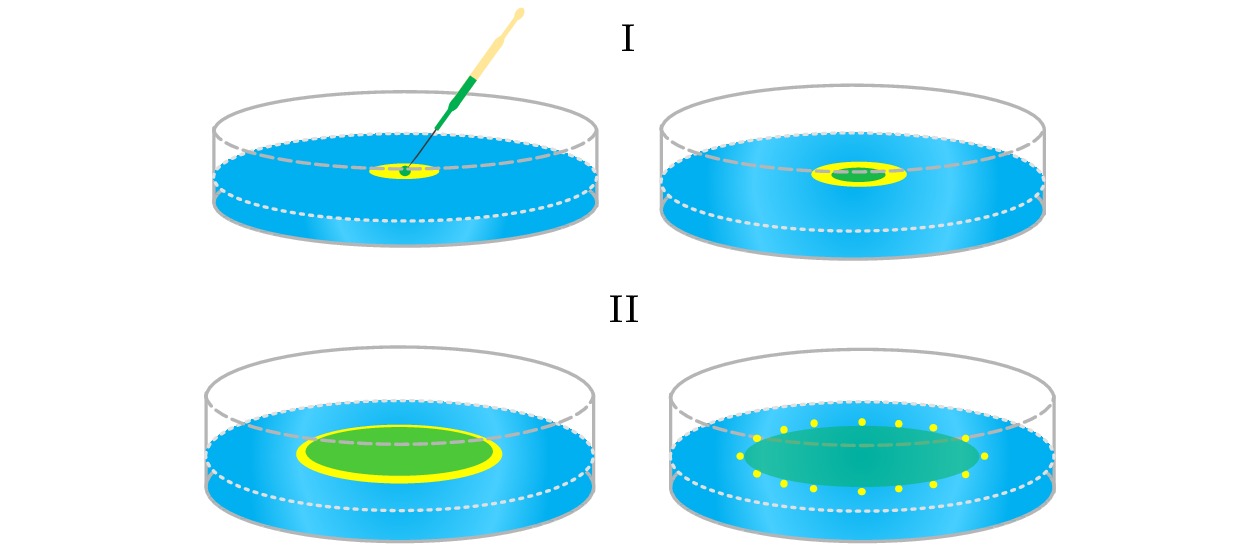
-
Drop dynamics at liquid surfaces is existent in nature and industry, which is of great value in studying droplet self-propulsion, surface coating, and drug delivery, and possesses great potential applications in microfluidics and biological process. Here, we analyze the role of Marangoni effect in the spontaneously driving system by studying the driving effect of a low surface tension liquid at the liquid substrate on another liquid. A three-phase liquid system is established to explore the liquid-driven spreading process, including non-volatile silicone oil as driving solvent, n-hexadecane as driven solvent, and sodium dodecyl sulfate (SDS) solution with different concentrations as aqueous substrates. The spreading process of n-hexadecane driven by silicone oil can be divided into two stages. N-hexadecane is first driven to form a thin rim, and then the rim breaks up into small liquid beads. Afterwards, the driving mechanism, spreading scaling laws and instability characteristic parameters of the liquid-driven spreading process are analyzed theoretically. The analysis of driving mechanism indicates that the differences in surface tension among silicone oil, n-hexadecane and SDS solution cause surface tension gradient at the liquid-liquid interface, which plays a crucial role in spreading the n-hexadecane. The results also demonstrate that the maximum spreading radius of n-hexadecane is affected by the concentration of the aqueous substrate. When the concentration of SDS solution is lower than the critical micelle concentration, the maximum spreading radius of n-hexadecane is proportional to the concentration of SDS solution. Meanwhile, the scaling law between the spreading radius R and time t driven by silicone oil conforms to the classical theoretical
$ \mathrm{r}\mathrm{e}\mathrm{l}\mathrm{a}\mathrm{t}\mathrm{i}\mathrm{o}\mathrm{n}\;R\left(t\right)\propto {t}^{3/4} $ . In addition, the classical analysis model is used to explain the instability pattern of n-hexadecane breaking into small beads from rim in the liquid-driven spreading process, which is called Rayleigh-Plateau instability. The fastest instability wavelength$ {\lambda }_{\mathrm{s}} $ and the constant radius$ {r}_{\mathrm{c}} $ of the n-hexadecane liquid rim are related by$ {\lambda }_{\mathrm{s}}\approx 9{r}_{\mathrm{c}} $ . Our results prove the applicability of the spreading scaling law to the liquid-driven spreading process, and also help to understand in depth the mechanism of the liquid-driven spreading and the instability pattern in the spreading process.-
Keywords:
- driven spreading /
- drop dynamics /
- interface instability /
- surface tension
[1] 袁泉子, 沈文豪, 赵亚溥 2016 力学进展 46 343
Google Scholar
Yuan Q Z, Shen W H, Zhao Y B 2016 Adv. Mech. 46 343
Google Scholar
[2] Yeo Y, Chen A, Basaran O, Park K 2004 Pharm. Res. 21 1419
Google Scholar
[3] Charve J, Reineccius G 2009 J. Agric. Food Chem. 57 2486
Google Scholar
[4] Blanco-Pascual N, Koldeweij R, Stevens R, Montero P, Gomez-Guillen M, Ten Cate A T 2014 Food Bioprocess Technol. 7 2472
Google Scholar
[5] Zhao X, Kim J, Cezar C, Huebsch N, Lee K, Bouhadir K, Mooney D 2011 Proc. Natl. Acad. Sci. U. S. A. 108 67
Google Scholar
[6] Seemann R, Fleury J B, Maass C C 2016 Eur. Phys. J.-Spec. Top. 225 2227
Google Scholar
[7] Kang D, Nadim A, Chugunova M 2017 Phys. Fluids 29 495
Google Scholar
[8] Roman B, Bico J 2010 J. Phys. Condens. Matter 22 493101
Google Scholar
[9] Elfring G, Goyal G 2015 J. Non-Newtonian Fluid Mech. 234 8
Google Scholar
[10] Tanner L H 1979 J. Phys. D; Appl. Phys. 12 13
Google Scholar
[11] Milchev A, Binder K 2002 J. Chem. Phys. 116 7691
Google Scholar
[12] Franklin B, Brownrigg W, Farish A 1774 Philos. Trans. R. Soc. Lond 64 445
Google Scholar
[13] Hoult D P 1972 Annu. Rev. Fluid Mech. 4 341
Google Scholar
[14] Fraaije J G E M, Cazabat A 1989 J. Colloid Interface Sci. 133 452
Google Scholar
[15] Berg S 2009 Phys. Fluids 21 398
Google Scholar
[16] Wodlei F, Sebilleau J, Magnaudet J, Pimienta V 2018 Nat. Commun. 9 820
Google Scholar
[17] Afsar-Siddiqui A, Luckham P, Matar O 2004 Adv. Colloid Interface Sci. 106 183
Google Scholar
[18] Lee K S, Starov V 2009 J. Colloid Interface Sci. 329 361
Google Scholar
[19] Afsar-Siddiqui A, Luckham P, Matar O 2003 Langmuir 19 703
Google Scholar
[20] Lee K S, Starov V 2007 J. Colloid Interface Sci. 314 631
Google Scholar
[21] Koldeweij R, Capelleveen B, Lohse D, Visser C W 2019 Soft Matter 15 8525
Google Scholar
[22] Chengara A, Nikolov A D, Wasan D T 2007 Ind. Eng. Chem. Res. 46 2987
Google Scholar
[23] Wilkinson K, Bain C, Matsubara H, Aratono M 2005 ChemPhysChem 6 547
Google Scholar
[24] Bonn D, Ross D 2001 Rep. Prog. Phys. 64 1085
Google Scholar
[25] Bergeron V, Langevin D 1996 Phys. Rev. Lett. 76 3152
Google Scholar
[26] Brochardwyart F, Dimeglio J M, Quere D, Degennes P G 1991 Langmuir 7 335
Google Scholar
[27] Rayleigh J 1878 Proc. London Math. Soc. 10 4
Google Scholar
[28] Eggers J, Dupont T 2001 J. Fluid Mech. 262 205
Google Scholar
[29] Frankel I, Weihs D 1987 J. Fluid Mech. 185 361
Google Scholar
-
图 1 实验装置示意图
Figure 1. Schematic diagram of experimental device.
图 2 不同浓度SDS溶液表面正十六烷初始铺展状态(比例尺为2 mm)
Figure 2. Initial spreading state of n-hexadecane on the surface of SDS solutions with different concentrations (scale bar = 2 mm).
图 3 SDS溶液(8 mmol/L)表面正十六烷受驱动铺展过程(比例尺为10 mm)
Figure 3. Spreading process of n-hexadecane on the surface of SDS solution (8 mmol/L) (scale bar = 10 mm).
图 4 硅油的驱动作用示意图. 1, 2和3分别为驱动溶剂硅油、受驱动溶剂正十六烷和基底SDS溶液,
$ {\sigma }_{23} $ 和$ {\sigma }_{12} $ 分别表示正十六烷与SDS基底溶液之间和硅油与正十六烷之间的界面张力,$ \nabla \sigma $ 表示界面张力梯度,${\Delta }P$ 表示压力梯度Figure 4. Schematic diagram of the driving effect of silicone oil. 1, 2 and 3 are driving solvent (silicone oil), driven solvent (n-hexadecane) and aqueous substrate (SDS solution),
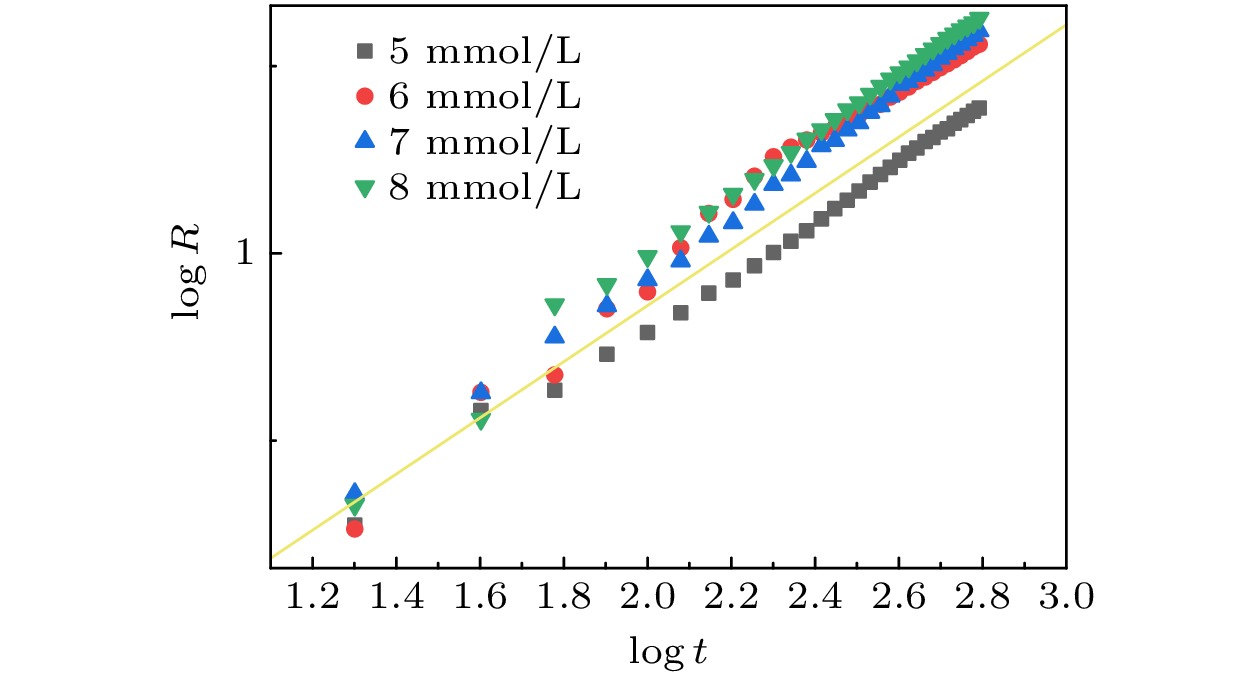
$ {\sigma }_{23} $ and$ {\sigma }_{12} $ represent the interfacial tension between n-hexadecane and SDS solution and between silicone oil and n-hexadecane, respectively.$ \nabla \sigma $ represents the interfacial tension gradient, and$ {\Delta }P $ represents the pressure gradient.
图 5 正十六烷液滴受驱动铺展阶段示意图
Figure 5. Schematic diagram of the driven-spreading stage of n-hexadecane.
图 6 失稳前不同浓度的SDS溶液表面正十六烷铺展半径R随时间的变化(在5, 6, 7和8 mmol/L的SDS溶液表面, 正十六烷受驱动铺展的标度率分别为0.73, 0.80, 0.82, 0.83, 黄线为理论值0.75)
Figure 6. Variations of the spreading radius R of n-hexadecane on the surface of SDS solutions with different concentrations before instability occurs. The driven-spreading scale rate of n-hexadecane is 0.73, 0.80, 0.82, and 0.83 on the surface of 5, 6, 7 and 8 mmol/L SDS solution, respectively. The yellow line is the theoretical rate of 0.75.
表 1 表/界面张力
$ \sigma $ (单位: mN·m–1)Table 1. Surface/Interfacial tension
$ \sigma $ (in mN·m–1)SDS溶液浓度/(mmol·L–1) 5 6 7 8 表面张力 33.19 32.82 32.62 34.53 与正十六烷界面张力 6.52 6.29 6.56 6.60 
DownLoad: CSV
表 2 铺展系数S
Table 2. Spreading coefficient S.
SDS溶液浓度/(mmol·L–1) 5 6 7 8 S –0.13 –0.27 –0.74 1.12
DownLoad: CSV
表 3 增长最快波长
$ {\lambda }_{\mathrm{s}} $ 、半径$ {r}_{\mathrm{c}} $ 和$ {\lambda }_{\mathrm{s}}/{r}_{\mathrm{c}} $ 的实验值Table 3. Experimental values of the fastest growing wavelength
$ {\lambda }_{\mathrm{s}} $ , the radius$ {r}_{\mathrm{c}} $ and$ {\lambda }_{\mathrm{s}}/{r}_{\mathrm{c}} $ .SDS溶液浓度/(mmol·L–1) 5 6 7 8 $ {\lambda }_{\mathrm{s}} $/mm 3.38 3.12 3.04 3.79 $ {r}_{\mathrm{c}} $/mm 0.36 0.32 0.31 0.41 $ {\lambda }_{\mathrm{s}}/{r}_{\mathrm{c}} $ 9.39 9.75 9.81 9.24
DownLoad: CSV
-
[1] 袁泉子, 沈文豪, 赵亚溥 2016 力学进展 46 343
Google Scholar
Yuan Q Z, Shen W H, Zhao Y B 2016 Adv. Mech. 46 343
Google Scholar
[2] Yeo Y, Chen A, Basaran O, Park K 2004 Pharm. Res. 21 1419
Google Scholar
[3] Charve J, Reineccius G 2009 J. Agric. Food Chem. 57 2486
Google Scholar
[4] Blanco-Pascual N, Koldeweij R, Stevens R, Montero P, Gomez-Guillen M, Ten Cate A T 2014 Food Bioprocess Technol. 7 2472
Google Scholar
[5] Zhao X, Kim J, Cezar C, Huebsch N, Lee K, Bouhadir K, Mooney D 2011 Proc. Natl. Acad. Sci. U. S. A. 108 67
Google Scholar
[6] Seemann R, Fleury J B, Maass C C 2016 Eur. Phys. J.-Spec. Top. 225 2227
Google Scholar
[7] Kang D, Nadim A, Chugunova M 2017 Phys. Fluids 29 495
Google Scholar
[8] Roman B, Bico J 2010 J. Phys. Condens. Matter 22 493101
Google Scholar
[9] Elfring G, Goyal G 2015 J. Non-Newtonian Fluid Mech. 234 8
Google Scholar
[10] Tanner L H 1979 J. Phys. D; Appl. Phys. 12 13
Google Scholar
[11] Milchev A, Binder K 2002 J. Chem. Phys. 116 7691
Google Scholar
[12] Franklin B, Brownrigg W, Farish A 1774 Philos. Trans. R. Soc. Lond 64 445
Google Scholar
[13] Hoult D P 1972 Annu. Rev. Fluid Mech. 4 341
Google Scholar
[14] Fraaije J G E M, Cazabat A 1989 J. Colloid Interface Sci. 133 452
Google Scholar
[15] Berg S 2009 Phys. Fluids 21 398
Google Scholar
[16] Wodlei F, Sebilleau J, Magnaudet J, Pimienta V 2018 Nat. Commun. 9 820
Google Scholar
[17] Afsar-Siddiqui A, Luckham P, Matar O 2004 Adv. Colloid Interface Sci. 106 183
Google Scholar
[18] Lee K S, Starov V 2009 J. Colloid Interface Sci. 329 361
Google Scholar
[19] Afsar-Siddiqui A, Luckham P, Matar O 2003 Langmuir 19 703
Google Scholar
[20] Lee K S, Starov V 2007 J. Colloid Interface Sci. 314 631
Google Scholar
[21] Koldeweij R, Capelleveen B, Lohse D, Visser C W 2019 Soft Matter 15 8525
Google Scholar
[22] Chengara A, Nikolov A D, Wasan D T 2007 Ind. Eng. Chem. Res. 46 2987
Google Scholar
[23] Wilkinson K, Bain C, Matsubara H, Aratono M 2005 ChemPhysChem 6 547
Google Scholar
[24] Bonn D, Ross D 2001 Rep. Prog. Phys. 64 1085
Google Scholar
[25] Bergeron V, Langevin D 1996 Phys. Rev. Lett. 76 3152
Google Scholar
[26] Brochardwyart F, Dimeglio J M, Quere D, Degennes P G 1991 Langmuir 7 335
Google Scholar
[27] Rayleigh J 1878 Proc. London Math. Soc. 10 4
Google Scholar
[28] Eggers J, Dupont T 2001 J. Fluid Mech. 262 205
Google Scholar
[29] Frankel I, Weihs D 1987 J. Fluid Mech. 185 361
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 6925
- PDF Downloads: 192
- Cited By: 0
