-
Exploring phase transition behaviors and constructing phase diagrams are of importance for theoretically and experimentally studying ferroelectric physics and materials. Because of the rapid development of computers and artificial intelligence, especially machine learning methods combined with other computational methods such as first principle calculation, it is possible to predict and choose appropriate materials that meet the target requirements from a large number of material data, which greatly saves the cost of experiments. In this work, we use neural network method and phenomenological theoretical calculations to accurately predict the phase structures that may appear in the phase diagrams of different orientated Pb(Zr0.52Ti0.48)O3 ferroelectric films, and establish the temperature-strain phase diagrams of (001), (110) and (111) oriented thin film, and calculate the polarization and dielectric properties of different oriented films at room temperature. By analyzing the changes of prediction accuracy and loss with the number of iterations, it is found that the deep neural network method has the advantages of high accuracy and speed in the construction of the film temperature-strain phase diagram and the prediction of the types of phases. Through the analysis of the room temperature polarization and dielectric properties, it is found that the (111)-oriented PbZr0.52Ti0.48O3 film has the largest out-of-plane polarization and the smallest out-of-plane dielectric coefficient, and they are insensitive to misfit strain. This work provides guidelines for designing micro-nano devices that require the stable dielectric coefficient and polarization performance in the special working environment and operation.
-
Keywords:
- ferroelectric thin films /
- phase diagram /
- machine learning /
- phenomenological theory
[1] Scott J 2007 Science 315 954
Google Scholar
[2] Dawber M, Rabe K, Scott J 2005 Rev. Mod. Phys. 77 1083
Google Scholar
[3] Schlom D, Chen L, Eom C, Rabe K, Streiffer S, Triscone J 2007 Annu. Rev. Mater. Res. 37 589
Google Scholar
[4] Agar J, Pandya S, Xu R, Yadav A, Liu Z, Angsten T, Saremi S, Asta M, Ramesh R, Martin L 2016 MRS Commun. 6 151
Google Scholar
[5] Martin L, Chu Y, Ramesh R 2010 Mater. Sci. Eng. 68 89
Google Scholar
[6] Schlom D, Chen L, Pan X, Schmehl A, Zurbuchen M 2008 J. Am. Ceram. Soc. 91 2429
Google Scholar
[7] Choi K, Biegalski M, Li Y, Sharan A, Schubert J, Uecker R, Reiche P, Chen Y, Pan X, Gopalan V, Chen L, Schlom D, Eom C 2004 Science 306 1005
Google Scholar
[8] Haeni J, Irvin P, Chang W, Uecker R, Reiche P, Li Y, Choudhury S, Tian W, Hawley M, Craigo B, Tagantsev A, Pan X, Streiffer S, Chen L, Kirchoefer S, Levy J, Schlom D 2004 Nature (London) 430 758
Google Scholar
[9] Sone K, Naganuma H, Miyazaki T, Nakajima T, Okamura S 2010 Jpn. J. Appl. Phys. 49 09MB03
Google Scholar
[10] Xu R, Liu S, Grinberg I, Karthik J, Damodaran A, Rappe A, Martin L 2015 Nat. Mater. 14 79
Google Scholar
[11] Simon W, Akdogan E, Safari A 2005 J. Appl. Phys. 97 103530
Google Scholar
[12] Simon W, Akdogan E, Safari A, Bellotti J 2005 Appl. Phys. Lett. 87 082906
Google Scholar
[13] Simon W, Akdogan E, Safari A, Bellotti J 2006 Appl. Phys. Lett. 88 132902
Google Scholar
[14] Gui Z, Prosandeev S, Bellaiche L 2011 Phys. Rev. B 84 214112
Google Scholar
[15] Raeliarijaona A, Fu H 2014 J. Appl. Phys. 115 054105
Google Scholar
[16] Oja R, Johnston K, Frantti J, Nieminen R 2008 Phys. Rev. B 78 094102
Google Scholar
[17] Angsten T, Martin L, Asta M 2017 Phys. Rev. B 95 174110
Google Scholar
[18] Tagantsev A K, Pertsev N A, Muralt P, Setter N 2002 Phys. Rev. B 65 012104
Google Scholar
[19] Akcay G, Misirlioglu I B, Alpay S P 2006 Appl. Phys. Lett. 89 042903
Google Scholar
[20] Zhang J X, Li Y L, Wang Y, Lliu Z K, Chen L Q, Chu Y H, Zavaliche F, Ramesh R 2007 J. Appl. Lett. 101 114105
Google Scholar
[21] Wu H, Ma X, Zhang Z, Zeng J, Wang J, Chai G 2016 AIP Adv. 6 015309
Google Scholar
[22] Mtebwa M, Tagantsev A K, Yamada T, Gemeiner P, Dkhil B, Setter N 2016 Phys. Rev. B 93 144113
Google Scholar
[23] Wang F, Ma W 2019 J. Appl. Lett. 125 082528
Google Scholar
[24] Qiu J, Chen Z, Wang X, Yuan N, Ding J 2016 Solid State Comm. 246 5
Google Scholar
[25] Qiu J, Chen Z, Wang X, Yuan N, Ding J 2016 Solid State Comm. 236 1
Google Scholar
[26] Li L, Yang Y, Zhang D, Ye Z, Jesse S, Kalinin S, Vasudevan R 2018 Sci. Adv. 4 eaap8672
Google Scholar
[27] Yuan R, Tian Y, Xue D, Xue D, Zhou Y, Ding X, Sun J, Lookman T 2019 Adv. Sci. 6 1901395
Google Scholar
[28] Pertsev N, Zembilgotov A, Tagantsev A 1998 Phys. Rev. Lett. 80 1988
Google Scholar
[29] Liu Y and Li J 2011 Phys. Rev. B 84 132104
Google Scholar
[30] Chen L 2007 Landau Free-Energy Coefficients, Physics of Ferroelectrics: A Modern Perspective (Berlin: Springer-Verlag)
[31] Liu D, Bai G, Gao C 2020 J. Appl. Lett. 127 154101
Google Scholar
[32] Hornik K, Stinchcombe M, White H 1989 Neural. Netw. 2 359
Google Scholar
[33] Zhu Z, Li J, Lai F, Zhen Y, Lin Y, Nan C, Li L 2007 Appl. Phys. Lett. 91 222910
Google Scholar
[34] Peng B, Zhang Q, Bai G, Leighton G, Shaw C, Milne S, Zou B, Sun W, Huang H, Wang Z 2019 Energy Environ. Sci. 12 1708
Google Scholar
[35] Huang H, Zhang G, Ma X, Liang D, Wang J, Liu Y, Wang Q, Chen L 2018 J. Am. Ceram. Soc. 101 1566
Google Scholar
-
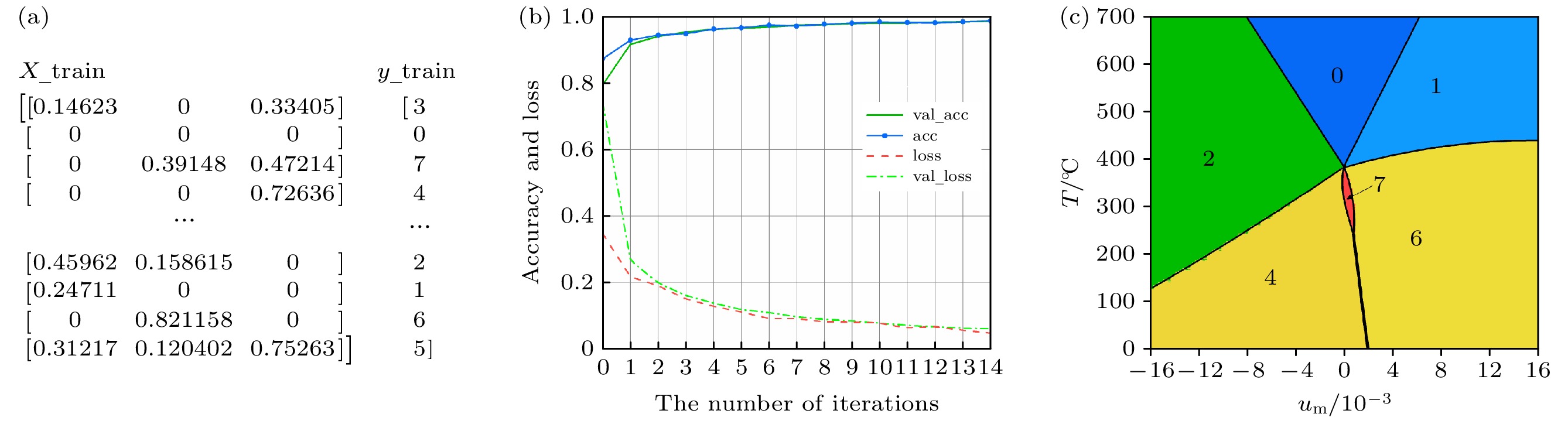
图 1 (a) 定向构造的训练集示例; (b) DNNs预测准确率及损失随迭代次数的变化; (c) DNNs预测的(110)取向的PZT52/48相图
Figure 1. (a) Constructed training set example; (b) the accuracy and loss of DNNs as a function of the number of iterations; (c) the temperature-misfit strain phase diagram of (110) oriented PZT52/48 thin film obtained by DNNs classification.
图 2 (a), (c), (e)分别为(001), (110), (111)取向薄膜可能存在相的结构示意图; (b), (d), (f)分别为(001), (110), (111)取向PZT52/48薄膜的相图, 其中粗线表示一级相变, 细线表示二级相变
Figure 2. Schematic diagrams of phase structures for (001) (a), (110) (c) and (111) (e) oriented ferroelectric PZT52/48 films; temperature-strain phase diagrams of (001) (b), (110) (d) and (111) (f) oriented PZT52/48 films. Thick and thin lines denote the first order and second order transitions, respectively.
图 3 (a) (001), (b) (110)和(c) (111)取向PZT54/48薄膜的室温极化随应变的变化
Figure 3. Strain dependent polarization of (a) (001), (b) (110), (c) (111) oriented PZT52/48 films at room temperature
图 4 在室温下, (a) (001), (b) (110)和(c) (111)取向PZT52/48薄膜的介电系数随应变的变化
Figure 4. Strain dependent dielectric coefficients of (a) (001), (b) (110) and (c) (111) oriented PZT52/48 films at room temperature.
表 1 不同取向PZT52/48薄膜相图中出现的相的极化分量的特征
Table 1. Polarization components of the different phases occurring in strain-temperature phase diagrams of (001), (110), and (111) oriented PZT52/48 films.
相结构 全局坐标 晶体坐标 (001) 顺电p ${P_1} = {P_2} = {P_3} = 0$ ${P_1} = {P_2} = {P_3} = 0$ 四方T相 ${P_1} = {P_2} = 0, {P_3} \ne 0$ ${P_1} = {P_2} = 0, {P_3} \ne 0$ 单斜M相 ${P_1} = {P_2} \ne 0, {P_3} \ne 0$ ${P_1} = {P_2} \ne 0, {P_3} \ne 0$ 正交O相 ${P_1} = {P_2} \ne 0, {P_3} = 0$ ${P_1} = {P_2} \ne 0, {P_3} = 0$ (110) 顺电p ${P'_1} = {P'_2} = {P'_3} = 0$ ${P_1} = {P_2} = {P_3} = 0$ 正交O ${P'_1} = {P'_2} = 0, {P'_3}\ne 0$ ${P_1} = {P_2} \ne 0, {P_3} = 0$ 单斜MB ${P'_1} < {P'_{\rm{3} } } {\rm{/} }\sqrt {\rm{2} }, {P'_{\rm{2} } } = 0$ ${P_1} = {P_2} > {P_3}$ 单斜MA ${P'_1} > {P'_{\rm{2} } } {\rm{/} }\sqrt {\rm{2} }, {P'_3} = 0$ ${P_1} = {P_2} < {P_3}$ 四方T ${P'_1} \ne {\rm{0 } },{P'_{\rm{2} } } = 0, {P'_3} = 0$ $ {P}_{1}{} = {P}_{2}=0, {P}_{3}\ne \rm{0}$ (111) 顺电p ${P''_1}= {P''_2} = {P''_3} = 0$ ${P_1} = {P_2} = {P_3} = 0$ 三方R ${P''_1} = {P''_2} = 0, \;{P''_3} \ne 0$ ${P_1} = {P_2} = {P_3} \ne 0$ 单斜MB ${P''_1} = 0, {P''_2} \ne 0, {P''_3} \ne 0$ ${P_1} = {P_2} > {P_3} \ne {\rm{0}}$ 
DownLoad: CSV
-
[1] Scott J 2007 Science 315 954
Google Scholar
[2] Dawber M, Rabe K, Scott J 2005 Rev. Mod. Phys. 77 1083
Google Scholar
[3] Schlom D, Chen L, Eom C, Rabe K, Streiffer S, Triscone J 2007 Annu. Rev. Mater. Res. 37 589
Google Scholar
[4] Agar J, Pandya S, Xu R, Yadav A, Liu Z, Angsten T, Saremi S, Asta M, Ramesh R, Martin L 2016 MRS Commun. 6 151
Google Scholar
[5] Martin L, Chu Y, Ramesh R 2010 Mater. Sci. Eng. 68 89
Google Scholar
[6] Schlom D, Chen L, Pan X, Schmehl A, Zurbuchen M 2008 J. Am. Ceram. Soc. 91 2429
Google Scholar
[7] Choi K, Biegalski M, Li Y, Sharan A, Schubert J, Uecker R, Reiche P, Chen Y, Pan X, Gopalan V, Chen L, Schlom D, Eom C 2004 Science 306 1005
Google Scholar
[8] Haeni J, Irvin P, Chang W, Uecker R, Reiche P, Li Y, Choudhury S, Tian W, Hawley M, Craigo B, Tagantsev A, Pan X, Streiffer S, Chen L, Kirchoefer S, Levy J, Schlom D 2004 Nature (London) 430 758
Google Scholar
[9] Sone K, Naganuma H, Miyazaki T, Nakajima T, Okamura S 2010 Jpn. J. Appl. Phys. 49 09MB03
Google Scholar
[10] Xu R, Liu S, Grinberg I, Karthik J, Damodaran A, Rappe A, Martin L 2015 Nat. Mater. 14 79
Google Scholar
[11] Simon W, Akdogan E, Safari A 2005 J. Appl. Phys. 97 103530
Google Scholar
[12] Simon W, Akdogan E, Safari A, Bellotti J 2005 Appl. Phys. Lett. 87 082906
Google Scholar
[13] Simon W, Akdogan E, Safari A, Bellotti J 2006 Appl. Phys. Lett. 88 132902
Google Scholar
[14] Gui Z, Prosandeev S, Bellaiche L 2011 Phys. Rev. B 84 214112
Google Scholar
[15] Raeliarijaona A, Fu H 2014 J. Appl. Phys. 115 054105
Google Scholar
[16] Oja R, Johnston K, Frantti J, Nieminen R 2008 Phys. Rev. B 78 094102
Google Scholar
[17] Angsten T, Martin L, Asta M 2017 Phys. Rev. B 95 174110
Google Scholar
[18] Tagantsev A K, Pertsev N A, Muralt P, Setter N 2002 Phys. Rev. B 65 012104
Google Scholar
[19] Akcay G, Misirlioglu I B, Alpay S P 2006 Appl. Phys. Lett. 89 042903
Google Scholar
[20] Zhang J X, Li Y L, Wang Y, Lliu Z K, Chen L Q, Chu Y H, Zavaliche F, Ramesh R 2007 J. Appl. Lett. 101 114105
Google Scholar
[21] Wu H, Ma X, Zhang Z, Zeng J, Wang J, Chai G 2016 AIP Adv. 6 015309
Google Scholar
[22] Mtebwa M, Tagantsev A K, Yamada T, Gemeiner P, Dkhil B, Setter N 2016 Phys. Rev. B 93 144113
Google Scholar
[23] Wang F, Ma W 2019 J. Appl. Lett. 125 082528
Google Scholar
[24] Qiu J, Chen Z, Wang X, Yuan N, Ding J 2016 Solid State Comm. 246 5
Google Scholar
[25] Qiu J, Chen Z, Wang X, Yuan N, Ding J 2016 Solid State Comm. 236 1
Google Scholar
[26] Li L, Yang Y, Zhang D, Ye Z, Jesse S, Kalinin S, Vasudevan R 2018 Sci. Adv. 4 eaap8672
Google Scholar
[27] Yuan R, Tian Y, Xue D, Xue D, Zhou Y, Ding X, Sun J, Lookman T 2019 Adv. Sci. 6 1901395
Google Scholar
[28] Pertsev N, Zembilgotov A, Tagantsev A 1998 Phys. Rev. Lett. 80 1988
Google Scholar
[29] Liu Y and Li J 2011 Phys. Rev. B 84 132104
Google Scholar
[30] Chen L 2007 Landau Free-Energy Coefficients, Physics of Ferroelectrics: A Modern Perspective (Berlin: Springer-Verlag)
[31] Liu D, Bai G, Gao C 2020 J. Appl. Lett. 127 154101
Google Scholar
[32] Hornik K, Stinchcombe M, White H 1989 Neural. Netw. 2 359
Google Scholar
[33] Zhu Z, Li J, Lai F, Zhen Y, Lin Y, Nan C, Li L 2007 Appl. Phys. Lett. 91 222910
Google Scholar
[34] Peng B, Zhang Q, Bai G, Leighton G, Shaw C, Milne S, Zou B, Sun W, Huang H, Wang Z 2019 Energy Environ. Sci. 12 1708
Google Scholar
[35] Huang H, Zhang G, Ma X, Liang D, Wang J, Liu Y, Wang Q, Chen L 2018 J. Am. Ceram. Soc. 101 1566
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4664
- PDF Downloads: 82
- Cited By: 0
