基于机器学习J1-J2反铁磁海森伯自旋链相变点的识别方法

王伟; 揭泉林

doi:10.7498/aps.70.20210711

摘要

通过序参量来研究量子相变是比较传统的做法, 而从机器学习的角度研究相变是一块全新的领域. 本文提出了先采用无监督学习算法中的高斯混合模型对J₁-J₂反铁磁海森伯自旋链系统的态矢量进行分类, 再使用监督学习算法中的卷积神经网络鉴别无监督学习算法给出的分类点是否是相变点的方法, 并使用交叉验证的方法对学习效果进行验证. 结果表明, 上述机器学习方法可以从基态精确找到J₁–J₂反铁磁海森伯自旋链系统的一阶相变点、无法找到无穷阶相变点, 从第一激发态不仅能找到一阶相变点, 还能找到无穷阶相变点.

关键词:

Abstract

Studying quantum phase transitions through order parameters is a traditional method, but studying phase transitions by machine learning is a brand new field. The ability of machine learning to classify, identify, or interpret massive data sets may provide physicists with similar analyses of the exponentially large data sets embodied in the Hilbert space of quantum many-body system. In this work, we propose a method of using unsupervised learning algorithm of the Gaussian mixture model to classify the state vectors of the J₁-J₂ antiferromagnetic Heisenberg spin chain system, then the supervised learning algorithm of the convolutional neural network is used to identify the classification point given by the unsupervised learning algorithm, and the cross-validation method is adopted to verify the learning effect. Using this method, we study the J₁-J₂ Heisenberg spin chain system with chain length N = 8, 10, 12, 16 and obtain the same conclusion. The first order phase transition point of J₁-J₂ antiferromagnetic Heisenberg spin chain system can be accurately found from the ground state vector, but the infinite order phase transition point cannot be found from the ground state vector. The first order and the infinite order phase transition point can be found from the first excited state vector, which indirectly shows that the first excited state may contain more information than the ground state of J₁-J₂ antiferromagnetic Heisenberg spin chain system. The visualization of the state vector shows the reliability of the machine learning algorithm, which can extract the feature information from the state vector. The result reveals that the machine learning techniques can directly find some possible phase transition points from a large set of state vectorwithout prior knowledge of the energy or locality conditions of the Hamiltonian, which may assists us in studying unknown systems. Supervised learning can verify the phase transition points given by unsupervised learning, thereby indicating that we can discover some useful information about unknown systems only through machine learning techniques. Machine learning techniques can be a basic research tool in strong quantum-correlated systems, and it can be adapted to more complex systems, which can help us dig up hidden information.

Keywords:

作者及机构信息

王伟,
揭泉林

武汉大学物理科学与技术学院, 武汉　430000

通信作者: 揭泉林, qljie@whu.edu.cn

基金项目: 国家自然科学基金(批准号: 1217050658)资助的课题

Authors and contacts

School of Physics and Technology, Wuhan University, Wuhan 430000, China

Corresponding author: Jie Quan-Lin, qljie@whu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 1217050658)

文章全文 : translate this paragraph

参考文献

[1]	Lei W 2016 Phys. Rev. B. 94 195105 Google Scholar
[2]	Wetzel S J 2017 Phys. Rev. E 96 022140 Google Scholar
[3]	Huang L, Wang L 2017 Phys. Rev. B 95 035105 Google Scholar
[4]	Phiala E S, Daniel T, William D 2018 Phys. Rev. D 97 094506 Google Scholar
[5]	LakovlevI A, SotnikovO M, MazurenkoV V 2018 Phys. Rev. B 98 174411 Google Scholar
[6]	Dong X Y, Pollmann F, Zhang X F 2019 Phys. Rev. B 99 121104 Google Scholar
[7]	Tan D R, Jiang F J 2020 Phys. Rev. B 102 224434 Google Scholar
[8]	Tan D R, Li C D, Zhu W P, Jiang F J 2020 New J. Phys. 22 063016 Google Scholar
[9]	Maskara N, Buchhold M, Endres M 2021 arXiv: 2103.15855 [quant-ph]
[10]	Tanja D 2021 arXiv: 2103.07236[quant-ph]
[11]	Sondhi S L, Girvin S M, Carini J P, Shahar D 1997 Rev. Mod. Phys 69 315 Google Scholar
[12]	Bulla R, Vojta M 2003 Rep. Prog. Phys. 66 2069 Google Scholar
[13]	Walker N, Tam K M, Novak B, Jarrell M 2018 Phys. Rev. E. 98 053305 Google Scholar
[14]	Jadrich R B, Lindquist B A, Pineros W D, Truskeet T M 2018 J. Chem. Phys. 149 194109 Google Scholar
[15]	Canabarro A, Fanchini F F, Malvezzi A L, Pereira R, Chaves R 2019 Phys. Rev. B 100 045129 Google Scholar
[16]	Carrasquilla J, Melko R G 2017 Nat. Phys. 13 431 Google Scholar
[17]	Ahmadreza A, Michel P 2020 arXiv: 2007.09764 [cond-mat. stat-mech]
[18]	Chitra R, Pati S, Krishnamurthy H R, Sen D, Ramasesha S 1995 Phys. Rev. B 52 6581 Google Scholar
[19]	Castilla G, Chakravarty S, Emery V J 1995 Phys. Rev. L 75 1823 Google Scholar
[20]	Shu C, Li W, Shi J G, Wang Y P 2007 Phys. Rev. E 76 061108 Google Scholar
[21]	Qian X F, Shi T, Li Y, Song Z, Sun C P 2005 Phys. Rev. A 72 012333 Google Scholar
[22]	周志华 2016 机器学习 (北京: 清华大学出版社) 第206页 Zhou Z H 2016 Machine Learning (Beijing: Tsinghua University Press) p206 (in Chinese)
[23]	李航 2012 统计机器学习 (北京: 清华大学出版社) 第162页 Li H 2012The Elements of Statistical Learning (Beijing: Tsinghua University Press) p162 (in Chinese)
[24]	徐启伟, 王佩佩, 曾镇佳, 黄泽斌, 周新星, 刘俊敏, 李瑛, 陈书青, 范滇元 2020 69 014209 Google Scholar Xu Q W, Wang P P, Zeng Z J, Huang Z B, Zhou X X, Liu J M, Li Y, Chen S Q, Fan D Y 2020 Acta Phys. Sin. 69 014209 Google Scholar
[25]	伊恩·古德费洛, 约书亚·本吉奥, 亚伦·库维尔著 (赵申剑, 黎彧君, 符天凡, 李凯译) 2017 深度学习 (北京:人民邮电出版社) 第143—317页 Goodfellow L, Bengio Y, Courville A（translated by Zhang SJ, Li Y J, Fu T F, Li K）2017 Deep Learning (Beijing: The People's Posts and Telecommunications Press) pp143–317 (in Chinese)

施引文献

图 1 不同链长的 J₁-J₂海森伯自旋链系统基态与第一激发态能量随J₂/J₁的变化　(a) N = 8; (b) N = 10; (c) N = 12; (d) N = 16

Fig. 1. Ground and first excited energy level diagram in J₁-J₂ Heisenberg spin chain system with chain length: (a) N = 8; (b) N = 10; (c) N = 12; (d) N = 16.

下载: 全尺寸图片幻灯片

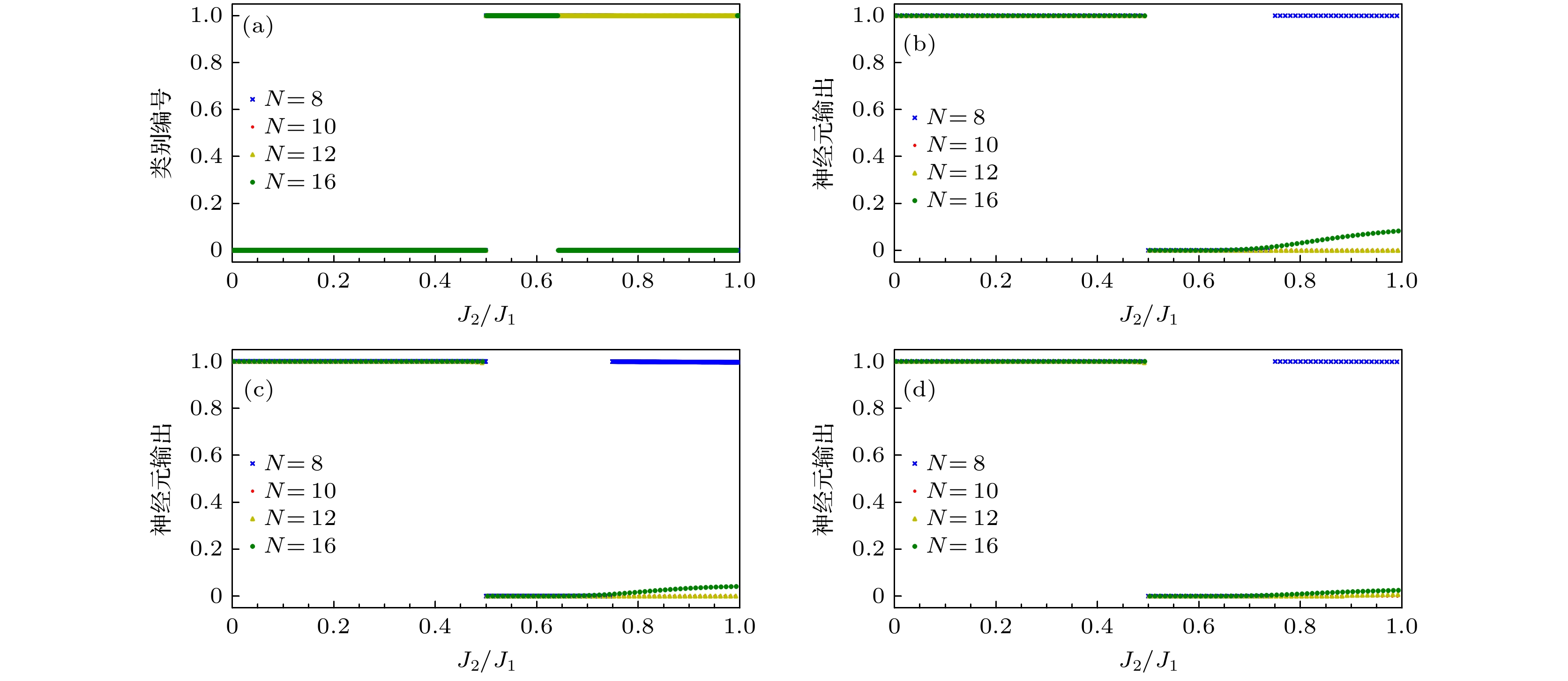
图 2 (a) 训练数据为J₂/J₁$\in $[0, 1)的海森伯J₁-J₂模型基态矢量生成的GMM对基态矢量的分类结果; (b)采用标记为0的J₂/J₁$\in $[0.35, 0.45)和为1的J₂/J₁$\in $[0.55, 0.65); (c)标记为0的J₂/J₁$\in $[0.3, 0.4)和为1的J₂/J₁$\in $[0.55, 0.65); (d)标记为0的J₂/J₁$\in $[0.2, 0.3)和为1的J₂/J₁$\in $[0.55, 0.65)的基态态矢量作为训练数据, 训练所得的CNN模型对基态态矢量的预测结果

Fig. 2. (a) Ground state vector classification results of the GMM generated by the Heisenberg J₁-J₂ model ground state vector with the training data of J₂/J₁$\in $ [0, 1); (b) using the ground state vector of J₂/J₁$\in $[0.35, 0.45) marked as 0 and J₂/J₁$\in $[0.55, 0.65) marked as 1; (c) J₂/J₁$\in $[0.3, 0.4) marked as 0 and J₂/J₁$\in $[0.55, 0.65) marked as 1; (d) J₂/J₁$\in $[0.2, 0.3) marked as 0 and J₂/J₁$\in $[0.55, 0.65) marked as 1 as training data, the prediction results of the ground state vector by the trained convolutional neural network model.

下载: 全尺寸图片幻灯片

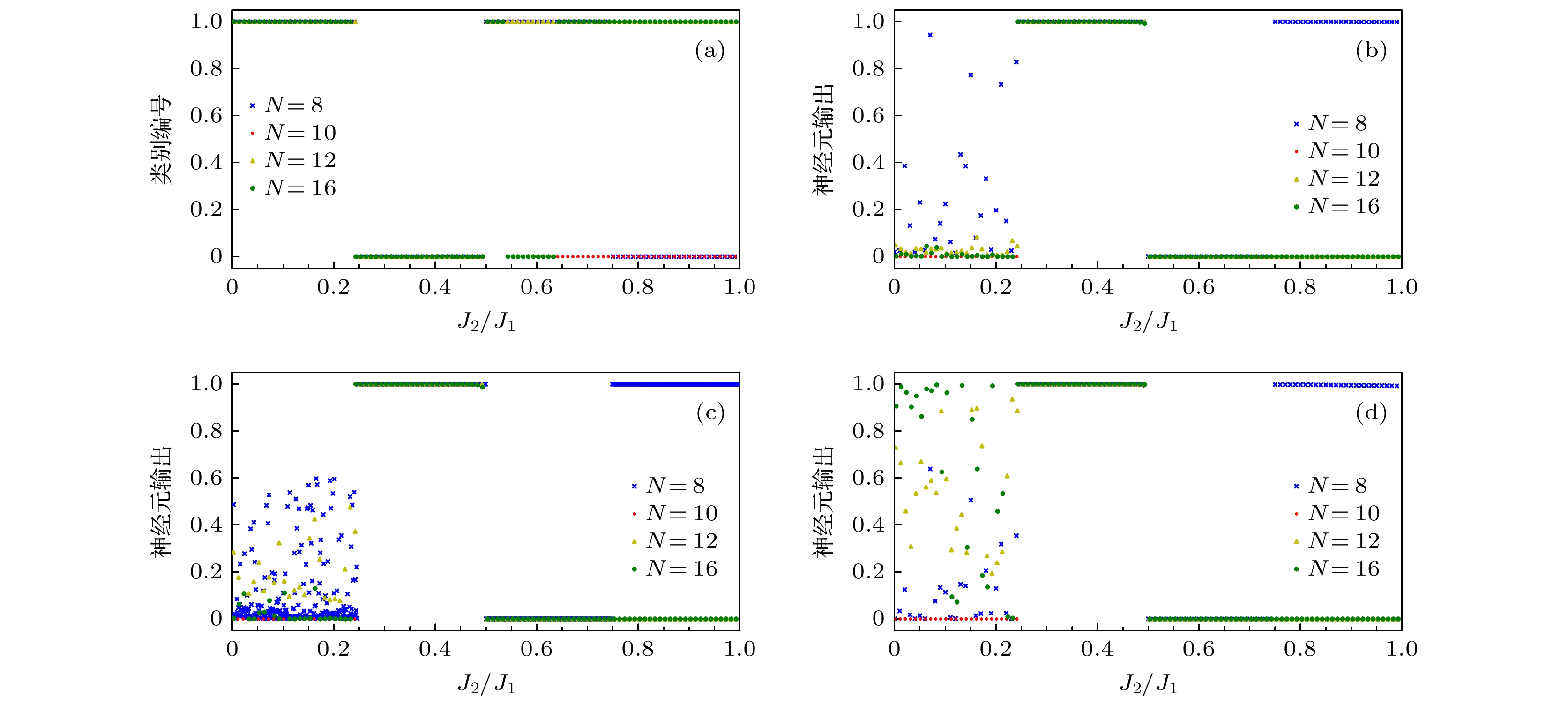
图 3 (a) 训练数据为J₂/J₁$\in $[0, 0.5)的海森伯J₁-J₂模型基态态矢量生成的GMM对基态态矢量的分类结果; (b) 分别为采用标记为0的J₂/J₁$\in $[0.25, 0.3)和为1的J₂/J₁$\in $[0.35, 0.4); (c)标记为0的J₂/J₁$\in $[0.2, 0.25)和为1的J₂/J₁$\in $[0.35, 0.4); (d)标记为0的J₂/J₁$\in $[0.2, 0.25)和为1的J₂/J₁$\in $[0.35, 0.4)(标记为1的数据是标记为0的5倍)的基态态矢量作为训练数据, 训练所得的CNN模型对基态态矢量的预测结果

Fig. 3. (a) Ground state vector classification results of the GMM generated by the Heisenberg J₁-J₂ model ground state vector with the training data of J₂/J₁$\in $ [0, 0.5); (b) respectively usingthe ground state vector of J₂/J₁$\in $[0.25, 0.3) marked as 0 and J₂/J₁$\in $[0.35, 0.4) marked as 1; (c) J₂/J₁$\in $[0.2, 0.25) marked as 0 and J₂/J₁$\in $[0.35, 0.4) marked as 1; (d) J₂/J₁$\in $[0.2, 0.25) marked as 0 and J₂/J₁$\in $[0.35, 0.4) marked as 1 (the data marked as 1 is 5 times as much as the data marked as 0)as training data, the prediction results of the ground state vector by the trained convolutional neural network model.

下载: 全尺寸图片幻灯片

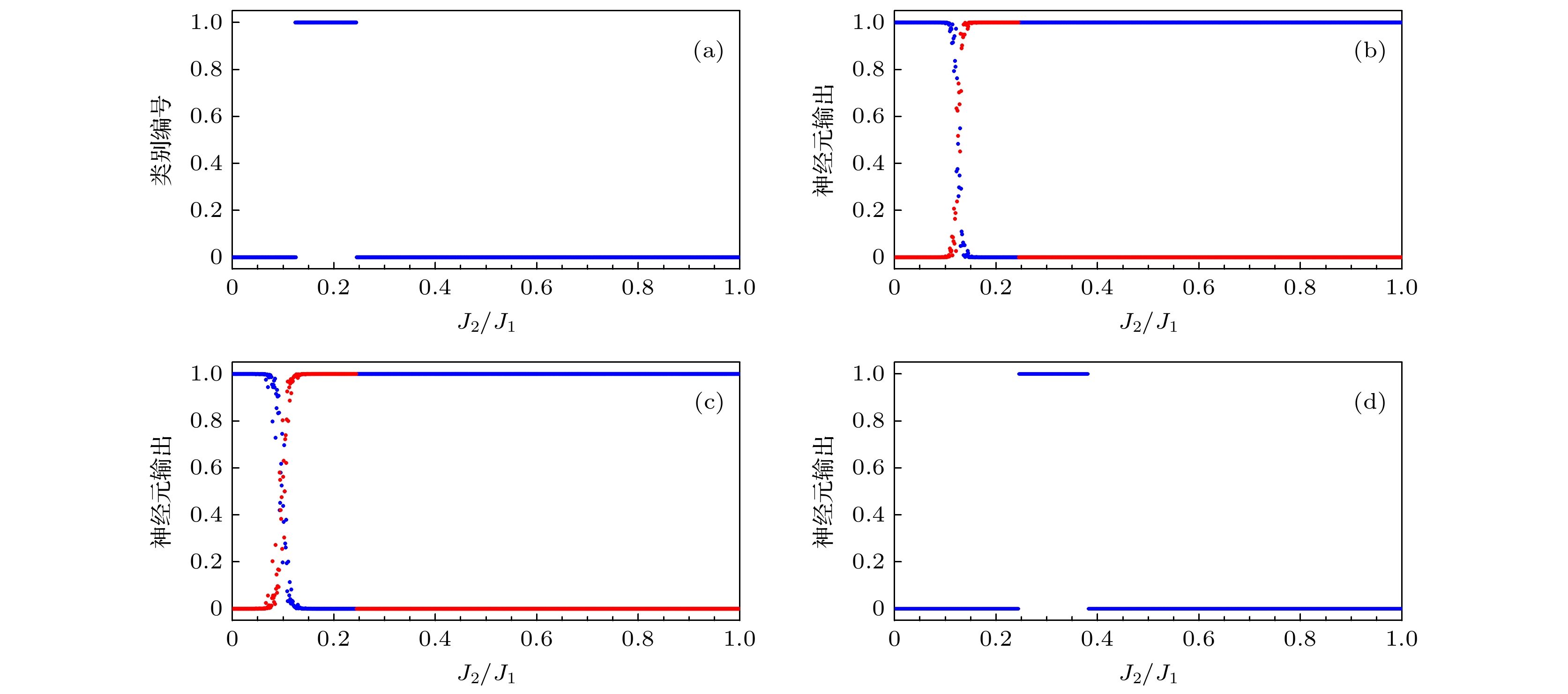
图 4 (a) 训练数据为J₂/J₁$\in $[0, 1)的海森伯J₁-J₂模型第一激发态态矢量生成的GMM对第一激发态态矢量的分类结果; (b)分别为采用标记为0的J₂/J₁$\in $[0.35, 0.45)和为1的J₂/J₁$\in $[0.55, 0.65); (c)标记为0的J₂/J₁$\in $[0.3, 0.4)和为1的J₂/J₁$\in $[0.55, 0.65); (d)标记为0的J₂/J₁$\in $[0.35, 0.45)和为1的J₂/J₁$\in $[0.55, 0.65) (标记为1的数据是标记为0的5倍)的第一激发态态矢量作为训练数据, 训练所得的CNN模型对第一激发态态矢量的预测结果

Fig. 4. (a) The first excited state vector classification results of the GMM generated by the Heisenberg J₁-J₂ model first excited state vector with the training data of J₂/J₁ $\in $ [0, 1); (b) using the first excited state vector of J₂/J₁$\in $[0.35, 0.45) marked as 0 and J₂/J₁$\in $[0.55, 0.65) marked as 1; (c) J₂/J₁$\in $[0.3, 0.4) marked as 0 and J₂/J₁$\in $[0.55, 0.65) marked as 1; (d) J₂/J₁$\in $[0.35, 0.45) marked as 0 and J₂/J₁$\in $[0.55, 0.65) marked as 1 (the data marked as 1 is 5 times as much as the data marked as 0)as training data, the prediction results of the first excited state vector by the trained convolutional neural network model.

下载: 全尺寸图片幻灯片

图 5 (a)采用标记为0的J₂/J₁$\in $[0.1, 0.2), 标记为1的J₂/J₁$\in $[0.3, 0.4); (b)标记为0的J₂/J₁$\in $[0, 0.1), 标记为1的J₂/J₁$\in $[0.3, 0.4)的第一激发态态矢量作为训练数据, 训练所得的CNN模型对第一激发态态矢量的预测结果

Fig. 5. (a) Using the first excited state vector of J₂/J₁$\in $[0.1, 0.2) marked as 0 and J₂/J₁$\in $[0.3, 0.4) marked as 1; (b)J₂/J₁$\in $[0, 0.1) marked as 0 and J₂/J₁$\in $[0.3, 0.4) marked as 1 as training data, the prediction results of the first excited state vector by the trained convolutional neural network model.

下载: 全尺寸图片幻灯片

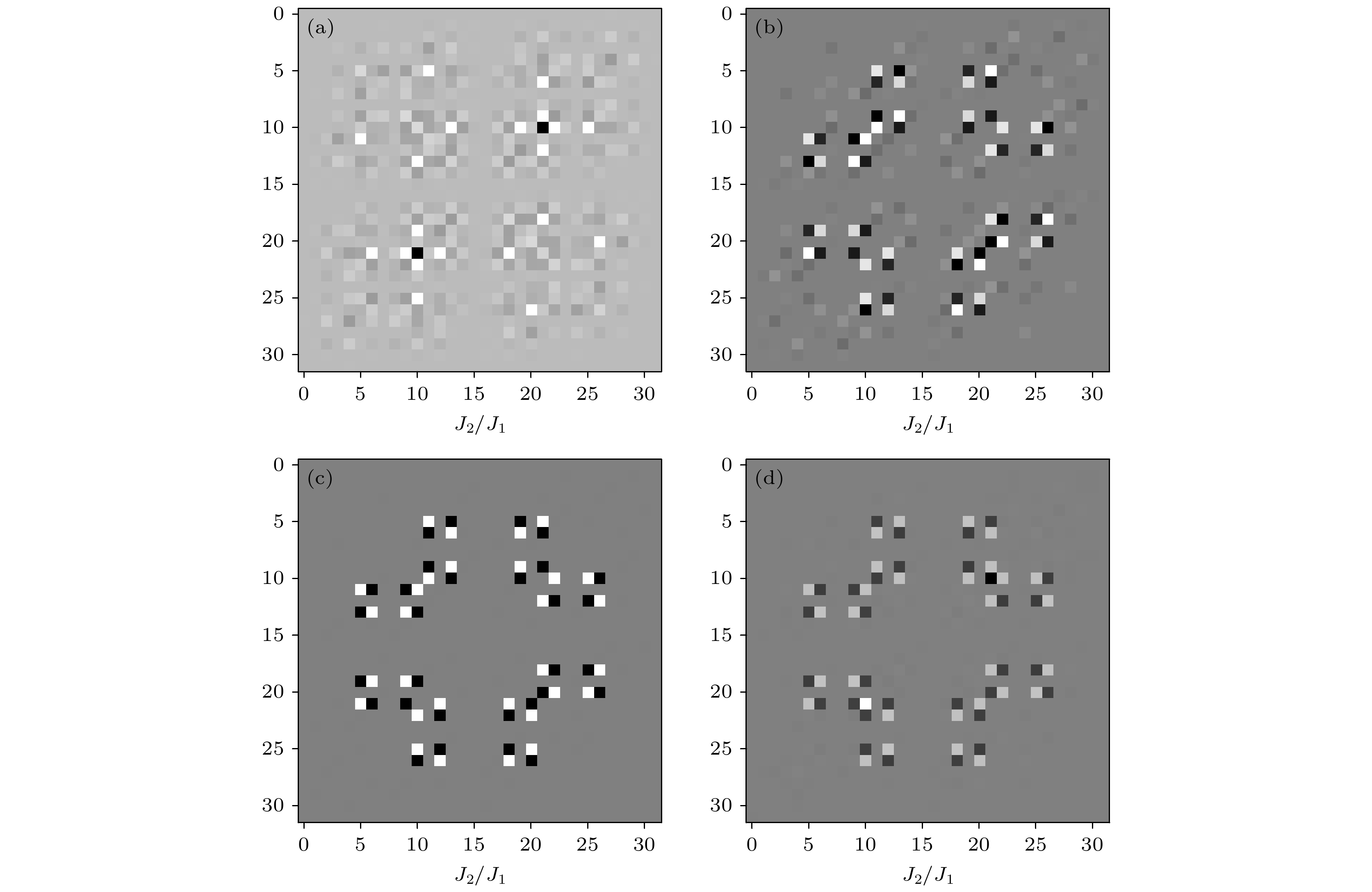
图 6 (a) 训练数据为J₂/J₁$\in $[0, 0.24)的海森伯J₁-J₂模型第一激发态态矢量生成的GMM对第一激发态态矢量的分类结果; (b), (c)分别为采用标记为0的J₂/J₁$\in $[0.05, 0.1)和为1的J₂/J₁$\in $[0.15, 0.2); 标记为0的J₂/J₁$\in $[0, 0.05)和为1的J₂/J₁$\in $[0.15, 0.2)的第一激发态态矢量作为训练数据, 训练所得的CNN模型对第一激发态态矢量的预测结果; (d) 训练数据为J₂/J₁$\in $[0.25, 0.5)的海森伯J₁-J₂模型第一激发态态矢量生成的GMM对第一激发态态矢量的分类结果

Fig. 6. (a) The first excited state vector classification results of the GMM generated by the Heisenberg J₁-J₂ model first excited state vector with the training data of J₂/J₁ $\in $ [0, 0.24); (b) (c) respectively using the first excited state vector of J₂/J₁$\in $[0.05, 0.1) marked as 0 and J₂/J₁$\in $[0.15, 0.2) marked as 1; J₂/J₁$\in $[0, 0.05) marked as 0 and J₂/J₁$\in $[0.15, 0.2) marked as 1 as training data, the prediction results of the first excited state vector by the trained convolutional neural network model; (d) he first excited state vector classification results of the GMM generated by the Heisenberg J₁-J₂ model first excited state vector with the training data of J₂/J₁$\in $[0.25, 0.5).

下载: 全尺寸图片幻灯片

图 7 海森伯 J₁-J₂模型基态态矢量变换而来的灰度图　(a) J₂/J₁ = 0.44; (b) J₂/J₁ = 0.49; (c) J₂/J₁ = 0.51; (d) J₂/J₁ = 0.58

Fig. 7. Gray scale images transformed from the ground state vector of the Heisenberg J₁-J₂ model: (a) J₂/J₁ = 0.44; (b) J₂/J₁ = 0.49; (c) J₂/J₁ = 0.51; (d) J₂/J₁ = 0.58.

下载: 全尺寸图片幻灯片

图 8 海森伯J₁-J₂模型第一激发态态矢量变换而来的灰度图　(a) J₂/J₁ = 0.24; (b) J₂/J₁ = 0.25; (c) J₂/J₁ = 0.49; (d) J₂/J₁ = 0.51

Fig. 8. Gray scale images transformed from the first excited state vector of the Heisenberg J₁-J₂ model: (a) J₂/J₁ = 0.24; (b) J₂/J₁ = 0.25; (c) J₂/J₁ = 0.49; (d) J₂/J₁ = 0.51.

下载: 全尺寸图片幻灯片

map

[1]	Lei W 2016 Phys. Rev. B. 94 195105 Google Scholar
[2]	Wetzel S J 2017 Phys. Rev. E 96 022140 Google Scholar
[3]	Huang L, Wang L 2017 Phys. Rev. B 95 035105 Google Scholar
[4]	Phiala E S, Daniel T, William D 2018 Phys. Rev. D 97 094506 Google Scholar
[5]	LakovlevI A, SotnikovO M, MazurenkoV V 2018 Phys. Rev. B 98 174411 Google Scholar
[6]	Dong X Y, Pollmann F, Zhang X F 2019 Phys. Rev. B 99 121104 Google Scholar
[7]	Tan D R, Jiang F J 2020 Phys. Rev. B 102 224434 Google Scholar
[8]	Tan D R, Li C D, Zhu W P, Jiang F J 2020 New J. Phys. 22 063016 Google Scholar
[9]	Maskara N, Buchhold M, Endres M 2021 arXiv: 2103.15855 [quant-ph]
[10]	Tanja D 2021 arXiv: 2103.07236[quant-ph]
[11]	Sondhi S L, Girvin S M, Carini J P, Shahar D 1997 Rev. Mod. Phys 69 315 Google Scholar
[12]	Bulla R, Vojta M 2003 Rep. Prog. Phys. 66 2069 Google Scholar
[13]	Walker N, Tam K M, Novak B, Jarrell M 2018 Phys. Rev. E. 98 053305 Google Scholar
[14]	Jadrich R B, Lindquist B A, Pineros W D, Truskeet T M 2018 J. Chem. Phys. 149 194109 Google Scholar
[15]	Canabarro A, Fanchini F F, Malvezzi A L, Pereira R, Chaves R 2019 Phys. Rev. B 100 045129 Google Scholar
[16]	Carrasquilla J, Melko R G 2017 Nat. Phys. 13 431 Google Scholar
[17]	Ahmadreza A, Michel P 2020 arXiv: 2007.09764 [cond-mat. stat-mech]
[18]	Chitra R, Pati S, Krishnamurthy H R, Sen D, Ramasesha S 1995 Phys. Rev. B 52 6581 Google Scholar
[19]	Castilla G, Chakravarty S, Emery V J 1995 Phys. Rev. L 75 1823 Google Scholar
[20]	Shu C, Li W, Shi J G, Wang Y P 2007 Phys. Rev. E 76 061108 Google Scholar
[21]	Qian X F, Shi T, Li Y, Song Z, Sun C P 2005 Phys. Rev. A 72 012333 Google Scholar
[22]	周志华 2016 机器学习 (北京: 清华大学出版社) 第206页 Zhou Z H 2016 Machine Learning (Beijing: Tsinghua University Press) p206 (in Chinese)
[23]	李航 2012 统计机器学习 (北京: 清华大学出版社) 第162页 Li H 2012The Elements of Statistical Learning (Beijing: Tsinghua University Press) p162 (in Chinese)
[24]	徐启伟, 王佩佩, 曾镇佳, 黄泽斌, 周新星, 刘俊敏, 李瑛, 陈书青, 范滇元 2020 69 014209 Google Scholar Xu Q W, Wang P P, Zeng Z J, Huang Z B, Zhou X X, Liu J M, Li Y, Chen S Q, Fan D Y 2020 Acta Phys. Sin. 69 014209 Google Scholar
[25]	伊恩·古德费洛, 约书亚·本吉奥, 亚伦·库维尔著 (赵申剑, 黎彧君, 符天凡, 李凯译) 2017 深度学习 (北京:人民邮电出版社) 第143—317页 Goodfellow L, Bengio Y, Courville A（translated by Zhang SJ, Li Y J, Fu T F, Li K）2017 Deep Learning (Beijing: The People's Posts and Telecommunications Press) pp143–317 (in Chinese)

[1]	张嘉晖. 蛋白质计算中的机器学习. , 2024, 73(6): 069301. doi: 10.7498/aps.73.20231618
[2]	欧阳鑫健, 张岩星, 王之龙, 张锋, 陈韦嘉, 庄园, 揭晓, 刘来君, 王大威. 面向铁电相变的机器学习: 基于图卷积神经网络的分子动力学模拟. , 2024, 73(8): 086301. doi: 10.7498/aps.73.20240156
[3]	罗启睿, 沈一凡, 罗孟波. 高分子塌缩相变和临界吸附相变的计算机模拟和机器学习. , 2023, 72(24): 240502. doi: 10.7498/aps.72.20231058
[4]	张逸凡, 任卫, 王伟丽, 丁书剑, 李楠, 常亮, 周倩. 机器学习结合固溶强化模型预测高熵合金硬度. , 2023, 72(18): 180701. doi: 10.7498/aps.72.20230646
[5]	郭唯琛, 艾保全, 贺亮. 机器学习回归不确定性揭示自驱动活性粒子的群集相变. , 2023, 72(20): 200701. doi: 10.7498/aps.72.20230896
[6]	田城, 蓝剑雄, 王苍龙, 翟鹏飞, 刘杰. BaF ₂高压相变行为的第一性原理研究. , 2022, 71(1): 017102. doi: 10.7498/aps.71.20211163
[7]	赵中华, 渠广昊, 姚佳池, 闵道敏, 翟鹏飞, 刘杰, 李盛涛. 热峰作用下单斜ZrO₂相变过程的分子动力学模拟. , 2021, 70(13): 136101. doi: 10.7498/aps.70.20201861
[8]	田城, 蓝剑雄, 王苍龙, 翟鹏飞, 刘杰. BaF2高压相变行为的第一性原理研究. , 2021, (): . doi: 10.7498/aps.70.20211163
[9]	刘武, 朱成皖, 李昊天, 赵谡玲, 乔泊, 徐征, 宋丹丹. 基于机器学习和器件模拟对Cu(In,Ga)Se₂电池中Ga含量梯度的优化分析. , 2021, 70(23): 238802. doi: 10.7498/aps.70.20211234
[10]	张瑶, 张云波, 陈立. 基于深度学习的光学表面杂质检测. , 2021, 70(16): 168702. doi: 10.7498/aps.70.20210403
[11]	孙立望, 李洪, 汪鹏君, 高和蓓, 罗孟波. 利用神经网络识别高分子链在表面的吸附相变. , 2019, 68(20): 200701. doi: 10.7498/aps.68.20190643
[12]	徐婷婷, 李毅, 陈培祖, 蒋蔚, 伍征义, 刘志敏, 张娇, 方宝英, 王晓华, 肖寒. 基于AZO/VO2/AZO结构的电压诱导相变红外光调制器. , 2016, 65(24): 248102. doi: 10.7498/aps.65.248102
[13]	曲艳东, 孔祥清, 李晓杰, 闫鸿浩. 热处理对爆轰合成的纳米TiO2混晶的结构相变的影响. , 2014, 63(3): 037301. doi: 10.7498/aps.63.037301
[14]	刘志强, 常胜江, 王晓雷, 范飞, 李伟. 基于VO2薄膜相变原理的温控太赫兹超材料调制器. , 2013, 62(13): 130702. doi: 10.7498/aps.62.130702
[15]	李炎, 唐刚, 宋丽建, 寻之朋, 夏辉, 郝大鹏. Erds Rnyi随机网络上爆炸渗流模型相变性质的数值模拟研究. , 2013, 62(4): 046401. doi: 10.7498/aps.62.046401
[16]	韩秀琴, 姜虹, 石玉仁, 刘妍秀, 孙建华, 陈建敏, 段文山. 一维 Frenkel-Kontorova(FK)模型原子链的相变研究. , 2011, 60(11): 116801. doi: 10.7498/aps.60.116801
[17]	樊华, 李理, 袁坚, 山秀明. 互联网流量控制的朗之万模型及相变分析. , 2009, 58(11): 7507-7513. doi: 10.7498/aps.58.7507
[18]	石筑一, 吉世印. 微观核芯+两准粒子模型中热核148—158Sm的比热容及其相变. , 2003, 52(1): 42-47. doi: 10.7498/aps.52.42
[19]	袁坚, 任勇, 刘锋, 山秀明. 复杂计算机网络中的相变和整体关联行为. , 2001, 50(7): 1221-1225. doi: 10.7498/aps.50.1221
[20]	. 神经网络的自适应删剪学习算法及其应用. , 2001, 50(4): 674-681. doi: 10.7498/aps.50.674

计量

文章访问数: 4509
PDF下载量: 112
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

基于机器学习J₁-J₂反铁磁海森伯自旋链相变点的识别方法