-
合金化是增加材料结构和性能多样性的重要手段. 本文先从考虑最近邻相互作用的Ising模型出发, 通过铁磁耦合研究二元合金的低温相分离、高温固溶体系, 通过反铁磁耦合研究低温有序固溶、高温无序体系. 以储氢合金中的Laves相V2x Fe2(1–x)Zr和ScxY1–x Fe2材料为例, 采用基于结构识别的高通量第一原理计算, 考虑结构简并度对配分函数的贡献, 可以对合金材料进行有限温度下的理论预测. 先通过第一原理计算得到基态 (零温下) 形成能, 形成能大于零的体系ScxY1–x Fe2在低温下相分离, 根据自由能符号确定合金固溶的临界温度; 形成能小于零的体系V2x Fe2(1–x)Zr在低温下倾向于形成有序相, 根据比热的计算可以确定体系出现有序-无序转变的临界温度. 其中, 高通量第一原理计算和对应的结构简并度统计可以通过我们课题组发布的程序SAGAR (structures of alloy generation and recognition)实现.Alloying is an important way to increase the diversity of material structure and properties. In this paper, we start from Ising model considering nearest neighbor interaction, in which a ferromagnetic system corresponds to a low temperature phase separation and high temperature solid solution of binary alloy, while antiferromagnetic system corresponds to a low temperature ordered solid solution and a high temperature disorder. The high-throughput first-principles calculation based on the structure recognition is realized by the program SAGAR (structures of alloy generation and recognition) developed by our research group. By considering the contribution of structural degeneracy to the partition function, theoretical prediction of alloy materials can be carried out at finite temperature. Taking hydrogen storage alloy (ScxY1–x Fe2 and V2x Fe2(1–x)Zr) for example, the formation energy of ground state (at zero temperature) can be obtained by the first-principles calculations. It is found that the formation energy of ScxY1–x Fe2 is greater than zero, thereby inducing the phase separation at low temperature. The free energy will decrease with the temperature and concentration increasing, where the critical temperature of solid solution of alloy is determined according to the zero point of free energy. The formation energies of V2x Fe2(1–x)Zr are all lower than zero, and the ordered phase occurs at low temperature. The order-disorder transition temperature of V0.5Fe1.5Zr and V1.5Fe0.5Zr are both about 100 K, while the transition temperature of VFeZr is nearly 50 K. The calculation process will effectively improve the high throughput screening efficiency of alloy, and also provide relevant theoretical reference for experimental research.
-
Keywords:
- alloy /
- structural optimization /
- solution temperature /
- phase diagram
[1] Le T, Epa V C, Burden F R, Winkler D A 2012 Chem. Rev. 5 112
Google Scholar
[2] Oganov A R, Pickard C J, Zhu Q, Needs R J 2019 Nat. Rev. Mater. 5 4
Google Scholar
[3] Woodley S M, Catlow R 2008 Nat. Mater. 12 7
Google Scholar
[4] Wang Y, Lv J, Zhu L, Ma Y 2010 Phys. Rev. B 82 094116
Google Scholar
[5] Lyakhov A O, Oganov A R, Stokes H T, Zhu Q 2013 Comput. Phys. Commun. 4 184
Google Scholar
[6] Pickard C J, Needs R J 2011 J. Phys. Condens. Matter 5 23
Google Scholar
[7] 黄文军, 乔君威, 陈顺华, 王雪姣, 吴玉程 2021 70 106201
Google Scholar
Huang W J, Qiao J W, Chen S H, Wang X J, Wu Y C 2021 Acta Phys. Sin. 70 106201
Google Scholar
[8] Li Z M, Wang H, Ouyang L Z, Liu J W, Zhu M 2016 J. Alloys Compd. 689 154865
Google Scholar
[9] 王鹏程, 曹亦, 谢红光, 殷归, 王伟, 王泽蓥, 马欣辰, 王琳, 黄维 2020 69 117501
Google Scholar
Wang P C, Cao Y, Xie H G, Yin Y, Wang W, Wang Z Y, Ma X C, Wang L, Huang W 2020 Acta Phys. Sin. 69 117501
Google Scholar
[10] 王大能, Olsen A, 叶恒强 1985 34 681
Google Scholar
Wang D N, Olsen A, Ye H Q 1985 Acta Phys. Sin. 34 681
Google Scholar
[11] van de Walle A 2008 Nat. Mater. 7 455
Google Scholar
[12] Hart G L W, Blum V, Walorski M J, Zunger A 2005 Nat. Mater. 4 391
Google Scholar
[13] Yuge K 2009 Phys. Rev. B 79 144109
Google Scholar
[14] Zunger A, Wei S H, Ferreira L G, Bernard J E 1990 Phys. Rev. Lett. 65 353
Google Scholar
[15] Xia Z G, Liu G K, Wen J G, Mei Z G, Balasubramanian M, Molokeev M S, Peng L C, Gu L, Miller D J, Liu Q L, Poeppelmeier K R 2016 J. Am. Chem. Soc. 138 1158
Google Scholar
[16] Banerjee S, Kumar A, Pillai C G S 2014 Intermetallics 51 30
Google Scholar
[17] Budzyński M, Sarzyński J, Wiertel M, Surowiec Z 2001 Acta Phys. Pol. A 100 717
Google Scholar
[18] Li X X, Yang C, Lu H Z, Luo X, Li Y Y, Ivasishin O M 2019 J. Alloys Compd. 787 112
Google Scholar
[19] Luo S L, Li T S, Wang X J, Faizan M, Zhang L J 2021 Comput. Mol. Sci. 11 7690
Google Scholar
[20] 何长春, 廖继海, 杨小宝 2017 66 163601
Google Scholar
He C C, Liao J H, Yang X B 2017 Acta Phys. Sin. 66 163601
Google Scholar
[21] Xu S G, Li X T, Zhao Y J, Liao J H, Xu W P, Yang X B, Xu H 2017 J. Am. Chem. Soc. 134 48
Google Scholar
[22] Cheng Y H, Liao J H, Zhao Y J, Ni J, Yang X B 2019 Carbon 154 140
Google Scholar
[23] Hart G L W, Forcade R W 2008 Phys. Rev. B 77 224115
Google Scholar
[24] Cheng Y H, Liao J H, Zhao Y J, Yang X B 2017 Sci. Rep. 7 16211
Google Scholar
[25] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[26] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
Google Scholar
[27] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[28] Perdew J P, Burke K, Ernzerhof M 1998 Phys. Rev. Lett. 80 891
Google Scholar
[29] Yuan S R, Ouyyang L Z, Zhu M, Zhao Y J 2018 J. Magn. Magn. Mater. 460 61
Google Scholar
[30] 汪志诚 2013 热力学·统计物理 (北京: 高等教育出版社) 第256页
Wang Z C 2013 Thermodynamics·Statistical Physics (5th Ed.) (Beijing: Higher Education Press) p256 (in Chinese)
[31] Sivardière J, Lajzerowicz J 1975 Phys. Rev. A 11 2090
Google Scholar
[32] Jiang Y L, Chen Y Z, Wang H, Yang X B 2020 Phys. Lett. A 284 126658
Google Scholar
-
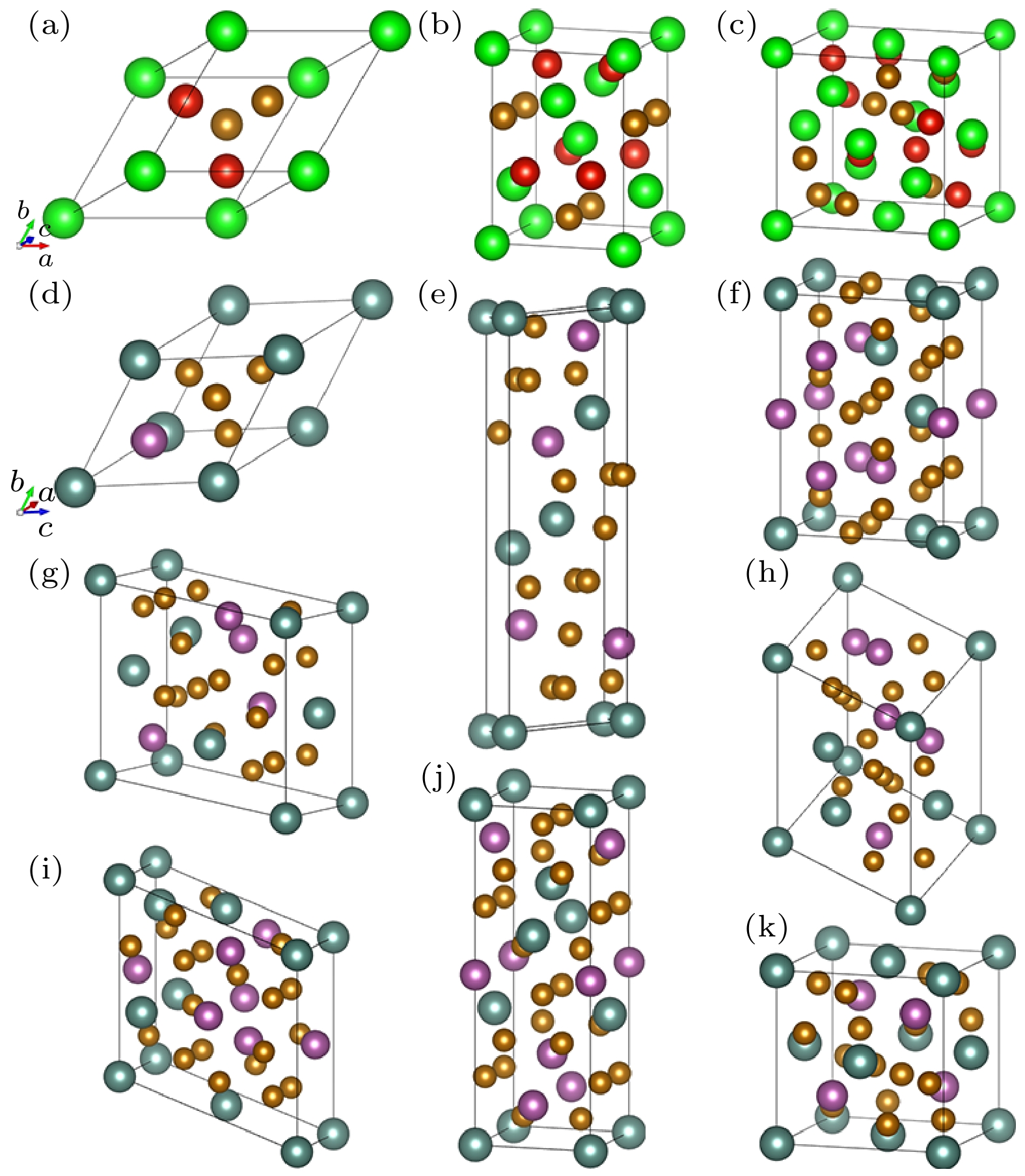
图 1 取代含量为半数时的一种构型下, V2x Fe2(1–x)Zr的(a)原胞结构和体积扩大为原胞(b) 2倍、(c) 4倍时对应的晶格; ScxY1–x Fe2合金体系的(d)原胞结构和(e)—(f)体积扩大为原胞4倍时对应的7种晶格(红色、绿色、黄色、青色和紫色小球分别代表 V, Zr, Fe, Y和Sc原子)
Fig. 1. Lattices of V2x Fe2(1–x)Zr (a) primitive cell and corresponding to volumes expanded respectively from primitive cell by (b) 2 times, (c) 4 times under a half of replacement content. (d) Primitive cell structure of ScxY1–x Fe2 alloys system and (e)−(f) the 7 kinds of lattices with 4 times volume of that of primitive one (red, green, yellow cyan and purple sphere represent respectively V, Zr, Fe, Y and Sc atom).
图 2 根据Ising模型拟合结果得出的体系 (a)自由能符号; (c)热容; (b), (d)相转变温度
Fig. 2. Systems obtained according to the fitting results of Ising model: (a) Free energy signal; (c) heat capacity; (b), (d) phase transition temperature.
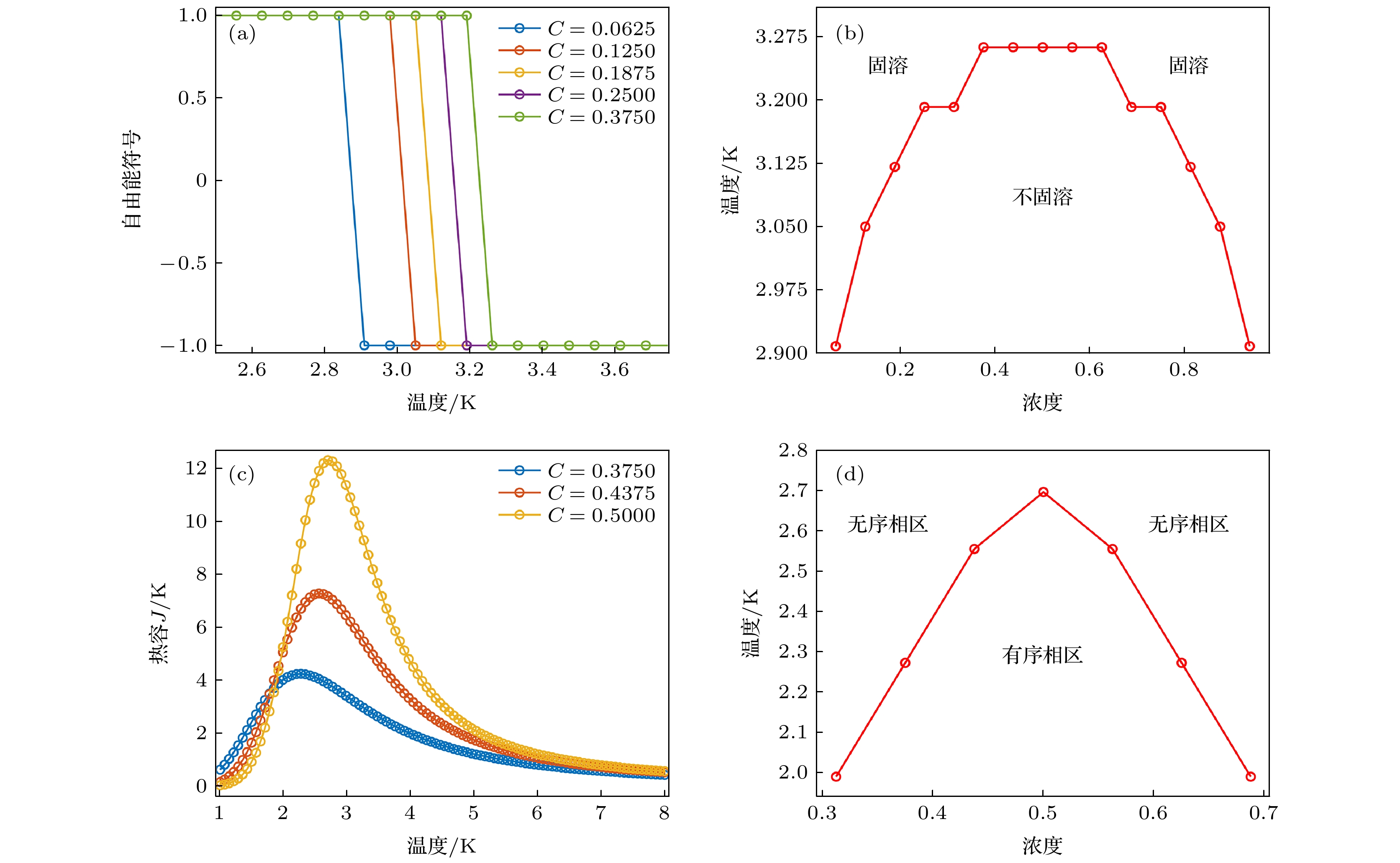
图 3 (a) ScxY1–x Fe2 体系立方晶格中不同取代浓度下随温度变化的自由能符号; (b) 1/8和(c) 5/8取代浓度下不同晶格结构随温度变化的自由能; (d) ScxY1–x Fe2体系温度-浓度相图
Fig. 3. (a) Free energy signal induced by temperature under different replacement concentration in ScxY1–x Fe2 cubic structure. Free energy versus temperature for different crystal structures at (b) 1/8 and (c) 5/8 replacement concentration, respectively. (d) Temperature-concentration phase diagram of ScxY1–x Fe2 alloy system
图 4 (a) V2x Fe2(1–x)Zr体系的温度-浓度相图(图中浓度指代为
$ x $ ); (b) V0.5Fe1.5Zr, (c) VFeZr和(d) V1.5Fe0.5Zr组分及邻近组分合金结构的热容Fig. 4. (a) Phase diagram of temperature versus concentration in V2x Fe2(1–x)Zr system (concentration in Fig. 4 is devoted to x). Heat capacities of (b) V0.5Fe1.5Zr, (c) VFeZr and (d) V1.5Fe0.5Zr components and their adjacent components
-
[1] Le T, Epa V C, Burden F R, Winkler D A 2012 Chem. Rev. 5 112
Google Scholar
[2] Oganov A R, Pickard C J, Zhu Q, Needs R J 2019 Nat. Rev. Mater. 5 4
Google Scholar
[3] Woodley S M, Catlow R 2008 Nat. Mater. 12 7
Google Scholar
[4] Wang Y, Lv J, Zhu L, Ma Y 2010 Phys. Rev. B 82 094116
Google Scholar
[5] Lyakhov A O, Oganov A R, Stokes H T, Zhu Q 2013 Comput. Phys. Commun. 4 184
Google Scholar
[6] Pickard C J, Needs R J 2011 J. Phys. Condens. Matter 5 23
Google Scholar
[7] 黄文军, 乔君威, 陈顺华, 王雪姣, 吴玉程 2021 70 106201
Google Scholar
Huang W J, Qiao J W, Chen S H, Wang X J, Wu Y C 2021 Acta Phys. Sin. 70 106201
Google Scholar
[8] Li Z M, Wang H, Ouyang L Z, Liu J W, Zhu M 2016 J. Alloys Compd. 689 154865
Google Scholar
[9] 王鹏程, 曹亦, 谢红光, 殷归, 王伟, 王泽蓥, 马欣辰, 王琳, 黄维 2020 69 117501
Google Scholar
Wang P C, Cao Y, Xie H G, Yin Y, Wang W, Wang Z Y, Ma X C, Wang L, Huang W 2020 Acta Phys. Sin. 69 117501
Google Scholar
[10] 王大能, Olsen A, 叶恒强 1985 34 681
Google Scholar
Wang D N, Olsen A, Ye H Q 1985 Acta Phys. Sin. 34 681
Google Scholar
[11] van de Walle A 2008 Nat. Mater. 7 455
Google Scholar
[12] Hart G L W, Blum V, Walorski M J, Zunger A 2005 Nat. Mater. 4 391
Google Scholar
[13] Yuge K 2009 Phys. Rev. B 79 144109
Google Scholar
[14] Zunger A, Wei S H, Ferreira L G, Bernard J E 1990 Phys. Rev. Lett. 65 353
Google Scholar
[15] Xia Z G, Liu G K, Wen J G, Mei Z G, Balasubramanian M, Molokeev M S, Peng L C, Gu L, Miller D J, Liu Q L, Poeppelmeier K R 2016 J. Am. Chem. Soc. 138 1158
Google Scholar
[16] Banerjee S, Kumar A, Pillai C G S 2014 Intermetallics 51 30
Google Scholar
[17] Budzyński M, Sarzyński J, Wiertel M, Surowiec Z 2001 Acta Phys. Pol. A 100 717
Google Scholar
[18] Li X X, Yang C, Lu H Z, Luo X, Li Y Y, Ivasishin O M 2019 J. Alloys Compd. 787 112
Google Scholar
[19] Luo S L, Li T S, Wang X J, Faizan M, Zhang L J 2021 Comput. Mol. Sci. 11 7690
Google Scholar
[20] 何长春, 廖继海, 杨小宝 2017 66 163601
Google Scholar
He C C, Liao J H, Yang X B 2017 Acta Phys. Sin. 66 163601
Google Scholar
[21] Xu S G, Li X T, Zhao Y J, Liao J H, Xu W P, Yang X B, Xu H 2017 J. Am. Chem. Soc. 134 48
Google Scholar
[22] Cheng Y H, Liao J H, Zhao Y J, Ni J, Yang X B 2019 Carbon 154 140
Google Scholar
[23] Hart G L W, Forcade R W 2008 Phys. Rev. B 77 224115
Google Scholar
[24] Cheng Y H, Liao J H, Zhao Y J, Yang X B 2017 Sci. Rep. 7 16211
Google Scholar
[25] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[26] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
Google Scholar
[27] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[28] Perdew J P, Burke K, Ernzerhof M 1998 Phys. Rev. Lett. 80 891
Google Scholar
[29] Yuan S R, Ouyyang L Z, Zhu M, Zhao Y J 2018 J. Magn. Magn. Mater. 460 61
Google Scholar
[30] 汪志诚 2013 热力学·统计物理 (北京: 高等教育出版社) 第256页
Wang Z C 2013 Thermodynamics·Statistical Physics (5th Ed.) (Beijing: Higher Education Press) p256 (in Chinese)
[31] Sivardière J, Lajzerowicz J 1975 Phys. Rev. A 11 2090
Google Scholar
[32] Jiang Y L, Chen Y Z, Wang H, Yang X B 2020 Phys. Lett. A 284 126658
Google Scholar
下载:
计量
- 文章访问数: 5525
- PDF下载量: 104
- 被引次数: 0
