-
Rare-earth iron garnet films with perpendicular magnetic anisotropy could open new perspectives for spintronics. Holmium iron garnet (Ho3Fe5O12, HoIG) films with thickness ranging from 2 to 100 nm are epitaxially grown on (111) orientated gadolinium gallium garnet single crystal substrate doped with yttrium and scandium (Gd0.63Y2.37Sc2Ga3O12, GYSGG) by ultra-high vacuum magnetron sputtering. A 3-nm Pt film is further deposited on each of the HoIG films. The magnetic anisotropy and magneto-transport properties of heterostructures at room temperature are investigated. It is shown that the HoIG film as thin as 2 nm (less than two unit cells in thickness) exhibits the ferromagnetic properties at room temperature, and perpendicular magnetic anisotropy is achieved in the 2-60 nm thick films, and a maximum effective perpendicular anisotropy field reaches 350 mT due to the strain induced magnetoelastic anisotropy. The HoIG/Pt heterostructure shows significant anomalous Hall effect (AHE) and appreciable spin-Hall magnetoresistance (SMR) and/or anisotropic magnetoresistance (AMR). Remarkably, the AHE starts to decline gradually when the HoIG thickness is less than 4 nm, but the magnetoresistance decreases rapidly with the HoIG layer becoming less than 7 nm in thickness. The fact that the AHE in the heterostructure is less sensitive to the HoIG thickness suggests that the interface effect is more dominant in the AHE mechanism, whereas the bulk magnetic properties of the HoIG plays a more important role for the observed magnetoresistance. In addition, the spin Seebeck effect decreases exponentially with the decrease of HoIG thickness till the ultrathin limit, which was previously validated in the micrometer-thick YIG/Pt stacks in the frame of thermally excited magnon accumulation and propagation. The present results show that the nanometer HoIG/Pt heterostructure with tunable perpendicular magnetic anisotropy and efficient interfacial spin exchange interaction could be a promising candidate for insulating magnet based spintronic devices.
[1] 沃尔法斯E P著 (刘增民 等 译) 1993 铁磁材料(卷二)(北京: 电子工业出版社) 第1, 188, 225页
Wohlfarth E P (translated by Liu Z M) 1993 Ferromagnetic Materials (Vol. 2) (Beijing: Electronics Industry Press) pp1, 188, 225 (in Chinese)
[2] Uchida K, Takahashi S, Harii K, Ieda J, Koshibae W, Ando K, Maekawa S and Saitoh E 2008 Nature 455 778
Google Scholar
[3] Jaworski C M, Yang J, Mack S, Awschalom D D, Heremans J P, Myers R C, 2010 Nat. Mater. 9 898
Google Scholar
[4] Lang M, montazeri M, Onbasli M C, Kou X F, Fan Y, Upadhyaya P, Yao K, Liu F, Jiang Y, Jiang W J, Wong K L, Yu G Q, Tang J S, Nie T X, He L, Schwartz B N, Wang Y, Ross C A and Wang K L 2014 Nano Lett. 14 3459
Google Scholar
[5] Jiang Z, Chang C Z, Tang C, Wei P, Moodera J S, Shi J 2015 Nano Lett. 15 5835
Google Scholar
[6] Liu L, Lee O J, Gudmundsen T, Ralph D, Buhrman R 2012 Phys. Rev. Lett. 109 096602
Google Scholar
[7] Li P, Liu T, Chang H, Kalitsov A, Zhang W, Csaba G, Li W, Richardson D, Demann A, Rimal G, Dey H, Jiang J S, porod W, Field S B, Tang J, Marconi M C, Hoffmann A, Mryasov O, Wu M Z 2016 Nat. Commun. 7 12688
Google Scholar
[8] Avci C O, Rosenberg E, Baumgartner, Beran L, Quindeau A, Gambardella P, Ross C A, Beach C S D 2017 Appl. Phys. Lett. 111 072406
Google Scholar
[9] Kamada O, Nakaya T and Higuchi S 2005 Sens. Actuators, A 119 345
Google Scholar
[10] Xiao J and Bauer G E W 2012 Phys. Rev. Lett. 108 217204
Google Scholar
[11] Lee S W and Lee K J 2016 Proc. IEEE 104 1831
Google Scholar
[12] 郭贻诚 著 2014 铁磁学 (北京: 北京大学出版社) 第171页
Guo Y C 2014 Ferromagnetics (Beijing: Peking Univercity Press) p171 (in Chinese)
[13] 郝俊祥, 杨青慧, 张怀武, 等 2018 67 117801
Google Scholar
Hao J X, Yang Q H, Zhang H W, et al. 2018 Acta. Phys. Sin. 67 117801
Google Scholar
[14] Chen J L, Wang C T, Liu C P, 2019 Appl. Phy. Lett. 114 212401
Google Scholar
[15] Li G, Bai H, Su J 2019 APL Mater. 7 041104
Google Scholar
[16] Kubota M, Tsukazaki A, Kagawa F, Shibuya K, Tokunaga Y, Kawasaki M, Tokura Y 2012 Appl. Phys. Express. 5 103002
Google Scholar
[17] Rosenberg E R, Beran L, Avci C O, Zeledon C, Song B, Gonzalez-Fuentes C, Mendil J, Gambardella P, Veis M, Garcia C, Beach G S D, Ross C A 2018 Phys. Rev. M 2 094405
Google Scholar
[18] Yamahara H, Mikami M, Seki M, Tabata H 2011 J.M.M.M. 323 3143
Google Scholar
[19] Bauer J J, Rosenberg E R, Kundu S, Mkhoyan K A, Quarterman P, Grutter A J, Kirby B J, Borchers J A, Ross C A 2020 Adv. Elctron. Mater. 6 1900820
Google Scholar
[20] Zanjani S M, Onbasli M C 2019 AIP Adv. 9 035024
Google Scholar
[21] Nakayama H, Althammer M, Chen Y T, Uchida K, Kajiwara Y, Kikuchi D, Ohtani T, Geprags S, Opel M 2013 Phys. Rev. Lett. 110 206601
Google Scholar
[22] Lu Y M, Choi Y, Ortega C M, Cheng X M, Cai J W, Huang S Y, Sun L, Chien C L 2013 Phys. Rev. Lett. 110 147207
Google Scholar
[23] Tang C, Sellappan P, Liu Y, Xu Y, Garay J E, Shi J 2016 Phys. Rev. B 94 140403(R
Google Scholar
[24] Aldosary M, Li J, Tang C, Xu Y, Zheng J-G, Bozhilov K N, Shi J 2016 Appl. Phys. Lett. 108 242401
Google Scholar
[25] Chen Y-T, Takahashi S, Nakayama H, Althammer M, Goennenwein S T B, Saitoh E, Bauer G E W 2013 Phys. Rev. B 87 144411
Google Scholar
[26] Bai H, Zhan X Z, Li G, Su J, Zhu Z Z, Zhang Y, Zhu T, Cai J W 2019 Appl. Phys. Lett. 115 182401
Google Scholar
[27] Bergholz R, Gradmann U 1984 J.M.M.M. 45 389
Google Scholar
[28] Zhou L K, Zhang Y, Gu L, Cai J W, Sun L 2016 Phys. Rev. B 93 075309
Google Scholar
[29] Philippi-Kobs A, Farhadi A, Matheis L, Chuvilin L A, Oepen H P 2019 Phys. Rev. Lett. 123 137201
Google Scholar
[30] Guo E-J, CraMer J, Kehlberger A, Ferguson C A, MacLaren D A, Jakob G, Klaul M 2016 Phys. Rev. X 6 031012
Google Scholar
[31] Prakash A, Flebus B, Brangham J, Yang F, Tserkovnyak, Heremans J P 2018 Phys. Rev. B 97 020408
Google Scholar
[32] Kikkawa T, Uchida K, Daimon S, Qiu Z, Shiomi Y, Saitoh E 2015 Phys. Rev. B 92 064413
Google Scholar
-
图 1 (a) 10 nm和(b) 40 nm HoIG薄膜的X射线衍射图, 图中虚线为块体HoIG (444)衍射峰位, 图中插图分别为对应HoIG薄膜的(444)衍射峰的摇摆曲线. (c) 10 nm和(d) 40 nm HoIG薄膜围绕其(486)衍射的X射线衍射倒易空间图, 虚线对应衬底
$(486) _{//}$ [$ 1\overline 1 0$ ]的qx值Figure 1. XRD spectra for (a)10 nm and (b) 40 nm HoIG film, the dashed line is the (444) peak position of bulk HoIG, the inset is rocking curve of 10 nm and 40 nm HoIG (444) peak, respectively. The reciprocal space mapping of XRD around (486) diffraction for (c) 10 nm and (d) 40 nm HoIG film, the dashed line corresponds to the qx value of the GYSGG
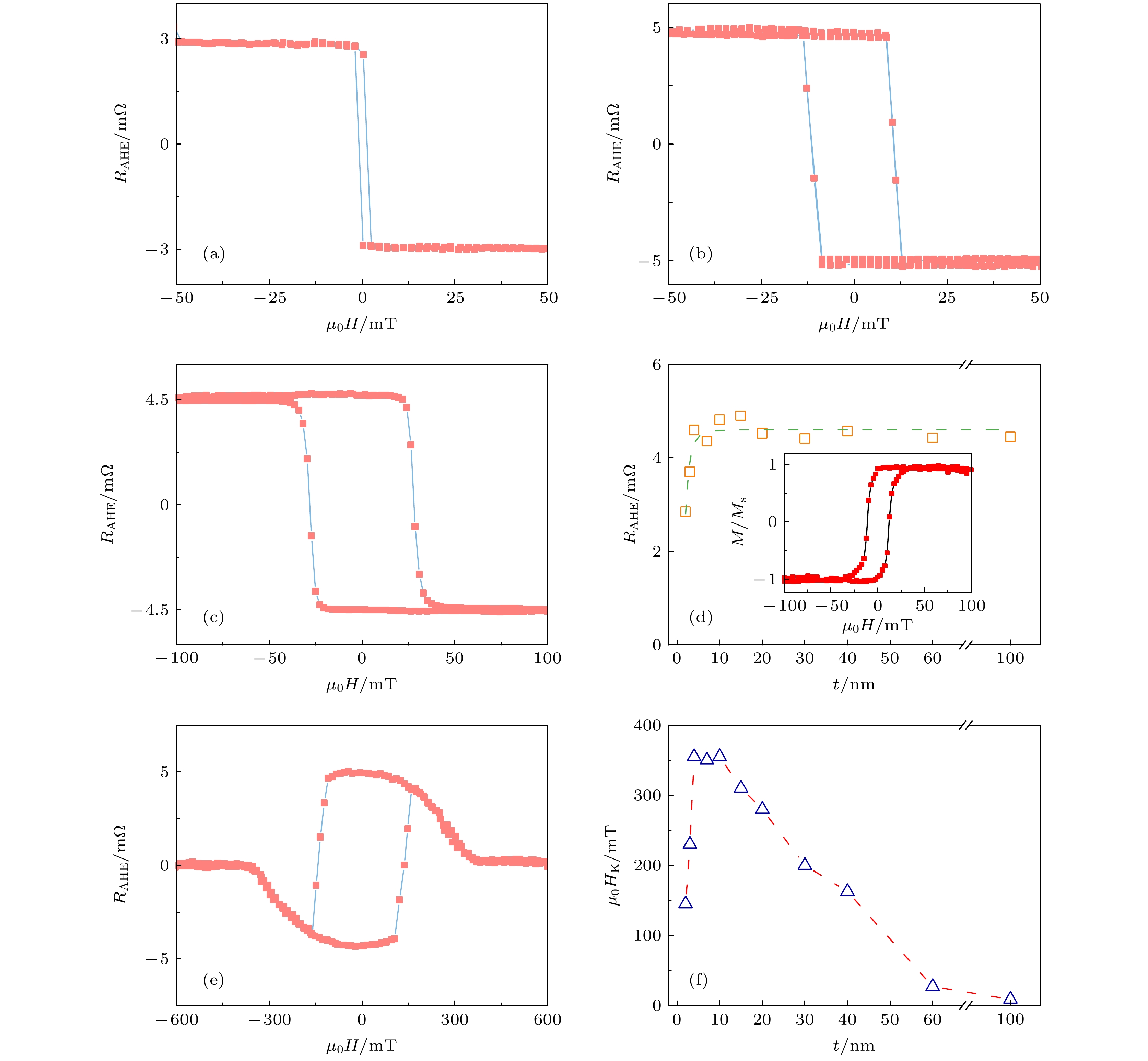
$(486) _{//}$ [$1\overline 1 0$ ].
图 2 (a) 2 nm, (b) 10 nm和(c) 40 nm HoIG/Pt的反常霍尔电阻随垂直外磁场的变化; (d) 饱和反常霍尔电阻随HoIG厚度的变化, 插图是60 nm HoIG的VSM测量磁滞回线; (e) 10 nm HoIG/Pt的平面霍尔电阻随面内磁场的变化; (f)不同厚度HoIG薄膜的有效垂直各向异性场
Figure 2. The anomalous Hall resistance as a function of external field for (a) 2 nm, (b)10 nm and (c) 40 nm HoIG/Pt heterostructure; (d) thickness dependence of the saturated anomalous Hall resistance. The inset is the M-H loop for the 60 nm HoIG film; (e) plane Hall resistance against the in-plane field for 10 nm HoIG/Pt; (f) thickness dependence of the effective perpendicular anisotropy field, the dashed line is the guide to the eye.
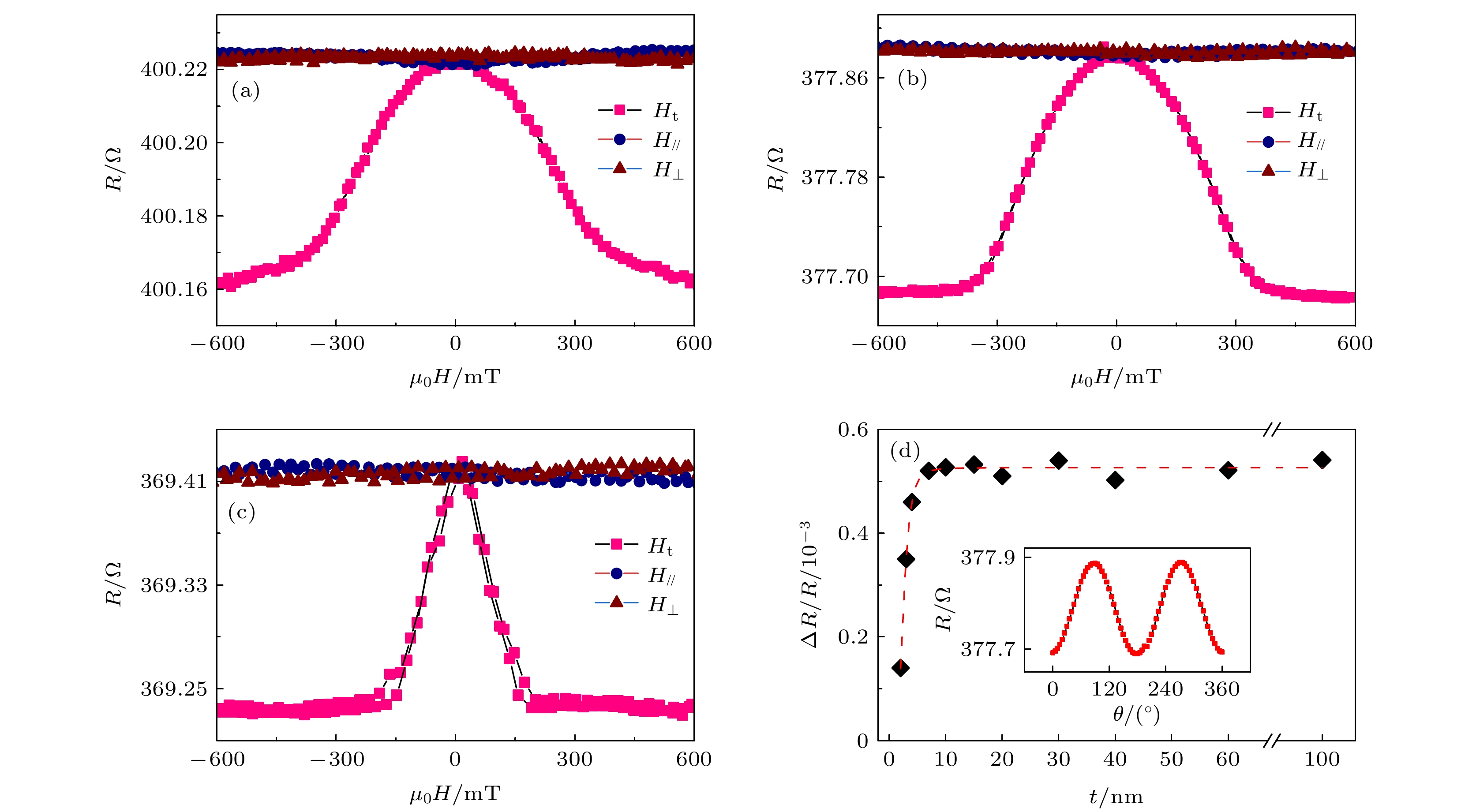
图 3 (a) 2 nm, (b) 10 nm和(c) 40 nm HoIG/Pt的电阻随磁场变化的曲线, 包括平行于膜面且与电流方向垂直的磁场(Ht), 平行于电流方向的磁场(
${{H}} _{//}$ ), 以及垂直于膜面的磁场(H⊥); (d) HoIG/Pt异质结构样品的磁电阻比值随HoIG厚度的变化, 插图为10 nm HoIG/3 nm Pt样品在平行膜面360 mT外磁场下的电阻随电流与磁场方向夹角的变化Figure 3. Field dependence of resistance for the sample (a) 2 nm, (b) 10 nm and (c) 40 nm HoIG/Pt with the field parallel to the film plane and orthogonal to the current (Ht), parallel to the current (
$H _{//}$ ), and perpendicular to the film plane (H⊥); (d) thickness dependence of magnetoresistance ratio ΔR/R for the HoIG/Pt heterostructure. The inset is the angular dependence resistance for the 10 nm HoIG/3 nm Pt stack with the in-plane field magnitude fixed at 360 mT.
图 4 纵向自旋塞贝克几何构型下 (a) 2 nm, (b) 10 nm和(c) 40 nm HoIG/Pt样品的热电压随面内磁场的变化; (d)自旋塞贝克电压随HoIG厚度的变化, 图中虚线为根据(5)式的拟合曲线
Figure 4. In-plane field dependence of thermal voltage for (a) 2 nm, (b) 10 nm and (c) 40 nm HoIG/Pt heterostructure under the longitudinal spin Seebeck geometry; (d) thickness dependence of spin-Seebeck voltage, the dashed line is fitting curve using Eq. (5).
表 1 10和40 nm HoIG薄膜的面内应变
$\varepsilon _{//}$ 、面外应变$\varepsilon _{\bot} $ 、平行膜面内应力$\sigma _{//}$ 和有效垂直各向异性场HKTable 1. The in-plane strain (
$\varepsilon _{//}$ ), perpendicular strain ($\varepsilon _{\bot} $ ), in-plane stress ($\sigma _{//}$ ) and effective perpendicular anisotropy field (Hk) for 10 and 40 nm HoIG films.t /nm $\varepsilon _{//}$/% ε⊥/% $\sigma _{//}$/109 N·m–2 μ0HK/mT 10 1.06 –0.84 3.0 487 40 0.87 –0.43 2.25 348 
DownLoad: CSV
-
[1] 沃尔法斯E P著 (刘增民 等 译) 1993 铁磁材料(卷二)(北京: 电子工业出版社) 第1, 188, 225页
Wohlfarth E P (translated by Liu Z M) 1993 Ferromagnetic Materials (Vol. 2) (Beijing: Electronics Industry Press) pp1, 188, 225 (in Chinese)
[2] Uchida K, Takahashi S, Harii K, Ieda J, Koshibae W, Ando K, Maekawa S and Saitoh E 2008 Nature 455 778
Google Scholar
[3] Jaworski C M, Yang J, Mack S, Awschalom D D, Heremans J P, Myers R C, 2010 Nat. Mater. 9 898
Google Scholar
[4] Lang M, montazeri M, Onbasli M C, Kou X F, Fan Y, Upadhyaya P, Yao K, Liu F, Jiang Y, Jiang W J, Wong K L, Yu G Q, Tang J S, Nie T X, He L, Schwartz B N, Wang Y, Ross C A and Wang K L 2014 Nano Lett. 14 3459
Google Scholar
[5] Jiang Z, Chang C Z, Tang C, Wei P, Moodera J S, Shi J 2015 Nano Lett. 15 5835
Google Scholar
[6] Liu L, Lee O J, Gudmundsen T, Ralph D, Buhrman R 2012 Phys. Rev. Lett. 109 096602
Google Scholar
[7] Li P, Liu T, Chang H, Kalitsov A, Zhang W, Csaba G, Li W, Richardson D, Demann A, Rimal G, Dey H, Jiang J S, porod W, Field S B, Tang J, Marconi M C, Hoffmann A, Mryasov O, Wu M Z 2016 Nat. Commun. 7 12688
Google Scholar
[8] Avci C O, Rosenberg E, Baumgartner, Beran L, Quindeau A, Gambardella P, Ross C A, Beach C S D 2017 Appl. Phys. Lett. 111 072406
Google Scholar
[9] Kamada O, Nakaya T and Higuchi S 2005 Sens. Actuators, A 119 345
Google Scholar
[10] Xiao J and Bauer G E W 2012 Phys. Rev. Lett. 108 217204
Google Scholar
[11] Lee S W and Lee K J 2016 Proc. IEEE 104 1831
Google Scholar
[12] 郭贻诚 著 2014 铁磁学 (北京: 北京大学出版社) 第171页
Guo Y C 2014 Ferromagnetics (Beijing: Peking Univercity Press) p171 (in Chinese)
[13] 郝俊祥, 杨青慧, 张怀武, 等 2018 67 117801
Google Scholar
Hao J X, Yang Q H, Zhang H W, et al. 2018 Acta. Phys. Sin. 67 117801
Google Scholar
[14] Chen J L, Wang C T, Liu C P, 2019 Appl. Phy. Lett. 114 212401
Google Scholar
[15] Li G, Bai H, Su J 2019 APL Mater. 7 041104
Google Scholar
[16] Kubota M, Tsukazaki A, Kagawa F, Shibuya K, Tokunaga Y, Kawasaki M, Tokura Y 2012 Appl. Phys. Express. 5 103002
Google Scholar
[17] Rosenberg E R, Beran L, Avci C O, Zeledon C, Song B, Gonzalez-Fuentes C, Mendil J, Gambardella P, Veis M, Garcia C, Beach G S D, Ross C A 2018 Phys. Rev. M 2 094405
Google Scholar
[18] Yamahara H, Mikami M, Seki M, Tabata H 2011 J.M.M.M. 323 3143
Google Scholar
[19] Bauer J J, Rosenberg E R, Kundu S, Mkhoyan K A, Quarterman P, Grutter A J, Kirby B J, Borchers J A, Ross C A 2020 Adv. Elctron. Mater. 6 1900820
Google Scholar
[20] Zanjani S M, Onbasli M C 2019 AIP Adv. 9 035024
Google Scholar
[21] Nakayama H, Althammer M, Chen Y T, Uchida K, Kajiwara Y, Kikuchi D, Ohtani T, Geprags S, Opel M 2013 Phys. Rev. Lett. 110 206601
Google Scholar
[22] Lu Y M, Choi Y, Ortega C M, Cheng X M, Cai J W, Huang S Y, Sun L, Chien C L 2013 Phys. Rev. Lett. 110 147207
Google Scholar
[23] Tang C, Sellappan P, Liu Y, Xu Y, Garay J E, Shi J 2016 Phys. Rev. B 94 140403(R
Google Scholar
[24] Aldosary M, Li J, Tang C, Xu Y, Zheng J-G, Bozhilov K N, Shi J 2016 Appl. Phys. Lett. 108 242401
Google Scholar
[25] Chen Y-T, Takahashi S, Nakayama H, Althammer M, Goennenwein S T B, Saitoh E, Bauer G E W 2013 Phys. Rev. B 87 144411
Google Scholar
[26] Bai H, Zhan X Z, Li G, Su J, Zhu Z Z, Zhang Y, Zhu T, Cai J W 2019 Appl. Phys. Lett. 115 182401
Google Scholar
[27] Bergholz R, Gradmann U 1984 J.M.M.M. 45 389
Google Scholar
[28] Zhou L K, Zhang Y, Gu L, Cai J W, Sun L 2016 Phys. Rev. B 93 075309
Google Scholar
[29] Philippi-Kobs A, Farhadi A, Matheis L, Chuvilin L A, Oepen H P 2019 Phys. Rev. Lett. 123 137201
Google Scholar
[30] Guo E-J, CraMer J, Kehlberger A, Ferguson C A, MacLaren D A, Jakob G, Klaul M 2016 Phys. Rev. X 6 031012
Google Scholar
[31] Prakash A, Flebus B, Brangham J, Yang F, Tserkovnyak, Heremans J P 2018 Phys. Rev. B 97 020408
Google Scholar
[32] Kikkawa T, Uchida K, Daimon S, Qiu Z, Shiomi Y, Saitoh E 2015 Phys. Rev. B 92 064413
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 6544
- PDF Downloads: 197
- Cited By: 0
