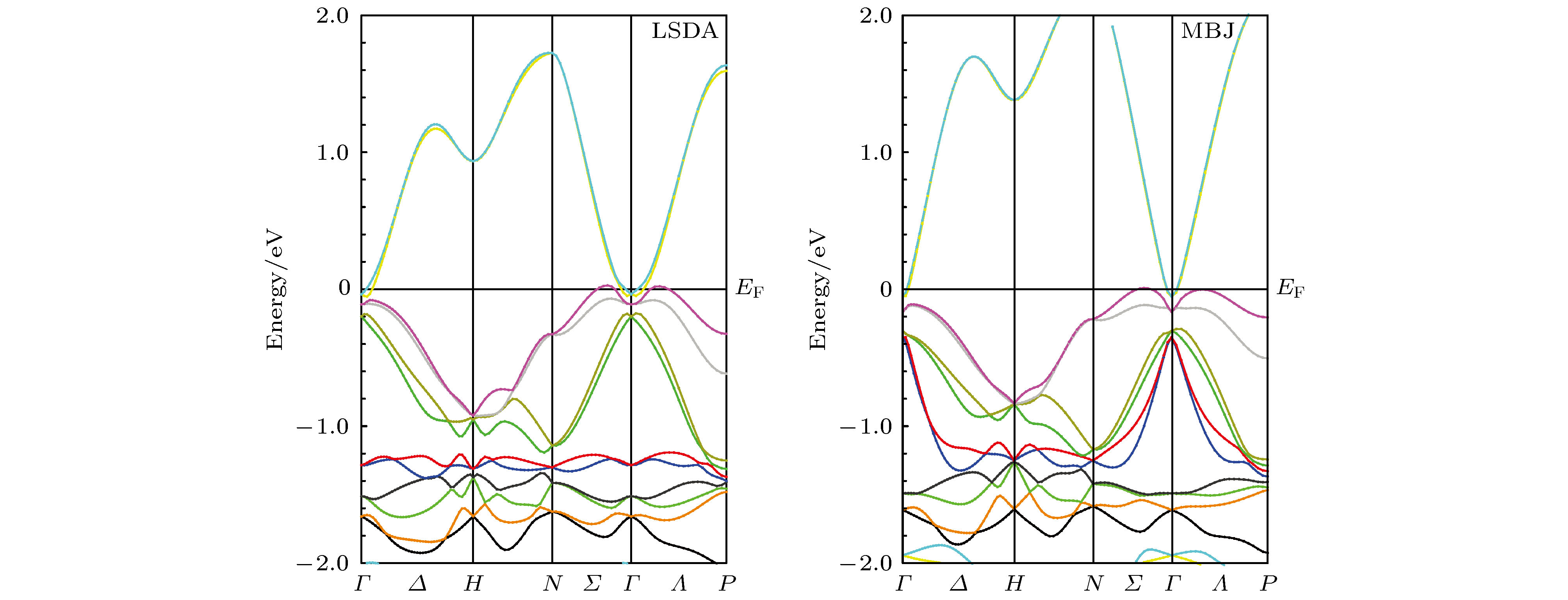
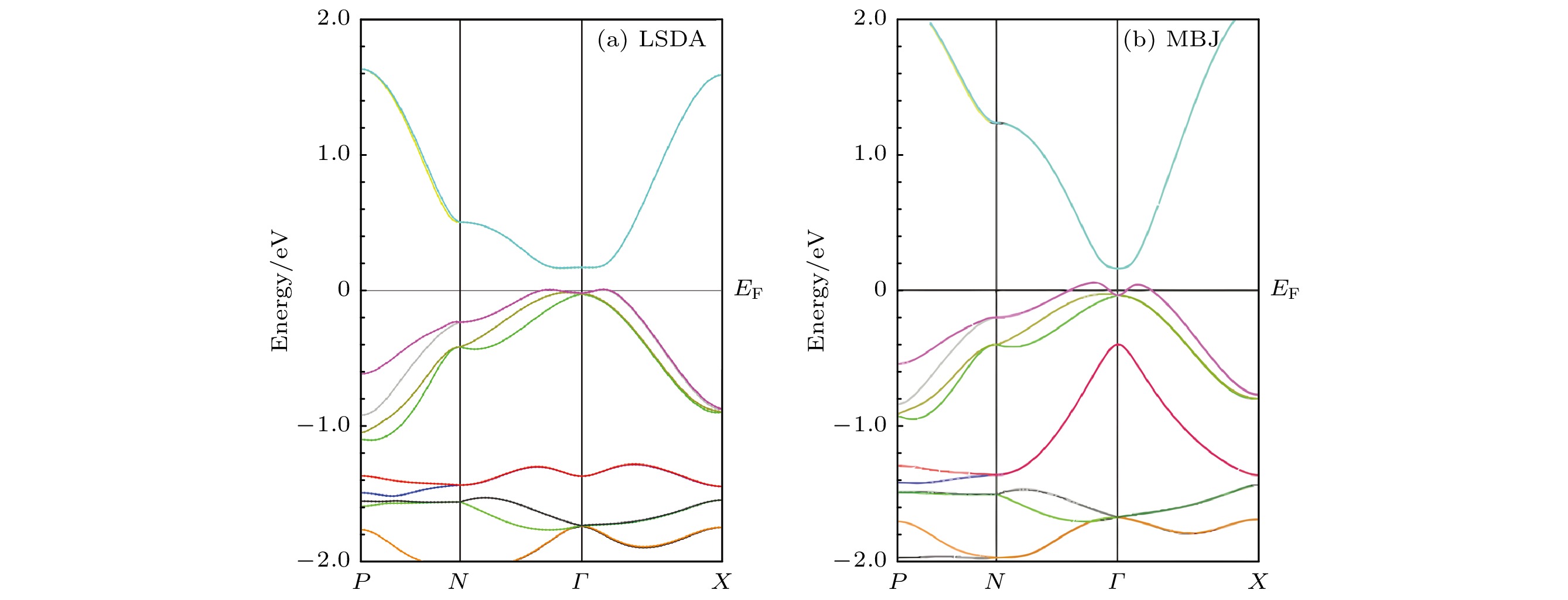
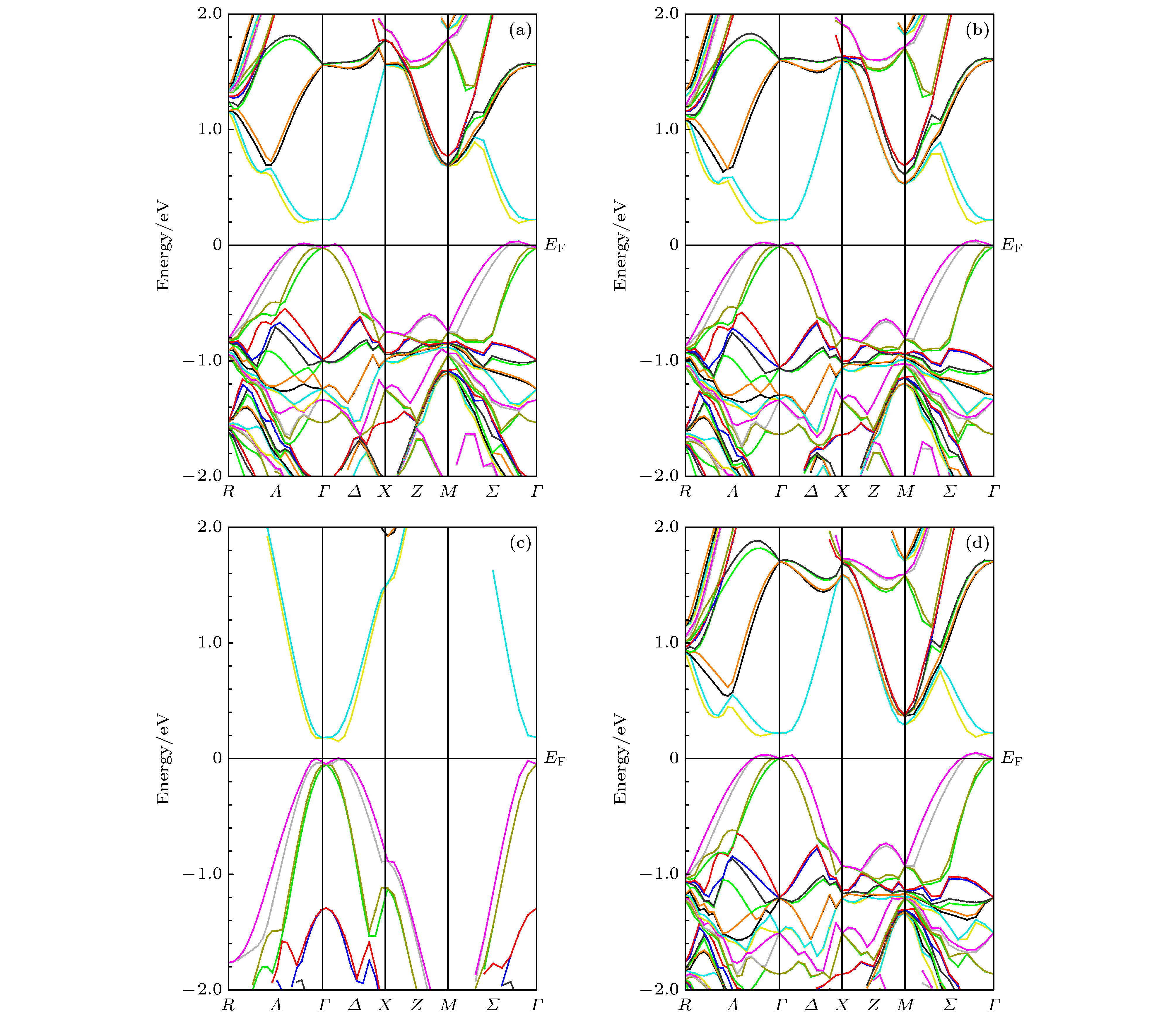
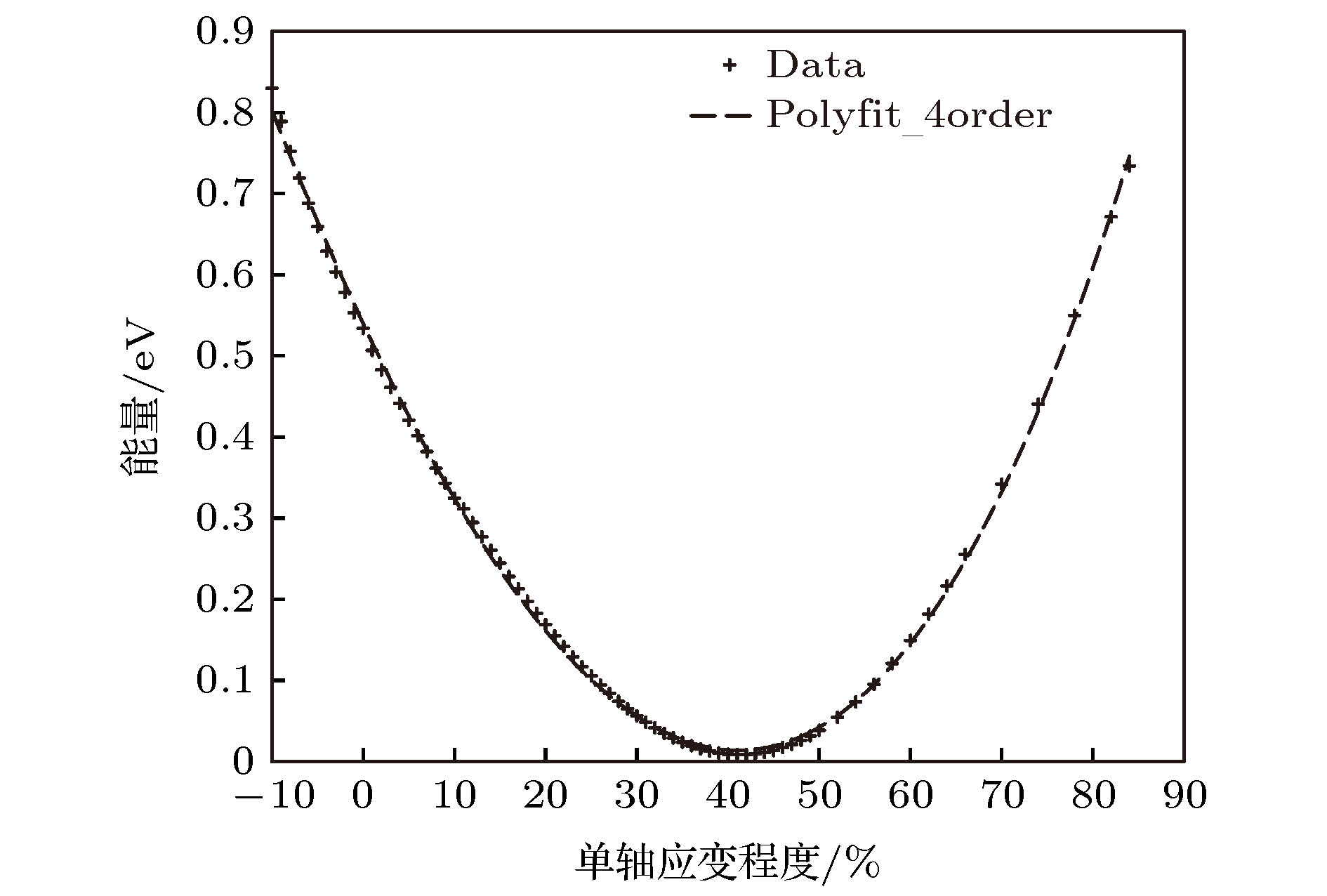
-
Half-Heusler semiconductors exhibit similar properties: the differences among their properties lie only in the fact that in ternary compositions the zinc-blende binary substructure does not provide the required 18 electrons, but this is improved by adding an extra transition metal, which restores the electronic balance. Half-Heusler ternary compound with 18 valence electrons under an appropriate uniaxial strain is a topological insulating phase. Most importantly, it is proposed that in the half-Heusler family, the topological insulator should allow the incorporating of superconductivity and magnetism. Using the first-principle full-potential linearized augmented wave method we study the band structure of a series of Li(Na)AuS topological insulators. The electronic and magnetic properties of Heusler alloys are investigated by the WIEN2k package. The exchange-correlations are treated within the generalized gradient approximation of PerdeweBurke and Ernzerhof (GGA), the local spin density approximation (LSDA), by using the modified Becke-Johnson exchange potential and the correlation potential of the local-density approximation (MBJ). Spin-orbit coupling is treated by means of the second variational procedure with the scalar-relativistic calculation as basis. We first determine the equilibrium lattice constants by calculating the total energy. The theoretical lattice constant of LiAuS full-potential GGA is 6.02 Å, which is somewhat greater than the result of pseudopotential(5.99 Å). The calculated equilibrium lattice parameter is 5.86 Å for LSDA. Most of the half-Heusler compounds have band inversion, and open the nature band gaps, but the gap of MBJ is not very good. Smaller uniaxial stress damages the cubic structure and also such a natural band gap of topological insulators. By applying uniaxial tensile stress until the equilibrium position is reached in all directions of the structure, the system band gap value is about 0.2 eV, which is consistent with the result obtained from the band gap of cubic structure equilibrium position. When uniaxial tensile stress is 41%, the system turns into a tetragonal structure, the equilibrium lattice constant is a = 5.2477 Å and c/a = 1.41. We use the method of substitution of homologous elements to ensure the properties of topological insulator of materials without changing the cubic structure, and open the bandgap of materials under the equilibrium lattice constant of the system, thereby improving the feasibility of experimental synthesis of topological insulator materials. Our results for the doping suggest that epitaxial strain encountered during experiment can result in electronic topological transition. We hope that the results presented here conduce to further experimental investigation of the electronic topological transition in half-Heusler compounds.
-
Keywords:
- topological insulators /
- Heusler alloys /
- first principle /
- band structure
[1] Tang F, Po H C, Vishwanath A, Wan X 2019 Nature 566 486
Google Scholar
[2] Zhang T, Jiang Y, Song Z, Huang H, He Y, Fang Z, Weng H, Fang C 2019 Nature 566 475
Google Scholar
[3] Feng W, Xiao D, Zhang Y, Yao Y 2010 Phys. Rev. B 82 235121
Google Scholar
[4] Zhang X 2018 Chin. Phys. B 27 127101
Google Scholar
[5] Shi F, Si M S, Xie J, Mi K, Xiao C, Luo Q 2017 J. Appl. Phys. 122 215701
Google Scholar
[6] Zhang X M, Xu G Z, Du Y, Liu E K, Liu Z Y, Wang W H, Wu G H 2014 J. Appl. Phys. 115 083704
Google Scholar
[7] Chadov S, Qi X, Kübler J, Fecher G H, Felser C, Zhang S C 2010 Nat. Mater. 9 541
Google Scholar
[8] Lin H, Wray L A, Xia Y, Xu S, Jia S, Cava R J, Bansil A, Hasan M Z 2010 Nat. Mater. 9 546
Google Scholar
[9] Yang H, Yu J, Parkin S S P, Felser C, Liu C X, Yan B 2017 Phys. Rev. Lett. 119 136401
Google Scholar
[10] Xiao D, Yao Y G, Feng W, Wen J, Zhu W, Chen X Q, Stocks G M, Zhang Z 2010 Phys. Rev. Lett. 105 096404
Google Scholar
[11] Xu G, Wang W, Zhang X, Du Y, Liu E, Wang S, Wu G, Liu Z, Zhang X X 2014 Sci. Rep. 4 5709
[12] Wang W, Du Y, Xu G, Zhang X, Liu E, Liu Z, Shi Y, Chen J, Wu G, Zhang X X 2013 Sci. Rep. 3 2181
Google Scholar
[13] Zhang X M, Xu G Z, Liu E K, Liu Z Y, Wang W H, Wu G H 2015 J. Appl. Phys. 117 045706
Google Scholar
[14] Zhang X M, Wang W H, Liu E K, Liu G D, Liu Z Y, Wu G H 2011 Appl. Phys. Lett 99 071901
Google Scholar
[15] Vidal J, Zhang X, Stevanović V, Luo J W, Zunger A 2012 Phys. Rev. B 86 075316
Google Scholar
[16] Lin S Y, Chen M, Yang X B, Zhao Y J, Wu S C, Felser C, Yan B 2015 Phys. Rev. B 91 094107
Google Scholar
[17] Ding G, Gao G Y, Yu L, Ni Y, Yao K 2016 J. Appl. Phys. 119 025105
Google Scholar
[18] Barman C K, Alam A 2018 Phys. Rev. B 97 075302
Google Scholar
[19] Wang G, Wei J 2016 Comput. Mater. Sci. 124 311
Google Scholar
[20] 张小明, 刘国栋, 杜音, 刘恩克, 王文洪, 吴光恒, 柳宗元 2012 61 123101
Google Scholar
Zhang X M, Liu G D, Du Y, Liu E K, Wang W H, Wu G H, Liu Z Y 2012 Acta Phys. Sin. 61 123101
Google Scholar
[21] 王啸天, 代学芳, 贾红英, 王立英, 张小明, 崔玉亭, 王文洪, 吴光恒, 刘国栋 2014 63 053103
Google Scholar
Wang X T, Dai X F, Jia H Y, Wang L Y, Zhang X M, Cui Y T, Wang W H, Wu G H, Liu G D 2014 Acta Phys. Sin. 63 053103
Google Scholar
[22] 王啸天, 代学芳, 贾红英, 王立英, 刘然, 李勇, 刘笑闯, 张小明, 王文洪, 吴光恒, 刘国栋 2014 63 023101
Google Scholar
Wang X T, Dai X F, Jia H Y, W L Y, Liu R, Li Y, Liu X C, Zhang X M, Wang W H, Wu G H, Liu G D 2014 Acta Phys. Sin. 63 023101
Google Scholar
-
图 1 对LiAuS在保证体积不变的基础上对c轴施加单轴应力得到的能量优化曲线, c/a = 1.41时得到平衡晶格常数为a = 0.52477 nm
Figure 1. Calculated total energies as functions of the uniaxial strain along [001] direction with constant volume for LiAuS, the equilibrium lattice constant is a = 0.52477 nm and c/a = 1.41.
图 2 施加1%拉应力后破坏立方结构的能带结构, 左图为LSDA 计算能带图, 右图为MBJ计算得到的能带图
Figure 2. Band structure of the LiAuS compound with 1% uniaxial tensile stress, on the left with LSDA, and on the right with MBJ.
图 3 四方结构平衡晶格常数(a = 0.52477 nm, c/a = 1.41)下的能带结构 (a) LSDA 计算能带图; (b) MBJ计算得到的能带图
Figure 3. Band structure of the tetragonal structure LiAuS compound with the equilibrium lattice constant (a = 0.52477 nm and c/a = 1.41): (a) LSDA; (b) MBJ.
图 4 掺杂结构在各自平衡晶格常数下的能带结构 (a) Li0.125Na0.875AuS; (b) Li0.375Na0.625AuS; (c) Li0.5Na0.5AuS; (d) Li0.875Na0.125AuS
Figure 4. Band structure of the LixNa1–xAuS compound with the equilibrium lattice constant: (a) Li0.125Na0.875AuS; (b) Li0.375Na0.625AuS; (c) Li0.5Na0.5AuS; (d) Li0.875Na0.125AuS.
-
[1] Tang F, Po H C, Vishwanath A, Wan X 2019 Nature 566 486
Google Scholar
[2] Zhang T, Jiang Y, Song Z, Huang H, He Y, Fang Z, Weng H, Fang C 2019 Nature 566 475
Google Scholar
[3] Feng W, Xiao D, Zhang Y, Yao Y 2010 Phys. Rev. B 82 235121
Google Scholar
[4] Zhang X 2018 Chin. Phys. B 27 127101
Google Scholar
[5] Shi F, Si M S, Xie J, Mi K, Xiao C, Luo Q 2017 J. Appl. Phys. 122 215701
Google Scholar
[6] Zhang X M, Xu G Z, Du Y, Liu E K, Liu Z Y, Wang W H, Wu G H 2014 J. Appl. Phys. 115 083704
Google Scholar
[7] Chadov S, Qi X, Kübler J, Fecher G H, Felser C, Zhang S C 2010 Nat. Mater. 9 541
Google Scholar
[8] Lin H, Wray L A, Xia Y, Xu S, Jia S, Cava R J, Bansil A, Hasan M Z 2010 Nat. Mater. 9 546
Google Scholar
[9] Yang H, Yu J, Parkin S S P, Felser C, Liu C X, Yan B 2017 Phys. Rev. Lett. 119 136401
Google Scholar
[10] Xiao D, Yao Y G, Feng W, Wen J, Zhu W, Chen X Q, Stocks G M, Zhang Z 2010 Phys. Rev. Lett. 105 096404
Google Scholar
[11] Xu G, Wang W, Zhang X, Du Y, Liu E, Wang S, Wu G, Liu Z, Zhang X X 2014 Sci. Rep. 4 5709
[12] Wang W, Du Y, Xu G, Zhang X, Liu E, Liu Z, Shi Y, Chen J, Wu G, Zhang X X 2013 Sci. Rep. 3 2181
Google Scholar
[13] Zhang X M, Xu G Z, Liu E K, Liu Z Y, Wang W H, Wu G H 2015 J. Appl. Phys. 117 045706
Google Scholar
[14] Zhang X M, Wang W H, Liu E K, Liu G D, Liu Z Y, Wu G H 2011 Appl. Phys. Lett 99 071901
Google Scholar
[15] Vidal J, Zhang X, Stevanović V, Luo J W, Zunger A 2012 Phys. Rev. B 86 075316
Google Scholar
[16] Lin S Y, Chen M, Yang X B, Zhao Y J, Wu S C, Felser C, Yan B 2015 Phys. Rev. B 91 094107
Google Scholar
[17] Ding G, Gao G Y, Yu L, Ni Y, Yao K 2016 J. Appl. Phys. 119 025105
Google Scholar
[18] Barman C K, Alam A 2018 Phys. Rev. B 97 075302
Google Scholar
[19] Wang G, Wei J 2016 Comput. Mater. Sci. 124 311
Google Scholar
[20] 张小明, 刘国栋, 杜音, 刘恩克, 王文洪, 吴光恒, 柳宗元 2012 61 123101
Google Scholar
Zhang X M, Liu G D, Du Y, Liu E K, Wang W H, Wu G H, Liu Z Y 2012 Acta Phys. Sin. 61 123101
Google Scholar
[21] 王啸天, 代学芳, 贾红英, 王立英, 张小明, 崔玉亭, 王文洪, 吴光恒, 刘国栋 2014 63 053103
Google Scholar
Wang X T, Dai X F, Jia H Y, Wang L Y, Zhang X M, Cui Y T, Wang W H, Wu G H, Liu G D 2014 Acta Phys. Sin. 63 053103
Google Scholar
[22] 王啸天, 代学芳, 贾红英, 王立英, 刘然, 李勇, 刘笑闯, 张小明, 王文洪, 吴光恒, 刘国栋 2014 63 023101
Google Scholar
Wang X T, Dai X F, Jia H Y, W L Y, Liu R, Li Y, Liu X C, Zhang X M, Wang W H, Wu G H, Liu G D 2014 Acta Phys. Sin. 63 023101
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 7239
- PDF Downloads: 160
- Cited By: 0
