First-principle study on effects of Zn-doping on electronic structure, magnetism and martensitic transformation of Heusler type MSMAs Ni2FeGa1–xZnx (x = 0–1)

Sun Kai-Chen; Liu Shuang; Gao Rui-Rui; Shi Xiang-Yu; Liu He-Yan; Luo Hong-Zhi

doi:10.7498/aps.70.20202179

Abstract

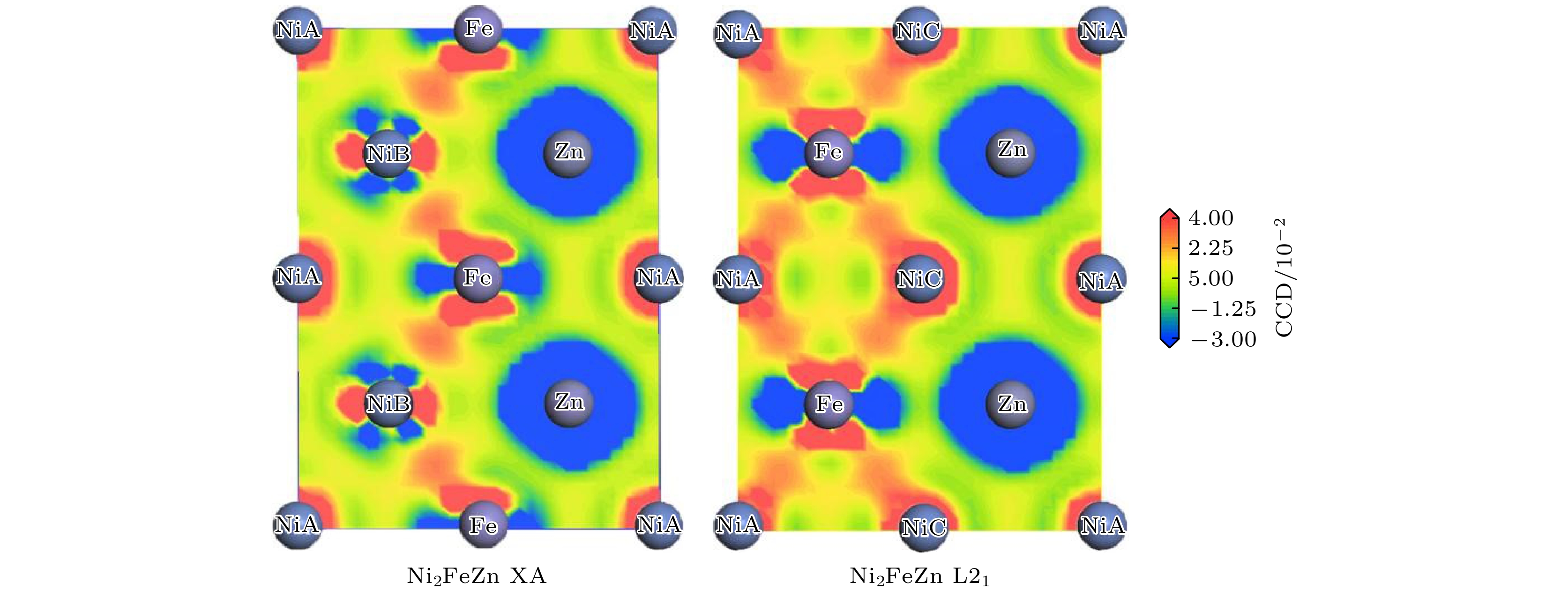
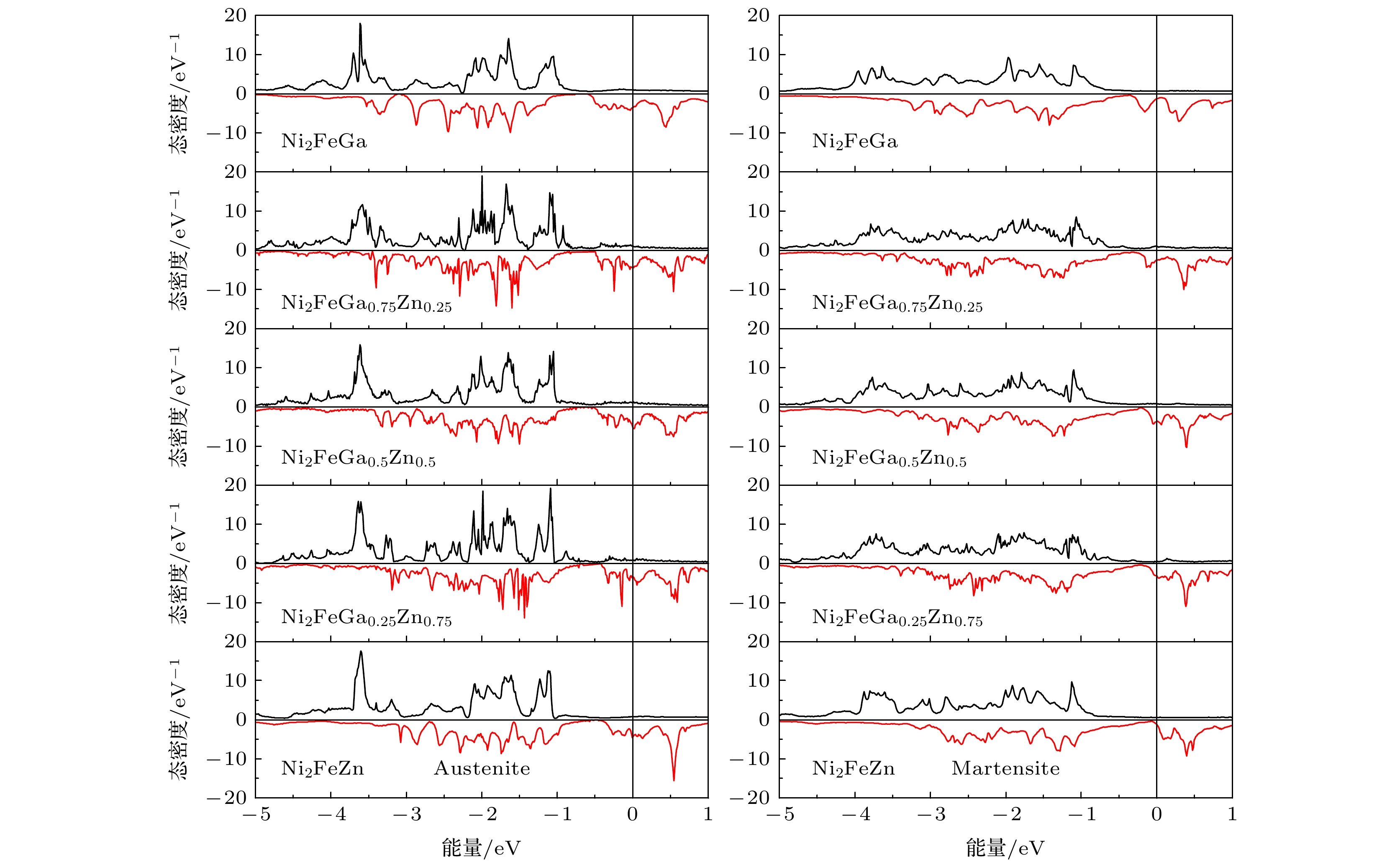
The magnetic shape memory alloys (MSMAs) have both martensitic transformation and ferromagnetism in the same material, thus external magnetic field can be used to induce/control the phase transformation or the reorientation of martensite variant. MSMAs have received considerable attention for their interesting properties and wide applications in different fields. For practical applications, the martensitic transformation temperature T_M is an important factor and a high T_M is preferable. Recently, Zn-doping has been found to be a possible way to elevate the value of T_M of Ni-Mn based MSMA, but this effect on other kinds of MSMAs is not very clear yet. Heusler alloy Ni₂FeGa is a typical MSMA with unique properties, however, its T_M is relatively low. So it can be meaningful to find possible ways to increase its phase transition temperature. In this paper, the influences of Zn-doping on the electronic structure, martensitic transformation and magnetic properties of Heusler-type magnetic shape memory alloy Ni₂FeGa are investigated by first-principle calculations. Total energy calculation and charge density difference indicate that Zn atom prefers to occupy the Ga (D) site when substituting for Ga in Ni₂FeGa_1–xZn_x (x = 0, 0.25, 0.5, 0.75, 1). This main-group-element-like behavior is related to the closed 3d shell of Zn. Due to the similar atomic radii of Ga and Zn, Zn-doping does not lead the lattice constant to change greatly. The variation of the energy difference ΔE_M between the martensite and austenite with Zn content increasing is calculated, and the result shows that ΔE_M increases with Zn-doping increasing, and thus conducing to increasing the stability of the martensite phase and to evaluating the transformation temperature T_M in Ni₂FeGa_1–xZn_x. This trend can be explained by the Jahn-Teller effect observed in the DOS structure. The Zn-doping does not change the magnetic structure of Ni₂FeGa. A ferromagnetic coupling between Fe spin moment and Ni spin moment can be observed within the whole range studied. The calculated total spin moment increases with Zn content increasing. The variation of formation energy E_f with Zn-doping is investigated. In Ni₂FeGa_1–xZn_x a negative E_f is retained within the whole range studied, though it increases slightly with the doping of Zn. It is also found that the Zn-doping can increase the stability of L2₁ Heusler phase in Ni₂FeGa_1–xZn_x and suppress the formation of the FCC L1₂ phase.

Keywords:

Authors and contacts

School of Materials Science and Engineering, Hebei University of Technology, Tianjin 300130, China

Corresponding author: Luo Hong-Zhi, luo_hongzhi@hebut.edu.cn

Funds: Project supported by the Natural Science Foundation of Hebei Province, China (Grant Nos. E2018202097, E2019202143)

FullText HTML

References

[1]	Dunand D C, Müllner P 2011 Adv. Mater. 23 216 Google Scholar
[2]	Gottschall T, Skokov K P, Scheibel F, Acet M, Ghorbani Zavareh M, Skourski Y, Wosnitza J, Farle M, Gutfleisch O 2016 Phys. Rev. Appl. 5 024013 Google Scholar
[3]	Ghosh S, Ghosh A, Mandal K 2018 J. Alloys Compd. 746 200 Google Scholar
[4]	Ullakko K, Huang J K, Kanter C, Kokorin V V, O’Handley R C 1996 Appl. Phys. Lett. 69 1966 Google Scholar
[5]	Liu Z H, Zhang M, Cui Y T, Zhou Y Q, Wang W H, Wu G H, Zhang X X 2003 Appl. Phys. Lett. 82 424 Google Scholar
[6]	Karaca H E, Karaman I, Lagoudas D C, Maier H J, Chumlyakov Y I 2003 Scr. Mater. 49 831 Google Scholar
[7]	Kainuma R, Imano Y, Ito W, Sutou Y, Morito H, Okamoto S, Kitakami O, Oikawa K, Fujita A, Kanomata T, Ishida K 2006 Nature 439 957 Google Scholar
[8]	Omori T, Watanabe K, Umetsu R, Kainuma R, Ishida K 2009 Appl. Phys. Lett. 95 082508 Google Scholar
[9]	Webster P J, Ziebeck K R A, Town S L, Peak M S 1984 Philos. Mag. B 49 295 Google Scholar
[10]	Liu G D, Chen J L, Liu Z H, Dai X F, Wu G H, Zhang B, Zhang X X 2005 Appl. Phys. Lett. 87 262504 Google Scholar
[11]	Han Z D, Wang D H, Zhang C L, Xuan H C, Zhang J R, Gu B X, Du Y W 2008 J. Appl. Phys. 104 053906 Google Scholar
[12]	Algethami Obaidallah A, 李歌天, 柳祝红, 马星桥 2020 69 058102 Google Scholar Algethami O A, Li T G, Liu Z H, Ma X Q 2020 Acta Phys. Sin. 69 058102 Google Scholar
[13]	Zhang H H, Qian M F, Zhang X X, Wei L S, Cao F Y, Xing D W, Cui X P, Sun J F, Geng L 2016 J. Alloys Compd. 689 481 Google Scholar
[14]	Luo H Z, Meng F B, Jiang Q X, Liu H Y, Liu E K, Wu G H, Wang Y X 2010 Scr. Mater. 63 569 Google Scholar
[15]	Barton L S, Lazott R T, Marsten E R 2014 J. Appl. Phys. 115 17A908 Google Scholar
[16]	Ni Z N, Guo X M, Li Q S, Liang Z Y, Luo H Z, Meng F B 2018 J. Magn. Magn. Mater. 464 65 Google Scholar
[17]	Janovec J, Straka L, Sozinov A, Heczko O, Zelený M 2020 Mater. Res. Express 7 026101
[18]	Ghotbi Varzaneh A, Kameli P, Abdolhosseini Sarsari I, Ghorbani Zavareh M, Salazar M C, Amiri T, Skourski Y, Luo J L, Etsell T H, Chernenko V A 2020 Phys. Rev. B 101 134403 Google Scholar
[19]	Payne M C, Teter M P, Allan D C, Arias T A, Joannopoulos J D 1992 Rev. Mod. Phys. 64 1065
[20]	Segall M D, Lindan P L D, Probert M J, Pickard C J, Hasnip P J, Clark S J, Payne M C 2002 J. Phys. Condens. Matter 14 2717 Google Scholar
[21]	Vanderbilt D 1990 Phys. Rev. B 41 7892 Google Scholar
[22]	Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865 Google Scholar
[23]	Kandpal H C, Fecher G H, Felser C 2007 J. Phys. D: Appl. Phys. 40 1507
[24]	Zhang R F, Veprek S, Argon A S 2008 Phys. Rev. B 77 172103 Google Scholar
[25]	Gilleßen M, Dronskowski R 2010 J. Comput. Chem. 31 612
[26]	Zhang Y J, Wang W H, Zhang H G, Liu E K, Ma R S, Wu G H 2013 Physica B 420 86 Google Scholar
[27]	Cordero B, Gomez V, Platero-Prats A E, Reves M, Echeverría J, Cremades E, Barragan F, Alvarez S 2008 Dalton Trans. 21 2832
[28]	赵建涛, 赵昆, 王家佳, 余新泉, 于金, 吴三械 2012 61 213102 Google Scholar Zhao J T, Zhao K, Wang J J, Yu X Q, Yu J, Wu S X 2012 Acta Phys. Sin. 61 213102 Google Scholar
[29]	Wollmann L, Chadov S, Kübler J, Felser C 2011 Phys. Rev. B 92 064417
[30]	Paul S, Ghosh S 2011 J. Appl. Phys. 110 063523 Google Scholar
[31]	Luo H Z, Meng F B, Liu G D, Liu H Y, Jia P Z, Liu E K, Wang W H, Wu G H 2013 Intermetallics 38 139 Google Scholar
[32]	Barman S R, Chakrabarti A, Singh S, Banik S, Bhardwaj S, Paulose P L, Chalke B A, Panda A K, Mitra A, Awasthi A M 2008 Phys. Rev. B 78 134406 Google Scholar
[33]	Entel P, Dannenberg A, Siewert M, Herper H C, Gruner M E, Buchelnikov V D, Chernenko V A 2011 Mater. Sci. Forum 684 1 Google Scholar
[34]	Winterlik J, Chadov S, Gupta A, Alijani V, Gasi T, Filsinger K, Balke B, Fecher G H, Jenkins C A, Casper F, Kubler J, Gao L, Parkin S S P, Felser C 2012 Adv. Mater. 24 6283 Google Scholar
[35]	Faleev S V, Ferrante Y, Jeong J, Samant M G, Jones B, Parkin S S P 2017 Phys. Rev. Appl. 7 034022 Google Scholar
[36]	Brown P J, Bargawi A Y, Crangle J, Neumann K U, Ziebeck K R A 1999 J. Phys. Condens. Matter 11 4715 Google Scholar
[37]	Barman S R, Banik S, Shukla A K, Kamal C, Chakrabart A 2007 EPL 80 57002 Google Scholar
[38]	Soykan C, Özdemir Kart S, Sevik C, Çağın T 2014 J. Alloys Compd. 611 225 Google Scholar

Cited By

图 1 计算得到的Ni₂FeGa_1–_xZn_x(x = 0, 0.25, 0.5, 0.75, 1)合金总能量与晶格常数的关系曲线, 其中Zn(C)和Zn(D)分别表示Zn进入C和D晶位

Figure 1. Calculated total energies of Ni₂FeGa_1–_xZn_x (x = 0, 0.25, 0.5, 0.75, 1) as functions of lattice constant. Here Zn (C) and Zn (D) indicate that Zn enters the C and D sites, respectively.

DownLoad: Full-Size Img PowerPoint

图 2 Ni₂FeZn合金XA(左)和L2₁(右)结构在(110)面上的差分电荷密度

Figure 2. The charge density difference on the (110) plane of Ni₂FeZn alloy with XA (left) and L2₁ (right) structures.

DownLoad: Full-Size Img PowerPoint

图 3 Ni₂FeGa_1–_xZn_x中马氏体和奥氏体相能量差ΔE_M随c/a比值的变化关系. 在图中, 零点对应于每种合金的立方奥氏体能量(c/a = 1)

Figure 3. Variation of the energy difference ΔE_M between the martensitic and austenitic phase with the c/a ratio in Ni₂FeGa_1–_xZn_x. Here the zero point corresponds to the cubic austenite (c/a = 1) of each alloy.

DownLoad: Full-Size Img PowerPoint

图 4 Ni₂FeGa_1–_xZn_x奥氏体和马氏体相总态密度的对比

Figure 4. Comparison between the total DOS of austenitic and martensitic type Ni₂FeGa_1–_xZn_x.

DownLoad: Full-Size Img PowerPoint

图 5 Ni₂FeGa, Ni₂FeGa_0.5Zn_0.5和Ni₂FeZn在L2₁和L1₂结构下的总能量与晶胞体积的函数关系. 图中ΔE表示L1₂和L2₁相之间的能量差

Figure 5. The calculated total energies as functions of cell volume for Ni₂FeGa, Ni₂FeGa_0.5Zn_0.5 and Ni₂FeZn with L2₁ and L1₂ structures. Here ΔE is the energy difference between the L1₂ and L2₁ phases.

DownLoad: Full-Size Img PowerPoint

图 6 L2₁和L1₂型Ni₂FeGa_1–_xZn_x(x = 0, 0.5, 1.0)的总态密度对比

Figure 6. The total DOS of L2₁ and L1₂ type of Ni₂FeGa_1–_xZn_x (x = 0, 0.5, 1.0).

DownLoad: Full-Size Img PowerPoint

表 1 计算得到的Ni₂FeGa_1–_xZn_x(x = 0, 0.25, 0.5, 0.75, 1)合金立方奥氏体相的平衡晶格常数a, 形成能E_f和磁性参数

Table 1. The calculated equilibrium lattice constant a, formation energy E_f and magnetic properties of Ni₂FeGa_1–_xZn_x (x = 0, 0.25, 0.5, 0.75, 1) alloys in cubic austenitic state.

x	0.00	0.25	0.5	0.75	1.00
a/Å	5.76	5.76	5.75	5.74	5.74
E_f/(eV·f.u.^–1)	–0.75	–0.64	–0.54	–0.43	–0.34
M_t (μ_B/f.u.)	3.40	3.50	3.61	3.72	3.82
M_Ni/μ_B	0.16	0.20	0.25	0.30	0.35
M_Fe/μ_B	3.11	3.13	3.15	3.16	3.17
M_Ga/μ_B	–0.03	–0.02	–0.01	0.00	—
M_Zn/μ_B	—	–0.06	–0.06	–0.05	–0.04

DownLoad: CSV

表 2 计算得到的Ni₂FeGa_1–_xZn_x(x = 0, 0.25, 0.5, 0.75, 1)合金在马氏体状态下的马氏体与奥氏体之间能量差ΔE_M, c/a比值和磁性参数

Table 2. The calculated energy difference ΔE_M between the martensite and austenite, c/a ratio and magnetic properties of Ni₂FeGa_1–_xZn_x (x = 0, 0.25, 0.5, 0.75, 1) alloys in tetragonal martensitic state.

x	0.00	0.25	0.5	0.75	1.00
ΔE_M/(eV·f.u.^–1)	–0.110	–0.119	–0.128	–0.144	–0.151
c/a	1.36	1.36	1.34	1.33	1.32
M_t/(μ_B·f.u.^–1)	3.38	3.48	3.63	3.73	3.85
M_Ni/μ_B	0.28	0.32	0.35	0.38	0.41
M_Fe/μ_B	2.92	3.00	3.02	3.06	3.11
M_Ga/μ_B	–0.11	–0.10	–0.08	–0.07	—
M_Zn/μ_B	—	–0.13	–0.11	–0.10	–0.09

DownLoad: CSV

map

[1]	Dunand D C, Müllner P 2011 Adv. Mater. 23 216 Google Scholar
[2]	Gottschall T, Skokov K P, Scheibel F, Acet M, Ghorbani Zavareh M, Skourski Y, Wosnitza J, Farle M, Gutfleisch O 2016 Phys. Rev. Appl. 5 024013 Google Scholar
[3]	Ghosh S, Ghosh A, Mandal K 2018 J. Alloys Compd. 746 200 Google Scholar
[4]	Ullakko K, Huang J K, Kanter C, Kokorin V V, O’Handley R C 1996 Appl. Phys. Lett. 69 1966 Google Scholar
[5]	Liu Z H, Zhang M, Cui Y T, Zhou Y Q, Wang W H, Wu G H, Zhang X X 2003 Appl. Phys. Lett. 82 424 Google Scholar
[6]	Karaca H E, Karaman I, Lagoudas D C, Maier H J, Chumlyakov Y I 2003 Scr. Mater. 49 831 Google Scholar
[7]	Kainuma R, Imano Y, Ito W, Sutou Y, Morito H, Okamoto S, Kitakami O, Oikawa K, Fujita A, Kanomata T, Ishida K 2006 Nature 439 957 Google Scholar
[8]	Omori T, Watanabe K, Umetsu R, Kainuma R, Ishida K 2009 Appl. Phys. Lett. 95 082508 Google Scholar
[9]	Webster P J, Ziebeck K R A, Town S L, Peak M S 1984 Philos. Mag. B 49 295 Google Scholar
[10]	Liu G D, Chen J L, Liu Z H, Dai X F, Wu G H, Zhang B, Zhang X X 2005 Appl. Phys. Lett. 87 262504 Google Scholar
[11]	Han Z D, Wang D H, Zhang C L, Xuan H C, Zhang J R, Gu B X, Du Y W 2008 J. Appl. Phys. 104 053906 Google Scholar
[12]	Algethami Obaidallah A, 李歌天, 柳祝红, 马星桥 2020 69 058102 Google Scholar Algethami O A, Li T G, Liu Z H, Ma X Q 2020 Acta Phys. Sin. 69 058102 Google Scholar
[13]	Zhang H H, Qian M F, Zhang X X, Wei L S, Cao F Y, Xing D W, Cui X P, Sun J F, Geng L 2016 J. Alloys Compd. 689 481 Google Scholar
[14]	Luo H Z, Meng F B, Jiang Q X, Liu H Y, Liu E K, Wu G H, Wang Y X 2010 Scr. Mater. 63 569 Google Scholar
[15]	Barton L S, Lazott R T, Marsten E R 2014 J. Appl. Phys. 115 17A908 Google Scholar
[16]	Ni Z N, Guo X M, Li Q S, Liang Z Y, Luo H Z, Meng F B 2018 J. Magn. Magn. Mater. 464 65 Google Scholar
[17]	Janovec J, Straka L, Sozinov A, Heczko O, Zelený M 2020 Mater. Res. Express 7 026101
[18]	Ghotbi Varzaneh A, Kameli P, Abdolhosseini Sarsari I, Ghorbani Zavareh M, Salazar M C, Amiri T, Skourski Y, Luo J L, Etsell T H, Chernenko V A 2020 Phys. Rev. B 101 134403 Google Scholar
[19]	Payne M C, Teter M P, Allan D C, Arias T A, Joannopoulos J D 1992 Rev. Mod. Phys. 64 1065
[20]	Segall M D, Lindan P L D, Probert M J, Pickard C J, Hasnip P J, Clark S J, Payne M C 2002 J. Phys. Condens. Matter 14 2717 Google Scholar
[21]	Vanderbilt D 1990 Phys. Rev. B 41 7892 Google Scholar
[22]	Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865 Google Scholar
[23]	Kandpal H C, Fecher G H, Felser C 2007 J. Phys. D: Appl. Phys. 40 1507
[24]	Zhang R F, Veprek S, Argon A S 2008 Phys. Rev. B 77 172103 Google Scholar
[25]	Gilleßen M, Dronskowski R 2010 J. Comput. Chem. 31 612
[26]	Zhang Y J, Wang W H, Zhang H G, Liu E K, Ma R S, Wu G H 2013 Physica B 420 86 Google Scholar
[27]	Cordero B, Gomez V, Platero-Prats A E, Reves M, Echeverría J, Cremades E, Barragan F, Alvarez S 2008 Dalton Trans. 21 2832
[28]	赵建涛, 赵昆, 王家佳, 余新泉, 于金, 吴三械 2012 61 213102 Google Scholar Zhao J T, Zhao K, Wang J J, Yu X Q, Yu J, Wu S X 2012 Acta Phys. Sin. 61 213102 Google Scholar
[29]	Wollmann L, Chadov S, Kübler J, Felser C 2011 Phys. Rev. B 92 064417
[30]	Paul S, Ghosh S 2011 J. Appl. Phys. 110 063523 Google Scholar
[31]	Luo H Z, Meng F B, Liu G D, Liu H Y, Jia P Z, Liu E K, Wang W H, Wu G H 2013 Intermetallics 38 139 Google Scholar
[32]	Barman S R, Chakrabarti A, Singh S, Banik S, Bhardwaj S, Paulose P L, Chalke B A, Panda A K, Mitra A, Awasthi A M 2008 Phys. Rev. B 78 134406 Google Scholar
[33]	Entel P, Dannenberg A, Siewert M, Herper H C, Gruner M E, Buchelnikov V D, Chernenko V A 2011 Mater. Sci. Forum 684 1 Google Scholar
[34]	Winterlik J, Chadov S, Gupta A, Alijani V, Gasi T, Filsinger K, Balke B, Fecher G H, Jenkins C A, Casper F, Kubler J, Gao L, Parkin S S P, Felser C 2012 Adv. Mater. 24 6283 Google Scholar
[35]	Faleev S V, Ferrante Y, Jeong J, Samant M G, Jones B, Parkin S S P 2017 Phys. Rev. Appl. 7 034022 Google Scholar
[36]	Brown P J, Bargawi A Y, Crangle J, Neumann K U, Ziebeck K R A 1999 J. Phys. Condens. Matter 11 4715 Google Scholar
[37]	Barman S R, Banik S, Shukla A K, Kamal C, Chakrabart A 2007 EPL 80 57002 Google Scholar
[38]	Soykan C, Özdemir Kart S, Sevik C, Çağın T 2014 J. Alloys Compd. 611 225 Google Scholar

[1]	WANG Jiaxu, ZHANG Yixin, MA Shengran, LI Haoze, LUO Hongzhi. First principles study of electronic structure, mechanical properties and possible martensitic transformation in Ni₂Cu-based Heusler alloys. Acta Physica Sinica, 2025, 74(4): 047101. doi: 10.7498/aps.74.20241485
[2]	Jin Miao, Bai Jing, Xu Jia-Xin, Jiang Xin-Jun, Zhang Yu, Liu Xin, Zhao Xiang, Zuo Liang. Effects of Fe doping on Martensitic Transformation and magnetic properties of Ni-Mn-Ti All-d-metal Heusler Alloy. Acta Physica Sinica, 2023, 72(4): 046301. doi: 10.7498/aps.72.20222037
[3]	Algethami Obaidallah A, Li Ge-Tian, Liu Zhu-Hong, Ma Xing-Qiao. Phase transformation, magnetic properties, and exchange bias of Heusler alloy Mn_50–xCr_xNi₄₂Sn₈. Acta Physica Sinica, 2020, 69(5): 058102. doi: 10.7498/aps.69.20191551
[4]	Xin Yue-Peng, Ma Yue-Xing, Hao Hong-Yue, Meng Fan-Bin, Liu He-Yan, Luo Hong-Zhi. Site preference in isoelectronic Heusler alloy Fe2RuSi. Acta Physica Sinica, 2016, 65(14): 147102. doi: 10.7498/aps.65.147102
[5]	Zhang Yuan-Lei, Li Zhe, Xu Kun, Jing Chao. Martensitic transformation and magnetic features in Ni-Fe-Mn-In Heusler alloy. Acta Physica Sinica, 2015, 64(6): 066402. doi: 10.7498/aps.64.066402
[6]	Jiang En-Hai, Zhu Xing-Feng, Chen Ling-Fu. First-principles study of the electronic structure, magnetism, and spin-polarization in Heusler alloy Co2MnAl(100) surface. Acta Physica Sinica, 2015, 64(14): 147301. doi: 10.7498/aps.64.147301
[7]	Wang Xiao-Tian, Dai Xue-Fang, Jia Hong-Ying, Wang Li-Ying, Liu Ran, Li Yong, Liu Xiao-Chuang, Zhang Xiao-Ming, Wang Wen-Hong, Wu Guang-Heng, Liu Guo-Dong. The band inversion and topological insulating state of Heusler alloys：X2RuPb (X=Lu, Y). Acta Physica Sinica, 2014, 63(2): 023101. doi: 10.7498/aps.63.023101
[8]	Zhang Hong-Wu, Zhou Wen-Ping, Liu En-Ke, Wang Wen-Hong, Wu Guang-Heng. Magnetic field-induced martensitic transformation, superspin glass and exchange bias in Heusler alloys NiCoMnSn. Acta Physica Sinica, 2013, 62(14): 147501. doi: 10.7498/aps.62.147501
[9]	Zhang Yu-Jie, Liu En-Ke, Zhang Hong-Guo, Li Gui-Jiang, Chen Jing-Lan, Wang Wen-Hong, Wu Guang-Heng. Martensitic transformation and magnetic properties in Ga-doped MMX alloy MnNiGe1-x Gax (x=00.30). Acta Physica Sinica, 2013, 62(19): 197501. doi: 10.7498/aps.62.197501
[10]	Luo Li-Jin, Zhong Chong-Gui, Dong Zheng-Chao, Fang Jing-Huai, Zhou Peng-Xia, Jiang Xue-Fan. A band Jahn-Teller effect in the martensitic phase transition of the Heusler alloy Mn2NiGe. Acta Physica Sinica, 2012, 61(20): 207503. doi: 10.7498/aps.61.207503
[11]	Zhu Wei, Liu En-Ke, Zhang Chang-Zai, Qin Yuan-Bin, Luo Hong-Zhi, Wang Wen-Hong, Du Zhi-Wei, Li Jian-Qi, Wu Guang-Heng. Magnetic property and structure of Heusler alloy: Fe2CrGa. Acta Physica Sinica, 2012, 61(2): 027502. doi: 10.7498/aps.61.027502
[12]	Song Rui-Ning, Zhu Wei, Liu En-Ke, Li Gui-Jiang, Chen Jing-Lan, Wang Wen-Hong, Li Xiang, Wu Guang-Heng. Effect of internal stress on sructure, martensitic transformation and magnetic properties of ferromagnetic shape memory alloy Mn2NiGa. Acta Physica Sinica, 2012, 61(2): 027501. doi: 10.7498/aps.61.027501
[13]	Zhao Jing-Jing, Shu Di, Qi Xin, Liu En-Ke, Zhu Wei, Feng Lin, Wang Wen-Hong, Wu Guang-Heng. Structural phase transition and magnetic properties of Co50Fe50-xSix alloys. Acta Physica Sinica, 2011, 60(10): 107203. doi: 10.7498/aps.60.107203.1
[14]	Zhang Hao-Lei, Li Zhe, Qiao Yan-Fei, Cao Shi-Xun, Zhang Jin-Cang, Jing Chao. Martensitic transformation and magnetocaloric effect in Ni-Co-Mn-Sn Heusler alloy. Acta Physica Sinica, 2009, 58(11): 7857-7863. doi: 10.7498/aps.58.7857
[15]	Wang Qing-Zhou, Lu Dong-Mei, Cui Chun-Xiang, Han Fu-Sheng. Effects of quenched-in vacancies on the reverse martensitic phase transformation temperature of the Cu-11.9Al-2.5Mn(wt%) shape memory alloy studied by internal friction. Acta Physica Sinica, 2008, 57(11): 7083-7087. doi: 10.7498/aps.57.7083
[16]	Jing Chao, Chen Ji-Ping, Li Zhe, Cao Shi-Xun, Zhang Jin-Cang. Martensitic transformation and magnetocaloric effect in Ni50Mn35In15 Heusler alloy. Acta Physica Sinica, 2008, 57(7): 4450-4455. doi: 10.7498/aps.57.4450
[17]	Jing Chao, Li Zhe, Chen Ji-Ping, Lu Yu-Ming, Cao Shi-Xun, Zhang Jin-Cang. Investigation of martensitic transition and inverse magnetocaloric property in Ni-Mn-Sn Heusler alloys. Acta Physica Sinica, 2008, 57(6): 3780-3785. doi: 10.7498/aps.57.3780
[18]	Dai Xue-Fang, Liu He-Yan, Yan Li-Qin, Qu Jing-Ping, Li Yang-Xian, Chen Jing-Lan, Wu Guang-Heng. Structure and martensitic transformation of the CoNiZ alloys. Acta Physica Sinica, 2006, 55(5): 2534-2538. doi: 10.7498/aps.55.2534
[19]	Gao Shu-Xia, Wang Wen-Hong, Liu Zhu-Hong, Chen Jing-Lan, Wu Guang-Heng, Liang Ting, Xu Hui-Bin, ＣａｉＷｅｉ, ＺｈｅｎｇＹｕＦｅｎｇ, Zhao Lian-Cheng. . Acta Physica Sinica, 2002, 51(2): 332-336. doi: 10.7498/aps.51.332
[20]	Wang Wen-Hong, Liu Zhu-Hong, Chen Jing-Lan, Wu Guang-Heng, Liang Ting, Xu Hui-Bin, Chai Wei, Zheng Yu-Feng, Zhao Lian-Cheng. . Acta Physica Sinica, 2002, 51(3): 635-639. doi: 10.7498/aps.51.635

Catalog

Vol. 70, Issue 13 - 2021-07-05

Metrics

Abstract views: 4970
PDF Downloads: 78
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板

First-principle study on effects of Zn-doping on electronic structure, magnetism and martensitic transformation of Heusler type MSMAs Ni₂FeGa_1–_xZn_x(x = 0–1)

Abstract

Authors and contacts

Corresponding author: Luo Hong-Zhi, luo_hongzhi@hebut.edu.cn

FullText HTML

References

Cited By

Catalog

Metrics

Authors

Referees

About Journal

About

Search

Article

留言板