-
According to the first-principles calculation within PBE+U method and tight-binding model, the magnetic properties and electronic structures of two-dimensional (2D) CrPSe3 monolayer were investigated. Constructed by a Cr-honeycomb hexagonal lattice, 2D CrPSe3 was predicted to be in a half-metallic ferromagnetic state with dynamic stability, confirmed by the phonon spectrum with no imaginary dispersion. The Curie temperature was estimated as 224 K by Monte Carlo simulation within the Metropolis algorithm under the periodic boundary condition. The thermal stability of CrPSe3 monolayer was estimated at 300 K by a first-principles molecular dynamics simulation. It is found that the magnetic ground state of CrPSe3 monolayer is determined by a competition between the antiferomagnetic d-d direct exchange interactions and the Se-p orbitals mediated ferromagnetic p-d superexchange interactions. Most interestingly, in the half-metallic state the band structure exhibits multiple Dirac cones in the first Brillouin zone: two cones at K point showing a very high Fermi velocity
${v_{\rm F}{(K)}} = 15.8 \times 10^5 \;{\rm m \!\cdot\! s^{-1}}$ about twice larger than the$ v_{\rm F} $ of graphene in the vicinity of Fermi level, and six cones at$ K'/2 $ points with${ v_{\rm F} {(K'/2)}} = 10.1 \times 10^5\;{\rm m \!\cdot\! s^{-1}}$ close to the graphene's value. These spin-polarized Dirac cones are mostly composed of Cr${\rm d}_{xz}$ and${\rm d}_{yz}$ orbitals. The novel electronic structure of CrPSe3 monolayer is also confirmed by the HSE06 functional. A tight-binding model was built based on the Cr-honeycomb structure with two Cr-d orbitals as the basic with the first, second and third nearest-neighboring interactions, further demonstrating that the multiple Dirac cones are protected by the Cr-honeycomb lattice symmetry. Our findings indicate that 2D CrPSe3 monolayer is a candidate with potential applications in the low-dimensional, high speed and temperature spintronics.-
Keywords:
- two-dimensional ferromagnetism /
- Dirac cone /
- first-principles calculation /
- tight-binding method
[1] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[2] 王兴悦, 张辉, 阮子林, 郝振亮, 杨孝天, 蔡金明, 卢建臣 2020 69 118101
Wang X Y, Zhang H, Ruan Z L, Hao Z L, Yang X T, Cai J M, Lu J C 2020 Acta Phys. Sin. 69 118101
[3] Li L, Yu Y, Ye G J, Ge Q, Ou X, Wu H, Feng D, Chen X H, Zhang Y 2014 Nat. Nanotechnol. 9 372
Google Scholar
[4] Watanabe K, Taniguchi T, Kanda H 2004 Nat. Mater. 3 404
Google Scholar
[5] Han G H, Duong D L, Keum D H, Yun Se J, Lee Y H 2018 Chem. Rev. 118 6297
Google Scholar
[6] Burch K S, Mandrus D, Park J 2018 Nature 563 47
Google Scholar
[7] S Babar, Nadeem M, Dai Z, Fuhrer M S, Xue Q, Wang X, Bao Q 2018 Appl. Phys. Rev. 5 041105
Google Scholar
[8] Huang B, Clark G, Navarro-Moratalla E, Klein D R, Cheng R, Seyler K L, Zhong D, Schmidgall E, McGuire M A, Cobden D H, Yao W, Xiao D, Jarillo-Herrero P, Xu X 2017 Nature 546 270
Google Scholar
[9] 邓雨君, 於逸骏, 张远波 2019 物理 2 88
Google Scholar
Deng Y J, Yu Y J, Zhang Y B 2019 Physics 2 88
Google Scholar
[10] Gong C, Li L, Li Z, Ji H, Stern A, Xia Y, et al. 2017 Nature 546 265
Google Scholar
[11] Wang F, Shifa T A, Yu P, He P, Liu Y, et al. 2018 Adv. Funct. Mater. 28 1802151
Google Scholar
[12] 俞强, 郭琨, 张颖聪, 陈捷, 王涛, 汪进, 史鑫尧, 吴坚, 张凯, 周朴 2020 69 184208
Google Scholar
Yu Q, Guo K, Zhang Y C, Chen J, Wang T, Wang J, Shi X Y, Wu J, Zhang K, Zhou P 2020 Acta Phys. Sin. 69 184208
Google Scholar
[13] 龚吉祥, 严秀, 杨军, 葛敏, 皮雳, 朱文卡, 张昌锦 2018 低温 40 22
Google Scholar
Gong J X, Yan X, Yang J, Ge M, Pi L, Zhu W K, Zhang C J 2018 Chin. J. Low Temp. Phys. 40 22
Google Scholar
[14] Sivadas N, Daniels M W, Swendsen R H, Okamoto S, Xiao D 2015 Phys. Rev. B 91 235425
Google Scholar
[15] Chittari B L, Park Y, Lee, Han D M, MacDonald A H, Hwang E, Jung J 2016 Phys. Rev. B 94 184428
Google Scholar
[16] Kim S Y, Kim T Y, Sandilands L J, Sinn S, Lee M C, et al. 2018 Phys. Rev. Lett. 120 136402
Google Scholar
[17] Li X, Cao T, Niu Q, Shi J, Feng J 2013 Proc. Natl. Acad. Sci. U.S.A. 110 3738
Google Scholar
[18] Pei Q, Wang X, Zou J, Mi W 2018 J. Mater. Chem. C 6 8092
Google Scholar
[19] Gu Y, Zhang Q, Le C, Li Y, Xiang T, Hu J 2019 Phys. Rev. B 100 165405
Google Scholar
[20] Sugita Y, Miyake T, Motome Y 2018 Phys. Rev. B 197 035125
[21] Gusmão R, Sofer Z, Sedmidubský D, Huber Š, Martin P 2017 ACS Catal. 7 8159
Google Scholar
[22] Kresse G, Hafner J 1994 J. Phys. Condens. Matter 6 8245
Google Scholar
[23] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
[24] Perdew J, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[25] Dudarev S L, Botton G A, Savrasov S Y, Humphreys C J, Sutton A P 1998 Phys. Rev. B 57 1505
Google Scholar
[26] Grimme S 2006 J. Comput. Chem. 27 1787
Google Scholar
[27] Blöchl P E, Jepsen O, Andersen O 1994 Phys. Rev. B 49 16223
Google Scholar
[28] Chaput L, Togo A, Tanaka I, Hug G 2011 Phys. Rev. B 84 094302
Google Scholar
[29] Togo A, Tanaka I 2015 Scr. Mater. 108 1
Google Scholar
[30] Metropolis N, Rosenbluth A W, Rosenbluth M N, Teller A H, Teller E 1953 J. Chem. Phys. 21 1087
Google Scholar
[31] Pizzi G, Vitale V, Arita R, et al. 2019 J. Phys. Condens. Matter 32 165902
Google Scholar
[32] Wang V, Xu N, Liu J C, Tang G, Geng W T 2019 arXiv: 1908.08269
[33] Goodenough J B 1955 Phys. Rev. B 100 564
Google Scholar
[34] Kanamori J 1960 J. Appl. Phys. 31 S14
Google Scholar
[35] Anderson P W 1959 Phys. Rev. B 115 2
Google Scholar
[36] Heyd J, Scuseria G E, Ernzerhof M 2003 J. Chem. Phys. 118 8207
Google Scholar
-
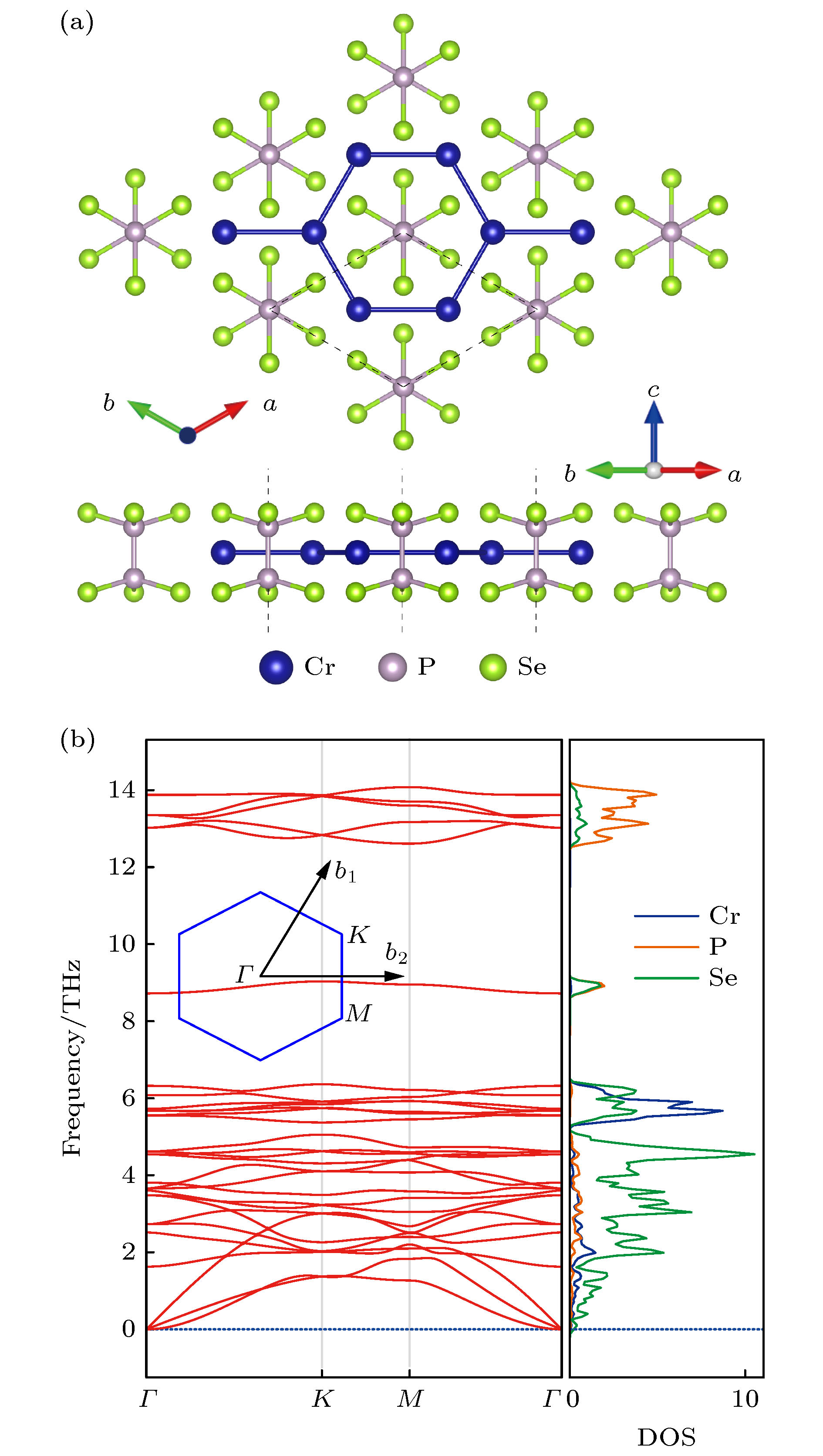
图 1 二维CrPSe3的(a) 晶体结构俯视图和侧视图(灰色虚线表示晶体单胞), (b) 声子谱(插图为布里渊区及高对称点)和声子态密度
Figure 1. (a) Top and side views of two-dimensional (2D) CrPSe3 monolayer with the hexagonal unit cell denoted by grey broken lines; (b) the phonon spectrum and the corresponding density of states (DOS) for Cr, P and Se atoms, respectively, the inset shows the Brillouin zone of 2D CrPSe3 monolayer
图 2 二维CrPSe3的交换作用能
$\Delta E$ (meV) 随(a)面内双轴压力(负值表示压缩, 正值表示膨胀)和(b)$U$ 值的变化曲线, 图(a)内插图是铁磁态和反铁磁态的自旋电荷密度图, 等值面密度为 3 × 10–3${\rm{e}}$ ·Å–3, 淡黄和浅蓝分别代表自旋向上和自旋向下的电荷密度分布Figure 2. Exchange parameters
$\Delta E=E({\rm{AFM}})-E({\rm{FM}})$ (meV) with respect to (a) in-plane biaxial strain (negative value denotes compressive and postive means tensile) and (b) U value of 2D CrPSe3. The insects of panel (a) is the spin electron density of 2D CrPSe3 in FM and AFM states with isovalue of 3 × 10–3${\rm{e }}$ ·Å–3. The yellow and cyan colors represent spin-up and down electrons, respectively
图 3 二维CrPSe3 (a)相对磁矩和比热容相对于温度的蒙特卡罗模拟变化曲线, 以及(b) 300 K温度下
$4\times4\times1$ 超胞总能随时间的变化, 插图是弛豫6 ps 后的晶体结构图Figure 3. (a) The Monte Carlo simulated magnetic moment and specific heat capacity as a function of temperature and (b) total energy fluctuations with respect to the simulation time at 300 K of CrPSe3 monolayer. The inset shows the corresponding structure at 300 K after the simulation for 6 ps
图 4 二维CrPSe3的(a) 自旋向上和自旋向下的能带图, 以及(b) 费米面附近的三维能带图(费米能级设置为0 eV)
Figure 4. (a) Spin up and spin down band structures along high symmetry
$k$ -points and (b) three-dimensional band structures near Fermi level of 2D CrPSe3. Fermi level is set to 0 eV
图 5 二维CrPSe3的总态密度和各元素的分波态密度图(费米能级设置为0 eV)
Figure 5. Total and partial density of states of 2D CrPSe3. Fermi level is set to 0 eV
图 A1 (a) HSE06杂化泛函与PBE + U计算结果比较; (b)几个典型的U值的计算结果; (c) 几个典型的压力值下二维CrPSe3自旋向上和自旋向下的能带图; 费米能级设置为0 eV
Figure A1. Spin up and spin down band structures along high symmetry
$k$ -points: (a) Calculated by HSE06 functional results compared with that of PBE + U method; (b) calculated by some typical U value; (c) under typical strain effeccts of 2D CrPSe3. Fermi level is set to 0 eV
图 6 紧束缚近似模型 (a) 二维CrPSe3轨道跃迁示意图; (b)考虑
$t_1$ ,$t_2$ 和$t_3$ 跃迁系数时二维CrPSe3的紧束缚近似能带图; (c)仅考虑$t_3$ 跃迁系数时二维CrPSe3的紧束缚近似能带图Figure 6. Tight binding model: (a) Hoping parameters illustration and band structures considered (b) all
$t_1$ ,$t_2$ and$t_3$ hopping parameters and (c) only$t_3$ hopping parameters of 2D CrPSe3 monolayer表 1 二维CrPSe3的优化结果(晶格常数
$a$ (Å)、晶体厚度$h$ (Å)、部分键长$d$ (Å) 和原子间的夹角$\theta\;(^\circ)$ )Table 1. Optimized lattice constants
$a$ (Å), monolayer thickness$h$ (Å), some bond legths$d$ (Å) and angle$\theta\;(^\circ)$ between some atoms of 2D CrPSe3 monolayer晶格常数/Å 键长 d/Å 原子夹角$\theta /(^\circ)$ a 6.364 Cr—Cr 3.674 Cr—Se—Cr 85.3 b 6.364 Cr—Se 2.711 Se—P—Se 113.5 h 3.394 P—Se 2.211 
DownLoad: CSV
-
[1] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[2] 王兴悦, 张辉, 阮子林, 郝振亮, 杨孝天, 蔡金明, 卢建臣 2020 69 118101
Wang X Y, Zhang H, Ruan Z L, Hao Z L, Yang X T, Cai J M, Lu J C 2020 Acta Phys. Sin. 69 118101
[3] Li L, Yu Y, Ye G J, Ge Q, Ou X, Wu H, Feng D, Chen X H, Zhang Y 2014 Nat. Nanotechnol. 9 372
Google Scholar
[4] Watanabe K, Taniguchi T, Kanda H 2004 Nat. Mater. 3 404
Google Scholar
[5] Han G H, Duong D L, Keum D H, Yun Se J, Lee Y H 2018 Chem. Rev. 118 6297
Google Scholar
[6] Burch K S, Mandrus D, Park J 2018 Nature 563 47
Google Scholar
[7] S Babar, Nadeem M, Dai Z, Fuhrer M S, Xue Q, Wang X, Bao Q 2018 Appl. Phys. Rev. 5 041105
Google Scholar
[8] Huang B, Clark G, Navarro-Moratalla E, Klein D R, Cheng R, Seyler K L, Zhong D, Schmidgall E, McGuire M A, Cobden D H, Yao W, Xiao D, Jarillo-Herrero P, Xu X 2017 Nature 546 270
Google Scholar
[9] 邓雨君, 於逸骏, 张远波 2019 物理 2 88
Google Scholar
Deng Y J, Yu Y J, Zhang Y B 2019 Physics 2 88
Google Scholar
[10] Gong C, Li L, Li Z, Ji H, Stern A, Xia Y, et al. 2017 Nature 546 265
Google Scholar
[11] Wang F, Shifa T A, Yu P, He P, Liu Y, et al. 2018 Adv. Funct. Mater. 28 1802151
Google Scholar
[12] 俞强, 郭琨, 张颖聪, 陈捷, 王涛, 汪进, 史鑫尧, 吴坚, 张凯, 周朴 2020 69 184208
Google Scholar
Yu Q, Guo K, Zhang Y C, Chen J, Wang T, Wang J, Shi X Y, Wu J, Zhang K, Zhou P 2020 Acta Phys. Sin. 69 184208
Google Scholar
[13] 龚吉祥, 严秀, 杨军, 葛敏, 皮雳, 朱文卡, 张昌锦 2018 低温 40 22
Google Scholar
Gong J X, Yan X, Yang J, Ge M, Pi L, Zhu W K, Zhang C J 2018 Chin. J. Low Temp. Phys. 40 22
Google Scholar
[14] Sivadas N, Daniels M W, Swendsen R H, Okamoto S, Xiao D 2015 Phys. Rev. B 91 235425
Google Scholar
[15] Chittari B L, Park Y, Lee, Han D M, MacDonald A H, Hwang E, Jung J 2016 Phys. Rev. B 94 184428
Google Scholar
[16] Kim S Y, Kim T Y, Sandilands L J, Sinn S, Lee M C, et al. 2018 Phys. Rev. Lett. 120 136402
Google Scholar
[17] Li X, Cao T, Niu Q, Shi J, Feng J 2013 Proc. Natl. Acad. Sci. U.S.A. 110 3738
Google Scholar
[18] Pei Q, Wang X, Zou J, Mi W 2018 J. Mater. Chem. C 6 8092
Google Scholar
[19] Gu Y, Zhang Q, Le C, Li Y, Xiang T, Hu J 2019 Phys. Rev. B 100 165405
Google Scholar
[20] Sugita Y, Miyake T, Motome Y 2018 Phys. Rev. B 197 035125
[21] Gusmão R, Sofer Z, Sedmidubský D, Huber Š, Martin P 2017 ACS Catal. 7 8159
Google Scholar
[22] Kresse G, Hafner J 1994 J. Phys. Condens. Matter 6 8245
Google Scholar
[23] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
[24] Perdew J, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[25] Dudarev S L, Botton G A, Savrasov S Y, Humphreys C J, Sutton A P 1998 Phys. Rev. B 57 1505
Google Scholar
[26] Grimme S 2006 J. Comput. Chem. 27 1787
Google Scholar
[27] Blöchl P E, Jepsen O, Andersen O 1994 Phys. Rev. B 49 16223
Google Scholar
[28] Chaput L, Togo A, Tanaka I, Hug G 2011 Phys. Rev. B 84 094302
Google Scholar
[29] Togo A, Tanaka I 2015 Scr. Mater. 108 1
Google Scholar
[30] Metropolis N, Rosenbluth A W, Rosenbluth M N, Teller A H, Teller E 1953 J. Chem. Phys. 21 1087
Google Scholar
[31] Pizzi G, Vitale V, Arita R, et al. 2019 J. Phys. Condens. Matter 32 165902
Google Scholar
[32] Wang V, Xu N, Liu J C, Tang G, Geng W T 2019 arXiv: 1908.08269
[33] Goodenough J B 1955 Phys. Rev. B 100 564
Google Scholar
[34] Kanamori J 1960 J. Appl. Phys. 31 S14
Google Scholar
[35] Anderson P W 1959 Phys. Rev. B 115 2
Google Scholar
[36] Heyd J, Scuseria G E, Ernzerhof M 2003 J. Chem. Phys. 118 8207
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 8872
- PDF Downloads: 289
- Cited By: 0
