-
In the last two decades, a wide variety of plasmoids events have been observed, ranging from space and astrophysical phenomenon to magnetically confined laboratory plasmas, in which there are a lot of evidence of observational plasmoid-like features supported by direct large-scaled computer simulations. A super-Alfvénic instability, named plasmoid instability, occurs in an extended current sheet, when the Lundquist number exceeds a critical value. The large-aspect-ratio current sheet is fragmented by generating, growing, coalescing and ejecting of plasmoids so that this phenomenon has been proposed as a possible mechanism for fast reconnection scenario. This super-Alfvénic plasmoid instability has been usedin the significant new development of reconnection theory, and thus can provide alternative and more convincing mechanism for fast reconnection. In this work, a “driving” kind of shear flow in the out-of-plane direction is imposed on a two-dimensional, three-component magnetohydrodynamic model with a current sheet system to study the dynamic process of the plasmoids in a current sheet system. The effect of the width and strength of the driving flow on the reconnection rate of plasmoids are numerically analyzed in detail. It is found that the plasmoids are easily formed in the case of strong and wide out-of-plane driving flow. The reconnection rate and the number of the plasmoids increase with the driving flow width and/or driving flow strength increasing. In the presence of guiding field, it is found that the symmetry of the plasmoids is broken in the reconnection plane. In addition, for the fixed guiding field, the growth rate of plasmoids increases much faster when the strength of driving flow increases.
-
Keywords:
- magnetic reconnection /
- plasma driving flow /
- plasmiods /
- guiding field
[1] Parker E N 1963 Astrophys. J. Suppl. Ser. 8 177
Google Scholar
[2] Sweet P A 1969 Annu. Rev. Astron. Astr. 7 149
Google Scholar
[3] Yokoyama T, Akita K, Morimoto T, Inoue K, Newmark J 2001 Astrophys. J. Lett. 546 L69
Google Scholar
[4] Dungey J W 1961 Phys. Rev. Lett. 6 47
Google Scholar
[5] Bhattacharjee A 2004 Annu. Rev. Astron. Astr. 42 365
Google Scholar
[6] Hastie R J 1997 Astrophys. Space Sci. 256 177
Google Scholar
[7] Chapman I T, Scannell R, Cooper W A, Graves J P, Hastie R J, Naylor G, Zocco A 2010 Phys. Rev. Lett. 105 255002
Google Scholar
[8] Wei L, Wang Z X, Fan D M, Wang F, Liu Y 2011 Phys. Plasmas 18 042503
Google Scholar
[9] Zhang C L, Ma Z W 2009 Phys. Plasmas 16 122113
Google Scholar
[10] Jin S P, Yang H A, Wang X G 2005 Phys. Plasmas 12 042902
Google Scholar
[11] Ishizawa A, Waelbroeck F L, Fitzpatrick R, Horton W, Nakajima N 2012 Phys. Plasmas 19 072312
Google Scholar
[12] Biskamp D 1986 Phys. Fluids. 29 1520
Google Scholar
[13] Daughton W, Scudder J, Karimabadi H 2006 Phys. Plasmas 13 072101
Google Scholar
[14] Drake J F, Swisdak M, Che H, Shay M A 2006 Nature 443 553
Google Scholar
[15] Loureiro N F, Schekochihin A A, Cowley S C 2007 Phys. Plasmas 14 100703
[16] Lapenta G 2008 Phys. Rev. Lett. 100 235001
Google Scholar
[17] Lin J, Cranmer S R, Farrugia C J 2008 J. Geophys. Res. 113 D11107
Google Scholar
[18] Bhattacharjee A, Huang Y M, Yang H, Rogers B 2009 Phys. Plasmas 16 112102
Google Scholar
[19] Daughton W, Roytershteyn V, Albright B J, Karimabadi H, Yin L, Bowers K J 2009 Phys. Rev. Lett. 103 065004
Google Scholar
[20] Huang Y M, Bhattacharjee A, Sullivan B P 2011 Phys. Plasmas 18 072109
Google Scholar
[21] Nemati M J, Wang Z X, Wei L, Selim B I 2015 Phys. Plasmas 22 012106
Google Scholar
[22] Samtaney R, Loureiro N F, Uzdensky D A, Schekochihin A A, Cowley S C 2009 Phys. Rev. Lett. 103 105004
Google Scholar
[23] Pritchett P L, Lee Y C, Drake J F 1980 Phys. Fluids 23 1368
Google Scholar
[24] Ishii Y, Azumi M, Kishimoto Y 2002 Phys. Rev. Lett. 89 205002
Google Scholar
[25] Wang Z X, Wang X, Dong J Q, Kishimoto Y, Li J Q 2008 Phys. Plasmas 15 082109
Google Scholar
[26] Wang Z X, Wang X G, Dong J Q, Lei Y A, Long Y X, Mou Z Z, Qu W X 2007 Phys. Rev. Lett. 99 185004
Google Scholar
[27] Bierwage A, Hamaguchi S, Wakatani M, Benkadda S, Leoncini X 2005 Phys. Rev. Lett. 94 065001
Google Scholar
[28] Bowers K, Li H 2007 Phys. Rev. Lett. 98 035002
Google Scholar
[29] Nemati M J, Wang Z X, Wei L 2016 Astrophys. J. 821 128
Google Scholar
[30] Nemati M J, Wang Z X, Wei L 2017 Astrophys. J. 835 191
Google Scholar
[31] La BelleHamer A L, Otto A, Lee L C 1994 Phys. Plasmas 1 706
Google Scholar
[32] Wang J, Xiao C, Wang X 2012 Phys. Plasmas 19 032905
Google Scholar
[33] Wang L, Wang X Q, Wang X G, Liu Y 2014 Chin. Phys. B 23 025203
Google Scholar
-
图 1 垂直磁重联平面的驱动流剖面
Figure 1. Profiles of the out-of-plane driving flows.
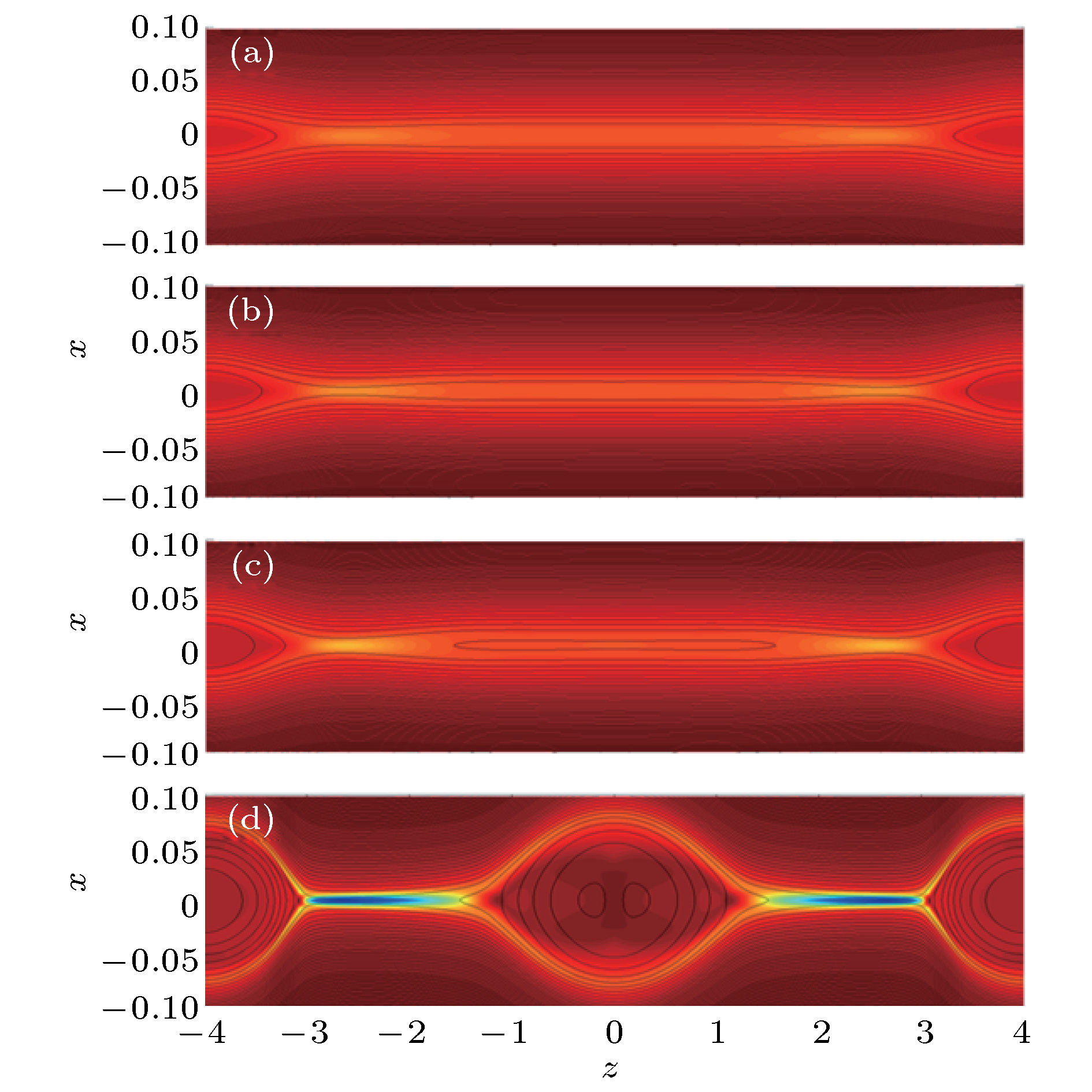
图 2 无驱动流情况下的磁岛位型 (a), (b), (c), (d)分别为时间t = 70, t = 74, t = 80和t = 120的结构, 黑线和背景颜色分别为重联平面的磁力线分布和垂直平面的电流密度
Figure 2. The magnetic configuration without driving flow at (a) t = 70, (b) t = 74, (c) t = 80, (d) t = 120. The black lines and background colors indicate the magnetic field line in the reconnection plane and the current density in out-of-plane direction, respectively.
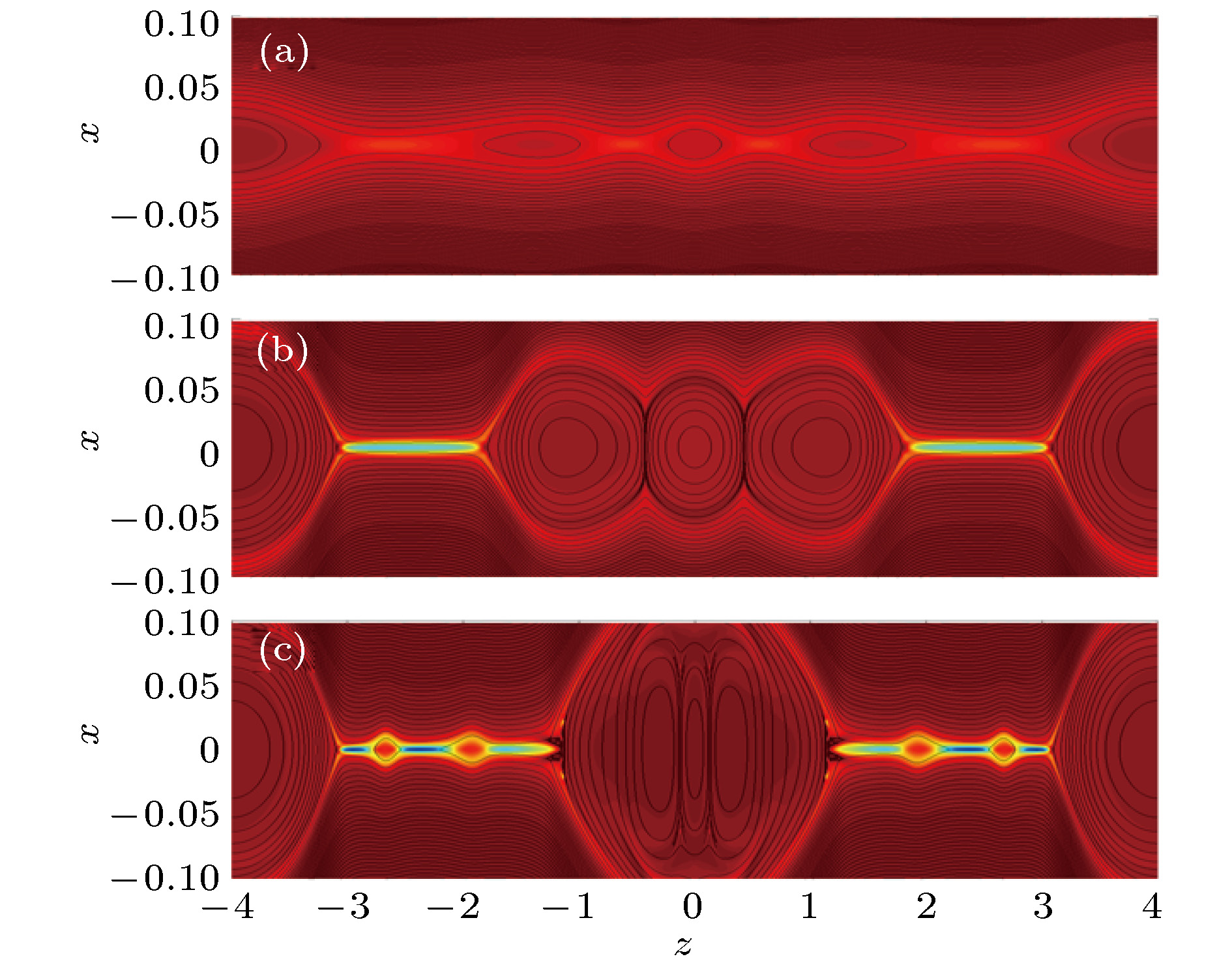
图 3 加入宽度LS = 0.05、强度U0 = 0.1的驱动流时, 磁岛链的演化过程 (a), (b), (c)分别为时间t = 70, t = 80和t = 110的结构, 黑线和背景颜色分别为重联平面的磁力线分布和垂直平面的电流密度
Figure 3. Evolution of magnetic configuration with out-of-plane driving flow for LS = 0.05, U0 = 0.1 at (a) t = 70, (b) t = 80, (c) t = 110. The black lines and background colors indicate the magnetic field line in the reconnection plane and the current density in out-of-plane direction, respec-tively.
图 4 加入宽度LS = 0.3, 强度U0 = 0.1 驱动流情况下的磁岛位型 (a), (b), (c)为时间t = 80, t = 110和t = 118的结构, 黑线和背景颜色分别为重联平面的磁力线分布和垂直平面的电流密度
Figure 4. The magnetic configuration with out-of-plane dri-ving flow for LS = 0.3, U0 = 0.1 at (a) t = 80, (b) t = 110, (c) t = 118. The black lines and background colors indicate the magnetic field line in the reconnection plane and the current density in out-of-plane direction, respectively.
图 5 相同宽度LS = 0.3, 不同强度的驱动流, 重联通量随时间的演化
Figure 5. The evolution of reconnected flux with different driving flow strength.
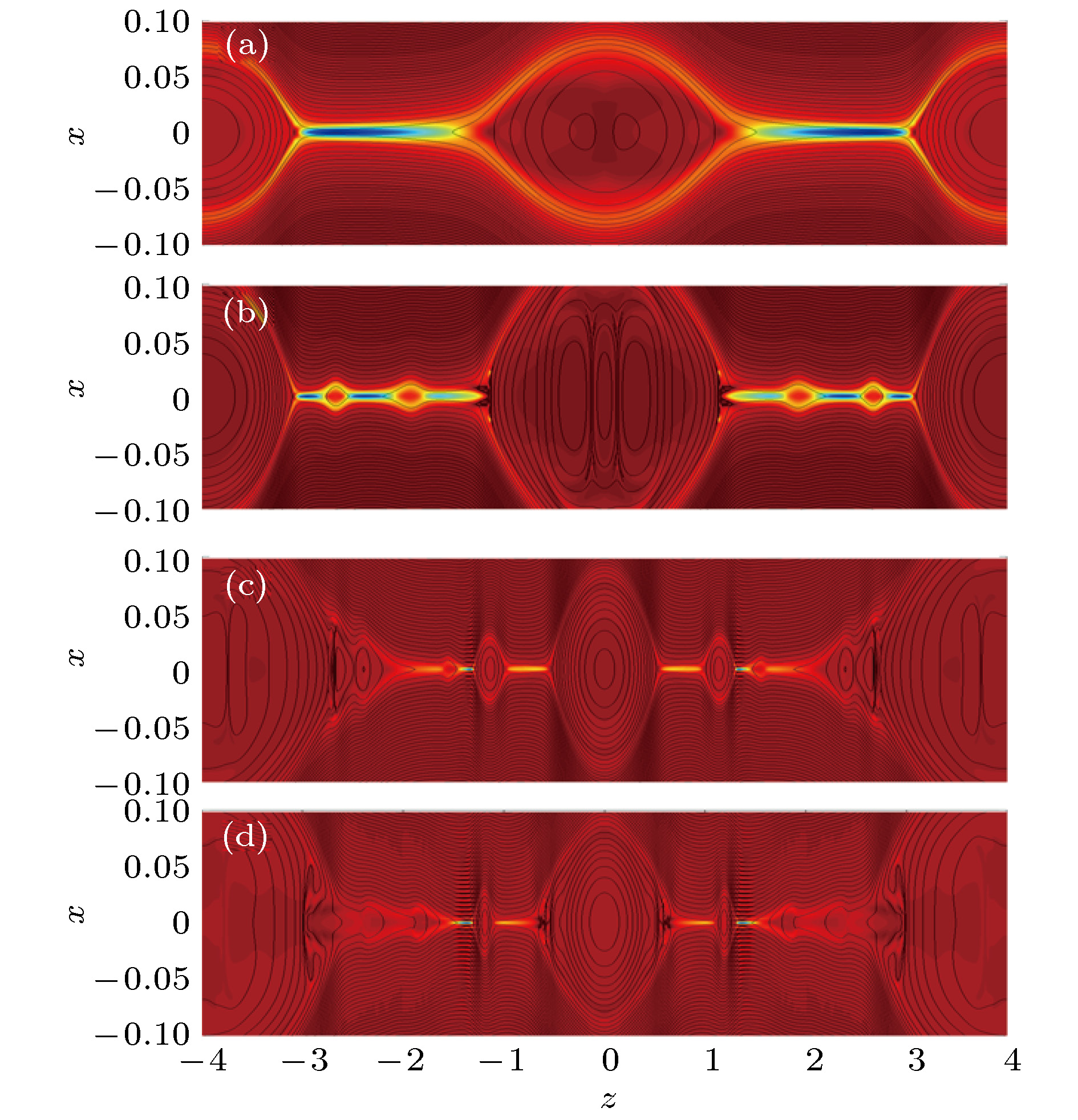
图 6 加入相同宽度LS = 0.3, 不同强度的驱动流时的典型磁岛位型, 黑线和背景颜色分别为重联平面的磁力线分布和垂直平面的电流密度 (a)无驱动流的情况t = 120的结构; (b)加入强度U0 = 0.1的情况在t = 118的结构; (c)加入强度U0 = 0.2的情况在t = 97的结构; (d)加入强度U0 = 0.3的情况在t = 88的结构
Figure 6. The magnetic configuration with out-of-plane dri-ving flow with different strength for LS = 0.3 in the same phase. The black lines and background colors indicate the magnetic field line in the reconnection plane and the current density in out-of-plane direction, respectively: (a) Without driving flow at t = 120; (b) with U0 = 0.1 at t = 118; (c) with U0 = 0.2 at t = 97; (d) with U0 = 0.3 at t = 88.
图 7 导向场By = 0.1, 驱动流宽度LS = 0.05、强度U0 = 0.1情况下的磁岛位型 (a), (b), (c)分别为时间t = 100, t = 110和t = 119的结构, 黑线和背景颜色分别为重联平面的磁力线分布和垂直平面的电流密度
Figure 7. The magnetic configuration is effected by out-of-plane driving flow with LS = 0.05, U0 = 0.1 and guilding field By = 0.1 at (a) t = 100, (b) t = 110, (c) t = 119. The black lines and background colors indicate the magnetic field line in the reconnection plane and the current density in out-of-plane direction, respectively.
图 8 驱动流宽度LS = 0.3, 强度U0 = 0.1, 导向场By = 0.1的情况下, 磁岛位形的演化 (a), (b), (c)分别为时间t = 70, t = 80和t = 88的结构, 黑线和背景颜色分别为重联平面的磁力线分布和垂直平面的电流密度
Figure 8. Evolution of the magnetic configuration with out-of-plane driving flow for LS = 0.3, U0 = 0.1 and guilding field By = 0.1 at (a) t = 70; (b) t = 80; (c) t = 88. The black lines and background colors indicate the magnetic field line in the reconnection plane and the current density in out-of-plane direction, respectively.
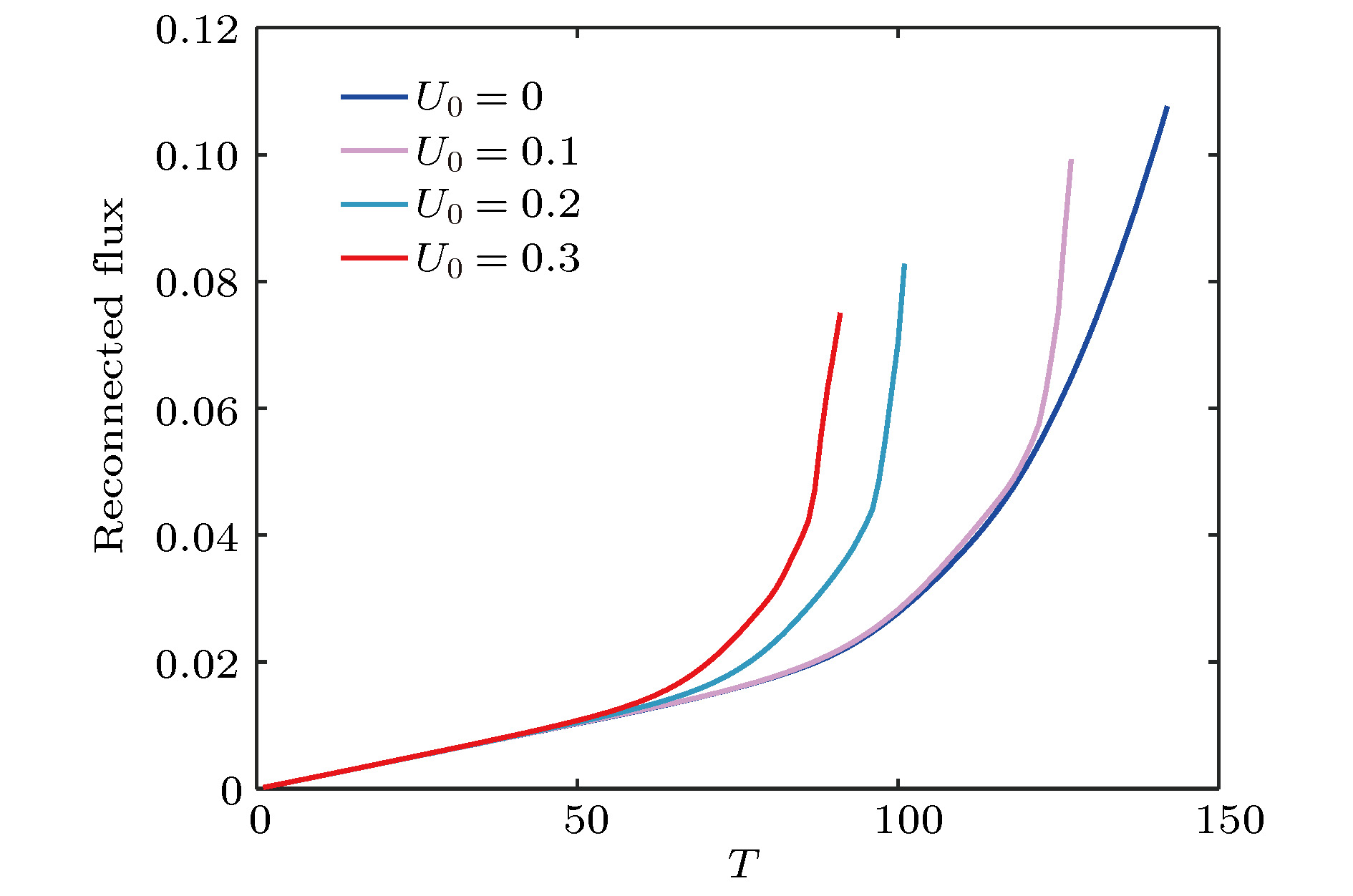
图 9 (a)驱动流宽度LS = 0.05, 强度U0 = 0.1时, 不同导向场By重联通量随时间的演化; (b)导向场By = 0.1, 驱动流宽度LS = 0.05时, 不同强度U0下重联通量随时间的演化; (c)导向场By = 0.1, 驱动流宽度LS = 0.05时, 小磁岛宽度的增长速度对强度U0的依赖关系
Figure 9. (a) Evolution of the reconnection flux with out-of-plane driving flow for LS = 0.05, U0 = 0.1 and different guilding field; (b) evolution of the reconnection flux with different driving flow strength and guilding field By = 0.1; (c) dependence of the growth rate of plasmoid on different driving flow strength with guilding field By = 0.1.
-
[1] Parker E N 1963 Astrophys. J. Suppl. Ser. 8 177
Google Scholar
[2] Sweet P A 1969 Annu. Rev. Astron. Astr. 7 149
Google Scholar
[3] Yokoyama T, Akita K, Morimoto T, Inoue K, Newmark J 2001 Astrophys. J. Lett. 546 L69
Google Scholar
[4] Dungey J W 1961 Phys. Rev. Lett. 6 47
Google Scholar
[5] Bhattacharjee A 2004 Annu. Rev. Astron. Astr. 42 365
Google Scholar
[6] Hastie R J 1997 Astrophys. Space Sci. 256 177
Google Scholar
[7] Chapman I T, Scannell R, Cooper W A, Graves J P, Hastie R J, Naylor G, Zocco A 2010 Phys. Rev. Lett. 105 255002
Google Scholar
[8] Wei L, Wang Z X, Fan D M, Wang F, Liu Y 2011 Phys. Plasmas 18 042503
Google Scholar
[9] Zhang C L, Ma Z W 2009 Phys. Plasmas 16 122113
Google Scholar
[10] Jin S P, Yang H A, Wang X G 2005 Phys. Plasmas 12 042902
Google Scholar
[11] Ishizawa A, Waelbroeck F L, Fitzpatrick R, Horton W, Nakajima N 2012 Phys. Plasmas 19 072312
Google Scholar
[12] Biskamp D 1986 Phys. Fluids. 29 1520
Google Scholar
[13] Daughton W, Scudder J, Karimabadi H 2006 Phys. Plasmas 13 072101
Google Scholar
[14] Drake J F, Swisdak M, Che H, Shay M A 2006 Nature 443 553
Google Scholar
[15] Loureiro N F, Schekochihin A A, Cowley S C 2007 Phys. Plasmas 14 100703
[16] Lapenta G 2008 Phys. Rev. Lett. 100 235001
Google Scholar
[17] Lin J, Cranmer S R, Farrugia C J 2008 J. Geophys. Res. 113 D11107
Google Scholar
[18] Bhattacharjee A, Huang Y M, Yang H, Rogers B 2009 Phys. Plasmas 16 112102
Google Scholar
[19] Daughton W, Roytershteyn V, Albright B J, Karimabadi H, Yin L, Bowers K J 2009 Phys. Rev. Lett. 103 065004
Google Scholar
[20] Huang Y M, Bhattacharjee A, Sullivan B P 2011 Phys. Plasmas 18 072109
Google Scholar
[21] Nemati M J, Wang Z X, Wei L, Selim B I 2015 Phys. Plasmas 22 012106
Google Scholar
[22] Samtaney R, Loureiro N F, Uzdensky D A, Schekochihin A A, Cowley S C 2009 Phys. Rev. Lett. 103 105004
Google Scholar
[23] Pritchett P L, Lee Y C, Drake J F 1980 Phys. Fluids 23 1368
Google Scholar
[24] Ishii Y, Azumi M, Kishimoto Y 2002 Phys. Rev. Lett. 89 205002
Google Scholar
[25] Wang Z X, Wang X, Dong J Q, Kishimoto Y, Li J Q 2008 Phys. Plasmas 15 082109
Google Scholar
[26] Wang Z X, Wang X G, Dong J Q, Lei Y A, Long Y X, Mou Z Z, Qu W X 2007 Phys. Rev. Lett. 99 185004
Google Scholar
[27] Bierwage A, Hamaguchi S, Wakatani M, Benkadda S, Leoncini X 2005 Phys. Rev. Lett. 94 065001
Google Scholar
[28] Bowers K, Li H 2007 Phys. Rev. Lett. 98 035002
Google Scholar
[29] Nemati M J, Wang Z X, Wei L 2016 Astrophys. J. 821 128
Google Scholar
[30] Nemati M J, Wang Z X, Wei L 2017 Astrophys. J. 835 191
Google Scholar
[31] La BelleHamer A L, Otto A, Lee L C 1994 Phys. Plasmas 1 706
Google Scholar
[32] Wang J, Xiao C, Wang X 2012 Phys. Plasmas 19 032905
Google Scholar
[33] Wang L, Wang X Q, Wang X G, Liu Y 2014 Chin. Phys. B 23 025203
Google Scholar
-
[1] Yang Zhen-Yu, Zhang Yuan-Zhe, Fan Wei, Yang Guang-Jie, Han Xian-Wei. Fluid simulation for detachment process in magnetic nozzle of magnetoplasma rocket engine. Acta Physica Sinica, 2024, 73(10): 105201. doi: 10.7498/aps.73.20231862 [2] . Preface to the special topic: Energetic particles in magnetic confinement fusion. Acta Physica Sinica, 2023, 72(21): 210101. doi: 10.7498/aps.72.210101 [3] Zhou Li-Na, Hu Han-Qing, Liu Yue-Qiang, Duan Ping, Chen Long, Zhang Han-Yu. Modelling study of fluid and kinetic responses of plasmas to resonant magnetic perturbation. Acta Physica Sinica, 2023, 72(7): 075202. doi: 10.7498/aps.72.20222196 [4] Su Xiang, Wang Xian-Qu, Fu Tian, Xu Yu-Hong. Suppression mechanism of equilibrium magnetic islands in CFQS low- $\boldsymbol \beta$ plasma. Acta Physica Sinica, 2023, 72(21): 215205. doi: 10.7498/aps.72.20230546[5] Man Liang, Deng Hao-Chuan, Wu Yang, Yu Xi-Long, Xiao Zhi-He. Echo spectrum modulation characteristics of plasma flow field simulated by wind tunnel. Acta Physica Sinica, 2022, 71(3): 035203. doi: 10.7498/aps.71.20211471 [6] Chen Guo-Hua, Shi Ke-Jun, Chu Jin-Ke, Wu Hao, Zhou Chi-Lou, Xiao Shu. Numerical simulation and optimization of cooling flow field of cylindrical cathode with annular magnetic field. Acta Physica Sinica, 2021, 70(7): 075203. doi: 10.7498/aps.70.20201368 [7] . Experimental study on echo spectrum modulation characteristics of plasma flow field simulated by wind tunnel. Acta Physica Sinica, 2021, (): . doi: 10.7498/aps.70.20211471 [8] Su Chun-Yan, Mou Mao-Lin, Chen Shao-Yong, Guo Wen-Ping, Tang Chang-Jian. Field amplification effect of resonant magnetic perturbation on ion orbits in tokamak plasma. Acta Physica Sinica, 2021, 70(9): 095207. doi: 10.7498/aps.70.20201860 [9] Yu Jia-Cheng, Zhong Jia-Yong, An Wei-Ming, Ping Yong-Li. Potential distribution behind target in intense and short pulsed laser-driven magnetic reconnection. Acta Physica Sinica, 2021, 70(6): 065201. doi: 10.7498/aps.70.20201339 [10] Yuan Xiao-Xia, Zhong Jia-Yong. Simulations for two colliding plasma bubbles embedded into an external magnetic field. Acta Physica Sinica, 2017, 66(7): 075202. doi: 10.7498/aps.66.075202 [11] Zhang Kai, Zhong Jia-Yong, Pei Xiao-Xing, Li Yu-Tong, Sakawa Youichi, Wei Hui-Gang, Yuan Da-Wei, Li Fang, Han Bo, Wang Chen, He Hao, Yin Chuan-Lei, Liao Guo-Qian, Fang Yuan, Yang Su, Yuan Xiao-Hui, Liang Gui-Yun, Wang Fei-Lu, Zhu Jian-Qiang, Ding Yong-Kun, Zhang Jie, Zhao Gang. Measurement of jet evolution and electron energy spectrum during the process of laser-driven magnetic reconnection. Acta Physica Sinica, 2015, 64(16): 165201. doi: 10.7498/aps.64.165201 [12] Hu Ming, Wan Shu-De, Zhong Lei, Liu Hao, Wang Hai. Magnetic control of the constant-current glow discharge plasma characteristics. Acta Physica Sinica, 2012, 61(4): 045201. doi: 10.7498/aps.61.045201 [13] Liu Hui-Ping, Zou Xiu, Zou Bin-Yan, Qiu Ming-Hui. Bohm criterion for an electronegative magnetized plasma sheath. Acta Physica Sinica, 2012, 61(3): 035201. doi: 10.7498/aps.61.035201 [14] Li Gang, Li Yi-Ming, Xu Yan-Ji, Zhang Yi, Li Han-Ming, Nie Chao-Qun, Zhu Jun-Qiang. Experimental study of near wall region flow control by dielectric barrier discharge plasma. Acta Physica Sinica, 2009, 58(6): 4026-4033. doi: 10.7498/aps.58.4026 [15] Zheng Yong-Zhen, Qi Chang-Wei, Ding Xuan-Tong, Lee Wen-Zhong. Internal magnetic fluctuation in the HL-1M tokamak. Acta Physica Sinica, 2006, 55(1): 294-298. doi: 10.7498/aps.55.294 [16] WU CHENG, YE MAO-FU, ZHANG BAO-ZHEN, LI YIN-AN. THE COMPRESSION OF AND THE FLUX TRAPPED IN A FIELD-REVERSED PINCH PLASMA. Acta Physica Sinica, 1987, 36(9): 1105-1111. doi: 10.7498/aps.36.1105 [17] DONG JIA-QI. DOUBLE TEARING MODE IN PLASMA WITH MAGNETIC BRAIDING. Acta Physica Sinica, 1984, 33(10): 1341-1349. doi: 10.7498/aps.33.1341 [18] LI JIA-QUAN. LOW TEMPERATURE PLASMA IN A MAGNETIC WELL. Acta Physica Sinica, 1980, 29(11): 1471-1478. doi: 10.7498/aps.29.1471 [19] SHI CHANG-HE. MAGNETOHYDRODYNAMIC INSTABILITY OF A NONHOMOGE-NEOUS PLASMA LAMINAR STREAM. Acta Physica Sinica, 1979, 28(2): 263-267. doi: 10.7498/aps.28.263 [20] . . Acta Physica Sinica, 1965, 21(1): 227-230. doi: 10.7498/aps.21.227
DownLoad:
Catalog
Metrics
- Abstract views: 6208
- PDF Downloads: 72
- Cited By: 0
