-
首先, 利用MARS-F程序模拟了HL-2A装置中不同电阻率、环向旋转频率和环向模数条件下等离子体对共振磁扰动(RMP)的线性响应过程, 分析了不同情况下共振场放大效应对三维扰动磁场的影响; 然后, 利用Boris算法追踪了三维场作用下的离子轨道, 并详细探究了不同扰动磁场改变离子轨道特性的物理机制. 研究发现, 考虑等离子体响应后的扰动磁场可增强离子轨道径向展宽, 且轨道最大径向展宽随轨道上扰动磁场平均值的增大而增大; 同时, 离子在通过扰动磁场被强烈放大的区域时轨道径向展宽会显著增加. 该物理机制可用于解释RMP缓解边界局域模实验中离子直接损失增加和等离子体径向输运增强现象.
The fast ion transport associated with resonant magnetic perturbation (RMP) contains rich physical spanning single particle motion of fast particle and plasmas response physics with RMP and their interaction. Full numerical simulation considering such physical ingredients should be performed in a long run for clarifying the underlying physical features of the fast ion confinement with RMP. Thus, the appropriate application of RMP is not only to avoid the detrimental effects but also to serve as an actuator to exert targeted control over the energetic particle profile. To achieve this goal, a comprehensive knowledge of the effects of RMP including plasma response on fast ions is necessary. In this work, the plasma response to RMP in HL-2A device is simulated by the MARS-F code under different parameters including finite resistivity, toroidal rotation frequency and toroidal mode number, and the three-dimensional (3D) magnetic field topology considering RMP is obtained. Then, Boris algorithm is used to track the ion orbit under these 3D fields, and the physical mechanism of ion orbit characteristics changed by the perturbed field is explored in detail. It is found that with the increase of finite resistivity, the average value of perturbed magnetic field decreases, and the orbit radial expansion turns smaller. The variation of toroidal rotation frequency can change the distribution of perturbed magnetic field, resulting in different orbit radial expansions for different kinds of orbits. What is more, if the toroidal mode number increases, the amplitude of perturbed magnetic field after response decreases obviously, thus resulting in little effect on orbit radial expansion. In a word, the plasma responded RMP field enhances the orbit radial expansion, and the maximum orbital radial expansion increases with the augment of average value of perturbed magnetic field on the orbit. Meanwhile, the amplitude of orbit expansion increases significantly when the ions pass through the region where the perturbed magnetic field is strongly amplified. This effect can explain the increase of ion prompt loss and enhancement of plasma radial transport in edge localized mode mitigation experiments by RMP. -
Keywords:
- resonant magnetic perturbation /
- plasma response /
- resonant field amplification /
- ion orbit radial expansion
[1] Wan Y, Li J, Liu Y, et al. 2017 Nucl. Fusion 57 102009
Google Scholar
[2] Buttery R J, Boozer A H, Liu Y Q, et al. 2012 Phys. Plasma 19 056111
Google Scholar
[3] Evans T E, Moyer R A, Thomas P R, et al. 2004 Phys. Rev. Lett. 92 235003
Google Scholar
[4] Evans T E, Fenstermacher M E, Moyer R A, et al. 2008 Nucl. Fusion 48 024002
Google Scholar
[5] Liu Y, Ham C J, Kirk A, et al. 2016 Plasma Phys. Controlled Fusion 58 114005
Google Scholar
[6] Liu Y, Kirk A, Li L, et al. 2017 Phys. Plasmas 24 056111
Google Scholar
[7] Liu Y, Paz-Soldan C, Li L, Sun Y 2020 Nucl. Fusion 60 036018
Google Scholar
[8] Van Zeeland M A, Ferraro N M, Heidbrink W W, et al. 2014 Plasma Phys. Controlled Fusion 56 015009
Google Scholar
[9] Van Zeeland M A, Ferraro N M, Grierson B A, et al. 2015 Nucl. Fusion 55 073028
Google Scholar
[10] Kim K, Jhang H, Kim J, Rhee T 2018 Phys. Plasmas 25 122511
Google Scholar
[11] Garcia-Munoz M, Äkäslompolo S, de Marne P, et al. 2013 Plasma Phys. Controlled Fusion 55 124014
Google Scholar
[12] McClements K G, Akers R J, Boeglin W U, et al. 2015 Plasma Phys. Controlled Fusion 57 075003
Google Scholar
[13] Mou M L, Wang Z T, Wu N, Chen S Y, Tang C J 2017 Nucl. Fusion 57 046023
Google Scholar
[14] He K, Wan B N, Sun Y, Jia M, Shi T, Wang H H, Zhang X J 2019 Nucl. Fusion 59 126026
Google Scholar
[15] Turnbull A D, Ferraro N M, Izzo V A, et al. 2013 Phys. Plasmas 20 056114
Google Scholar
[16] Liu Y, Kirk A, Nardon E 2010 Phys. Plasmas 17 122502
Google Scholar
[17] Reimerdes H, Bialek J, Chance M S, et al. 2005 Nucl. Fusion 45 368
Google Scholar
[18] Gryaznevich M P, Hender T C, Howell D F, et al. 2008 Plasma Phys. Controlled Fusion 50 124030
Google Scholar
[19] Park J K, Schaffer M J, Menard J E, Boozer A H 2007 Phys. Rev. Lett. 99 195003
Google Scholar
[20] Kirk A, Nardon E, Akers R, et al. 2010 Nucl. Fusion 50 034008
Google Scholar
[21] Kirk A, Liu Y, Nardon E, et al. 2011 Plasma Phys. Controlled Fusion 53 065011
Google Scholar
[22] Liu Y, Kirk A, Gribov Y, Gryaznevich M P, Hender T C, Nardon E 2011 Nucl. Fusion 51 083002
Google Scholar
[23] Liu Y, Kirk A, Thornton A J 2014 Plasma Phys. Controlled Fusion 56 104002
Google Scholar
[24] Liu Y, Ryan D, Kirk A, et al. 2016 Nucl. Fusion 56 056015
Google Scholar
[25] Boris J 1970 Proceedings of the Fourth Conference on Numerical Simulation of Plasmas Washington DC, USA, November 2–3, 1970 pp3−67
[26] Birdsall C, Langdon A 1985 Plasma Physics via Computer Simulation (New York: McGraw-Hill)
[27] Qin H, Zhang S, Xiao J, Liu J, Sun Y, Tang W M 2013 Phys. Plasmas 20 0845031
[28] 邵福球 2002 等离子体粒子模拟 (北京: 科学出版社) 第101页
Shao F Q 2002 Plasma Particle Simulation (Beijing: Science Press) p101 (in Chinese)
[29] Lao L, John H S, Stambaugh R D, Kellman A G, Pfeiffer W 1985 Nucl. Fusion 25 1611
[30] 陈撷宇, 牟茂淋, 苏春燕, 陈少永, 唐昌建 2020 69 195201
Google Scholar
Chen X Y, Mou M L, Su C Y, Chen S Y, Tang C J 2020 Acta Phys. Sin. 69 195201
Google Scholar
[31] Yang X, Sun Y, Liu Y, et al. 2016 Plasma Phys. Controlled Fusion 58 114006
Google Scholar
-
图 1 HL-2A第29676炮放电的平衡参数分布(1 G = 10–4 T) (a)平衡磁场分布; (b)安全因子径向剖面; (c)归一化的等离子体环向旋转频率; (d)归一化电阻率; 黑色竖直虚线分别代表
$ q=2, 3, 4 $ 的共振面位置Fig. 1. Distribution of the plasma equilibrium parameters for HL-2A discharge 29676: (a) Equilibrium magnetic field; (b) safety factor; (c) plasma toroidal rotation, normalized to the Alfvén frequency at the plasma center; (d) normalized plasma resistivity. The vertical lines indicate the radial locations of resonant surfaces for
$ q=2, 3, 4 $ , respectively.
图 2 Case 1—Case 4情况下, 真空RMP磁场(蓝线)和等离子体响应后总扰动磁场(红线)各极向谐波分量的径向剖面, 黑色虚线代表共振面位置
Fig. 2. Comparison of radial profiles between the vacuum (blue lines) and plasma response (red lines) RMP fields for the resonant poloidal Fourier harmonics in Case 1–Case 4. The dashed vertical lines indicate the radial locations of resonant surfaces.
图 3 在(a)平衡场、(b)真空近似RMP场、(c)−(f) Case1−Case 4等离子体响应扰动磁场作用下的R-Z平面磁场庞加莱图(图中红点分别代表
$ q=2, 3, 4 $ 的共振面位置)Fig. 3. Poincare plots of magnetic field configuration on the R-Z plane for (a) unperturbed field, (b) vacuum RMP field and (c)–(f) plasma response RMP field for Case 1–Case 4. The red dots represent
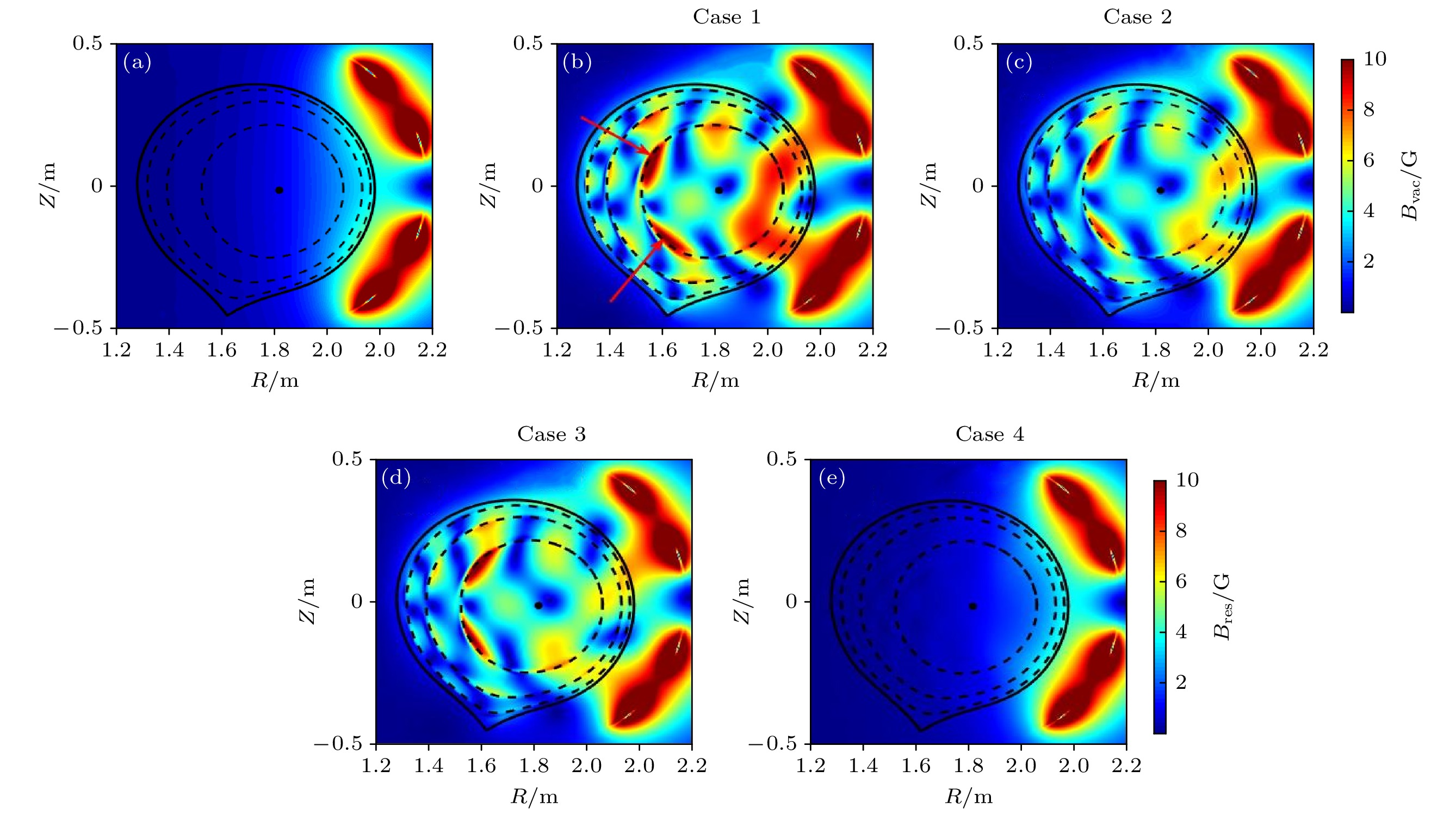
$ q=2, 3, 4 $ resonant surfaces.
图 4 (a)真空近似RMP场、(b)−(e) Case 1−Case 4情况下等离子体响应后的总扰动磁场幅值在
$ \phi =0 $ 小截面的分布; 其中黑色实线代表最外闭合磁面位置, 黑色虚线分别代表$ q=2, 3, 4 $ 的共振面位置, 黑色实心点代表磁轴位置, 最外闭合磁面外的两个强磁场区域代表RMP线圈位置, 图4(b)中红色箭头处为共振场强放大区域Fig. 4. Contour plots of peak amplitudes of the perturbed field due to (a) vacuum RMP and (b)–(e) plasma response RMP fields for Case 1–Case 4 in cross section with
$ \phi =0 $ , respectively. The black solid line is the LCFS, the black dashed lines are the positions of$ q=2, 3, 4 $ resonant surfaces, the black point represents the position of the magnetic axis. The two strong magnetic field regions outside the LCFS represent the positions of RMP coils. The areas pointed by red arrows in Fig. 4(b) are the regions with strong field amplification.
图 5 无扰动磁场作用时, 分别从外中平面上q = (a) 2, (b) 3, (c) 4共振面(黑色虚线)出发的基本离子轨道, 其中红线代表通行轨道, 蓝线代表捕获轨道
Fig. 5. Fundamental ion orbits with unperturbed magnetic fields which launched from (a)
$ q=2 $ , (b)$ q=3 $ , (c)$ q=4 $ resonant surfaces, respectively. The red lines represent passing orbits, and the blue lines represent trapped orbits.
图 6 在Case 1—Case 4的扰动磁场作用下, 从外中平面上
$ q=2 $ 共振面出发反向入射的典型通行(红线)和捕获(蓝线)轨道(黑色虚线分别代表$ q=2, 3, 4 $ 的共振面位置)Fig. 6. Typical ion orbits with Case 1–Case4 plasma response RMP field which launched from outer middle plane of
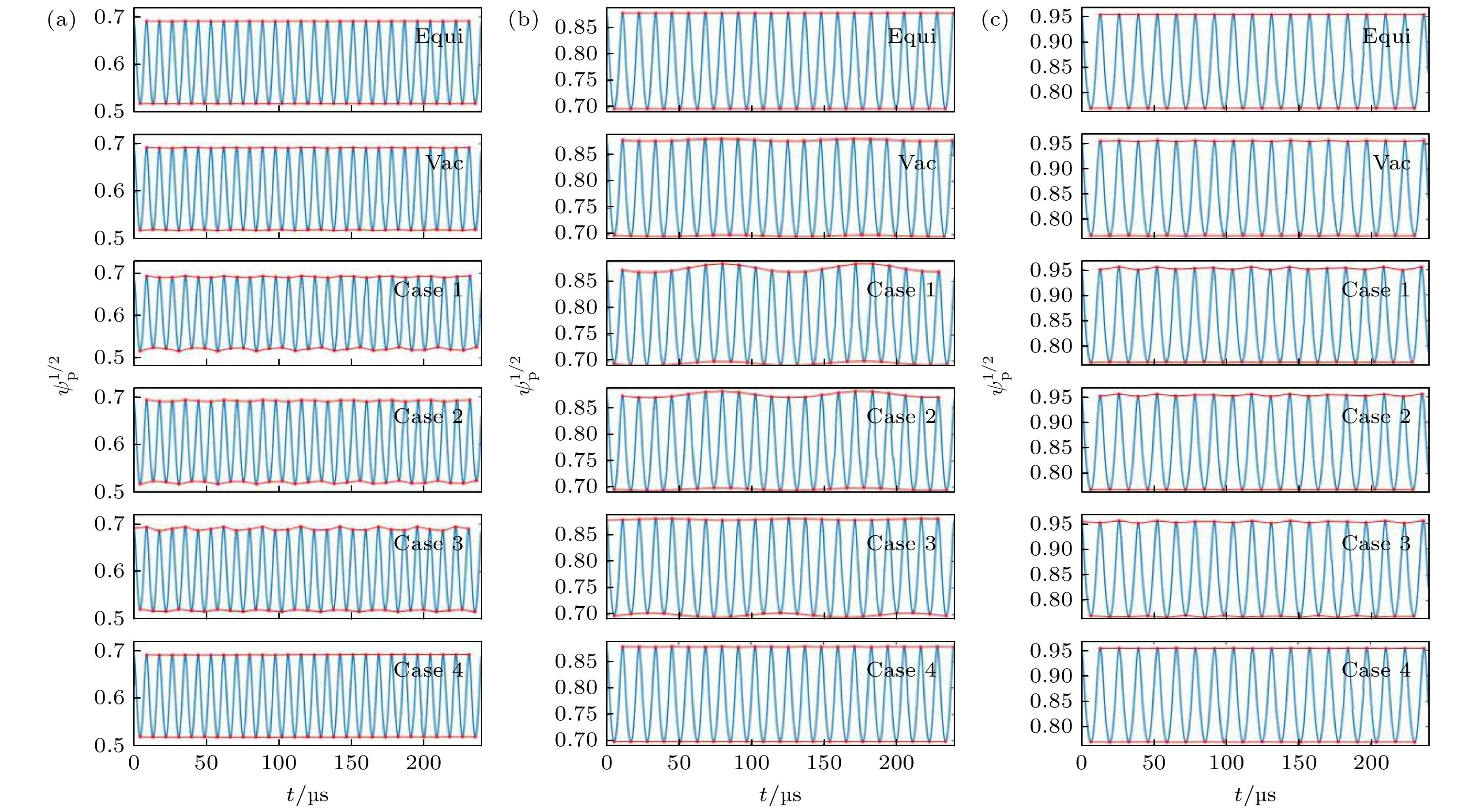
$ q=2 $ resonant surface. The red lines represent passing orbits, and the blue lines represent trapped orbits. The black dashed lines are the positions of$ q=2, 3, 4 $ resonant surfaces.
图 7 不同扰动磁场作用下通行轨道极向磁通随时间的变化 (a)—(c)离子轨道初始位置分别为
$ q=2, 3, 4 $ 的有理面, 离子初始能量$ {E}_{0}=30\;\mathrm{k}\mathrm{e}\mathrm{V} $ Fig. 7. Variations of the poloidal flux for passing orbits launched from (a)
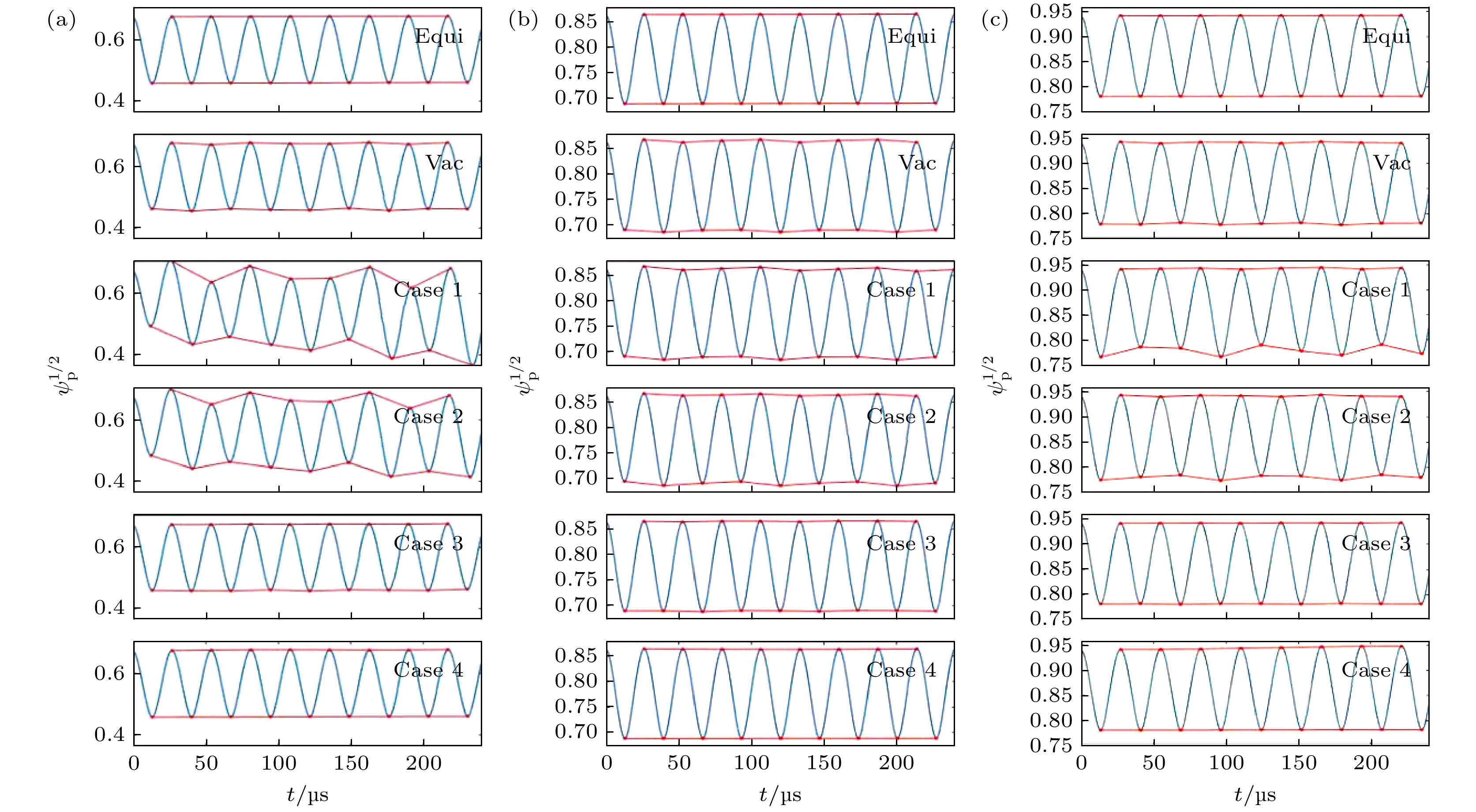
$ q=2 $ , (b)$ q=3 $ , (c)$ q=4 $ resonant surfaces with different perturbation magnetic fields. The ion initial energy$ {E}_{0}=30\;\mathrm{k}\mathrm{e}\mathrm{V} $ .
图 8 不同扰动磁场作用下捕获轨道极向磁通 (a)—(c)离子轨道初始位置分别为
$ q=2, 3, 4 $ 的有理面, 离子初始能量${E}_{0}= $ $ 30\;\mathrm{k}\mathrm{e}\mathrm{V}$ Fig. 8. Variations of the poloidal flux for trapped orbits launched from (a)
$ q=2 $ , (b)$ q=3 $ , (c)$ q=4 $ resonant surfaces with different perturbation magnetic fields. The ion initial energy$ {E}_{0}=30\;\mathrm{k}\mathrm{e}\mathrm{V} $ .
图 9 初始能量
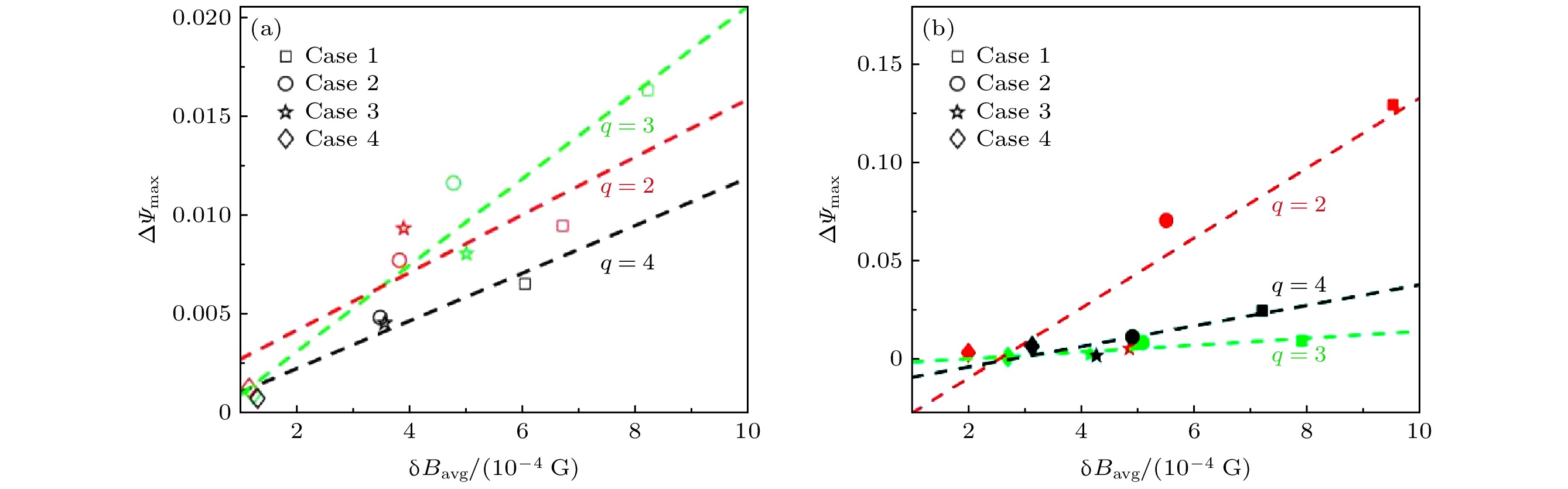
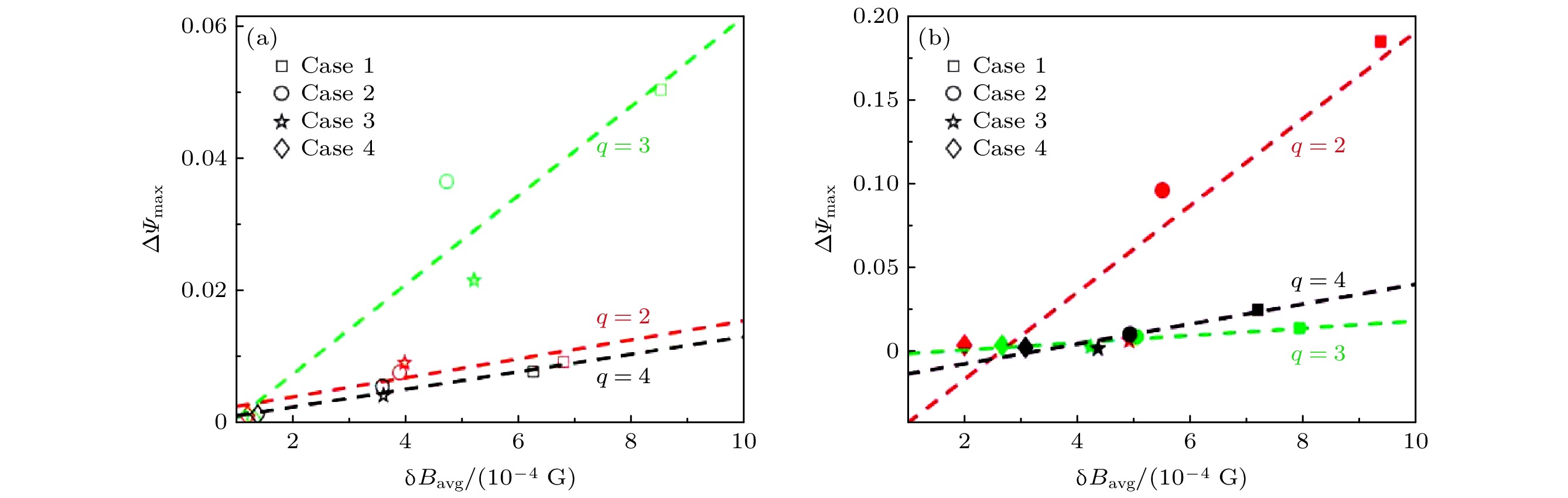
$ {E}_{0}=30\;\mathrm{k}\mathrm{e}\mathrm{V} $ 的通行(a)和捕获(b)离子轨道最大径向展宽($ \Delta {\varPsi }_{\mathrm{m}\mathrm{a}\mathrm{x}} $ )随轨道上扰动磁场平均值($ \text{δ}{B}_{\mathrm{a}\mathrm{v}\mathrm{g}} $ )的变化(图中正方形、圆形、五角星和菱形依次对应Case 1—Case 4扰动磁场的情况, 红、绿、黑色图形分别表示初始位置为$ q=2, 3, 4 $ 共振面上轨道参量的计算结果, 红、绿、黑色虚线为对相同颜色图形进行数值拟合的结果)Fig. 9. Variations of the maximum expansion distance (
$ \Delta {\varPsi }_{\mathrm{m}\mathrm{a}\mathrm{x}} $ ) and the average value of the orbital response field ($\text{δ}{B}_{\mathrm{a}\mathrm{v}\mathrm{g}}$ ) for (a) passing orbits and (b) trapped orbits with$ {E}_{0}=30\;\mathrm{k}\mathrm{e}\mathrm{V} $ . The square, circle, pentagram and diamond in the figure correspond to perturbation magnetic field in Case 1–Case 4, respectively. The red, green and black labels represent the calculation results for ions launched from$ q=2, 3, 4 $ resonant surfaces, respectively. The red, green and black dashed lines are the numerical fitting results of the same-colored labels.
图 10 理想等离子体响应场(Case 1)下, (a)从
$ q=3 $ 共振面出发的通行离子轨道(红线), (b)从$ q=2 $ 共振面出发的捕获离子轨道(蓝线)(图中彩色背景为理想等离子体响应后的总扰动磁场在$ \phi =0 $ 小截面的分布, 黑色实线代表最外闭合磁面位置, 黑色虚线分别代表$ q=2, 3, 4 $ 的共振面位置, 红(蓝)色箭头所指为强(弱)场侧两个扰动磁场明显增强的区域)Fig. 10. Trajectory projection of ions orbit in ideal plasma response field (Case 1): (a) Passing orbit (red line) launched from
$ q=3 $ resonant surface; (b) trapped orbit (blue line) launched from$ q=2 $ resonant surface. The background is the distribution of plasma response RMP field in$ \phi =0 $ cross section, and the black solid line is the LCFS, the black dashed lines are the positions of$ q=\;2, 3, 4 $ resonant surfaces. The areas pointed by the red (blue) arrows are two regions with the strong field amplification in high (low) field side.
图 11 初始能量为
$ {E}_{0}=40\;\mathrm{k}\mathrm{e}\mathrm{V} $ 的通行(a)和捕获(b)离子轨道最大径向展宽($ \Delta {\varPsi }_{\mathrm{m}\mathrm{a}\mathrm{x}} $ )随轨道上扰动磁场平均值($ \text{δ}{B}_{\mathrm{a}\mathrm{v}\mathrm{g}} $ )的变化(图中正方形、圆形、五角星和菱形依次对应Case 1—Case 4扰动磁场的情况, 红、绿、黑色图形分别表示初始位置为$ q=2, 3, 4 $ 共振面上轨道参量的计算结果, 红、绿、黑色虚线为对相同颜色图形进行数值拟合的结果)Fig. 11. Variations of the maximum expansion distance (
$ \Delta {\varPsi }_{\mathrm{m}\mathrm{a}\mathrm{x}} $ ) and the average value of the orbital response field ($ \text{δ}{B}_{\mathrm{a}\mathrm{v}\mathrm{g}} $ ) for (a) passing orbits and (b) trapped orbits with$ {E}_{0}=40\;\mathrm{k}\mathrm{e}\mathrm{V} $ . The square, circle, pentagram and diamond in the figure correspond to perturbation magnetic field in Case 1–Case 4, respectively. The red, green and black labels represent the calculation results for ions launched from$ q=2, 3, 4 $ resonant surfaces, respectively. The red, green and black dashed lines are the numerical fitting results of the same-colored labels.表 1 不同算例的等离子参数
Table 1. Plasma parameters of different calculation cases.
Case n $ {\omega }_{0}/{\omega }_{\mathrm{A}} $ $ {\eta }_{0} $ 1 1 2.341$ \times {10}^{-2} $ 0 2 1 2.341$ \times {10}^{-2} $ $ 1.7524\times {10}^{-8} $ 3 1 1.000$ \times {10}^{-2} $ $ 1.7524\times {10}^{-8} $ 4 3 2.341$ \times {10}^{-2} $ $ 1.7524\times {10}^{-8} $ 
下载: 导出CSV
-
[1] Wan Y, Li J, Liu Y, et al. 2017 Nucl. Fusion 57 102009
Google Scholar
[2] Buttery R J, Boozer A H, Liu Y Q, et al. 2012 Phys. Plasma 19 056111
Google Scholar
[3] Evans T E, Moyer R A, Thomas P R, et al. 2004 Phys. Rev. Lett. 92 235003
Google Scholar
[4] Evans T E, Fenstermacher M E, Moyer R A, et al. 2008 Nucl. Fusion 48 024002
Google Scholar
[5] Liu Y, Ham C J, Kirk A, et al. 2016 Plasma Phys. Controlled Fusion 58 114005
Google Scholar
[6] Liu Y, Kirk A, Li L, et al. 2017 Phys. Plasmas 24 056111
Google Scholar
[7] Liu Y, Paz-Soldan C, Li L, Sun Y 2020 Nucl. Fusion 60 036018
Google Scholar
[8] Van Zeeland M A, Ferraro N M, Heidbrink W W, et al. 2014 Plasma Phys. Controlled Fusion 56 015009
Google Scholar
[9] Van Zeeland M A, Ferraro N M, Grierson B A, et al. 2015 Nucl. Fusion 55 073028
Google Scholar
[10] Kim K, Jhang H, Kim J, Rhee T 2018 Phys. Plasmas 25 122511
Google Scholar
[11] Garcia-Munoz M, Äkäslompolo S, de Marne P, et al. 2013 Plasma Phys. Controlled Fusion 55 124014
Google Scholar
[12] McClements K G, Akers R J, Boeglin W U, et al. 2015 Plasma Phys. Controlled Fusion 57 075003
Google Scholar
[13] Mou M L, Wang Z T, Wu N, Chen S Y, Tang C J 2017 Nucl. Fusion 57 046023
Google Scholar
[14] He K, Wan B N, Sun Y, Jia M, Shi T, Wang H H, Zhang X J 2019 Nucl. Fusion 59 126026
Google Scholar
[15] Turnbull A D, Ferraro N M, Izzo V A, et al. 2013 Phys. Plasmas 20 056114
Google Scholar
[16] Liu Y, Kirk A, Nardon E 2010 Phys. Plasmas 17 122502
Google Scholar
[17] Reimerdes H, Bialek J, Chance M S, et al. 2005 Nucl. Fusion 45 368
Google Scholar
[18] Gryaznevich M P, Hender T C, Howell D F, et al. 2008 Plasma Phys. Controlled Fusion 50 124030
Google Scholar
[19] Park J K, Schaffer M J, Menard J E, Boozer A H 2007 Phys. Rev. Lett. 99 195003
Google Scholar
[20] Kirk A, Nardon E, Akers R, et al. 2010 Nucl. Fusion 50 034008
Google Scholar
[21] Kirk A, Liu Y, Nardon E, et al. 2011 Plasma Phys. Controlled Fusion 53 065011
Google Scholar
[22] Liu Y, Kirk A, Gribov Y, Gryaznevich M P, Hender T C, Nardon E 2011 Nucl. Fusion 51 083002
Google Scholar
[23] Liu Y, Kirk A, Thornton A J 2014 Plasma Phys. Controlled Fusion 56 104002
Google Scholar
[24] Liu Y, Ryan D, Kirk A, et al. 2016 Nucl. Fusion 56 056015
Google Scholar
[25] Boris J 1970 Proceedings of the Fourth Conference on Numerical Simulation of Plasmas Washington DC, USA, November 2–3, 1970 pp3−67
[26] Birdsall C, Langdon A 1985 Plasma Physics via Computer Simulation (New York: McGraw-Hill)
[27] Qin H, Zhang S, Xiao J, Liu J, Sun Y, Tang W M 2013 Phys. Plasmas 20 0845031
[28] 邵福球 2002 等离子体粒子模拟 (北京: 科学出版社) 第101页
Shao F Q 2002 Plasma Particle Simulation (Beijing: Science Press) p101 (in Chinese)
[29] Lao L, John H S, Stambaugh R D, Kellman A G, Pfeiffer W 1985 Nucl. Fusion 25 1611
[30] 陈撷宇, 牟茂淋, 苏春燕, 陈少永, 唐昌建 2020 69 195201
Google Scholar
Chen X Y, Mou M L, Su C Y, Chen S Y, Tang C J 2020 Acta Phys. Sin. 69 195201
Google Scholar
[31] Yang X, Sun Y, Liu Y, et al. 2016 Plasma Phys. Controlled Fusion 58 114006
Google Scholar
下载:
计量
- 文章访问数: 5193
- PDF下载量: 76
- 被引次数: 0
