Some recent progresses on the study of ultracold quantum gases with spin-orbit coupling

Shi Ting-Ting; Wang Liu-Jiu; Wang Jing-Kun; Zhang Wei

doi:10.7498/aps.69.20191241

Abstract

Artificial synthetic gauge field and spin-orbit coupling has been extensively studied following their experimental realization in ultracold atomic systems. Thanks for the versatile controllability, such systems not only provide possibilities to simulate and study important models in multidisciplinary fields of physics, but also work as an excellent platform to engineer novel states of matter and quantum phenomena. This paper reviews some recent progresses on the study of ultracold atomic systems with spin-orbit coupling, focusing on the effects induced by dissipation, novel interaction forms, large symmetry of spins, and long-range interactions. The investigation in these aspects is closely related to the characteristics of ultracold atomic systems, hence can bring new inspirations and perspectives on the understanding of spin-orbit coupling. In this review, we firstly investigate the appearance of a topological superradiant state in a quasi-one-dimensional Fermi gas with cavity-assisted Raman process. A cavity-assisted spin-orbit coupling and a bulk gap opening at half filling will be induced by the superradiant light generated in the transversely driven cavity mode. The topological superradiant state and the corresponding topological phase transition in the system can be driven by this mechanism. Then, symmetry-protected topological states of interacting fermions will be introduced in a quasi-one-dimensional cold gas of alkaline-earth-like atoms. Raman-assisted spin-orbit couplings in the clock states, together with the spin-exchange interactions in the clock-state manifolds will give rise to symmetry-protected topological states for interacting fermions, by taking advantage of the separation of orbital and nuclear-spin degrees of freedom in these alkaline-earth-like atoms. Furthermore, we show that an exotic topological defect, double-quantum spin vortices, which are characterized by doubly quantized circulating spin currents and unmagnetized filled cores, can exist in the ground states of SU(3) spin-orbit-coupled Bose-Einstein condensates. It is found that the combined effects of SU(3) spin-orbit coupling and spin-exchange interaction determine the ground-state phase diagram. Finally, we demonstrate that spin-orbit coupling and soft-core long-range interaction can induce an exotic supersolid phase of Bose gas, with the emergence of spontaneous circulating particle current. This implies that a finite angular momentum can be generated with neither external rotation nor synthetic magnetic field, and the direction of the angular momentum can be altered by adjusting the strength of spin-orbit coupling or interatomic interaction.

Keywords:

Authors and contacts

1.
Department of Physics, Renmin University of China, Beijing 100872, China
2.
Beijing Key Laboratory of Opto-electronic Functional Materials and Micro-nano Devices, Renmin University of China, Beijing 100872, China

Corresponding author: Wang Jing-Kun, jkwang@ruc.edu.cn ; Zhang Wei, wzhangl@ruc.edu.cn

Funds: Project supported by the National Key R&D Program of China (Grant No. 2018YFA0306501), the National Natural Science Foundation of China (Grant Nos. 11434011, 11522436, 11774425), and the Research Funds of Renmin University of China (Grant Nos. 16XNLQ03, 18XNLQ15)

FullText HTML : translate this paragraph

References

[1]	Gong M, Tewari S, Zhang C 2011 Phys. Rev. Lett. 107 195303 Google Scholar
[2]	Hu H, Jiang L, Liu X J, Pu H 2011 Phys. Rev. Lett. 107 195304 Google Scholar
[3]	Han L, Melo C A R S' a de 2012 Phys. Rev. A 85 011606(R)Google Scholar
[4]	Yu Z Q, Zhai H 2011 Phys. Rev. Lett. 107 195305 Google Scholar
[5]	Iskin M, Subası A L 2011 Phys. Rev. Lett. 107 050402 Google Scholar
[6]	Yi W, Guo G C 2011 Phys. Rev. A 84 031608(R)Google Scholar
[7]	Lin Y J, Jiménez-García K, Spielman I B 2011 Nature (London) 471 83 Google Scholar
[8]	Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H, Chai S J, Zhai H, Zhang J 2012 Phys. Rev. Lett. 109 095301 Google Scholar
[9]	Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302 Google Scholar
[10]	Huang L H, Meng Z M, Wang P J, Peng P, Zhang S L, Chen L C, Li D H, Zhou Q, Zhang J 2016 Nat. Phys. 12 540 Google Scholar
[11]	Wu Z, Zhang L, Sun W, Xu X T, Wang B Z, Ji S C, Deng Y, Chen S, Liu X J, Pan J W 2016 Science 354 83 Google Scholar
[12]	Chen H R, Lin K Y, Chen P K, Chiu N C, Wang J B, Chen C A, Huang P P, Yip S K, Kawaguchi Y, Lin Y J 2018 Phys. Rev. Lett. 121 113204 Google Scholar
[13]	Zhang D F, Gao T Y, Zou P, Kong L R, Li R Z, Shen X, Chen X L, Peng S G, Zhan M S, Pu H, Jiang K J 2019 Phys. Rev. Lett. 122 110402 Google Scholar
[14]	Zhai H 2015 Rep. Prog. Phys. 78 026001 Google Scholar
[15]	Vyasanakere J P, Shenoy V B 2011 Phys. Rev. B 83 094515 Google Scholar
[16]	Vyasanakere J P, Zhang S Z, Shenoy V B 2011 Phys. Rev. B 84 014512 Google Scholar
[17]	Xu Z F, Lü R, You L 2011 Phys. Rev. A 83 053602 Google Scholar
[18]	Kawakami T, Mizushima T, Machida K 2011 Phys. Rev. A 84 011607 Google Scholar
[19]	Wu C J, Mondragon-Shem I, Zhou X F 2011 Chin. Phys. Lett. 28 097102 Google Scholar
[20]	Stanescu T D, Anderson B, Galitski V 2008 Phys. Rev. A 78 023616 Google Scholar
[21]	Deng Y, Cheng J, Jing H, Sun C P, Yi S 2012 Phys. Rev. Lett. 108 125301 Google Scholar
[22]	Kawakami T, Mizushima T, Nitta M, Machida K 2012 Phys. Rev. Lett. 109 015301 Google Scholar
[23]	Li Y, Zhou X F, Wu C J 2016 Phys. Rev. A 93 033628 Google Scholar
[24]	Sinha S, Nath R, Santos L 2011 Phys. Rev. Lett. 107 270401 Google Scholar
[25]	Hu H, Ramachandhran B, Pu H, Liu X J 2012 Phys. Rev. Lett. 108 010402 Google Scholar
[26]	Ramachandhran B, Hu H, Pu H 2013 Phys. Rev. A 87 033627 Google Scholar
[27]	Li Y, Zhou X F, Wu C J 2012 Phys. Rev. B 85 125122 Google Scholar
[28]	Campbell D L, Juzeliūnas G, Spielman I B 2011 Phys. Rev. A 84 025602 Google Scholar
[29]	Sau J D, Sensarma R, Powell S, Spielman I B, Sarma S D 2011 Phys. Rev. B 83 140510(R)Google Scholar
[30]	Xu Z F, You L 2012 Phys. Rev. A 85 043605 Google Scholar
[31]	Liu X J, Law K T, Ng T K 2014 Phys. Rev. Lett. 112 086401 Google Scholar
[32]	Anderson B M, Spielman I B, Juzeliūnas 2013 Phys. Rev. Lett. 111 125301 Google Scholar
[33]	Xu Z F, You L, Ueda M 2013 Phys. Rev. A 87 063634 Google Scholar
[34]	Anderson B M, Juzeliünas G, Galitski V M, Spielman I B 2012 Phys. Rev. Lett. 108 235301 Google Scholar
[35]	Zhou J, Zhang W, Yi W 2011 Phys. Rev. A 84 063603 Google Scholar
[36]	Chen J, Hu H, Gao X L 2014 Phys. Rev. A 90 023619 Google Scholar
[37]	Chen C 2013 Phys. Rev. Lett. 111 235302 Google Scholar
[38]	Qu C L, Zheng Z, Gong M, Xu Y, Mao L, Zou X B, Guo G C, Zhang C W 2013 Nat. Commun. 4 2710 Google Scholar
[39]	Liu X J, Hu H 2013 Phys. Rev. A 88 023622 Google Scholar
[40]	Zhang W, Yi W 2013 Nat. Commun. 4 2711 Google Scholar
[41]	Cui X L, Yi W 2014 Phys. Rev. X 4 031026
[42]	Shi Z Y, Cui X L, Zhai H 2014 Phys. Rev. Lett 112 013201 Google Scholar
[43]	Pan J S, Liu X J, Zhang W, Yi W, Guo G C 2015 Phys. Rev. Lett. 115 045303 Google Scholar
[44]	Han W, Zhang X F, Song S W, Saito H, Zhang W, Liu W M, Zhang S G 2016 Phys. Rev. A 94 033629 Google Scholar
[45]	Han W, Zhang X F, Wang D S, Jiang K J, Zhang W, Zhang S G 2018 Phys. Rev. Lett. 121 030404 Google Scholar
[46]	Zhou X F, Pan J S, Liu Z X, Zhang W, Yi W, Chen G, Jia S T 2017 Phys. Rev. Lett. 119 185701 Google Scholar
[47]	Dalibard J, Gerbier F, Juzeliūnas G, Öberg P 2011 Rev. Mod. Phys. 83 1523 Google Scholar
[48]	Yi W, Zhang W, Cui X 2015 Sci. China Phys. Mech. Astron. 58 1-11
[49]	Lin Y J, Compton R L, Jiménez-García K, Porto J V, Spielman I B 2009 Nature (London) 462 628 Google Scholar
[50]	Lin Y J, Compton R L, Jiménez-García K, Porto J V, Spielman I B 2011 Nat. Phys. 7 531 Google Scholar
[51]	Ruseckas J, Juzeliūnas G, Öhberg P, Fleischhauer M 2005 Phys. Rev. Lett. 95 010404 Google Scholar
[52]	Zhang L, Liu X J 2018 arXiv 1806 05628
[53]	Liu X J, Liu Z X, Cheng M 2013 Phys. Rev. Lett. 110 076401 Google Scholar
[54]	Sun W, Wang B Z, Xu X T, Yi C R, Zhang L, Wu Z, Deng Y, Liu X J, Chen S, Pan J W 2018 Phys. Rev. Lett. 121 150401 Google Scholar
[55]	Baumann K, Guerlin C, Brennecke F, Esslinger T 2010 Nature (London) 464 1301 Google Scholar
[56]	Ritsch H, Domokos P, Brennecke F, Esslinger T 2013 Rev. Mod. Phys. 85 553 Google Scholar
[57]	Dalla Torre E G, Diehl S, Lukin M D, Sachdev S, Strack P 2013 Phys. Rev. A 87 023831 Google Scholar
[58]	Gopalakrishnan S, Lev B L, Goldbart P M 2009 Nat. Phys. 5 845 Google Scholar
[59]	Strack P, Sachdev S 2011 Phys. Rev. Lett. 107 277202 Google Scholar
[60]	Müller M, Strack P, Sachdev S 2012 Phys. Rev. A 86 023604 Google Scholar
[61]	Domokos P, Ritsch H 2002 Phys. Rev. Lett. 89 253003 Google Scholar
[62]	Dimer F, Estienne B, Parkins A S, Carmichael H J 2007 Phys. Rev. A 75 013804 Google Scholar
[63]	Nagy D, Konya G, Szirmai G, Domokos P 2010 Phys. Rev. Lett. 104 130401 Google Scholar
[64]	Keeling J, Bhaseen M J, Simons B D 2014 Phys. Rev. Lett. 112 143002 Google Scholar
[65]	Piazza F, Strack P 2014 Phys. Rev. Lett. 112 143003 Google Scholar
[66]	Chen Y, Yu Z, Zhai H 2014 Phys. Rev. Lett. 112 143004 Google Scholar
[67]	Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045 Google Scholar
[68]	Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057 Google Scholar
[69]	Lin Y J, Jiménez-García K, Spielman I B 2011 Nature 471 83
[70]	Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H, Chai S J, Zhai H, Zhang J 2012 Phys. Rev. Letter 109 095301
[71]	Galitski V, Spielman I B 2013 Nature (London) 494 49 Google Scholar
[72]	Goldman N, Juzeliūnas G, Öberg P, Spielman I B 2014 Rep. Prog. Phys. 77 126401 Google Scholar
[73]	Zhou X, Li Y, Cai Z, Wu C 2013 J. Phys. B 46 134001 Google Scholar
[74]	Deng Y, Cheng J, Jing H, Yi S 2014 Phys. Rev. Lett. 112 143007 Google Scholar
[75]	Dong L, Zhou L, Wu B, Ramachandhran B, Pu H 2014 Phys. Rev. A 89 011602(R)Google Scholar
[76]	Pan J S, Liu X J, Zhang W, Yi W, Guo G C 2015 Phys. Rev. Letter 115 045303
[77]	Liu X J, Law K T, Ng T K 2014 Phys. Rev. Letter 112 086401
[78]	Gu Z C, Wen X G 2009 Phys. Rev. B 80 155131 Google Scholar
[79]	Pollmann F, Berg E, Turner A M, Oshikawa M 2012 Phys. Rev. B 85 075125 Google Scholar
[80]	Wen X G 1989 Phys. Rev. B 40 7387
[81]	Wen X G, Niu Q 1990 Phys. Rev. B 41 9377 Google Scholar
[82]	Wen X G 1990 Int. J. Mod. Phys. B 04 239 Google Scholar
[83]	Haldane F D M 1983 Phys. Rev. Lett. 50 1153 Google Scholar
[84]	Kane C L, Mele E J 2005 Phys. Rev. Lett. 95 146802 Google Scholar
[85]	Bernevig B A, Zhang S C 2006 Phys. Rev. Lett. 96 106802 Google Scholar
[86]	Moore J E, Balents L 2007 Phys. Rev. B 75 121306(R)Google Scholar
[87]	Fu L, Kane C L, Mele E J 2007 Phys. Rev. Lett. 98 106803 Google Scholar
[88]	Qi X L, Hughes T L, Zhang S C 2008 Phys. Rev. B 78 195424 Google Scholar
[89]	Chen X, Gu Z C, Wen X G 2011 Phys. Rev. B 83 035107 Google Scholar
[90]	Chen X, Gu Z C, Liu Z X, Wen X G 2013 Phys. Rev. B 87 155114 Google Scholar
[91]	Kitaev A 2009 AIP Conf. Proc. 1134 22
[92]	Ryu S, Schnyder A, Furusaki A, Ludwig A 2010 New J. Phys. 12 065010 Google Scholar
[93]	Fidkowski L, Kitaev A 2010 Phys. Rev. B 81 134509 Google Scholar
[94]	Gu Z C, Wen X G 2014 Phys. Rev. B 90 115141 Google Scholar
[95]	Wang C, Potter A C, Senthil T 2014 Science 343 629 Google Scholar
[96]	Wu H Q, He Y Y, You Y Z, Yoshida T, Kawakami N, Xu C, Meng Z Y, Lu Z Y 2016 Phys. Rev. B 94 165121 Google Scholar
[97]	Takamoto M, Hong F L, Higashi R, Katori H 2005 Nature (London) 435 321 Google Scholar
[98]	Ludlow A D, Boyd M M, Zelevinsky T, Foreman S M, Blatt S, Notcutt M, Ido T, Ye J 2006 Phys. Rev. Lett. 96 033003 Google Scholar
[99]	Swallows M D, Bishof M, Lin Y, Blatt S, Martin M J, Rey A M, Ye J 2011 Science 331 1043 Google Scholar
[100]	Bloom B J, Nicholson T L, Williams J R, Campbell S L, Bishof M, Zhang X, Zhang W, Bromley S L, Ye J 2014 Nature (London) 506 71 Google Scholar
[101]	Cazalilla M A, Rey A M 2014 Rep. Prog. Phys. 77 124401 Google Scholar
[102]	Gorshkov A V, Rey A M, Daley A J, Boyd M M, Ye J, Zoller P, Lukin M D 2009 Phys. Rev. Lett. 102 110503 Google Scholar
[103]	Wu C, Hu J P, Zhang S C 2003 Phys. Rev. Lett. 91 186402 Google Scholar
[104]	Fukuhara T, Takasu Y, Kumakura M, Takahashi Y 2007 Phys. Rev. Lett. 98 030401 Google Scholar
[105]	Cazalilla M A, Ho A F, Ueda M 2009 New J. Phys. 11 103033 Google Scholar
[106]	Stellmer S, Tey M K, Huang B, Grimm R, Schreck F 2009 Phys. Rev. Lett. 103 200401 Google Scholar
[107]	DeSalvo B J, Yan M, Mickelson P G, Martinez de Escobar Y N, Killian T C 2010 Phys. Rev. Lett. 105 030402 Google Scholar
[108]	Gorshkov A V, Hermele M, Gurarie V, Xu C, Julienne P S, Ye J, Zoller P, Demler E, Lukin M D, Rey A M 2010 Nat. Phys. 6 289 Google Scholar
[109]	Kobayashi K, Okumura M, Ota Y, Yamada S, Machida M 2012 Phys. Rev. Lett. 109 235302 Google Scholar
[110]	Nonne H, Moliner M, Capponi S, Lecheminant P, Totsuka K 2013 Europhys. Lett. 102 37008 Google Scholar
[111]	Duivenvoorden K, Quella T 2013 Phys. Rev. B 87 125145
[112]	Zhang X, Bishof M, Bromley S L, Kraus C V, Safronova M S, Zoller P, Rey A M, Ye J 2014 Science 345 1467 Google Scholar
[113]	Scazza F, Hofrichter C, Höfer M, De Groot P C, Bloch I, Fölling S 2014 Nat. Phys. 10 779 Google Scholar
[114]	Cappellini G, Mancini M, Pagano G, Lombardi P, Livi L, Siciliani de Cumis M, Cancio P, Pizzocaro M, Calonico D, Levi F, Sias C, Catani J, Inguscio M, Fallani L 2014 Phys. Rev. Lett. 113 120402 Google Scholar
[115]	Mancini M, Pagano G, Cappellini G, Livi L, Rider M, Catani J, Sias C, Zoller P, Inguscio M, Dalmonte M, Fallani L 2015 Science 349 1510 Google Scholar
[116]	Bois V, Capponi S, Lecheminant P, Moliner M, Totsuka K 2015 Phys. Rev. B 91 075121
[117]	Roy A, Quella T arXiv: 1512.05229
[118]	Hofrichter C, Riegger L, Scazza F, Höfer M, Fernandes D R, Bloch I, Fölling S 2016 Phys. Rev. X 6 021030
[119]	Bois V, Fromholz P, Lecheminant P 2016 Phys. Rev. B 93 134415 Google Scholar
[120]	Capponi S, Lecheminant P, Totsuka K 2016 Ann. Phys. (Amsterdam) 367 50 Google Scholar
[121]	Wall M L, Koller A P, Li S, Zhang X, Cooper N R, Ye J, Rey A M 2016 Phys. Rev. Lett. 116 035301 Google Scholar
[122]	Kolkowitz S, Bromley S L, Bothwell T, Wall M L, Marti G E, Koller A P, Zhang X, Rey A M, Ye J 2017 Nature (London) 542 66 Google Scholar
[123]	Livi L F, Cappellini G, Diem M, Franchi L, Clivati C, Frittelli M, Levi F, Calonico D, Catani J, Inguscio M, Fallani L 2016 Phys. Rev. Lett. 117 220401 Google Scholar
[124]	Song B, He C, Zhang S, Hajiyev E, Huang W, Liu X J, Jo G B 2016 Phys. Rev. A 94 061604 Google Scholar
[125]	Zhang R, Cheng Y, Zhai H, Zhang Z 2015 Phys. Rev. Lett. 115 135301 Google Scholar
[126]	Pagano G, Mancini M, Cappellini G, Livi L, Sias C, Catani J, Inguscio M, Fallani L 2015 Phys. Rev. Lett. 115 265301 Google Scholar
[127]	Höfer M, Riegger L, Scazza F, Hofrichter C, Fernandes D R, Parish M M, Levinsen J, Bloch I, Fölling S 2015 Phys. Rev. Lett. 115 265302 Google Scholar
[128]	Zhou X F, Pan J S, Liu Z X, Zhang W, Yi W, Chen G, Jia S T 2017 Phys. Rev. Letter 119 185701
[129]	Dzuba V A, Derevianko A 2010 J. Phys. B 43 074011 Google Scholar
[130]	Porsev S G, Derevianko A, Fortson E N 2004 Phys. Rev. A 69 021403 Google Scholar
[131]	Zhou L, Cui X 2015 Phys. Rev. B 92 140502(R)Google Scholar
[132]	Yu D, Pan J S, Liu X J, Zhang W, Yi W 2017 Front. Phys. 13 136701
[133]	Pan J S, Zhang W, Yi W, Guo G C 2016 Phys. Rev. A 94 043619 Google Scholar
[134]	Zhang R, Zhang D, Cheng Y, Chen W, Zhang P, Zhai H 2016 Phys. Rev. A 93 043601 Google Scholar
[135]	Liu X J, Liu Z X, Cheng M 2013 Phys. Rev. Letter 110 076401
[136]	Tang E, Wen X G 2012 Phys. Rev. Lett. 109 096403 Google Scholar
[137]	Morimoto T, Furusaki A, Mudry C 2015 Phys. Rev. B 92 125104 Google Scholar
[138]	Zhao J Z, Hu S J, Zhang P 2015 Phys. Rev. Lett. 115 195302 Google Scholar
[139]	Yoshida T, Peters R, Fujimoto S, Kawakami N 2014 Phys. Rev. Lett. 112 196404 Google Scholar
[140]	Pollmann F, Turner A M, Berg E, Oshikawa M 2010 Phys. Rev. B 81 064439 Google Scholar
[141]	Turner A M, Pollmann F, Berg E 2011 Phys. Rev. B 83 075102 Google Scholar
[142]	Fidkowski L 2010 Phys. Rev. Lett. 104 130502 Google Scholar
[143]	Flammia S T, Hamma A, Hughes T L, Wen X G 2009 Phys. Rev. Lett. 103 261601 Google Scholar
[144]	Li H, Haldane F D M 2008 Phys. Rev. Lett. 101 010504 Google Scholar
[145]	Hastings M B, González I, Kallin A B, Melko R G 2010 Phys. Rev. Lett. 104 157201 Google Scholar
[146]	Daley A J, Pichler H, Schachenmayer J, Zoller P 2012 Phys. Rev. Lett. 109 020505 Google Scholar
[147]	Abanin D A, Demler E 2012 Phys. Rev. Lett. 109 020504 Google Scholar
[148]	Jiang H C, Wang Z H, Balents L 2012 Nat. Phys. 8 902 Google Scholar
[149]	Islam R, Ma R, Preiss P M, Tai M E, Lukin A, Rispoli M, Greiner M 2015 Nature (London) 528 77 Google Scholar
[150]	Calabrese P, Cardy J 2004 J. Stat. Mech: Theory Exp. 06 P06002
[151]	Nielsen A E B, Sierra G, Cirac J I 2011 Phys. Rev. A 83 053807 Google Scholar
[152]	Wang C, Gao C, Jian C M, Zhai H 2010 Phys. Rev. Lett. 105 160403 Google Scholar
[153]	Barnett R, Boyd G R, Galitski V 2012 Phys. Rev. Lett. 109 235308 Google Scholar
[154]	Kawaguchi Y, Ueda M 2012 Phys. Rep. 520 253 Google Scholar
[155]	Isoshima T, Machida K, Ohmi T 2001 J. Phys. Soc. Jpn. 70 1604 Google Scholar
[156]	Mizushima T, Kobayashi N, Machida K 2004 Phys. Rev. A 70 043613 Google Scholar
[157]	Stamper-Kurn D M, Ueda M 2013 Rev. Mod. Phys. 85 1191 Google Scholar
[158]	Wang D S, Shi Y R, Chow K W, Yu Z X, Li X G 2013 Eur. Phys. J. D 67 242 Google Scholar
[159]	Wang D S, Ma Y Q, Li X G 2014 Commun. Nonlinear Sci. Numer. Simul. 19 3556 Google Scholar
[160]	Xu Z F, Kawaguchi Y, You L, Ueda M 2012 Phys. Rev. A 86 033628 Google Scholar
[161]	Lan Z, Ohberg P 2014 Phys. Rev. A 89 023630 Google Scholar
[162]	Han W, Zhang X F, Song S W, Saito H, Zhang W, Liu W M, Zhang S C 2016 Physics Review A 94 033629
[163]	Zhang Y, Mao L, Zhang C 2012 Phys. Rev. Lett. 108 035302 Google Scholar
[164]	Li Y, Pitaevskii L P, Stringari S 2012 Phys. Rev. Lett. 108 225301 Google Scholar
[165]	Saito H, Kawaguchi Y, Ueda M 2006 Phys. Rev. Lett. 96 065302 Google Scholar
[166]	Saito H, Kawaguchi Y, Ueda M 2007 Phys. Rev. A 75 013621 Google Scholar
[167]	Kawaguchi Y, Saito H, Kudo K, Ueda M 2010 Phys. Rev. A 82 043627 Google Scholar
[168]	Lovegrove J, Borgh M O, Ruostekoski J 2012 Phys. Rev. A 86 013613 Google Scholar
[169]	Su S W, Liu I K, Tsai Y C, Liu W M, Gou S C 2012 Phys. Rev. A 86 023601 Google Scholar
[170]	Shinjo T, Okuno T, Hassdorf R, Shigeto K, Ono T 2000 Science 289 930 Google Scholar
[171]	Wachowiak A, Wiebe J, Bode M, Pietzsch O, Morgenstern M, Wiesendanger R 2002 Science 298 577 Google Scholar
[172]	Yi S, Pu H 2006 Phys. Rev. Lett. 97 020401 Google Scholar
[173]	Leslie L S, Hansen A, Wright K C, Deutsch B M, Bigelow N P 2009 Phys. Rev. Lett. 103 250401 Google Scholar
[174]	Sadler L E, Higbie J M, Leslie S R, Vengalattore M, Stamper-Kurn D M 2006 Nature (London) 443 312 Google Scholar
[175]	Savard T A, Granade S R, O'Hara K M, Gehm M E, Thomas J E 1999 Phys. Rev. A 60 4788 Google Scholar
[176]	McGuire B A, Carroll P B, Loomis R A, Finneran I A, Jewell P R, Remijan A J, Blake G A 2016 Science 352 1449 Google Scholar
[177]	Yoon M, Srirambalaji R, Kim K 2012 Chem. Rev. 112 1196 Google Scholar
[178]	Kallin C, Berlinsky J 2016 Rep. Prog. Phys. 79 054502 Google Scholar
[179]	Weng H, Fang C, Fang Z, Bernevig B A, Dai X 2015 Phys. Rev. X 5 011029
[180]	Ryu K S, Thomas L, Yang S H, Parkin S 2013 Nat. Nanotechnol. 8 527 Google Scholar
[181]	Emori S, Bauer U, Ahn S M, Martinez E, Beach G S D 2013 Nat. Mater. 12 611 Google Scholar
[182]	Chen G, Ma T, N'Diaye A T, Kwon H, Won C, Wu Y, Schmid A K 2013 Nat. Commun. 4 2671
[183]	Shibata K, Yu X Z, Hara T, Morikawa D, Kanazawa N, Kimoto K, Ishiwata S, Matsui Y, Tokura Y 2013 Nat. Nanotechnol. 8 723 Google Scholar
[184]	Zhai H 2012 Int. J. Mod. Phys. B 26 1230001 Google Scholar
[185]	Wu C 2009 Mod. Phys. Lett. B 23 1
[186]	Wilson R M, Anderson B M, Clark C W 2013 Phys Rev. Lett. 111 185303 Google Scholar
[187]	Gopalakrishnan S, Martin I, Demler E A 2013 Phys. Rev. Lett. 111 185304 Google Scholar
[188]	Henkel N, Nath R, Pohl T 2010 Phys. Rev. Lett. 104 195302 Google Scholar
[189]	Hsueh C H, Tsai Y C, Wu K S, Chang M S, Wu W C 2013 Phys. Rev. A 88 043646 Google Scholar
[190]	Heidemann R, Raitzsch U, Bendkowsky V, Butscher B, Löw R, Pfau T 2008 Phys. Rev. Lett. 100 033601 Google Scholar
[191]	Boninsegni M, Prokof'ev N V 2012 Rev. Mod. Phys. 84 759 Google Scholar
[192]	Boninsegni M 2012 J. Low Temp. Phys. 168 137 Google Scholar
[193]	Balibar S 2010 Nature (London) 464 176 Google Scholar
[194]	Andreev A F, Lifshitz I M 1969 Zh. Eksp. Teor. Fiz. 56 2057
[195]	Chester G V 1970 Phys. Rev. A 2 256 Google Scholar
[196]	Leggett A J 1970 Phys. Rev. Lett. 25 1543 Google Scholar
[197]	Kim E, Chan M H W 2004 Nature (London) 427 225 Google Scholar
[198]	Luo X, Wu L, Chen J, Guan Q, Gao K, Xu Z F, You L, Wang R 2016 Sci. Rep. 6 18983 Google Scholar
[199]	Yefsah T, Desbuquois R, Chomaz L, Günter K J, Dalibard J 2011 Phys. Rev. Lett. 107 130401 Google Scholar
[200]	Han W, Zhang X F, Wang D S, Jiang H F, Zhang W, Zhang S G 2018 Phys. Rev. Letter 121 030404
[201]	Ruokokoski E, Huhtamäki, MöttÖnen M 2012 Phys. Rev. A 86 051607(R)Google Scholar
[202]	Xu Z F, Kobayashi S, Ueda M 2013 Phys. Rev. A 88 013621 Google Scholar
[203]	Su S W, Gou S C, Sun Q, Wen L, Liu W M, Ji A C, Ruseckas J, Juzeliūnas G 2016 Phys. Rev. A 93 053630 Google Scholar
[204]	Nagaosa N, Tokura Y 2013 Nat. Nanotechnol. 8 899 Google Scholar
[205]	Mühlbauer S, Binz B, Jonietz F, Pfleiderer C, Rosch A, Neubauer A, Georgii R, Böni P 2009 Science 323 915 Google Scholar
[206]	Yu X Z, Onose Y, Kanazawa N, Park J H, Han J H, Matsui Y, Nagaosa N, Tokura Y 2010 Nature (London) 465 901 Google Scholar
[207]	Seki S, Yu X Z, Ishiwata S, Tokura Y 2012 Science 336 198 Google Scholar
[208]	Ozawa T, Baym G 2012 Phys. Rev. A 85 063623 Google Scholar
[209]	Fetter A L 2014 Phys. Rev. A 89 023629 Google Scholar
[210]	Madison K W, Chevy F, Wohlleben W, Dalibard J 2000 Phys. Rev. Lett. 84 806 Google Scholar
[211]	Abo-Shaeer J R, Raman C, Vogels J M, Ketterle W 2001 Science 292 476 Google Scholar
[212]	Li J R, Lee J, Huang W, Burchesky S, Shteynas B, Top F C, Jamison A O, Ketterle W 2017 Nature (London) 543 91 Google Scholar
[213]	Ho T L, Zhang S 2011 Phys. Rev. Lett. 107 150403 Google Scholar
[214]	Ji S C, Zhang J Y, Zhang L, Du Z D, Zheng W, Deng Y J, Zhai H, Chen S, Pan J W 2014 Nat. Phys. 10 314 Google Scholar
[215]	Hall D S, Matthews M R, Ensher J R, Wieman C E, Cornell E A 1998 Phys. Rev. Lett. 81 1539 Google Scholar

Cited By

图 1 (a) 一维自旋轨道耦合的实验实现方案； (b) 利用tripod方案实现二维人造规范势的方案；(c) 利用Raman晶格实现二维人造自旋轨道耦合的实验方案

Figure 1. (a) The NIST scheme to realize 1D spin-orbit coupling; (b) the tripod scheme to realize 2D synthetic gauge field; (c) the Raman optical lattice scheme to realize 2D spin-orbit coupling

DownLoad: Full-Size Img PowerPoint

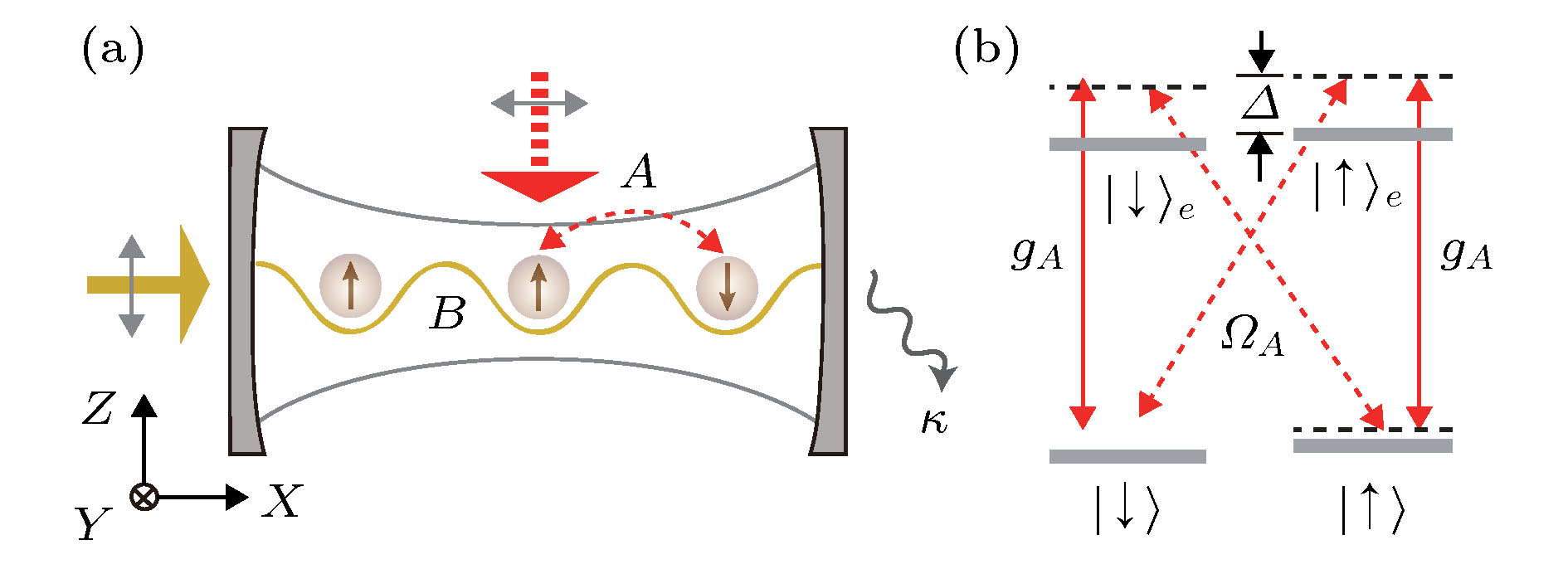
图 2 (a)准一维费米气体与双模光腔耦合的示意图. 在腔轴向(沿$ \hat{x} $轴)和径向(沿$ -\hat{z} $轴)均有泵浦光; (b)原子能级和光耦合的示意图^[76]

Figure 2. (a) A quasi-one-dimensional Fermi gas, which is coupled to a two-mode optical cavity, is under both transverse (along $ -\hat{z} $) and longitudinal (along $ \hat{x} $) pumping; (b) the level scheme of atom^[76]

DownLoad: Full-Size Img PowerPoint

图 3 开边界条件下准一维晶格中TSR态的一些特征　(a) 腔场强度$ |\alpha| $随有效泵浦$ \eta_ {\rm A} $的变化; (b) 序参量$ \theta(x) $在TSR相变前(点线表示, $ \eta_ {\rm A} = 1 E_{\rm r} $)和相变后(实线和点划线表示, $ \eta_ {\rm A} = 3 E_{\rm r} $)在中心六个格点中的变化情况. 临界点位于$ \eta^ {\rm c}_ {\rm A}\sim 2.05 E_{\rm r} $处. 在TSR相中, 由于自发对称性破缺, 腔场$ \alpha $可以取正值或负值, 对应序参量由实线或点划线表示; (c) 当系统穿过相边界进入TSR态后, 系统会由于超辐射相变打开一个体能隙, 同时出现一对零能的边缘态 (d) 当$ \eta_ {\rm A} = 3 E_{\rm r} $时, 图(c)中的边缘态所对应的实空间波函数. 本图中考虑一个拥有80个格点的半满晶格体系, 体系参数选取为: $ k_ {\rm B}T = E_{\rm r}/200 $, $ m_z = 0 $, $ V_0 = 5 E_{\rm r} $, $ \kappa = 100 E_{\rm r} $, $ \varDelta_ {\rm A} = -10 E_{\rm r} $, $ \xi_ {\rm A} = 5 E_{\rm r} $. 对于$ ^6 {\rm {Li}} $原子, 通过选取$ \kappa\approx7.4 $MHz, $ g_ {\rm A}\approx 27.1 $MHz, $ |\varDelta|\approx 0.74 $MHz, $ \varDelta\approx2 $GHz和$ T\approx17.7 $nK可以满足上述参数条件^[76]

Figure 3. TSR state in a quasi-one-dimensional lattice with open boundary conditions: (a) The cavity field $ |\alpha| $ varies with $ \eta_ {\rm A} $ across the TSR transition; (b) $ \theta(x) $ on the central six sites. The dotted curve corresponds to the $ \theta(x) $ before the TSR phase transition, where $ \eta_ {\rm A} = 1 E_{\rm r} $. The solid and dash-dotted curves to the $ \theta(x) $ after the TSR phase transition, where $ \eta_ {\rm A} = 3 E_{\rm r} $. The transition point is around $ \eta^ {\rm c}_ {\rm A}\approx 2.05 E_{\rm r} $. Because of the spontaneous symmetry breaking, the cavity field of the TSR phase acquires a positive (negative) real part, corresponding to solid (dash-dotted) curve; (c) when the system crosses the phase boundary, a pair of edge states emerge in the superradiance-induced bulk gap. (d) the wave functions of the edge states in (c) with $ \eta_ {\rm A} = 3 E_{\rm r} $. In our calculation, we consider a half-filled lattice of 80 sites, with the parameters $ k_ {\rm B}T = E_{\rm r}/200 $, $ m_z = 0 $, $ V_0 = 5 E_{\rm r} $, $ \kappa = 100 E_{\rm r} $, $ \varDelta_ {\rm A} = -10 E_{\rm r }$, and $ \xi_ {\rm A} = 5 E_{\rm r} $. For $ ^6 {\rm {Li}} $ atoms, these parameters can be satisfied by choosing $ \kappa\approx7.4 $ MHz, $ g_ {\rm A}\approx 27.1 $ MHz, $ |\varDelta|\approx0.74 $ MHz, $ \varDelta\approx2 $ GHz, and $ T\approx17.7 $ nK^[76]

DownLoad: Full-Size Img PowerPoint

图 4 有限温度$ k_{\rm B}T = E_{\rm r}/200 $时系统的稳态相图. 图中实线为TSR相边界, 点线为TSR态和普通SR态间的拓扑相边界. 在$ m_{\rm c}\approx 0.132 E_{\rm r} $处的细虚线为金属态(M)和绝缘态(I)间的边界. 点划线为普通SR相与绝缘相的边界. 不同的相边界汇聚于$ \eta_{\rm A}\approx 2.614 E_{\rm r} $, $ m_{\rm c}\approx0.132 E_{\rm r} $处(如图中四相点所示). 图中其他参数与图(2)一致. 内嵌图展示了与大图中箭头对应的相变前后体能隙的变化. 图中实线为相变前的能带, 虚线为相变后的能带, 点线为相边界上的情况^[76]

Figure 4. The phase diagram of steady-state with $\small k_{\rm B}T $$ = E_{\rm r}/200 $. The solid curve corresponds to the TSR phase boundary, and the topological phase boundary between the TSR and the trivial SR states corresponds to dotted curve. The thin dashed curve at $ m_{\rm c}\approx0.132 E_{\rm r} $ is the boundary between the M and the I states, and the dash-dotted curve is the conventional SR phase boundary. At the tetracritical point (dot) with $ \eta_{\rm A}\approx2.614 E_{\rm r} $ and $ m_{\rm c}\approx0.132 E_{\rm r} $, the various boundaries merge. Other parameters are the same as those used in Fig. 2. Inset: change of bulk gap before (solid), after (dashed), and right (dotted) at the phase boundaries labeled by arrows^[76]

DownLoad: Full-Size Img PowerPoint

图 5 (a) 处在拉曼光中的准一维超冷原子气体; (b) 通过拉曼过程耦合的原子能级示意图. 图中绿色曲线指示了不同轨道态之间的自旋交换相互作用. 通过利用与自旋相关的激光频移, 可以将图中的四个核自旋态与其他核自旋态分离开来进行操控^[128]

Figure 5. A quasi-1D atomic gas under Raman lasers; (b) Raman level schemes in the clock-states manifold. The green curve corresponds to the interorbital spin-exchange interaction. By using spin-dependent laser shifts, the four nuclear spin states from $ ^1 S_0 $ and $ ^3 P_0 $ manifolds can be separated from the other nuclear spins^[128]

DownLoad: Full-Size Img PowerPoint

图 6 (a) 本征值最小的四个纠缠谱$ \xi_i(i = 1, 2, 3, 4) $随自旋交换相互作用的变化; (b) 开边界条件下, 在格点数$ N = 60 $的光晶格链中, 二阶Rényi熵$ S_2 $和von Neumann熵$ S_{\rm{vN}} $随$ V_{\rm{ex}}/t_s $的变化情况; (c) 周期边界条件下, 在格点数$ N = 12 $的光晶格链中, 体能隙$ E_{\rm {gap}} $的变化情况. 内嵌图为体能隙在临界点处随$ 1/N $的变化情况. 图中线性拟合的红色实线给出大$ N $极限下$ E_{\rm {gap}}/t_{\rm s}\approx-0.02\pm0.05 $; (d) 临界点$ V_{\rm{ex}}/t_s = 1.694 $处, 长度为$ j $且格点数$ N = 120 $的子链中von Neumann熵随$ \sin({\text{π}} l/N) $的变化. 通过线性拟合$ S_{\rm{vN}} = (C/6)\ln[\sin({\text{π}} l/N)]+1.87 $, 可以得到中心荷(central charge)$ C = 1.018 $. 图中所有计算均在半满状态下进行, 且固定参数$ \varGamma^{g/e}_z = 0 $, $ U = 0 $, $ t_{\rm{so}}/t_{\rm s} = 0.4 $^[128]

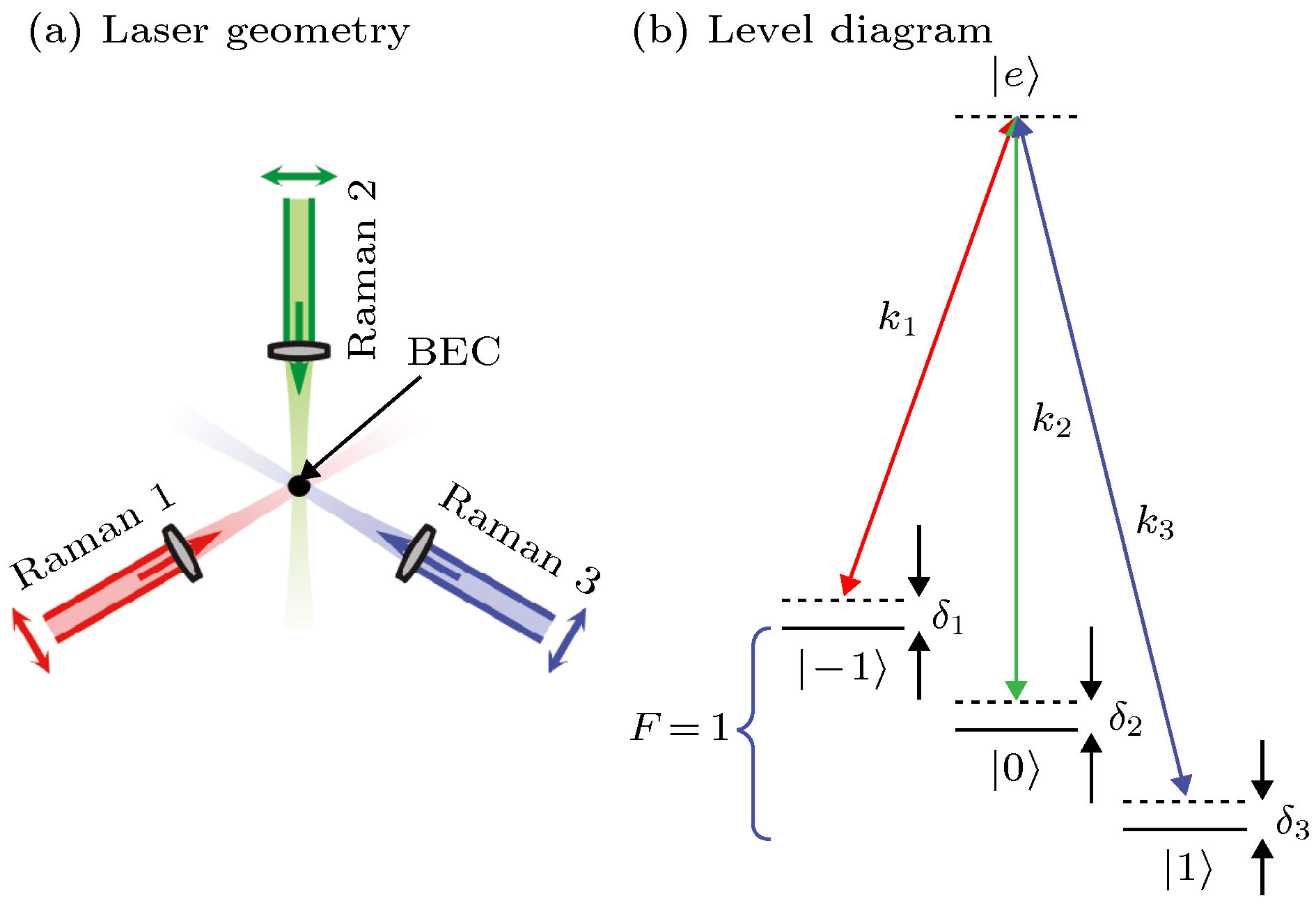
Figure 6. (a) The entanglement spectrum $ \xi_i(i = 1, 2, 3, 4) $; (b) in a chain with $ N = 60 $ lattice sites and under open boundary conditions, the second-order Rényi entropy $ S_2 $ and the von Neumann entropy $ S_{\rm{vN}} $ vary with $ V_{\rm{ex}}/t_{\rm s} $; (c) in a chain with $ N = 12 $ lattice sites and under the periodic boundary condition, the bulk energy gap $ E_{\rm {gap}} $ varies with $ V_{\rm{ex}}/t_{\rm s} $. Inset: The bulk gap as a function of $ 1/N $ at the critical point, and the red solid line is a linear fit with $ E_{\rm {gap}}/t_{\rm s}\approx-0.02\pm0.05 $ in the large-N limit. (d) in a chain with $ N = 120 $ lattice sites and at the critical point $ V_{\rm{ex}}/t_s = 1.694 $, the von Neumann entropy of a subchain of length $ l $ varied with $ \sin({\text{π}} l/N) $. The solid line is the linear fit with $ S_{\rm{vN}} = (C/6)\ln[\sin({\text{π}} l/N)]+1.87 $ and $ C = 1.018 $. The central charge is 6 times the slope of the linear fit. All calculations are performed at half filling and with the fixed parameters $ \varGamma^{g/2}_z = 0 $, $ U = 0 $, and $ t_{\rm{so}}/t_{\rm s} = 0.4 $^[128]

DownLoad: Full-Size Img PowerPoint

图 7 格点数$ N = 60 $且处于半满状态下的体系相图, 其中$ \varGamma^{\alpha}_z = 0 $^[128]

Figure 7. The phase diagram of a lattice with $ N = 60 $ sites at half filling with $ \varGamma^{\alpha}_z = 0 $^[128]

DownLoad: Full-Size Img PowerPoint

图 8 旋量BEC中产生SU(3)自旋轨道耦合的原理图　(a) 激光作用. 三束有不同频率和偏振的激光, 以$ 2{\text{π}}/3 $的角度作用于原子气体; (b) 能级图. 三个拉曼过程分别缀饰⁸⁷Rb中饰⁸⁷Rb中$ 5 {\rm S}_{1/2}, F = 1 $基态的超精细塞曼能级$|F = 1 $, $ m_{\rm F} = 1\rangle $, $ |F = 1, m_{\rm F} = 0\rangle $和$ |F = 1, m_{\rm F} = -1\rangle $. $ \delta_1 $, $ \delta_2 $和$ \delta_3 $与拉曼过程的失谐对应^[162]

Figure 8. Scheme for creating SU(3) spin-orbit coupling in spinor BECs: (a) Laser geometry. The cloud of atoms is illuminated by three laser beams with different frequencies and polarizations, intersecting at an angle of $ 2{\text{π}}/3 $ (b) Each of the three Raman lasers dresses one hyperfine Zeeman level from eman level from $ |F = 1, m_{\rm F} = 1\rangle $, $ |F \!=\! 1, m_{\rm F} \!=\! 0\rangle $ and $ |F \!=\! 1, m_{\rm F} \!=\! -1\rangle $ of the ⁸⁷Rb ${\rm 5 S}_{1/2}, F = 1 $. $ \delta_1, \delta_2 $, and $ \delta_3 $ are the detuning in the Raman transitions^[162]

DownLoad: Full-Size Img PowerPoint

图 9 有SU(3)自旋轨道耦合的BEC中的两种不同相　(a)−(d) 存在反铁磁自旋相互作用时($ c_2 > 0 $)的拓扑非平庸晶格相. 图(a)中的高度和颜色分别代表$ \varPsi_1 $的密度和相位. 在图(b)中, 一个单胞中呈现出涡旋(白色圆圈)和反涡旋(黑色圆圈)的位置. 图(c)和图(d)分别展示了晶格相的动量分布和相分离结构的示意图; (e), (f) 存在铁磁自旋相互作用时($ c_2 < 0 $)的三重简并磁化相. 图(e)和图(f)分别展示了$ \varPsi_1 $在实空间和动量空间的分布^[162]

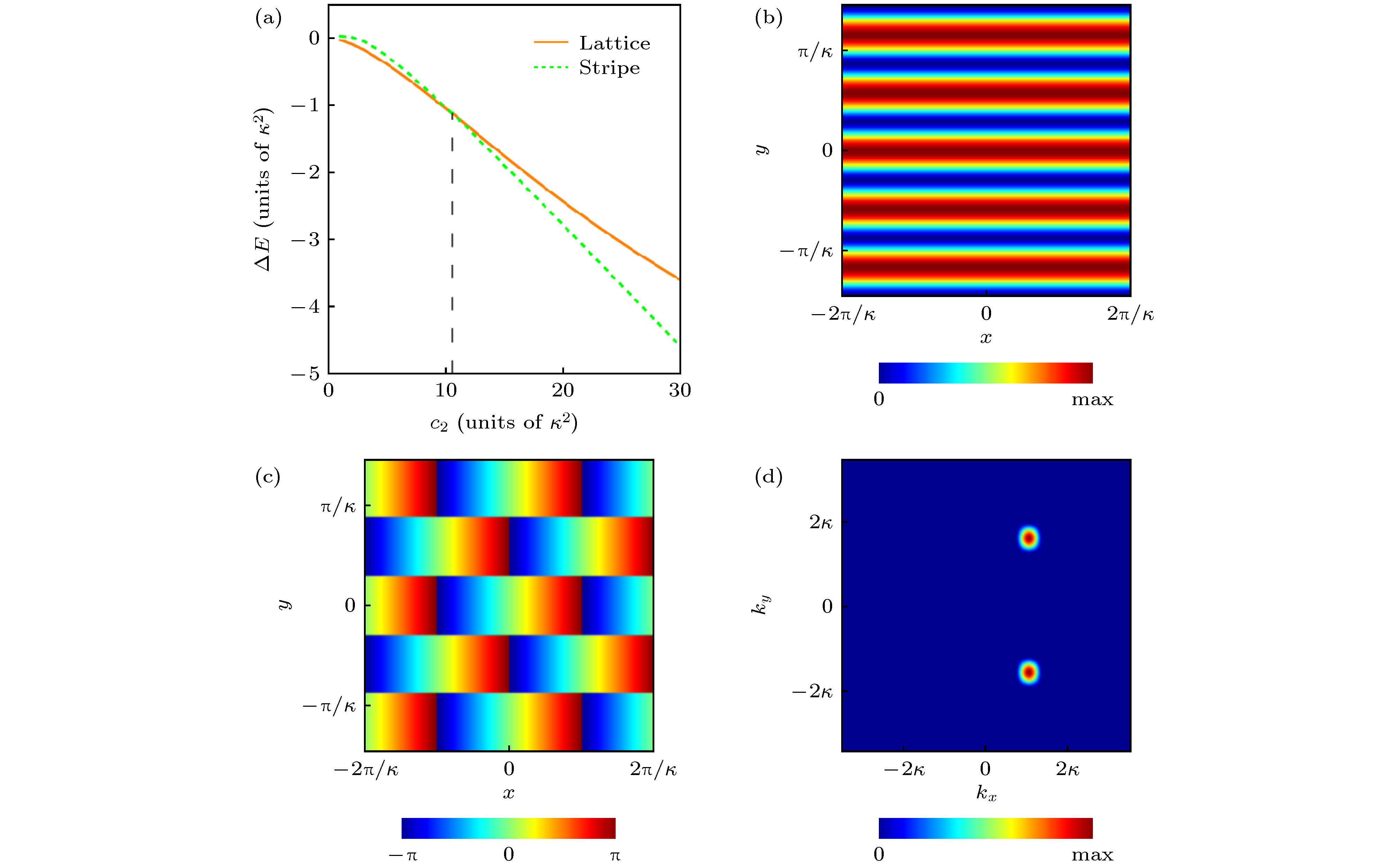
Figure 9. Two distinct phases of SU(3) spin-orbit-coupled BECS: (a)−(d) The topologically nontrivial lattice phase with antiferromagnetic spin interaction ($ c_2 > 0 $). (a) The heights and colors correspond to the density and phase of $ \varPsi_1 $ respectively, (b) the positions of vortices (white circles) and antivortices (black circles) in the phase within one unit cell, (c) the corresponding momentum distributions, (d) the structural schematic drawing of the phase separation; (e), (f) the threefold-degenerate magnetized phase for ferromagnetic spin interaction ($ c_2 < 0 $). (e) the density and phase distributions of $ \varPsi_1 $, (f) the corresponding momentum distributions^[162]

DownLoad: Full-Size Img PowerPoint

图 10 (a) 晶格相和条纹相的能量对比; (b)−(d) 参数$ c_2 = 20\kappa^2 $和$ c_0 = 10 c_2 $时, 条纹相基态的密度、相位和动量的分布^[162]

Figure 10. (a) Energy comparison between the lattice and stripe phases. The solid (lattice state) and dashed (stripe state) lines correspond to the energy difference $ \Delta E $ between the numerical simulation and the variational calculation; (b)−(d) the ground-state density, phase and momentum distributions of the stripe phase with the parameters $ c_2 = 20\kappa^2 $ and $ c_0 = 10 c_2 $^[162]

DownLoad: Full-Size Img PowerPoint

图 11 有SU(3)自旋轨道耦合的反铁磁旋量BEC中的涡旋结构. 图中描绘了三个自旋分量中的涡旋排列. 三种类型的涡旋包括: 一个不同自旋分量的环绕数组合为$ \langle -1, 0, 1 \rangle $的极化核心涡旋(蓝线所示), 两个环绕数组合分别为$ \langle 1, -1, 0 \rangle $(绿线所示)和$ \langle 0, 1, -1 \rangle $ (红线所示)的铁磁核心涡旋^[162]

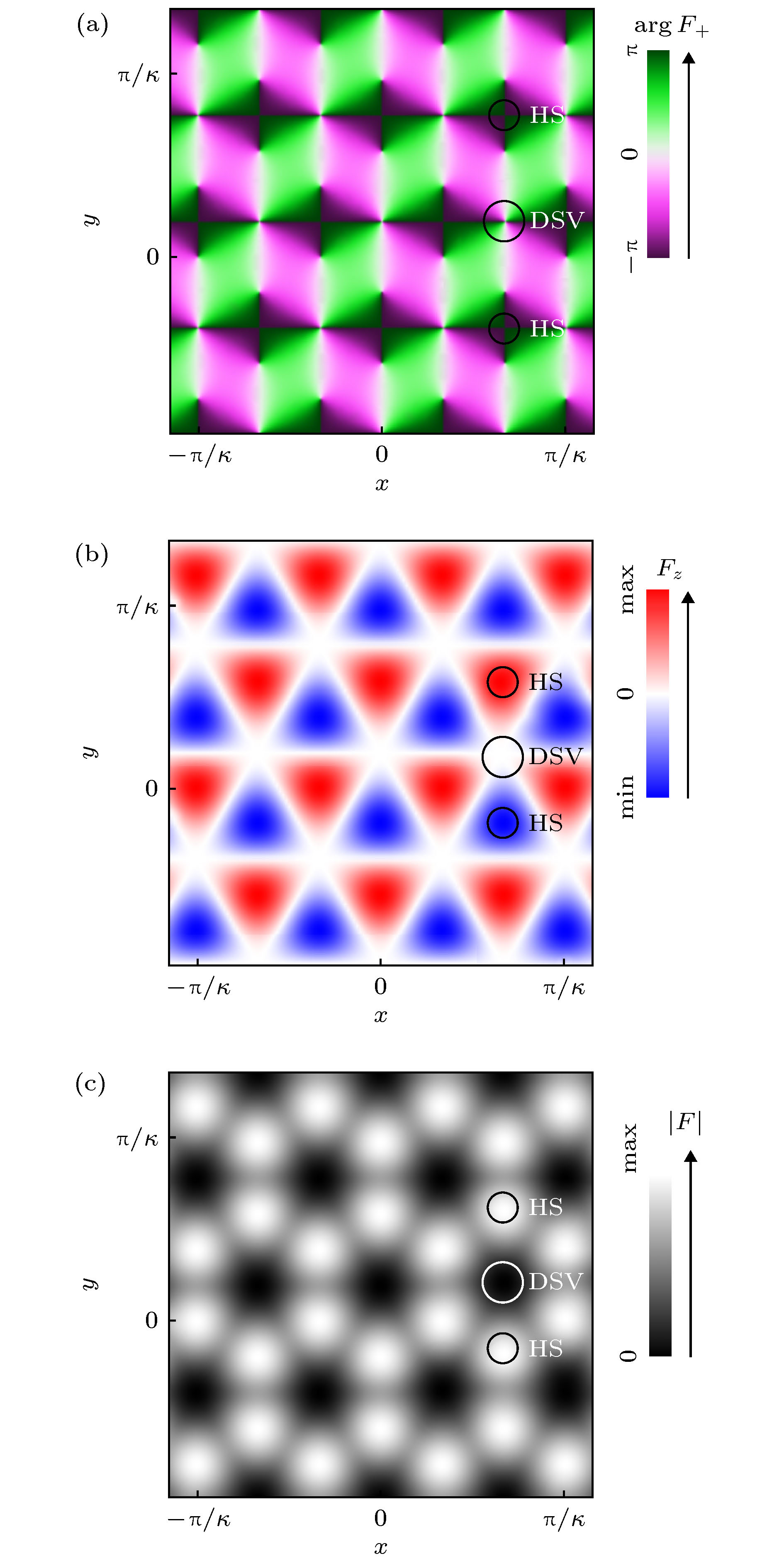
Figure 11. Vortex arrangement among the three components in antiferromagnetic spinor BECs with SU(3) spin-orbit coupling. There are three types of vortices, including a polar-core vortex with winding combination $ \langle -1, 0, 1 \rangle $ (blue line) and two ferromagnetic-core vortices with winding number combinations $ \langle 1, -1, 0 \rangle $ (green line) and $ \langle 0, 1, -1 \rangle $ (red line)^[162]

DownLoad: Full-Size Img PowerPoint

图 12 有SU(3)自旋轨道耦合的反铁磁自旋BEC中的自旋双涡旋 (a)横向磁化的空间分布, 其中颜色表示磁化方向; (b), (c)分别描绘了纵向磁化和总磁化幅度$ |{{F}}| $的分布. 图中分别用大圆圈和小圆圈标记了自旋双涡旋和half-Skyrmion这两种类型的拓扑缺陷^[162]

Figure 12. The double-quantum spin vortex in antiferromagnetic spinor BECs with SU(3) spin-orbit coupling: (a) Spatial maps of the transverse magnetization. The colors correspond to the magnetization orientation; (b) longitudinal magnetization; (c) amplitude of the total magnetization $ |{{F}}| $. The big and small circles represents the two kinds of topological defects: double-quantum spin vortex and half-Skyrmion.^[162]

DownLoad: Full-Size Img PowerPoint

图 13 由Rashba类型SOC和软核长程相互作用诱导产生的手性超固体. 图中的亮度和颜色分别表示密度和相位分布. 软核长程相互作用在图(a)和图(b)中为$ \tilde{C}_6^{(\uparrow\uparrow)}N = 2\tilde{C}_6^{(\downarrow\downarrow)}N = 2500\hbar^2 R_{\rm c}^4/M $, 在图(c)和图(d)中为$ \tilde{C}_6^{(\downarrow\downarrow)}N = 2\tilde{C}_6^{(\uparrow\uparrow)}N = 2500\hbar^2 R_{\rm c}^4/M $. 色标圆盘中的箭头方向表示相应物理量增加的方向. 其他固定参数分别为$ \tilde{C}_6^{(\uparrow\downarrow)}N = 1250\hbar^2 R_{\rm c}^4/M $, $ \kappa = 4\hbar/MR_{\rm c} $和$ gN = 1000\hbar^2/M $. 这里使用的软核长程相互作用强度$ \tilde{C}_6^{(ij)} $在实验中可以实现^[200]

Figure 13. Chiral supersolid induced by Rashba spin-orbit coupling and soft-core long-range interactions. The brightness and color represent the density and phase distributions respectively. The soft-core long-range interactions in (a) and (b) is $ \tilde{C}_6^{(\uparrow\uparrow)}N = 2\tilde{C}_6^{(\downarrow\downarrow)}N = 2500\hbar^2 R_{\rm c}^4/M $, and in (c) and (d) is $ \tilde{C}_6^{(\downarrow\downarrow)}N = 2\tilde{C}_6^{(\uparrow\uparrow)}N = 2500\hbar^2 R_{\rm c}^4/M $. The directions of the arrows in the color wheel indicate the elevation of the respective quantities. Other parameters are fixed at $ \tilde{C}_6^{(\uparrow\downarrow)}N = 1250\hbar^2 R_{\rm c}^4/M $, $ \kappa = 4\hbar/MR_{\rm c} $ and $ gN = 1000\hbar^2/M $^[200]

DownLoad: Full-Size Img PowerPoint

图 14 由Rashba类型SOC((a), (b))和Dresselhaus类型SOC((c), (d))诱导产生的粒子流$ {{j}} $和自旋的径向磁化$ {{S}}_z $. 其中颜色从蓝到红代表$ {{S}}_z $从小到大, 黑色箭头代表环流方向$ {{j}} $. 本图中参数与图12相同^[200]

Figure 14. Particle currents $ {{j}} $ and longitudinal magnetizations $ {{S}}_z $ of the spin induced by Rashba spin-orbit coupling ( (a), (b)) and Dresselhaus spin-orbit coupling ((c), (d) ). $ {{S}}_z $ and $ {{j}} $ are represented by the color map and black arrows, respectively. The colors ranging from blue to red represent the values from the minimum to the maximum. The parameters used here are same as those in Fig. 12^[200]

DownLoad: Full-Size Img PowerPoint

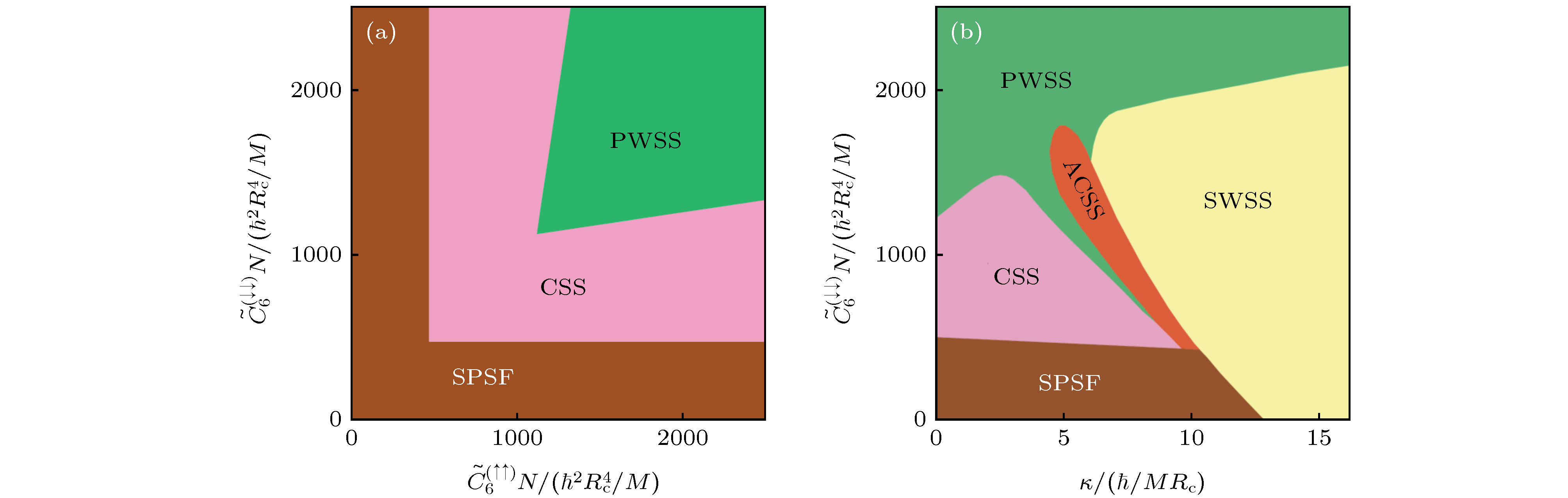
图 15 (a) 通过改变软核长程相互作用强度$ \tilde{C}_6^{(\uparrow\uparrow)} $和$ \tilde{C}_6^{(\downarrow\downarrow)} $的体系相图; (b) 通过改变SOC强度$ \kappa $和软核长程相互作用强度$ \tilde{C}_6^{(\downarrow\downarrow)} $的体系相图. 图(a)中SOC强度固定为$ \kappa = 4\hbar/MR_{\rm c} $, 图(b)中软核长程相互作用强度固定为$ \tilde{C}_6^{(\uparrow\uparrow)} = 2500\hbar^2 R_{\rm c}^4/M $. 其他参数为$ \tilde{C}_6^{(\uparrow\downarrow)}N = 1250\hbar^2 R_{\rm c}^4/M $和$ gN = 1000\hbar^2/M $^[200]

Figure 15. (a) The phase diagram by varying the soft-core long-range interaction strengths $ \tilde{C}_6^{(\uparrow\uparrow)} $ and $ \tilde{C}_6^{(\downarrow\downarrow)} $; (b) the phase diagram by varying the Rashba spin-orbit-coupling strength $ \kappa $ and the soft-core long-range interaction strength $ \tilde{C}_6^{(\downarrow\downarrow)} $. The spin-orbit-coupling strength in (a) is fixed at $ \kappa = 4\hbar/MR_{\rm c} $, and the soft-core long-range interaction strength in (b) is fixed at $ \tilde{C}_6^{(\uparrow\uparrow)} = 2500\hbar^2 R_{\rm c}^4/M $. Other parameters are taken as $ \tilde{C}_6^{(\uparrow\downarrow)}N = 1250\hbar^2 R_{\rm c}^4/M $ and $ gN = 1000\hbar^2/M $^[200]

DownLoad: Full-Size Img PowerPoint

map

[1]	Gong M, Tewari S, Zhang C 2011 Phys. Rev. Lett. 107 195303 Google Scholar
[2]	Hu H, Jiang L, Liu X J, Pu H 2011 Phys. Rev. Lett. 107 195304 Google Scholar
[3]	Han L, Melo C A R S' a de 2012 Phys. Rev. A 85 011606(R)Google Scholar
[4]	Yu Z Q, Zhai H 2011 Phys. Rev. Lett. 107 195305 Google Scholar
[5]	Iskin M, Subası A L 2011 Phys. Rev. Lett. 107 050402 Google Scholar
[6]	Yi W, Guo G C 2011 Phys. Rev. A 84 031608(R)Google Scholar
[7]	Lin Y J, Jiménez-García K, Spielman I B 2011 Nature (London) 471 83 Google Scholar
[8]	Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H, Chai S J, Zhai H, Zhang J 2012 Phys. Rev. Lett. 109 095301 Google Scholar
[9]	Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302 Google Scholar
[10]	Huang L H, Meng Z M, Wang P J, Peng P, Zhang S L, Chen L C, Li D H, Zhou Q, Zhang J 2016 Nat. Phys. 12 540 Google Scholar
[11]	Wu Z, Zhang L, Sun W, Xu X T, Wang B Z, Ji S C, Deng Y, Chen S, Liu X J, Pan J W 2016 Science 354 83 Google Scholar
[12]	Chen H R, Lin K Y, Chen P K, Chiu N C, Wang J B, Chen C A, Huang P P, Yip S K, Kawaguchi Y, Lin Y J 2018 Phys. Rev. Lett. 121 113204 Google Scholar
[13]	Zhang D F, Gao T Y, Zou P, Kong L R, Li R Z, Shen X, Chen X L, Peng S G, Zhan M S, Pu H, Jiang K J 2019 Phys. Rev. Lett. 122 110402 Google Scholar
[14]	Zhai H 2015 Rep. Prog. Phys. 78 026001 Google Scholar
[15]	Vyasanakere J P, Shenoy V B 2011 Phys. Rev. B 83 094515 Google Scholar
[16]	Vyasanakere J P, Zhang S Z, Shenoy V B 2011 Phys. Rev. B 84 014512 Google Scholar
[17]	Xu Z F, Lü R, You L 2011 Phys. Rev. A 83 053602 Google Scholar
[18]	Kawakami T, Mizushima T, Machida K 2011 Phys. Rev. A 84 011607 Google Scholar
[19]	Wu C J, Mondragon-Shem I, Zhou X F 2011 Chin. Phys. Lett. 28 097102 Google Scholar
[20]	Stanescu T D, Anderson B, Galitski V 2008 Phys. Rev. A 78 023616 Google Scholar
[21]	Deng Y, Cheng J, Jing H, Sun C P, Yi S 2012 Phys. Rev. Lett. 108 125301 Google Scholar
[22]	Kawakami T, Mizushima T, Nitta M, Machida K 2012 Phys. Rev. Lett. 109 015301 Google Scholar
[23]	Li Y, Zhou X F, Wu C J 2016 Phys. Rev. A 93 033628 Google Scholar
[24]	Sinha S, Nath R, Santos L 2011 Phys. Rev. Lett. 107 270401 Google Scholar
[25]	Hu H, Ramachandhran B, Pu H, Liu X J 2012 Phys. Rev. Lett. 108 010402 Google Scholar
[26]	Ramachandhran B, Hu H, Pu H 2013 Phys. Rev. A 87 033627 Google Scholar
[27]	Li Y, Zhou X F, Wu C J 2012 Phys. Rev. B 85 125122 Google Scholar
[28]	Campbell D L, Juzeliūnas G, Spielman I B 2011 Phys. Rev. A 84 025602 Google Scholar
[29]	Sau J D, Sensarma R, Powell S, Spielman I B, Sarma S D 2011 Phys. Rev. B 83 140510(R)Google Scholar
[30]	Xu Z F, You L 2012 Phys. Rev. A 85 043605 Google Scholar
[31]	Liu X J, Law K T, Ng T K 2014 Phys. Rev. Lett. 112 086401 Google Scholar
[32]	Anderson B M, Spielman I B, Juzeliūnas 2013 Phys. Rev. Lett. 111 125301 Google Scholar
[33]	Xu Z F, You L, Ueda M 2013 Phys. Rev. A 87 063634 Google Scholar
[34]	Anderson B M, Juzeliünas G, Galitski V M, Spielman I B 2012 Phys. Rev. Lett. 108 235301 Google Scholar
[35]	Zhou J, Zhang W, Yi W 2011 Phys. Rev. A 84 063603 Google Scholar
[36]	Chen J, Hu H, Gao X L 2014 Phys. Rev. A 90 023619 Google Scholar
[37]	Chen C 2013 Phys. Rev. Lett. 111 235302 Google Scholar
[38]	Qu C L, Zheng Z, Gong M, Xu Y, Mao L, Zou X B, Guo G C, Zhang C W 2013 Nat. Commun. 4 2710 Google Scholar
[39]	Liu X J, Hu H 2013 Phys. Rev. A 88 023622 Google Scholar
[40]	Zhang W, Yi W 2013 Nat. Commun. 4 2711 Google Scholar
[41]	Cui X L, Yi W 2014 Phys. Rev. X 4 031026
[42]	Shi Z Y, Cui X L, Zhai H 2014 Phys. Rev. Lett 112 013201 Google Scholar
[43]	Pan J S, Liu X J, Zhang W, Yi W, Guo G C 2015 Phys. Rev. Lett. 115 045303 Google Scholar
[44]	Han W, Zhang X F, Song S W, Saito H, Zhang W, Liu W M, Zhang S G 2016 Phys. Rev. A 94 033629 Google Scholar
[45]	Han W, Zhang X F, Wang D S, Jiang K J, Zhang W, Zhang S G 2018 Phys. Rev. Lett. 121 030404 Google Scholar
[46]	Zhou X F, Pan J S, Liu Z X, Zhang W, Yi W, Chen G, Jia S T 2017 Phys. Rev. Lett. 119 185701 Google Scholar
[47]	Dalibard J, Gerbier F, Juzeliūnas G, Öberg P 2011 Rev. Mod. Phys. 83 1523 Google Scholar
[48]	Yi W, Zhang W, Cui X 2015 Sci. China Phys. Mech. Astron. 58 1-11
[49]	Lin Y J, Compton R L, Jiménez-García K, Porto J V, Spielman I B 2009 Nature (London) 462 628 Google Scholar
[50]	Lin Y J, Compton R L, Jiménez-García K, Porto J V, Spielman I B 2011 Nat. Phys. 7 531 Google Scholar
[51]	Ruseckas J, Juzeliūnas G, Öhberg P, Fleischhauer M 2005 Phys. Rev. Lett. 95 010404 Google Scholar
[52]	Zhang L, Liu X J 2018 arXiv 1806 05628
[53]	Liu X J, Liu Z X, Cheng M 2013 Phys. Rev. Lett. 110 076401 Google Scholar
[54]	Sun W, Wang B Z, Xu X T, Yi C R, Zhang L, Wu Z, Deng Y, Liu X J, Chen S, Pan J W 2018 Phys. Rev. Lett. 121 150401 Google Scholar
[55]	Baumann K, Guerlin C, Brennecke F, Esslinger T 2010 Nature (London) 464 1301 Google Scholar
[56]	Ritsch H, Domokos P, Brennecke F, Esslinger T 2013 Rev. Mod. Phys. 85 553 Google Scholar
[57]	Dalla Torre E G, Diehl S, Lukin M D, Sachdev S, Strack P 2013 Phys. Rev. A 87 023831 Google Scholar
[58]	Gopalakrishnan S, Lev B L, Goldbart P M 2009 Nat. Phys. 5 845 Google Scholar
[59]	Strack P, Sachdev S 2011 Phys. Rev. Lett. 107 277202 Google Scholar
[60]	Müller M, Strack P, Sachdev S 2012 Phys. Rev. A 86 023604 Google Scholar
[61]	Domokos P, Ritsch H 2002 Phys. Rev. Lett. 89 253003 Google Scholar
[62]	Dimer F, Estienne B, Parkins A S, Carmichael H J 2007 Phys. Rev. A 75 013804 Google Scholar
[63]	Nagy D, Konya G, Szirmai G, Domokos P 2010 Phys. Rev. Lett. 104 130401 Google Scholar
[64]	Keeling J, Bhaseen M J, Simons B D 2014 Phys. Rev. Lett. 112 143002 Google Scholar
[65]	Piazza F, Strack P 2014 Phys. Rev. Lett. 112 143003 Google Scholar
[66]	Chen Y, Yu Z, Zhai H 2014 Phys. Rev. Lett. 112 143004 Google Scholar
[67]	Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045 Google Scholar
[68]	Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057 Google Scholar
[69]	Lin Y J, Jiménez-García K, Spielman I B 2011 Nature 471 83
[70]	Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H, Chai S J, Zhai H, Zhang J 2012 Phys. Rev. Letter 109 095301
[71]	Galitski V, Spielman I B 2013 Nature (London) 494 49 Google Scholar
[72]	Goldman N, Juzeliūnas G, Öberg P, Spielman I B 2014 Rep. Prog. Phys. 77 126401 Google Scholar
[73]	Zhou X, Li Y, Cai Z, Wu C 2013 J. Phys. B 46 134001 Google Scholar
[74]	Deng Y, Cheng J, Jing H, Yi S 2014 Phys. Rev. Lett. 112 143007 Google Scholar
[75]	Dong L, Zhou L, Wu B, Ramachandhran B, Pu H 2014 Phys. Rev. A 89 011602(R)Google Scholar
[76]	Pan J S, Liu X J, Zhang W, Yi W, Guo G C 2015 Phys. Rev. Letter 115 045303
[77]	Liu X J, Law K T, Ng T K 2014 Phys. Rev. Letter 112 086401
[78]	Gu Z C, Wen X G 2009 Phys. Rev. B 80 155131 Google Scholar
[79]	Pollmann F, Berg E, Turner A M, Oshikawa M 2012 Phys. Rev. B 85 075125 Google Scholar
[80]	Wen X G 1989 Phys. Rev. B 40 7387
[81]	Wen X G, Niu Q 1990 Phys. Rev. B 41 9377 Google Scholar
[82]	Wen X G 1990 Int. J. Mod. Phys. B 04 239 Google Scholar
[83]	Haldane F D M 1983 Phys. Rev. Lett. 50 1153 Google Scholar
[84]	Kane C L, Mele E J 2005 Phys. Rev. Lett. 95 146802 Google Scholar
[85]	Bernevig B A, Zhang S C 2006 Phys. Rev. Lett. 96 106802 Google Scholar
[86]	Moore J E, Balents L 2007 Phys. Rev. B 75 121306(R)Google Scholar
[87]	Fu L, Kane C L, Mele E J 2007 Phys. Rev. Lett. 98 106803 Google Scholar
[88]	Qi X L, Hughes T L, Zhang S C 2008 Phys. Rev. B 78 195424 Google Scholar
[89]	Chen X, Gu Z C, Wen X G 2011 Phys. Rev. B 83 035107 Google Scholar
[90]	Chen X, Gu Z C, Liu Z X, Wen X G 2013 Phys. Rev. B 87 155114 Google Scholar
[91]	Kitaev A 2009 AIP Conf. Proc. 1134 22
[92]	Ryu S, Schnyder A, Furusaki A, Ludwig A 2010 New J. Phys. 12 065010 Google Scholar
[93]	Fidkowski L, Kitaev A 2010 Phys. Rev. B 81 134509 Google Scholar
[94]	Gu Z C, Wen X G 2014 Phys. Rev. B 90 115141 Google Scholar
[95]	Wang C, Potter A C, Senthil T 2014 Science 343 629 Google Scholar
[96]	Wu H Q, He Y Y, You Y Z, Yoshida T, Kawakami N, Xu C, Meng Z Y, Lu Z Y 2016 Phys. Rev. B 94 165121 Google Scholar
[97]	Takamoto M, Hong F L, Higashi R, Katori H 2005 Nature (London) 435 321 Google Scholar
[98]	Ludlow A D, Boyd M M, Zelevinsky T, Foreman S M, Blatt S, Notcutt M, Ido T, Ye J 2006 Phys. Rev. Lett. 96 033003 Google Scholar
[99]	Swallows M D, Bishof M, Lin Y, Blatt S, Martin M J, Rey A M, Ye J 2011 Science 331 1043 Google Scholar
[100]	Bloom B J, Nicholson T L, Williams J R, Campbell S L, Bishof M, Zhang X, Zhang W, Bromley S L, Ye J 2014 Nature (London) 506 71 Google Scholar
[101]	Cazalilla M A, Rey A M 2014 Rep. Prog. Phys. 77 124401 Google Scholar
[102]	Gorshkov A V, Rey A M, Daley A J, Boyd M M, Ye J, Zoller P, Lukin M D 2009 Phys. Rev. Lett. 102 110503 Google Scholar
[103]	Wu C, Hu J P, Zhang S C 2003 Phys. Rev. Lett. 91 186402 Google Scholar
[104]	Fukuhara T, Takasu Y, Kumakura M, Takahashi Y 2007 Phys. Rev. Lett. 98 030401 Google Scholar
[105]	Cazalilla M A, Ho A F, Ueda M 2009 New J. Phys. 11 103033 Google Scholar
[106]	Stellmer S, Tey M K, Huang B, Grimm R, Schreck F 2009 Phys. Rev. Lett. 103 200401 Google Scholar
[107]	DeSalvo B J, Yan M, Mickelson P G, Martinez de Escobar Y N, Killian T C 2010 Phys. Rev. Lett. 105 030402 Google Scholar
[108]	Gorshkov A V, Hermele M, Gurarie V, Xu C, Julienne P S, Ye J, Zoller P, Demler E, Lukin M D, Rey A M 2010 Nat. Phys. 6 289 Google Scholar
[109]	Kobayashi K, Okumura M, Ota Y, Yamada S, Machida M 2012 Phys. Rev. Lett. 109 235302 Google Scholar
[110]	Nonne H, Moliner M, Capponi S, Lecheminant P, Totsuka K 2013 Europhys. Lett. 102 37008 Google Scholar
[111]	Duivenvoorden K, Quella T 2013 Phys. Rev. B 87 125145
[112]	Zhang X, Bishof M, Bromley S L, Kraus C V, Safronova M S, Zoller P, Rey A M, Ye J 2014 Science 345 1467 Google Scholar
[113]	Scazza F, Hofrichter C, Höfer M, De Groot P C, Bloch I, Fölling S 2014 Nat. Phys. 10 779 Google Scholar
[114]	Cappellini G, Mancini M, Pagano G, Lombardi P, Livi L, Siciliani de Cumis M, Cancio P, Pizzocaro M, Calonico D, Levi F, Sias C, Catani J, Inguscio M, Fallani L 2014 Phys. Rev. Lett. 113 120402 Google Scholar
[115]	Mancini M, Pagano G, Cappellini G, Livi L, Rider M, Catani J, Sias C, Zoller P, Inguscio M, Dalmonte M, Fallani L 2015 Science 349 1510 Google Scholar
[116]	Bois V, Capponi S, Lecheminant P, Moliner M, Totsuka K 2015 Phys. Rev. B 91 075121
[117]	Roy A, Quella T arXiv: 1512.05229
[118]	Hofrichter C, Riegger L, Scazza F, Höfer M, Fernandes D R, Bloch I, Fölling S 2016 Phys. Rev. X 6 021030
[119]	Bois V, Fromholz P, Lecheminant P 2016 Phys. Rev. B 93 134415 Google Scholar
[120]	Capponi S, Lecheminant P, Totsuka K 2016 Ann. Phys. (Amsterdam) 367 50 Google Scholar
[121]	Wall M L, Koller A P, Li S, Zhang X, Cooper N R, Ye J, Rey A M 2016 Phys. Rev. Lett. 116 035301 Google Scholar
[122]	Kolkowitz S, Bromley S L, Bothwell T, Wall M L, Marti G E, Koller A P, Zhang X, Rey A M, Ye J 2017 Nature (London) 542 66 Google Scholar
[123]	Livi L F, Cappellini G, Diem M, Franchi L, Clivati C, Frittelli M, Levi F, Calonico D, Catani J, Inguscio M, Fallani L 2016 Phys. Rev. Lett. 117 220401 Google Scholar
[124]	Song B, He C, Zhang S, Hajiyev E, Huang W, Liu X J, Jo G B 2016 Phys. Rev. A 94 061604 Google Scholar
[125]	Zhang R, Cheng Y, Zhai H, Zhang Z 2015 Phys. Rev. Lett. 115 135301 Google Scholar
[126]	Pagano G, Mancini M, Cappellini G, Livi L, Sias C, Catani J, Inguscio M, Fallani L 2015 Phys. Rev. Lett. 115 265301 Google Scholar
[127]	Höfer M, Riegger L, Scazza F, Hofrichter C, Fernandes D R, Parish M M, Levinsen J, Bloch I, Fölling S 2015 Phys. Rev. Lett. 115 265302 Google Scholar
[128]	Zhou X F, Pan J S, Liu Z X, Zhang W, Yi W, Chen G, Jia S T 2017 Phys. Rev. Letter 119 185701
[129]	Dzuba V A, Derevianko A 2010 J. Phys. B 43 074011 Google Scholar
[130]	Porsev S G, Derevianko A, Fortson E N 2004 Phys. Rev. A 69 021403 Google Scholar
[131]	Zhou L, Cui X 2015 Phys. Rev. B 92 140502(R)Google Scholar
[132]	Yu D, Pan J S, Liu X J, Zhang W, Yi W 2017 Front. Phys. 13 136701
[133]	Pan J S, Zhang W, Yi W, Guo G C 2016 Phys. Rev. A 94 043619 Google Scholar
[134]	Zhang R, Zhang D, Cheng Y, Chen W, Zhang P, Zhai H 2016 Phys. Rev. A 93 043601 Google Scholar
[135]	Liu X J, Liu Z X, Cheng M 2013 Phys. Rev. Letter 110 076401
[136]	Tang E, Wen X G 2012 Phys. Rev. Lett. 109 096403 Google Scholar
[137]	Morimoto T, Furusaki A, Mudry C 2015 Phys. Rev. B 92 125104 Google Scholar
[138]	Zhao J Z, Hu S J, Zhang P 2015 Phys. Rev. Lett. 115 195302 Google Scholar
[139]	Yoshida T, Peters R, Fujimoto S, Kawakami N 2014 Phys. Rev. Lett. 112 196404 Google Scholar
[140]	Pollmann F, Turner A M, Berg E, Oshikawa M 2010 Phys. Rev. B 81 064439 Google Scholar
[141]	Turner A M, Pollmann F, Berg E 2011 Phys. Rev. B 83 075102 Google Scholar
[142]	Fidkowski L 2010 Phys. Rev. Lett. 104 130502 Google Scholar
[143]	Flammia S T, Hamma A, Hughes T L, Wen X G 2009 Phys. Rev. Lett. 103 261601 Google Scholar
[144]	Li H, Haldane F D M 2008 Phys. Rev. Lett. 101 010504 Google Scholar
[145]	Hastings M B, González I, Kallin A B, Melko R G 2010 Phys. Rev. Lett. 104 157201 Google Scholar
[146]	Daley A J, Pichler H, Schachenmayer J, Zoller P 2012 Phys. Rev. Lett. 109 020505 Google Scholar
[147]	Abanin D A, Demler E 2012 Phys. Rev. Lett. 109 020504 Google Scholar
[148]	Jiang H C, Wang Z H, Balents L 2012 Nat. Phys. 8 902 Google Scholar
[149]	Islam R, Ma R, Preiss P M, Tai M E, Lukin A, Rispoli M, Greiner M 2015 Nature (London) 528 77 Google Scholar
[150]	Calabrese P, Cardy J 2004 J. Stat. Mech: Theory Exp. 06 P06002
[151]	Nielsen A E B, Sierra G, Cirac J I 2011 Phys. Rev. A 83 053807 Google Scholar
[152]	Wang C, Gao C, Jian C M, Zhai H 2010 Phys. Rev. Lett. 105 160403 Google Scholar
[153]	Barnett R, Boyd G R, Galitski V 2012 Phys. Rev. Lett. 109 235308 Google Scholar
[154]	Kawaguchi Y, Ueda M 2012 Phys. Rep. 520 253 Google Scholar
[155]	Isoshima T, Machida K, Ohmi T 2001 J. Phys. Soc. Jpn. 70 1604 Google Scholar
[156]	Mizushima T, Kobayashi N, Machida K 2004 Phys. Rev. A 70 043613 Google Scholar
[157]	Stamper-Kurn D M, Ueda M 2013 Rev. Mod. Phys. 85 1191 Google Scholar
[158]	Wang D S, Shi Y R, Chow K W, Yu Z X, Li X G 2013 Eur. Phys. J. D 67 242 Google Scholar
[159]	Wang D S, Ma Y Q, Li X G 2014 Commun. Nonlinear Sci. Numer. Simul. 19 3556 Google Scholar
[160]	Xu Z F, Kawaguchi Y, You L, Ueda M 2012 Phys. Rev. A 86 033628 Google Scholar
[161]	Lan Z, Ohberg P 2014 Phys. Rev. A 89 023630 Google Scholar
[162]	Han W, Zhang X F, Song S W, Saito H, Zhang W, Liu W M, Zhang S C 2016 Physics Review A 94 033629
[163]	Zhang Y, Mao L, Zhang C 2012 Phys. Rev. Lett. 108 035302 Google Scholar
[164]	Li Y, Pitaevskii L P, Stringari S 2012 Phys. Rev. Lett. 108 225301 Google Scholar
[165]	Saito H, Kawaguchi Y, Ueda M 2006 Phys. Rev. Lett. 96 065302 Google Scholar
[166]	Saito H, Kawaguchi Y, Ueda M 2007 Phys. Rev. A 75 013621 Google Scholar
[167]	Kawaguchi Y, Saito H, Kudo K, Ueda M 2010 Phys. Rev. A 82 043627 Google Scholar
[168]	Lovegrove J, Borgh M O, Ruostekoski J 2012 Phys. Rev. A 86 013613 Google Scholar
[169]	Su S W, Liu I K, Tsai Y C, Liu W M, Gou S C 2012 Phys. Rev. A 86 023601 Google Scholar
[170]	Shinjo T, Okuno T, Hassdorf R, Shigeto K, Ono T 2000 Science 289 930 Google Scholar
[171]	Wachowiak A, Wiebe J, Bode M, Pietzsch O, Morgenstern M, Wiesendanger R 2002 Science 298 577 Google Scholar
[172]	Yi S, Pu H 2006 Phys. Rev. Lett. 97 020401 Google Scholar
[173]	Leslie L S, Hansen A, Wright K C, Deutsch B M, Bigelow N P 2009 Phys. Rev. Lett. 103 250401 Google Scholar
[174]	Sadler L E, Higbie J M, Leslie S R, Vengalattore M, Stamper-Kurn D M 2006 Nature (London) 443 312 Google Scholar
[175]	Savard T A, Granade S R, O'Hara K M, Gehm M E, Thomas J E 1999 Phys. Rev. A 60 4788 Google Scholar
[176]	McGuire B A, Carroll P B, Loomis R A, Finneran I A, Jewell P R, Remijan A J, Blake G A 2016 Science 352 1449 Google Scholar
[177]	Yoon M, Srirambalaji R, Kim K 2012 Chem. Rev. 112 1196 Google Scholar
[178]	Kallin C, Berlinsky J 2016 Rep. Prog. Phys. 79 054502 Google Scholar
[179]	Weng H, Fang C, Fang Z, Bernevig B A, Dai X 2015 Phys. Rev. X 5 011029
[180]	Ryu K S, Thomas L, Yang S H, Parkin S 2013 Nat. Nanotechnol. 8 527 Google Scholar
[181]	Emori S, Bauer U, Ahn S M, Martinez E, Beach G S D 2013 Nat. Mater. 12 611 Google Scholar
[182]	Chen G, Ma T, N'Diaye A T, Kwon H, Won C, Wu Y, Schmid A K 2013 Nat. Commun. 4 2671
[183]	Shibata K, Yu X Z, Hara T, Morikawa D, Kanazawa N, Kimoto K, Ishiwata S, Matsui Y, Tokura Y 2013 Nat. Nanotechnol. 8 723 Google Scholar
[184]	Zhai H 2012 Int. J. Mod. Phys. B 26 1230001 Google Scholar
[185]	Wu C 2009 Mod. Phys. Lett. B 23 1
[186]	Wilson R M, Anderson B M, Clark C W 2013 Phys Rev. Lett. 111 185303 Google Scholar
[187]	Gopalakrishnan S, Martin I, Demler E A 2013 Phys. Rev. Lett. 111 185304 Google Scholar
[188]	Henkel N, Nath R, Pohl T 2010 Phys. Rev. Lett. 104 195302 Google Scholar
[189]	Hsueh C H, Tsai Y C, Wu K S, Chang M S, Wu W C 2013 Phys. Rev. A 88 043646 Google Scholar
[190]	Heidemann R, Raitzsch U, Bendkowsky V, Butscher B, Löw R, Pfau T 2008 Phys. Rev. Lett. 100 033601 Google Scholar
[191]	Boninsegni M, Prokof'ev N V 2012 Rev. Mod. Phys. 84 759 Google Scholar
[192]	Boninsegni M 2012 J. Low Temp. Phys. 168 137 Google Scholar
[193]	Balibar S 2010 Nature (London) 464 176 Google Scholar
[194]	Andreev A F, Lifshitz I M 1969 Zh. Eksp. Teor. Fiz. 56 2057
[195]	Chester G V 1970 Phys. Rev. A 2 256 Google Scholar
[196]	Leggett A J 1970 Phys. Rev. Lett. 25 1543 Google Scholar
[197]	Kim E, Chan M H W 2004 Nature (London) 427 225 Google Scholar
[198]	Luo X, Wu L, Chen J, Guan Q, Gao K, Xu Z F, You L, Wang R 2016 Sci. Rep. 6 18983 Google Scholar
[199]	Yefsah T, Desbuquois R, Chomaz L, Günter K J, Dalibard J 2011 Phys. Rev. Lett. 107 130401 Google Scholar
[200]	Han W, Zhang X F, Wang D S, Jiang H F, Zhang W, Zhang S G 2018 Phys. Rev. Letter 121 030404
[201]	Ruokokoski E, Huhtamäki, MöttÖnen M 2012 Phys. Rev. A 86 051607(R)Google Scholar
[202]	Xu Z F, Kobayashi S, Ueda M 2013 Phys. Rev. A 88 013621 Google Scholar
[203]	Su S W, Gou S C, Sun Q, Wen L, Liu W M, Ji A C, Ruseckas J, Juzeliūnas G 2016 Phys. Rev. A 93 053630 Google Scholar
[204]	Nagaosa N, Tokura Y 2013 Nat. Nanotechnol. 8 899 Google Scholar
[205]	Mühlbauer S, Binz B, Jonietz F, Pfleiderer C, Rosch A, Neubauer A, Georgii R, Böni P 2009 Science 323 915 Google Scholar
[206]	Yu X Z, Onose Y, Kanazawa N, Park J H, Han J H, Matsui Y, Nagaosa N, Tokura Y 2010 Nature (London) 465 901 Google Scholar
[207]	Seki S, Yu X Z, Ishiwata S, Tokura Y 2012 Science 336 198 Google Scholar
[208]	Ozawa T, Baym G 2012 Phys. Rev. A 85 063623 Google Scholar
[209]	Fetter A L 2014 Phys. Rev. A 89 023629 Google Scholar
[210]	Madison K W, Chevy F, Wohlleben W, Dalibard J 2000 Phys. Rev. Lett. 84 806 Google Scholar
[211]	Abo-Shaeer J R, Raman C, Vogels J M, Ketterle W 2001 Science 292 476 Google Scholar
[212]	Li J R, Lee J, Huang W, Burchesky S, Shteynas B, Top F C, Jamison A O, Ketterle W 2017 Nature (London) 543 91 Google Scholar
[213]	Ho T L, Zhang S 2011 Phys. Rev. Lett. 107 150403 Google Scholar
[214]	Ji S C, Zhang J Y, Zhang L, Du Z D, Zheng W, Deng Y J, Zhai H, Chen S, Pan J W 2014 Nat. Phys. 10 314 Google Scholar
[215]	Hall D S, Matthews M R, Ensher J R, Wieman C E, Cornell E A 1998 Phys. Rev. Lett. 81 1539 Google Scholar

[1]	Zhang Jie, Chen Ai-Xi, Peng Ze-An. Spatially oriented correlated emission based on selective drive of diatomic superradiance states. Acta Physica Sinica, 2024, 73(14): 144202. doi: 10.7498/aps.73.20240521
[2]	Wang Huan, He Xia-Yao, Li Shuai, Liu Bo. Quench dynamics of a spin-orbital coupled Bose-Einstein condensate with nonlinear interactions. Acta Physica Sinica, 2023, 72(10): 100309. doi: 10.7498/aps.72.20222401
[3]	Wang Zhi-Mei, Wang Hong, Xue Nai-Tao, Cheng Gao-Yan. Quantum coherence in spin-orbit coupled quantum dots system. Acta Physica Sinica, 2022, 71(7): 078502. doi: 10.7498/aps.71.20212111
[4]	Wu Jin, Lu Zhan-Peng, Xu Zhi-Hao, Guo Li-Ping. Mobility edges and reentrant localization induced by superradiance. Acta Physica Sinica, 2022, 71(11): 113702. doi: 10.7498/aps.71.20212246
[5]	Li Jia-Rui, Wang Zi-An, Xu Tong-Tong, Zhang Lian-Lian, Gong Wei-Jiang. Topological properties of the one-dimensional ${\cal {PT}}$-symmetric non-Hermitian spin-orbit-coupled Su-Schrieffer-Heeger model. Acta Physica Sinica, 2022, 71(17): 177302. doi: 10.7498/aps.71.20220796
[6]	Chen Xing, Xue Xiao-Bo, Zhang Sheng-Kang, Ma Yu-Quan, Fei Peng, Jiang Yuan, Ge Jun. Ground energy level transition for two-body interacting Fermionic system with spin-orbit coupling and Zeeman interaction. Acta Physica Sinica, 2021, 70(8): 083401. doi: 10.7498/aps.70.20201456
[7]	Zhang Ai-Xia, Jiang Yan-Fang, Xue Ju-Kui. Nonlinear energy band structure of spin-orbit coupled Bose-Einstein condensates in optical lattice. Acta Physica Sinica, 2021, 70(20): 200302. doi: 10.7498/aps.70.20210705
[8]	Xue Hai-Bin, Duan Zhi-Lei, Chen Bin, Chen Jian-Bin, Xing Li-Li. Electron transport through Su-Schrieffer-Heeger chain with spin-orbit coupling. Acta Physica Sinica, 2021, 70(8): 087301. doi: 10.7498/aps.70.20201742
[9]	Liang Tao, Li Ming. Integer quantum Hall effect in a spin-orbital coupling system. Acta Physica Sinica, 2019, 68(11): 117101. doi: 10.7498/aps.68.20190037
[10]	Li Zhi-Qiang, Wang Yue-Ming. One-dimensional spin-orbit coupling Bose gases with harmonic trapping. Acta Physica Sinica, 2019, 68(17): 173201. doi: 10.7498/aps.68.20190143
[11]	Yang Yuan, Chen Shuai, Li Xiao-Bing. Topological phase transitions in square-octagon lattice with Rashba spin-orbit coupling. Acta Physica Sinica, 2018, 67(23): 237101. doi: 10.7498/aps.67.20180624
[12]	Liu Sheng-Li, Li Jian-Zheng, Cheng Jie, Wang Hai-Yun, Li Yong-Tao, Zhang Hong-Guang, Li Xing-Ao. Doping and Raman scattering of strong spin-orbit-coupling compound Sr2-xLaxIrO4. Acta Physica Sinica, 2015, 64(20): 207103. doi: 10.7498/aps.64.207103
[13]	Chen Dong-Hai, Yang Mou, Duan Hou-Jian, Wang Rui-Qiang. Electronic transport properties of graphene pn junctions with spin-orbit coupling. Acta Physica Sinica, 2015, 64(9): 097201. doi: 10.7498/aps.64.097201
[14]	Chen Guang-Ping. Ground state of a rotating spin-orbit-coupled Bose-Einstein condensate in a harmonic plus quartic potential. Acta Physica Sinica, 2015, 64(3): 030302. doi: 10.7498/aps.64.030302
[15]	Gong Shi-Jing, Duan Chun-Gang. Recent progress in Rashba spin orbit coupling on metal surface. Acta Physica Sinica, 2015, 64(18): 187103. doi: 10.7498/aps.64.187103
[16]	Chen Zai-Gao, Wang Jian-Guo, Wang Yue, Zhu Xiang-Qin, Zhang Dian-Hui, Qiao Hai-Liang. Numerical simulation of generation and radiation of super-radiation from relativistic backward wave oscillators. Acta Physica Sinica, 2014, 63(3): 038402. doi: 10.7498/aps.63.038402
[17]	Zhang Lei, Li Hui-Wu, Hu Liang-Bin. Study of stability of persistent spin helix in two-dimensional electron gases with spin-orbit coupling. Acta Physica Sinica, 2012, 61(17): 177203. doi: 10.7498/aps.61.177203
[18]	Dong Quan-Li, Zhang Jie, Yang Jie, Jiang Zhao-Tan. Electronic energy band structures of carbon nanotubeswith spin-orbit coupling interaction. Acta Physica Sinica, 2011, 60(7): 075202. doi: 10.7498/aps.60.075202
[19]	Yu Zhi-Qiang, Xie Quan, Xiao Qing-Quan. Effects of the spin-orbit coupling on X-ray spectrum in special relativity. Acta Physica Sinica, 2010, 59(2): 925-931. doi: 10.7498/aps.59.925
[20]	Zhou Qing-Chun, Wang Jia-Fu, Xu Rong-Qing. . Acta Physica Sinica, 2002, 51(7): 1639-1644. doi: 10.7498/aps.51.1639

Catalog

Vol. 69, Issue 1 - 2020-01-05

Metrics

Abstract views: 19853
PDF Downloads: 477
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板