-
对于两个二能级原子组成的系统, 当受到方向性可调的激光场驱动, 同时原子间存在偶极-偶极相互作用和自发辐射相干的条件下, 我们研究系统的双光子发射现象. 对于全同原子系统, 原子在特殊的几何构型下, 能够实现将原子系统选择性激发到超辐射态或亚辐射态, 详细讨论了关联函数的角分布情况. 对于非全同原子系统, 由于原子间的失谐, 选择性驱动减弱, 但是通过失谐的改变, 激光方向调节能够对能级间耦合强度产生显著影响. 研究发现调整激光的入射角度和原子的失谐, 能够改变原子一阶相干, 进而可以优化关联函数的角分布图样, 以获得良好的对称性. 本研究能够实现单侧或双侧高定向双光子发射, 这为纳米天线的双光子发射提供了理论依据.
In recent years, the radiative properties of atomic systems have been a hot topic in the research fields of quantum optics and quantum information. With the continuous development of nanophotonics, quantum antennas have become an important model for studying atomic radiation. In order to investigate these phenomena in depth, we investigate a system composed of two two-level atoms, and study the two-photon emission phenomenon of diatomic system under conditions of driving directional tunable laser field, interatomic dipole-dipole interaction, and spontaneous emission coherence. In this study, we diagonalize the atomic Hamiltonian to obtain the eigenvalues and entangled states of the system (symmetric and asymmetric states of two atoms), and use the rotating wave approximation to rotate the system into the laser frame. The evolution of the system is characterized mainly by the evolution of symmetric and asymmetric state, as well as the evolution of coherent terms. In our studies it is found that for identical atoms, certain laser directions and geometric configurations can exclusively drive the superradiant and subradiant states of atoms, which can enhance the first-order interference effect of the atoms and markedly increase the probability of two-photon emission in a specific detection direction. When the superradiant state of the atom is solely driven, there will be no coupling between the superradiant state and subradiant state, resulting in a correlation function angular distribution that is symmetric along the direction perpendicular to atomic axis. Further adjusting the laser direction causes the atomic interference patterns to shift, and the system will exhibit two-photon emission characteristics on one side or both sides. For nonidentical atomic systems, due to detuning between the two atoms, the laser cannot drive the superradiant state or subradiant state individually, and the influence of changing the laser direction on the coupling strength diminishes with the increase of detuning between the atoms. When the laser is in resonance with one of the atoms, due to the atomic interactions, the other atom can achieve the strongest coherent effect without resonating with the laser. This research reveals that atomic detuning is crucial for the correlation values and angular distribution of the correlation function. By adjusting the atomic detuning and laser direction, the system can display highly directed one-sided two-photon emission characteristics. However, different dissipation rates will lead the probability of two-photon emission to decrease. Our studies can achieve highly directional two-photon emission on one side or both sides, which provides a theoretical basis for studying the two-photon emission of nanoantennas. -
Keywords:
- superradiance /
- subradiance /
- correlation function /
- nanoantenna.
[1] Saffman M 2016 J. Phys. B: At. Mol. Opt. Phys. 49 202001
 Google Scholar
Google Scholar
[2] Li N, Jiang H W, Xia X W, Zhu C J, Xie S Y, Xu J P, Yang Y P 2021 Phys. Lett. A 420 127772
 Google Scholar
Google Scholar
[3] Vilas N B, Hallas C, Anderegg L, Robichaud P, Winnicki A, Mitra D, Doyle J M 2022 Nature 606 70
 Google Scholar
Google Scholar
[4] Cidrim A, doEspiritoSanto T S, Schachenmayer J, Kaiser R, Bachelard R 2020 Phys. Rev. Lett. 125 073601
 Google Scholar
Google Scholar
[5] Moreno-Cardoner M, Plankensteiner D, Ostermann L, Chang D E, Ritsch H 2019 Phys. Rev. A 100 023806
 Google Scholar
Google Scholar
[6] Nelson K D, Li X, Weiss D S 2007 Nat. Phys. 3 556
 Google Scholar
Google Scholar
[7] Dicke R H 1954 Phys. Rev. 93 99
 Google Scholar
Google Scholar
[8] Goban A, Hung C L, Hood J D, Yu S P, Muniz J A, Painter O, Kimble H J 2015 Phys. Rev. Lett. 115 063601
 Google Scholar
Google Scholar
[9] Scully M O, Svidzinsky A A 2009 Science 325 1510
 Google Scholar
Google Scholar
[10] Hildred G P, Puri R R, Hassan S S, Bullough R K 1984 J. Phys. B: At. Mol. Phys. 17 L535
 Google Scholar
Google Scholar
[11] Corman L, Ville J L, Saint-Jalm R, Aidelsburger M, Bienaimé T, Nascimbène S, Dalibard J, Beugnon J 2017 Phys. Rev. A 96 053629
 Google Scholar
Google Scholar
[12] Rui J, Wei D, Rubio-Abadal A, Hollerith S, Zeiher J, Stamper-Kurn D M, Gross C, Bloch I 2020 Nature 583 369
 Google Scholar
Google Scholar
[13] Peng J S, Li G X 1993 Phys. Rev. A 47 4212
 Google Scholar
Google Scholar
[14] Richter S, Wolf S, von Zanthier J, Schmidt-Kaler F 2023 Phys. Rev. Res. 5 013163
 Google Scholar
Google Scholar
[15] Das S, Agarwal G S, Scully M O 2008 Phys. Rev. Lett. 101 153601
 Google Scholar
Google Scholar
[16] Downing C, Del Valle E, Fernández-Domínguez A I 2023 Phys. Rev. A 107 023717
 Google Scholar
Google Scholar
[17] Shegai T, Chen S, Miljković V D, Zengin G, Johansson P, Käll M 2011 Nat. Commun. 2 481
 Google Scholar
Google Scholar
[18] Vivas-Viaña A, Sánchez Muñoz C 2021 Phys. Rev. Res. 3 033136
 Google Scholar
Google Scholar
[19] Gulfam Q-u-A, Evers J 2010 J. Phys. B: At. Mol. Opt. Phys. 43 045501
 Google Scholar
Google Scholar
[20] Araneda G, Higginbottom D B, Slodička L, Colombe Y, Blatt R 2018 Phys. Rev. Lett. 120 193603
 Google Scholar
Google Scholar
[21] Onofrio R 2016 Can. J. Chem. 59 1129
[22] Anderson B P, Gustavson T L, Kasevich M A 1996 Phys. Rev. A 53 R3727
 Google Scholar
Google Scholar
[23] Anderson S E, Younge K C, Raithel G 2011 Phys. Rev. Lett. 107 263001
 Google Scholar
Google Scholar
[24] Lvovsky A I, Sanders B C, Tittel W 2009 Nat. Photonics 3 706
 Google Scholar
Google Scholar
[25] Bouwmeester D, Pan J-W, Mattle K, Eibl M, Weinfurter H, Zeilinger A 1997 Nature 390 575
 Google Scholar
Google Scholar
[26] Akram U, Ficek Z, Swain S 2000 Phys. Rev. A 62 013413
 Google Scholar
Google Scholar
[27] Motes K R, Mann R L, Olson J P, Studer N M, Bergeron E A, Gilchrist A, Dowling J P, Berry D W, Rohde P P 2016 Phys. Rev. A 94 012344
 Google Scholar
Google Scholar
[28] Norcia M A, Cline J R K, Muniz J A, Robinson J M, Hutson R B, Goban A, Marti G E, Ye J, Thompson J K 2018 Phys. Rev. X 8 021036
[29] Kim W J, Brownell J H, Onofrio R 2006 Phys. Rev. Lett. 96 200402
 Google Scholar
Google Scholar
[30] Georgescu I M, Ashhab S, Nori F 2014 Rev. Mod. Phys. 86 153
 Google Scholar
Google Scholar
[31] O'Brien J L, Furusawa A, Vučković J 2009 Nat. Photonics 3 687
 Google Scholar
Google Scholar
[32] Clemens J, Horvath L, Sanders B, Carmichael H 2003 Phys. Rev. A 68 023809
 Google Scholar
Google Scholar
[33] Gulfam Q-u-A, Ficek Z 2016 Phys. Rev. A 94 053831
 Google Scholar
Google Scholar
[34] Peng Z A, Chen A X, Li G X 2022 Phys. Rev. A 106 063702
 Google Scholar
Google Scholar
[35] Lembessis V E, Rsheed A A, Aldossary O M, Ficek Z 2013 Phys. Rev. A 88 053814
 Google Scholar
Google Scholar
[36] Chu X L, Angelopoulou V, Lodahl P, Rotenberg N 2022 Phys. Rev. A 106 053702
 Google Scholar
Google Scholar
[37] Loudon R, Knight P L 1987 J. Mod. Opt. 34 709
 Google Scholar
Google Scholar
[38] Afek I, Ambar O, Silberberg Y 2010 Science 328 879
 Google Scholar
Google Scholar
[39] Lichtenegger H C, Schoberl T, Bartl M H, Waite H, Stucky G D 2002 Science 298 389
 Google Scholar
Google Scholar
[40] Carmichael H J, Walls D F 1976 J. Phys. B: At. Mol. Phys. 9 1199
 Google Scholar
Google Scholar
[41] Kimble H, Dagenais M, Mandel L 1978 Phys. Rev. A 18 201
 Google Scholar
Google Scholar
[42] Sierra E, Masson S J, Asenjo-Garcia A 2022 Phys. Rev. Res. 4 023207
 Google Scholar
Google Scholar
[43] Masson S J, Ferrier-Barbut I, Orozco L A, Browaeys A, Asenjo-Garcia A 2020 Phys. Rev. Lett. 125 263601
 Google Scholar
Google Scholar
[44] Gulfam Q-u-A 2023 J. Mod. Opt. 70 217
 Google Scholar
Google Scholar
[45] Gulfam Q-u-A 2023 Phys. Lett. A 480 128966
 Google Scholar
Google Scholar
[46] Ficek Z, Tanaś R 2002 Phys. Rep. 372 369
 Google Scholar
Google Scholar
[47] Alaee R, Safari A, Sandoghdar V, Boyd R W 2020 Phys. Rev. Res. 2 043409
 Google Scholar
Google Scholar
[48] van Loo A F, Fedorov A, Lalumière K, Sanders B C, Blais A, Wallraff A 2013 Science 342 1494
 Google Scholar
Google Scholar
[49] Ben-Reuven A 1980 Phys. Rev. A 22 2572
 Google Scholar
Google Scholar
[50] Lembessis V E, Lyras A, Rsheed A A, Aldossary O M, Ficek Z 2015 Phys. Rev. A 92 023850
 Google Scholar
Google Scholar
[51] Gulfam Q-u-A, Ficek Z 2018 Phys. Rev. A 98 063824
 Google Scholar
Google Scholar
-
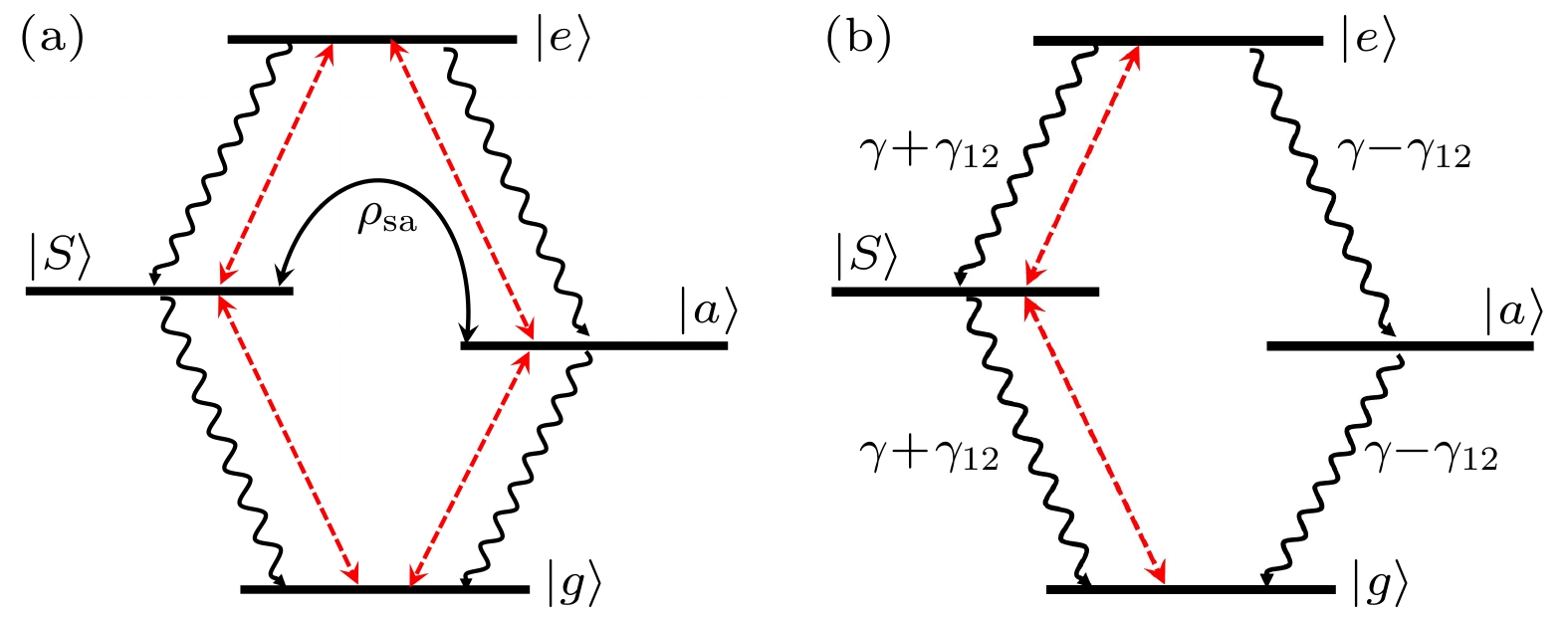
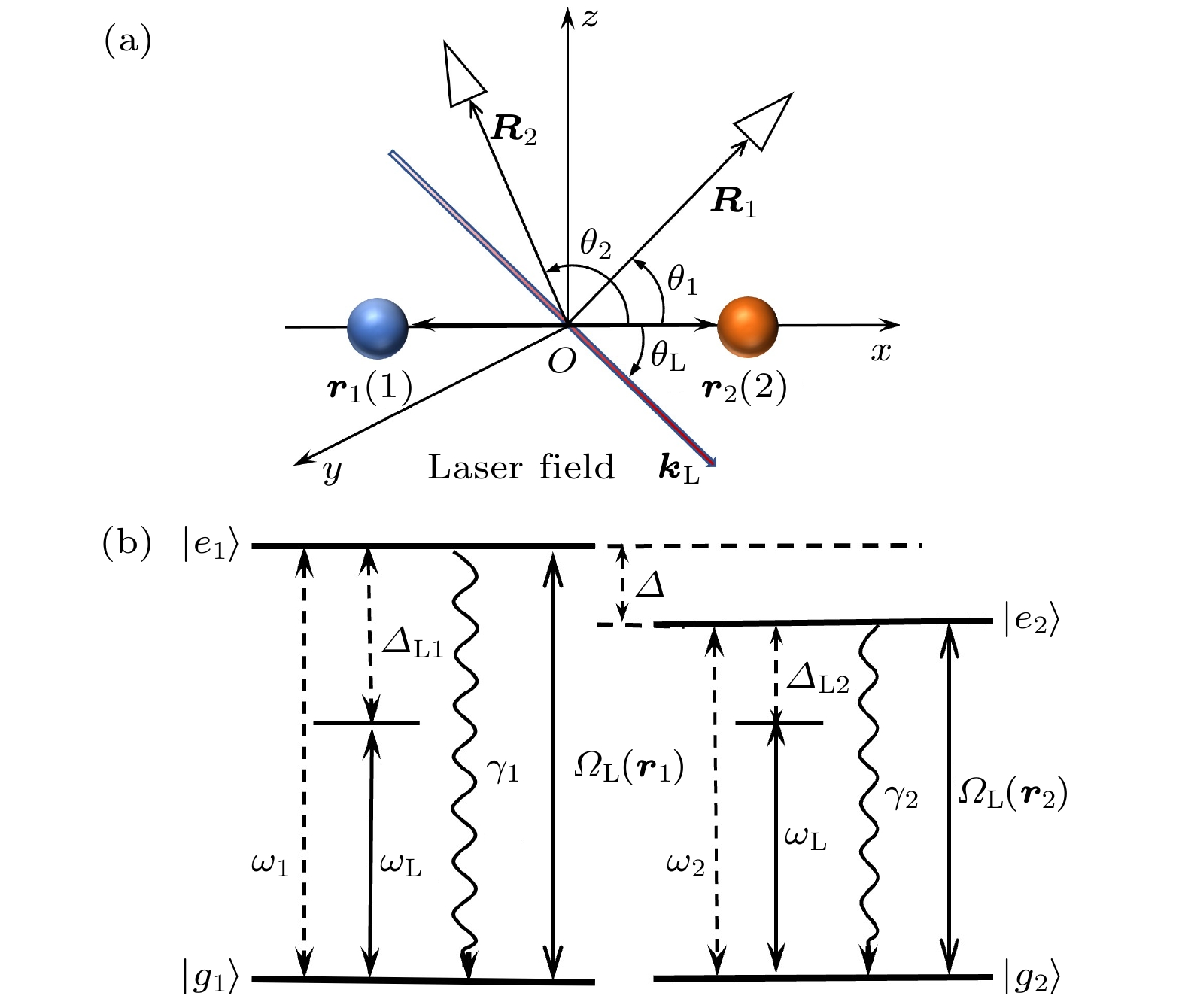
图 1 (a) 双原子系统的模型图, 两原子受到一个方向与${r_{12}}$成${\theta _{\text{L}}}$, 频率为${\omega _{\text{L}}}$的激光场驱动. 两个探测器分别位于${{\boldsymbol{R}}_1}$和${{\boldsymbol{R}}_2}$, 与原子轴成${\theta _1}$和${\theta _2}$处探测原子发射的光子; (b) 原子能级图, 显示了原子1, 2的跃迁频率${\omega _1}$和${\omega _2}$, 自发辐射速率${\gamma _1}$, ${\gamma _2}$, 以及激光耦合强度等
Fig. 1. (a) Schematic of the two-atom system, the atoms are driven by a laser field with frequency ${\omega _{\text{L}}}$ at an angle ${\theta _{\text{L}}}$ with respect to the interatomic vector ${r_{12}}$, detectors are placed at positions ${{\boldsymbol{R}}_1}$ and ${{\boldsymbol{R}}_2}$, detecting photons emitted by the atoms at angles ${\theta _1}$ and ${\theta _2}$ relative to the atomic axis; (b) presents the atomic energy level diagram, displaying the transition frequencies ${\omega _1}$ and ${\omega _2}$ of atoms 1 and 2, spontaneous emission rates ${\gamma _1}$ and ${\gamma _2}$, as well as laser coupling strengths.
图 2 对角化处理后两原子能级分布 (a) 非全同原子能级分布, 对称态与反对称态存在耦合; (b)全同原子能级分布, 激光只驱动对称态$\left| s \right\rangle $
Fig. 2. Energy level distribution of two atoms after diagonalization: (a) Non-identical atomic energy level distribution, with coupling between symmetric and antisymmetric states; (b) identical atomic energy level distribution, where the laser drives only the symmetric state $\left| s \right\rangle $.
图 4 全同原子能级布居分布, $\varDelta = 0$, ${\varDelta _{\text{L}}} = 0$, ${r_{12}} = 0.5\lambda $, ${\gamma _1} = {\gamma _2}$ (a) 原子能级布居随$\varOmega $的变化, 激光入${\theta _{\text{L}}} = {\text{π }}/2$; (b) 原子能级布居随激光入射角${\theta _{\text{L}}}$的变化, $\varOmega = 0.5\gamma $
Fig. 4. Energy level population distribution of identical atoms with $\varDelta = 0$, ${\varDelta _{\text{L}}} = 0$, ${r_{12}} = 0.5\lambda $, ${\gamma _1} = {\gamma _2}$: (a) The atomic energy level population as a function of $\varOmega $ with laser incidence at ${\theta _{\text{L}}} = {\text{π }}/2$; (b) the change in atomic energy level population with the laser incidence angle ${\theta _{\text{L}}}$, for $\varOmega = 0.5\gamma $.
图 5 非全同原子能级布居分布, ${\theta _{\text{L}}} = {\text{π }}/2$, $\varOmega = 0.5\gamma $, ${\omega _2} = $$ {\omega _{\text{L}}}$, ${r_{12}} = 0.5\lambda $ (a) 原子能级布居随$\varDelta $的变化, ${\gamma _1} = {\gamma _2}$; (b) 原子能级布居随耗散比${\gamma _1}/{\gamma _2}$的变化, $\varDelta = 0$
Fig. 5. Energy level population distribution for non-identical atoms with ${\theta _{\text{L}}} = {\text{π }}/2$, $\varOmega = 0.5\gamma $, ${\omega _2} = {\omega _{\text{L}}}$, and ${r_{12}} = 0.5\lambda $: (a) The variation of atomic energy level population with $\varDelta $, ${\gamma _1} = {\gamma _2}$; (b) the change in atomic energy level population with the dissipative ratio ${\gamma _1}/{\gamma _2}$, $\varDelta = 0$.
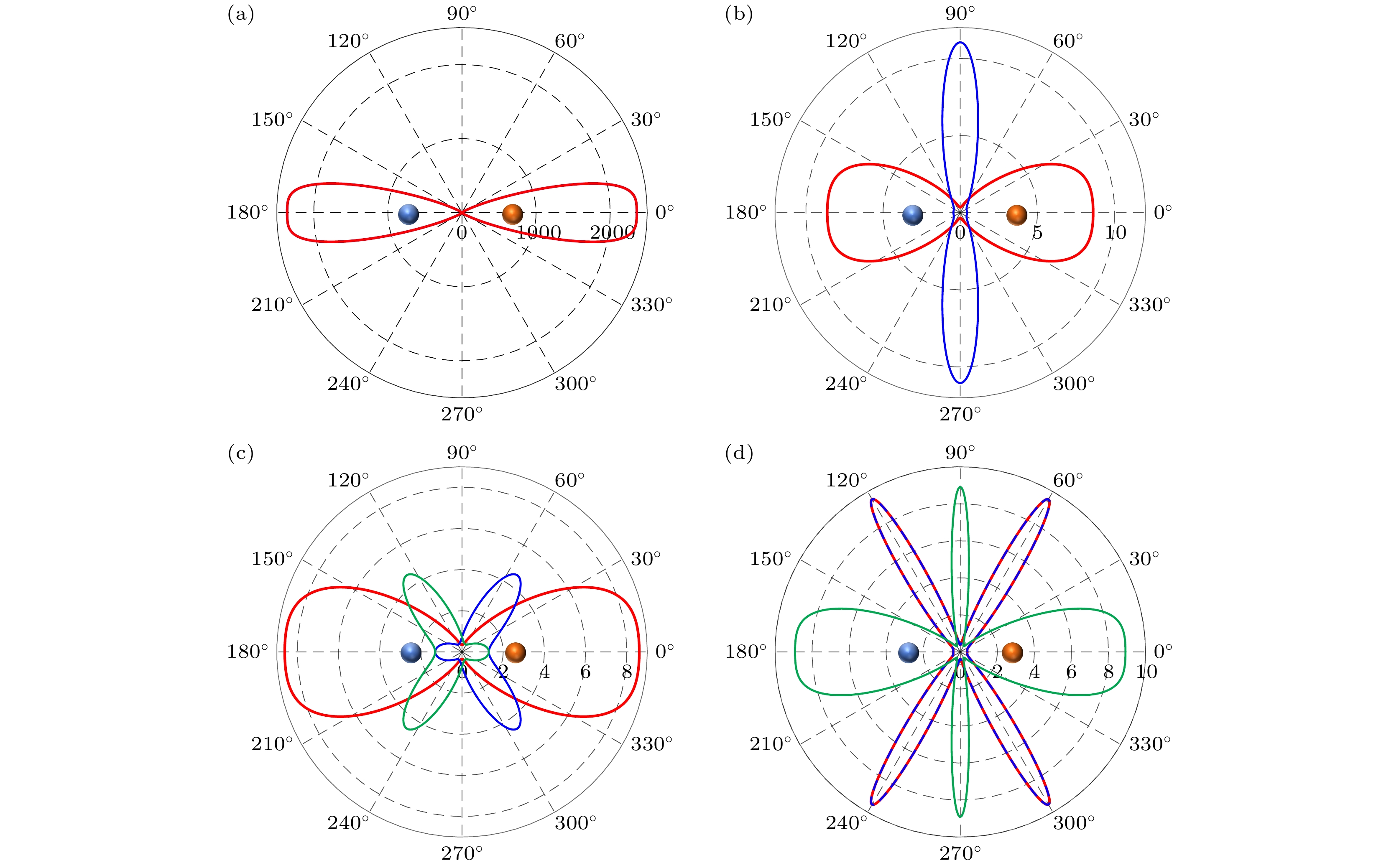
图 6 全同原子${g^{(2)}}({\boldsymbol{R}}, {\boldsymbol{R}})$关联值随探测角的分布, ${\varDelta _{\text{L}}} = 0$ (a) ${\theta _{\text{L}}} = {\text{π }}/2$, ${r_{12}} = 0.5\lambda $, $\varOmega = 0.1\gamma $; (b) 红线${\theta _{\text{L}}} = {\text{π }}/2$, 蓝线${\theta _{\text{L}}} = {\text{π }}$, ${r_{12}} = 0.5\lambda $, $\varOmega = 0.5\gamma $; (c) ${\theta _{\text{L}}} = {\text{π }}/2$红线, ${\theta _{\text{L}}} = {\text{π }}/3$蓝线, ${\theta _{\text{L}}} = 2{\text{π }}/3$绿线, ${r_{12}} = 0.5\lambda $, $\varOmega = 0.5\gamma $; (d) ${\theta _{\text{L}}} = {\text{π }}/2$红线, ${\theta _{\text{L}}} = {\text{π }}$蓝虚线, ${\theta _{\text{L}}} = {\text{π }}/3$绿线. ${r_{12}} = \lambda $, $\varOmega = 0.5\gamma $
Fig. 6. Distribution of the second-order correlation function ${g^{(2)}}({\boldsymbol{R}}, {\boldsymbol{R}})$ for identical atoms as a function of the detection angle with ${\varDelta _{\text{L}}} = 0$: (a) For ${\theta _{\text{L}}} = {\text{π }}/2$, ${r_{12}} = 0.5\lambda $, and $\varOmega = 0.1\gamma $; (b) for ${\theta _{\text{L}}} = {\text{π }}/2$ represented by the red line and ${\theta _{\text{L}}} = {\text{π }}$ by the blue line, with ${r_{12}} = 0.5\lambda $ and $\varOmega = 0.5\gamma $; (c) for ${\theta _{\text{L}}} = {\text{π }}/2$ shown as the red line, ${\theta _{\text{L}}} = {\text{π }}/3$ as the blue line, and ${\theta _{\text{L}}} = 2{\text{π }}/3$ as the green line, with ${r_{12}} = 0.5\lambda $ and $\varOmega = 0.5\gamma $; (d) for ${\theta _{\text{L}}} = {\text{π }}/2$ by the red solid line, ${\theta _{\text{L}}} = {\text{π }}$ by the blue dashed line, and ${\theta _{\text{L}}} = {\text{π }}/3$ as the green line, with ${r_{12}} = \lambda $ and $\varOmega = 0.5\gamma $.
图 7 非全同原子${g^{(2)}}({\boldsymbol{R}}, {\boldsymbol{R}})$关联值随探测角的分布, ${r_{12}} = 0.5\lambda $, $\varOmega = 0.5\gamma $ (a) ${\theta _{\text{L}}} = {\text{π }}/2$, ${\gamma _1} = {\gamma _2}$, ${\omega _{\text{L}}} = {\omega _2}$, 蓝线$\varDelta = 0.75\gamma $, 绿线$\varDelta = - 0.75\gamma $; (b) ${\theta _{\text{L}}} = {\text{π }}/2$, ${\gamma _1} = {\gamma _2}$, ${\omega _{\text{L}}} = {\omega _1}$, 蓝线$\varDelta = 0.75\gamma $, 绿线$\varDelta = - 0.75\gamma $; (c) ${\theta _{\text{L}}} = {\text{π }}$, ${\gamma _1} = {\gamma _2}$, ${\omega _{\text{L}}} = {\omega _1}$, 蓝线$\varDelta = 0.75\gamma $, 绿线$\varDelta = - 0.75\gamma $; (d) ${\theta _{\text{L}}} = {\text{π }}/2$, $\varDelta = 0$, ${\gamma _1} = {\gamma _2}$红线, ${\gamma _1} = 2{\gamma _2}$蓝线, ${\gamma _1} = 10{\gamma _2}$绿线
Fig. 7. Distribution of the second-order correlation value ${g^{(2)}}({\boldsymbol{R}}, {\boldsymbol{R}})$ for distinguishable atoms as a function of the detection angle with ${r_{12}} = 0.5\lambda $ and $\varOmega = 0.5\gamma $: (a) ${\theta _{\text{L}}} = {\text{π }}/2$, ${\gamma _1} = {\gamma _2}$, ${\omega _{\text{L}}} = {\omega _2}$, the blue line corresponds to $\varDelta = 0.75\gamma $ and the green line to $\varDelta = - 0.75\gamma $; (b) ${\theta _{\text{L}}} = {\text{π }}/2$, ${\gamma _1} = {\gamma _2}$, ${\omega _{\text{L}}} = {\omega _1}$, the blue line is for $\varDelta = 0.75\gamma $ and the green line for $\varDelta = - 0.75\gamma $; (c) ${\theta _{\text{L}}} = {\text{π }}$, ${\gamma _1} = {\gamma _2}$, ${\omega _{\text{L}}} = {\omega _1}$, the blue line is for $\varDelta = 0.75\gamma $ and the green line for $\varDelta = - 0.75\gamma $; (d) with ${\theta _{\text{L}}} = {\text{π }}/2$ and $\varDelta = 0$, the red line is for ${\gamma _1} = {\gamma _2}$, the blue line for ${\gamma _1} = 2{\gamma _2}$, and the green line for ${\gamma _1} = 10{\gamma _2}$.
-
[1] Saffman M 2016 J. Phys. B: At. Mol. Opt. Phys. 49 202001
 Google Scholar
Google Scholar
[2] Li N, Jiang H W, Xia X W, Zhu C J, Xie S Y, Xu J P, Yang Y P 2021 Phys. Lett. A 420 127772
 Google Scholar
Google Scholar
[3] Vilas N B, Hallas C, Anderegg L, Robichaud P, Winnicki A, Mitra D, Doyle J M 2022 Nature 606 70
 Google Scholar
Google Scholar
[4] Cidrim A, doEspiritoSanto T S, Schachenmayer J, Kaiser R, Bachelard R 2020 Phys. Rev. Lett. 125 073601
 Google Scholar
Google Scholar
[5] Moreno-Cardoner M, Plankensteiner D, Ostermann L, Chang D E, Ritsch H 2019 Phys. Rev. A 100 023806
 Google Scholar
Google Scholar
[6] Nelson K D, Li X, Weiss D S 2007 Nat. Phys. 3 556
 Google Scholar
Google Scholar
[7] Dicke R H 1954 Phys. Rev. 93 99
 Google Scholar
Google Scholar
[8] Goban A, Hung C L, Hood J D, Yu S P, Muniz J A, Painter O, Kimble H J 2015 Phys. Rev. Lett. 115 063601
 Google Scholar
Google Scholar
[9] Scully M O, Svidzinsky A A 2009 Science 325 1510
 Google Scholar
Google Scholar
[10] Hildred G P, Puri R R, Hassan S S, Bullough R K 1984 J. Phys. B: At. Mol. Phys. 17 L535
 Google Scholar
Google Scholar
[11] Corman L, Ville J L, Saint-Jalm R, Aidelsburger M, Bienaimé T, Nascimbène S, Dalibard J, Beugnon J 2017 Phys. Rev. A 96 053629
 Google Scholar
Google Scholar
[12] Rui J, Wei D, Rubio-Abadal A, Hollerith S, Zeiher J, Stamper-Kurn D M, Gross C, Bloch I 2020 Nature 583 369
 Google Scholar
Google Scholar
[13] Peng J S, Li G X 1993 Phys. Rev. A 47 4212
 Google Scholar
Google Scholar
[14] Richter S, Wolf S, von Zanthier J, Schmidt-Kaler F 2023 Phys. Rev. Res. 5 013163
 Google Scholar
Google Scholar
[15] Das S, Agarwal G S, Scully M O 2008 Phys. Rev. Lett. 101 153601
 Google Scholar
Google Scholar
[16] Downing C, Del Valle E, Fernández-Domínguez A I 2023 Phys. Rev. A 107 023717
 Google Scholar
Google Scholar
[17] Shegai T, Chen S, Miljković V D, Zengin G, Johansson P, Käll M 2011 Nat. Commun. 2 481
 Google Scholar
Google Scholar
[18] Vivas-Viaña A, Sánchez Muñoz C 2021 Phys. Rev. Res. 3 033136
 Google Scholar
Google Scholar
[19] Gulfam Q-u-A, Evers J 2010 J. Phys. B: At. Mol. Opt. Phys. 43 045501
 Google Scholar
Google Scholar
[20] Araneda G, Higginbottom D B, Slodička L, Colombe Y, Blatt R 2018 Phys. Rev. Lett. 120 193603
 Google Scholar
Google Scholar
[21] Onofrio R 2016 Can. J. Chem. 59 1129
[22] Anderson B P, Gustavson T L, Kasevich M A 1996 Phys. Rev. A 53 R3727
 Google Scholar
Google Scholar
[23] Anderson S E, Younge K C, Raithel G 2011 Phys. Rev. Lett. 107 263001
 Google Scholar
Google Scholar
[24] Lvovsky A I, Sanders B C, Tittel W 2009 Nat. Photonics 3 706
 Google Scholar
Google Scholar
[25] Bouwmeester D, Pan J-W, Mattle K, Eibl M, Weinfurter H, Zeilinger A 1997 Nature 390 575
 Google Scholar
Google Scholar
[26] Akram U, Ficek Z, Swain S 2000 Phys. Rev. A 62 013413
 Google Scholar
Google Scholar
[27] Motes K R, Mann R L, Olson J P, Studer N M, Bergeron E A, Gilchrist A, Dowling J P, Berry D W, Rohde P P 2016 Phys. Rev. A 94 012344
 Google Scholar
Google Scholar
[28] Norcia M A, Cline J R K, Muniz J A, Robinson J M, Hutson R B, Goban A, Marti G E, Ye J, Thompson J K 2018 Phys. Rev. X 8 021036
[29] Kim W J, Brownell J H, Onofrio R 2006 Phys. Rev. Lett. 96 200402
 Google Scholar
Google Scholar
[30] Georgescu I M, Ashhab S, Nori F 2014 Rev. Mod. Phys. 86 153
 Google Scholar
Google Scholar
[31] O'Brien J L, Furusawa A, Vučković J 2009 Nat. Photonics 3 687
 Google Scholar
Google Scholar
[32] Clemens J, Horvath L, Sanders B, Carmichael H 2003 Phys. Rev. A 68 023809
 Google Scholar
Google Scholar
[33] Gulfam Q-u-A, Ficek Z 2016 Phys. Rev. A 94 053831
 Google Scholar
Google Scholar
[34] Peng Z A, Chen A X, Li G X 2022 Phys. Rev. A 106 063702
 Google Scholar
Google Scholar
[35] Lembessis V E, Rsheed A A, Aldossary O M, Ficek Z 2013 Phys. Rev. A 88 053814
 Google Scholar
Google Scholar
[36] Chu X L, Angelopoulou V, Lodahl P, Rotenberg N 2022 Phys. Rev. A 106 053702
 Google Scholar
Google Scholar
[37] Loudon R, Knight P L 1987 J. Mod. Opt. 34 709
 Google Scholar
Google Scholar
[38] Afek I, Ambar O, Silberberg Y 2010 Science 328 879
 Google Scholar
Google Scholar
[39] Lichtenegger H C, Schoberl T, Bartl M H, Waite H, Stucky G D 2002 Science 298 389
 Google Scholar
Google Scholar
[40] Carmichael H J, Walls D F 1976 J. Phys. B: At. Mol. Phys. 9 1199
 Google Scholar
Google Scholar
[41] Kimble H, Dagenais M, Mandel L 1978 Phys. Rev. A 18 201
 Google Scholar
Google Scholar
[42] Sierra E, Masson S J, Asenjo-Garcia A 2022 Phys. Rev. Res. 4 023207
 Google Scholar
Google Scholar
[43] Masson S J, Ferrier-Barbut I, Orozco L A, Browaeys A, Asenjo-Garcia A 2020 Phys. Rev. Lett. 125 263601
 Google Scholar
Google Scholar
[44] Gulfam Q-u-A 2023 J. Mod. Opt. 70 217
 Google Scholar
Google Scholar
[45] Gulfam Q-u-A 2023 Phys. Lett. A 480 128966
 Google Scholar
Google Scholar
[46] Ficek Z, Tanaś R 2002 Phys. Rep. 372 369
 Google Scholar
Google Scholar
[47] Alaee R, Safari A, Sandoghdar V, Boyd R W 2020 Phys. Rev. Res. 2 043409
 Google Scholar
Google Scholar
[48] van Loo A F, Fedorov A, Lalumière K, Sanders B C, Blais A, Wallraff A 2013 Science 342 1494
 Google Scholar
Google Scholar
[49] Ben-Reuven A 1980 Phys. Rev. A 22 2572
 Google Scholar
Google Scholar
[50] Lembessis V E, Lyras A, Rsheed A A, Aldossary O M, Ficek Z 2015 Phys. Rev. A 92 023850
 Google Scholar
Google Scholar
[51] Gulfam Q-u-A, Ficek Z 2018 Phys. Rev. A 98 063824
 Google Scholar
Google Scholar
计量
- 文章访问数: 2204
- PDF下载量: 55
- 被引次数: 0













 下载:
下载: