-
Spin switching and spin transfer are essential prerequisites for designing the spin-logic devices based on endohedral fullerenes. In this paper by combining the theoreticalΛ-process model with a self-designed genetic algorithm, we are able to theoretically observe spin-switching and spin-transfer scenarios on the subpicosecond time scale in the endohedral fullerene Y2C2@C82-C2(1) from first principles. The results show that the geometry of the optimized enclosed Y2C2 cluster is consistent with the experimental data. There exists a certain repulsive force between the external C82-C2(1) cage and the encaged cluster. However, the whole system still maintains its integral cage structure due to the excellent stability of the fullerene. In the Y2C2@C82-C2(1) system, it is found that the spin density is highly localized on the two Y atoms and only minimally distributed on the carbon cage. By analyzing the spin-density distribution and the evolution of the spin expectation values as influenced by the laser pulses, it is found that global spin switching can be achieved on the two Y atoms, while spin transfer between the two Y atoms actually results from the redistribution of the spin density among the two magnetic centers and the carbon cage under the action of the optimized laser pulses. The achieved spin-switching scenario completes within about 1000 fs and its fidelity reaches 97.8%, while the obtained spin-transfer process completes within 200 fs and its fidelity reaches 95.1%. The electron absorption spectra of the system verify that optical transitions are possible between the main intermediate states and the initial and final states involved in the spin-switching and spin-transfer scenarios. Therefore, by analyzing the electron absorption spectra corresponding to the initial and final states, the energy of the laser pulses adopted for the studied spin-dynamics process can be predicted, and the spin transferability can be evaluated. In addition, it is found that the smaller the detuning between the required energy difference and the applied laser pulse energy is, the greater the probability for spin switching/transfer scenarios becomes. The present results reveal the mechanisms of the laser-induced ultrafast spin dynamics in Y2C2@C82-C2(1) and can provide a theoretical basis for designing the spin-logic devices on realistic endohedral fullerenes.
-
Keywords:
- endohedral fullerenes /
- Λ process /
- spin dynamics /
- first-principles method
[1] Li J L, Yang G W 2009 Appl. Phys. Lett. 95 085411
[2] Li J L, Yang G W 2009 J. Phys. Chem. C 113 18292
Google Scholar
[3] Wang J, Ma L, Liang Y, Gao M, Wang G 2014 J. Theor. Comput. Chem. 13 162
[4] Cox B J, Thamwattana N, Hill J M 2007 Proc. Math. Phys. Eng. Sci. 463 461
Google Scholar
[5] Li C, Liu J, Zhang S, Lefkidis G, Hübner W 2015 Carbon 87 153
Google Scholar
[6] Jin P, Hou Q, Tang C, Chen Z 2015 Theor. Chem. Acc. 134 1
Google Scholar
[7] Xiao Y, Zhu S E, Liu D J, Suzuki M, Lu X, Wang G W 2014 Angew. Chem. Int. Edit. 126 3050
Google Scholar
[8] Zhang N, Zhang Y, Yang M Q, Tang Z R, Xu Y J 2013 J. Catal. 299 210
Google Scholar
[9] Ren J M, Subbiah J, Zhang B, Ishitake K, Satoh K, Kamigaito M, Qiao G G, Wong E H, Wong W W 2016 Chem. Commun. 52 3356
Google Scholar
[10] Johnston H J, Hutchison G R, Christensen F M, Aschberger K, Stone V 2010 Toxicol. Sci. 114 162
Google Scholar
[11] Shu C, Corwin F D, Zhang J, Chen Z, Reid J E, Sun M, Xu W, Sim J H, Wang C, Fatouros P P 2009 Bioconjugate. Chem. 20 1186
Google Scholar
[12] Chai Y, Guo T, Jin C, Haufler R E, Chibante L P F, Fure J, Wang L, Alford J M, Smalley R E 1991 J. Phys. Chem. 95 557
[13] Wang C R, Kai T, Dr T T, Yoshida T, Dr Y K, Dr E N, Dr M T, Sakata M, Dr H S 2001 Angew. Chem. Int. Ed. Engl. 40 397
Google Scholar
[14] Chen N, Chaur M N, Moore C, Pinzón J R, Valencia R, Rodríguezfortea A, Poblet J M, Echegoyen L 2010 Chem. Commun. 46 4818
Google Scholar
[15] Li F F, Chen N, Muletgas M, Triana V, Murillo J, Rodríguezfortea A, Poblet J M, Echegoyen L 2013 Chem. Sci. 4 3404
Google Scholar
[16] Jin P, Tang C, Chen Z 2014 Coordin. Chem. Rev. 270-271 89
Google Scholar
[17] Dunsch L, Yang S, Zhang L, Svitova A, Oswald S, Popov A A 2010 J. Am. Chem. Soc. 132 5413
Google Scholar
[18] Harneit W 2002 Phys. Rev. A 65 184
[19] Benjamin S C, Ardavan A, Briggs G A D, Britz D A, Gunlycke D, Jefferson J, Jones M A G, Leigh D F, Lovett B W, Khlobystov A N 2005 J. Phys-Condens. Matt. 18 1599
[20] Ju C, Suter D, Du J 2011 Phys. Lett. A 375 1441
Google Scholar
[21] Beaurepaire E, Merle J, Daunois A, Bigot J 1996 Phys. Rev. Lett. 76 4250
Google Scholar
[22] Koopmans B, Ruigrok J J, Longa F D, de Jonge W J 2005 Phys. Rev. Lett. 95 267207
Google Scholar
[23] Bigot J Y, Vomir M, Beaurepaire E 2009 Nat. Phys. 5 515
Google Scholar
[24] Battiato M, Carva K, Oppeneer P M 2010 Phys. Rev. Lett. 105 027203
Google Scholar
[25] Stöhr J, Siegmann H C 2006 Magnetism-From Fundamentals to Nanoscale Dynamics (Berlin Heidelberg: Springer-Verlag) p753
[26] Li C, Liu J, Zhang S, Lefkidis G, Hübner W 2015 IEEE. T. Magn. 51 11
[27] Li C, Liu J, Lefkidis G, Hübner W 2017 Phys. Chem. Chem. Phys. 19 673
Google Scholar
[28] Jin F, Tamm N B, Troyanov S I, Yang S 2018 J. Am. Chem. Soc. 140 3496
Google Scholar
[29] 李春, 杨帆, Georgios Lefkidis, Wolfgang Hübner 2011 60 017802
Li C, Yang F, Lefkidis G, Hübner W 2011 Acta Phys. Sin. 60 017802
[30] Li C, Jin W, Xiang H, Lefkidis G, Hübner W 2011 Phys. Rev. B 84 2250
[31] 李春, 张少斌, 金蔚, Georgios Lefkidis, Wolfgang Hübner 2012 61 177502
Google Scholar
Li C, Zhang S B, Jin W, Lefkidis G, Hubner W 2012 Acta Phys. Sin. 61 177502
Google Scholar
[32] Zhang N, Du H, Chang J, Jin W, Li C, Lefkidis G, Hubner W 2018 Phys. Rev. B 98 104431
Google Scholar
[33] Frisch M J, Trucks G W, Schlegel H B, Scuseria G E, Robb M A, Cheeseman J R 2009 Gaussian 09, Revision A.1. (Wallingford: Gaussian Inc.)
[34] Nakatsuji H 1979 Chem. Phys. Lett. 67 329
Google Scholar
[35] Hay P J 1985 J. Chem. Phys. 82 299
Google Scholar
-
图 1 双磁性中心内嵌富勒烯Y2C2@C82-C2(1)体系 (a) 内嵌富勒烯Y2C2@C82C2(1); (b) 内嵌的双金属碳化物团簇(Y2C2)
Figure 1. Double-magnetic-center endohedral fullerene Y2C2@C82-C2(1) system: (a) Endohedral fullerene Y2C2@C82C2(1); (b) endohedral bimetallic carbide clusters (Y2C2).
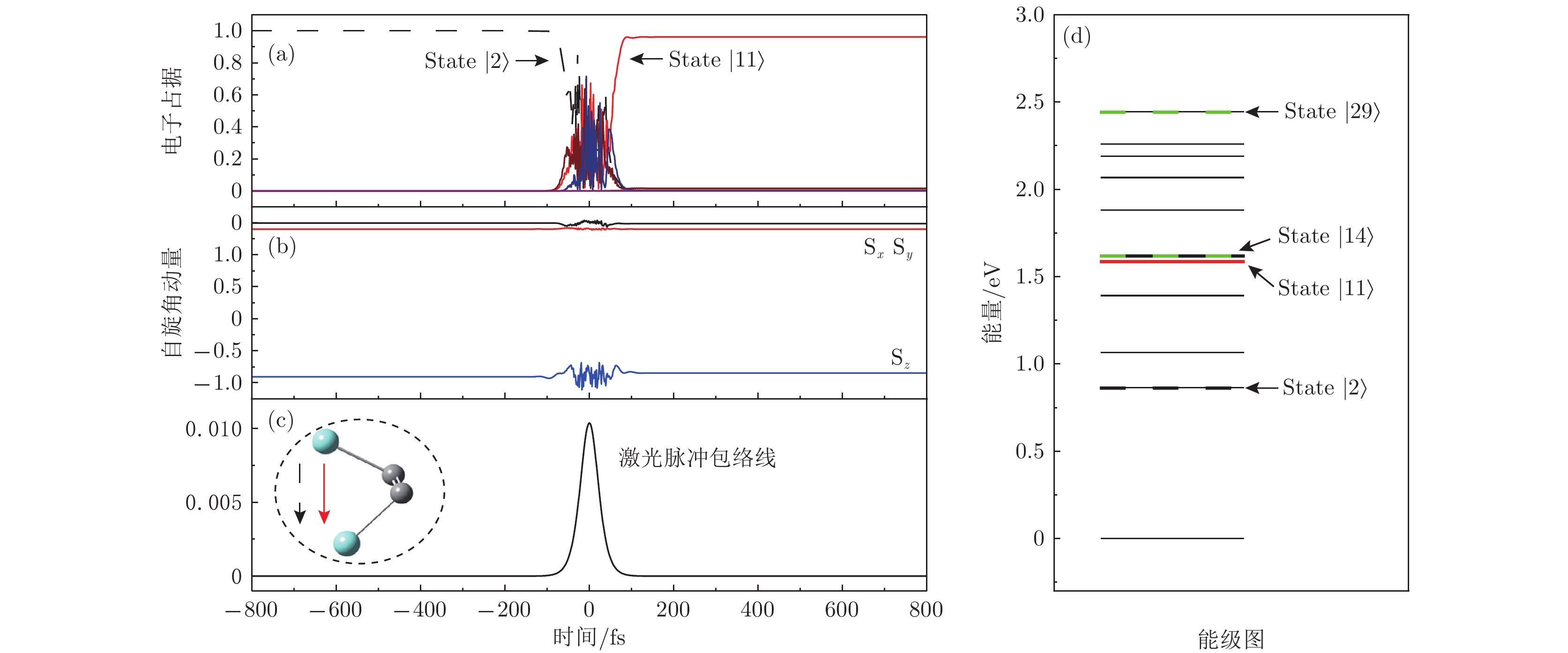
图 2 激光脉冲作用下, Y2C2@C82-C2(1)体系中的超快自旋翻转过程 (a) 自旋翻转过程中所涉及的初始态(黑色虚线)、最终态(红色实线)和中间态(彩色实线)随时间变化的占据情况; (b) 自旋角动量分量的期望值随时间的变化情况; (c) 实现自旋翻转所用的激光脉冲包络线, 插图为结构优化后的内嵌Y2C2团簇, 碳笼用虚线表示; (d)SAC-CI计算得到的能级图(考虑SOC)
Figure 2. Ultrafast spin-switching scenario achieved in Y2C2@C82-C2(1) system under laser pulses: (a) Time evolution of the occupation of the initial state (dashed black line), final state (solid red line), and the intermediate states (colorized solid lines) involved in spin-switching scenario; (b) variation of the expectation values of the spin angular momentum components along the x, y and z axis with time; (c) laser pulse envelope of the spin-switching scenario, inset represents structurally optimized endohedral Y2C2 cluster, the dashed circle represents the carbon cage; (d)energy levels of Y2C2@C82-C2(1) calculated by SAC-CI (including SOC).
图 3 激光脉冲作用下, Y2C2@C82-C2(1)体系中的超快自旋转移过程 (a) 自旋转移过程中所涉及的初始态(黑色虚线)、最终态(红色实线)和中间态(彩色实线)随时间变化的占据情况; (b) 自旋角动量分量的期望值随时间的变化情况; (c) 实现自旋转移所用的激光脉冲包络线, 插图为结构优化后的内嵌Y2C2团簇, 碳笼用虚线表示; (d)SAC-CI计算得到的能级图(考虑SOC)
Figure 3. Ultrafast spin-transfer scenario achieved in Y2C2@C82-C2(1) system under laser pulses: (a) Time evolution of the occupation of the initial state (dashed black line), final state (solid red line), and the intermediate states (colorized solid lines) involved in spin-transfer scenario; (b) variation of the expectation values of the spin angular momentum components along the x, y and z axis with time; (c) laser pulse envelope of the spin-transfer scenario, inset represents structurally optimized endohedral Y2C2 cluster, the dashed circle represents the carbon cage; (d) energy levels of Y2C2@C82-C2(1) calculated by SAC-CI (including SOC).
图 4 Y2C2@C82-C2(1)体系自旋翻转和自旋转移过程中初始态
$\left| 2 \right\rangle $ 和最终态$\left| 11 \right\rangle $ 的电子吸收光谱图Figure 4. Electronic absorption spectra of the initial (state
$\left| 2 \right\rangle $ ) and final (state$\left| 11 \right\rangle $ ) state of the spin-switching and spin-transfer scenarios in Y2C2@C82-C2(1).表 1 Y2C2@C82-C2(1)体系中能量最低的五个三重态的自旋密度分布情况(未考虑SOC)
Table 1. Spin density distributions of the five lowest triplet states in Y2C2@C82-C2(1) system (without SOC).
Atom State 1 State 2 State 3 State 4 State 5 Y1 0.448 0.479 0.125 0.368 0.198 Y2 0.606 0.831 0.148 0.801 0.173 C (max) 0.058 0.046 0.059 0.042 0.066 
DownLoad: CSV
表 2 自旋翻转过程涉及能态的局域化位置、能量和自旋期望值(考虑SOC)
Table 2. Localization positions, energies and spin expectation values of the involved states in spin-switching scenario (including SOC).
State Spin localization Energy 〈Sx〉 〈Sy〉 〈Sz〉 〈S〉 eV 31 2.444 0.001 0.001 0.918 0.918 30 2.443 −0.003 −0.003 0.000 0.004 29 2.443 0.002 0.001 −0.918 0.918 4 Y1 = Y2 0.863 −0.001 −0.043 0.962 0.962 3 0.862 0.002 0.083 0.000 0.083 2 Y1 = Y2 0.862 −0.001 −0.040 −0.962 0.963
DownLoad: CSV
表 3 Y2C2@C82-C2(1)体系中自旋密度分布情况(考虑SOC)
Table 3. Spin density distribution in Y2C2@C82-C2(1) system (including SOC).
Energy/eV Y1 Y2 C (max) Total spin density (C atom) State $\left| 2 \right\rangle $ 0.862 −0.504 −0.680 −0.016 −1.062 State $\left| 11 \right\rangle $ 1.586 −0.414 −0.900 −0.032 −0.933 Difference value 0.724 0.090 −0.220 — 0.129
DownLoad: CSV
-
[1] Li J L, Yang G W 2009 Appl. Phys. Lett. 95 085411
[2] Li J L, Yang G W 2009 J. Phys. Chem. C 113 18292
Google Scholar
[3] Wang J, Ma L, Liang Y, Gao M, Wang G 2014 J. Theor. Comput. Chem. 13 162
[4] Cox B J, Thamwattana N, Hill J M 2007 Proc. Math. Phys. Eng. Sci. 463 461
Google Scholar
[5] Li C, Liu J, Zhang S, Lefkidis G, Hübner W 2015 Carbon 87 153
Google Scholar
[6] Jin P, Hou Q, Tang C, Chen Z 2015 Theor. Chem. Acc. 134 1
Google Scholar
[7] Xiao Y, Zhu S E, Liu D J, Suzuki M, Lu X, Wang G W 2014 Angew. Chem. Int. Edit. 126 3050
Google Scholar
[8] Zhang N, Zhang Y, Yang M Q, Tang Z R, Xu Y J 2013 J. Catal. 299 210
Google Scholar
[9] Ren J M, Subbiah J, Zhang B, Ishitake K, Satoh K, Kamigaito M, Qiao G G, Wong E H, Wong W W 2016 Chem. Commun. 52 3356
Google Scholar
[10] Johnston H J, Hutchison G R, Christensen F M, Aschberger K, Stone V 2010 Toxicol. Sci. 114 162
Google Scholar
[11] Shu C, Corwin F D, Zhang J, Chen Z, Reid J E, Sun M, Xu W, Sim J H, Wang C, Fatouros P P 2009 Bioconjugate. Chem. 20 1186
Google Scholar
[12] Chai Y, Guo T, Jin C, Haufler R E, Chibante L P F, Fure J, Wang L, Alford J M, Smalley R E 1991 J. Phys. Chem. 95 557
[13] Wang C R, Kai T, Dr T T, Yoshida T, Dr Y K, Dr E N, Dr M T, Sakata M, Dr H S 2001 Angew. Chem. Int. Ed. Engl. 40 397
Google Scholar
[14] Chen N, Chaur M N, Moore C, Pinzón J R, Valencia R, Rodríguezfortea A, Poblet J M, Echegoyen L 2010 Chem. Commun. 46 4818
Google Scholar
[15] Li F F, Chen N, Muletgas M, Triana V, Murillo J, Rodríguezfortea A, Poblet J M, Echegoyen L 2013 Chem. Sci. 4 3404
Google Scholar
[16] Jin P, Tang C, Chen Z 2014 Coordin. Chem. Rev. 270-271 89
Google Scholar
[17] Dunsch L, Yang S, Zhang L, Svitova A, Oswald S, Popov A A 2010 J. Am. Chem. Soc. 132 5413
Google Scholar
[18] Harneit W 2002 Phys. Rev. A 65 184
[19] Benjamin S C, Ardavan A, Briggs G A D, Britz D A, Gunlycke D, Jefferson J, Jones M A G, Leigh D F, Lovett B W, Khlobystov A N 2005 J. Phys-Condens. Matt. 18 1599
[20] Ju C, Suter D, Du J 2011 Phys. Lett. A 375 1441
Google Scholar
[21] Beaurepaire E, Merle J, Daunois A, Bigot J 1996 Phys. Rev. Lett. 76 4250
Google Scholar
[22] Koopmans B, Ruigrok J J, Longa F D, de Jonge W J 2005 Phys. Rev. Lett. 95 267207
Google Scholar
[23] Bigot J Y, Vomir M, Beaurepaire E 2009 Nat. Phys. 5 515
Google Scholar
[24] Battiato M, Carva K, Oppeneer P M 2010 Phys. Rev. Lett. 105 027203
Google Scholar
[25] Stöhr J, Siegmann H C 2006 Magnetism-From Fundamentals to Nanoscale Dynamics (Berlin Heidelberg: Springer-Verlag) p753
[26] Li C, Liu J, Zhang S, Lefkidis G, Hübner W 2015 IEEE. T. Magn. 51 11
[27] Li C, Liu J, Lefkidis G, Hübner W 2017 Phys. Chem. Chem. Phys. 19 673
Google Scholar
[28] Jin F, Tamm N B, Troyanov S I, Yang S 2018 J. Am. Chem. Soc. 140 3496
Google Scholar
[29] 李春, 杨帆, Georgios Lefkidis, Wolfgang Hübner 2011 60 017802
Li C, Yang F, Lefkidis G, Hübner W 2011 Acta Phys. Sin. 60 017802
[30] Li C, Jin W, Xiang H, Lefkidis G, Hübner W 2011 Phys. Rev. B 84 2250
[31] 李春, 张少斌, 金蔚, Georgios Lefkidis, Wolfgang Hübner 2012 61 177502
Google Scholar
Li C, Zhang S B, Jin W, Lefkidis G, Hubner W 2012 Acta Phys. Sin. 61 177502
Google Scholar
[32] Zhang N, Du H, Chang J, Jin W, Li C, Lefkidis G, Hubner W 2018 Phys. Rev. B 98 104431
Google Scholar
[33] Frisch M J, Trucks G W, Schlegel H B, Scuseria G E, Robb M A, Cheeseman J R 2009 Gaussian 09, Revision A.1. (Wallingford: Gaussian Inc.)
[34] Nakatsuji H 1979 Chem. Phys. Lett. 67 329
Google Scholar
[35] Hay P J 1985 J. Chem. Phys. 82 299
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 8009
- PDF Downloads: 50
- Cited By: 0
