Spin noise spectroscopy of cesium vapor in micron-scale cell

Guo Zhi-Chao; Zhang Tong-Yao; Zhang Jing

doi:10.7498/aps.69.20191623

Abstract

In this paper, the spin dynamics and broadening mechanism of cesium vapor in cells without buffer gas is investigated by means of spin noise spectroscopy. In a macro atomic vapor cell, the lineshape of detuning frequency spectrum of spin relaxation rate is of Gaussian distribution. For a micron-scaled vapor cell with strong spatial locality, the lineshape of detuning frequency spectrum of spin relaxation rate is of Lorentzian distribution. The parameter dependence of detuning frequency spectrum of spin relaxation rate, such as temperature, is studied quantitatively. The detuning frequency spectrum of the spin relaxation rate is measured experimentally to be broadened by $ \sim $4 GHz, which is obviously larger than the unhomogeneous Doppler broadening of $ \sim $500 MHz for a macro atomic vapor cell. At the same time, the detuning frequency spectrum of total noise in the two atomic vapor cells is studied. In the macro atomic vapor cell, the total noise intensity strongly relies on the detuning frequency of the laser with respect to the atomic resonance transition. In the micron-scaled vapor cell, due to the strong homogeneous broadening, the center of the detuning frequency spectrum of the total noise is observed to dip. Finally, a simplified physical model is established to compute the broadening of the micron-scaled vapor cell. The homogeneous broadening of atoms is explained experimentally and theoretically in the micron-scaled vapor cell.

Keywords:

Authors and contacts

1.
State Key Laboratory of Quantum Optics and Quantum Optics Devices, Institute of Opto-Electronics, Shanxi University, Taiyuan 030006, China
2.
Collaborative Innovation Center of Extreme Optics, Shanxi University, Taiyuan 030006, China

Corresponding author: Guo Zhi-Chao, sxdxguozhichao@gmail.com

Funds: Supported by the National Key Research and Development Program of China (Grant No. 2016 YFA0301602) and the National Natural Science Foundation of China (Grant No. 61574087)

FullText HTML : translate this paragraph

References

[1]	Kubo R 1966 Rep. Prog. Phys. 29 255 Google Scholar
[2]	Römer M, Hübner J, Oestreich M 2007 Rev. Sci. Instrum. 78 103903 Google Scholar
[3]	Sørensen J L, Hald J, Polzik E S 1998 Phys Rev. Lett. 80 3487 Google Scholar
[4]	Crooker S A, Rickel D G, Balatsky A V, Smith D L 2004 Nature 431 49 Google Scholar
[5]	Oestreich M, Römer M, Haug R J, Hägele D 2005 Phys. Rev. Lett. 95 216603 Google Scholar
[6]	Sterin P, Wiegand J, Hübner Jens, Oestreich M 2018 Phys. Rev. Appl. 9 034003 Google Scholar
[7]	Lucivero V G, Jiménez-Martínez Ricardo, Kong J, Mitchell M W 2016 Phys. Rev. A. 93 053802 Google Scholar
[8]	史平, 马健, 钱轩, 姬杨, 李伟 2017 66 017201 Google Scholar Shi P, Ma J, Qian X, Ji Y, Li W 2017 Acta Phys. Sin. 66 017201 Google Scholar
[9]	Roy D, Yang L, Crooker S A, Sinitsyn N A 2015 Sci. Rep. 5 9573 Google Scholar
[10]	Petrov M Y, Kamenskii A N, Zapasskii V S, Bayer M, Greilich A 2018 Phys. Rev. B 97 125202 Google Scholar
[11]	Cronenberger S, Scalbert D 2016 Rev. Sci. Instrum. 87 093111 Google Scholar
[12]	李晨, 丁畅, 张桐耀, 曹丹华, 吴裕斌, 陈院森 2017 量子光学学报 23 228 Li C, Ding C, Zhang T Y, Cao D H, Wu Y B, Chen Y S 2017 J. Quant. Opt. 23 228
[13]	Müller G M, Römer M, Hübner J, Oestreich M 2010 App. Phys. Lett. 97 192109 Google Scholar
[14]	Poltavtsev S V, Ryzhov I I, Glazov M M, Kozlov G G, Zapasskii V S, Kavokin A V, Lagoudakis P G, Smirnov D S, Ivchenko E L 2014 Phys. Rev. B 89 081304 Google Scholar
[15]	Pershin Y V, Slipko V A, Roy D, Sinitsyn N A 2013 Appl. Phys. Lett. 102 202405 Google Scholar
[16]	Dahbashi R, Hübner J, Berski F, Pierz K, Oestreich M 2014 Phys. Rev. Lett. 112 156601 Google Scholar
[17]	Yang L, Glasenapp P, Greilich A, Reuter D, Wieck A D, Yakovlev D R, Bayer M, Crooker S A 2014 Nat. Commun. 5 4949 Google Scholar
[18]	Crooker S A, Brandt J, Sandfort C, Greilich A, Yakovlev D R, Reuter D, Wieck A D, Bayer M 2010 Phys. Rev. Lett. 104 036601 Google Scholar
[19]	Kozlov G G, Ryzhov I I, Zapasskii V S 2017 Phys. Rev. A 95 043810 Google Scholar
[20]	Kozlov G G, Ryzhov I I, Zapasskii V S 2018 Phys. Rev. A 97 013848 Google Scholar
[21]	Keaveney J 2013 Ph. D. Dissertation (Durham: Durham University)
[22]	Ma J, Shi P, Qian X, Li W, Ji Y 2016 Chin. Phys. B 25 117203 Google Scholar
[23]	Hübner J, Berski F, Dahbashi R, Oestreich M 2014 Phys. Status. Solidi. 251 1824 Google Scholar
[24]	Petrov M Y, Ryzhov I I, Smirnov D S, Belyaev L Y, Potekhin R A, Glazov M M, Kulyasov V N, Kozlov G G, Aleksandrov E B, Zapasskii1 V S 2018 Phys. Rev. A 97 032502 Google Scholar
[25]	Zapasskii V S, Greilich A, Crooker S A, Li Y, Kozlov G G, Yakovlev D R, Reuter D, Wieck A D, Bayer M 2013 Phys. Rev. Lett. 110 176601 Google Scholar
[26]	Ghosh R K 2009 Ph. D. Dissertation (Princeton: Princeton University)
[27]	Rajroop J 2018 Ph. D. Dissertation (London: University College London)
[28]	Christopher J F 2005 Atomic Physics (Oxford: Oxford University Press) p142
[29]	Ma J, Shi P, Qian X, Shang Y, Ji Y 2017 Sci. Rep. 7 10238 Google Scholar
[30]	Buckingham A D, Stephens P J 1966 Annu. Rev. Phys. Chem. 17 399 Google Scholar
[31]	Zapasskii V S 2013 Adv. Opt. Photon. 5 131 Google Scholar
[32]	尚雅轩, 马健, 史平, 钱轩, 李伟, 姬杨 2018 67 087201 Google Scholar Shang Y X, Ma J, Shi P, Qian X, Li W, Ji Y 2018 Acta Phys. Sin. 67 087201 Google Scholar
[33]	Chalupczak W, Godun R M 2011 Phys. Rev. A. 83 032512 Google Scholar
[34]	Zhang W J, You L X, Li H, Huang J, Lv C L, Zhang L, Liu X Y, Wu J J, Wang Z, Xie X M 2017 Sci: China Phys. Mech. Astron. 60 120314 Google Scholar
[35]	Yang W H, Shi S P, Wang Y J, Ma W G, Zheng Y H, Peng K C 2017 Opt. Lett. 42 21 Google Scholar

Cited By

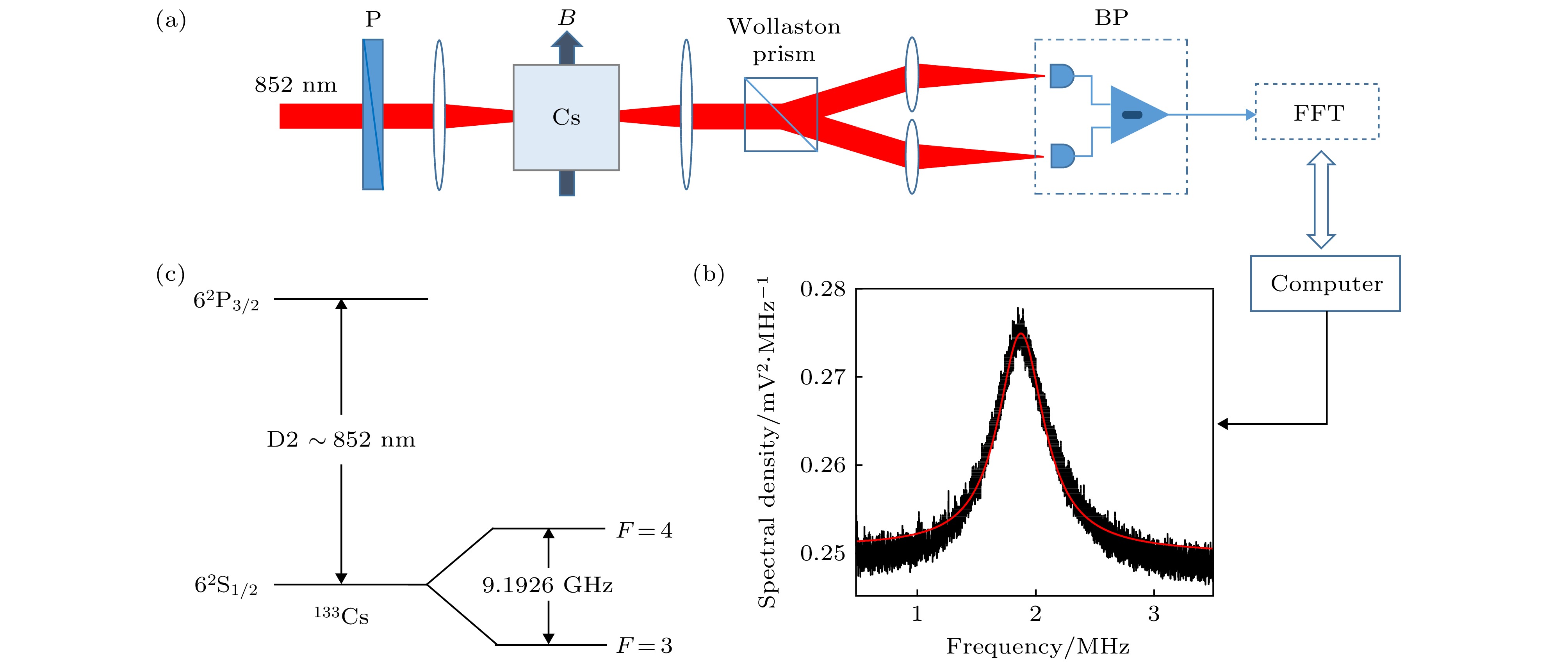
图 1 (a)自旋噪声谱的实验原理图(P是偏振片, B是外加磁场, Wollaston prism是沃拉斯顿棱镜, BP是平衡零拍探测器, FFT是快速傅里叶变换); (b)气室1中经典的¹³³Cs自旋噪声谱(激光失谐频率Ω_D2 = + 600 MHz于D2线(6²S_1/2 (F = 4) → 6²P_3/2), 原子气室温度T = 296 K, 激光功率P = 500 μW, 外加磁场B = 5 G); (c) ¹³³Cs的D2跃迁线和基态超精细结构

Figure 1. (a) Schematic of the experimental apparatus (P-polarizer, B-magnetic fields, BP-balanced homodyne detector, FFT-fast Fourier transform); (b) typical spin noise spectrum of ¹³³Cs in cell 1 (the laser is detuned Ω_D2 = + 600 MHz from the D2 transition (6²S_1/2 (F = 4) → 6²P_3/2). The temperature of atomic cell is 296 K. The laser power P = 500 μW. Magnetic field B = 5 G); (c) D2 line transition and ground-state hyperfine structure of ¹³³Cs.

DownLoad: Full-Size Img PowerPoint

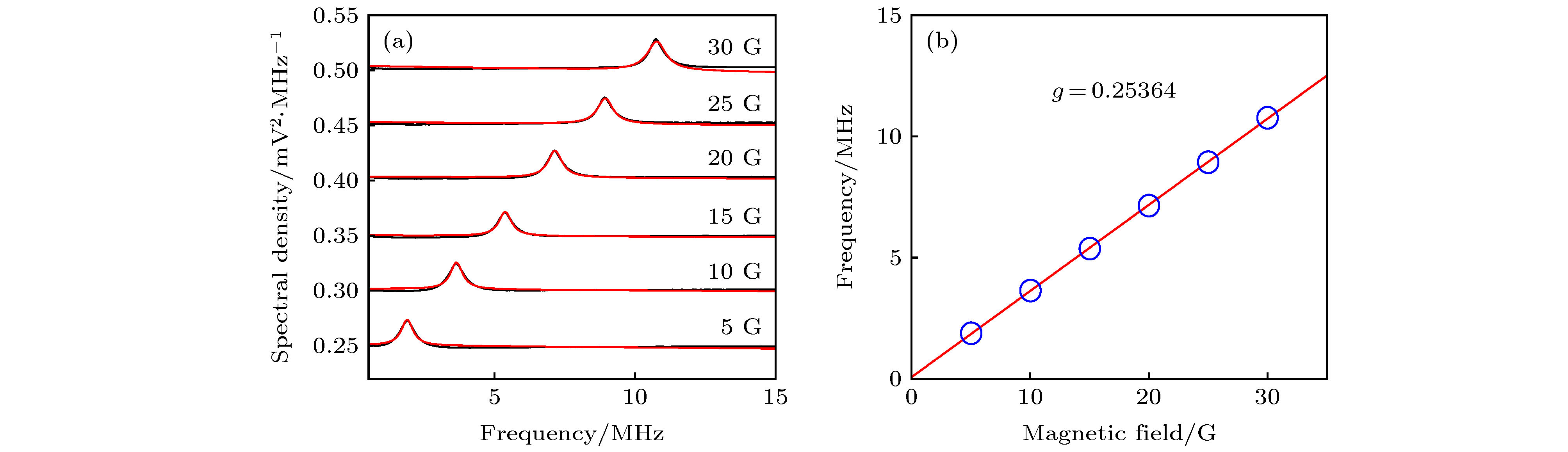
图 2 气室1中磁场相关的自旋噪声谱　(a)不同磁场下的自旋噪声谱(黑线)和拟合曲线(红线); (b)自旋噪声谱中心频率(蓝圆圈)与外场关系图, 以及拟合曲线(红线); 激光失谐频率Ω_D2 = + 600 MHz于D2线(6²S_1/2 (F = 4) → 6²P_3/2), 激光功率为P = 500 μW, 原子气室温度T = 296 K

Figure 2. Magnetic-field dependent spin noise spectrum in cell 1: (a) Spin noise spectrum (black lines) and fitting curve (red lines) versus the magnetic fields; (b) dependence of center frequency of spin noise spectrum on magnetic fields. The blue circles are the experimental data. The red line is the fitting curve. The laser is detuned Ω_D2 = + 600 MHz from the D2 transition (6²S_1/2(F = 4) → 6²P_3/2). The laser power P = 500 μW. The temperature of atomic cell is 296 K.

DownLoad: Full-Size Img PowerPoint

图 3 气室1中自旋噪声谱的失谐频率依赖关系　(a)自旋弛豫速率与失谐频率的对应关系图; (b)总噪声与失谐频率的对应关系图; 激光失谐频率在D2线(6²S_1/2(F = 3) → 6²P_3/2)附近, 激光功率P = 500 μW, 外磁场B = 15 G, 实验数据为(蓝圆圈)和拟合曲线(红线), 原子气室温度T = 296 K

Figure 3. Detuning frequency dependent spin noise spectrum in cell 1: (a) Spin relaxation rate versus the detuning frequency; (b) total noise versus the detuning frequency. The laser is detuned from the D2 transition (6²S_1/2(F = 3) → 6²P_3/2). The laser power P = 500 μW. Magnetic field B = 15 G. The blue circles are the experimental data. The red line is the fitting curve. The temperature of atomic cell is 296 K.

DownLoad: Full-Size Img PowerPoint

图 4 气室2中自旋噪声谱的失谐频率依赖关系　(a) T = 387 K时自旋弛豫速率与失谐频率的关系; (b) T = 431 K时自旋弛豫速率与失谐频率的关系; (c) T = 387 K时总噪声与失谐频率的关系; (d) T = 431 K时总噪声与失谐频率的关系; 激光失谐频率在D2线(6²S_1/2(F = 3) → ²P_3/2)附近, 激光功率为P = 5 mW, 蓝圆圈表示实验数据, 红线为拟合曲线

Figure 4. The detuning frequency dependent spin noise spectrum in cell 2: (a) Spin relaxation rate versus the detuning frequency at T = 387 K; (b) spin relaxation rate versus the detuning frequency at T = 431 K; (c) total noise versus the detuning frequency at T = 387 K; (d) total noise versus the detuning frequency at T = 431 K. The laser is detuned from the D2 transition (6²S_1/2(F = 3) → 6²P_3/2). The laser power P = 5 mW. The blue circles are the experimental data, and the red line is the fitting curve.

DownLoad: Full-Size Img PowerPoint

map

[1]	Kubo R 1966 Rep. Prog. Phys. 29 255 Google Scholar
[2]	Römer M, Hübner J, Oestreich M 2007 Rev. Sci. Instrum. 78 103903 Google Scholar
[3]	Sørensen J L, Hald J, Polzik E S 1998 Phys Rev. Lett. 80 3487 Google Scholar
[4]	Crooker S A, Rickel D G, Balatsky A V, Smith D L 2004 Nature 431 49 Google Scholar
[5]	Oestreich M, Römer M, Haug R J, Hägele D 2005 Phys. Rev. Lett. 95 216603 Google Scholar
[6]	Sterin P, Wiegand J, Hübner Jens, Oestreich M 2018 Phys. Rev. Appl. 9 034003 Google Scholar
[7]	Lucivero V G, Jiménez-Martínez Ricardo, Kong J, Mitchell M W 2016 Phys. Rev. A. 93 053802 Google Scholar
[8]	史平, 马健, 钱轩, 姬杨, 李伟 2017 66 017201 Google Scholar Shi P, Ma J, Qian X, Ji Y, Li W 2017 Acta Phys. Sin. 66 017201 Google Scholar
[9]	Roy D, Yang L, Crooker S A, Sinitsyn N A 2015 Sci. Rep. 5 9573 Google Scholar
[10]	Petrov M Y, Kamenskii A N, Zapasskii V S, Bayer M, Greilich A 2018 Phys. Rev. B 97 125202 Google Scholar
[11]	Cronenberger S, Scalbert D 2016 Rev. Sci. Instrum. 87 093111 Google Scholar
[12]	李晨, 丁畅, 张桐耀, 曹丹华, 吴裕斌, 陈院森 2017 量子光学学报 23 228 Li C, Ding C, Zhang T Y, Cao D H, Wu Y B, Chen Y S 2017 J. Quant. Opt. 23 228
[13]	Müller G M, Römer M, Hübner J, Oestreich M 2010 App. Phys. Lett. 97 192109 Google Scholar
[14]	Poltavtsev S V, Ryzhov I I, Glazov M M, Kozlov G G, Zapasskii V S, Kavokin A V, Lagoudakis P G, Smirnov D S, Ivchenko E L 2014 Phys. Rev. B 89 081304 Google Scholar
[15]	Pershin Y V, Slipko V A, Roy D, Sinitsyn N A 2013 Appl. Phys. Lett. 102 202405 Google Scholar
[16]	Dahbashi R, Hübner J, Berski F, Pierz K, Oestreich M 2014 Phys. Rev. Lett. 112 156601 Google Scholar
[17]	Yang L, Glasenapp P, Greilich A, Reuter D, Wieck A D, Yakovlev D R, Bayer M, Crooker S A 2014 Nat. Commun. 5 4949 Google Scholar
[18]	Crooker S A, Brandt J, Sandfort C, Greilich A, Yakovlev D R, Reuter D, Wieck A D, Bayer M 2010 Phys. Rev. Lett. 104 036601 Google Scholar
[19]	Kozlov G G, Ryzhov I I, Zapasskii V S 2017 Phys. Rev. A 95 043810 Google Scholar
[20]	Kozlov G G, Ryzhov I I, Zapasskii V S 2018 Phys. Rev. A 97 013848 Google Scholar
[21]	Keaveney J 2013 Ph. D. Dissertation (Durham: Durham University)
[22]	Ma J, Shi P, Qian X, Li W, Ji Y 2016 Chin. Phys. B 25 117203 Google Scholar
[23]	Hübner J, Berski F, Dahbashi R, Oestreich M 2014 Phys. Status. Solidi. 251 1824 Google Scholar
[24]	Petrov M Y, Ryzhov I I, Smirnov D S, Belyaev L Y, Potekhin R A, Glazov M M, Kulyasov V N, Kozlov G G, Aleksandrov E B, Zapasskii1 V S 2018 Phys. Rev. A 97 032502 Google Scholar
[25]	Zapasskii V S, Greilich A, Crooker S A, Li Y, Kozlov G G, Yakovlev D R, Reuter D, Wieck A D, Bayer M 2013 Phys. Rev. Lett. 110 176601 Google Scholar
[26]	Ghosh R K 2009 Ph. D. Dissertation (Princeton: Princeton University)
[27]	Rajroop J 2018 Ph. D. Dissertation (London: University College London)
[28]	Christopher J F 2005 Atomic Physics (Oxford: Oxford University Press) p142
[29]	Ma J, Shi P, Qian X, Shang Y, Ji Y 2017 Sci. Rep. 7 10238 Google Scholar
[30]	Buckingham A D, Stephens P J 1966 Annu. Rev. Phys. Chem. 17 399 Google Scholar
[31]	Zapasskii V S 2013 Adv. Opt. Photon. 5 131 Google Scholar
[32]	尚雅轩, 马健, 史平, 钱轩, 李伟, 姬杨 2018 67 087201 Google Scholar Shang Y X, Ma J, Shi P, Qian X, Li W, Ji Y 2018 Acta Phys. Sin. 67 087201 Google Scholar
[33]	Chalupczak W, Godun R M 2011 Phys. Rev. A. 83 032512 Google Scholar
[34]	Zhang W J, You L X, Li H, Huang J, Lv C L, Zhang L, Liu X Y, Wu J J, Wang Z, Xie X M 2017 Sci: China Phys. Mech. Astron. 60 120314 Google Scholar
[35]	Yang W H, Shi S P, Wang Y J, Ma W G, Zheng Y H, Peng K C 2017 Opt. Lett. 42 21 Google Scholar

[1]	Liang Peng-Jun, Zhu Tian-Xiang, Xiao Yi-Xin, Wang Yi-Yang, Han Yong-Jian, Zhou Zong-Quan, Li Chuan-Feng. Concentration-dependent optical and spin inhomogeneous linewidth of europium-doped yttrium orthosilicate crystals. Acta Physica Sinica, 2024, 73(10): 100301. doi: 10.7498/aps.73.20240116
[2]	Yang Xu, Feng Hong-Mei, Liu Jia-Nan, Zhang Xiang-Qun, He Wei, Cheng Zhao-Hua. Ultrafast spin dynamics: From femtosecond magnetism to attosecond magnetism. Acta Physica Sinica, 2024, 73(15): 157501. doi: 10.7498/aps.73.20240646
[3]	. From Transverse Field Ising Chain to Quantum E8 Integrable Model. Acta Physica Sinica, 2022, (): . doi: 10.7498/aps.71.20211836
[4]	Wang Xiao, Yang Jia-Hao, Wu Jian-Da. From the transverse field Ising chain to the quantum E₈ integrable model. Acta Physica Sinica, 2021, 70(23): 230504. doi: 10.7498/aps.70.20211836
[5]	Zhang Peng, Liu Zheng, Dai Jian-Ming, Yang Zhao-Rong, Su Fu-Hai. Anisotropic resonance absorptions induced by high magnetic field in ZnCr₂Se₄. Acta Physica Sinica, 2020, 69(20): 207501. doi: 10.7498/aps.69.20201507
[6]	Yang Yu-Lin, Bai Le-Le, Zhang Lu-Lu, He Jun, Wen Xin, Wang Jun-Min. Experimental investigation of spin noise spectroscopy of rubidium atomic ensemble. Acta Physica Sinica, 2020, 69(23): 233201. doi: 10.7498/aps.69.20201103
[7]	Yu Yang, Zhang Wen-Jie, Zhao Wan-Ying, Lin Xian, Jin Zuan-Ming, Liu Wei-Min, Ma Guo-Hong. Dynamics of A-exciton and spin relaxation in WS₂ and WSe₂ monolayer. Acta Physica Sinica, 2019, 68(1): 017201. doi: 10.7498/aps.68.20181769
[8]	Huang Rui, Li Chun, Jin Wei, Georgios Lefkidis, Wolfgang Hübner. Ultrafast spin dynamics in double-magnetic-center endohedral fullerene Y₂C₂@C₈₂-C₂(1). Acta Physica Sinica, 2019, 68(2): 023101. doi: 10.7498/aps.68.20181887
[9]	Xiang Tian, Cheng Liang, Qi Jing-Bo. Ultrafast charge and spin dynamics on topological insulators. Acta Physica Sinica, 2019, 68(22): 227202. doi: 10.7498/aps.68.20191433
[10]	Gong Dong-Liang, Luo Hui-Qian. Antiferromagnetic order and spin dynamics in iron-based superconductors. Acta Physica Sinica, 2018, 67(20): 207407. doi: 10.7498/aps.67.20181543
[11]	Shang Ya-Xuan, Ma Jian, Shi Ping, Qian Xuan, Li Wei, Ji Yang. Measurement and improvement of rubidium spin noise spectroscopy. Acta Physica Sinica, 2018, 67(8): 087201. doi: 10.7498/aps.67.20180098
[12]	Shi Ping, Ma Jian, Qian Xuan, Ji Yang, Li Wei. Signal-to-noise ratio of spin noise spectroscopy in rubidium vapor. Acta Physica Sinica, 2017, 66(1): 017201. doi: 10.7498/aps.66.017201
[13]	Zhu Meng-Long, Dong Yu-Lan, Zhong Hai-Zheng, He Jun. Exciton spin relaxation dynamics in CdTe quantum dots at room temperature. Acta Physica Sinica, 2014, 63(12): 127202. doi: 10.7498/aps.63.127202
[14]	Li Xia, Feng Dong-Hai, Pan Xian-Qun, Jia Tian-Qing, Shan Lu-Fan, Deng Li, Sun Zhen-Rong. Room-temperature ultrafast spin dynamics in colloidal CdSe quantum dots. Acta Physica Sinica, 2012, 61(20): 207202. doi: 10.7498/aps.61.207202
[15]	Li Chun, Yang Fan, Georgios Lefkidis, Wolfgang Hübner. Laser-induced ultrafast spin dynamics research on magnetic nanostructures. Acta Physica Sinica, 2011, 60(1): 017802. doi: 10.7498/aps.60.017802
[16]	Sun Zheng, Xu Zhong-Ying, Ruan Xue-Zhong, Ji Yang, Sun Bao-Quan, Ni Hai-Qiao. Spin relaxation dynamics in InAs monolayer and submonolayer. Acta Physica Sinica, 2007, 56(5): 2958-2961. doi: 10.7498/aps.56.2958
[17]	Qin Ji-Hong, Xu Su-Fen, Feng Shi-Ping. Spin dynamics in quasi-one-dimensional strongly correlated zigzag materials. Acta Physica Sinica, 2006, 55(10): 5511-5515. doi: 10.7498/aps.55.5511
[18]	Guo Li-Jun, Wüstenberg Jan-Peter, Oleksiy Andreyev, Bauer Michael, Aeschlimann Martin. Spin dynamics of GaAs(100) by two-photon photoemission. Acta Physica Sinica, 2005, 54(7): 3200-3205. doi: 10.7498/aps.54.3200
[19]	Liu Bin, Liang Ying, Feng Shi-Ping. Spin dynamics in doped spatially anisotropic triangular antiferromagnets. Acta Physica Sinica, 2004, 53(10): 3540-3544. doi: 10.7498/aps.53.3540
[20]	MA BEN-KUN. SPIN-LATTICE RELAXATION. Acta Physica Sinica, 1965, 21(7): 1419-1436. doi: 10.7498/aps.21.1419

Catalog

Vol. 69, Issue 3 - 2020-02-05

Metrics

Abstract views: 9697
PDF Downloads: 142
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板