-
This review reports a series of theoretical and experimental progress on researches of the transverse field Ising chain (TFIC) and the quantum E8 integrable model. For the TFIC, on one hand, a unique exotic quantum critical behavior of Grüneisen ratio (a ratio from magnetic or thermal expansion coefficient to specific heat) is theoretically established; on the other hand microscopic models can accommodate the TFIC universality class are substantially expanded. These progresses successfully promote a series of experiments collaborations to first-time realize the TFIC universality class in quasi one-dimensional anti-ferromagnetic material BaCo2V2O8 and SrCo2V2O8. For the quantum E8 integrable model, the low temperature local dynamics and the dynamical structure factor with zero transfer momentum of this system are analytically determined, where a cascade of edge singularities with power-law divergences are obtained in the continuum region of the dynamical structure factor. After combining with detailed quantum critical scaling behaviors analysis and large scale iTEBD calculation, it successfully facilitates a series of experiments, including THz spectrum measurements, inelastic neutron scattering and NMR experiments, to realize the quantum E8 integrable model in BaCo2V2O8 for the first time. The experimental realization of the quantum E8 integrable model substantially extends the frontiers of studying quantum integrable models in real materials. The series of progress and developments on the TFIC and the quantum E8 integrable model lay down a concrete ground to go beyond quantum integrability, and can inspire studies in condensed matter systems, cold atom systems, statistical field theory and conformal field theory.
-
Keywords:
- integrable systems /
- quantum magnetism /
- universality class /
- spin dynamics
[1] Sachdev S 2011 Quantum Phase Transitions (Cambridge: Cambridge University Press) pp96−115
[2] [3] Belavin A, Polyakov A, Zamolodchikov A 1984 Nucl. Phys. B 241 333
Google Scholar
[4] Zamolodchikov A B, Zamolodchikov A B 1979 Ann. Phys. 120 253
Google Scholar
[5] Belavin A A, Belavin V A, Litvinov A V, Pugai Y P, Zamolodchikov A B 2004 Nucl. Phys. B 676 587
Google Scholar
[6] Delfino G, Mussardo G 1994 Phys. Lett. B 324 40
Google Scholar
[7] Berg B, Karowski M, Weisz P 1979 Phys. Rev. D 19 2477
Google Scholar
[8] Löhneysen V H 2010 J. Low Temp. Phys. 161 1
Google Scholar
[9] Si Q M, Rabello S, Ingersent K, Smith J L 2001 Nature 413 804
Google Scholar
[10] Schuberth E, Tippmann M, Steinke L, Lausberg S, Steppke A, Brando M, Krellner C, Geibel C, Yu R, Si Q M, Steglich F 2016 Science 351 485
Google Scholar
[11] Wang Z, Wu J D, Yang W, Bera A K, Kamenskyi D, Islam A T M N, Xu S L, Law J M, Lake B, Wu C J, Loidl A 2018 Nature 554 219
Google Scholar
[12] Fan Y C, Yang J H, Yu W Q, Wu J D, Yu R 2020 Phys. Rev. Res. 2 013345
Google Scholar
[13] Isakov S V, Moessner R 2003 Phys. Rev. B 68 104409
Google Scholar
[14] Essler F H L, Konik R M 2005 in From Fields to Strings: Circumnavigating Theoretical Physics (Vol. 1) (Singapore: World Scientific) pp684−830
[15] Amico L, Fazio R, Osterloh A, Vedral V 2008 Rev. Mod. Phys. 80 517
Google Scholar
[16] Mussardo G 2010 Statistical Field Theory (New York: Oxford University Press) pp744–785
[17] Ku W, Yildirim Y 2015 Phys. Rev. B 92 180501(R
Google Scholar
[18] Ku W, Yildirim Y 2011 Phys. Rev. X 1 011011
[19] Kitaev A 2006 Ann. Phys. 321 2
Google Scholar
[20] Banerjee A, Yan J Q, Knolle J, Bridges C, Stone M, Lumsden M, Mandrus D, Tennant D, Moessner R, Nagler S 2017 Science 356 1055
Google Scholar
[21] Balents L 2010 Nature 464 199
Google Scholar
[22] Pfeuty P 1970 Ann. Phys. 57 79
Google Scholar
[23] Zamolodchikov A B 1989 Int. J. Mod. Phys. A 04 4235
Google Scholar
[24] Fateev V A, Zamolodchikov A B 1990 Int. J. Mod. Phys. A 05 06
[25] 杨文力, 杨战营, 杨涛等 2019 可积模型方法及其应用 (北京: 科学出版社) 第137—180页
Yang W L, Yang Z Y, Yang T, et al. 2019 Integrable Model Method and Its Applications (Beijing: Science Press) pp137–180
[26] Wu T T, McCoy B M, Tracy C A, Barouch E 1976 Phys. Rev. B 13 316
Google Scholar
[27] Zamolodchikov A B 1989 Adv. Stud. Pure Math. 19 641
[28] Zamolodchikov A B 1991 Nucl. Phys. B 348 619
Google Scholar
[29] Braden H W, Corrigan E, Dorey P E, Sasaki R 1990 Nucl. Phys. B 338 3
[30] Wang X, Zou H Y, Hódsági K, Kormos M, Takács G, Wu J D 2021 Phys. Rev. B 103 235117
Google Scholar
[31] Delfino G, Mussardo G 1995 Nucl. Phys. B 455 724
Google Scholar
[32] Wu J D, Zhu L J, Si Q M 2018 Phys. Rev. B 97 245127
Google Scholar
[33] Wang Z, Lorenz T, Gorbunov D I, Cong P T, Kohama Y, Niesen S, Breunig O, Engelmayer J, Herman A, Wu J D, Kindo K, Wosnitza J, Zherlitsyn S, Loidl A 2018 Phys. Rev. Lett. 120 207205
Google Scholar
[34] Cui Y, Zou H, Xi N, He Z Z, Yang Y X, Shu L, Zhang G H, Hu Z, Chen T, Yu R, Wu J D, Yu W Q 2019 Phys. Rev. Lett. 123 067203
Google Scholar
[35] Zou H Y, Yu R, Wu J D 2020 J. Phys.: Condens. Matter. 32 045602
Google Scholar
[36] Zhang Z, Amelin K, Wang X, Zou H Y, Yang J H, Nagel U, Rõõm T, Dey T, Nugroho A A, Lorenz T, Wu J D, Wang Z 2020 Phys. Rev. B 101 220411(R
Google Scholar
[37] Zou H Y, Cui Y, Wang X, Zhang Z, Yang J H, Xu G, Okutani A, Hagiwara M, Matsuda M, Wang G, Mussardo G, Hódsági K, Kormos M, He Z Z, Kimura S, Yu R, Yu W Q, Ma J, Wu J D 2021 Phys. Rev. Lett. 127 077201
Google Scholar
[38] Francesco P D, Mathieu P, Sénéchal D 1997 Conformal Field Theory (New York: Springer) pp200–235
[39] Wu T T, McCoy B 2013 The Two-Dimensional Ising Model (Cambridge: Harvard University Press) pp213–238
[40] Wu J D, Zhu L J, Si Q M 2011 J. Phys. Conf. Ser. 273 012019
Google Scholar
[41] Rost A, Perry R, Mercure J F, Mackenzie A, Grigera S 2009 Science 325 1360
Google Scholar
[42] Grube K, Zaum S, Stockert O, Si Q M, Löhneysen H V 2017 Nat. Phys. 13 742
Google Scholar
[43] Shiba H, Ueda Y, Okunishi K, Kimura S, Kindo K 2003 J. Phys. Soc. Jpn. 72 2326
Google Scholar
[44] Dmitriev V D, Krivnov Y V, Ovchinnikov A A, Langari A 2002 J. Exp. Theor. Phys. 95 538
Google Scholar
[45] Kimura S, Okunishi K, Hagiwara M, Kindo K, He Z Z, Taniyama T, Itoh M, Koyama K, and Watanabe K 2013 J. Phys. Soc. Jpn. Lett. 82 033706
Google Scholar
[46] Wu J D, Kormos M, Si Q M 2014 Phys. Rev. Lett. 113 247201
Google Scholar
[47] Hódsági K, Kormos M, Takács G 2019 J. High Energy Phys. 08 047
[48] Delfino G, Simonetti P, Cardy J 1996 Phys. Lett. B 387 327
Google Scholar
[49] Delfino G, Simonetti P 1996 Phys. Lett. B 383 450
Google Scholar
[50] Delfino G, Mussardo G, Simonetti P 1996 Phys. Lett. B 473 469
[51] O'Brien E, Fendley P 2018 Phys. Rev. Lett. 120 206403
Google Scholar
[52] O'Brien E, Fendley P 2020 SciPost Phys. 9 88
Google Scholar
[53] Zou Y J, Vidal G 2020 Phys. Rev. B 101 045132
Google Scholar
[54] Gehlen G 1990 Nucl. Phys. B 330 741
Google Scholar
[55] Deserno M 1997 Phys. Rev. E 56 5204
Google Scholar
[56] Xiang C H, Chen A M, Su H Y, Wang H L 2020 Physica A 553 124274
Google Scholar
[57] Balbao D B, Felicio J R D D 1987 J. Phys. A: Math. Gen. 20 L207
Google Scholar
[58] Fateev V 1994 Phys. Lett. B 324 45
Google Scholar
[59] Fonseca P, Zamolodchikov A 2006 arxiv: hep-th/0612304
[60] McCoy B M, Wu T T 1978 Phys. Rev. D 18 1259
Google Scholar
[61] Pozsgay B, Takács G 2008 Nucl. Phys. B 788 167
Google Scholar
[62] Pozsgay B, Takács G 2008 Nucl. Phys. B 788 209
Google Scholar
[63] Kormos M, Takács G 2010 J. High Energy Phys. 2010 112
[64] Kormos M, Takács G 2008 Nucl. Phys. B 803 277
Google Scholar
[65] Coldea R, Tennant D A, Wheeler E M, Wawrzynska E, Prabhakaran D, Telling M, Habicht K, Smeibidl P, Kiefer K 2010 Science 327 177
Google Scholar
[66] Amelin K, Engelmayer J, Viirok J, Nagel U, Rõõm T, Lorenz T, Wang Z 2020 Phys. Rev. B 102 104431
Google Scholar
[67] Morris M C, Desai N, Viirok J, Hüvonen D, Nagel U, Rõõm T, Krizan W J, Cava J R, McQueen T M, Koohpayeh S M, Kaul K R, Armitage N P 2020 Nat. Phys. 17 832
[68] Favaa M, Coldea R, Parameswaran S 2020 PNAS 117 41
Google Scholar
-
图 1 TFIC模型相图.
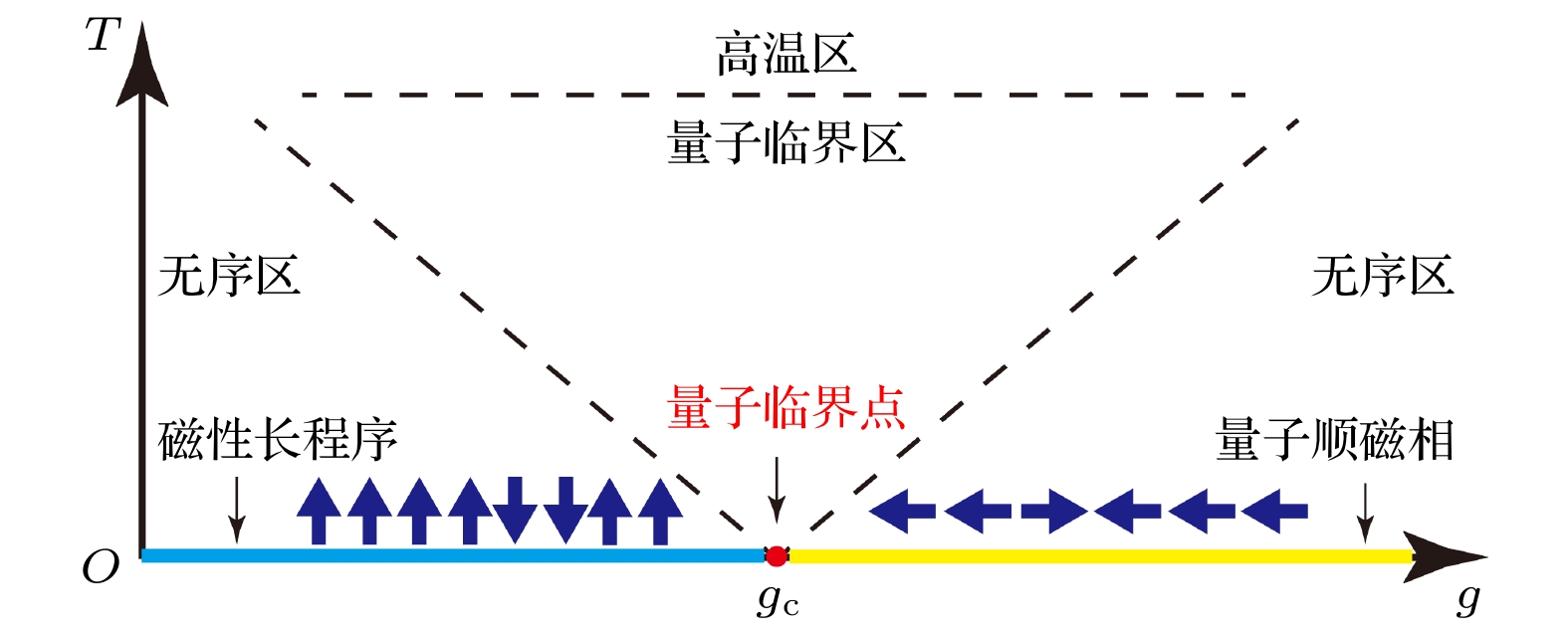
$ g $ 为横场参数,$ g_{{\rm{c}}} = 1 $ 为QCP. 在相变点左侧, 青蓝色实线代表零温下的铁磁相; 在相变点右侧, 黄色实线代表零温下的顺磁相. 相图中的两斜虚线为低温下各个不同无序区域的过渡边界, 而上方虚线则是量子临界区域到经典区域的过渡边界Figure 1. A phase diagram of TFIC, where
$ g $ labels transverse field, and$ g_{{\rm{c}}} = 1 $ is the QCP. The cyan solid line represents for ferromagnetic phase at zero temperature on the left of the critical point, while on the right the yellow solid line stands for a paramagnetic phase at zero temperature. The two tilted dotted lines illustrate the crossovers of different disorder phases in low temperature region, while the dotted line above shows a crossover from quantum critical region to classical region.
图 3 (a)交错磁化
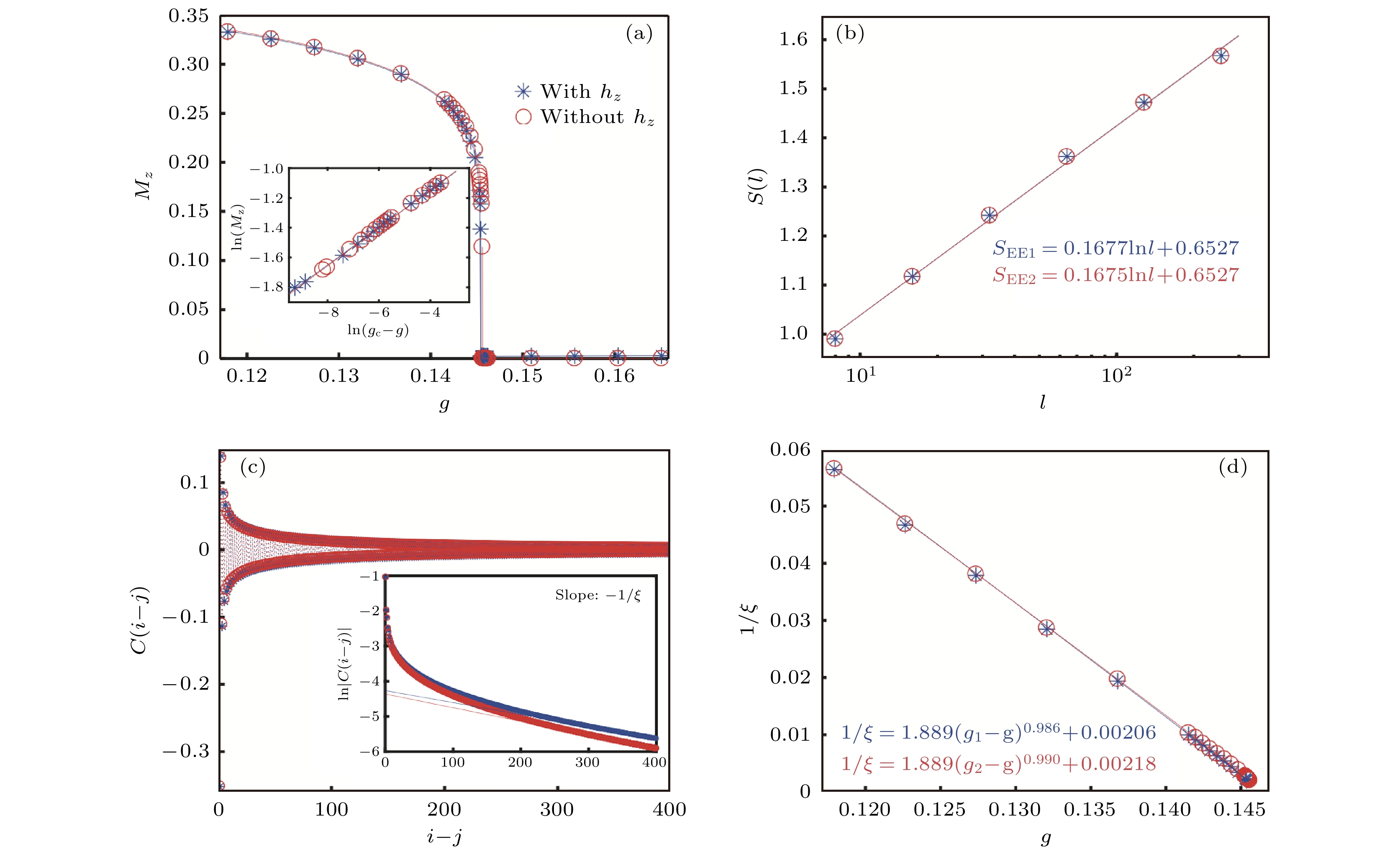
$ M_{z}(g) $ 的计算结果. 蓝线和红线分别代表了带有和不带有4周期项的数据. 这两条曲线可以分别用$M_{z} = $ $ 0.524(g_{{\rm{c}}1}-g)^{0.126}$ 和$ M_{z} = 0.530(g_{{\rm{c}}2}-g)^{0.128} $ 来拟合, 其中$ g_{{\rm{c}}1} = 0.1454 $ ,$ g_{{\rm{c}}2} = 0.1456 $ , 在误差精度范围内均可得到临界指数为$ 1/8 $ 的结论. 内嵌图是利用对数坐标轴画出的$ M_{z} $ 的标度行为. (b)半对数坐标轴下纠缠熵$ S_{{\rm{EE}}}(l) $ 和链长$ l $ 的关系, 两种情况都符合伊辛普适类的$ 1/2 $ 中心荷. (c)当$ g = 0.1448 $ 时, 自旋两点关联函数和距离$ i-j $ 间的函数关系. 内嵌图展示了$ \ln C(i-j) $ 在长程时与$ i-j $ 成正比. (d)关联长度的倒数和$ g $ 的函数关系, 两种情况都符合伊辛普适类中关联长度指数$ \nu = 1 $ 的结论(图片经文献[35]允许转载, 版权归2019 IOP Publishing Ltd 所有)Figure 3. (a) iTEBD results for staggered magnetization
$ M_{z}(g) $ . The blue line and red line represent iTEBD data with and without the four periodic term, respectively. The two curves can be fitted with$ M_{z} = 0.524(g_{{\rm{c}}1}-g)^{0.126} $ and$ M_{z} = 0.530(g_{{\rm{c}}2}-g)^{0.128} $ , where$ g_{{\rm{c}}1} = 0.1454 $ ,$ g_{{\rm{c}}2} = 0.1456 $ , and critical exponent$ \delta = 1/8 $ is obtained within error bar for both cases. Inset shows scaling behavior of$ M_{z} $ in$ \log-\log $ plot. (b) Entanglement entropy$ S_{{\rm{EE}}}(l) $ versus length$ l $ in a semi-$ \log $ plot, both fall into central charge$ c = 1/2 $ of TFIC universality. (c) Spin-spin correlation function versus distance$ i-j $ at$ g = 0.1448 $ . Inset shows$ \ln C(i-j) $ being proportional to$ i-j $ in long range region. (d) The inverse of correlation length in terms of$ g $ , both agree with correlation length exponent$ \nu = 1 $ . (Reprinted with permission from Ref. [35]. Copyright 2019 IOP Publishing Ltd.)
图 4 (a) CoO6八面体的结构; (b) CoO6四周期螺旋链的单元结构及局域坐标系
$ \xi\psi\zeta $ ; (c)局域坐标系$ \xi\psi\zeta $ 与实验室坐标系XYZ的关系Figure 4. (a) Structure of CoO6 octahedron; (b) a unit structure of CoO6 four-period screw chain and the local coordinate
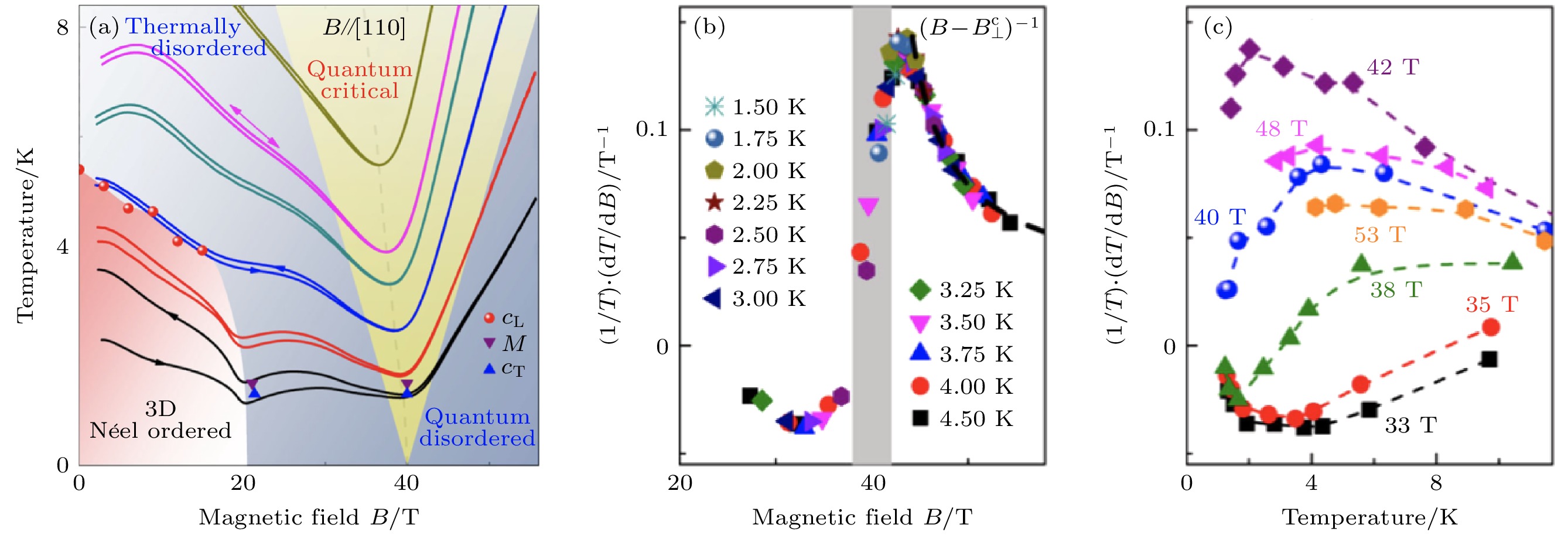
$ \xi\psi\zeta $ ; (c) relation between the local coordinate$ \xi\psi\zeta $ and the lab coordinate XYZ.
图 5 (a) BCVO材料在加[110]方向磁场后的相图, 利用从不同起始温度出发的绝热磁热测量得出. 红色区域内直到
$ 20 $ T处标记了一有限温的三维序. 一维QCP出现在$ B_{\perp}^{{\rm{c}}} = 40 $ T附近, 上方黄色区域为量子临界区. (b), (c)以磁场和温度作为自变量的格林艾森比率实验数据. 在图(b)中, 大于QCP时不同温度的数据展现了$ \varGamma_{B}\approx (B-B_{\perp}^{{\rm{c}}})^{-1} $ 的发散行为. 相对应地, 当场强在临界场强附近时, 低温下格林艾森比率$ \varGamma_{B}(T) $ 趋于收敛(图片经文献[33]允许转载, 版权归2018 American Physical Society 所有)Figure 5. (a) Phase diagram of BCVO material with a transverse field along [110], obtained from adiabatic magnetocaloric-effect measurements starting from different temperatures. Red area labels a finite-temperature three dimensional (3D) order until 20 T. The one dimensional (1D) QCP appears around
$ B_{\perp}^{{\rm{c}}} = 40 $ T, while the yellow area above labels quantum critical region. (b), (c) Experimental data of Grüneisen ratio in terms of magnetic field and temperature. In panel (b) data of different temperature above the QCP shows divergent behavior of$ \varGamma_{B}\approx (B-B_{\perp}^{{\rm{c}}})^{-1} $ . Correspondingly, with the field being around the critical field, Grüneisen ratio converges in low temperature. (Reprinted with permission from Ref. [33]. Copyright 2018 American Physical Society)
图 6 外加[100]方向磁场的SCVO材料相图. 图中AFM, QCR和QD分别代表反铁磁相、量子临界区和量子无序区. 两个QCPs分别为
$ H_{{\rm{c}}1}\approx 7.03\; {\rm{T}} $ 和$ H_{{\rm{c}}2}\approx 7.7\; {\rm{T}} $ . 蓝色线可以被曲线$ T_{{\rm{cr}}}^{{\rm{L, H}}}\sim \vert H_{{\rm{c}}2}-H \vert $ 所拟合, 揭示了该QCP对应伊辛普适类. 内嵌图是两QCPs附近拟合曲线的放大 (图片经文献[34]允许转载, 版权归2019 American Physical Society 所有)Figure 6. Phase diagram of SCVO with transverse field along [100]. The AFM, QCR and QD in the figure corresponds to anti-ferromagnetic phase, quantum critical region and quantum disordered region, respectively. The two QCPs are
$ H_{{\rm{c}}1}\approx 7.03 \;{\rm{T}} $ and$ H_{{\rm{c}}2}\approx 7.7\; {\rm{T}} $ . The blue line can be fitted with$ T_{{\rm{cr}}}^{{\rm{L, H}}}\sim \vert H_{{\rm{c}}2}-H \vert $ , implying the Ising universality of the 1D QCP. Inset is an enlarged figure of the fitting curves near two QCPs. (Reprinted with permission from Ref. [34]. Copyright 2019 American Physical Society)
图 7
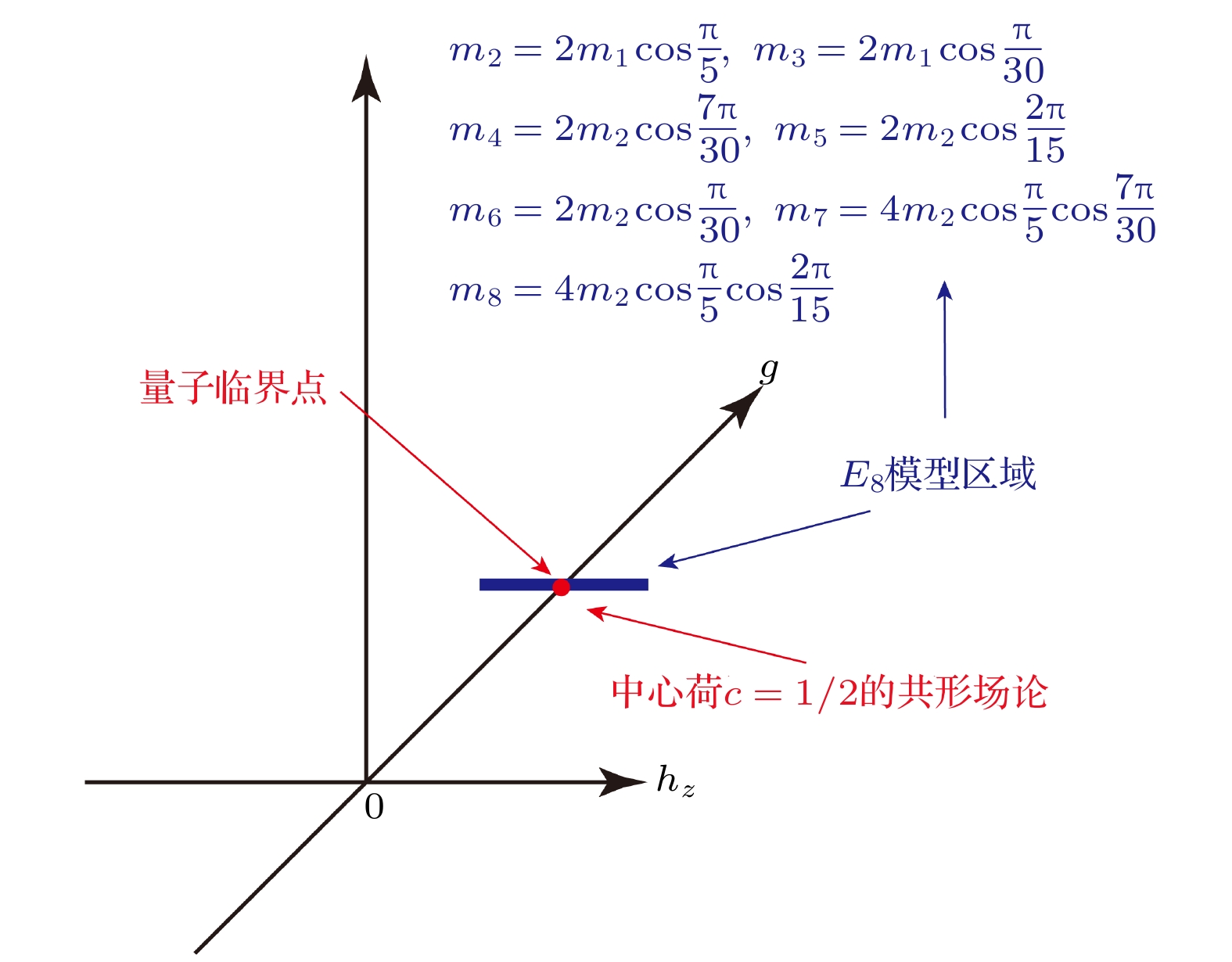
$ E_{8} $ 模型相图. 图中蓝色实线代表了$ E_{8} $ 模型涌现的区域, 其中$ g $ 和$ h_{z} $ 分别对应于横场参数$ g $ 和方程(17)中的$ h_{z} $ . 其余$ E_{8} $ 准粒子的质量和$ m_{1} $ 和$ m_{2} $ 的关系列于右上, 其中$ m_{1} $ 为最轻准粒子的质量Figure 7. A phase diagram of
$ E_{8} $ model. The blue solid line in the figure implies region of$ E_{8} $ model emerging, where$ g $ and$ h_{z} $ correspond to transverse parameter$ g $ and$ h_{z} $ in Eq. (17) respectively. Relations of other$ E_{8} $ particles' masses to$ m_{1} $ and$ m_{2} $ are listed in the top right-hand corner, where$ m_{1} $ is mass of the lightest particle.
图 8
$ D^{yy}(\omega) $ 的解析计算结果 (a)单粒子通道的谱权重贡献, 8个峰的位置依次对应$ E_{8} $ 模型8种粒子的质量, 其中$ m_{1} = 1 $ ; (b)—(d)二粒子、三粒子和四粒子通道的谱权重贡献, 图中分别标出了谱线和峰的具体贡献来源(图片经文献[30]允许转载, 版权归2021 American Physical Society 所有)Figure 8. (a) Spectrum contribution from single particle channel, the eight delta peaks one-by-one correspond to the masses of the eight particles in the quantum
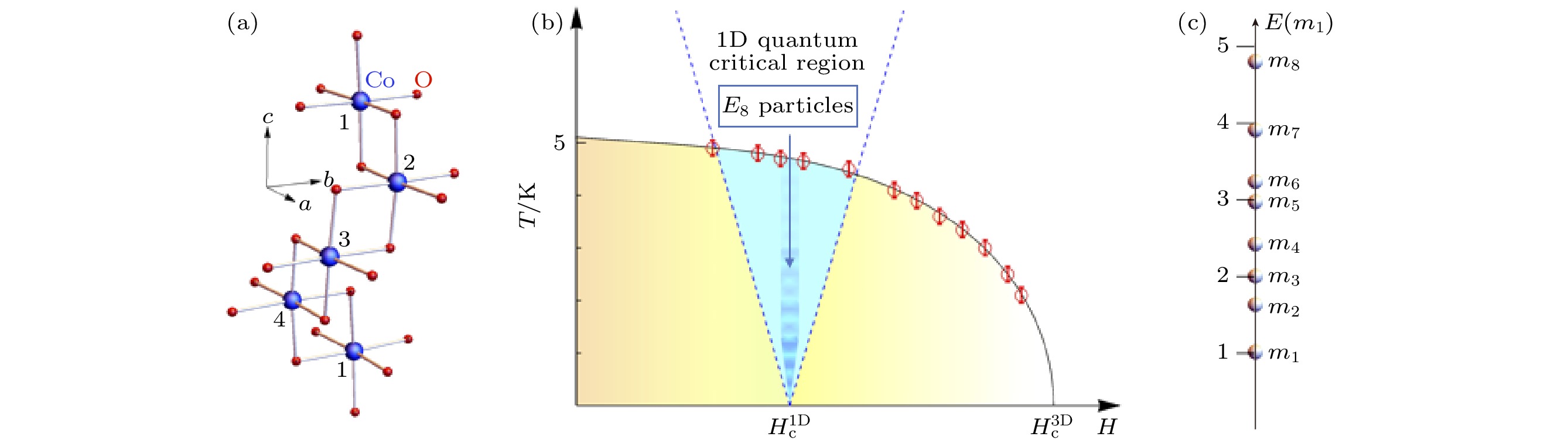
$ E_{8} $ model with$ m_{1} = 1 $ ; (b)–(d) contributions from two, three and four particle channels. The detailed contributions of different peaks and curves are highlighted in the sub-figures. (Reprinted with permission from Ref. [30]. Copyright 2021 American Physical Society)
图 9 (a) BCVO的晶格结构. (b) 加沿[010]方向磁场后BCVO的相图, QCP为
$ H_{{\rm{c}}}^{1 {\rm{D}}} $ , 隐藏在三维序中, 其一维量子临界行为可在三维序外测得. 棕色圆圈代表由NMR实验测出的尼尔(Néel)温度$ T_{{\rm{N}}} $ .$H_{{\rm{c}}}^{3{\rm{ D}}} = (10.4\pm 0.1)\;\text{T}, \; H_{{\rm{c}}}^{1 {\rm{D}}} = (4.7\pm 0.3)$ T.$ E_{8} $ 模型在蓝色缎带区涌现. (c) 8种$ E_{8} $ 粒子的质量以$ m_{1} $ 为单位沿能量轴分布(图片经文献[37]允许转载, 版权归2021 American Physical Society 所有)Figure 9. (a) Crystal structure of BCVO. (b) Phase diagram of BCVO with a transverse field along [010] direction. QCP is at
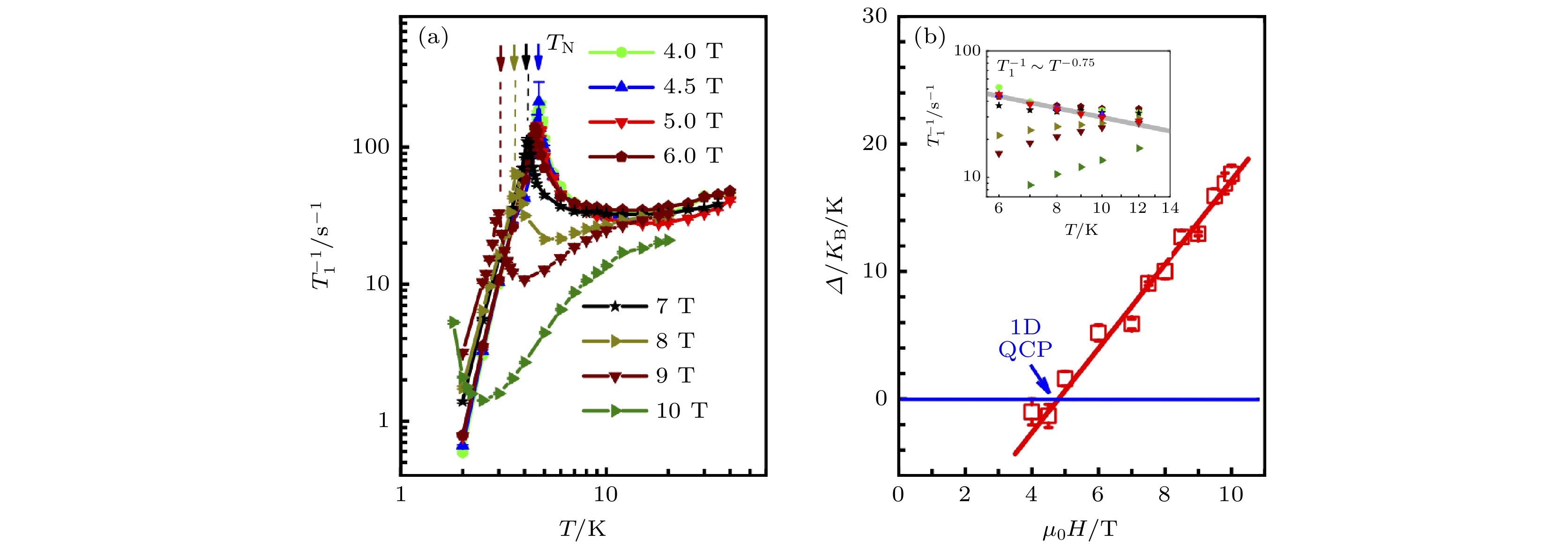
$ H_{{\rm{c}}}^{1{\rm{ D}}} $ , hidden in the 3D order. Its quantum criticality can be measured outside the 3D ordering phase. Brown circles represent for Néel temperature$ T_{{\rm{N}}} $ measured by NMR experiments.$H_{{\rm{c}}}^{3 {\rm{D}}} = (10.4\pm 0.1)\;\rm {T}, \; H_{{\rm{c}}}^{1 {\rm{D}}} = (4.7\pm 0.3)\;\rm {T}$ .$ E_{8} $ model emerges in the blue ribbon area. (c) Masses of eight$ E_{8} $ particles along energy axis in unit of$ m_{1} $ . (Reprinted with permission from Ref. [37]. Copyright 2021 American Physical Society)
图 10 三维反铁磁序外NMR实验测量结果 (a) 在不同横场下测得自旋-晶格弛豫率
$ 1/T_{1} $ 关于温度的函数. 下箭头标记出决定$T_{{\rm{N}}}$ 的$ 1/T_{1} $ 的峰. (b)拟合$ 1/T_{1} $ 得到能隙, 温度区间为6—12 K. 具体细节见文献[37]. QCP位于$ (4.7\pm 0.3) $ T. 内嵌图是对数坐标下放大的低温区数据. 灰色直线区域内的量子临界行为可以拟合为$ 1/T_{1}\sim T^{-0.75} $ (图片经文献[37]允许转载, 版权归2021 American Physical Society 所有)Figure 10. NMR experiment result outside the 3D anti-ferromagnetic ordering phase. (a) Spin-lattice relaxation rate
$ 1/T_{1} $ with different transverse fields as a function of temperature. The down arrows label$ 1/T_{1} $ peaks that determining$T_{{\rm{N}}}$ . (b) Energy gap by fitting$ 1/T_{1} $ , with temperature being from 6 K to 12 K. The details can be found in Ref. [37]. QCP is at$(4.7\pm 0.3)$ T. Inset: a$ \log-\log $ plot of enlarged data in low-temperature region. The quantum critical behavior around the gray line area can be fitted by$ 1/T_{1}\sim T^{-0.75} $ . (Reprinted with permission from Ref. [37]. Copyright 2021 American Physical Society)
图 11 (a)
$ Q = (002) $ QCP处INS测量数据, 场强$ H = 4.7 $ T, 温度$ 0.4 $ K. 蓝色方块及误差棒为实验测量数据, 黑线是高斯函数的拟合. 红色竖线对应于8种$ E_{8} $ 模型的粒子. 其他的峰来自于多粒子通道的连续谱贡献以及布里渊区折叠效应(标记于$ F_{1} $ 和$ F_{2} $ 处). (b) 解析计算的$ D^{xx}(\omega) $ , 数据经过$ 0.08 m_{1} $ 洛伦兹展宽.$m_{1} = 1.2\;\text{meV}$ . 红色线和黑色线分别代表单粒子激发贡献及引入多粒子激发贡献的总和. (c) iTEBD数值计算有效模型在转移动量$ q = 0 $ 处的激发谱, 其中蓝线和黑线代表不包含及包含折叠效应的数据. (d)—(g) 单粒子到四粒子激发通道的贡献,$ ijkl $ 代表激发来自于$ m_{i}m_{j}m_{k}m_{l} $ 通道(图片经文献[37]允许转载, 版权归2021 American Physical Society 所有)Figure 11. (a) INS data obtained from
$ Q = (002) $ at QCP with$ H = 4.7 $ T, temperature$ 0.4 $ K. The blue diamonds with error bars are experimental data, the black curve is fitted with Gaussian functions. The red vertical lines correspond to eight particles of$ E_{8} $ model. Other peaks are contributed from multi-particle channels and zone-folding effect (labelled at$ F_{1} $ and$ F_{2} $ ). (b) Analytical result of$ D^{xx}(\omega) $ with a$ 0.08 m_{1} $ Lorentzian boradening.$m_{1} = 1.2\;\text{meV}$ . Red curve and black curve are single particle spectrum and total spectrum including multi-particle excitations respectively. (c) Effective model spectrum of iTEBD calculation at$ q = 0 $ , where blue curve and black curve are results of without and with zone-folding effect. (d)–(g) single particle-four particle excited channels' contributions, where$ ijkl $ represents for$ m_{i}m_{j}m_{k}m_{l} $ channel. (Reprinted with permission from Ref. [37]. Copyright 2021 American Physical Society) -
[1] Sachdev S 2011 Quantum Phase Transitions (Cambridge: Cambridge University Press) pp96−115
[2] [3] Belavin A, Polyakov A, Zamolodchikov A 1984 Nucl. Phys. B 241 333
Google Scholar
[4] Zamolodchikov A B, Zamolodchikov A B 1979 Ann. Phys. 120 253
Google Scholar
[5] Belavin A A, Belavin V A, Litvinov A V, Pugai Y P, Zamolodchikov A B 2004 Nucl. Phys. B 676 587
Google Scholar
[6] Delfino G, Mussardo G 1994 Phys. Lett. B 324 40
Google Scholar
[7] Berg B, Karowski M, Weisz P 1979 Phys. Rev. D 19 2477
Google Scholar
[8] Löhneysen V H 2010 J. Low Temp. Phys. 161 1
Google Scholar
[9] Si Q M, Rabello S, Ingersent K, Smith J L 2001 Nature 413 804
Google Scholar
[10] Schuberth E, Tippmann M, Steinke L, Lausberg S, Steppke A, Brando M, Krellner C, Geibel C, Yu R, Si Q M, Steglich F 2016 Science 351 485
Google Scholar
[11] Wang Z, Wu J D, Yang W, Bera A K, Kamenskyi D, Islam A T M N, Xu S L, Law J M, Lake B, Wu C J, Loidl A 2018 Nature 554 219
Google Scholar
[12] Fan Y C, Yang J H, Yu W Q, Wu J D, Yu R 2020 Phys. Rev. Res. 2 013345
Google Scholar
[13] Isakov S V, Moessner R 2003 Phys. Rev. B 68 104409
Google Scholar
[14] Essler F H L, Konik R M 2005 in From Fields to Strings: Circumnavigating Theoretical Physics (Vol. 1) (Singapore: World Scientific) pp684−830
[15] Amico L, Fazio R, Osterloh A, Vedral V 2008 Rev. Mod. Phys. 80 517
Google Scholar
[16] Mussardo G 2010 Statistical Field Theory (New York: Oxford University Press) pp744–785
[17] Ku W, Yildirim Y 2015 Phys. Rev. B 92 180501(R
Google Scholar
[18] Ku W, Yildirim Y 2011 Phys. Rev. X 1 011011
[19] Kitaev A 2006 Ann. Phys. 321 2
Google Scholar
[20] Banerjee A, Yan J Q, Knolle J, Bridges C, Stone M, Lumsden M, Mandrus D, Tennant D, Moessner R, Nagler S 2017 Science 356 1055
Google Scholar
[21] Balents L 2010 Nature 464 199
Google Scholar
[22] Pfeuty P 1970 Ann. Phys. 57 79
Google Scholar
[23] Zamolodchikov A B 1989 Int. J. Mod. Phys. A 04 4235
Google Scholar
[24] Fateev V A, Zamolodchikov A B 1990 Int. J. Mod. Phys. A 05 06
[25] 杨文力, 杨战营, 杨涛等 2019 可积模型方法及其应用 (北京: 科学出版社) 第137—180页
Yang W L, Yang Z Y, Yang T, et al. 2019 Integrable Model Method and Its Applications (Beijing: Science Press) pp137–180
[26] Wu T T, McCoy B M, Tracy C A, Barouch E 1976 Phys. Rev. B 13 316
Google Scholar
[27] Zamolodchikov A B 1989 Adv. Stud. Pure Math. 19 641
[28] Zamolodchikov A B 1991 Nucl. Phys. B 348 619
Google Scholar
[29] Braden H W, Corrigan E, Dorey P E, Sasaki R 1990 Nucl. Phys. B 338 3
[30] Wang X, Zou H Y, Hódsági K, Kormos M, Takács G, Wu J D 2021 Phys. Rev. B 103 235117
Google Scholar
[31] Delfino G, Mussardo G 1995 Nucl. Phys. B 455 724
Google Scholar
[32] Wu J D, Zhu L J, Si Q M 2018 Phys. Rev. B 97 245127
Google Scholar
[33] Wang Z, Lorenz T, Gorbunov D I, Cong P T, Kohama Y, Niesen S, Breunig O, Engelmayer J, Herman A, Wu J D, Kindo K, Wosnitza J, Zherlitsyn S, Loidl A 2018 Phys. Rev. Lett. 120 207205
Google Scholar
[34] Cui Y, Zou H, Xi N, He Z Z, Yang Y X, Shu L, Zhang G H, Hu Z, Chen T, Yu R, Wu J D, Yu W Q 2019 Phys. Rev. Lett. 123 067203
Google Scholar
[35] Zou H Y, Yu R, Wu J D 2020 J. Phys.: Condens. Matter. 32 045602
Google Scholar
[36] Zhang Z, Amelin K, Wang X, Zou H Y, Yang J H, Nagel U, Rõõm T, Dey T, Nugroho A A, Lorenz T, Wu J D, Wang Z 2020 Phys. Rev. B 101 220411(R
Google Scholar
[37] Zou H Y, Cui Y, Wang X, Zhang Z, Yang J H, Xu G, Okutani A, Hagiwara M, Matsuda M, Wang G, Mussardo G, Hódsági K, Kormos M, He Z Z, Kimura S, Yu R, Yu W Q, Ma J, Wu J D 2021 Phys. Rev. Lett. 127 077201
Google Scholar
[38] Francesco P D, Mathieu P, Sénéchal D 1997 Conformal Field Theory (New York: Springer) pp200–235
[39] Wu T T, McCoy B 2013 The Two-Dimensional Ising Model (Cambridge: Harvard University Press) pp213–238
[40] Wu J D, Zhu L J, Si Q M 2011 J. Phys. Conf. Ser. 273 012019
Google Scholar
[41] Rost A, Perry R, Mercure J F, Mackenzie A, Grigera S 2009 Science 325 1360
Google Scholar
[42] Grube K, Zaum S, Stockert O, Si Q M, Löhneysen H V 2017 Nat. Phys. 13 742
Google Scholar
[43] Shiba H, Ueda Y, Okunishi K, Kimura S, Kindo K 2003 J. Phys. Soc. Jpn. 72 2326
Google Scholar
[44] Dmitriev V D, Krivnov Y V, Ovchinnikov A A, Langari A 2002 J. Exp. Theor. Phys. 95 538
Google Scholar
[45] Kimura S, Okunishi K, Hagiwara M, Kindo K, He Z Z, Taniyama T, Itoh M, Koyama K, and Watanabe K 2013 J. Phys. Soc. Jpn. Lett. 82 033706
Google Scholar
[46] Wu J D, Kormos M, Si Q M 2014 Phys. Rev. Lett. 113 247201
Google Scholar
[47] Hódsági K, Kormos M, Takács G 2019 J. High Energy Phys. 08 047
[48] Delfino G, Simonetti P, Cardy J 1996 Phys. Lett. B 387 327
Google Scholar
[49] Delfino G, Simonetti P 1996 Phys. Lett. B 383 450
Google Scholar
[50] Delfino G, Mussardo G, Simonetti P 1996 Phys. Lett. B 473 469
[51] O'Brien E, Fendley P 2018 Phys. Rev. Lett. 120 206403
Google Scholar
[52] O'Brien E, Fendley P 2020 SciPost Phys. 9 88
Google Scholar
[53] Zou Y J, Vidal G 2020 Phys. Rev. B 101 045132
Google Scholar
[54] Gehlen G 1990 Nucl. Phys. B 330 741
Google Scholar
[55] Deserno M 1997 Phys. Rev. E 56 5204
Google Scholar
[56] Xiang C H, Chen A M, Su H Y, Wang H L 2020 Physica A 553 124274
Google Scholar
[57] Balbao D B, Felicio J R D D 1987 J. Phys. A: Math. Gen. 20 L207
Google Scholar
[58] Fateev V 1994 Phys. Lett. B 324 45
Google Scholar
[59] Fonseca P, Zamolodchikov A 2006 arxiv: hep-th/0612304
[60] McCoy B M, Wu T T 1978 Phys. Rev. D 18 1259
Google Scholar
[61] Pozsgay B, Takács G 2008 Nucl. Phys. B 788 167
Google Scholar
[62] Pozsgay B, Takács G 2008 Nucl. Phys. B 788 209
Google Scholar
[63] Kormos M, Takács G 2010 J. High Energy Phys. 2010 112
[64] Kormos M, Takács G 2008 Nucl. Phys. B 803 277
Google Scholar
[65] Coldea R, Tennant D A, Wheeler E M, Wawrzynska E, Prabhakaran D, Telling M, Habicht K, Smeibidl P, Kiefer K 2010 Science 327 177
Google Scholar
[66] Amelin K, Engelmayer J, Viirok J, Nagel U, Rõõm T, Lorenz T, Wang Z 2020 Phys. Rev. B 102 104431
Google Scholar
[67] Morris M C, Desai N, Viirok J, Hüvonen D, Nagel U, Rõõm T, Krizan W J, Cava J R, McQueen T M, Koohpayeh S M, Kaul K R, Armitage N P 2020 Nat. Phys. 17 832
[68] Favaa M, Coldea R, Parameswaran S 2020 PNAS 117 41
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 6896
- PDF Downloads: 241
- Cited By: 0
