-
作为典型的具有螺旋磁结构的材料, ZnCr2Se4承载着诸如磁电耦合、磁致伸缩和负热膨胀等有趣特性, 并可能具备多种不同的量子基态. 本文利用太赫兹时域光谱技术研究了ZnCr2Se4在低温强磁场(T = 4—60 K, H = 0—10 T)下的自旋动力学行为. 当外加磁场高于4 T时, 可以观察到亚太赫兹频率范围的磁共振吸收, 并呈现出随磁场增加蓝移特征. 当磁场( H )方向垂直于太赫兹波矢( k )方向时, 仅观察到单个共振吸收, 且其磁场行为符合线性拉莫尔进动关系. 这种磁场依赖性对应传统的铁磁共振, 意味着螺旋自旋态在高磁场下演化为线性铁磁态. 然而, 在 H 和 k 同时平行于样品的
$ \langle 111\rangle $ 晶向配置下, 当磁场强度高于7 T时, 其太赫兹共振明显劈裂为高频和低频两个吸收峰, 并且其高频吸收表现出非线性磁场依赖关系. 这种奈尔温度以下特有的各向异性太赫兹自旋动力学效应可能与最近发现的量子临界区域有关.As a typical helimagnet, ZnCr2Se4 possesses fascinating effects including magnetoelectric coupling, magnetostriction, negative thermal expansion, as well as possible diversity in quantum ground states. Here in this work, we investigate magnetic excitation arising from spiral spin structure in ZnCr2Se4 single crystal by using terahertz (THz) time domain spectroscopy (THz-TDS) under magnetic fields up to 10 T and at low temperatures. The magnetic resonance absorption is observed in a sub-THz region as the applied magnetic field is above 4 T, featuring the blue shift with magnetic field increasing. As the THz wave vector ( k ) is vertical to the external magnetic field (H), the single resonance frequency conforms well with the linear Larmor relation, corresponding to a spin structure transformation from helical to ferromagnetic state with magnetic field increasing in ZnCr2Se4. However, in the geometry in which both k and H are along the$ \langle 111\rangle $ direction of crystal, a well-defined resonance splitting emerges when H > 7 T. Especially, the high-frequency absorption shows pronouncedly nonlinear magnetic field dependence. It is suggested that such anisotropic spin dynamics below Néel temperature be linked with the field-driven quantum criticality unveiled in recent work.-
Keywords:
- ZnCr2Se4 /
- spin dynamics /
- high magnetic field /
- terahertz
[1] Harris M J, Bramwell S T, McMorrow D F, Zeiske T, Godfrey K W 1988 Phys. Rev. Lett. 79 2554
[2] Chen G, Balents L, Schnyder A P 2009 Phys. Rev. Lett. 102 096406
Google Scholar
[3] Gu C C, Zhao Z Y, Chen X L, Lee M, Choi E S, Han Y Y, Ling L S, Pi L, Zhang Y H, Chen G, Yang Z R, Zhou H D, Sun X F 2018 Phys. Rev. Lett. 120 147204
Google Scholar
[4] Rudolf T, Kant Ch, Mayr F, Hemberger J, Tsurkan V, Loidl A 2007 Phys. Rev. B 75 052410
Google Scholar
[5] Akimitsu J, Siratori K, Shirane G, Iizumi M, Watanabe T 1978 J. Phys. Soc. Jpn. 44 172
Google Scholar
[6] Hidaka M, Tokiwa N, Fujii M, Watanabe S, Akimitsu J 2003 Phys. Status Solidi B 236 9
Google Scholar
[7] Plumier R J 1966 J. Appl. Phys. 37 964
Google Scholar
[8] Tymoshenko Y V, Onykiienko Y A, Müller T, Thomale R, Rachel S, Cameron A S, Portnichenko P Y, Efremov D V, Tsurkan V, Abernathy D L, Ollivier J, Schneidewind A, Piovano A, Felea V, Loidl A, Inosov D S 2017 Phys. Rev. X 7 041049
[9] Felea V, Yasin S, Gunther A, Deisenhofer J, Krug von Nidda H A, Zherlitsyn S, Tsurkan V, Lemmens P, Wosnitza J, Loidl A 2012 Phys. Rev. B 86 104420
Google Scholar
[10] Laurita N J, Deisenhofer J, Pan LiDong, Morris C M, Schmidt M, Johnsson M, Tsurkan V, Loidl A, Armitage N P 2015 Phys. Rev. Lett. 114 207201
Google Scholar
[11] Shuvaev A M, Travkin V D, Ivanov V Yu, Mukhin A A, Pimenov A 2010 Phys. Rev. Lett. 104 097202
Google Scholar
[12] 金钻明, 阮舜逸, 李炬赓, 林贤, 任伟, 曹世勋, 马国宏, 姚建铨 2019 68 167501
Google Scholar
Jin Z M, Yuan S Y, Li J G, Lin X, Ren W, Cao S X, Ma G H, Yao J S 2019 Acta Phys. Sin. 68 167501
Google Scholar
[13] van Mechelen J L M, van der Marel D, Crassee I, and Kolodiazhnyi T 2011 Phys. Rev. Lett. 106 217601
Google Scholar
[14] Kampfrath T, Sell A, Klatt G, Pashkin A, Mährlein S, Dekorsy T, Wolf M, Fiebig M, Leitenstorfer A, Huber R 2011 Nat. Photonics 5 31
Google Scholar
[15] Zhou R Z, Jin Z M, Li G F, Li G F, Ma G H, Cheng Z X, Wang X L, 2012 Appl. Phys. Lett. 100 061102
Google Scholar
[16] Liu X M, Xie T, Guo J J, Yang S M, Song Y, Xian Lin, S X Cao, Cheng Z X, Jin Z M, Wu A H, Ma G H, Yao J S 2018 Appl. Phys. Lett. 113 022401
Google Scholar
[17] Siratori K 1971 J. Phys. Soc. Jpn. 30 709
Google Scholar
[18] Zhang P, Su F H, Chen X L, Zhang S L, Mei H Y, Yang Z R, Dai J M, Pi L 2016 Appl. Phys. Express 9 10
[19] Hemberger J, Krug von Nidda H A, Tsurkan V, Loidl A 2007 Phys. Rev. Lett. 98 147203
Google Scholar
[20] Murakawa H, Onose Y, Ohgushi K, Ishiwata S, Tokura Y 2008 J. Phys. Soc. Jpn. 77 043709
Google Scholar
[21] Guo J J, Cheng L, Zhuang R, Zhang W J, Lin X, Jin Z M, Cao S X, Sheng Z G, Ma G H 2020 J. Phys. Condens. Matter. 32 185401
Google Scholar
[22] Li X W, Bamba M, Yuan N, Zhang Q, ZhaoY G, Xiang M L, Xu K, Jin Z M, Wei R, Ma G H, Cao S X, Turchinovich D, Kono J 2018 Science 361 794
Google Scholar
[23] Lin X, Jiang J J, Jin Z M, Wang D Y, Tian Z, Han J G, Cheng Z X, Ma G H 2015 Appl. Phys. Lett. 106 092403
Google Scholar
-
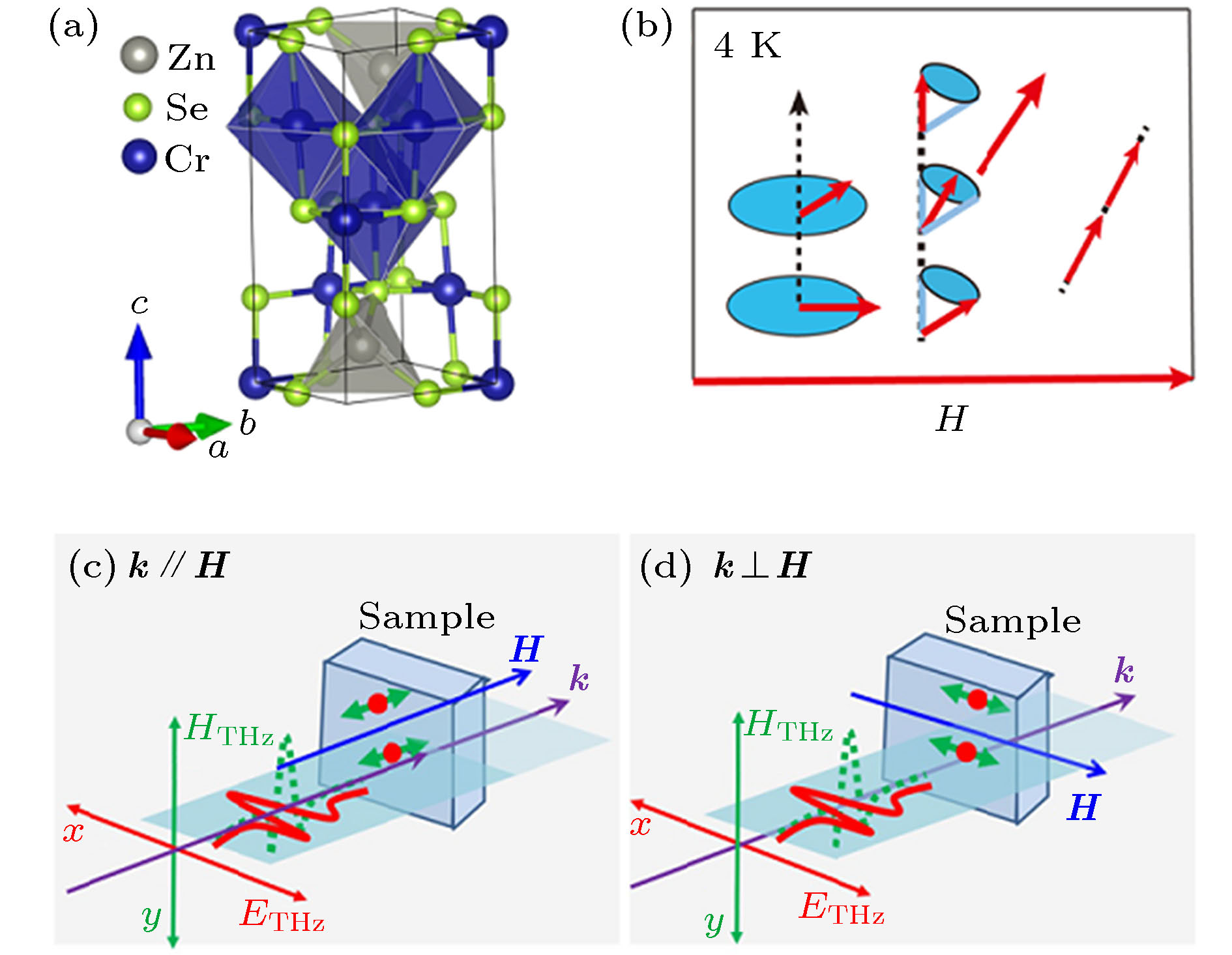
图 1 (a) ZnCr2Se4晶体结构; (b) 外磁场下ZnCr2Se4磁结构演化; (c) 外磁场下太赫兹透射测量配置示意图, 太赫兹波矢(k)平行于外磁场(H)方向; (d) k垂直于H配置, 对于两种测量配置, 太赫兹电场分量始终保持p型偏振, 从而确保太赫兹磁场分量和应用的稳态外部磁场保持正交
Fig. 1. (a) ZnCr2Se4 crystal structure; (b) the evolution of magnetic structure of ZnCr2Se4 under external magnetic field; (c) configuration of THz transmission measurements under external magnetic field, the THz wave vector (k) is parallel with external magnetic field (H); (d) the k is vertical with H. In both cases, the THz electric field (ETHz) is set as p polarization, and therefore the magnetic field component of THz waveform (HTHz) is perpendicular with steady external magnetic field.
图 2 (a) k∥H配置下, 不同外磁场下透过ZnCr2Se4单晶样品的THz时域波形图, 红色为不加样品时的时域信号, 幅度缩小5倍; (b) 这些时域波形图对应的快速傅里叶变换(FFT), 虚线为表征吸收位置变化的引导线
Fig. 2. (a) In the configuration of k//H, THz waveforms transmitted through ZnCr2Se4 single crystal measured under different magnetic fields at 4 K temperature. The red trace with the 0.2 scale factor is the reference waveform trough empty sample holder; (b) corresponding FFT amplitude spectra in frequency domain. The y axis is logarithmic scale. The dotted lines are guides for the eye.
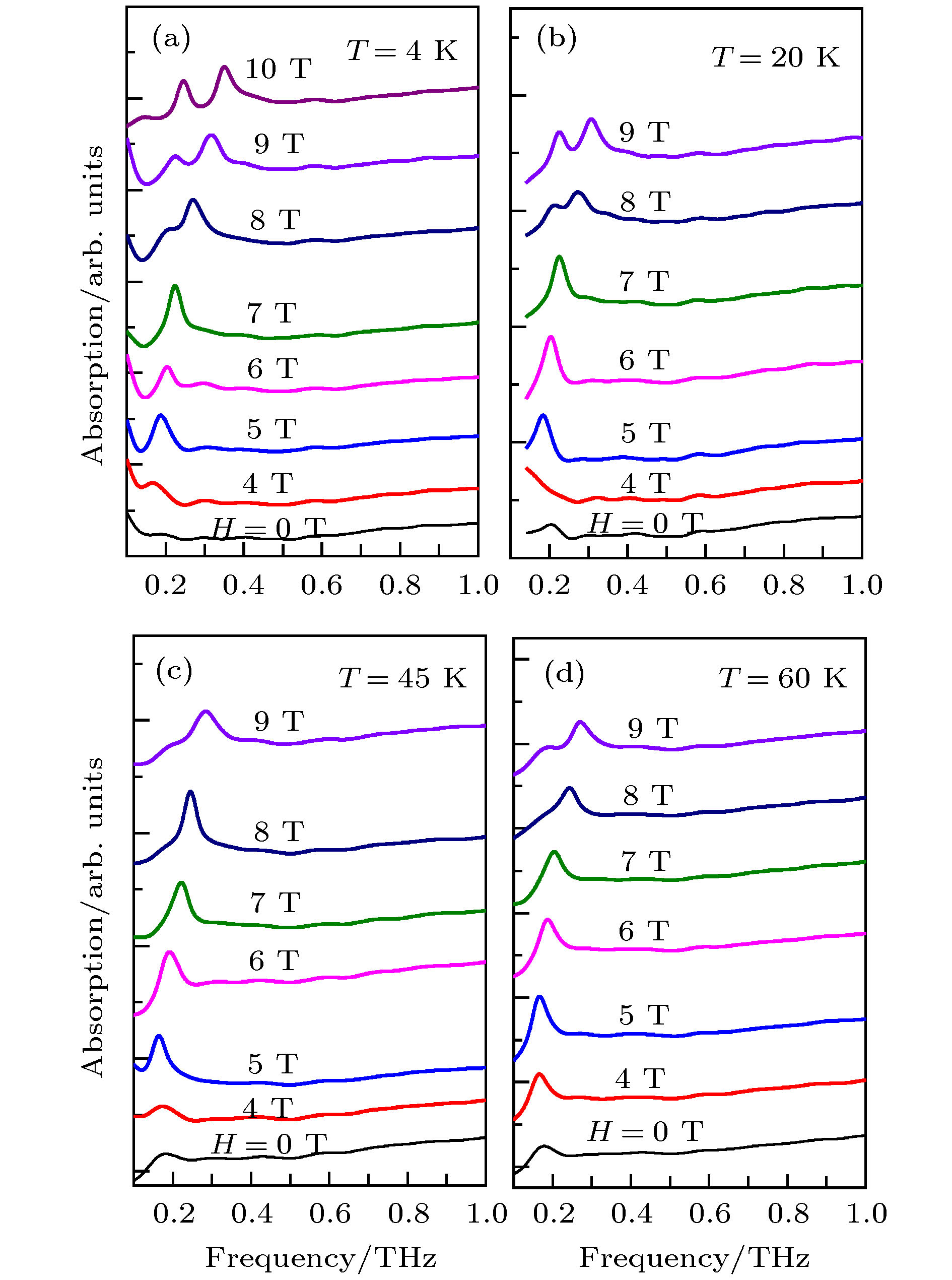
图 3 相对零磁场归一化的太赫兹透射谱 (a) 太赫兹波矢平行磁场配置; (b) 太赫兹波矢垂直磁场配置. 测量温度为4 K
Fig. 3. Normalized THz transmission spectra with respect to the spectrum without the application of external magnetic field: (a) THz wave vector is parallel with the external magnetic field; (b) THz wave vector is vertical with the external magnetic field. The measurement temperature is 4 K.
图 4 不同磁场下在如下温度中太赫兹吸收光谱曲线 (a) 4 K; (b) 20 K; (c) 45 K; (d) 60 K
Fig. 4. The THz absorption spectra obtained under different external magnetic fields at temperatures: (a) 4 K; (b) 20 K; (c) 45 K; (d) 60 K.
图 5 不同温度下共振频率随磁场的变化示意图 (a) 代表4 K温度下k∥H和k⊥H两种配置下测量结果, 红色实线表示根据关系式
$ \hslash \omega =g{\mu }_{\rm{B}}H$ , 对k⊥H配置测量数据的线性拟合, 灰色虚线代表对k∥H配置下的共振频率的线性外推; (b) k∥H配置下, 20, 45和60 K不同温度的测量结果, 红色实线代表根据关系式$ \hslash \omega =g{\mu }_{\rm{B}}H$ , 对T = 45 K数据的拟合Fig. 5. The frequencies at the maxima of the absorption spectra as a function of applied magnetic field at tempera-tures of 4 K (a), and 20, 45 and 60 K (b). In Fig.5 (a), the red solid line represents the fitting according to the equation
$ \hslash \omega =g{\mu }_{\rm{B}}H$ . The grey dash line denotes the linear extrapolation for the low-frequency absorption. In Fig. 5 (b), the red solid line is obtained from the fitting to the data taken at T = 45 K using the equation,$ \hslash \omega =g{\mu }_{\rm{B}}H$ . -
[1] Harris M J, Bramwell S T, McMorrow D F, Zeiske T, Godfrey K W 1988 Phys. Rev. Lett. 79 2554
[2] Chen G, Balents L, Schnyder A P 2009 Phys. Rev. Lett. 102 096406
Google Scholar
[3] Gu C C, Zhao Z Y, Chen X L, Lee M, Choi E S, Han Y Y, Ling L S, Pi L, Zhang Y H, Chen G, Yang Z R, Zhou H D, Sun X F 2018 Phys. Rev. Lett. 120 147204
Google Scholar
[4] Rudolf T, Kant Ch, Mayr F, Hemberger J, Tsurkan V, Loidl A 2007 Phys. Rev. B 75 052410
Google Scholar
[5] Akimitsu J, Siratori K, Shirane G, Iizumi M, Watanabe T 1978 J. Phys. Soc. Jpn. 44 172
Google Scholar
[6] Hidaka M, Tokiwa N, Fujii M, Watanabe S, Akimitsu J 2003 Phys. Status Solidi B 236 9
Google Scholar
[7] Plumier R J 1966 J. Appl. Phys. 37 964
Google Scholar
[8] Tymoshenko Y V, Onykiienko Y A, Müller T, Thomale R, Rachel S, Cameron A S, Portnichenko P Y, Efremov D V, Tsurkan V, Abernathy D L, Ollivier J, Schneidewind A, Piovano A, Felea V, Loidl A, Inosov D S 2017 Phys. Rev. X 7 041049
[9] Felea V, Yasin S, Gunther A, Deisenhofer J, Krug von Nidda H A, Zherlitsyn S, Tsurkan V, Lemmens P, Wosnitza J, Loidl A 2012 Phys. Rev. B 86 104420
Google Scholar
[10] Laurita N J, Deisenhofer J, Pan LiDong, Morris C M, Schmidt M, Johnsson M, Tsurkan V, Loidl A, Armitage N P 2015 Phys. Rev. Lett. 114 207201
Google Scholar
[11] Shuvaev A M, Travkin V D, Ivanov V Yu, Mukhin A A, Pimenov A 2010 Phys. Rev. Lett. 104 097202
Google Scholar
[12] 金钻明, 阮舜逸, 李炬赓, 林贤, 任伟, 曹世勋, 马国宏, 姚建铨 2019 68 167501
Google Scholar
Jin Z M, Yuan S Y, Li J G, Lin X, Ren W, Cao S X, Ma G H, Yao J S 2019 Acta Phys. Sin. 68 167501
Google Scholar
[13] van Mechelen J L M, van der Marel D, Crassee I, and Kolodiazhnyi T 2011 Phys. Rev. Lett. 106 217601
Google Scholar
[14] Kampfrath T, Sell A, Klatt G, Pashkin A, Mährlein S, Dekorsy T, Wolf M, Fiebig M, Leitenstorfer A, Huber R 2011 Nat. Photonics 5 31
Google Scholar
[15] Zhou R Z, Jin Z M, Li G F, Li G F, Ma G H, Cheng Z X, Wang X L, 2012 Appl. Phys. Lett. 100 061102
Google Scholar
[16] Liu X M, Xie T, Guo J J, Yang S M, Song Y, Xian Lin, S X Cao, Cheng Z X, Jin Z M, Wu A H, Ma G H, Yao J S 2018 Appl. Phys. Lett. 113 022401
Google Scholar
[17] Siratori K 1971 J. Phys. Soc. Jpn. 30 709
Google Scholar
[18] Zhang P, Su F H, Chen X L, Zhang S L, Mei H Y, Yang Z R, Dai J M, Pi L 2016 Appl. Phys. Express 9 10
[19] Hemberger J, Krug von Nidda H A, Tsurkan V, Loidl A 2007 Phys. Rev. Lett. 98 147203
Google Scholar
[20] Murakawa H, Onose Y, Ohgushi K, Ishiwata S, Tokura Y 2008 J. Phys. Soc. Jpn. 77 043709
Google Scholar
[21] Guo J J, Cheng L, Zhuang R, Zhang W J, Lin X, Jin Z M, Cao S X, Sheng Z G, Ma G H 2020 J. Phys. Condens. Matter. 32 185401
Google Scholar
[22] Li X W, Bamba M, Yuan N, Zhang Q, ZhaoY G, Xiang M L, Xu K, Jin Z M, Wei R, Ma G H, Cao S X, Turchinovich D, Kono J 2018 Science 361 794
Google Scholar
[23] Lin X, Jiang J J, Jin Z M, Wang D Y, Tian Z, Han J G, Cheng Z X, Ma G H 2015 Appl. Phys. Lett. 106 092403
Google Scholar
下载:
计量
- 文章访问数: 8123
- PDF下载量: 314
- 被引次数: 0
