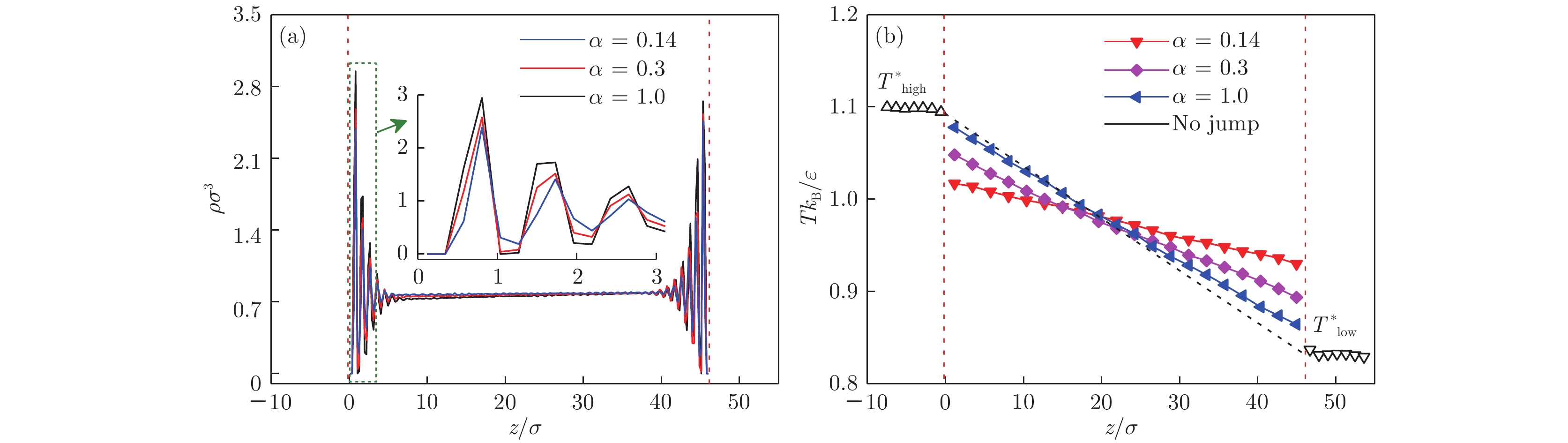
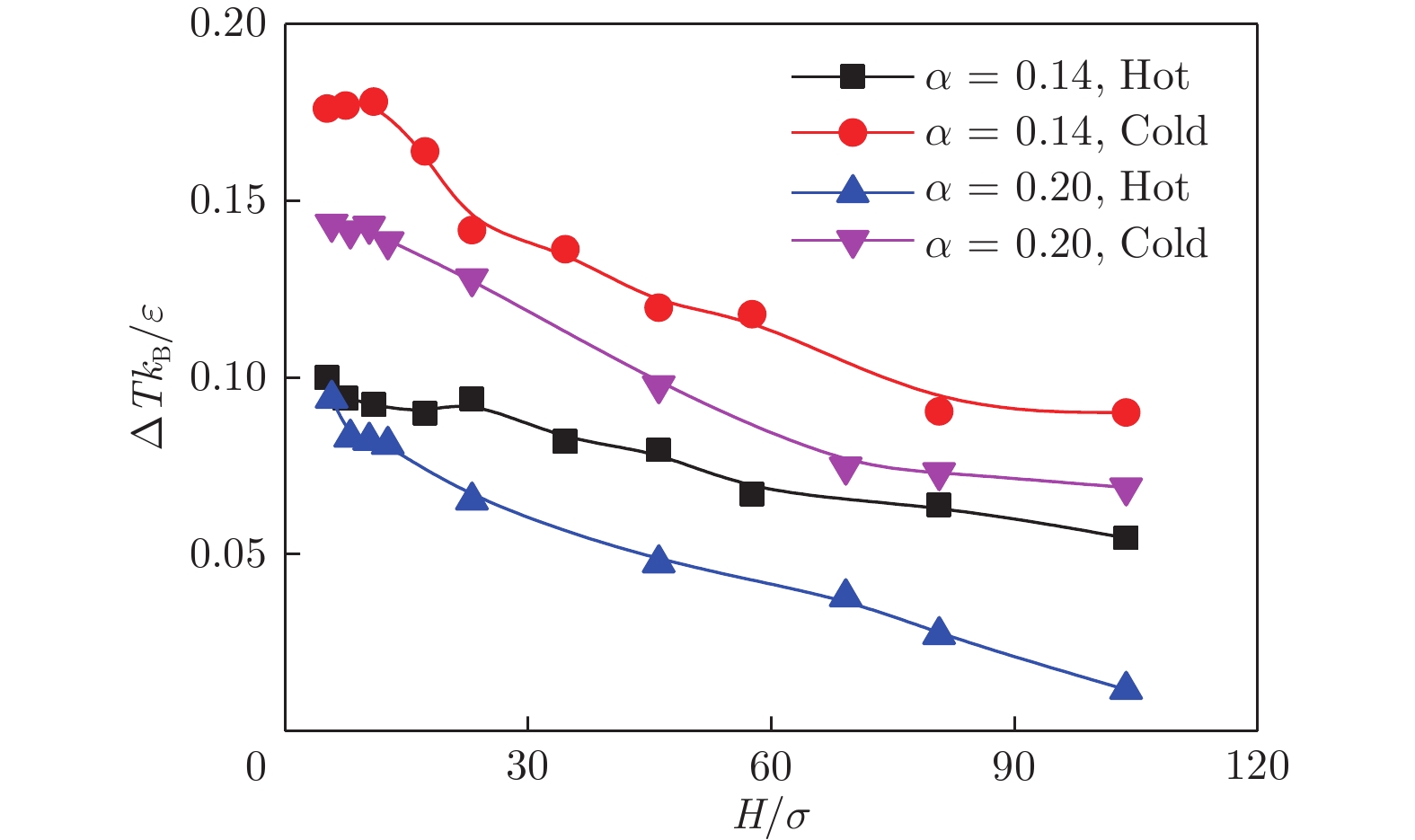
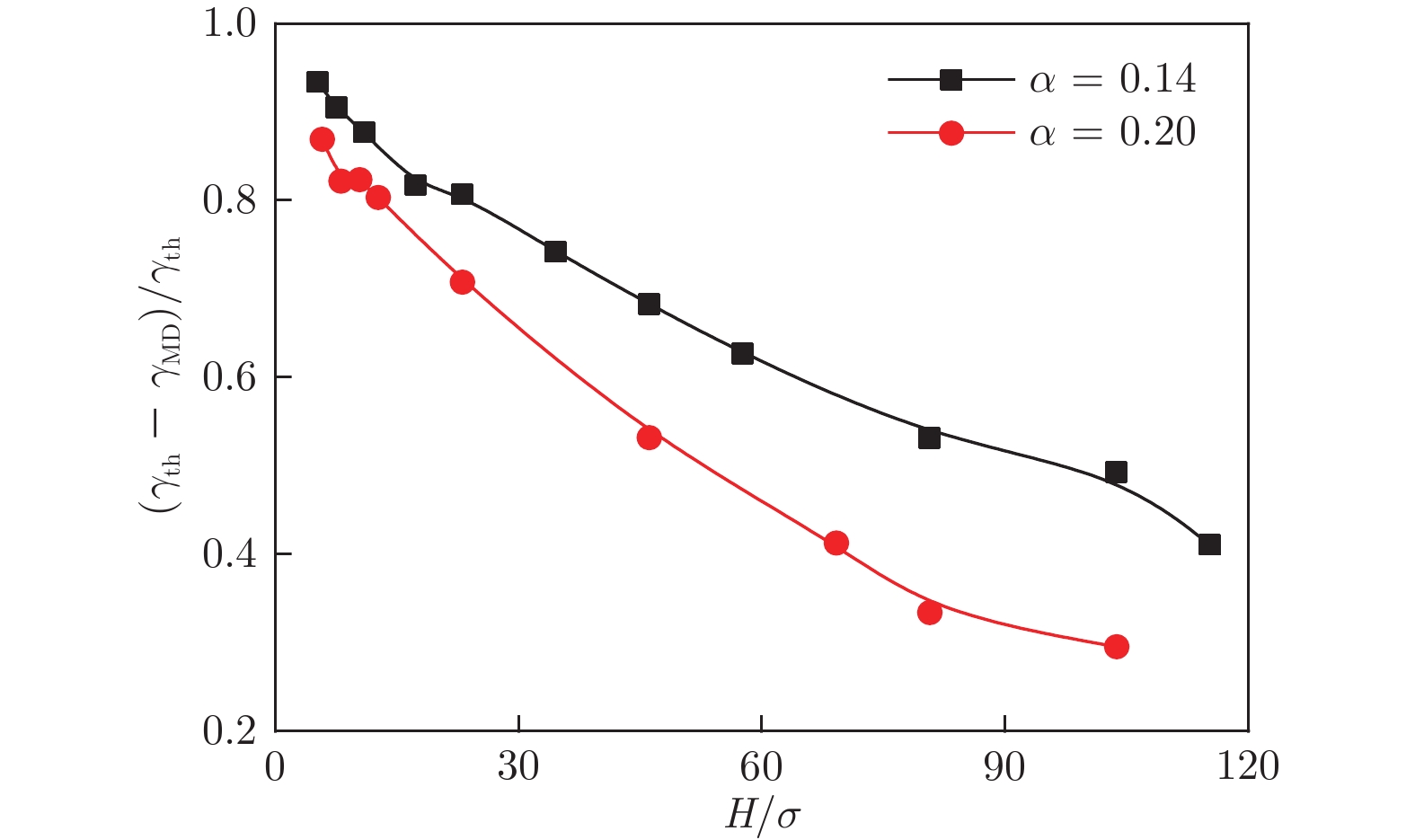
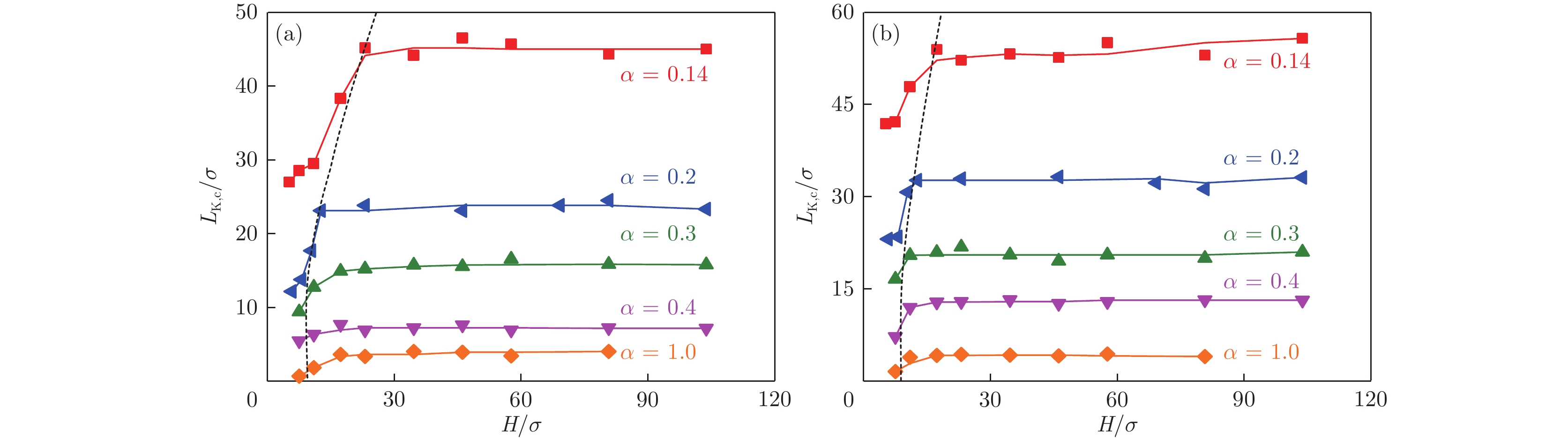
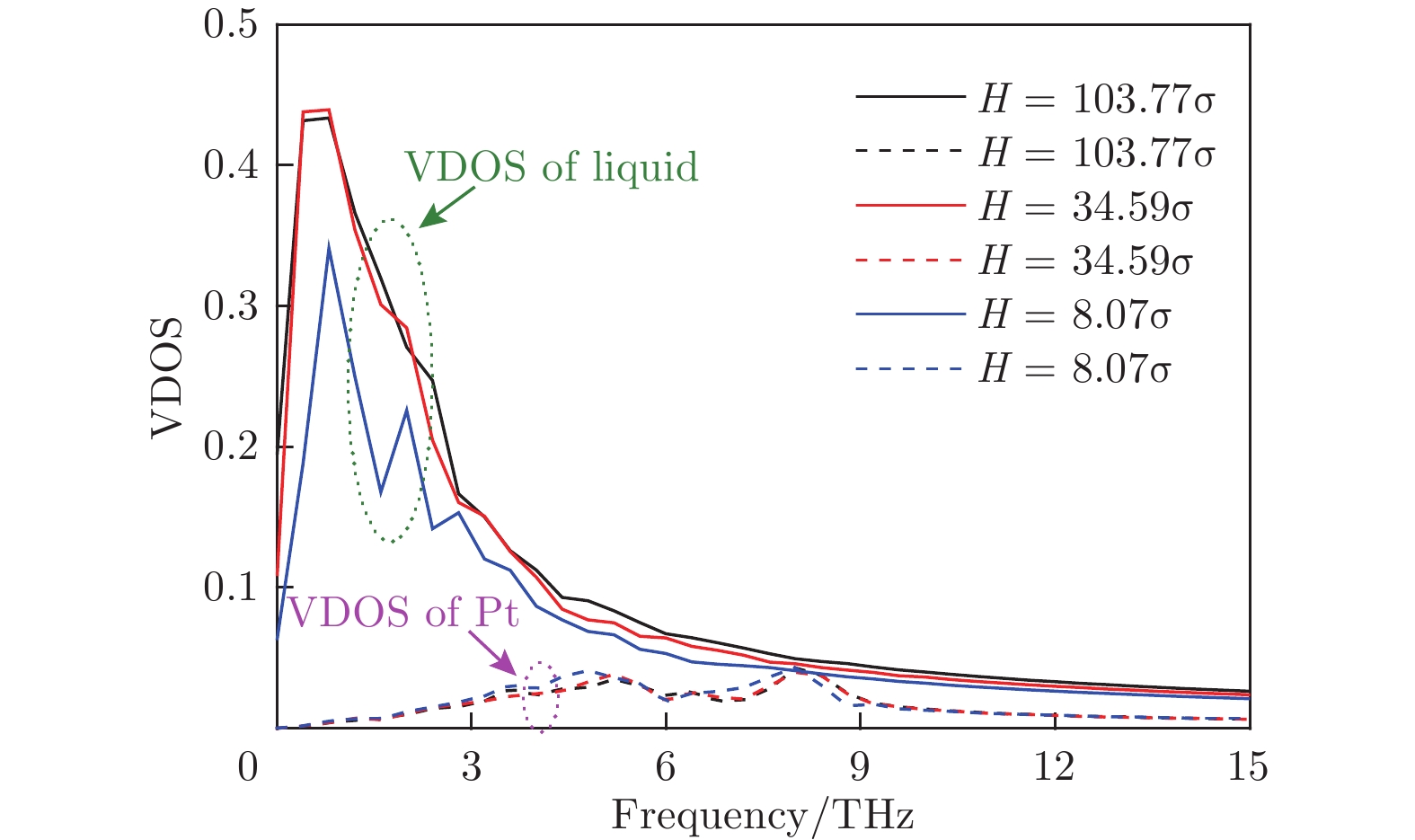
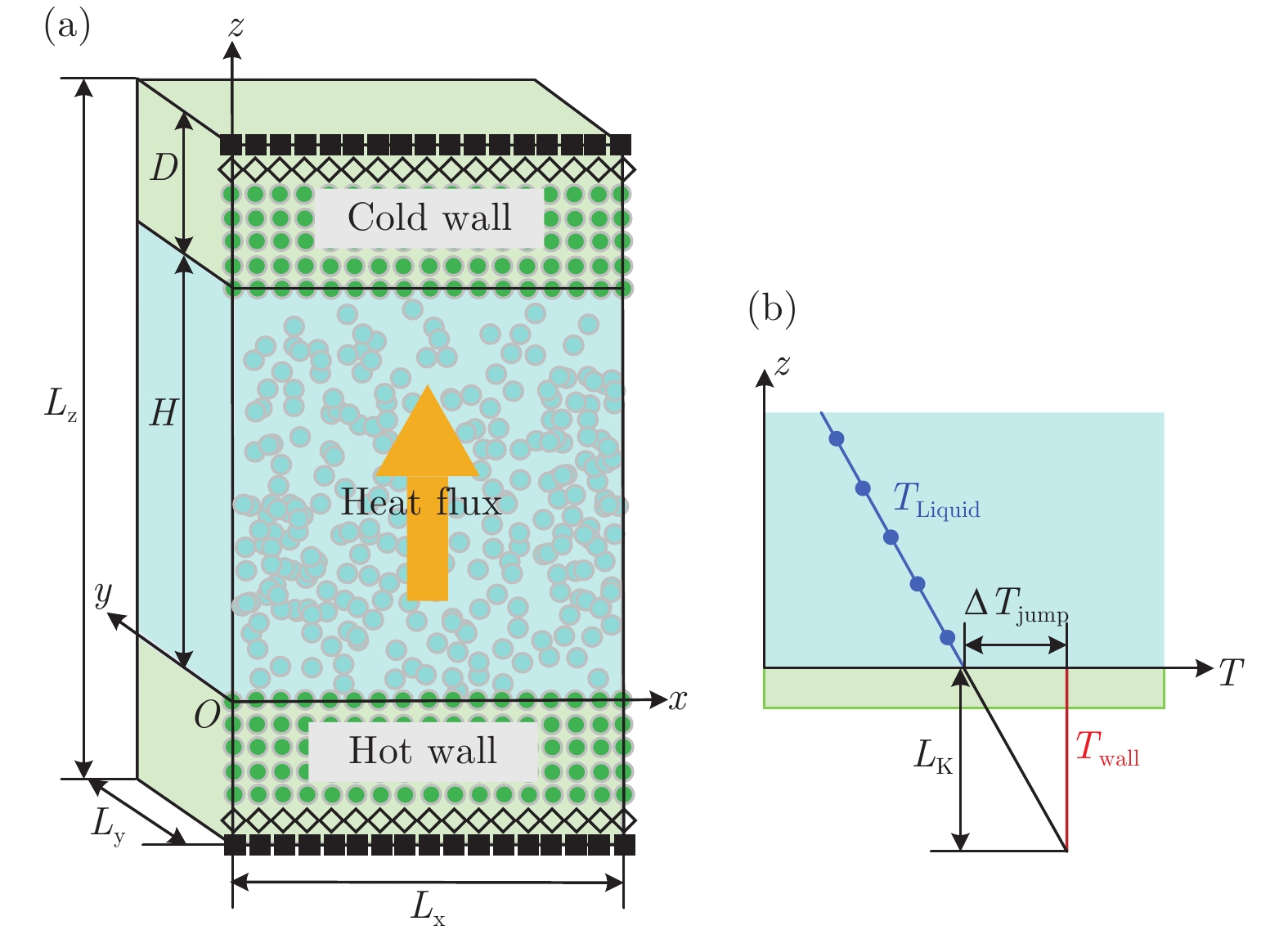
-
采用非平衡分子动力学方法模拟不同浸润性微通道内液体的传热过程, 分析了尺寸效应对固液界面热阻及温度阶跃的影响. 研究结果表明, 界面热阻随微通道尺寸的变化可分为两个阶段, 即小尺寸微通道的单调递增阶段和大尺寸微通道的恒定值阶段. 随着微通道尺寸的增加, 近壁区液体原子受对侧固体原子的约束程度降低, 微通道中央的液体原子自由移动, 固液原子振动态密度近似不变, 使得尺寸效应的影响忽略不计. 上述两种阶段的微通道尺寸过渡阈值受固液作用强度与壁面温度的共同作用: 减弱壁面浸润性, 过渡阈值向大尺寸区域迁移; 相较于低温壁面, 高温壁面处的过渡阈值更大. 增加微通道尺寸, 固液界面温度阶跃呈单调递减趋势, 致使壁面温度边界和宏观尺度下逐渐符合. 探讨尺寸效应有助于深刻理解固液界面能量输运及传递机制.The heat transfer in microchannel has attracted considerable attention due to many important applications in biology, chemistry, physics and engineering. When the fluid size shrinks to nanoscale, the energy transport of micro-system is significantly different from the conventional case. It is of great significance to study the size effect on heat transfer in a micro-system. However, there is a large size gap between existing molecular dynamics simulation and experimental measurement, in which the size effect on solid-liquid interfacial thermal resistance is rarely involved. Non-equilibrium molecular dynamics simulation is performed to investigate the heat transfer through the solid-liquid interface. Simple Lennard-Jones (LJ) fluid is simulated as the ultra-thin liquid film in a non-equilibrium simulation system. The liquid film is confined in a nanochannel composed of two solid surfaces. The potential function between solid and liquid atom is represented by a modified LJ function to control the solid-liquid interfaces of different surface wettabilities. We examine the size effect on temperature jump and thermal resistance at the solid-liquid interface. The fluid number density and temperature distribution in the perpendicular direction of solid wall are evaluated. It is found that the liquid atoms near wall are arranged as a solid-like structure. Particularly in the small channel, liquid atoms confined in the channel are affected by two solid walls. However, with the increase of channel height, the liquid atoms in the middle channel move freely, leading to the decrease of the size effect. The simulation results show that the dependence of thermal resistance on microchannel height exhibits two regimes: (i) monotonically increasing dependence for the small channel and (ii) keeping constant thermal resistance for the large channel. These two distinct trends can be explained by phonon vibrational density of states (VDOS) of solid wall and liquid. For the small channel, a stronger confinement of liquid leads to a weaker mismatch in VDOS of solid wall and liquid, thus resulting in a smaller thermal resistance. Whereas, for the large channel, the vibrational coupling between the solid and the liquid atom remains unchanged and the size effect is negligible. The size thresholds of the two regimes of the thermal resistance are both sensitive to the liquid-solid interaction strength, which decreases with solid-liquid interaction increasing. Furthermore, with the increase of the microchannel height, the temperature jump at the solid-liquid interface monotonically decreases and eventually approaches to the non-jump temperature boundary on a macroscopic scale. These findings may help to understand the mechanism of temperature boundary conditions on a microscopic scale and a macroscopic scale and provide a theoretical support for manufacturing new nano-devices.
-
Keywords:
- size effect /
- interface thermal resistance /
- temperature jump /
- molecular dynamics
[1] Bocquet L R, Barrat J 2007 Soft Matter 3 685
Google Scholar
[2] Caplan M E, Giri A, Hopkins P E 2014 J. Chem. Phys. 140 154701
Google Scholar
[3] Feng Y, Liang X 2015 Int. J. Thermophys. 36 1519
Google Scholar
[4] Swartz E T, Pohl R O 1989 Rev. Mod. Phys. 61 605
Google Scholar
[5] Cahill D G, Ford W K. Goodson K E, Mahan G D, Majumdar A, Maris H J, Merlin R, Phillpot S R 2003 J. Appl. Phys. 93 793
Google Scholar
[6] Barrat J L, Chiaruttini F 2003 Mol. Phys. 101 1605
Google Scholar
[7] Ge Z B, Cahill D G, Braun V 2006 Phys. Rev. Lett. 96 186101
Google Scholar
[8] 张程宾, 许兆林, 陈永平 2014 63 214706
Google Scholar
Zhang C B, Xu Z L, Chen Y P 2014 Acta Phys. Sin. 63 214706
Google Scholar
[9] Xu J L, Li Y X 2007 Int. J. Heat Mass Transf. 50 2571
Google Scholar
[10] Xue L, Keblinski P, Phillpot S R, Choi U S, Eastman J A 2004 Int. J. Heat Mass Transf. 47 4277
Google Scholar
[11] Caplan M E, Giri A, Hopkins P E 2014 J. Chem. Phys. 140 154701
Google Scholar
[12] 葛宋, 陈民 2013 62 110204
Google Scholar
Ge S, Chen M 2013 Acta Phys. Sin. 62 110204
Google Scholar
[13] Ge S, Chen M 2013 Int. J. Thermophys. 34 64
Google Scholar
[14] Li Q, Liu C 2012 Int. J. Heat Mass Transf. 55 8088
Google Scholar
[15] Chen Y, Zhang C 2014 Int. J. Heat Mass Transf. 78 624
Google Scholar
[16] Murad S, Puri I K 2008 Chem. Phys. Lett. 467 110
Google Scholar
[17] Vera J, Bayazitoglu Y 2015 Int. J. Heat Mass Transf. 86 433
Google Scholar
[18] Sun J, Wang W, Wang H S 2013 J. Chem. Phys. 87 234703
Google Scholar
[19] Liang Z, Tsai H L 2011 Phys. Rev. E. 83 061603
Google Scholar
[20] Wang X, Cheng P, Quan X 2016 Int. Commun. Heat Mass Transfer. 77 183
Google Scholar
[21] Kim B H, Beskok A, Cagin T 2008 J. Chem. Phys. 129 174701
Google Scholar
[22] Chen Z, Jang W, Bao W, Lau C N, Dames C 2009 Appl. Phys. Lett. 95 161910
Google Scholar
[23] Nagayama G, Tsuruta T, Cheng P 2006 Int. J. Heat Mass Transf. 49 4437
Google Scholar
[24] Nagayama G, Kawagoe M, Tokunaga A, Tsuruta T 2010 Int. J. Therm. Sci. 49 59
Google Scholar
[25] Pan Y, Poulikakos D, Walther J, Yadigaroglu G 2002 Int. J. Heat Mass Transf. 4 2087
Google Scholar
[26] She X, Shedd T A, Lindeman B, Yin Y, Zhang X 2016 Int. J. Heat Mass Transf. 95 278
Google Scholar
[27] Giri A, Hopkins P E 2014 Appl. Phys. Lett. 105 033106
Google Scholar
[28] Stevens R J, Zhigilei L V, Norris P M 2007 Int. J. Heat Mass Transf. 50 3977
Google Scholar
[29] Wilson R B, Cahill D G 2014 Nat. Commun. 5 5075
Google Scholar
[30] Hua Y C, Chan B Y 2017 Nanoscale Microscale Thermophys. Eng. 21 159
Google Scholar
[31] Hu H, Sun Y 2012 J. Appl. Phys. 112 053508
Google Scholar
-
图 1 (a) 物理模型图; (b) 界面热阻长度定义
Fig. 1. (a) Physical model of system; (b) definition of thermal resistance length at interface.
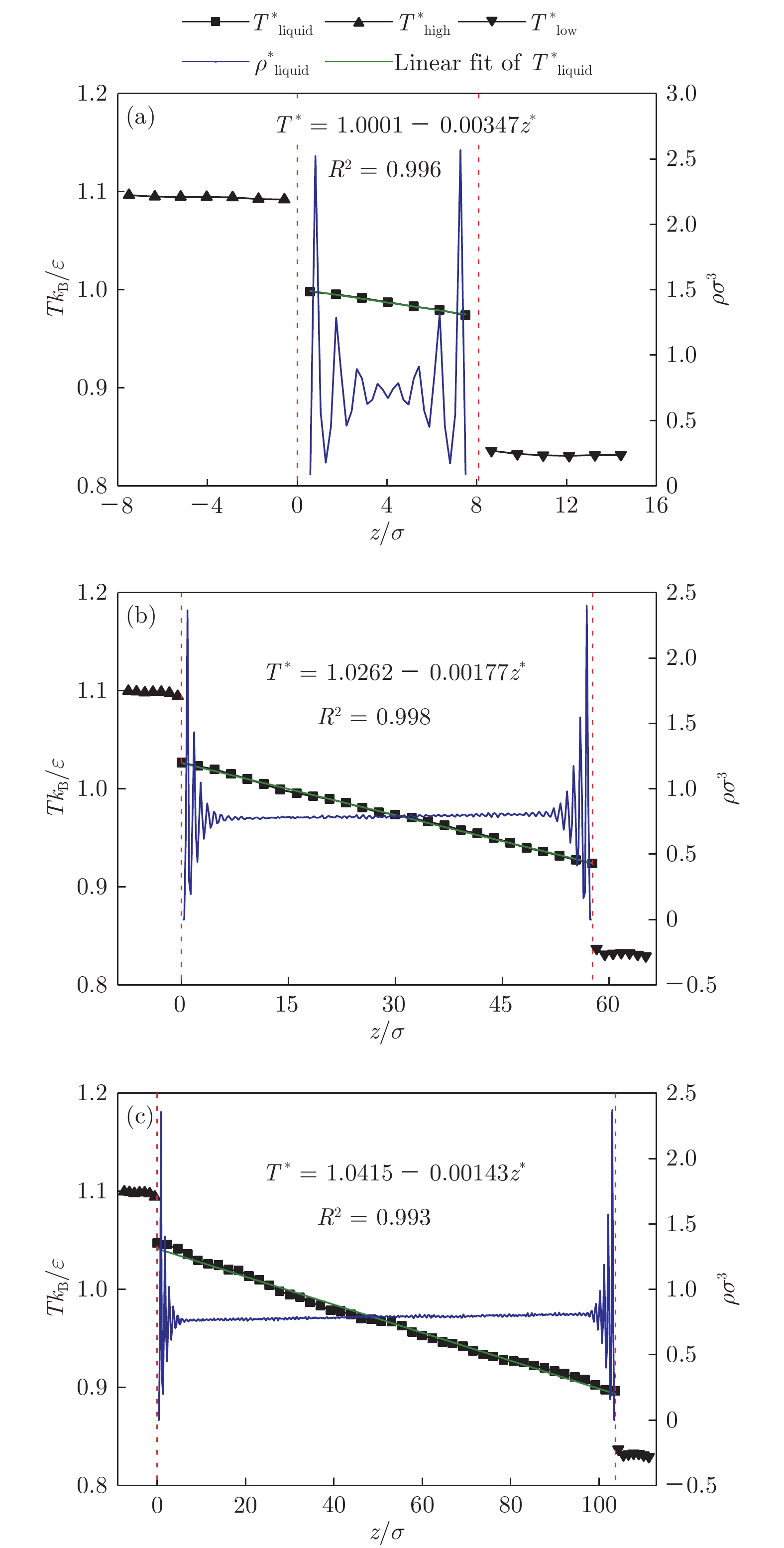
图 2 固液势能参数
$\alpha = 0.14$ 时, 流体沿着z方向的温度分布和密度分布 (a)$H = 8.07\sigma $ ; (b)$H = 57.65\sigma $ ; (c)$H = 103.77\sigma $ Fig. 2. Temperature and density profile of liquid along z direction when solid-liquid potential energy parameter
$\alpha = 0.14$ : (a)$H = 8.07\sigma $ ; (b)$H = 57.65\sigma $ ; (c)$H =$ $103.77\sigma $ .
图 3 微通道尺寸
$H = 46.12\sigma $ 时流体沿着z方向的(a) 密度分布和(b)温度分布Fig. 3. (a) Density profile and (b) temperature profile of liquid along z direction when microchannel height H = 46.12σ.
图 4 固液界面温度阶跃随微通道尺寸的变化
Fig. 4. Variation of temperature jump at solid-liquid interface with microchannel height.
图 5 液体实际温度与理论温度的接近程度随微通道尺寸的变化关系
Fig. 5. Degree of closeness of actual and theoretical temperature of liquid versus microchannel height.
图 6 固液界面热阻随微通道尺寸的变化 (a) 热壁面; (b) 冷壁面
Fig. 6. Variation of thermal resistance at solid-liquid interface with microchannel height: (a) Hot wall; (b) cold wall.
图 7 固液势能参数为
$\alpha = 0.14$ 时固液原子VDOS分布Fig. 7. VDOS profile of solid atoms and liquid atoms located at interfaces when solid-liquid potential energy parameter
$\alpha = 0.14$ . -
[1] Bocquet L R, Barrat J 2007 Soft Matter 3 685
Google Scholar
[2] Caplan M E, Giri A, Hopkins P E 2014 J. Chem. Phys. 140 154701
Google Scholar
[3] Feng Y, Liang X 2015 Int. J. Thermophys. 36 1519
Google Scholar
[4] Swartz E T, Pohl R O 1989 Rev. Mod. Phys. 61 605
Google Scholar
[5] Cahill D G, Ford W K. Goodson K E, Mahan G D, Majumdar A, Maris H J, Merlin R, Phillpot S R 2003 J. Appl. Phys. 93 793
Google Scholar
[6] Barrat J L, Chiaruttini F 2003 Mol. Phys. 101 1605
Google Scholar
[7] Ge Z B, Cahill D G, Braun V 2006 Phys. Rev. Lett. 96 186101
Google Scholar
[8] 张程宾, 许兆林, 陈永平 2014 63 214706
Google Scholar
Zhang C B, Xu Z L, Chen Y P 2014 Acta Phys. Sin. 63 214706
Google Scholar
[9] Xu J L, Li Y X 2007 Int. J. Heat Mass Transf. 50 2571
Google Scholar
[10] Xue L, Keblinski P, Phillpot S R, Choi U S, Eastman J A 2004 Int. J. Heat Mass Transf. 47 4277
Google Scholar
[11] Caplan M E, Giri A, Hopkins P E 2014 J. Chem. Phys. 140 154701
Google Scholar
[12] 葛宋, 陈民 2013 62 110204
Google Scholar
Ge S, Chen M 2013 Acta Phys. Sin. 62 110204
Google Scholar
[13] Ge S, Chen M 2013 Int. J. Thermophys. 34 64
Google Scholar
[14] Li Q, Liu C 2012 Int. J. Heat Mass Transf. 55 8088
Google Scholar
[15] Chen Y, Zhang C 2014 Int. J. Heat Mass Transf. 78 624
Google Scholar
[16] Murad S, Puri I K 2008 Chem. Phys. Lett. 467 110
Google Scholar
[17] Vera J, Bayazitoglu Y 2015 Int. J. Heat Mass Transf. 86 433
Google Scholar
[18] Sun J, Wang W, Wang H S 2013 J. Chem. Phys. 87 234703
Google Scholar
[19] Liang Z, Tsai H L 2011 Phys. Rev. E. 83 061603
Google Scholar
[20] Wang X, Cheng P, Quan X 2016 Int. Commun. Heat Mass Transfer. 77 183
Google Scholar
[21] Kim B H, Beskok A, Cagin T 2008 J. Chem. Phys. 129 174701
Google Scholar
[22] Chen Z, Jang W, Bao W, Lau C N, Dames C 2009 Appl. Phys. Lett. 95 161910
Google Scholar
[23] Nagayama G, Tsuruta T, Cheng P 2006 Int. J. Heat Mass Transf. 49 4437
Google Scholar
[24] Nagayama G, Kawagoe M, Tokunaga A, Tsuruta T 2010 Int. J. Therm. Sci. 49 59
Google Scholar
[25] Pan Y, Poulikakos D, Walther J, Yadigaroglu G 2002 Int. J. Heat Mass Transf. 4 2087
Google Scholar
[26] She X, Shedd T A, Lindeman B, Yin Y, Zhang X 2016 Int. J. Heat Mass Transf. 95 278
Google Scholar
[27] Giri A, Hopkins P E 2014 Appl. Phys. Lett. 105 033106
Google Scholar
[28] Stevens R J, Zhigilei L V, Norris P M 2007 Int. J. Heat Mass Transf. 50 3977
Google Scholar
[29] Wilson R B, Cahill D G 2014 Nat. Commun. 5 5075
Google Scholar
[30] Hua Y C, Chan B Y 2017 Nanoscale Microscale Thermophys. Eng. 21 159
Google Scholar
[31] Hu H, Sun Y 2012 J. Appl. Phys. 112 053508
Google Scholar
下载:
计量
- 文章访问数: 8739
- PDF下载量: 116
- 被引次数: 0
