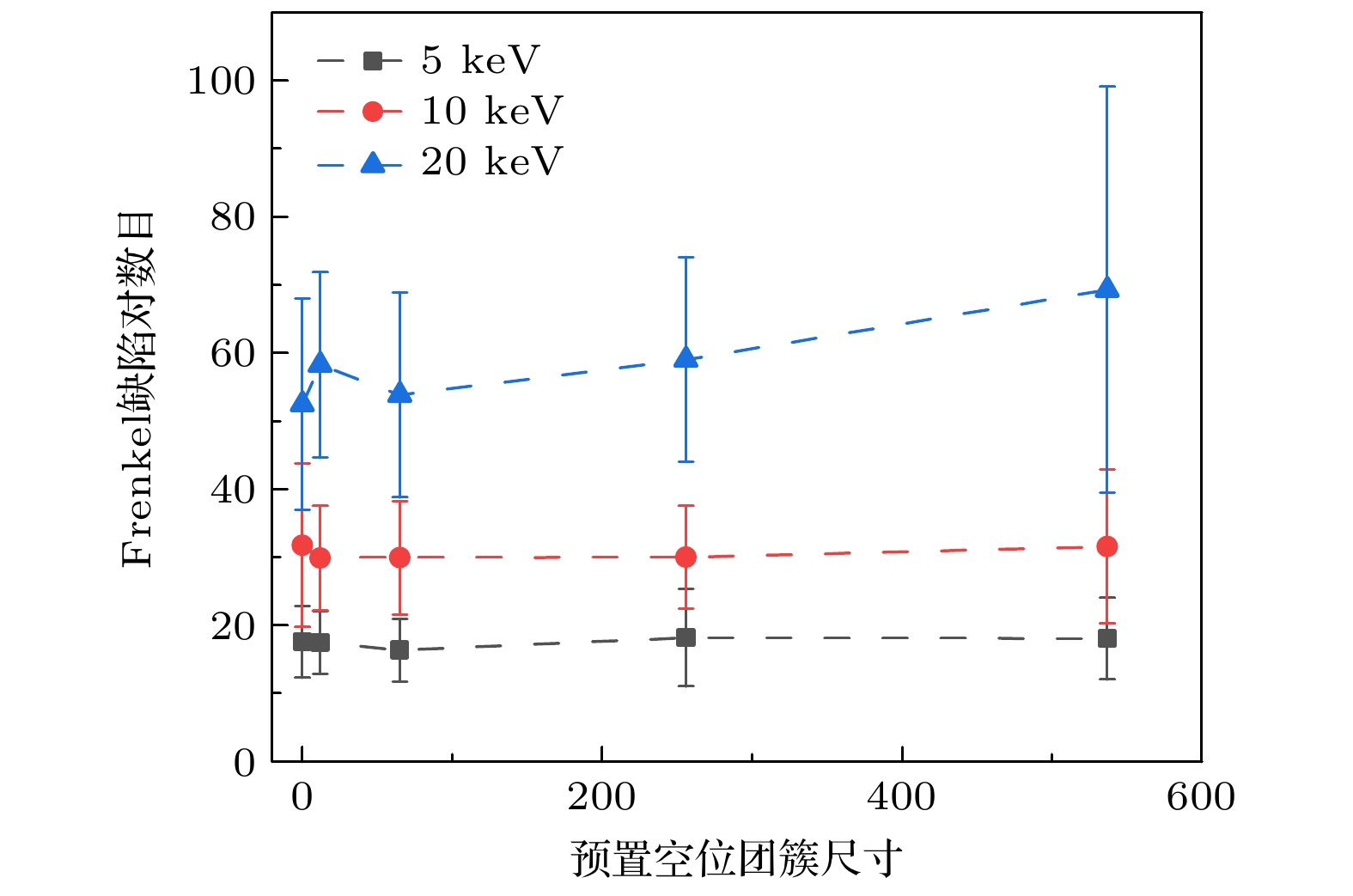
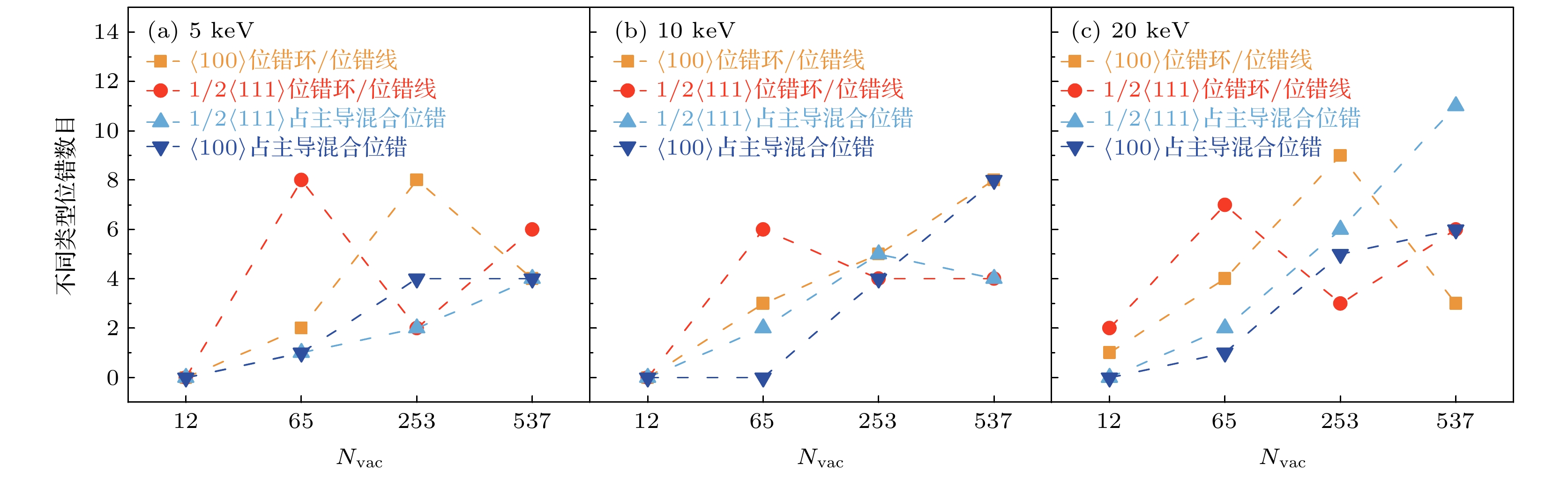
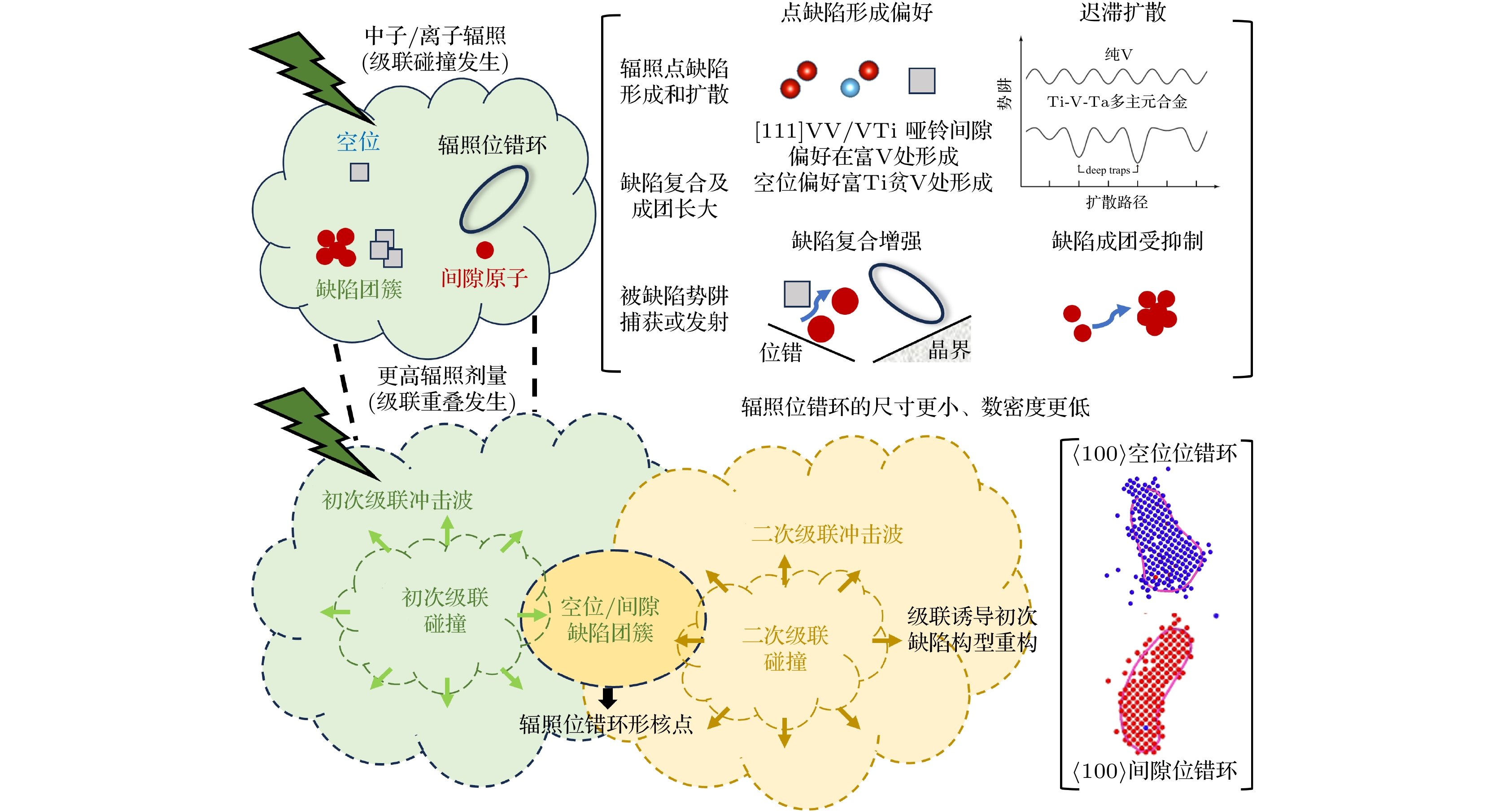
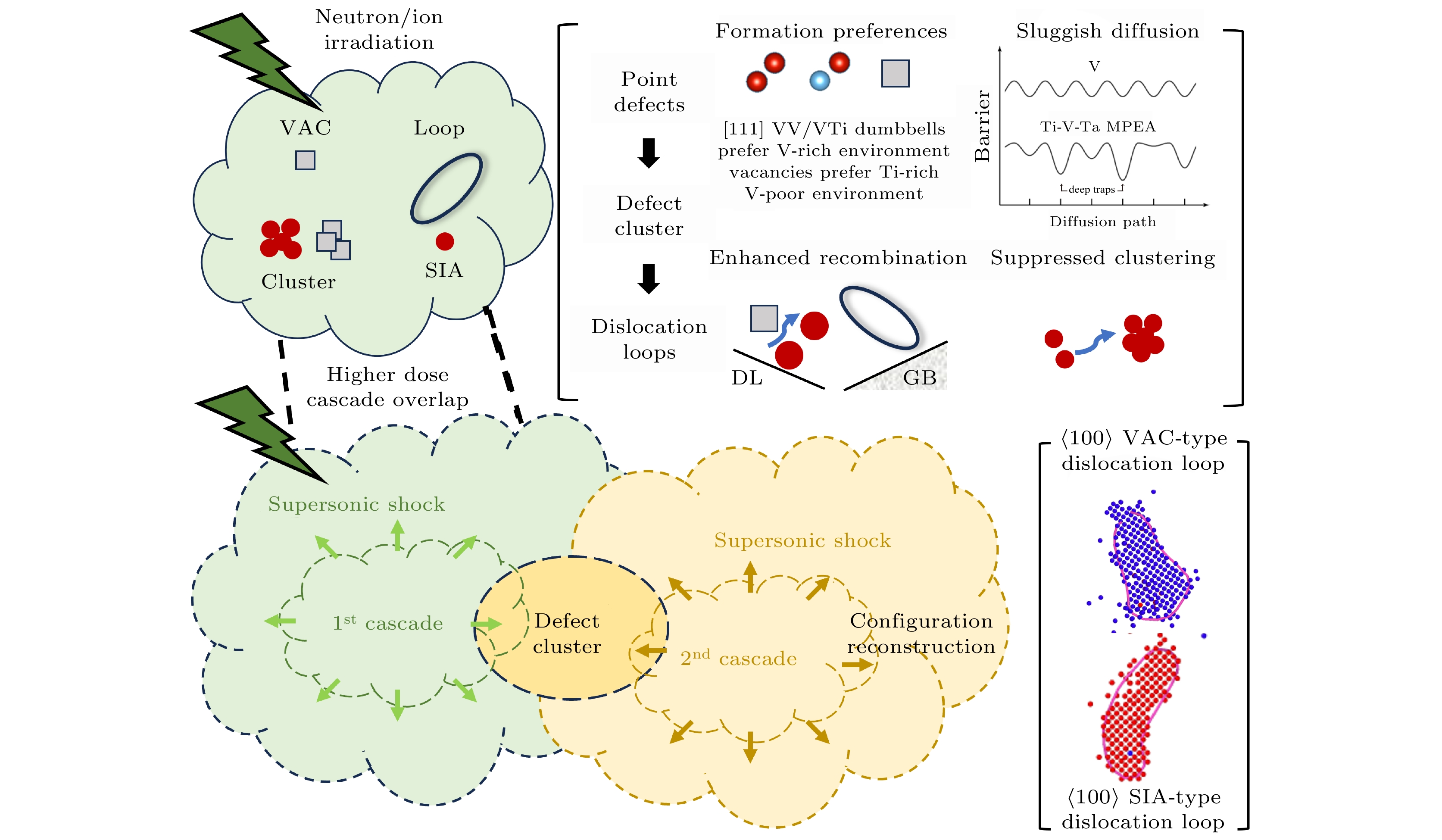
-
针对Ti-V-Ta多主元合金中辐照位错环的形成行为, 采用分子动力学方法开展了级联重叠模拟, 分析讨论了辐照位错环形成的级联重叠机制. 研究发现, 在Ti-V-Ta多主元合金中, 与缺陷团簇的级联重叠可以直接产生不同类型的位错结构, 级联重叠后的缺陷构型由PKA能量和预置缺陷团簇的类型和尺寸决定. 相比于单次级联碰撞, 级联重叠可以提高$ \left\langle {100} \right\rangle $取向位错环的形成概率. 与空位团簇的级联重叠是形成$ \left\langle {100} \right\rangle $空位位错环的重要机制, 而空位团簇的尺寸是形成$ \left\langle {100} \right\rangle $空位位错环的决定因素, 当PKA能量足以溶解缺陷团簇时, 更容易形成$ \left\langle {100} \right\rangle $空位位错环. 与间隙团簇的级联重叠是形成$ \left\langle {100} \right\rangle $间隙位错环的一种可能机制, 但发生概率较小. 本研究有助于理解Ti-V-Ta多主元合金中辐照缺陷的形成和演化行为, 促进材料抗辐照性能的评价, 并为难熔高熵合金的成分设计和优化提供理论支持.
-
关键词:
- Ti-V-Ta多主元合金 /
- 辐照位错环 /
- 级联重叠 /
- 分子动力学
Among the currently developed multi-principal element alloys (MPEAs), Ti-V-Ta MPEA stands out because of its good high-temperature strength, good room-temperature plasticity, stable organizational structure, and low neutron activation, and becomes a first option for cladding material of special power reactors. The radiation resistance of Ti-V-Ta MPEA is the focus of current research. Dislocation loops are the main irradiation defects in Ti-V-Ta MPEA, which can significantly affect the mechanical properties. Therefore, clarifying the formation mechanism of dislocation loops in Ti-V-Ta HEA can help to understand its radiation resistance. The formation behavior of dislocation loops in Ti-V-Ta MPEA is studied based on molecular dynamics method in this work. Cascade overlap simulations with vacancy clusters and interstitial clusters are carried out. The cascade overlap formation mechanism of dislocation loops is analyzed and discussed. In Ti-V-Ta MPEA, the cascade overlap with defect clusters can directly produce different types of dislocation structures. The defect configuration after cascade overlap is determined by the primary knock-on atom (PKA) energy and the type and size of the preset defect clusters. Cascade overlap can improve the formation probability of $ \left\langle {100} \right\rangle $ dislocation loops in Ti-V-Ta MPEA. Cascade overlap with vacancy clusters is an important mechanism for the formation of $ \left\langle {100} \right\rangle $ vacancy dislocation loops, and the size of vacancy clusters is the dominant factor for the formation of $ \left\langle {100} \right\rangle $ vacancy dislocation loops. When the PKA energy is enough to dissolve the defect clusters, $ \left\langle {100} \right\rangle $ vacancy dislocation loops are more likely to form. Furthermore, cascade overlap with interstitial clusters in Ti-V-Ta MPEA is a possible mechanism for the formation of $ \left\langle {100} \right\rangle $ interstitial dislocation loops. This study can contribute to understanding the evolution behavior of irradiation defects in Ti-V-Ta MPEA, and provide theoretical support for designing the composition and optimizing the high-entropy alloys.
-
Keywords:
- Ti-V-Ta multi-principal element alloy /
- dislocation loop /
- cascade overlap /
- molecular dynamics
[1] El-Genk M S, Tournier J M 2005 J. Nucl. Mater. 340 93
Google Scholar
[2] Busby J T, Leonard K J 2007 JOM 59 20
[3] Yeh J W, Chen S K, Lin S J, Gan J Y, Chin T S, Shun T T, Tsau C H, Chang S Y 2004 Adv. Eng. Mater. 6 299
Google Scholar
[4] Tsai M H, Yeh J W 2014 Mater. Res. Lett. 2 107
Google Scholar
[5] Ye Y F, Wang Q, Lu J, Liu C T, Yang Y 2016 Mater. Today 19 349
Google Scholar
[6] Miracle D B, Senkov O N 2017 Acta Mater. 122 448
Google Scholar
[7] George E P, Raabe D, Ritchie R O 2019 Nat. Rev. Mater. 4 515
Google Scholar
[8] Pickering E J, Carruthers A W, Barron P J, Middleburgh S C, Armstrong D E J, Gandy A S 2021 Entropy 23 98
Google Scholar
[9] Jia N N, Li Y K, Liu X, Zheng Y, Wang B P, Wang J S, Xue Y F, Jin K 2019 JOM. 71 3490
Google Scholar
[10] Hu B, Yao B, Wang J, Liu Y, Wang C J, Du Y, Yin H Q 2020 Intermetallics 118 106701
Google Scholar
[11] Yin X, Dou Y K, He X F, Jin K, Wang C L, Dong Y G, Wang H R, Zhong W H, Xue Y F, Yang W 2022 JOM 74 4326
Google Scholar
[12] Jia N N, Li Y, Huang H F, Chen S, Li D, Dou Y K, He X F, Yang W, Yin X, Jin K 2021 J. Nucl. Mater. 550 152937
Google Scholar
[13] Mei L, Zhang Q H, Dou Y K, Fu E G, Li L, Chen S, Dong Y G, Guo X, He X F, Yang W, Yin X, Jin K 2023 Scr. Mater. 223 115070
Google Scholar
[14] Dou Y K, Zhao Y P, He X F, Gao J, Cao J L, Yang W 2023 J. Nucl. Mater. 573 154096
Google Scholar
[15] Zhao Y P, Dou Y K, He X F, Deng H Q, Wang L F, Yang W 2023 Comput. Mater. Sci. 218 111943
Google Scholar
[16] Zhao Y P, Dou Y K, He X F, Cao H, Wang L F, Deng H Q, Yang W 2024 Chin. Phys. B 33 036104
Google Scholar
[17] Peng Q, Meng F J, Yang Y Z, Lu C Y, Deng H Q, Wang L M, De S, Gao F 2018 Nat. Commun. 9 4880
Google Scholar
[18] Granberg F, Byggmästar J, Sand A E, Nordlund K 2017 Europhys. Lett. 119 56003
Google Scholar
[19] Byggmästar J, Granberg F, Sand A E, Pirttikoski A, Alexander R, Marinica M C, Nordlund K 2019 J. Phys. Condens. Matter. 31 245402
Google Scholar
[20] Wang X Y, Gao N, Wang Y N, Liu H L, Shu G G, Li C L, Xu B, Liu W 2019 J. Nucl. Mater. 519 322
Google Scholar
[21] Marinica M C, Willaime F, Crocombette J P 2012 Phys. Rev. Lett. 108 025501
Google Scholar
[22] Gao J, Gaganidze E, Kaiser B, Aktaa J 2021 J. Nucl. Mater. 557 153212
Google Scholar
[23] Esfandiarpour A, Byggmästar J, Balbuena J P, Caturla M J, Nordlund K, Granberg F 2022 Materialia. 21 101344
Google Scholar
[24] Arakawa K, Hatanaka M, Kuramoto E, Ono K, Mori H 2006 Phys. Rev. Lett. 96 125506
Google Scholar
[25] Chen J, Gao N, Jung P, Sauvage T 2013 J. Nucl. Mater. 441 216
Google Scholar
[26] Gao N, Chen J, Kurtz R J, Wang Z G, Zhang R F, Gao F 2017 J. Phys. Condens. Matter. 29 455301
Google Scholar
[27] Xu H, Stoller R E, Osetsky Y N, Terentyev D 2013 Phys. Rev. Lett. 110 265503
Google Scholar
[28] Wang X Y, Gao N, Wang Y N, Wu X Y, Shu G G, Li C L, Li Q L, Xu B, Liu W 2019 Scr. Mater. 162 204
Google Scholar
[29] Plimpton S 1995 J. Comput. Phys. 117 1
Google Scholar
[30] Qiu R Y, Chen Y C, Liao X C, He X F, Yang W, Hu W Y, Deng H Q 2021 J. Nucl. Mater. 557 153231
Google Scholar
[31] Stukowski A 2010 Model. Simul. Mater. Sc. 18 015012
Google Scholar
[32] Fellman A, Sand A E 2022 J. Nucl. Mater. 572 154020
Google Scholar
[33] Fellman A, Sand A E, Byggmästar J, Nordlund K 2019 J. Phys. Condens. Matter. 31 405402
Google Scholar
[34] Qiu R Y, Chen Y C, Gao N, He X F, Dou Y K, Yang W, Hu W Y, Deng H Q 2023 Nucl. Mater. Energy 34 101394
Google Scholar
-
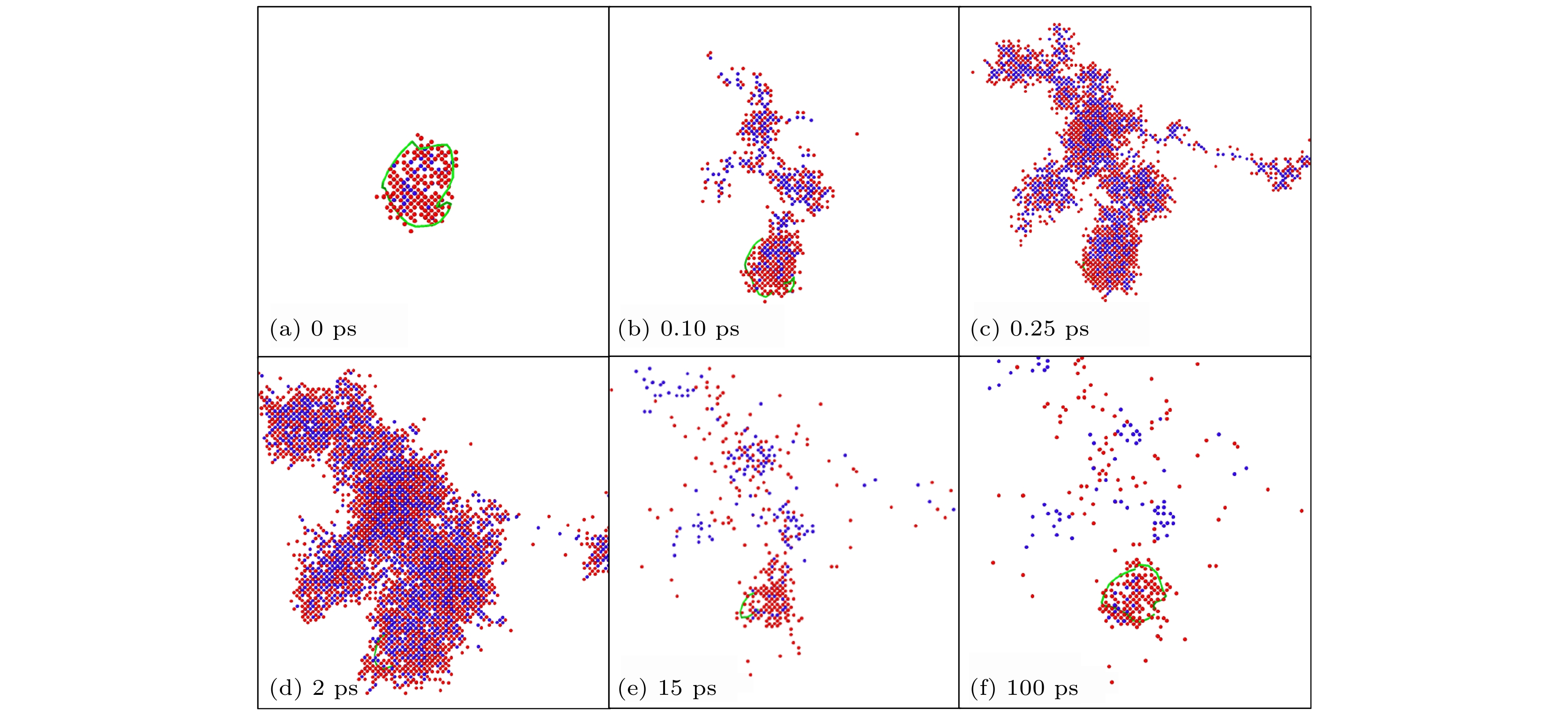
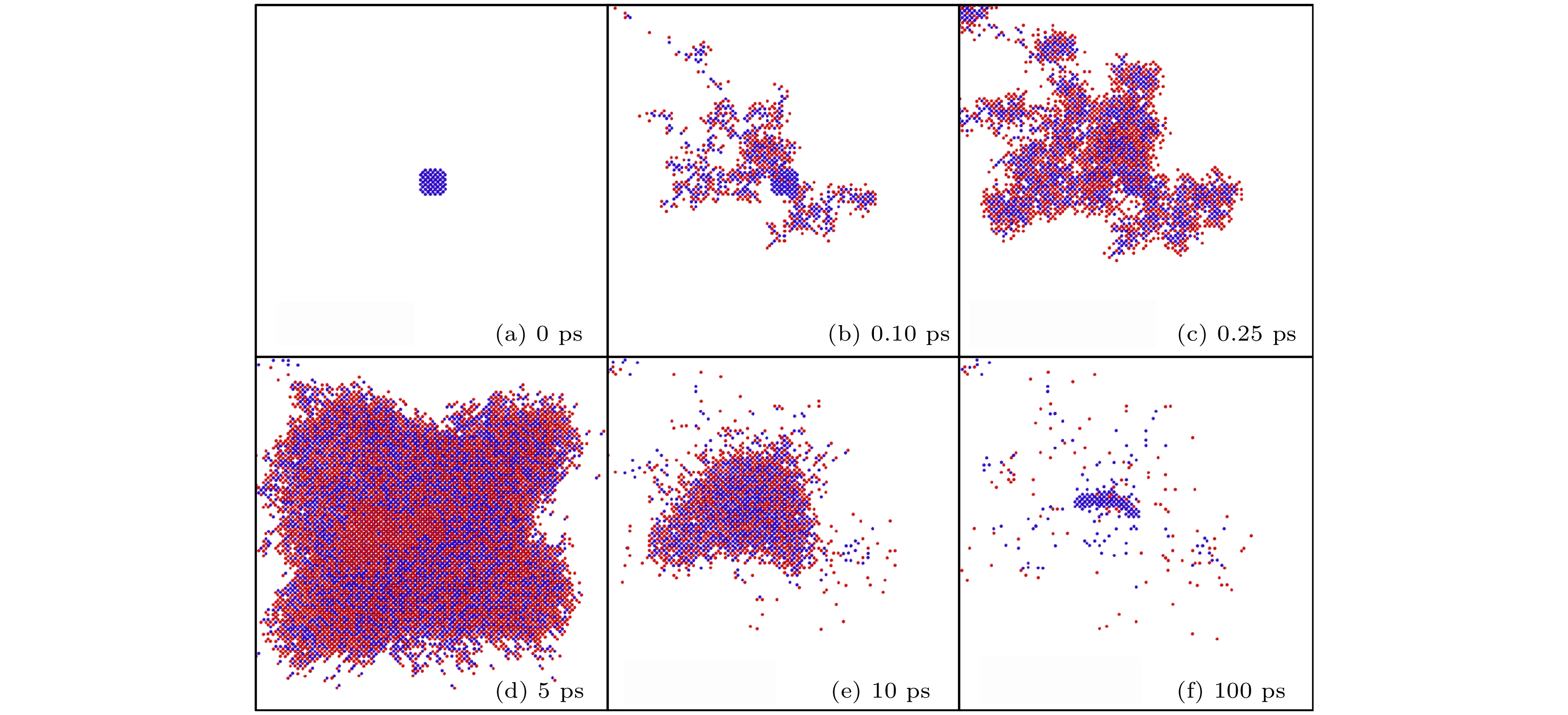
图 1 Ti-V-Ta多主元合金与空位团簇级联重叠模拟的缺陷演化过程(40 keV, $ {N}_{{\mathrm{V}}{\mathrm{A}}{\mathrm{C}}} = 253$), 红色和蓝色为间隙原子和空位
Fig. 1. Defect evolution process during the cascade overlap simulation with vacancy cluster (40 keV, $ {N}_{{\mathrm{V}}{\mathrm{A}}{\mathrm{C}}}= 253 $) in Ti-V-Ta multi-principal element alloy, red and blue are interstitials and vacancies.
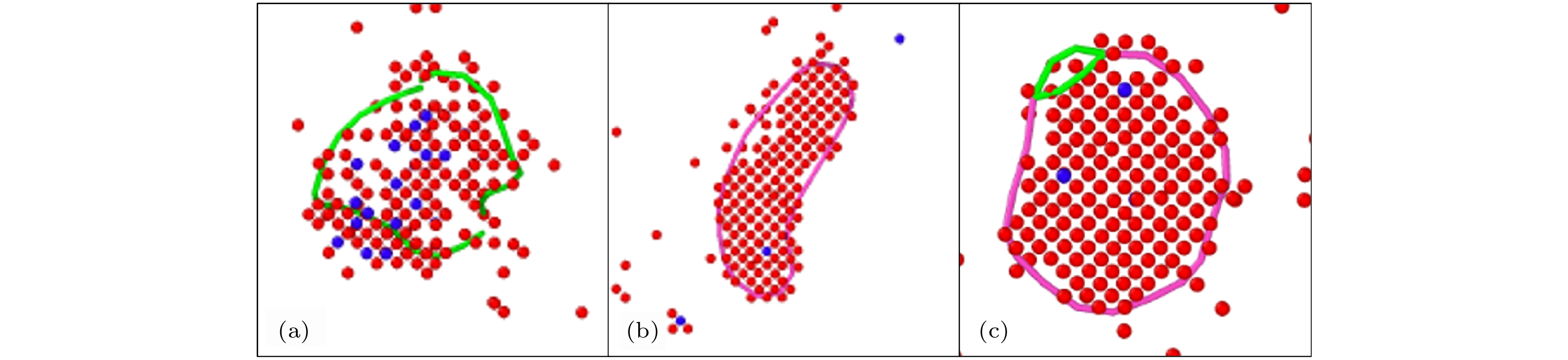
图 2 Ti-V-Ta多主元合金与空位团簇级联重叠形成的典型缺陷构型(40 keV, $ {N}_{{\mathrm{V}}{\mathrm{A}}{\mathrm{C}}} $= 253), 红色和蓝色为间隙原子和空位, 粉线和绿线为$ \left\langle {100} \right\rangle $和1/2$ \left\langle {111} \right\rangle $位错线
Fig. 2. Typical defect configuration during the cascade overlap simulation with vacancy cluster (40 keV, $ {N}_{{\mathrm{V}}{\mathrm{A}}{\mathrm{C}}} $= 253) in Ti-V-Ta multi-principal element alloy, red and blue are interstitials and vacancies, pink and green lines are $ \left\langle {100} \right\rangle $ and 1/2$ \left\langle {111} \right\rangle $ dislocation lines.
图 3 新产生的Frenkel缺陷对数目与预置空位团簇尺寸和PKA能量的关系
Fig. 3. Relationship between the number of newly generated Frenkel defect pairs and the size of preset vacancy cluster and PKA energy.
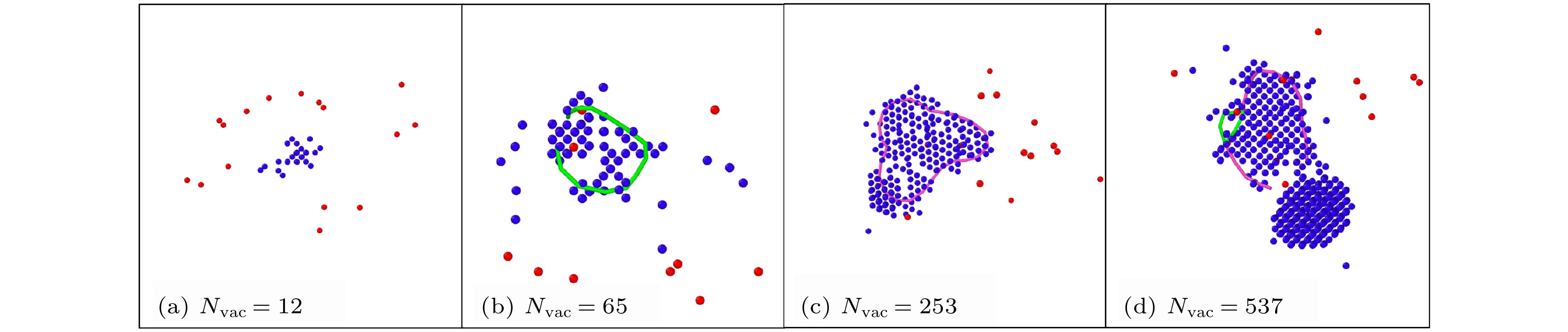
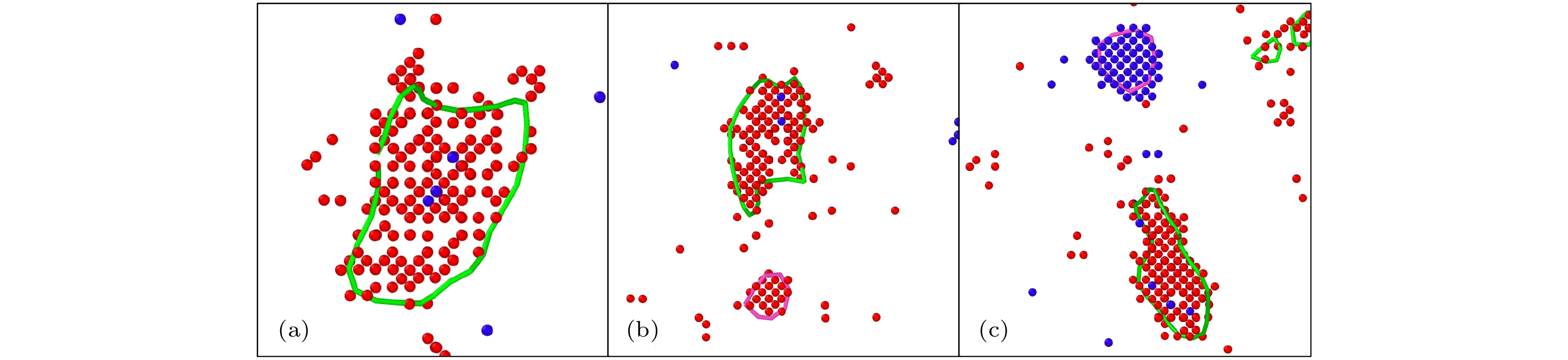
图 4 Ti-V-Ta多主元合金与不同尺寸空位团簇级联重叠形成的典型缺陷构型(5 keV), 红色和蓝色为间隙原子和空位, 粉线和绿线为$ \left\langle {100} \right\rangle $和1/2$ \left\langle {111} \right\rangle $位错线
Fig. 4. Typical defect configuration formed during the cascade overlap simulations with different size vacancy clusters in Ti-V-Ta multi-principal element alloy (5 keV), red and blue interstitials and vacancies, and pink and green lines are $ \left\langle {100} \right\rangle $ and 1/2$ \left\langle {111} \right\rangle $ dislocation lines.
图 5 Ti-V-Ta多主元合金中与空位团簇在不同条件下级联重叠后形成的缺陷构型数目
Fig. 5. Number of defect configurations formed in Ti-V-Ta multi-principal element alloy after cascade overlap simulations with vacancy clusters under different conditions.
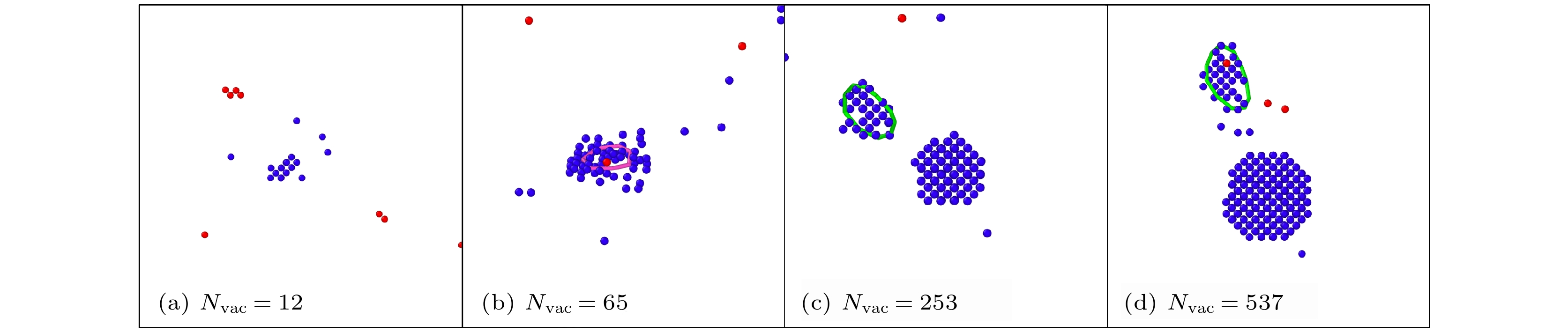
图 6 纯V与不同尺寸空位团簇级联重叠形成的典型缺陷构型(5 keV), 红色和蓝色为间隙原子和空位, 粉线和绿线为$ \left\langle {100} \right\rangle $和1/2$ \left\langle {111} \right\rangle $位错线
Fig. 6. Typical defect configuration formed during the cascade overlap simulations with different size vacancy clusters in pure V (5 keV), red and blue interstitials and vacancies, and pink and green lines are $ \left\langle {100} \right\rangle $ and 1/2$ \left\langle {111} \right\rangle $ dislocation lines.
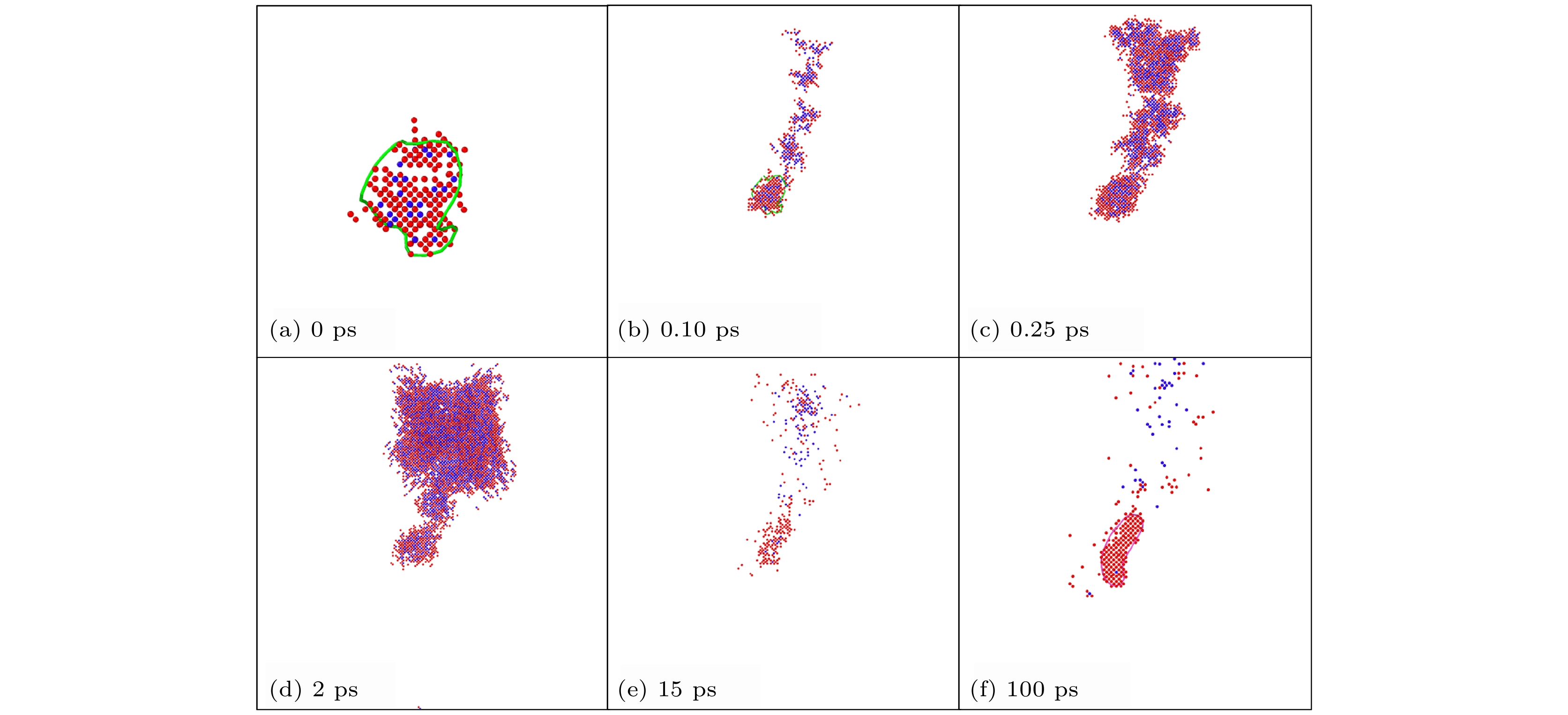
图 7 Ti-V-Ta多主元合金与间隙团簇级联重叠形成1/2$ \left\langle {111} \right\rangle $位错环的缺陷演化过程(40 keV, $ {N}_{{\mathrm{S}}{\mathrm{I}}{\mathrm{A}}} $= 183), 红色和蓝色为间隙原子和空位, 绿线为1/2$ \left\langle {111} \right\rangle $位错线
Fig. 7. Defect evolution process during the cascade overlap simulation with interstitial cluster (40 keV, $ {N}_{{\mathrm{S}}{\mathrm{I}}{\mathrm{A}}} $= 183) in Ti-V-Ta multi-principal element alloy, red and blue are interstitials and vacancies, green lines are 1/2$ \left\langle {111} \right\rangle $ dislocation lines.
图 8 Ti-V-Ta多主元合金与间隙团簇级联重叠形成$ \left\langle {100} \right\rangle $间隙位错环的缺陷演化过程(40 keV, $ {N}_{{\mathrm{S}}{\mathrm{I}}{\mathrm{A}}} $= 183), 红色和蓝色为间隙原子和空位, 粉线为$ \left\langle {100} \right\rangle $位错线
Fig. 8. Defect evolution process during the cascade overlap simulation with interstitial cluster (40 keV, $ {N}_{{\mathrm{S}}{\mathrm{I}}{\mathrm{A}}} $= 183) in Ti-V-Ta multi-principal element alloy, red and blue are interstitials and vacancies, pink lines are $ \left\langle {100} \right\rangle $ dislocation lines.
图 9 Ti-V-Ta多主元合金与间隙团簇级联重叠形成的典型缺陷构型(40 keV, $ {N}_{{\mathrm{S}}{\mathrm{I}}{\mathrm{A}}} $=183), 红色和蓝色为间隙原子和空位, 粉线和绿线为$ \left\langle {100} \right\rangle $和1/2$ \left\langle {111} \right\rangle $位错线
Fig. 9. Typical defect configuration during the cascade overlap simulation with interstitial cluster (40 keV, $ {N}_{{\mathrm{S}}{\mathrm{I}}{\mathrm{A}}} $=183) in Ti-V-Ta multi-principal element alloy, red and blue are interstitials and vacancies, pink and green lines are $ \left\langle {100} \right\rangle $ and 1/2$ \left\langle {111} \right\rangle $ dislocation lines.
图 10 纯V与间隙团簇级联重叠形成的典型缺陷构型(40 keV, $ {N}_{{\mathrm{S}}{\mathrm{I}}{\mathrm{A}}} $=183), 红色和蓝色为间隙原子和空位, 粉线和绿线为$ \left\langle {100} \right\rangle $和1/2$ \left\langle {111} \right\rangle $位错线
Fig. 10. Typical defect configuration during the cascade overlap simulation with interstitial cluster (40 keV, $ {N}_{{\mathrm{S}}{\mathrm{I}}{\mathrm{A}}} $=183) in pure V, red and blue are interstitials and vacancies, pink and green lines are $ \left\langle {100} \right\rangle $ and 1/2$ \left\langle {111} \right\rangle $ dislocation lines.
图 11 Ti-V-Ta多主元合金中辐照位错环形成的级联重叠机制示意图
Fig. 11. Schematic diagram of the cascade overlap mechanism of dislocation loops formation in Ti-V-Ta multi-principal element alloy.
-
[1] El-Genk M S, Tournier J M 2005 J. Nucl. Mater. 340 93
Google Scholar
[2] Busby J T, Leonard K J 2007 JOM 59 20
[3] Yeh J W, Chen S K, Lin S J, Gan J Y, Chin T S, Shun T T, Tsau C H, Chang S Y 2004 Adv. Eng. Mater. 6 299
Google Scholar
[4] Tsai M H, Yeh J W 2014 Mater. Res. Lett. 2 107
Google Scholar
[5] Ye Y F, Wang Q, Lu J, Liu C T, Yang Y 2016 Mater. Today 19 349
Google Scholar
[6] Miracle D B, Senkov O N 2017 Acta Mater. 122 448
Google Scholar
[7] George E P, Raabe D, Ritchie R O 2019 Nat. Rev. Mater. 4 515
Google Scholar
[8] Pickering E J, Carruthers A W, Barron P J, Middleburgh S C, Armstrong D E J, Gandy A S 2021 Entropy 23 98
Google Scholar
[9] Jia N N, Li Y K, Liu X, Zheng Y, Wang B P, Wang J S, Xue Y F, Jin K 2019 JOM. 71 3490
Google Scholar
[10] Hu B, Yao B, Wang J, Liu Y, Wang C J, Du Y, Yin H Q 2020 Intermetallics 118 106701
Google Scholar
[11] Yin X, Dou Y K, He X F, Jin K, Wang C L, Dong Y G, Wang H R, Zhong W H, Xue Y F, Yang W 2022 JOM 74 4326
Google Scholar
[12] Jia N N, Li Y, Huang H F, Chen S, Li D, Dou Y K, He X F, Yang W, Yin X, Jin K 2021 J. Nucl. Mater. 550 152937
Google Scholar
[13] Mei L, Zhang Q H, Dou Y K, Fu E G, Li L, Chen S, Dong Y G, Guo X, He X F, Yang W, Yin X, Jin K 2023 Scr. Mater. 223 115070
Google Scholar
[14] Dou Y K, Zhao Y P, He X F, Gao J, Cao J L, Yang W 2023 J. Nucl. Mater. 573 154096
Google Scholar
[15] Zhao Y P, Dou Y K, He X F, Deng H Q, Wang L F, Yang W 2023 Comput. Mater. Sci. 218 111943
Google Scholar
[16] Zhao Y P, Dou Y K, He X F, Cao H, Wang L F, Deng H Q, Yang W 2024 Chin. Phys. B 33 036104
Google Scholar
[17] Peng Q, Meng F J, Yang Y Z, Lu C Y, Deng H Q, Wang L M, De S, Gao F 2018 Nat. Commun. 9 4880
Google Scholar
[18] Granberg F, Byggmästar J, Sand A E, Nordlund K 2017 Europhys. Lett. 119 56003
Google Scholar
[19] Byggmästar J, Granberg F, Sand A E, Pirttikoski A, Alexander R, Marinica M C, Nordlund K 2019 J. Phys. Condens. Matter. 31 245402
Google Scholar
[20] Wang X Y, Gao N, Wang Y N, Liu H L, Shu G G, Li C L, Xu B, Liu W 2019 J. Nucl. Mater. 519 322
Google Scholar
[21] Marinica M C, Willaime F, Crocombette J P 2012 Phys. Rev. Lett. 108 025501
Google Scholar
[22] Gao J, Gaganidze E, Kaiser B, Aktaa J 2021 J. Nucl. Mater. 557 153212
Google Scholar
[23] Esfandiarpour A, Byggmästar J, Balbuena J P, Caturla M J, Nordlund K, Granberg F 2022 Materialia. 21 101344
Google Scholar
[24] Arakawa K, Hatanaka M, Kuramoto E, Ono K, Mori H 2006 Phys. Rev. Lett. 96 125506
Google Scholar
[25] Chen J, Gao N, Jung P, Sauvage T 2013 J. Nucl. Mater. 441 216
Google Scholar
[26] Gao N, Chen J, Kurtz R J, Wang Z G, Zhang R F, Gao F 2017 J. Phys. Condens. Matter. 29 455301
Google Scholar
[27] Xu H, Stoller R E, Osetsky Y N, Terentyev D 2013 Phys. Rev. Lett. 110 265503
Google Scholar
[28] Wang X Y, Gao N, Wang Y N, Wu X Y, Shu G G, Li C L, Li Q L, Xu B, Liu W 2019 Scr. Mater. 162 204
Google Scholar
[29] Plimpton S 1995 J. Comput. Phys. 117 1
Google Scholar
[30] Qiu R Y, Chen Y C, Liao X C, He X F, Yang W, Hu W Y, Deng H Q 2021 J. Nucl. Mater. 557 153231
Google Scholar
[31] Stukowski A 2010 Model. Simul. Mater. Sc. 18 015012
Google Scholar
[32] Fellman A, Sand A E 2022 J. Nucl. Mater. 572 154020
Google Scholar
[33] Fellman A, Sand A E, Byggmästar J, Nordlund K 2019 J. Phys. Condens. Matter. 31 405402
Google Scholar
[34] Qiu R Y, Chen Y C, Gao N, He X F, Dou Y K, Yang W, Hu W Y, Deng H Q 2023 Nucl. Mater. Energy 34 101394
Google Scholar
-
[1] 陈贝, 王小云, 刘涛, 高明, 文大东, 邓永和, 彭平. Pd-Si非晶合金动力学非均匀性的对称与有序. , 2024, 73(24): . doi: 10.7498/aps.73.20241051 [2] 余欣秀, 李多生, 叶寅, 朗文昌, 刘俊红, 陈劲松, 于爽爽. 硬质合金表面镍过渡层对碳原子沉积与石墨烯生长影响的分子动力学模拟. , 2024, 73(23): 238701. doi: 10.7498/aps.73.20241170 [3] 张宇航, 李孝宝, 詹春晓, 王美芹, 浦玉学. 单层MoSSe力学性质的分子动力学模拟研究. , 2023, 72(4): 046201. doi: 10.7498/aps.72.20221815 [4] 闻鹏, 陶钢. 温度对CoCrFeMnNi高熵合金冲击响应和塑性变形机制影响的分子动力学研究. , 2023, 0(0): 0-0. doi: 10.7498/aps.72.20221621 [5] 丁业章, 叶寅, 李多生, 徐锋, 朗文昌, 刘俊红, 温鑫. WC-Co硬质合金表面石墨烯沉积生长分子动力学仿真研究. , 2023, 72(6): 068703. doi: 10.7498/aps.72.20221332 [6] 闻鹏, 陶钢. 温度对CoCrFeMnNi高熵合金冲击响应和塑性变形机制影响的分子动力学研究. , 2022, 71(24): 246101. doi: 10.7498/aps.71.20221621 [7] 王瑾, 贺新福, 曹晗, 贾丽霞, 豆艳坤, 杨文. 不同温度下bcc-Fe中螺位错滑移及其与½[ ${{11}}\bar {{1}}$ ]位错环相互作用行为. , 2021, 70(6): 068701. doi: 10.7498/aps.70.20201659[8] 杨权, 马立, 耿松超, 林旖旎, 陈涛, 孙立宁. 多壁碳纳米管与金属表面间接触行为的分子动力学模拟. , 2021, 70(10): 106101. doi: 10.7498/aps.70.20202194 [9] 周明锦, 侯氢, 潘荣剑, 吴璐, 付宝勤. 锆铌合金的特殊准随机结构模型的分子动力学研究. , 2021, 70(3): 033103. doi: 10.7498/aps.70.20201407 [10] 朱琪, 王升涛, 赵福祺, 潘昊. 层错四面体对单晶铜层裂行为影响的分子动力学研究. , 2020, 69(3): 036201. doi: 10.7498/aps.69.20191425 [11] 袁伟, 彭海波, 杜鑫, 律鹏, 沈扬皓, 赵彦, 陈亮, 王铁山. 分子动力学模拟钠硼硅酸盐玻璃电子辐照诱导的结构演化效应. , 2017, 66(10): 106102. doi: 10.7498/aps.66.106102 [12] 邓永和, 文大东, 彭超, 韦彦丁, 赵瑞, 彭平. 二十面体团簇的遗传:一个与快凝Cu56Zr44合金玻璃形成能力有关的动力学参数. , 2016, 65(6): 066401. doi: 10.7498/aps.65.066401 [13] 王成龙, 王庆宇, 张跃, 李忠宇, 洪兵, 苏折, 董良. SiC/C界面辐照性能的分子动力学研究. , 2014, 63(15): 153402. doi: 10.7498/aps.63.153402 [14] 吴文平, 郭雅芳, 汪越胜, 徐爽. 镍基单晶高温合金界面位错网在剪切载荷作用下的演化. , 2011, 60(5): 056802. doi: 10.7498/aps.60.056802 [15] 陈敏. 分子动力学方法研究金属Ti中He小团簇的迁移. , 2011, 60(12): 126602. doi: 10.7498/aps.60.126602 [16] 王伟, 张凯旺, 孟利军, 李中秋, 左学云, 钟建新. 多壁碳纳米管外壁高温蒸发的分子动力学模拟. , 2010, 59(4): 2672-2678. doi: 10.7498/aps.59.2672 [17] 朱弢, 王崇愚, 干勇. 镍基单晶高温合金相界面错配位错网络的演化. , 2009, 58(13): 156-S160. doi: 10.7498/aps.58.156 [18] 周耐根, 周 浪. 采用纳米晶柱阵列衬底抑制失配位错形成的分子动力学模拟研究. , 2008, 57(5): 3064-3070. doi: 10.7498/aps.57.3064 [19] 张 超, 王永亮, 颜 超, 张庆瑜. 替位杂质对低能Pt原子与Pt(111)表面相互作用影响的分子动力学模拟. , 2006, 55(6): 2882-2891. doi: 10.7498/aps.55.2882 [20] 周耐根, 周 浪. 外延生长薄膜中失配位错形成条件的分子动力学模拟研究. , 2005, 54(7): 3278-3283. doi: 10.7498/aps.54.3278
下载:
计量
- 文章访问数: 245
- PDF下载量: 7
- 被引次数: 0
