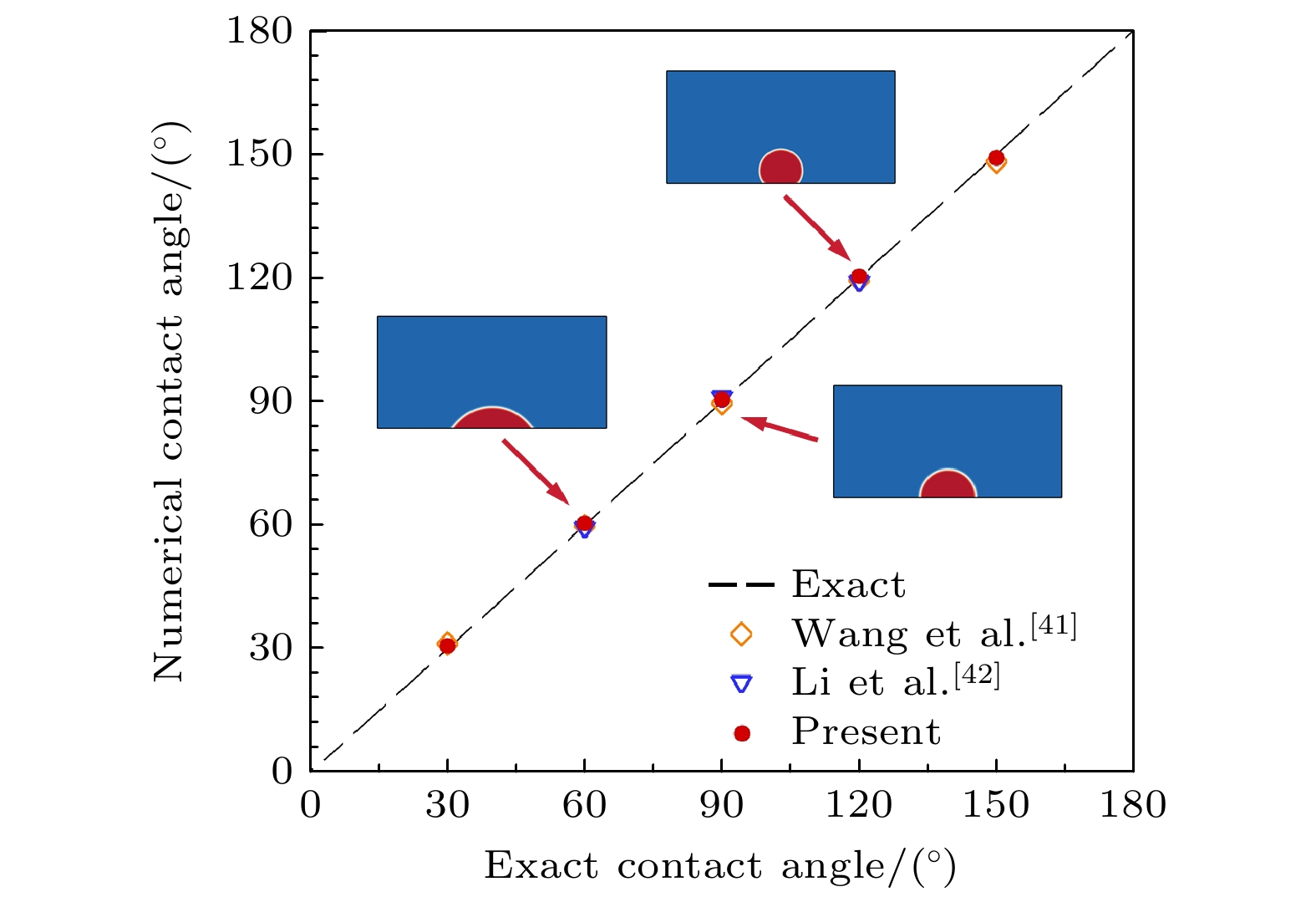
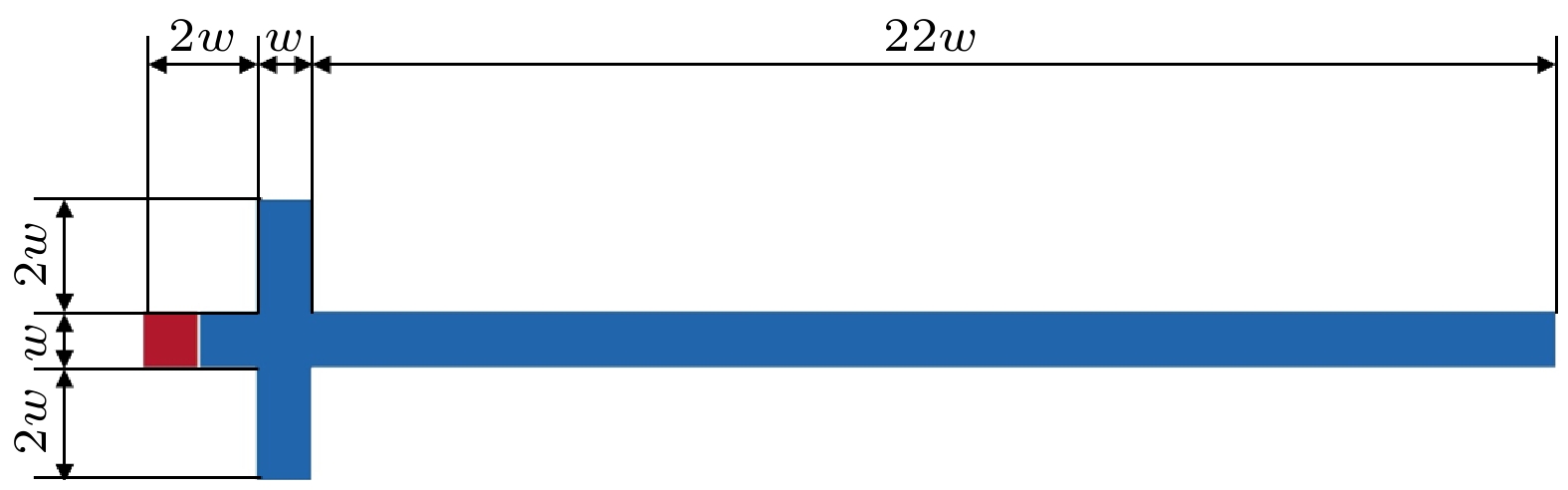
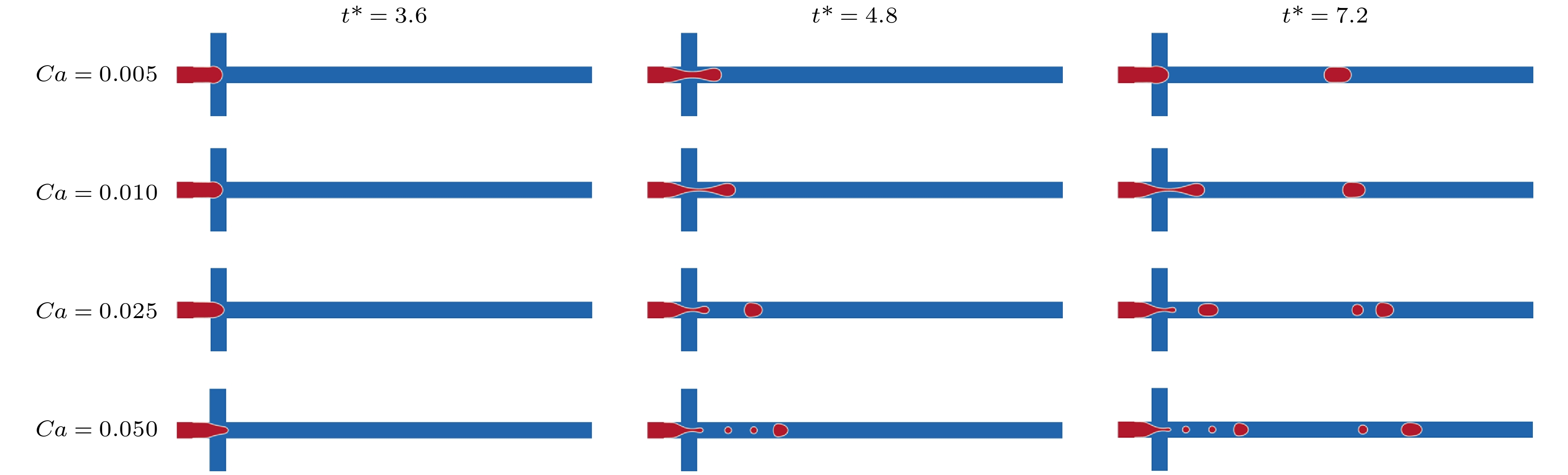
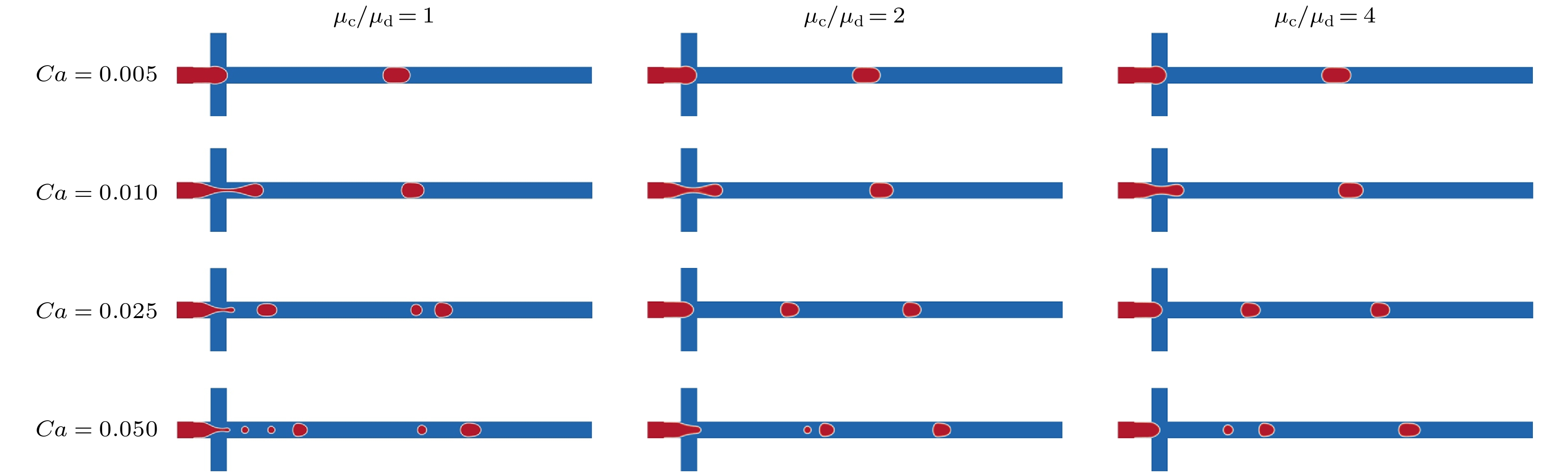
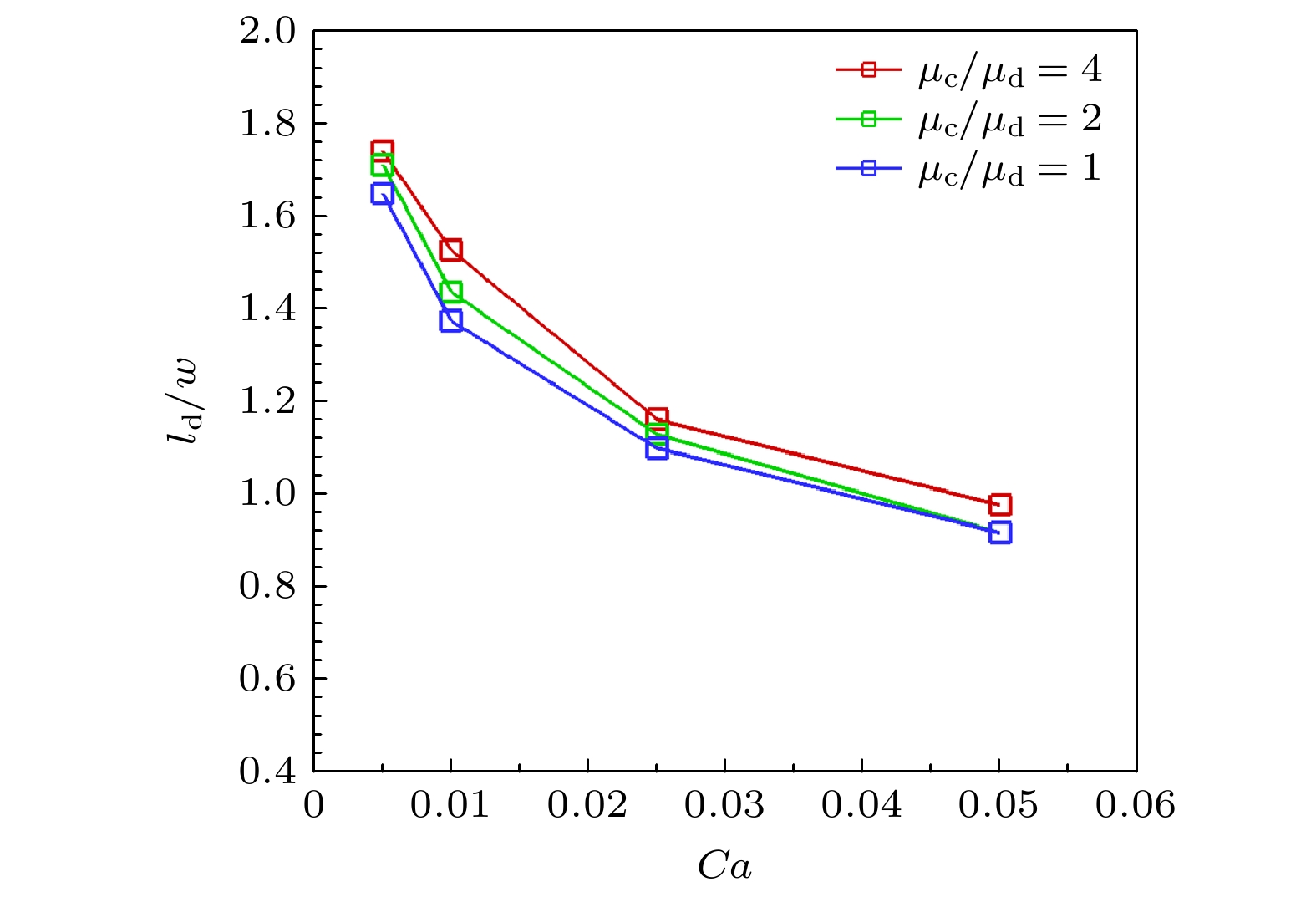
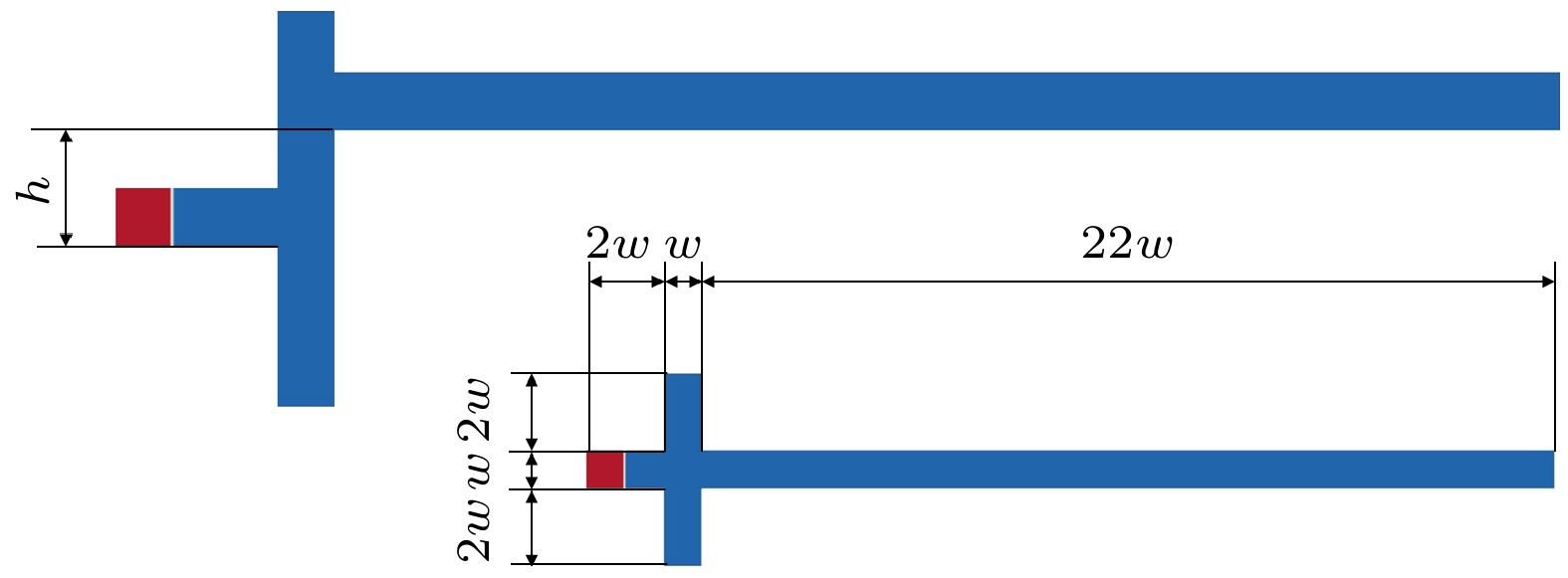
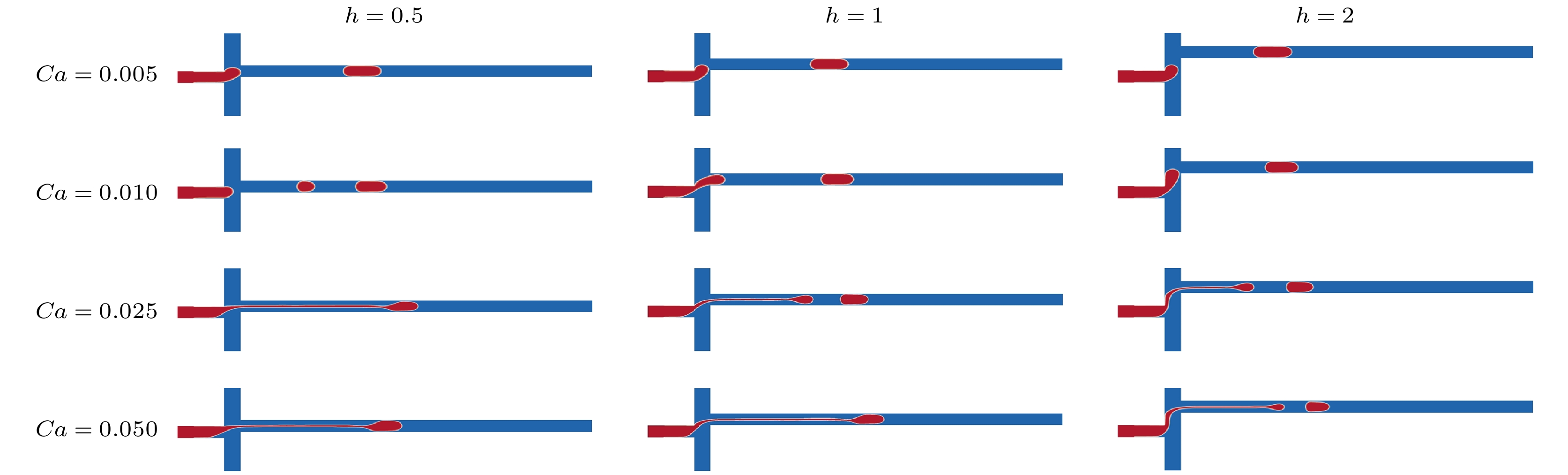
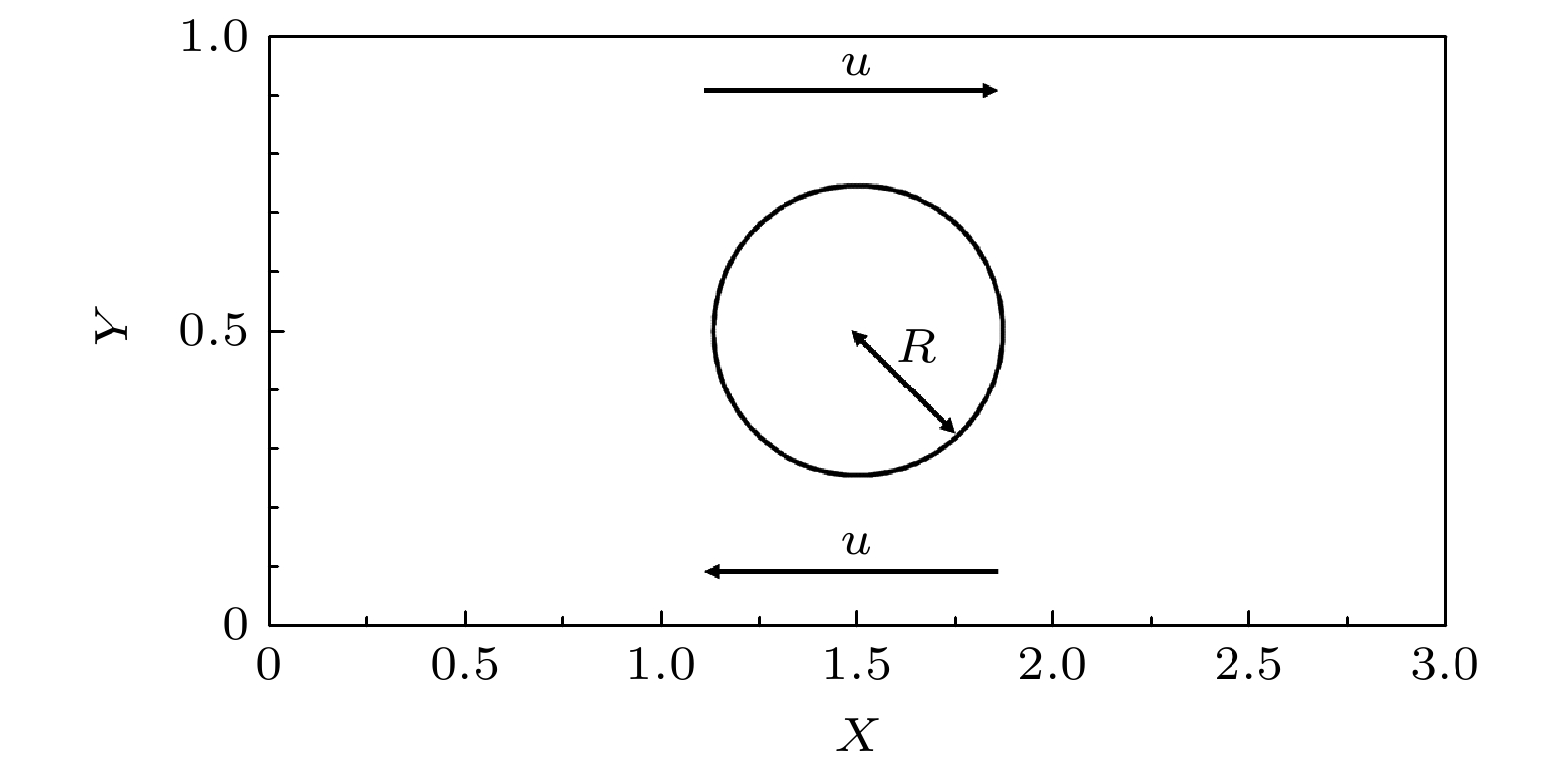
-
Droplet microfluidics technology presents significant potential for applications in chemical analysis, biological detection, and material preparation. Passive droplet generation methods can rapidly achieve droplet formation by relying on the geometric characteristics of microchannels and shear flow. As a typical structure, the influence of fluid parameters and symmetry differences in cross microchannels on the droplet generation process has not been fully studied. Therefore, this paper uses the lattice Boltzmann method to conduct numerical simulation studies on droplet generation in symmetric and asymmetric cross microchannels, systematically analyzing the action mechanisms of capillary number, viscosity ratio, and microchannel symmetry. First, this study verifies the computational reliability of the numerical model through two classic cases, i.e., the droplet deformation under planar shear flow and stationary droplets on ideal solid surfaces. Then, this work focuses on the three flow stages in symmetric cross microchannels, i.e., the interface immersion stage, the shear-induced breakup stage, and the droplet migration and coalescence stage, analyzing the collaborative mechanism of capillary number and viscosity ratio. In the symmetric cross microchannel structure, the capillary number is the main factor determining the droplet size in the cross microchannel. With the increase of the capillary number, the surface tension gradually weakens, causing the liquid bridge at the droplet neck to break more easily and generate droplets. In contrast, the effect of the viscosity ratio on the droplet size is relatively small, but it can suppress the generation of sub-droplets and improve the uniformity of droplets by adjusting the viscous resistance of the continuous phase. On this basis, the study further quantifies the impact of microchannel symmetry on the droplet generation process in cross microchannels. In the asymmetric cross microchannel structure, the microchannel deviation breaks the flow symmetry and weakens the cooperative shearing effect of the oil-phase fluid on the immersion structure of the water-phase fluid. When the microchannel deviates within the centerline range of the water-phase microchannel, the droplet size increases significantly with the increase of the microchannel deviation. This is mainly because the oil-phase fluid on the side far from the deviation first squeezes the immersion structure of the water-phase fluid, and then the oil-phase fluid near the deviation side performs secondary squeezing on the immersion structure, resulting in the continuous elongation of the neck liquid bridge of the immersion structure and the offset of the shear position along the microchannel deviation direction. When the microchannel deviation exceeds the centerline position of the water-phase microchannel, the interface fracture of the water-phase immersion structure mainly relies on the double squeeze effect of the oil-phase fluid and the surface tension of water-phase fluid, and the droplet size tends to be stable. The relevant research results provide a theoretical basis for microchannel design and fluid parameter regulation in droplet microfluidics and further promote the application and development of droplet microfluidic technology.
-
Keywords:
- Droplet Generation /
- Multiphase Flow /
- Lattice Boltzmann Method /
- Diffuse Interface Method /
- Microfluidic Technology
-
[1] Zhu P, Wang L 2017 Lab Chip 17 34
[2] Cybulski O, Garstecki P, Grzybowski B A 2019 Nat. Phys. 15 706
[3] Chen P-C, Wu M-H, Wang Y-N 2014 Microfluidic. Nanofluidic. 17 275
[4] Li X, Li D, Liu X, Chang H 2016 Sensor. Actuat. B-Chem. 229 466
[5] Theberge A B, Courtois F, Schaerli Y, Fischlechner M, Abell C, Hollfelder F, Huck W T S 2010 Angew. Chem. Int. Edit. 49 5846
[6] Teo A J T, Tan S H, Nguyen N-T 2020 Anal. Chem. 92 1147
[7] Zhang Q, Li H, Zhu C, Fu T, Ma Y, Li H Z 2018 Colloid. Surface. A 537 572
[8] Jin S, Wei X, Liu Z, Ren J, Jiang Z, Abell C, Yu Z 2019 Sensor. Actuat. B-Chem. 291 1
[9] Zhang H, Palit P, Liu Y, Vaziri S, Sun Y 2020 ACS Appl. Mater. Interfaces 12 26936
[10] Thorsen T, Roberts R W, Arnold F H, Quake S R 2001 Phys. Rev. Lett. 86 4163
[11] Zhang Y Y, Xia H M, Wu J W, Zhang J, Wang Z P 2019 Appl. Phys. Lett. 114 073701
[12] Zhang Y Y, Xia H M 2022 Sensor. Actuat. B-Chem. 368 132183
[13] Dreyfus R, Tabeling P, Willaime H 2003 Phys. Rev. Lett. 90 144505
[14] Tan Y-C, Cristini V, Lee A P 2006 Sensor. Actuat. B-Chem. 114 350
[15] Chae S-K, Lee C-H, Lee S H, Kim T-S, Kang J Y 2009 Lab Chip 9 1957
[16] Nisisako T, Hatsuzawa T 2010 Microfluidic. Nanofluidic. 9 427
[17] Liu H, Zhang Y 2011 Commun. Comput. Phys. 9 1235
[18] Rostami B, Morini G L 2019 Exp. Therm. Fluid Sci. 103 191
[19] Yu W, Liu X, Zhao Y, Chen Y 2019 Chem. Eng. Sci. 203 259
[20] Nozaki Y, Yoon D H, Furuya M, Fujita H, Sekiguchi T, Shoji S 2021 Sensor. Actuat. A-Phys. 331 112917
[21] Liu Z, Ma Y, Wang X, Pang Y, Ren Y, Li D 2022 Exp. Therm. Fluid Sci. 139 110739
[22] Umbanhowar P B, Prasad V, Weitz D A 2000 Langmuir 16 347
[23] Utada A S, Lorenceau E, Link D R, Kaplan P D, Stone H A, Weitz D A 2005 Science 308 537
[24] Garstecki P, Fuerstman M J, Whitesides G M 2005 Phys. Rev. Lett. 94 234502
[25] Deng C, Wang H, Huang W, Cheng S 2017 Colloid. Surface. A 533 1
[26] Liu H-T, Liu M-B, Chang J-Z, Su T-X 2013 Acta Phys. Sin.-Ch Ed 62 064705
[27] Liang H, Chai Z-H, Shi B-C 2016 Acta Phys. Sin.-Ch Ed 65 204701
[28] Zhang X-L, Huang J-J 2023 Acta Phys. Sin.-Ch Ed 72 024701
[29] Wang W, Liu Z, Jin Y, Cheng Y 2011 Chem. Eng. J. 173 828
[30] Ngo I-L, Dang T-D, Byon C, Joo S W 2015 Biomicrofluidics 9 024107
[31] Liu H, Zhang Y 2011 Phys. Fluids 23 082101
[32] Boruah M P, Sarker A, Randive P R, Pati S, Sahu K C 2021 Phys. Fluids 33 122101
[33] Wang H, Yuan X, Liang H, Chai Z, Shi B 2019 Capillarity 2 32
[34] Niu X-D, Li Y, Ma Y-R, Chen M-F, Li X, Li Q-Z 2018 Phys. Fluids 30 013302
[35] Li X, Dong Z-Q, Li Y, Wang L-P, Niu X-D, Yamaguchi H, Li D-C, Yu P 2022 Int. J. Multiphas. Flow 149 103982
[36] Li X, Yu P, Niu X-D, Li D-C, Yamaguchi H 2021 Appl. Math. and Comput. 393 125769
[37] Qian Y H, Humières D D, Lallemand P 1992 Europhysics Letters 17 479
[38] van der Graaf S, Nisisako T, Schroën C G P H, van der Sman R G M, Boom R M 2006 Langmuir 22 4144
[39] Ding H, Spelt P D M, Shu C 2007 J. Comput. Phys. 226 2078
[40] Liang H, Liu H, Chai Z, Shi B 2019 Phys. Rev. E 99 063306
[41] Shi Y, Tang G H, Xia H H 2014 Comput. Fluids 90 155
[42] Yue P, Feng J J, Liu C, Shen J 2004 J. Fluid Mech. 515 293
[43] Roths T, Friedrich C, Marth M, Honerkamp J 2002 Rheol. Acta 41 211
[44] Wang Y, Shu C, Huang H B, Teo C J 2015 J. Comput. Phys. 280 404
[45] Li X, Dong Z-Q, Wang L-P, Niu X-D, Yamaguchi H, Li D-C, Yu P 2023 Appl. Math. Model. 117 219
Metrics
- Abstract views: 93
- PDF Downloads: 11
- Cited By: 0
下载:
