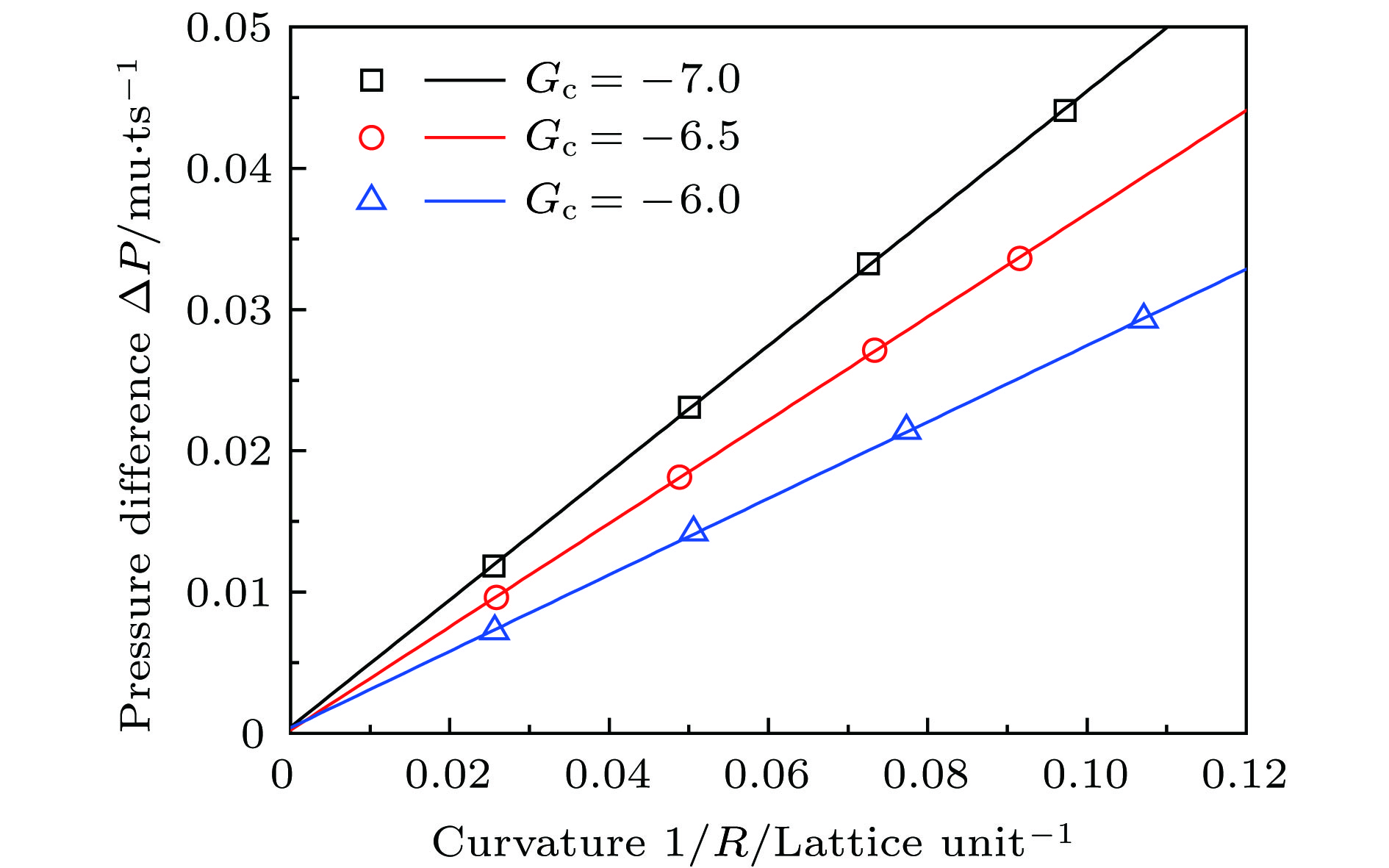
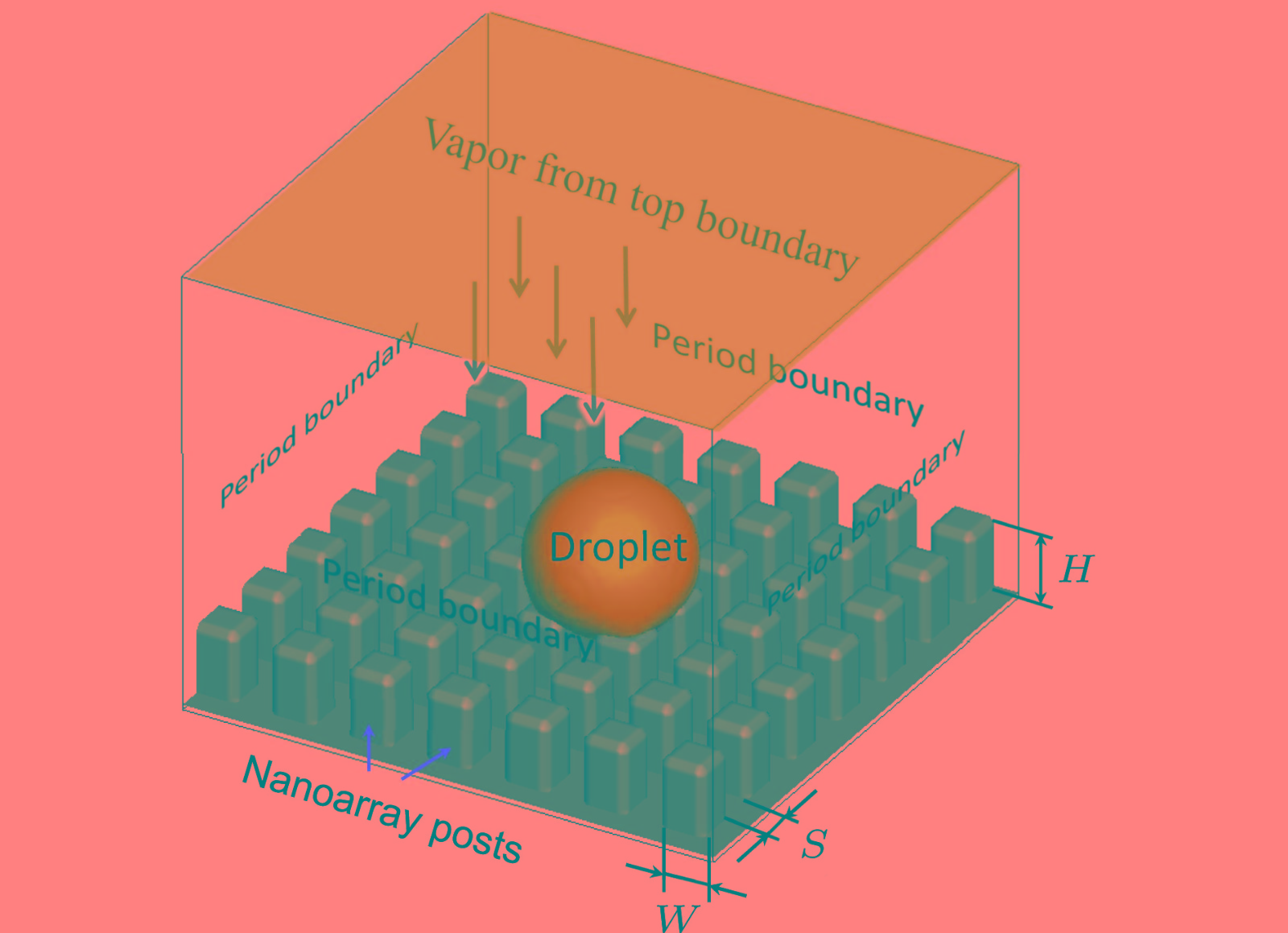
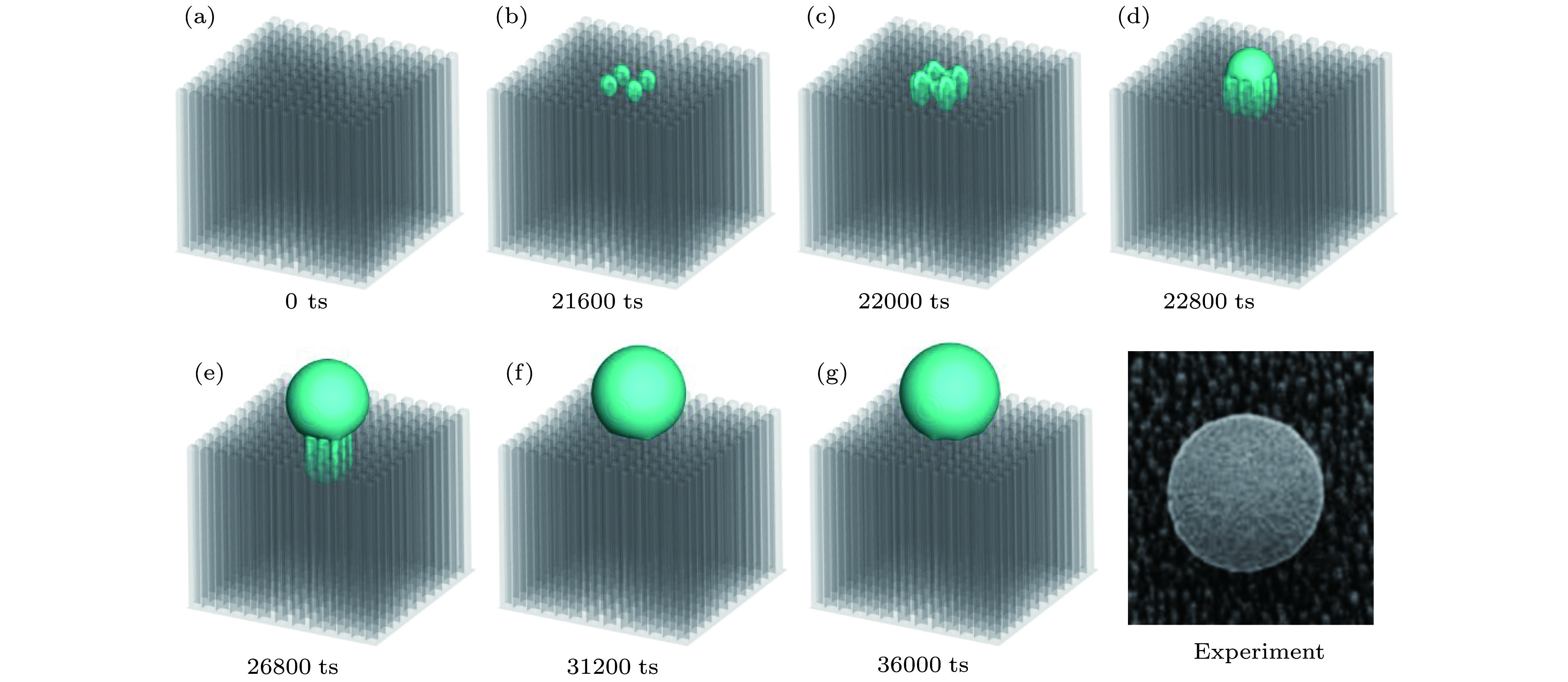
-
Superhydrophobic surfaces resulting from nanoarrays have good performance in anti-condensation. However, the study of droplet nucleation during water vapor condensation is a challenge because of the limitation of observation on a nanoscale, and therefore the fundamental understanding of the influence of geometrical parameters of nanoarrays on the condensation behavior is still less clear. In this work a three-dimensional (3D) multiphase lattice Boltzmann (LB) model is employed to simulate the phenomenon of droplet condensation on the superhydrophobic nanostructured surface. The model validation is carried out through the comparison of the simulations with the results from the Laplace's law and the intrinsic contact angle theory. The LB simulations accord well with the results from Laplace's law. The relative deviation between the simulated intrinsic contact angle and the theoretical value is less than 0.14%, demonstrating the validity of the LB model. Then, the 3D LB model is used to simulate the different preferential nucleation positions and final wetting states of condensate droplets by changing the geometrical parameters, including interpost space, post height and post width, and local wettability of the nanoarrays on superhydrophobic surfaces. It is found that for the nanostructured surfaces patterned with tall posts, the droplets nucleate in the upside interpost space and at the bottom of nanostructures simultaneously. By designing wider and thinner interpost spaces at the downside and upside of the tall nanostructures, respectively, the phenomenon of droplet nucleation at the bottom can be avoided. The simulation results show that the condensate droplets nucleated in the upside interpost space of tall nanostructures migrate upwards during growth, producing a Wenzel-to-Cassie wetting state transition. On the other hand, the condensate droplets nucleated at the bottom of nanostructured surface patterned with short posts produce the Wenzel state. However, by setting non-uniform hydrophilic and hydrophobic regions on the top of the short nanostructures, the condensate droplets are found to nucleate on the hydrophilic top and generate a Cassie state. The simulated final wetting states of condensate droplets on the nanostructures, having various geometrical parameters, compare reasonably well with the experimental observations reported in the literature. It is demonstrated that the migration of condensate droplets is correlated with the evolution of the statistical average force. If the direction of the statistical average force acting on the droplet is upward, the condensate droplets nucleated in the upside interpost space move upward during growth. The 3D LB simulations provide an insight into the physical mechanism of droplet nucleation, growth and wetting state transitions on superhydrophobic nanostructured surfaces.
-
Keywords:
- nanostructure /
- condensation /
- superhydrophobic /
- lattice Boltzmann method
[1] Zhang X, Shi F, Niu J, Jiang Y G, Wang Z Q 2008 J. Mater. Chem. 18 621
Google Scholar
[2] Park K C, Choi H J, Chang C H, Cohen R E, Mckinley G H, Barbastathis G 2012 ACS Nano 6 3789
Google Scholar
[3] Guo P, Zheng Y M, Wen M X, Song C, Lin Y C, Jiang L 2012 Adv. Mater. 24 2642
Google Scholar
[4] Zhang Y F, Yu X Q, Wu H, Wu J 2012 Appl. Surf. Sci. 258 8253
Google Scholar
[5] 柯清平, 李广录, 郝天歌, 何涛, 李雪梅 2010 化学进展 22 284
Ke Q P, Li G L, Hao T G, He T, Li X M 2010 Prog. Chem. 22 284
[6] 郗金明 2008 博士学位论文 (北京: 中国科学院研究生院)
Xi J M 2008 Ph. D. Dissertation (Beijing: Graduate University of Chinese Academy of Sciences) (in Chinese)
[7] 刘俊吉, 周亚平, 李松林 2009 物理化学(下卷) (北京: 高等教育出版社) 第487页
Liu J J, Zhou Y P, Li S L 2009 Physical Chemistry (Vol. 2) (Beijing: Higher Education Press) p487 (in Chinese)
[8] He M, Wang J J, Li H L, Song Y L 2011 Soft Matter 7 3993
Google Scholar
[9] Enright R, Miljkovic N, Dou N, Nam Y, Wang E N 2013 J. Heat Transfer 135 091304
Google Scholar
[10] Zhang S N, Huang J Y, Tang Y X, Li S H, Ge M Z, Chen Z, Zhang K Q, Lai Y K 2017 Small 13 1600687
Google Scholar
[11] Aili A, Ge Q Y, Zhang T J 2017 J. Heat Transfer 139 112401
Google Scholar
[12] Wenzel R N 1936 J. Ind. Eng. Chem. 28 988
Google Scholar
[13] Cassie A B D, Baxter S 1944 Trans. Faraday Soc. 40 546
Google Scholar
[14] Narhe R D, Beysens D A 2004 Phys. Rev. Lett. 93 076103
Google Scholar
[15] Narhe R D, Beysens D A 2006 Europhys. Lett. 75 98
Google Scholar
[16] Rykaczewski K 2012 Langmuir 28 7720
Google Scholar
[17] Lau K K S, Bico J, Teo K B K, Chhowalla M, Amaratunga G A J, Milne W I, McKinley G H, Gleason K K 2003 Nano Lett. 3 1701
Google Scholar
[18] 郭照立, 郑楚光 2009 格子Boltzmann方法的原理和应用 (第一版) (北京: 科学出版社) 第9, 10页
Guo Z L, Zheng C G 2009 Theory and Applications of Lattice Boltzmann Method (Beijing: Science Press) pp9, 10 (in Chinese)
[19] 张博 2016 博士学位论文 (北京: 北京化工大学)
Zhang B 2016 Ph. D. Dissertation (Beijing: Beijing University of Chemical Technology) (in Chinese)
[20] Kusumaatmaja H, Leopoldes J, Dupuis A, Yeomans J M 2006 Europhys. Lett. 73 740
Google Scholar
[21] Kusumaatmaja H, Yeomans J M 2007 Langmuir 23 6019
Google Scholar
[22] Cui J, Li W Z, Lam W H 2011 Comput. Math. Appl. 61 3678
Google Scholar
[23] Liu X L, Cheng P, Quan X J 2014 Int. J. Heat Mass Transfer 73 195
Google Scholar
[24] Zhang Q Y, Sun D K, Zhang Y F, Zhu M F 2014 Langmuir 30 12559
Google Scholar
[25] Zhang Q Y, Sun D K, Zhang Y F, Zhu M F 2016 Chin. Phys. B 25 066401
Google Scholar
[26] Shan X W, Chen H D 1993 Phys. Rev. E 47 1815
Google Scholar
[27] 何雅玲, 王勇, 李庆 2009 格子Boltzmann方法的理论及应用 (第一版) (北京: 科学出版社) 第49−52页
He Y L, Wang Y, Li Q 2009 Lattice Boltzmann Method: Theory and Applications (Beijing: Science Press) pp49−52 (in Chinese)
[28] 吴伟 2012 硕士学位论文(南京: 东南大学)
Wu W 2012 M. S. Thesis (Nanjing: Southeast University) (in Chinese)
[29] Sukop M C, Jr Thorne D T 2005 Lattice Boltzmann Modeling-An Introduction for Geoscientists and Engineers (2nd Ed.) (New York: Springer) p89
[30] Chen C H, Cai Q, Tsai C, Chen C L, Xiong G, Yu Y, Ren Z 2007 Appl. Phys. Lett. 90 173108
Google Scholar
-
图 2 模拟的液滴内外压力差与液滴曲率的关系(符号, 模拟值; 直线, 线性拟合)
Figure 2. Simulated pressure difference between inside and outside of a spherical droplet as a function of droplet curvature (symbols, simulated data; lines, linear fitting).
图 3 取Gc = −6.5时模拟的液滴本征接触角随流-固作用系数的变化
Figure 3. Simulated intrinsic contact angle as a function of the fluid-solid interaction strength at Gc = −6.5.
图 4 液滴冷凝的三维模拟区域示意图
Figure 4. Schematic of the three-dimensional domain for the simulation of droplet condensation.
图 5 模拟的液滴在纳米阵列上部侧面和底部同时形核、生长及合并演化过程
Figure 5. Simulated evolution of droplet nucleation, growth, and coalescence for the droplets that nucleate simultaneously in the upside space and the bottom corners between the posts of nanoarrays.
图 9 模拟的液滴在具有不均匀润湿性的纳米阵列顶端形核、生长及合并演化过程
Figure 9. Simulated evolution of droplet nucleation, growth, and coalescence on the nanoarrays non-uniformly patterned with hydrophilic and hydrophobic regions on the top of nanoarrays.
表 1 取Gc = −6.5时液滴本征接触角设定值与模拟值对比
Table 1. Comparison of intrinsic contact angles between the targeted data and the simulated results (Gc = −6.5).
Gads $\theta_{\rm c0} $ $\theta_{\rm c1} $ $\operatorname{Re} {\rm{lative \;error = }}\dfrac{{{\theta _{{\rm{c}}1}} - {\theta _{{\rm{c0}}}}}}{{{\theta _{{\rm{c}}0}}}}$/% −5.68 0° 0° 0 −3.33 90° 90.13° 0.14 −0.39 180° 180° 0 
DownLoad: CSV
-
[1] Zhang X, Shi F, Niu J, Jiang Y G, Wang Z Q 2008 J. Mater. Chem. 18 621
Google Scholar
[2] Park K C, Choi H J, Chang C H, Cohen R E, Mckinley G H, Barbastathis G 2012 ACS Nano 6 3789
Google Scholar
[3] Guo P, Zheng Y M, Wen M X, Song C, Lin Y C, Jiang L 2012 Adv. Mater. 24 2642
Google Scholar
[4] Zhang Y F, Yu X Q, Wu H, Wu J 2012 Appl. Surf. Sci. 258 8253
Google Scholar
[5] 柯清平, 李广录, 郝天歌, 何涛, 李雪梅 2010 化学进展 22 284
Ke Q P, Li G L, Hao T G, He T, Li X M 2010 Prog. Chem. 22 284
[6] 郗金明 2008 博士学位论文 (北京: 中国科学院研究生院)
Xi J M 2008 Ph. D. Dissertation (Beijing: Graduate University of Chinese Academy of Sciences) (in Chinese)
[7] 刘俊吉, 周亚平, 李松林 2009 物理化学(下卷) (北京: 高等教育出版社) 第487页
Liu J J, Zhou Y P, Li S L 2009 Physical Chemistry (Vol. 2) (Beijing: Higher Education Press) p487 (in Chinese)
[8] He M, Wang J J, Li H L, Song Y L 2011 Soft Matter 7 3993
Google Scholar
[9] Enright R, Miljkovic N, Dou N, Nam Y, Wang E N 2013 J. Heat Transfer 135 091304
Google Scholar
[10] Zhang S N, Huang J Y, Tang Y X, Li S H, Ge M Z, Chen Z, Zhang K Q, Lai Y K 2017 Small 13 1600687
Google Scholar
[11] Aili A, Ge Q Y, Zhang T J 2017 J. Heat Transfer 139 112401
Google Scholar
[12] Wenzel R N 1936 J. Ind. Eng. Chem. 28 988
Google Scholar
[13] Cassie A B D, Baxter S 1944 Trans. Faraday Soc. 40 546
Google Scholar
[14] Narhe R D, Beysens D A 2004 Phys. Rev. Lett. 93 076103
Google Scholar
[15] Narhe R D, Beysens D A 2006 Europhys. Lett. 75 98
Google Scholar
[16] Rykaczewski K 2012 Langmuir 28 7720
Google Scholar
[17] Lau K K S, Bico J, Teo K B K, Chhowalla M, Amaratunga G A J, Milne W I, McKinley G H, Gleason K K 2003 Nano Lett. 3 1701
Google Scholar
[18] 郭照立, 郑楚光 2009 格子Boltzmann方法的原理和应用 (第一版) (北京: 科学出版社) 第9, 10页
Guo Z L, Zheng C G 2009 Theory and Applications of Lattice Boltzmann Method (Beijing: Science Press) pp9, 10 (in Chinese)
[19] 张博 2016 博士学位论文 (北京: 北京化工大学)
Zhang B 2016 Ph. D. Dissertation (Beijing: Beijing University of Chemical Technology) (in Chinese)
[20] Kusumaatmaja H, Leopoldes J, Dupuis A, Yeomans J M 2006 Europhys. Lett. 73 740
Google Scholar
[21] Kusumaatmaja H, Yeomans J M 2007 Langmuir 23 6019
Google Scholar
[22] Cui J, Li W Z, Lam W H 2011 Comput. Math. Appl. 61 3678
Google Scholar
[23] Liu X L, Cheng P, Quan X J 2014 Int. J. Heat Mass Transfer 73 195
Google Scholar
[24] Zhang Q Y, Sun D K, Zhang Y F, Zhu M F 2014 Langmuir 30 12559
Google Scholar
[25] Zhang Q Y, Sun D K, Zhang Y F, Zhu M F 2016 Chin. Phys. B 25 066401
Google Scholar
[26] Shan X W, Chen H D 1993 Phys. Rev. E 47 1815
Google Scholar
[27] 何雅玲, 王勇, 李庆 2009 格子Boltzmann方法的理论及应用 (第一版) (北京: 科学出版社) 第49−52页
He Y L, Wang Y, Li Q 2009 Lattice Boltzmann Method: Theory and Applications (Beijing: Science Press) pp49−52 (in Chinese)
[28] 吴伟 2012 硕士学位论文(南京: 东南大学)
Wu W 2012 M. S. Thesis (Nanjing: Southeast University) (in Chinese)
[29] Sukop M C, Jr Thorne D T 2005 Lattice Boltzmann Modeling-An Introduction for Geoscientists and Engineers (2nd Ed.) (New York: Springer) p89
[30] Chen C H, Cai Q, Tsai C, Chen C L, Xiong G, Yu Y, Ren Z 2007 Appl. Phys. Lett. 90 173108
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 10329
- PDF Downloads: 0
- Cited By: 0
