-
The structural randomness of porous medium presents significant challenges for accurately simulating colloidal transport. The Boltzmann transport equation (BTE) provides a reliable way for simulating the microscopic dynamics of colloidal particles in random space. By using the Chapman-Enskog (CE) method, a macroscopic advection-diffusion transport model is derived from the BTE. It includes a diffusion term dependent on the particle velocity distribution, a velocity delay term, and a capture term reflecting the microscopic capture mechanism, which tends to preferentially capture high-speed moving particles. These terms explain the delay and capture effects in colloidal transport. Meanwhile, the explicit expressions of the three transport coefficients are presented to provide a quantitative basis for using the model. The model is effective at small mixing filtration coefficients λl. By comparing the outlet concentration profiles of different models, the influences of this mechanism on the advection velocity delay and capture efficiency are elucidated. The model solves some of the paradoxes of traditional colloidal transport models, and under specific conditions, it is consistent with previous models. -
Keywords:
- porous media /
- colloidal transport /
- capture /
- Boltzmann equation
[1] Luna M, Gastone F, Tosco T, Sethi R, Velimirovic M, Gemoets J, Muyshondt R, Sapionet H, Klaas N, Bastiaens L 2015 J. Contam. Hydrol. 181 46
 Google Scholar
Google Scholar
[2] Tosco T, Gastone F, Sethi R 2014 J. Contam. Hydrol. 166 34
 Google Scholar
Google Scholar
[3] Fakhreddine S, Prommer H, Gorelick S M, Dadakis J, Fendorf S 2020 Environ. Sci. Technol. 54 8728
 Google Scholar
Google Scholar
[4] Boccardo G, Sethi R, Marchisio D L 2019 Chem. Eng. Sci. 198 290
 Google Scholar
Google Scholar
[5] 杨秀清, 胡亦, 张景路, 王艳秋, 裴春梅, 刘飞 2014 63 048102
 Google Scholar
Google Scholar
Yang X Q, Hu Y, Zhang J L, Wang Y Q, Pei C M, Liu F 2014 Acta Phys. Sin. 63 048102
 Google Scholar
Google Scholar
[6] Salimi S, Ghalambor A 2011 Energies 4 1728
 Google Scholar
Google Scholar
[7] Winter C L, Tartakovsky D M 2002 Water Resour. Res. 38 23-1
 Google Scholar
Google Scholar
[8] Russell T, Dinariev O Y, Pessoa Rego L A, Bedrikovetsky P 2021 Water Resour. Res. 57 e2020WR029557
 Google Scholar
Google Scholar
[9] Zou Z K, Yu L, Li Y L, Niu S Y, Fan L L, Luo W B, Li W 2023 Water 15 2193
 Google Scholar
Google Scholar
[10] Shapiro A A 2024 Phys. Fluids 36 027118
 Google Scholar
Google Scholar
[11] Panfilov M, Rasoulzadeh M 2013 Comput. Geosci. 17 269
 Google Scholar
Google Scholar
[12] Shapiro A A 2022 Chem. Eng. Sci. 248 117247
 Google Scholar
Google Scholar
[13] Herzig J P, Leclerc D M, Goff P L 1970 Ind. Eng. Chem. 62 8
[14] Bedrikovetsky P 2008 Transp. Porous Med. 75 335
 Google Scholar
Google Scholar
[15] Bedrikovetsky P, Siqueira A G, de Souza A L S, Altoé J E, Shecaira F 2006 J. Pet. Sci. Eng. 51 68
 Google Scholar
Google Scholar
[16] Bradford S A, Leij F J 2018 Chem. Eng. Sci. 192 972
 Google Scholar
Google Scholar
[17] Molnar I L, Pensini E, Asad M A, Mitchell C A, Nitsche L C, Pyrak-Nolte L J, Miño G L, Krol M M 2019 Transp. Porous Med. 130 129
 Google Scholar
Google Scholar
[18] Zhang H, Malgaresi G V C, Bedrikovetsky P 2018 Int. J. Non - Linear Mech. 105 27
 Google Scholar
Google Scholar
[19] Arns C H 2004 Physica A 339 159
 Google Scholar
Google Scholar
[20] Arns C H, Knackstedt M A, Martys N S 2005 Phys. Rev. E 72 046304
 Google Scholar
Google Scholar
[21] Russell T, Bedrikovetsky P 2021 Phys. Fluids 33 053306
 Google Scholar
Google Scholar
[22] Russell T, Bedrikovetsky P 2023 J. Comput. Appl. Math. 422 114896
 Google Scholar
Google Scholar
[23] Bhatnagar P L, Gross E P, Krook M 1954 Phys. Rev. 94 511
 Google Scholar
Google Scholar
[24] Grad H 1963 Phys. Fluids 6 147
 Google Scholar
Google Scholar
[25] Bradford S A, Yates S R, Bettahar M, Simunek J 2002 Water Resour. Res. 38 63-1
 Google Scholar
Google Scholar
[26] Tufenkji N, Elimelech M 2004 Environ. Sci. Technol. 38 529
 Google Scholar
Google Scholar
[27] Andrade J S, Araújo A D, Vasconcelos T F, Herrmann H J 2008 Eur. Phys. J. B 64 433
 Google Scholar
Google Scholar
[28] Wang H Q, Lacroix M, Masséi N, Dupont J P 2000 C. R. Acad. Sci. - Ser. IIA - Earth Planet. Sci. 331 97
 Google Scholar
Google Scholar
[29] Yang Y, Bedrikovetsky P 2017 Transp. Porous Med. 119 351
 Google Scholar
Google Scholar
[30] Malgaresi G, Collins B, Alvaro P, Bedrikovetsky P 2019 Chem. Eng. J. 375 121984
 Google Scholar
Google Scholar
[31] Hashemi A, Nguyen C, Loi G, Khazali N, Yutong Y, Dang-Le B, Russell T, Bedrikovetsky P 2023 Chem. Eng. J. 474 145436
 Google Scholar
Google Scholar
-
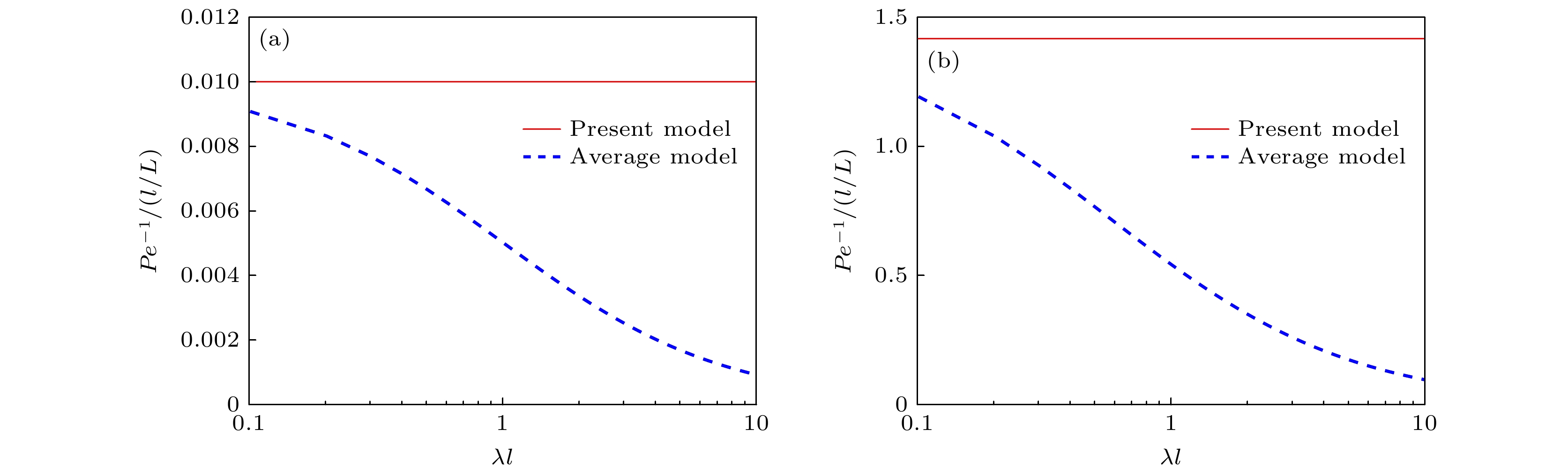
图 5 本模型和R-平均模型(average model)的$ 1/P{e^*} $比较 (a) $ {C_v} = 0.1\left( {\alpha = 1} \right) $; (b) $ {C_v} = 1.19\left( {\alpha = 0.8} \right) $
Figure 5. Comparison of the parameter $ 1/P{e^*} $ between the present model and the average model: (a) $ {C_v} = 0.1\left( {\alpha = 1} \right) $; (b) $ {C_v} = $$ 1.19\left( {\alpha = 0.8} \right) $.
图 6 本模型和R-平均模型(average model)的延迟数θ (a) $ {C_v} = 0.1\left( {\alpha = 1} \right) $; (b) $ {C_v} = 1.19\left( {\alpha = 0.8} \right) $
Figure 6. Comparison of the parameter θ between the present model and the average model: (a) $ {C_v} = 0.1\left( {\alpha = 1} \right) $; (b) $ {C_v} = $$ 1.19\left( {\alpha = 0.8} \right) $.
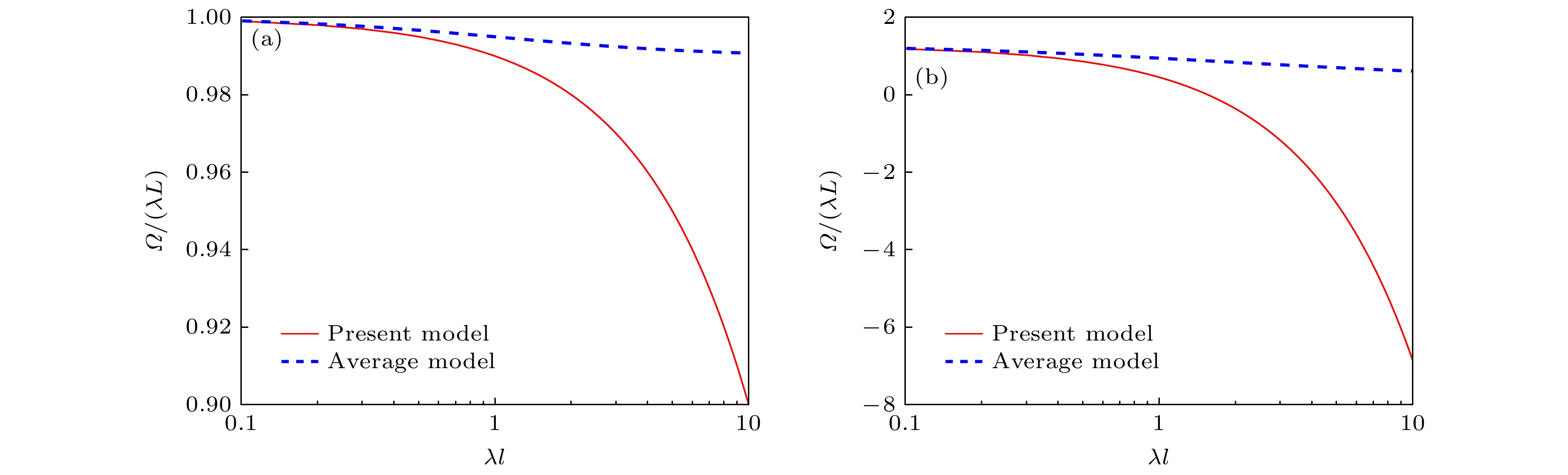
图 7 本模型和R-平均模型(average model)的参数$ \varPsi = \varOmega /\left( {\lambda L} \right) $比较 (a) $ {C_v} = 0.1\left( {\alpha = 1} \right) $; (b) $ {C_v} = 1.19\left( {\alpha = 0.8} \right) $
Figure 7. Comparison of the parameter $ \varPsi = \varOmega /\left( {\lambda L} \right) $ between the present model and the average model: (a) $ {C_v} = 0.1\left( {\alpha = 1} \right) $; (b) $ {C_v} = 1.19\left( {\alpha = 0.8} \right) $.
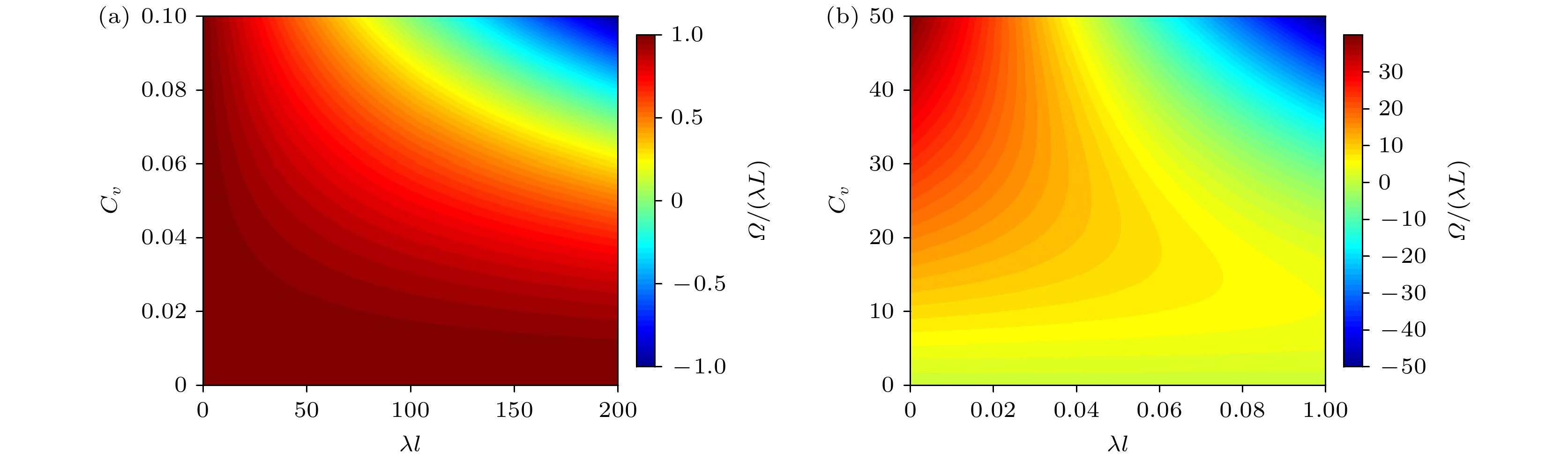
图 8 本文模型的宏观系数θ对$ {C_v} $和$ \lambda l $的敏感性分析 (a) $ {C_v} \in (0, 0.1],\; \lambda l \in [0, 200] $; (b) $ {C_v} \in (0, 50],\; \lambda l \in [0, 0.1] $
Figure 8. Sensitivity analysis of the macroscopic coefficient θ of the present model to $ {C_v} $ and $ \lambda l $: (a) $ {C_v} \in (0, 0.1], \;\lambda l \in [0, 200] $; (b) $ {C_v} \in (0, 50], \;\lambda l \in [0, 0.1] $.
图 9 本文模型的宏观系数$ \varPsi = \varOmega /\left( {\lambda L} \right) $对$ {C_v} $和$ \lambda l $的敏感性分析 (a) $ {C_v} \in (0, 0.1],\; \lambda l \in [0, 200] $; (b) $ {C_v} \in (0, 50], \;\lambda l \in [0, 0.1] $
Figure 9. Sensitivity analysis of the macroscopic coefficient $ \varPsi = \varOmega /\left( {\lambda L} \right) $ of the present model to $ {C_v} $ and $ \lambda l $: (a) $ {C_v} \in $$ (0, 0.1],\; \lambda l \in [0, 200] $; (b) $ {C_v} \in (0, 50], \;\lambda l \in [0, 0.1] $.
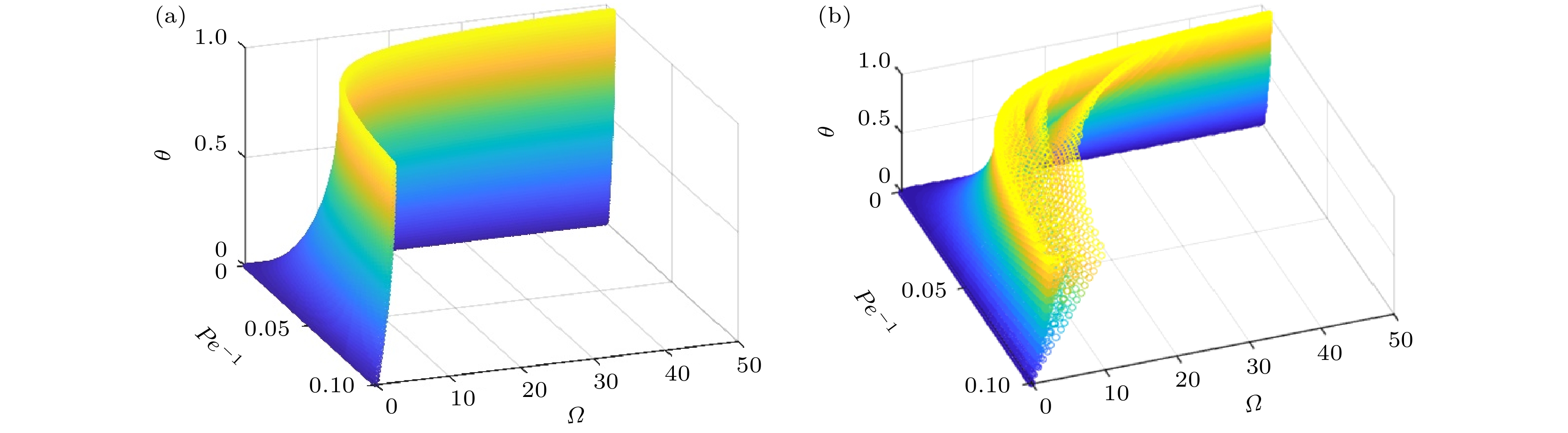
图 10 从微观参数对$ \left( {\lambda , l, {C_v}} \right) $计算宏观参数对$ \left( {P{e^{ - 1}}, \theta , \varOmega } \right) $的结果 (a) $ \alpha = 1 $; (b) $ \alpha < 1 $
Figure 10. Results of calculating the macroscopic parameter for $ \left( {P{e^{ - 1}}, \theta , \varOmega } \right) $ from the microscopic parameter for $ \left( {\lambda , l, {C_v}} \right) $: (a) $ \alpha = 1 $; (b) $ \alpha < 1 $.
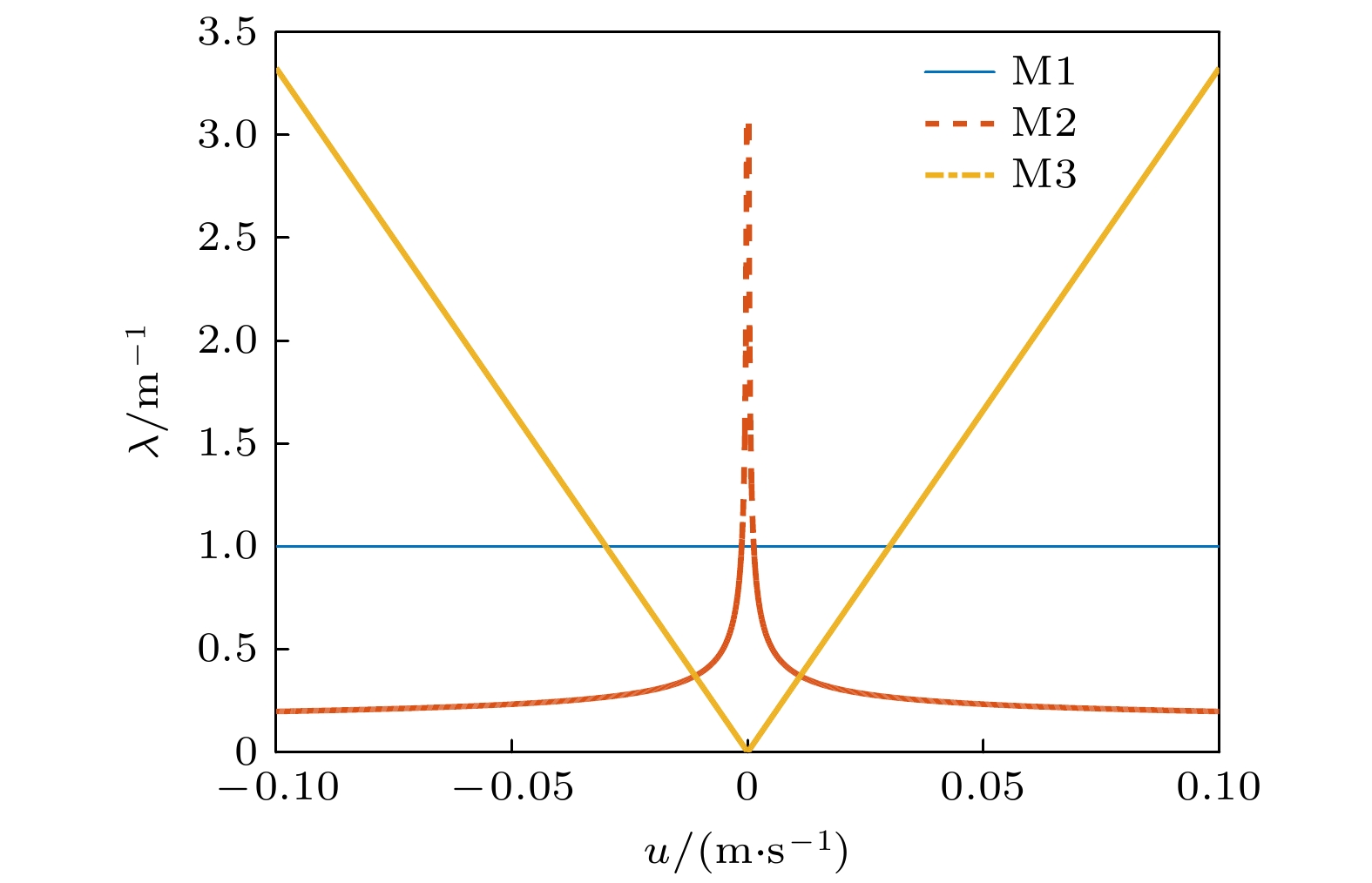
图 12 三种捕获机制下的宏观系数关于平均速度u的灵敏度研究($ s = {10^{ - 2}},\, l = {10^{ - 6}}, \,\alpha = 0.5—1 $) (a) $ P{e^{ - 1}} $; (b) $ \theta $; (c) $ \varOmega $
Figure 12. Sensitivity analysis of the macroscopic coefficient under three capture mechanisms to the average velocity u $( s = {10^{ - 2}}, \;l = {10^{ - 6}}, \;\alpha = 0.5-1 $): (a) $ P{e^{ - 1}} $; (b) θ; (c) Ω.
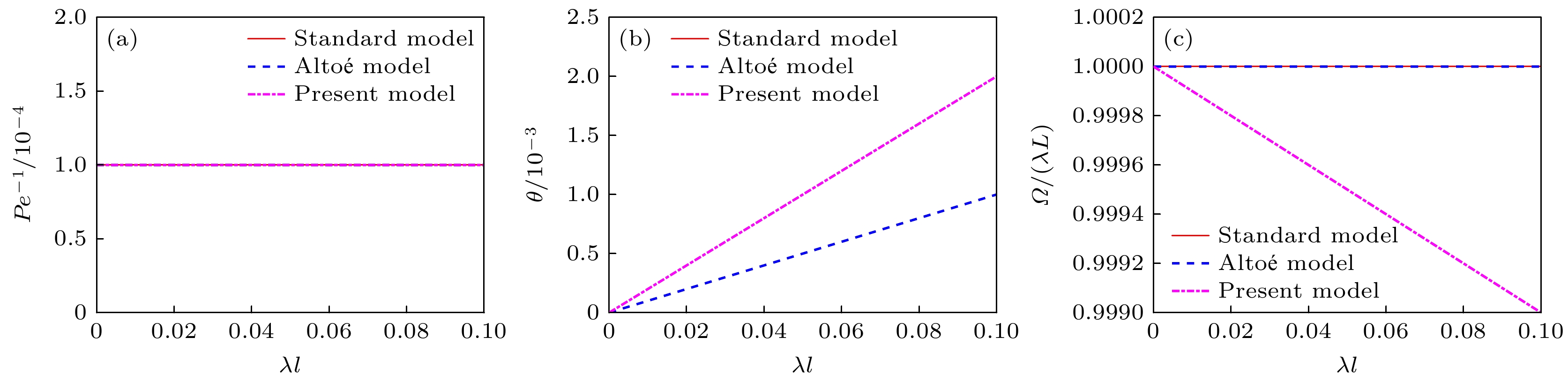
图 13 粒子速度为正值时本文模型与传统模型在$ \lambda l $很小时的系数比较 (a) $ P{e^{ - 1}} $; (b) $ \theta $; (c) $ \varOmega /\left( {\lambda L} \right) $
Figure 13. Comparison of the coefficients between the present model and traditional models at very small $ \lambda l $ when the particle velocities are positive: (a) $ P{e^{ - 1}} $; (b) $ \theta $; (c) $ \varOmega /\left( {\lambda L} \right) $.
表 1 宏观系数的显式表达
Table 1. Explicit expression of macroscopic coefficients.
α = 1 α < 1 $ {{Pe}}^{-1} $ $ {({l}{/}{L}){{C}}_{{v}}^{2}}_{}^{} $ $ ({l}{/}{L}){{C}}_{{v}}^{2} $ θ $ 2{ \lambda l}{{C}}_{{v}}^{2} $ $ 2{ \lambda l}\left\{\left(1+{{C}}_{{v}}^{2}\right)\left[2\varPhi\left(\displaystyle \frac{1}{{{C}}_{{v}}}\right)-1\right]+\displaystyle \frac{2{{C}}_{{v}}}{\sqrt{{2\pi}}}\text{exp}\left(-\frac{1}{2{{C}}_{{v}}^{2}}\right)\right\} $ Ω $ \lambda{ L}{(1}-{ \lambda l}{{C}}_{{v}}^{2}) $ $\lambda L \left[ \left[ 2\varPhi\left( \dfrac{1}{C_v} \right) - 1 \right] + \dfrac{2C_v}{\sqrt{2\pi}} \exp\left( -\dfrac{1}{2C_v^2} \right) - \lambda l \left( C_v^2 + 1 - \left\{ \left[ 2\varPhi\left( \dfrac{1}{C_v} \right) - 1 \right] + \dfrac{2C_v}{\sqrt{2\pi}} \exp\left( -\dfrac{1}{2C_v^2} \right) \right\}^2 \right) \right] $ 注:$ \varPhi \left( x \right) = \displaystyle \int_{ - \infty }^x {\frac{{\exp \left( { - {y^2}/2} \right)}}{{\sqrt {2{\pi{}}} }}} {\text{d}}y. $ -
[1] Luna M, Gastone F, Tosco T, Sethi R, Velimirovic M, Gemoets J, Muyshondt R, Sapionet H, Klaas N, Bastiaens L 2015 J. Contam. Hydrol. 181 46
 Google Scholar
Google Scholar
[2] Tosco T, Gastone F, Sethi R 2014 J. Contam. Hydrol. 166 34
 Google Scholar
Google Scholar
[3] Fakhreddine S, Prommer H, Gorelick S M, Dadakis J, Fendorf S 2020 Environ. Sci. Technol. 54 8728
 Google Scholar
Google Scholar
[4] Boccardo G, Sethi R, Marchisio D L 2019 Chem. Eng. Sci. 198 290
 Google Scholar
Google Scholar
[5] 杨秀清, 胡亦, 张景路, 王艳秋, 裴春梅, 刘飞 2014 63 048102
 Google Scholar
Google Scholar
Yang X Q, Hu Y, Zhang J L, Wang Y Q, Pei C M, Liu F 2014 Acta Phys. Sin. 63 048102
 Google Scholar
Google Scholar
[6] Salimi S, Ghalambor A 2011 Energies 4 1728
 Google Scholar
Google Scholar
[7] Winter C L, Tartakovsky D M 2002 Water Resour. Res. 38 23-1
 Google Scholar
Google Scholar
[8] Russell T, Dinariev O Y, Pessoa Rego L A, Bedrikovetsky P 2021 Water Resour. Res. 57 e2020WR029557
 Google Scholar
Google Scholar
[9] Zou Z K, Yu L, Li Y L, Niu S Y, Fan L L, Luo W B, Li W 2023 Water 15 2193
 Google Scholar
Google Scholar
[10] Shapiro A A 2024 Phys. Fluids 36 027118
 Google Scholar
Google Scholar
[11] Panfilov M, Rasoulzadeh M 2013 Comput. Geosci. 17 269
 Google Scholar
Google Scholar
[12] Shapiro A A 2022 Chem. Eng. Sci. 248 117247
 Google Scholar
Google Scholar
[13] Herzig J P, Leclerc D M, Goff P L 1970 Ind. Eng. Chem. 62 8
[14] Bedrikovetsky P 2008 Transp. Porous Med. 75 335
 Google Scholar
Google Scholar
[15] Bedrikovetsky P, Siqueira A G, de Souza A L S, Altoé J E, Shecaira F 2006 J. Pet. Sci. Eng. 51 68
 Google Scholar
Google Scholar
[16] Bradford S A, Leij F J 2018 Chem. Eng. Sci. 192 972
 Google Scholar
Google Scholar
[17] Molnar I L, Pensini E, Asad M A, Mitchell C A, Nitsche L C, Pyrak-Nolte L J, Miño G L, Krol M M 2019 Transp. Porous Med. 130 129
 Google Scholar
Google Scholar
[18] Zhang H, Malgaresi G V C, Bedrikovetsky P 2018 Int. J. Non - Linear Mech. 105 27
 Google Scholar
Google Scholar
[19] Arns C H 2004 Physica A 339 159
 Google Scholar
Google Scholar
[20] Arns C H, Knackstedt M A, Martys N S 2005 Phys. Rev. E 72 046304
 Google Scholar
Google Scholar
[21] Russell T, Bedrikovetsky P 2021 Phys. Fluids 33 053306
 Google Scholar
Google Scholar
[22] Russell T, Bedrikovetsky P 2023 J. Comput. Appl. Math. 422 114896
 Google Scholar
Google Scholar
[23] Bhatnagar P L, Gross E P, Krook M 1954 Phys. Rev. 94 511
 Google Scholar
Google Scholar
[24] Grad H 1963 Phys. Fluids 6 147
 Google Scholar
Google Scholar
[25] Bradford S A, Yates S R, Bettahar M, Simunek J 2002 Water Resour. Res. 38 63-1
 Google Scholar
Google Scholar
[26] Tufenkji N, Elimelech M 2004 Environ. Sci. Technol. 38 529
 Google Scholar
Google Scholar
[27] Andrade J S, Araújo A D, Vasconcelos T F, Herrmann H J 2008 Eur. Phys. J. B 64 433
 Google Scholar
Google Scholar
[28] Wang H Q, Lacroix M, Masséi N, Dupont J P 2000 C. R. Acad. Sci. - Ser. IIA - Earth Planet. Sci. 331 97
 Google Scholar
Google Scholar
[29] Yang Y, Bedrikovetsky P 2017 Transp. Porous Med. 119 351
 Google Scholar
Google Scholar
[30] Malgaresi G, Collins B, Alvaro P, Bedrikovetsky P 2019 Chem. Eng. J. 375 121984
 Google Scholar
Google Scholar
[31] Hashemi A, Nguyen C, Loi G, Khazali N, Yutong Y, Dang-Le B, Russell T, Bedrikovetsky P 2023 Chem. Eng. J. 474 145436
 Google Scholar
Google Scholar
Catalog
Metrics
- Abstract views: 479
- PDF Downloads: 16
- Cited By: 0














 DownLoad:
DownLoad: