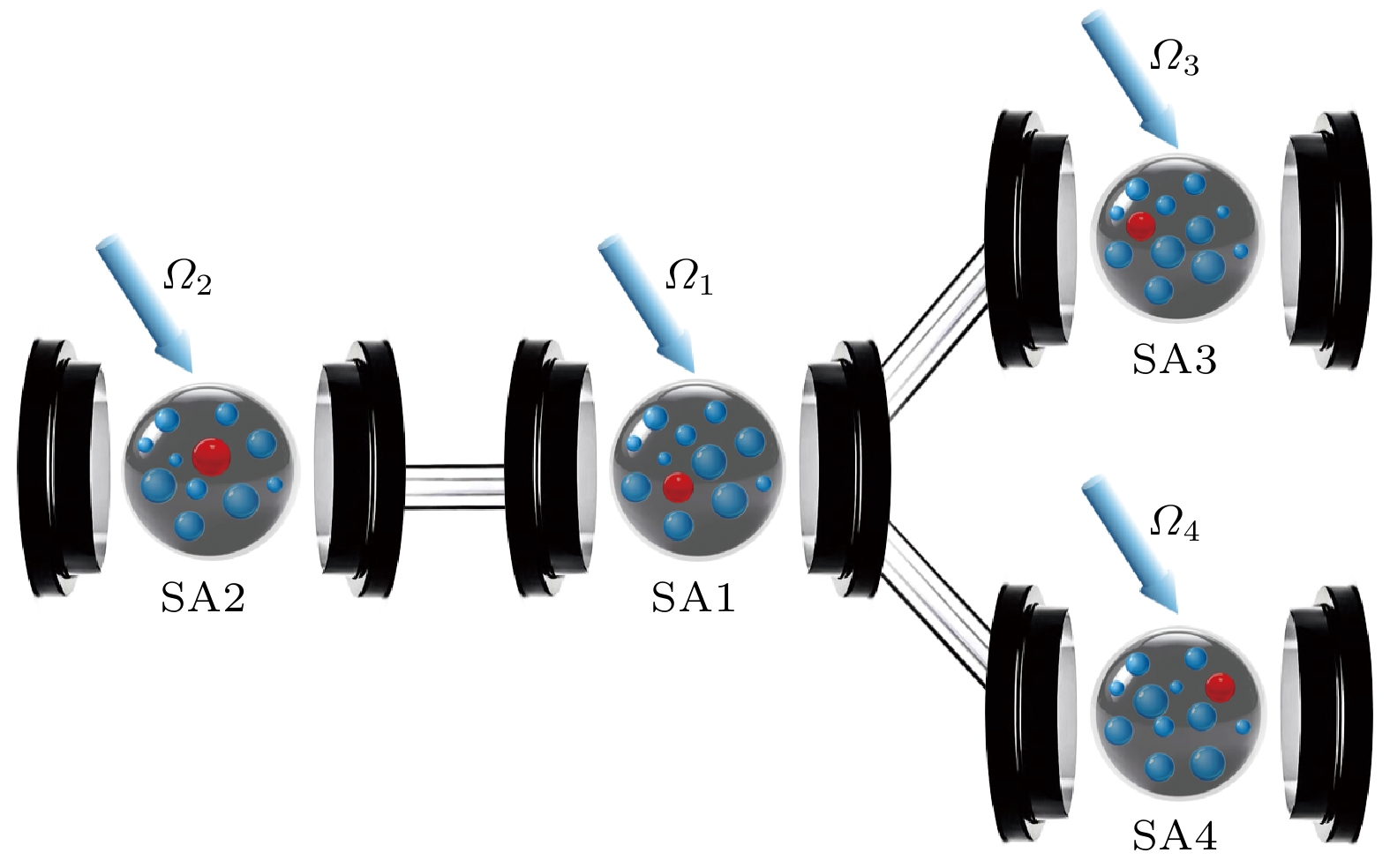
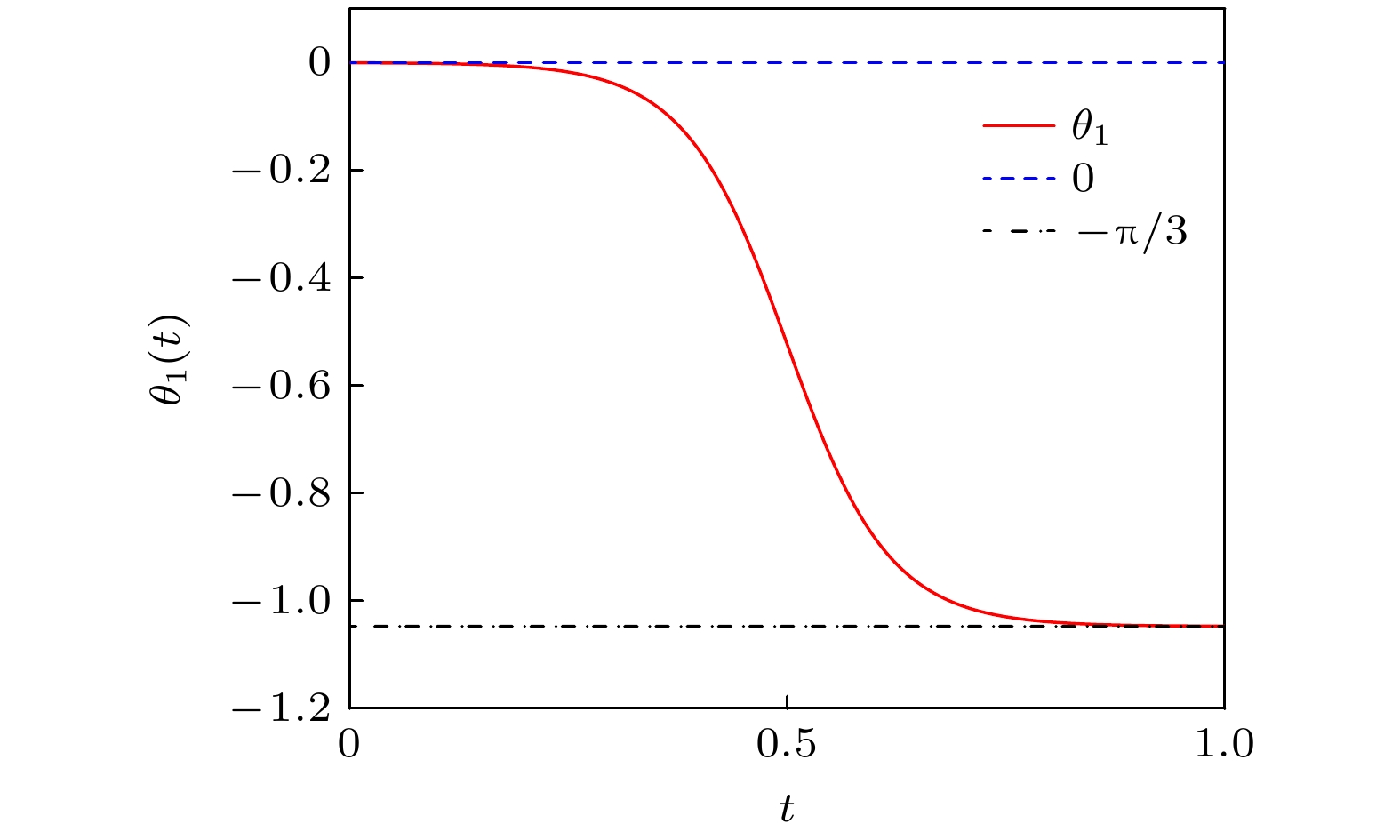
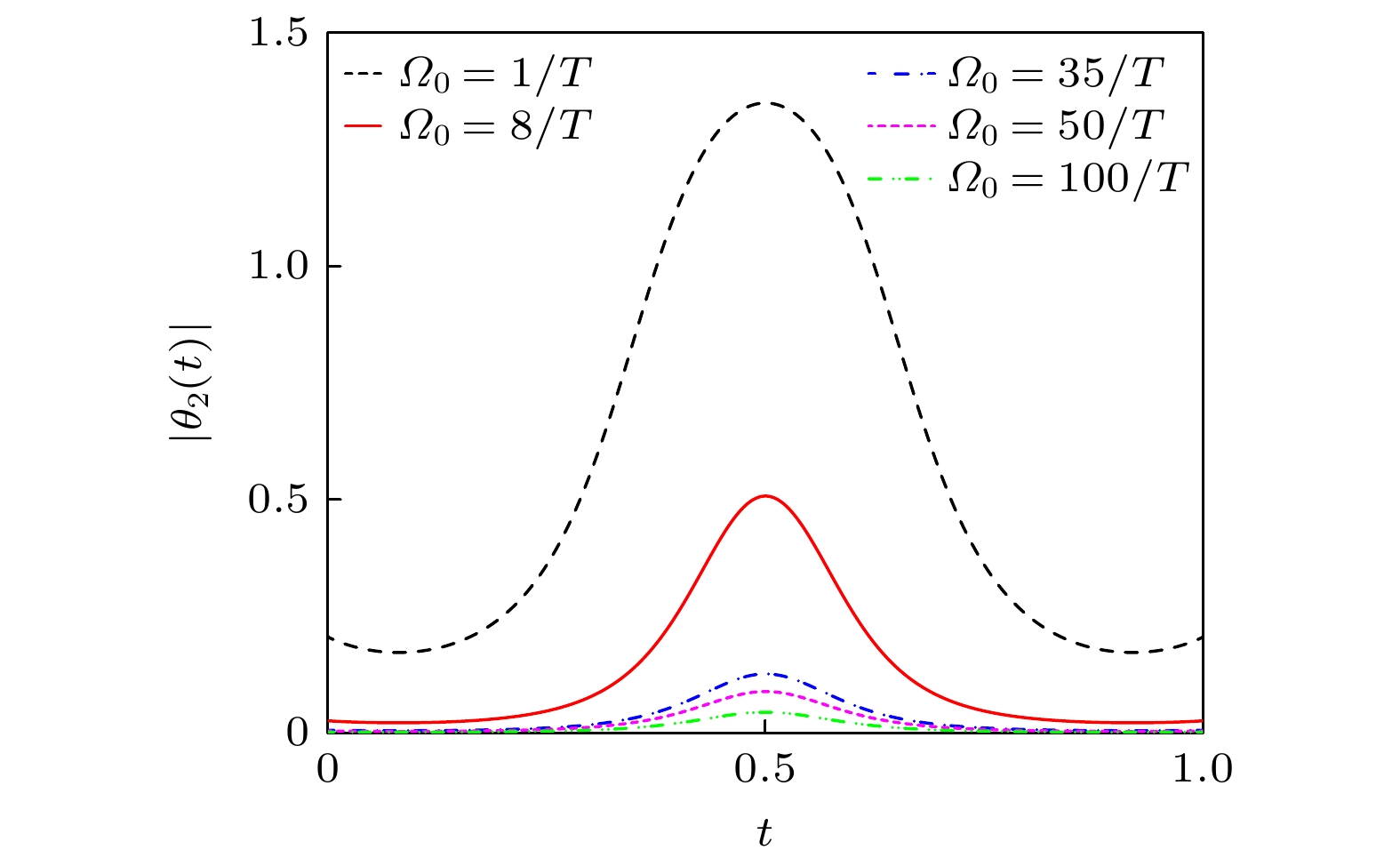
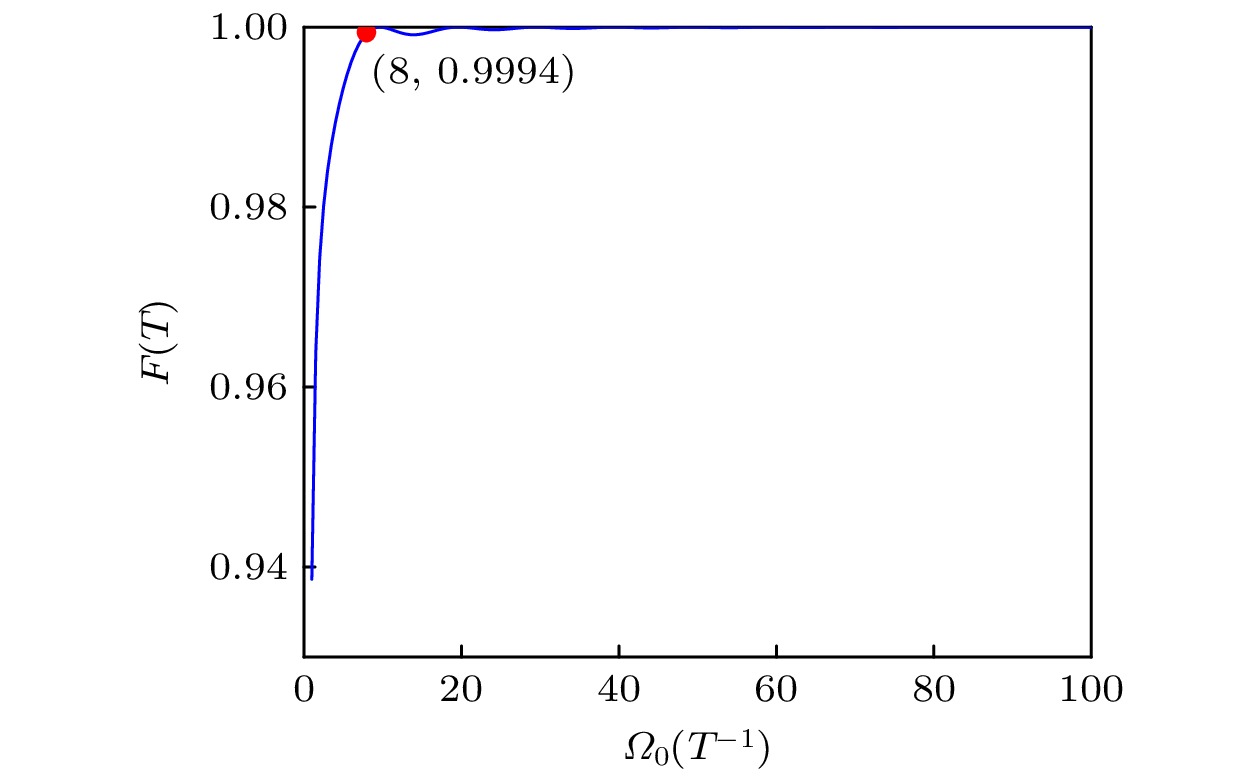
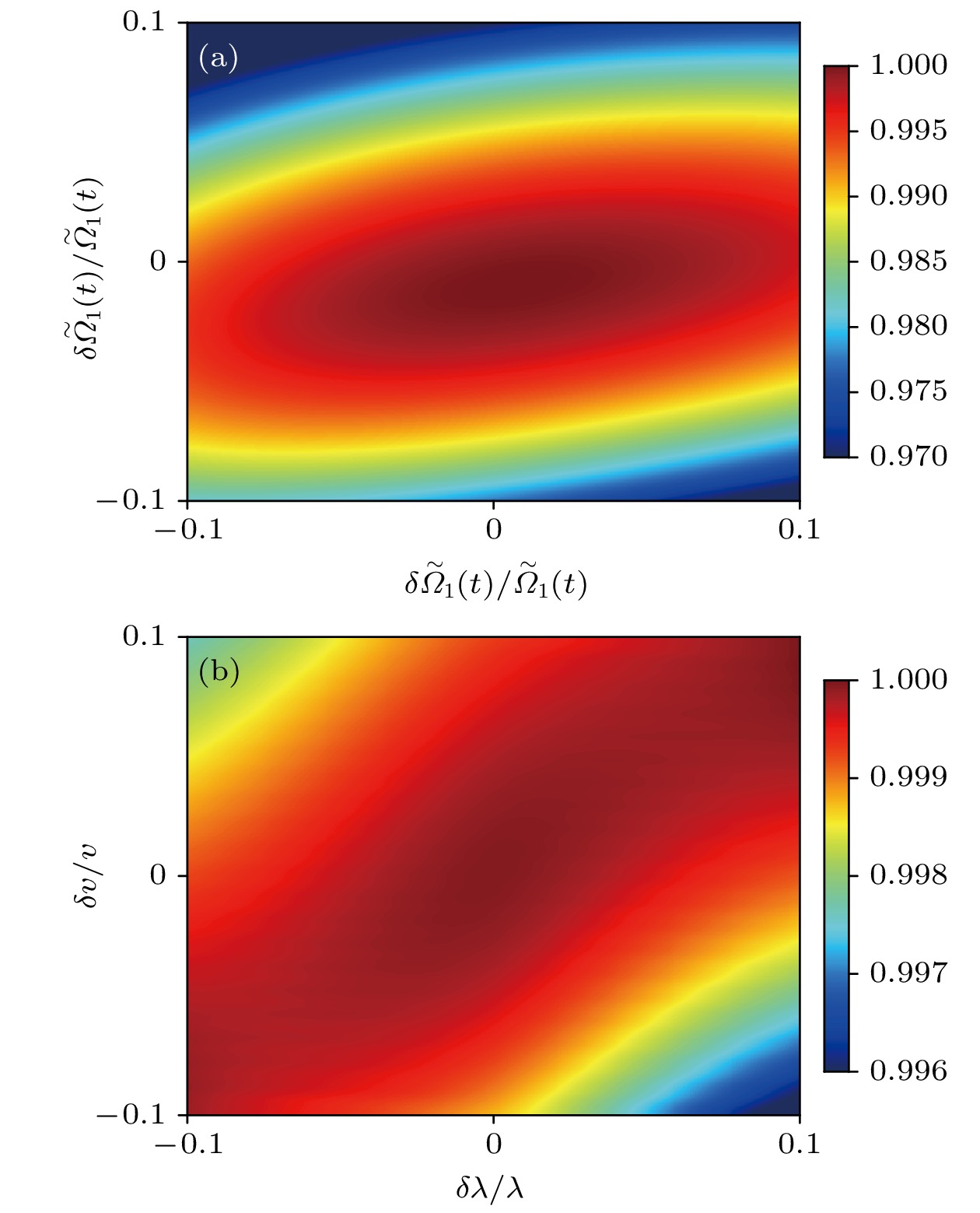
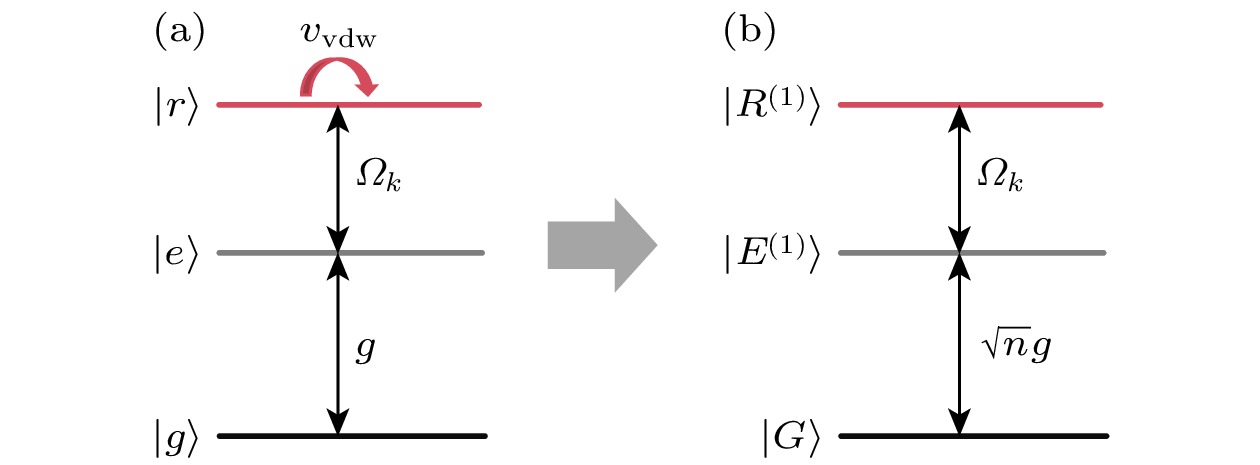
-
The W state, as a robust multipartite entangled state, plays an important role in quantum information processing, quantum network construction and quantum computing. In this paper, the three-level ladder-type Rydberg atomic system is put into the Rydberg blocking ball to form a superatom. Each superatom has many collective states including just one Rydberg excitation constrained by the Rydberg blockade effect. In the weak cavity field limit, at most one atom can be pumped into excited state, then we can describe the superatom by a three-level ladder-type system. Afterwards we encode quantum information on the effective energy levels of Rydberg superatoms and propose a fast scheme for preparing the Rydberg superatom W state based on the superadiabatic iterative technique and quantum Zeno dynamics, this scheme can be achieved in only one step by controlling the laser pulses. In the current scheme, the superatoms are trapped in spatially separated cavities connected by optical fibers, which significantly enhances the feasibility of experimental manipulation. A remarkable feature is that it does not require precise control of experimental parameters and interaction time. Meanwhile, the form of counterdiabatic Hamiltonian is the same as that of the effective Hamiltonian. Through numerical simulations, the fidelity of this scheme can reach 99.94%. Even considering decoherence effects, including atomic spontaneous emission and photon leakage, the fidelity can still exceed 97.5%, further demonstrating the strong robustness of the solution. In addition, the Rabi frequency can be characterized as a linear superposition of Gaussian functions, this representation significantly alleviates the complexity encountered in practical experiments. Futhermore, we also analyzed the impact of parameter fluctuations on the fidelity, the results show that this scheme is robust against parameter fluctuations. At last, the present scheme is extended to the cases of N Rydberg superatoms, which shows the scalability of our scheme.
-
Keywords:
- Superadiabatic /
- W state /
- Rydberg superatoms
-
图 1 (a)单个里德堡原子能级结构图; (b)里德堡超级原子的等效能级结构图
Figure 1. (a) Energy level structure diagram of a single Rydberg atom; (b) The equivalent energy level structure diagram of Rydberg superatom.
图 2 里德堡超级原子与腔系统的示意图. SA是里德堡超级原子, $ \Omega_k $是第k个腔中经典场的拉比频率
Figure 2. Schematic diagram of the structure of the Rydberg superatom-cavity system. SA denots the Rydberg superatom, and $ \Omega_k $ is the classical field Rabi frequency in the k-th cavity.
图 3 $ \theta_1(t) $随时间的变化关系. 选取的参数为 $ t_0 = 0.14 T $ 和 $ t_c = 0.19 T $
Figure 3. The $ \theta_1(t) $ as a function of the time. The parameters $ t_0 = 0.14 T $ and $ t_c = 0.19 T $.
图 4 $ \theta_2(t) $ 随时间的变化关系. 选取的参数为$ t_0 = 0.14 T $ 和 $ t_c = 0.19 T $
Figure 4. The $ \theta_2(t) $ as a function of the time. The parameters $ t_0 = 0.14 T $and $ t_c = 0.19 T $.
图 5 $ \Omega_0(T^{-1}) $对保真度$ F(T) $的影响图. 当$ \Omega_0 = 8 T^{-1} $时, 保真度$ F(T) = 0.9994 $
Figure 5. The influence of $ \Omega_0(T^{-1}) $ on fidelity $ F(T) $. When $ \Omega_0 = 8 T^{-1} $, the fidelity $ F(T) = 0.9994 $.
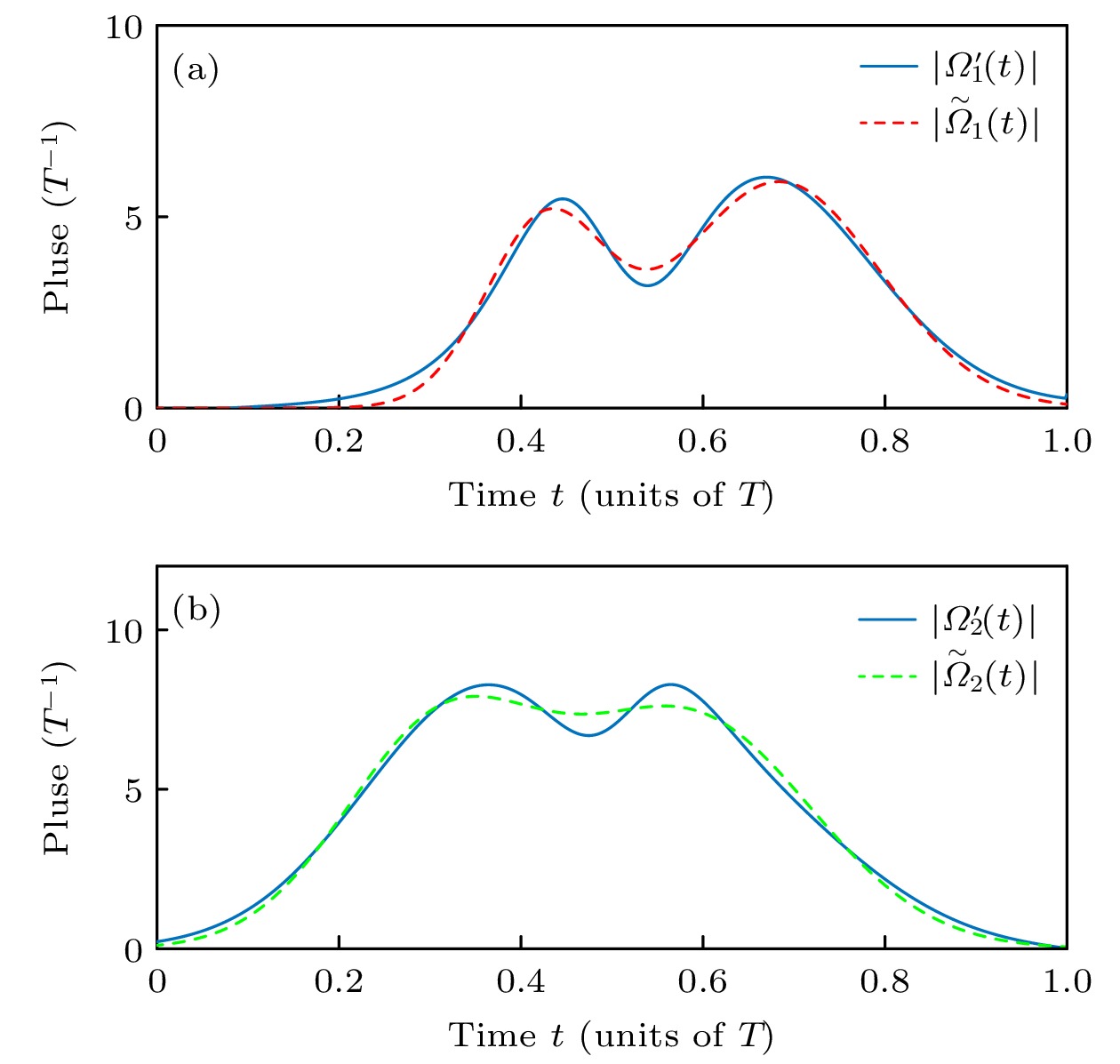
图 6 (a)对比脉冲$ \Omega'_1(t) $和拟合的高斯脉冲$ \widetilde{\Omega}_1(t) $. (b)对比脉冲$ \Omega'_2(t) $和拟合的高斯脉冲$ \widetilde{\Omega}_2(t) $
Figure 6. (a) Comparing the pulse $ \Omega '_1 (t) $ and the fitting of gaussian pulse $ \widetilde {\Omega} _1 (t) $. (b) Comparing the pulse $ \Omega '_2 (t) $ and the fitting of gaussian pulse $ \widetilde {\Omega} _2 (t) $.
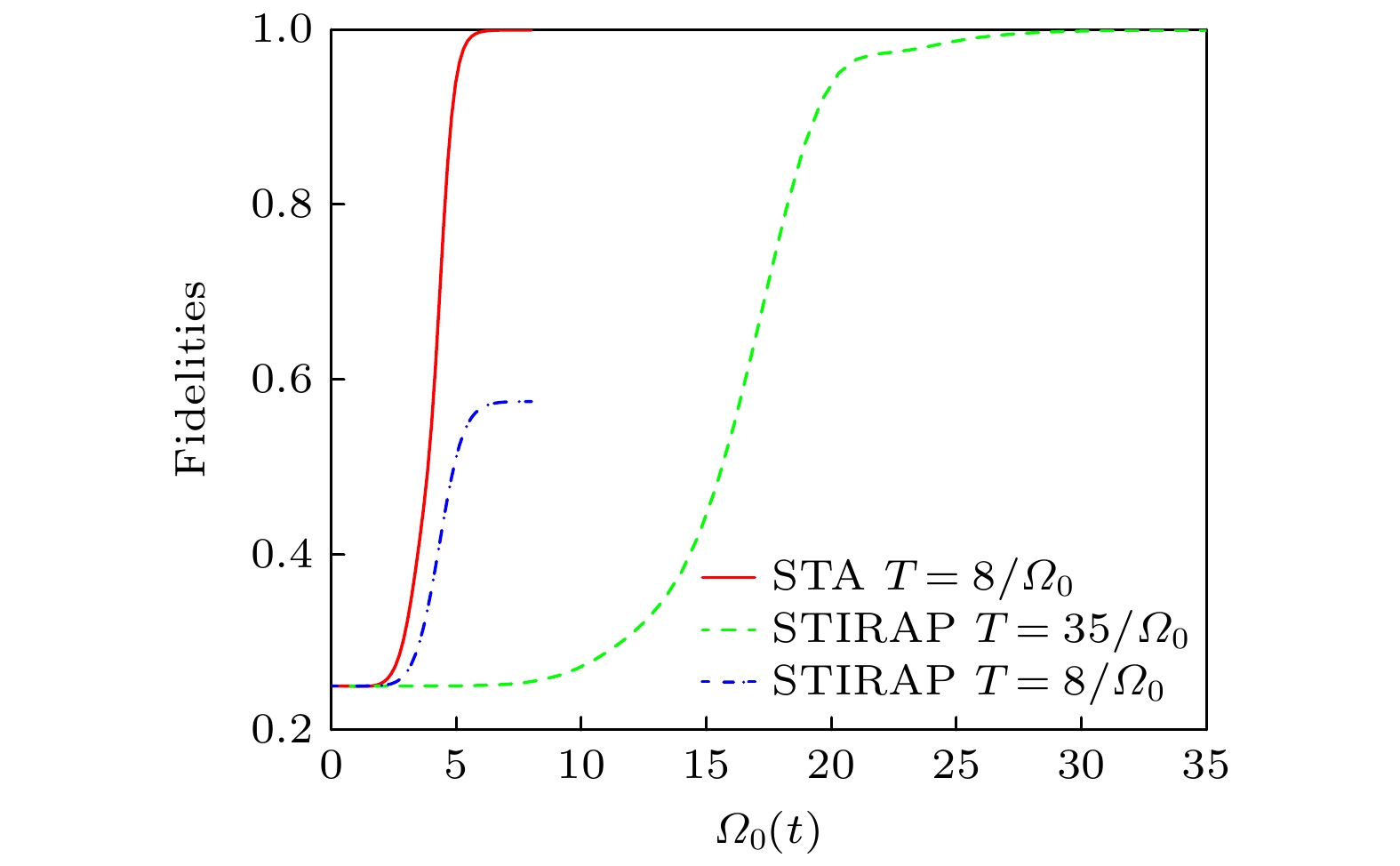
图 7 W态的保真度在超绝热迭代$ T = 8/\Omega_0 $、绝热演化$ T = 8/\Omega_0 $、绝热演化$ T = 35/\Omega_0 $三种不同情况下随时间的变化
Figure 7. Under the three different conditions: superadiabatic iteration $ T = 8/\Omega_0 $, adiabatic evolution $ T = 8/\Omega_0 $ and adiabatic evolution $ T = 35/\Omega_0 $, the fidelity of W state as a function of the time.
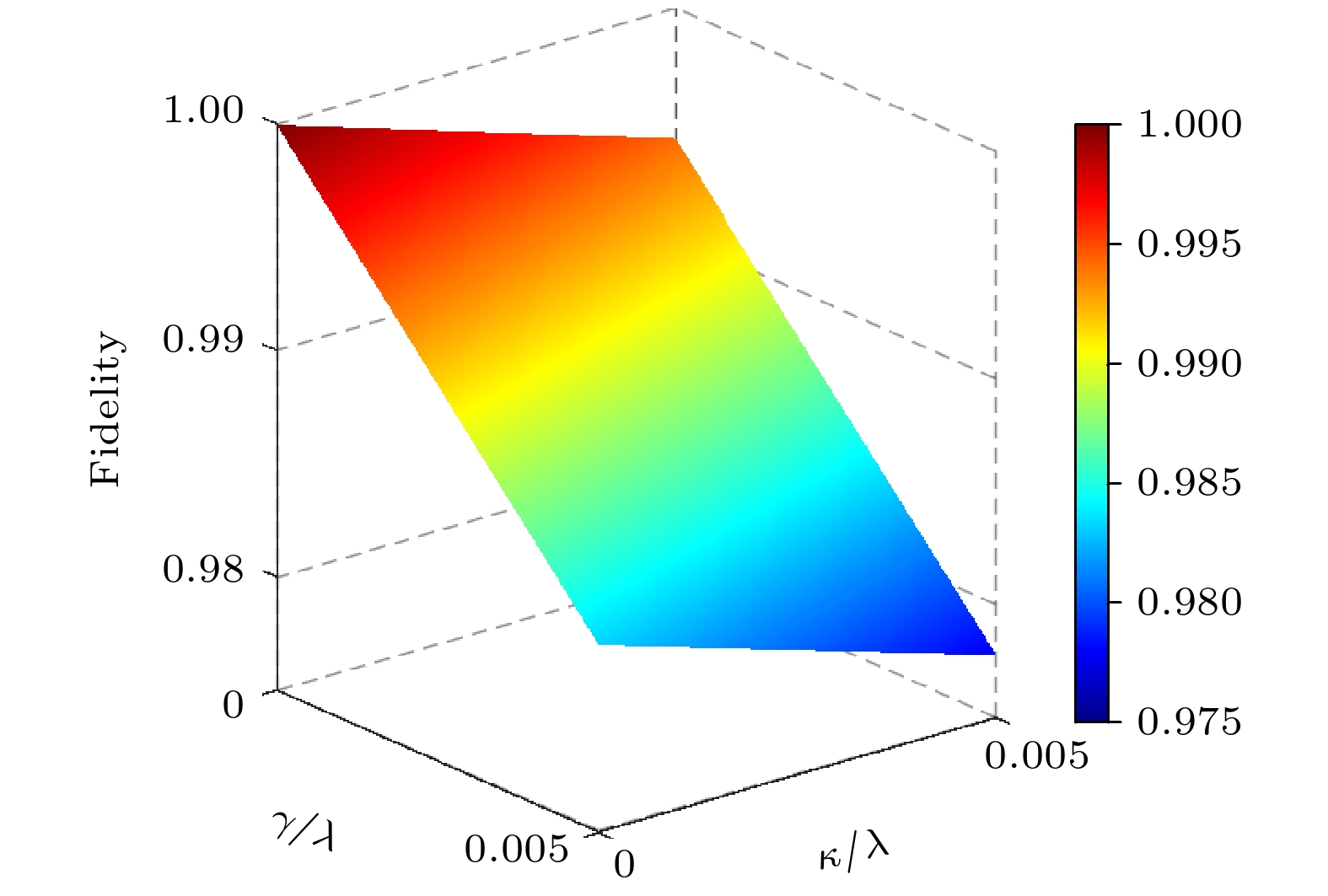
图 8 哈密顿量$ H_{tot} $的控制下的W态的保真度与$ \kappa/\lambda $和$ \gamma/\lambda $的关系. $ T = 8/\Omega_0, \Omega_0 = 0.1\lambda $
Figure 8. The relationship between the fidelity of the W state and $ \kappa/\lambda $, $ \gamma/\lambda $ by the Hamiltonian $ H_{tot} $. $ T = 8 / \Omega_0, $$ \Omega_0 = 0.1\lambda $.
图 9 (a)保真度随$ \delta \widetilde{\Omega}_1 $ 和 $ \delta \widetilde{\Omega}_2 $的变化. (b)保真度随$ \delta \lambda $和$ \delta v $的变化
Figure 9. (a)The fidelity versus $ \delta \widetilde{\Omega}_1 $ and $ \delta \widetilde{\Omega}_2 $. (b)The fidelity versus $ \delta \lambda $ and $ \delta v $.
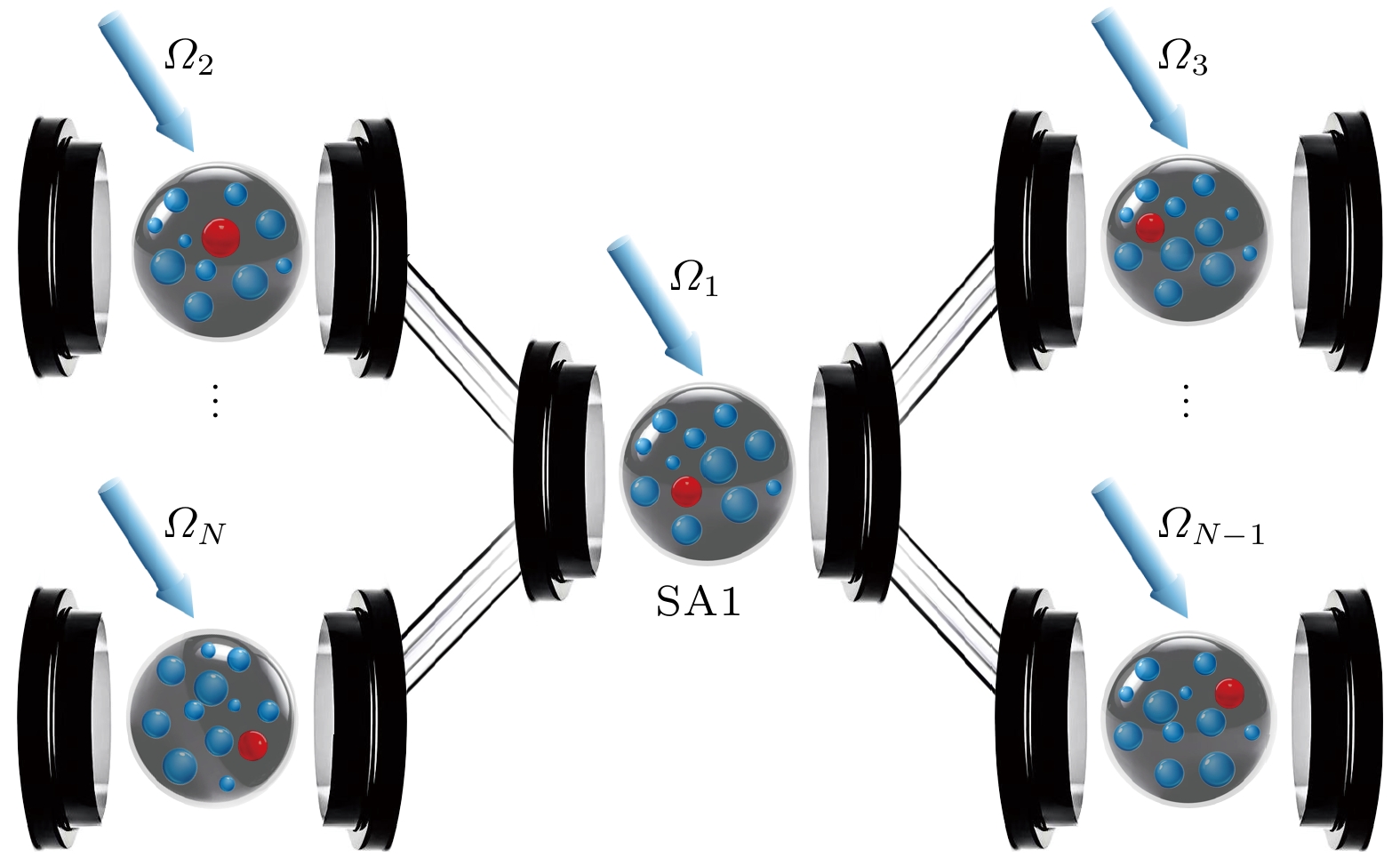
图 10 N个里德堡超级原子-腔系统结构型的示意图. 每个里德堡超级原子被分别放置在不同的真空腔中, 第 2 到第 N 个腔均与第 1 个腔相连. $ \Omega_N $是第N个腔中经典场驱动的拉比频率
Figure 10. A schematic diagram illustrating the structure of N-Rydberg superatom-cavity system. Each of the Rydberg superatom is placed in a separate vacuum cavity, with cavities 2 through N all connected to cavity 1. $ \Omega_N $ is the classical field-driven Rabi frequency in the N-th cavity.
-
[1] Gisin N, Ribordy G, Tittel W, Zbinden H 2002 Rev. Mod. Phys. 74 145
Google Scholar
[2] Markham D, Sanders B C 2008 Phys. Rev. A 78 042309
Google Scholar
[3] Bennett C H, Brassard G, Crépeau C, Jozsa R, Peres A, Wootters W K 1993 Phys. Rev. Lett. 70 1895
Google Scholar
[4] Luo Y H, Zhong H S, Erhard M, Wang X L, Peng L C, Krenn M, Jiang X, Li L, Liu N L, Lu C Y, et al 2019 Phys. Rev. Lett. 123 070505
Google Scholar
[5] Xia Y, Song J, Lu P M, Song H S 2010 JOSA B 27 A1
Google Scholar
[6] Ekert A K 1991 Phys. Rev. Lett. 67 661
Google Scholar
[7] Bennett C H, Brassard G, Mermin N D 1992 Phys. Rev. Lett. 68 557
Google Scholar
[8] Long G L, Liu X S 2002 Phys. Rev. A 65 032302
Google Scholar
[9] Scarani V, Bechmann-Pasquinucci H, Cerf N J, Dušek M, Lütkenhaus N, Peev M 2009 Rev. Mod. Phys. 81 1301
Google Scholar
[10] Briegel H J, Browne D E, Dür W, Raussendorf R, Van den Nest M 2009 Nat. Phys. 5 19
Google Scholar
[11] Zhao P Z, Cui X D, Xu G, Sjöqvist E, Tong D 2017 Phys. Rev. A 96 052316
Google Scholar
[12] Su S L, Gao Y, Liang E, Zhang S 2017 Phys. Rev. A 95 022319
Google Scholar
[13] Wu J L, Su S L, Wang Y, Song J, Xia Y, Jiang Y Y 2020 Opt. Lett. 45 1200
Google Scholar
[14] Li X H, Deng F G, Zhou H Y 2006 Phys. Rev. A 74 054302
Google Scholar
[15] Zhu A D, Xia Y, Fan Q B, Zhang S 2006 Phys. Rev. A 73 022338
Google Scholar
[16] Li T, Long G L 2020 New J. Phys. 22 063017
Google Scholar
[17] Greenberger D M, Horne M A, Zeilinger A 1989 In Bell’ s theorem, quantum theory and conceptions of the universe (Springer), pp 69–72
[18] Shao X Q, Liu F, Xue X W, Mu W, Li W b 2023 Phys. Rev. Appl 20 014014
Google Scholar
[19] Dür W, Vidal G, Cirac J I 2000 Phys. Rev. A 62 062314
Google Scholar
[20] Cabello A 2002 Phys. Rev. A 65 032108
Google Scholar
[21] Agrawal P, Pati A 2006 Phys. Rev. A 74 062320
Google Scholar
[22] Wang A, Wei Y Z, Li Z Y, Jiang M 2023 IET Quantum Commun. 4 200
Google Scholar
[23] Renner R 2008 Int. J. Quantum Inf. 6 1
Google Scholar
[24] Lo H K, Ma X, Chen K 2005 Phys. Rev. Lett. 94 230504
Google Scholar
[25] Lipinska V, Murta G, Wehner S 2018 Phys. Rev. A 98 052320
Google Scholar
[26] Miguel-Ramiro J, Riera-Sàbat F, Dür W 2023 PRX Quantum 4 040323
Google Scholar
[27] Su S L, Shao X Q, Wang H F, Zhang S 2014 Phys. Rev. A 90 054302
Google Scholar
[28] Han J X, Wu J L, Wang Y, Xia Y, Jiang Y Y, Song J 2021 Phys. Rev. A 103 032402
Google Scholar
[29] Shao X Q, Wang Z, Liu H, Yi X 2016 Phys. Rev. A 94 032307
Google Scholar
[30] Shao X Q, Su S L, Li L, Nath R, Wu J H, Li W 2024 Appl. Phys. Rev. 11
[31] Shao Q P, Wang J, Ji Y, Liu Y, Dong L, Xiu X M 2023 J. Opt. Soc. Am. B 41 143
[32] Zhang W Y, Wang C Q, Ji Y Q, Shao Q P, Wang J P, Wang J, Yang L P, Dong L, Xiu X M 2024 Adv. Quantum Technol. 7 2300140
Google Scholar
[33] Saffman M, Walker T G, Mølmer K 2010 Rev. Mod. Phys. 82 2313
Google Scholar
[34] Beguin L, Vernier A, Chicireanu R, Lahaye T, Browaeys A 2013 Phys. Rev. Lett. 110 263201
Google Scholar
[35] Xing T, Zhao P, Tong D 2021 Phys. Rev. A 104 012618
Google Scholar
[36] Lukin M D, Fleischhauer M, Cote R, Duan L, Jaksch D, Cirac J I, Zoller P 2001 Phys. Rev. Lett. 87 037901
Google Scholar
[37] Urban E, Johnson T A, Henage T, Isenhower L, Yavuz D, Walker T, Saffman M 2009 Nat. Phys. 5 110
Google Scholar
[38] Gaëtan A, Miroshnychenko Y, Wilk T, Chotia A, Viteau M, Comparat D, Pillet P, Browaeys A, Grangier P 2009 Nat. Phys. 5 115
Google Scholar
[39] Wilk T, Gaëtan A, Evellin C, Wolters J, Miroshnychenko Y, Grangier P, Browaeys A 2010 Phys. Rev. Lett. 104 010502
Google Scholar
[40] Dudin Y O, Li L, Bariani F, Kuzmich A 2012 Nat. Phys. 8 790
Google Scholar
[41] Shao X Q, Wu J, Yi X 2017 Phys. Rev. A 95 062339
Google Scholar
[42] Su S L, Li W 2021 Phys. Rev. A 104 033716
Google Scholar
[43] Wu J L, Wang Y, Han J X, Su S L, Xia Y, Jiang Y, Song J 2021 Phys. Rev. A 103 012601
Google Scholar
[44] Zeiher J, Schauß P, Hild S, Macrì T, Bloch I, Gross C 2015 Phys. Rev. X 5 031015
[45] Yang L, Wang J, Ji Y, Wang J, Zhang Z, Liu Y, Dong L, Xiu X 2024 Eur. Phys. J. Plus 139 1
Google Scholar
[46] Xu W, Venkatramani A V, Cantú S H, Šumarac T, Klüsener V, Lukin M D, Vuletić V 2021 Phys. Rev. Lett. 127 050501
Google Scholar
[47] Zhao P Z, Wu X, Xing T, Xu G, Tong D 2018 Phys. Rev. A 98 032313
Google Scholar
[48] Paris-Mandoki A, Braun C, Kumlin J, Tresp C, Mirgorodskiy I, Christaller F, Büchler H P, Hofferberth S 2017 Phys. Rev. X 7 041010
[49] Liu Y L, Ji Y Q, Han X, Cui W X, Zhang S, Wang H F 2023 Adv. Quantum Technol. 6 2200173
Google Scholar
[50] Baksic A, Ribeiro H, Clerk A A 2016 Phys. Rev. Lett. 116 230503
Google Scholar
[51] Wu J L, Ji X, Zhang S 2017 Sci. Rep. 7 46255
Google Scholar
[52] Chen Y H, Qin W, Wang X, Miranowicz A, Nori F 2021 Phys. Rev. Lett. 126 023602
Google Scholar
[53] Giannelli L, Arimondo E 2014 Phys. Rev. A 89 033419
Google Scholar
[54] Wang X M, Zhang A Q, Zhao S M 2022 ACTA PHYSICA SINICA 71
[55] Berry M V 2009 J. Phys. A: Math. Theor. 42 365303
Google Scholar
[56] Ibáñez S, Chen X, Muga J 2013 Phys. Rev. A 87 043402
Google Scholar
[57] Song X K, Ai Q, Qiu J, Deng F G 2016 Phys. Rev. A 93 052324
Google Scholar
[58] Huang B H, Chen Y H, Wu Q C, Song J, Xia Y 2016 Laser Phys. Lett. 13 105202
Google Scholar
[59] Wu J L, Su S L, Ji X, Zhang S 2017 Ann. Phys. 386 34
Google Scholar
[60] Löw R, Weimer H, Nipper J, Balewski J B, Butscher B, Büchler H P, Pfau T 2012 J. Phys. B: At., Mol. Opt. Phys. 45 113001
Google Scholar
[61] Su S L, Sun L N, Liu B J, Yan L L, Yung M H, Li W, Feng M 2023 Phys. Rev. Appl. 19 044007
Google Scholar
[62] Du F F, Fan Z G, Ren X M, Ma M, Liu W Y 2024 Chin. Phys. B
[63] Shao X Q 2020 Phys. Rev. A 102 053118
Google Scholar
[64] Facchi P, Pascazio S 2002 Phys. Rev. Lett. 89 080401
Google Scholar
[65] Wu J L, Song C, Xu J, Yu L, Ji X, Zhang S 2016 Quantum Inf. Process. 15 3663
Google Scholar
[66] Shore B W 2017 Adv. Opt. Photonics 9 563
Google Scholar
[67] Zhang X, Isenhower L, Gill A, Walker T, Saffman M 2010 Phys. Rev. A 82 030306
Google Scholar
[68] Isenhower L, Urban E, Zhang X, Gill A, Henage T, Johnson T A, Walker T, Saffman M 2010 Phys. Rev. Lett. 104 010503
Google Scholar
[69] Guerlin C, Brion E, Esslinger T, Mølmer K 2010 Phys. Rev. A 82 053832
Google Scholar
[70] Zhang X F, Sun Q, Wen Y C, Liu W M, Eggert S, Ji A C 2013 Phys. Rev. Lett. 110 090402
Google Scholar
DownLoad:
Metrics
- Abstract views: 334
- PDF Downloads: 19
- Cited By: 0
