-
Quantum entanglement is a key resource for performing quantum computing and building quantum communication networks. By injecting a microwave-optical dual-mode entanglement field into the system, as well as pumping the optical and microwave cavities, and by appropriately choosing the detuning relationship between the pumping field and the modes, it is shown in this work that microwave-phonon entanglement Eab and magnon-optics entanglement Ecm can be generated simultaneously in the cavity opto-magnomechanic system, and the entanglement can be in a steady state. Specifically, the model is based on a hybrid quantum system of magnons, where a microwave-light entanglement generated by an optically pulsed superconducting electro-optical device through spontaneous parametric down-conversion process is injected as the intracavity field, and a blue-detuned microwave field is used to excite the magnon modes to produce magnon-phonon entanglement. Through the interaction between an optomechanical beam splitter and microwave-magnon state-swap, steady microwave-phonon entanglement Eab and magnon-optics entanglement Ecm are successfully realized. The entanglement Eab and Ecm in the system are analyzed using the logarithmic negativity. The effects of several parameters of the system, such as environment temperature, coupling strength and dissipation rate, on the degree of entanglement are investigated. In particular, the entanglement Eab and Ecm generated in this system can exist both simultaneously and individually. Especially when gam=0, the entanglement Eab and Ecm still exist. Moreover, directly injecting entangled microwave-light into the system can significantly enhance the robustness of the entanglement against temperature, which will have broad application prospects in quantum information processing in quantum networks and hybrid quantum systems. Notably, the entanglement Eab and Ecm exist even at a temperature of 1.3 K. Our research has potential value for applications in the fields of quantum information processing and quantum networks.
-
Keywords:
- quantum entanglement /
- opto-magnomechanics /
- two-mode squeezing
-
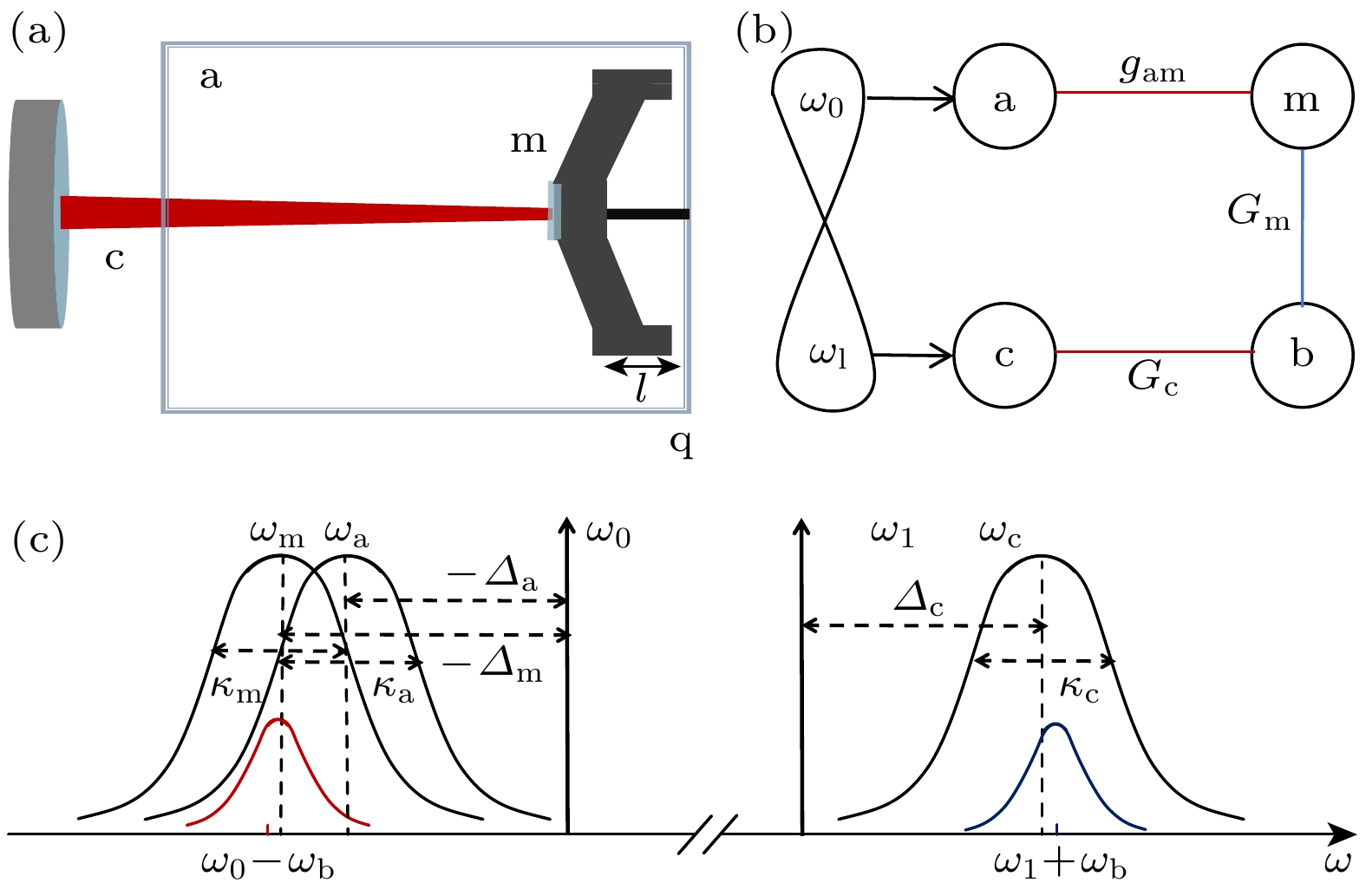
图 1 (a), (b) OMM系统示意图. YIG晶体中的磁振子模式m耦合到微波腔模a并且通过磁致伸缩引起振动声子b耦合到光学腔模c. 图(b)中蓝色(红色)线表示参量下转换(分束器)相互作用; (c)模型采用的模式频率和带宽. 当光学腔模与驱动激光场的反斯托克斯边带(蓝色)在频率为($ {\omega _{\text{l}}} + {\omega _{\text{b}}} $)处共振, 并且磁子模和微波腔模同时与微波驱动场的斯托克斯边带(红色)在频率为($ {\omega _0} - {\omega _{\text{b}}} $)处共振, 可以建立微波-声子以及光-磁子之间的稳态纠缠Eab和Ecm
Figure 1. (a), (b) OMM system scheme. A magnon mode m in a YIG crystal couples to a microwave cavity mode a and to an optical cavity mode c via the mechanical vibration b induced by the magnetostriction. The blue (red) line in Figure (b) denotes the effective parametric down-conversion (beam-splitter) interaction; (c) mode frequency and bandwidth used adopted in the protocol. When the optical cavity is resonant with the (blue) anti-Stokes sideband of the driving laser at frequency ($ {\omega _0} - {\omega _{\text{b}}} $), and the magnon and microwave cavity modes are resonant with the (red) Stokes sideband of the microwave drive field at frequency ($ {\omega _{\text{l}}} + {\omega _{\text{b}}} $), microwave-mechanics entanglement Eab and magnon-optics entanglement Ecm can be generated at steady state.
图 2 稳态纠缠随磁子模式和微波腔模式有效失谐量变化密度图 (a)微波-声子纠缠Eab; (b)光-磁纠缠Ecm
Figure 2. Density plot of steady-state entanglement versus effective detunings ${\varDelta _{\text{a}}}$ and ${\tilde \varDelta _{\text{m}}}$: (a) Microwave-mechanics entanglement Eab; (b) magnon-optics entanglement Ecm.
图 3 微波-声子, 光-磁以及微波-光纠缠随压缩参数r的变化
Figure 3. Stationary microwave-mechanics entanglement Eab, magnon-optics entanglement Ecm and microwave-optics entanglement Eac versus squeezing parameter r.
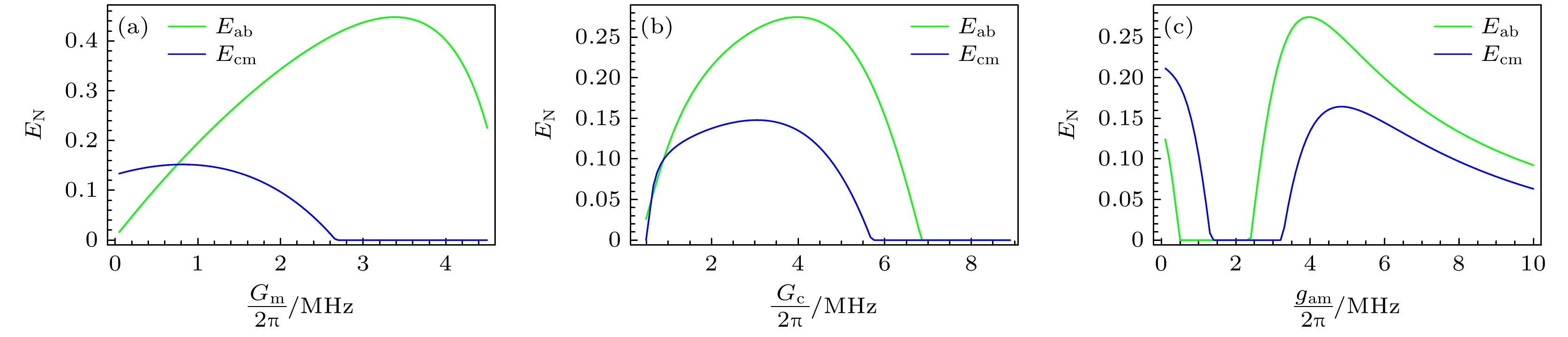
图 4 光-磁和微波-声子之间纠缠随有效耦合强度变化 (a)有效光力耦合强度Gc; (b)有效磁力耦合强度Gm; (c)微波-磁子耦合强度gam
Figure 4. Stationary magnon-optics entanglement Ecm and microwave-mechanics entanglement Eab versus effective coupling strength: (a) The effective optomechanical coupling strength Gc; (b) the effective magno-mechanical coupling strength Gm; (c) the microwave-magnon coupling rate gam.
图 5 微波-声子以及光-磁之间纠缠随不同模式耗散率变化 (a) 微波腔模耗散率$ {\kappa _{\text{a}}} $; (b) 磁振子模耗散率$ {\kappa _{\text{m}}} $; (c) 光学腔模耗散率$ {\kappa _{\text{c}}} $
Figure 5. Stationary microwave-mechanics entanglement Eab and magnon-optics entanglement Ecm versus dissipation rates: (a) Microwave cavity mode dissipation rate $ {\kappa _{\text{a}}} $; (b) magnon mode dissipation rate $ {\kappa _{\text{m}}} $; (c) optical cavity mode dissipation rate $ {\kappa _{\text{c}}} $.
图 6 微波-声子以及光-磁之间纠缠 (a) 随温度T变化; (b) 随机械振子耗散率$ {\gamma _{\text{b}}} $变化
Figure 6. Stationary microwave-mechanics entanglement Eab and magnon-optics entanglement Ecm versus: (a) Temperature T; (b) mechanical damping rate $ {\gamma _{\text{b}}} $.
-
[1] Tang J F, Hou Z B, Shang J W , Zhu H J, Xiang G Y, Li C F, Guo G C 2020 Phys. Rev. Lett. 124 060502
[2] Konrad T, De Melo F, Tiersch M, Kasztelan C, Aragão A, 2008 Buchleitner A Nat. Phys. 4 99
Google Scholar
[3] Aspelmeyer M, Böhm H R, Gyatso T, Jennewein T, Kaltenbaek R, Lindenthal M, Molina-Terriza G, Poppe A, Taraba M, Ursin R, Walther P, Zeilinger A 2003 Science 301 621
Google Scholar
[4] 杨荣国, 张超霞, 李妮, 张静, 郜江瑞 2019 68 094205
Google Scholar
Yang R G, Zhang C X, Li N, Zhang J, Gao J R 2019 Acta Phys. Sin. 68 094205
Google Scholar
[5] Liang Y Y, Yang R G, Zhang J, Zhang T C 2023 Opt. Express 31 11775
Google Scholar
[6] Zhang K, Wang W, Liu S H, Pan X Z, Du J J, Lou Y B, Yu S, Lü S C, Treps N, Fabre C, Jing J T 2020 Phys. Rev. Lett. 124 090501
Google Scholar
[7] 郝景晨, 杜培林, 孙恒信, 刘奎, 张静, 杨荣国, 郜江瑞 2024 73 074203
Google Scholar
Hao J C, Du P L, Sun H X, Liu K, Zhang J, Yang R G, Gao J R 2024 Acta Phys. Sin. 73 074203
Google Scholar
[8] 闫智辉, 贾晓军, 谢常德, 彭堃墀 2012 61 014206
Google Scholar
Yan Z H, Jia X J, Xie C D, Peng K C 2012 Acta Phys. Sin. 61 014206
Google Scholar
[9] Liu K, Cui S Z, Yang R G, Zhang J X, Gao J R 2012 Chin. Phys. Lett. 29 060304
Google Scholar
[10] 刘奎, 马龙, 苏必达, 李佳明, 孙恒信, 郜江瑞 2024 29 124203
Liu K, Ma L, Su B D, Li J M, Sun H X, Gao J R 2020 Acta Phys. Sin. 69 124203
[11] Yang R G, Zhang J, Klich I, González-Arciniegas C, Pfister O 2020 Phys. Rev. A 101 043832
Google Scholar
[12] Du P L, Wang Y, Liu K, Yang R G, Zhang J 2023 Opt. Express 31 479420
[13] Aspelmeyer M, Kippenberg T J, Marquardt F 2014 Rev. Mod. Phys. 86 1391
Google Scholar
[14] Liu X M, Yang R G, Zhang J, Zhang T C 2023 Opt. Express 31 30005
Google Scholar
[15] 罗均文, 吴德伟, 苗强, 魏天丽 2020 69 054203
Google Scholar
Luo J W, Wu D W, Miao Q, Wei T L 2020 Acta Phys. Sin. 69 054203
Google Scholar
[16] Palomaki T A, Teufel J D, Simmonds R W, Lehnert K W 2013 Science 342 710
Google Scholar
[17] Riedinger R, Wallucks A, Marinkovi´c I, Löschnauer C, Aspelmeyer M, Hong S, Gröblacher S 2018 Nature 556 473
Google Scholar
[18] Wollman E E, Lei C U, Weinstein A J, Suh J, Kronwald A, Marquardt F, Clerk A A, Schwab K C 2015 Science 349 952
Google Scholar
[19] Zhang X F, Zou C L, Jiang L, Tang H X 2016 Sci. Adv. 2 e1501286
Google Scholar
[20] Shen R C, Li J, Fan Z Y, Wang Y P, You J Q 2022 Phys. Rev. Lett. 129 123601
Google Scholar
[21] Huebl H, Zollitsch C W, Lotze J, Hocke F, Greifenstein M, Marx A, Gross R, Goennenwein S T B 2013 Phys. Rev. Lett. 111 127003
Google Scholar
[22] Tabuchi Y, Ishino S, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2014 Phys. Rev. Lett. 113 083603
Google Scholar
[23] Bai L, Harder M, Chen Y P, Fan X, Xiao J Q, Hu C M 2015 Phys. Rev. Lett. 114 227201
Google Scholar
[24] Osada A, Hisatomi R, Noguchi A, Tabuchi Y, Yamazaki R, Usami K, Sadgrove M, Yalla R, Nomura M, Nakamura Y 2016 Phys. Rev. Lett. 116 223601
Google Scholar
[25] Zhang X F, Zhu N, Zou C L, Tang H X 2016 Phys. Rev. Lett. 117 123605
Google Scholar
[26] Osada A, Gloppe A, Hisatomi R, Noguchi A, Yamazaki R, Nomura M, Nakamura Y, Usami K 2018 Phys. Rev. Lett. 120 133602
Google Scholar
[27] Zhu N, Zhang X, Han X, Zou C L, Zhong C, Wang C H, Jiang L, Tang H X 2020 Optica 7 1291
Google Scholar
[28] Haigh J A, Nunnenkamp A, Ramsay A J 2021 Phys. Rev. Lett. 127 143601
Google Scholar
[29] Yang Z B, Liu X D, Yin X Y, Ming Y, Liu H Y, Yang R C 2021 Phys. Rev. Appl. 15 024042
Google Scholar
[30] Yin X Y, Yang Z B, Huang Y M, Wan Q M, Yang R C, Liu H Y 2023 Ann. Phys. 535 2200603
Google Scholar
[31] Fan Z Y, Shen R C, Wang Y P, Li J, You J Q 2022 Phys. Rev. A 105 033507
Google Scholar
[32] Fan Z Y, Qian H, Li J 2023 Quantum Sci. Technol. 8 015014
Google Scholar
[33] Fan Z Y, Qiu L, Gröblacher S, Li J 2023 Laser Photonics Rev. 17 2200866
Google Scholar
[34] Rueda A, Hease W, Barzanjeh S, Fink J M 2019 npj Quantum Inf. 5 108
Google Scholar
[35] Hease W, Rueda A, Sahu R, Wulf M, Arnold G, Schwefel H G L, Fink J M 2020 PRX Quantum 1 020315
Google Scholar
[36] Andersen U L, Gehring T, Marquardt C, Leuchs G 2016 Phys. Scr. 91 053001
Google Scholar
[37] Sahu R, Qiu L, Hease W, Arnold G, Minoguchi Y, Rabl P, Fink J M 2023 Science 380 718
Google Scholar
[38] Li J, Zhu S Y, Agarwal G S 2018 Phys. Rev. Lett. 121 203601
Google Scholar
[39] Lachance-Quirion D, Tabuchi Y, Gloppe A, Usami K, Nakamura Y 2019 Appl. Phys. Express 12 070101
Google Scholar
[40] Heyroth F, Hauser C, Trempler P, Geyer P, Syrowatka F, Dreyer R, Ebbinghaus S G, Woltersdorf G, Schmidt G 2019 Phys. Rev. Appl. 12 054031
Google Scholar
[41] Vidal G, Werner R F 2002 Phys. Rev. A 65 032314
Google Scholar
[42] Plenio M B 2005 Phys. Rev. Lett. 95 090503
Google Scholar
[43] Tan H T, Deng W W, Li G X 2017 Phys. Rev. A 95 053842
Google Scholar
[44] Luo Y X, Cong L J, Zheng Z G, Liu H Y, Ming Y, Yang R C 2023 Opt. Express 31 34764
Google Scholar
DownLoad:
Metrics
- Abstract views: 430
- PDF Downloads: 10
- Cited By: 0
