Tunable Casimir equilibria in dual-liquid system

ZHOU Shuai; LIU Kaipeng; DAI Shiwei; GE Lixin

doi:10.7498/aps.74.20241126

Abstract

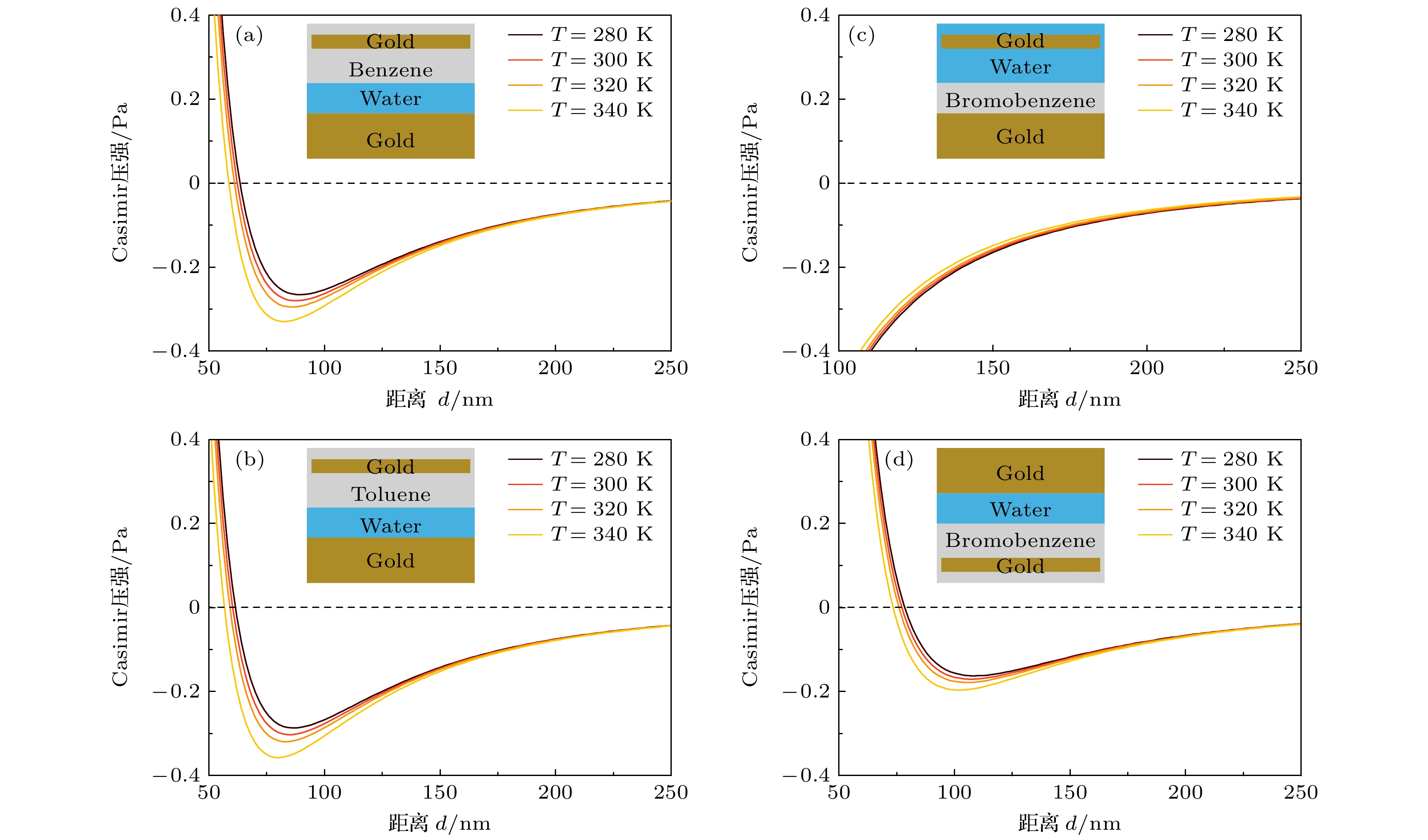
The Casimir effect, a macroscopic manifestation of quantum phenomena, arises from zero-point energy and thermal fluctuations. When two objects are brought into close proximity, the Casimir effect manifests as a repulsive force, while at greater separations, it transforms into an attractive force. There exists a specific distance at which the Casimir force vanishes, which is referred to as the stable Casimir equilibrium. Stable Casimir equilibrium arises from the curve minimum value of the Casimir energy, which can create spatial trapping. The manipulation of stable Casimir equilibrium provides promising applications in fields such as tunable optical resonators and self-assembly. This work presents a scheme for achieving tunable Casimir equilibrium in a dual-liquid system. The system comprises a multilayered stratified structure with a gold substrate. Above the gold substrate, a stratified liquid system is formed due to the immiscibility between organic solutions and water. The lower-density solution is at the top, while the higher-density solution is at the bottom. Our results suggest that a stable Casimir equilibrium for a suspended gold nanoplate can be realized, when the suspended gold nanoplate is immersed in organic solution of toluene or benzene. Moreover, the height of the suspended gold nanoplate, determined by the stable Casimir equilibrium, can be precisely tuned by changing the thickness of the water layer. The effects of finite temperature and ionic concentration on the Casimir equilibria are also analyzed in this work. The results suggest that the separation height of Casimir equilibrium decreases with the increase of temperature. Interestingly, when the Debye shielding length is comparable to or smaller than the separation length, the ion concentration in water significantly affects the Casimir pressure allowing for extensive modulations of Casimir equilibrium. This work opens up a new avenue for adjusting Casimir equilibrium and has important applications in “quantum trapping” of micro-nano particles.

Keywords:

Authors and contacts

1.
School of Physics and Electronic Engineering, Xinyang Normal University, Xinyang 464000, China
2.
Department of Basic Courses, Zhengzhou University of Science and Technology, Zhengzhou 450064, China
3.
College of Mathematics and Physics, Chengdu University of Technology, Chengdu 610059, China

Corresponding author: GE Lixin, lixinge@hotmail.com

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 11804288), the Natural Science Foundation of Henan Province, China (Grant No. 232300420120), and the Key Scientific Research Project of Higher Education Institutions of Henan Province, China (Grant No. 20231205164502999).

FullText HTML

References

[1]	Casimir H B 1948 Proceedings of the Koninklijke Nederlandse Akademie van Wetenschappen (Vol. 51) p793
[2]	苗兵 2020 69 080505 Google Scholar Miao B 2020 Acta Phys. Sin 69 080505 Google Scholar
[3]	Klimchitskaya G, Mohideen U, Mostepanenko V 2009 Rev. Mod. Phys. 81 1827 Google Scholar
[4]	Lamoreaux S K 1997 Phys. Rev. Lett. 78 5 Google Scholar
[5]	Mohideen U, Roy A 1998 Phys. Rev. Lett. 81 4549 Google Scholar
[6]	Garrett J L, Somers D A, Munday J N 2018 Phys. Rev. Lett. 120 040401 Google Scholar
[7]	Woods L, Dalvit D A R, Tkatchenko A, Rodriguez-Lopez P, Rodriguez A W, Podgornik R 2016 Rev. Mod. Phys. 88 045003 Google Scholar
[8]	Rosa F S, Dalvit D A, Milonni P W 2008 Phys. Rev. Lett. 100 183602 Google Scholar
[9]	Song G, Zeng R, Al-Amri M, Xu J P, Zhu C J, He P F, Yang Y P 2018 Opt. Express 26 34461 Google Scholar
[10]	Grushin A G, Cortijo A 2011 Phys. Rev. Lett. 106 020403 Google Scholar
[11]	Nie W J, Zeng R, Lan Y H, Zhu S Y 2013 Phys. Rev. B 88 085421 Google Scholar
[12]	Chen L, Wan S L 2012 Phys. Rev. B 85 115102 Google Scholar
[13]	Zhang Y C, Zhang H, Wang X X, Wang Y H, Liu Y C, Li S, Zhang T Y, Fan C, Zeng C G 2024 Nat. Phys. 20 1282 Google Scholar
[14]	Jiang Q D, Wilczek F 2019 Phys. Rev. B 99 125403 Google Scholar
[15]	Hu Y, Wu X H, Liu H T, Ge W X, Zhang J H, Huang X Q 2024 ACS Photonics 11 1998 Google Scholar
[16]	Gong T, Corrado M R, Mahbub A R, Shelden C, Munday J N 2020 Nanophotonics 10 523 Google Scholar
[17]	Bao F L, Shi K Z, Cao G J, Evans J S, He S L 2018 Phys. Rev. Lett. 121 130401 Google Scholar
[18]	Chen L, Chang K 2020 Phys. Rev. Lett. 125 047402 Google Scholar
[19]	Wang T B, Zhou Y, Mu H Q, Shehzad K, Zhang D J, Liu W X, Yu T B, Liao Q H 2022 Nanotechnology 33 245001 Google Scholar
[20]	Yu T, You W, Wang T B, Yu T B, Liao Q H 2023 Results Phys. 52 106902 Google Scholar
[21]	Wang L Q, Wu Y, Chen A X, Nie W J 2022 Results Phys. 41 105939 Google Scholar
[22]	Wang Y P, Zhang Z C, Yu Y F, Zhang Z M 2019 Chin. Phys. B 28 014202 Google Scholar
[23]	Esteso V, Frustaglia D, Carretero-Palacios S, Míguez H 2024 Adv. Phys. Res. 3 2300065 Google Scholar
[24]	Rodriguez A W, McCauley A P, Woolf D, Capasso F, Joannopoulos J D, Johnson S G 2010 Phys. Rev. Lett. 104 160402 Google Scholar
[25]	Ye Y Q, Hu Q, Zhao Q, Meng Y G 2018 Phys. Rev. B 98 035410 Google Scholar
[26]	Liu X L, Zhang Z M 2016 Phys. Rev. Appl. 5 034004 Google Scholar
[27]	Rahi S J, Kardar M, Emig T 2010 Phys. Rev. Lett. 105 070404 Google Scholar
[28]	Rong J N, Chen L, Chang K 2021 Chin. Phys. Lett. 38 084501 Google Scholar
[29]	Zhao R K, Li L, Yang S, Bao W, Xia Y, Ashby P, Wang Y, Zhang X 2019 Science 364 984 Google Scholar
[30]	Ge L X, Shi X, Xu Z J, Gong K 2020 Phys. Rev. B 101 104107 Google Scholar
[31]	Ge L X, Shi X, Liu L, Gong K 2020 Phys. Rev. B 102 075428 Google Scholar
[32]	Toyama H, Ikeda T, Iizuka H 2023 Phys. Rev. B 108 245402 Google Scholar
[33]	Ge L X, Liu K P, Gong K, Podgornik R 2024 Phys. Rev. Appl. 21 044040 Google Scholar
[34]	Esteso V, Carretero-Palacios S, Míguez H 2019 J. Phys. Chem. Lett. 10 5856 Google Scholar
[35]	Ge L X, Li B Z, Luo H, Gong K 2023 Phys. Rev. A 108 062814 Google Scholar
[36]	Munkhbat B, Canales A, Kucukoz B, Baranov D G, Shegai T O 2021 Nature 597 214 Google Scholar
[37]	Krasnov M, Mazitov A, Orekhov N, Baranov D G 2024 Phys. Rev. B 109 195411 Google Scholar
[38]	Küçüköz B, Kotov O V, Canales A, Polyakov A Y, Agrawal A V, Antosiewicz T J, Shegai T O 2024 Sci. Adv. 10 1825 Google Scholar
[39]	Lifshitz E 1956 Sov. Phys. JETP-USSR 2 73
[40]	Van Zwol P, Palasantzas G 2010 Phys. Rev. A 81 062502 Google Scholar
[41]	Rodriguez A W, Woolf D, McCauley A P, Capasso F, Joannopoulos J D, Johnson S G 2010 Phys. Rev. Lett. 105 060401 Google Scholar
[42]	Munday J N, Capasso F, Parsegian V A 2009 Nature 457 170 Google Scholar
[43]	Dou M F, Lou F, Boström M, Brevik I, Persson C 2014 Phys. Rev. B 89 201407 Google Scholar
[44]	Sehmi H S, Langbein W, Muljarov E A 2017 Phys. Rev. B 95 115444 Google Scholar
[45]	Fiedler J, Bostrom M, Persson C, Brevik I, Corkery R, Buhmann S Y, Parsons D F 2020 J Phys. Chem. B 124 3103 Google Scholar
[46]	Wang Y F, Narayanan S R, Wu W 2017 ACS Nano 11 8421 Google Scholar

Cited By

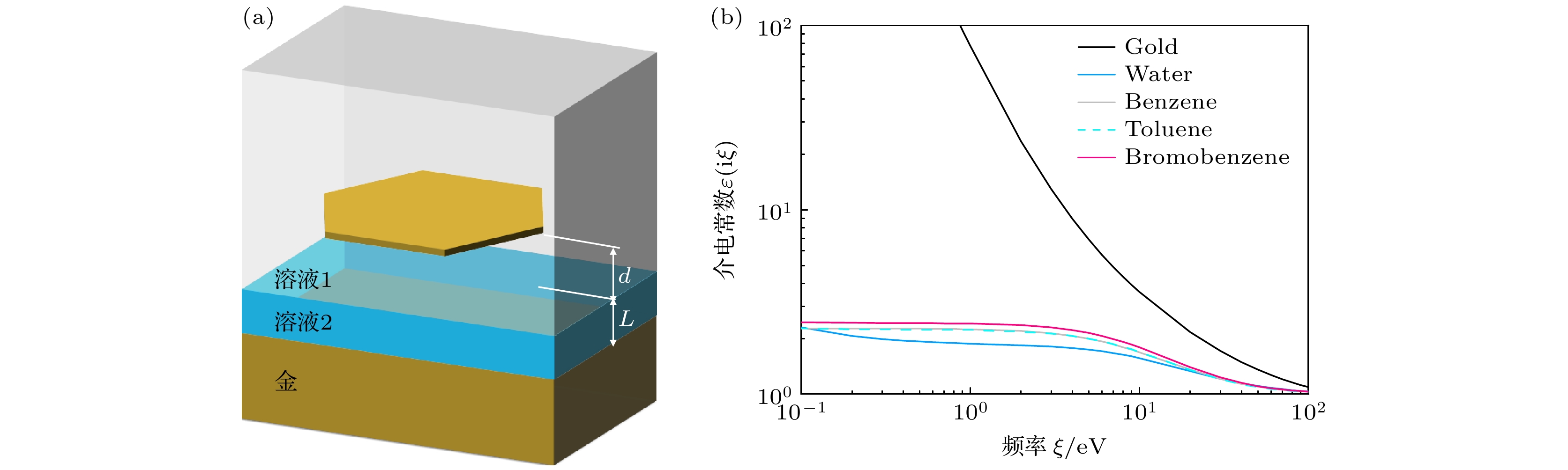
图 1 (a) 两不相溶液体中的Casimir悬浮示意图. 金纳米薄片距离液体分层界面为d. 溶液1的密度小于溶液2的密度; 而溶液2作为隔离层, 紧邻金属衬底且具有可调控厚度L. (b) 金、水、溴苯、苯和甲苯的介电常数随虚频率变化曲线

Figure 1. (a) Schematic diagram of Casimir suspension in two immiscible liquids. The distance between the gold nanoplate and the stratified liquid interface is d. The density of solution 1 is less than that of solution 2, while solution 2 acts as an isolation layer, close to the metal substrate and has a controllable thickness L. (b) Dielectric constants of gold, water, bromobenzene, benzene, and toluene change with the imaginary frequency.

DownLoad: Full-Size Img PowerPoint

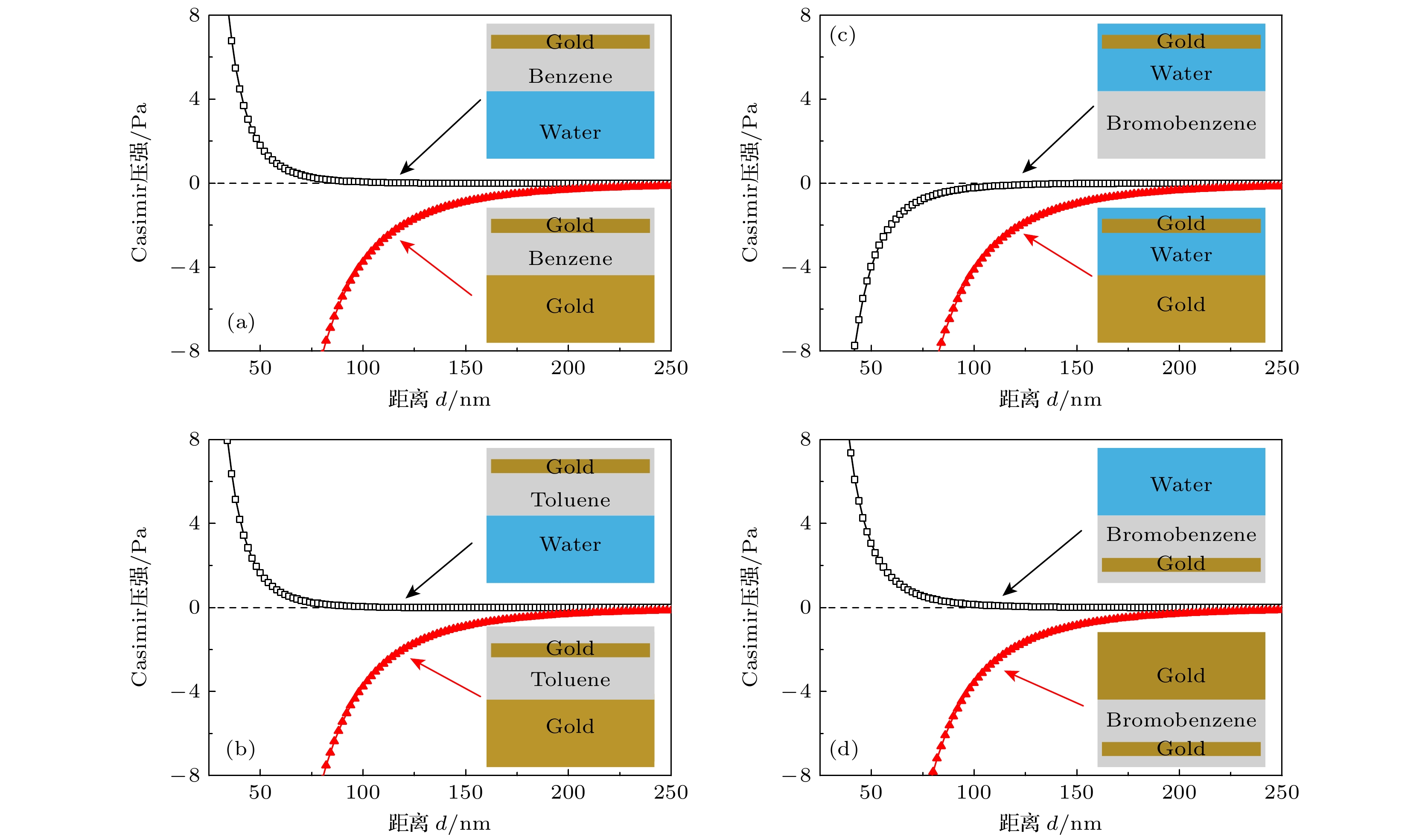
图 2 悬浮金纳米薄片受到的Casimir压强　(a) 苯@水; (b) 甲苯@水; (c) 溴苯@水; (d)倒置的溴苯@水. 压力的正号表示排斥力, 负号表示吸引力. 金纳米薄片的厚度L₀ = 40 nm, 系统温度T = 300 K

Figure 2. Casimir pressure acts on suspended metal nanoplates: (a) Benzene@water; (b) toluene@water; (c) bromobenzene@water; (d) tnverted bromobenzene@water. The positive sign of the pressure indicates repulsion, while the negative sign indicates attraction. Thickness of gold nanoplate L₀ = 40 nm, and the temperature of system T = 300 K.

DownLoad: Full-Size Img PowerPoint

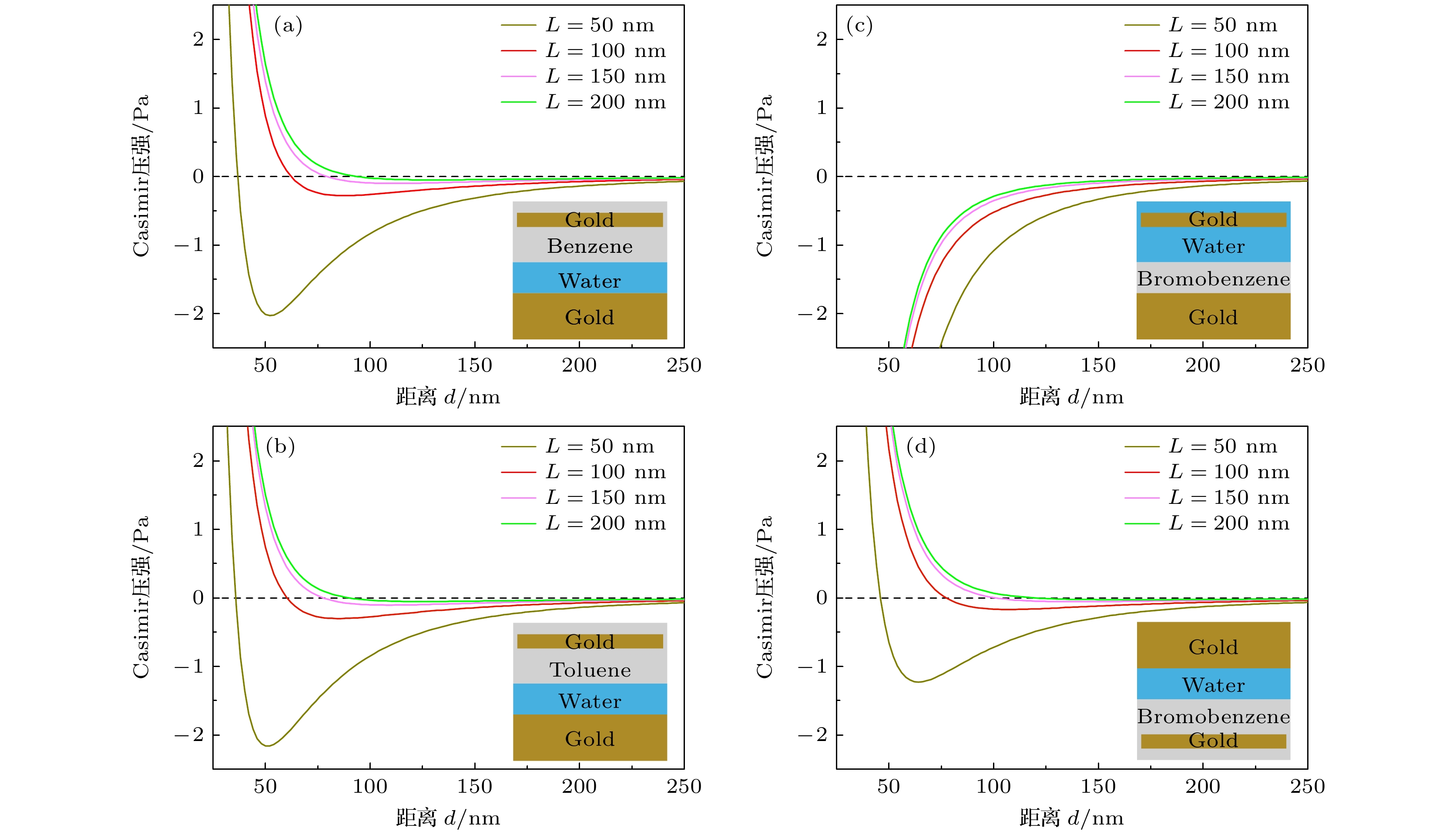
图 3 液体隔离层在有限厚度下, 双液体系统中金纳米薄片受到的Casimir压强　(a) 苯@水; (b) 甲苯@水; (c) 溴苯@水; (d) 倒置的溴苯@水

Figure 3. Casimir pressure acts on gold nanoplates in dual-liquid systems, where the layer thickness of liquid isolation is finite: (a) Benzene@water; (b) toluene@water; (c) bromobenzene@water; (d) inverted bromobenzene@water.

DownLoad: Full-Size Img PowerPoint

图 4 不同温度下的Casimir压强　(a) 苯@水; (b) 甲苯@水; (c) 溴苯@水; (d)倒置的溴苯@水. 液体隔离层L的厚度设定为100 nm

Figure 4. Casimir pressure at different temperatures: (a) Benzene@water; (b) toluene@water; (c) bromobenzene@water; (d) inverted bromobenzene@water. Thickness of the liquid isolation layer L is set to 100 nm.

DownLoad: Full-Size Img PowerPoint

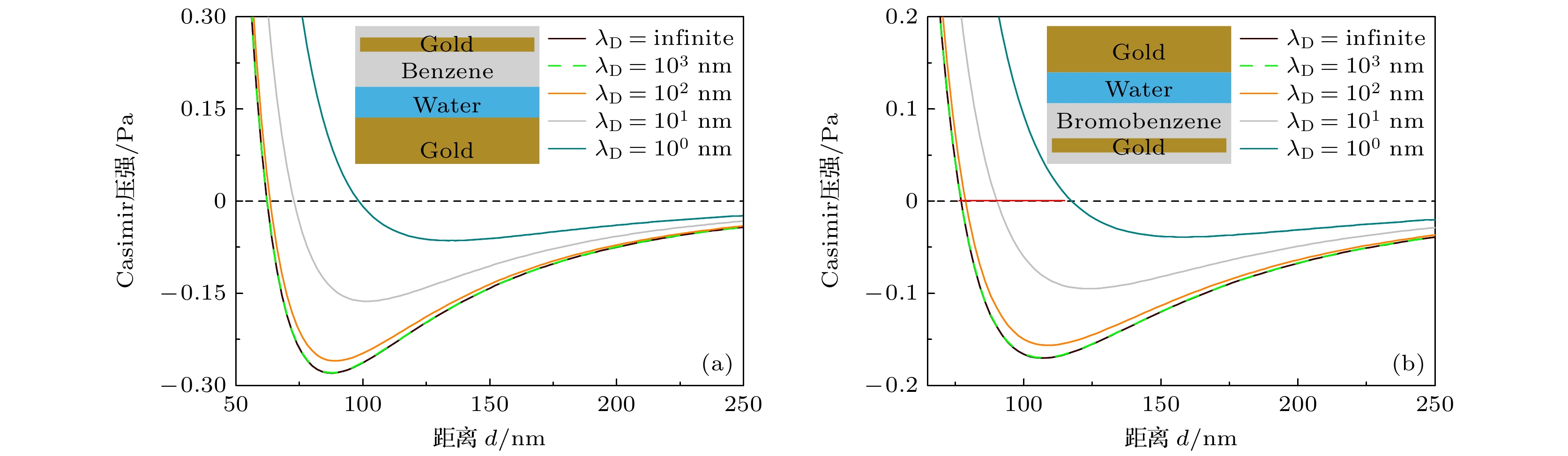
图 5 不同德拜屏蔽长度下, Casimir压强随距离d的变化曲线　(a) 苯@水; (b) 倒置的溴苯@水. 液体隔离层L的厚度设定为100 nm, 系统温度T = 300 K

Figure 5. Casimir pressure changes with separation d under different Debye screening lengths: (a) Benzene@water; (b) inverted bromobenzene@water. Thickness of the liquid isolation layer L is set to 100 nm, and the system temperature T = 300 K.

DownLoad: Full-Size Img PowerPoint

DownLoad: Full-Size Img PowerPoint

map

[1]	Casimir H B 1948 Proceedings of the Koninklijke Nederlandse Akademie van Wetenschappen (Vol. 51) p793
[2]	苗兵 2020 69 080505 Google Scholar Miao B 2020 Acta Phys. Sin 69 080505 Google Scholar
[3]	Klimchitskaya G, Mohideen U, Mostepanenko V 2009 Rev. Mod. Phys. 81 1827 Google Scholar
[4]	Lamoreaux S K 1997 Phys. Rev. Lett. 78 5 Google Scholar
[5]	Mohideen U, Roy A 1998 Phys. Rev. Lett. 81 4549 Google Scholar
[6]	Garrett J L, Somers D A, Munday J N 2018 Phys. Rev. Lett. 120 040401 Google Scholar
[7]	Woods L, Dalvit D A R, Tkatchenko A, Rodriguez-Lopez P, Rodriguez A W, Podgornik R 2016 Rev. Mod. Phys. 88 045003 Google Scholar
[8]	Rosa F S, Dalvit D A, Milonni P W 2008 Phys. Rev. Lett. 100 183602 Google Scholar
[9]	Song G, Zeng R, Al-Amri M, Xu J P, Zhu C J, He P F, Yang Y P 2018 Opt. Express 26 34461 Google Scholar
[10]	Grushin A G, Cortijo A 2011 Phys. Rev. Lett. 106 020403 Google Scholar
[11]	Nie W J, Zeng R, Lan Y H, Zhu S Y 2013 Phys. Rev. B 88 085421 Google Scholar
[12]	Chen L, Wan S L 2012 Phys. Rev. B 85 115102 Google Scholar
[13]	Zhang Y C, Zhang H, Wang X X, Wang Y H, Liu Y C, Li S, Zhang T Y, Fan C, Zeng C G 2024 Nat. Phys. 20 1282 Google Scholar
[14]	Jiang Q D, Wilczek F 2019 Phys. Rev. B 99 125403 Google Scholar
[15]	Hu Y, Wu X H, Liu H T, Ge W X, Zhang J H, Huang X Q 2024 ACS Photonics 11 1998 Google Scholar
[16]	Gong T, Corrado M R, Mahbub A R, Shelden C, Munday J N 2020 Nanophotonics 10 523 Google Scholar
[17]	Bao F L, Shi K Z, Cao G J, Evans J S, He S L 2018 Phys. Rev. Lett. 121 130401 Google Scholar
[18]	Chen L, Chang K 2020 Phys. Rev. Lett. 125 047402 Google Scholar
[19]	Wang T B, Zhou Y, Mu H Q, Shehzad K, Zhang D J, Liu W X, Yu T B, Liao Q H 2022 Nanotechnology 33 245001 Google Scholar
[20]	Yu T, You W, Wang T B, Yu T B, Liao Q H 2023 Results Phys. 52 106902 Google Scholar
[21]	Wang L Q, Wu Y, Chen A X, Nie W J 2022 Results Phys. 41 105939 Google Scholar
[22]	Wang Y P, Zhang Z C, Yu Y F, Zhang Z M 2019 Chin. Phys. B 28 014202 Google Scholar
[23]	Esteso V, Frustaglia D, Carretero-Palacios S, Míguez H 2024 Adv. Phys. Res. 3 2300065 Google Scholar
[24]	Rodriguez A W, McCauley A P, Woolf D, Capasso F, Joannopoulos J D, Johnson S G 2010 Phys. Rev. Lett. 104 160402 Google Scholar
[25]	Ye Y Q, Hu Q, Zhao Q, Meng Y G 2018 Phys. Rev. B 98 035410 Google Scholar
[26]	Liu X L, Zhang Z M 2016 Phys. Rev. Appl. 5 034004 Google Scholar
[27]	Rahi S J, Kardar M, Emig T 2010 Phys. Rev. Lett. 105 070404 Google Scholar
[28]	Rong J N, Chen L, Chang K 2021 Chin. Phys. Lett. 38 084501 Google Scholar
[29]	Zhao R K, Li L, Yang S, Bao W, Xia Y, Ashby P, Wang Y, Zhang X 2019 Science 364 984 Google Scholar
[30]	Ge L X, Shi X, Xu Z J, Gong K 2020 Phys. Rev. B 101 104107 Google Scholar
[31]	Ge L X, Shi X, Liu L, Gong K 2020 Phys. Rev. B 102 075428 Google Scholar
[32]	Toyama H, Ikeda T, Iizuka H 2023 Phys. Rev. B 108 245402 Google Scholar
[33]	Ge L X, Liu K P, Gong K, Podgornik R 2024 Phys. Rev. Appl. 21 044040 Google Scholar
[34]	Esteso V, Carretero-Palacios S, Míguez H 2019 J. Phys. Chem. Lett. 10 5856 Google Scholar
[35]	Ge L X, Li B Z, Luo H, Gong K 2023 Phys. Rev. A 108 062814 Google Scholar
[36]	Munkhbat B, Canales A, Kucukoz B, Baranov D G, Shegai T O 2021 Nature 597 214 Google Scholar
[37]	Krasnov M, Mazitov A, Orekhov N, Baranov D G 2024 Phys. Rev. B 109 195411 Google Scholar
[38]	Küçüköz B, Kotov O V, Canales A, Polyakov A Y, Agrawal A V, Antosiewicz T J, Shegai T O 2024 Sci. Adv. 10 1825 Google Scholar
[39]	Lifshitz E 1956 Sov. Phys. JETP-USSR 2 73
[40]	Van Zwol P, Palasantzas G 2010 Phys. Rev. A 81 062502 Google Scholar
[41]	Rodriguez A W, Woolf D, McCauley A P, Capasso F, Joannopoulos J D, Johnson S G 2010 Phys. Rev. Lett. 105 060401 Google Scholar
[42]	Munday J N, Capasso F, Parsegian V A 2009 Nature 457 170 Google Scholar
[43]	Dou M F, Lou F, Boström M, Brevik I, Persson C 2014 Phys. Rev. B 89 201407 Google Scholar
[44]	Sehmi H S, Langbein W, Muljarov E A 2017 Phys. Rev. B 95 115444 Google Scholar
[45]	Fiedler J, Bostrom M, Persson C, Brevik I, Corkery R, Buhmann S Y, Parsons D F 2020 J Phys. Chem. B 124 3103 Google Scholar
[46]	Wang Y F, Narayanan S R, Wu W 2017 ACS Nano 11 8421 Google Scholar

[1]	Liu Xue, Wang De-Hua. Internal disorder of hydrogenic-like atom trapped in dielectric spherical microcavity. Acta Physica Sinica, 2023, 72(10): 100301. doi: 10.7498/aps.72.20222413
[2]	Li Liang-Liang, Meng Fan-Wei, Zou Kun, Huang Yao, Peng Yi-Tian. Friction properties of suspended graphene. Acta Physica Sinica, 2021, 70(8): 086801. doi: 10.7498/aps.70.20201796
[3]	Wei Yan-Ju, Zhang Jie, Deng Sheng-Cai, Zhang Ya-Jie, Yang Ya-Jing, Liu Sheng-Hua, Chen Hao. Phenomenon study on heat induced atomization of acoustic levitated methanol droplet. Acta Physica Sinica, 2020, 69(18): 184702. doi: 10.7498/aps.69.20200562
[4]	You Jia-Xue, Wang Jin-Cheng, Wang Li-Lin, Wang Zhi-Jun, Li Jun-Jie, Lin Xin. Recent progress of solidification of suspensions. Acta Physica Sinica, 2019, 68(1): 018101. doi: 10.7498/aps.68.20181645
[5]	Li Jin-Qing, Luo Yun-Rong, Hai Wen-Hua. Quantum damping motion of a single trapped ion. Acta Physica Sinica, 2017, 66(23): 233701. doi: 10.7498/aps.66.233701
[6]	Qiao Xiao-Fen, Li Xiao-Li, Liu He-Nan, Shi Wei, Liu Xue-Lu, Wu Jiang-Bin, Tan Ping-Heng. Periodic oscillation in the reflection and photoluminescence spectra of suspended two-dimensional crystal flakes. Acta Physica Sinica, 2016, 65(13): 136801. doi: 10.7498/aps.65.136801
[7]	Li Ming-Hua, Yuan Zhen-Zhou, Xu Yan, Tian Jun-Fang. Randomness analysis of lane formation in pedestrian counter flow based on improved lattice gas model. Acta Physica Sinica, 2015, 64(1): 018903. doi: 10.7498/aps.64.018903
[8]	Xie Wen-Jun, Teng Peng-Fei. Study of acoustic levitation by lattice Boltzmann method. Acta Physica Sinica, 2014, 63(16): 164301. doi: 10.7498/aps.63.164301
[9]	Wu Li-Jun, Han Yu, Gong Wei-Jiang, Tan Tian-Ya. Decoupled states and anti-resonance in the Aharonov-Bohm interferometer with embodied quantum-dot ring. Acta Physica Sinica, 2011, 60(10): 107303. doi: 10.7498/aps.60.107303
[10]	Liu Wang-Yun, Bi Si-Wen, Dou Xi-Bo. Evolution properties of the field quantum entropy in the nonlinear Jaynes-Cummings model of a trapped ion. Acta Physica Sinica, 2010, 59(3): 1780-1785. doi: 10.7498/aps.59.1780
[11]	Zhang Miao, Jia Huan-Yu, Ji Xiao-Hui, Si Kun, Wei Lian-Fu. Generation of squeezed quantum states of a single trapped cold ion. Acta Physica Sinica, 2008, 57(12): 7650-7657. doi: 10.7498/aps.57.7650
[12]	Liu Su-Hua, Tang Jia-Shi. Anti-control of Hopf bifurcation at zero equilibrium of 4D Qi system. Acta Physica Sinica, 2008, 57(10): 6162-6168. doi: 10.7498/aps.57.6162
[13]	Cai Cheng-Yu, Zhou Wang-Min. The strain distribution and equilibrium morphology of Ge/Si semiconductor quantum dot. Acta Physica Sinica, 2007, 56(8): 4841-4846. doi: 10.7498/aps.56.4841
[14]	Chen Xiong-Wen, He Da-Jiang, Wu Shao-Quan, Song Ke-Hui. Magnetic polarization currents in parallel-coupled double-quantum-dot devices. Acta Physica Sinica, 2006, 55(8): 4287-4291. doi: 10.7498/aps.55.4287
[15]	Wu Yun-Wen, Hai Wen-Hua. Exact quantum motions of two ions in a two-dimensional paul trap. Acta Physica Sinica, 2006, 55(11): 5721-5727. doi: 10.7498/aps.55.5721
[16]	Ma Yun, Fu Li-Bin, Yang Zhi-An, Liu Jie. Dynamical phase changes of the self-trapping of Bose-Einstein condensates and its characteristic of entanglement. Acta Physica Sinica, 2006, 55(11): 5623-5628. doi: 10.7498/aps.55.5623
[17]	Zhang Lin, Li En-Pu, Feng Wei, Hong Zhen-Yu, Xie Wen-Jun, Ma Yang-Hua. A study of acoustic levitation process based on laser holographic interferometry. Acta Physica Sinica, 2005, 54(5): 2038-2042. doi: 10.7498/aps.54.2038
[18]	Ma Wei-Zeng, Ji Cheng-Chang, Li Jian-Guo, Xu Zhen-Ming. Temperature character of electromagnetic levitation melting. Acta Physica Sinica, 2003, 52(4): 834-839. doi: 10.7498/aps.52.834
[19]	LONG DE-SHUN, NING XI-JING. POPULATION TRAPPING PHENOMENA IN A TWO-LEVEL SYSTEM. Acta Physica Sinica, 2001, 50(12): 2335-2340. doi: 10.7498/aps.50.2335
[20]	JIA WEI-YI, XIONG JI-WU. ENHANCEMENT OF FLUORESCENCE INDUCED BY SCATTERING FROM SUSPENDED PARTICLES. Acta Physica Sinica, 1983, 32(11): 1471-1473. doi: 10.7498/aps.32.1471

Catalog

Vol. 74, Issue 1 - 2025-01-05

Metrics

Abstract views: 953
PDF Downloads: 37
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板