Mechanism of boundary bubble drag reduction of Couette flow in nano-confined domain

Zhang Peng; Zhang Yan-Ru; Zhang Fu-Jian; Liu Zhen; Zhang Zhong-Qiang

doi:10.7498/aps.73.20240474

Abstract

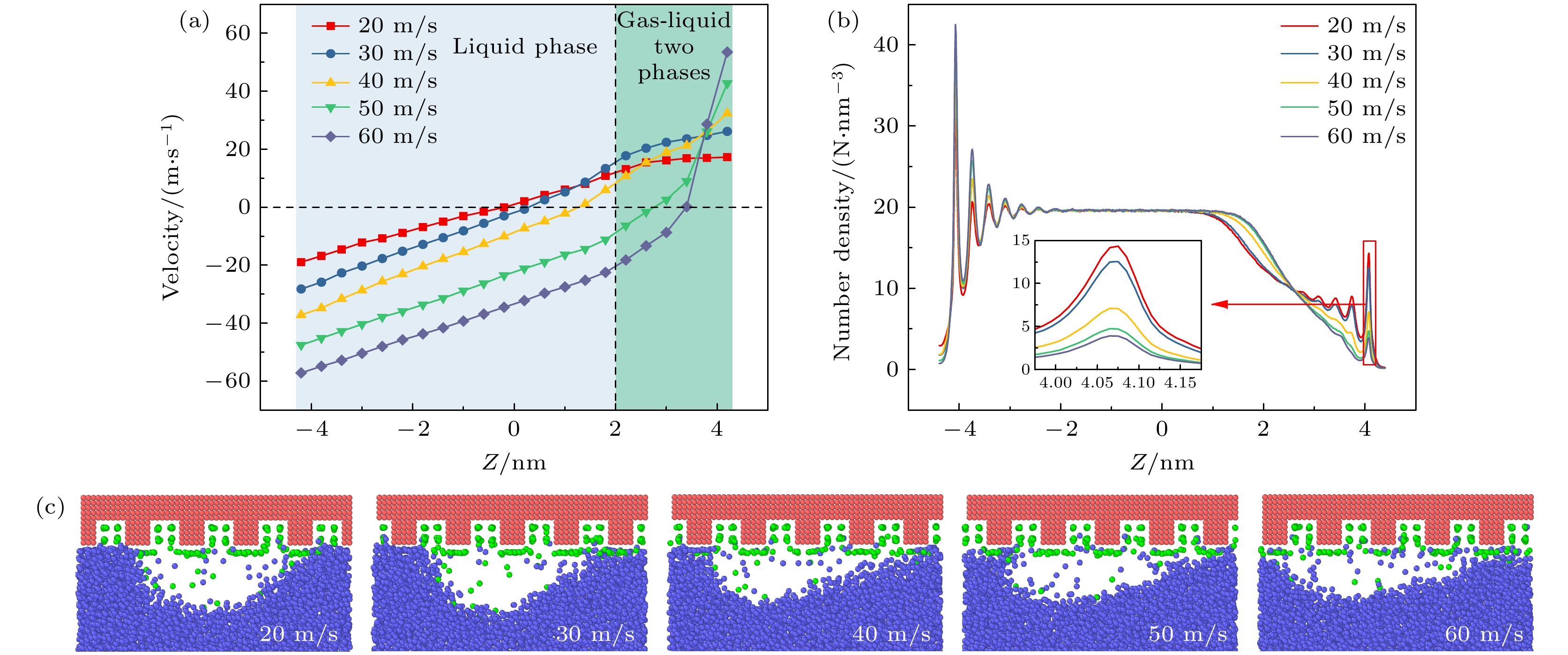
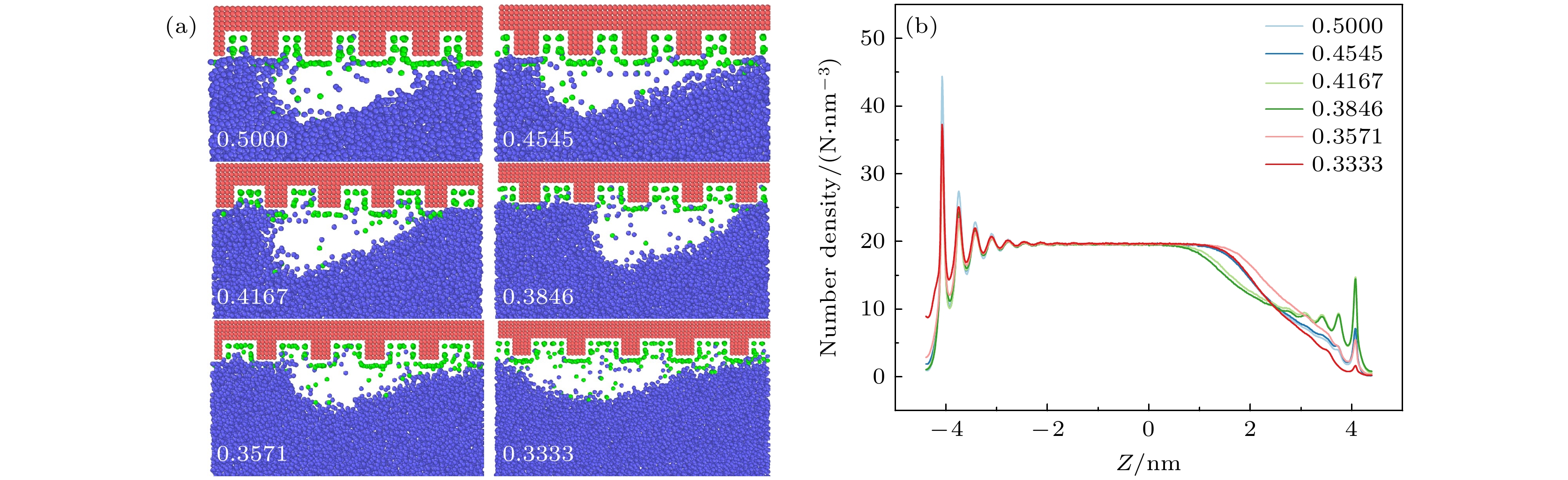
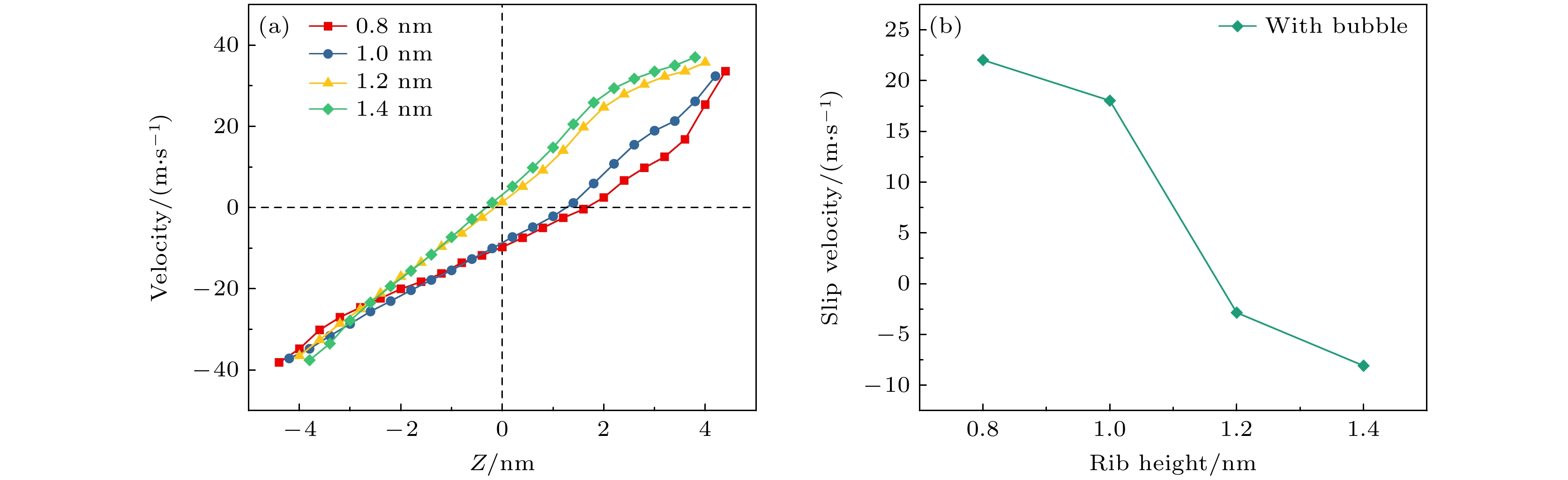
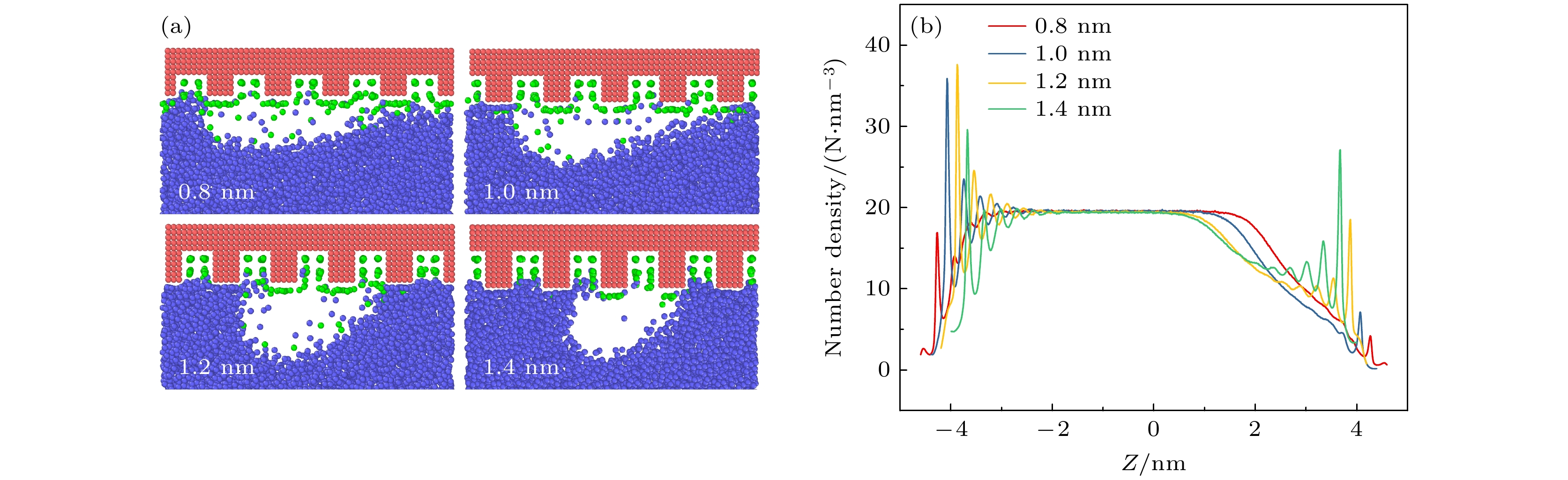
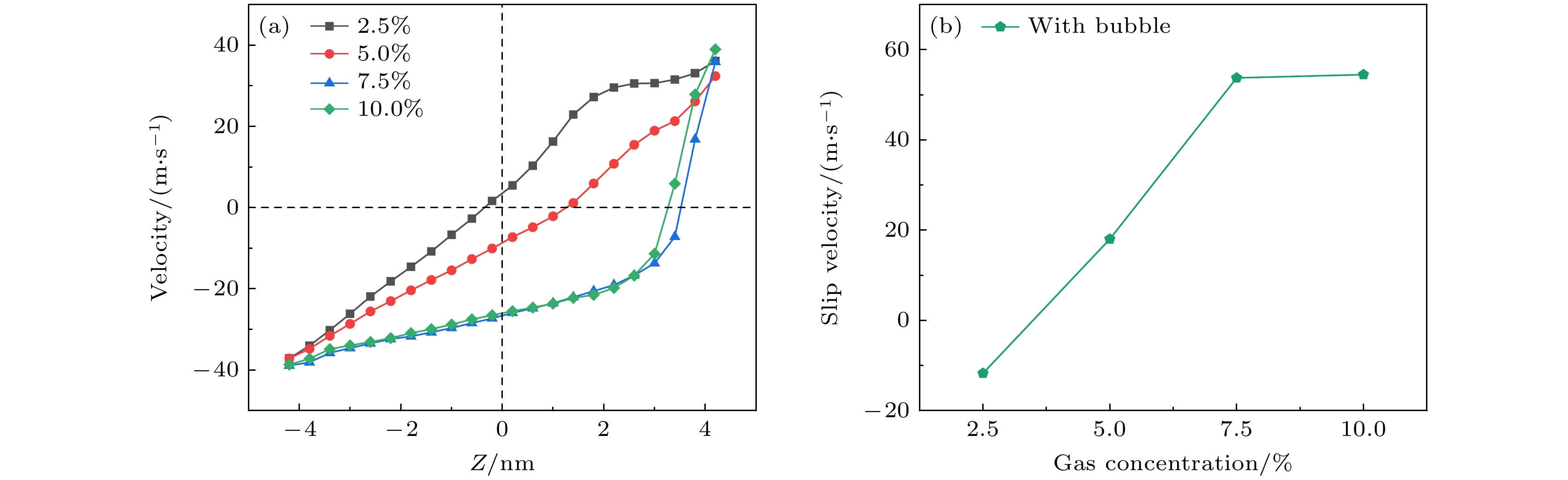
Bubble drag reduction technology is of great significance in improving the propulsion efficiency of underwater vehicle and reducing the comprehensive energy consumption during navigation. Bubble drag reduction is a highly effective method of reducing the frictional resistance encountered by large ships and underwater vehicles during navigation. It exhibits excellent stability in drag reduction, and has advantages such as environmental friendliness, adaptability to various flow environments, and suitability for all underwater components of ships. Therefore, it is greatly significant to conduct in-depth research on bubble drag reduction and its underlying mechanism. In this work, the flow characteristics and the boundary bubble drag reduction mechanism of gas-liquid Couette flow in parallel wall nanochannels are studied by molecular dynamics method, and the influences of surface wettability, wall roughness, and gas concentration on boundary slip velocity and bubble drag reduction effect are analyzed. The results indicate that the bubble drag reduction effect is enhanced with the increase of boundary slip velocity. In the gas-liquid two-phase flow region, with the increase of shear velocity, the lateral deformation of boundary adsorbed bubble and boundary slip velocity increase, thus enhancing the bubble drag reduction effect. The increase of solid-gas interaction strength and gas concentration can lead to the enrichment of gas atoms near the wall, improve the bubble spreading characteristics on the wall, and thus increase the slip velocity of the solid-liquid interface. The wall roughness can change the spreading characteristics of bubble, affect the boundary slip velocity, and then change the drag reduction effect of the fluid-solid interface. As the rib height increases, gas atoms accumulate in the grooves between ribs and the adsorption quantity of gas atoms on the upper surface of the rib decreases, which leads to the decrease of the boundary slip velocity of the solid-liquid interface and ultimately reduces the drag reduction effect. The research results will provide important theoretical guidance for implementing the boundary drag reduction technology in large ships and underwater vehicles.

Keywords:

Authors and contacts

1.
School of Mechanical Engineering, Jiangsu University, Zhenjiang 212013, China
2.
School of Ship and Ocean Engineering, Jiangsu University of Science and Technology, Zhenjiang 212003, China
3.
State Key Laboratory of Structural Analysis of Industrial Equipment, Dalian University of Technology, Dalian 116024, China

Corresponding author: Liu Zhen, liuzhen@just.edu.cn ; Zhang Zhong-Qiang, zhangzq@ujs.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12272151, 52005222, 92248301).

FullText HTML : translate this paragraph

References

[1]	Sindagi S, Vijayakumar R 2020 Ships Offshore Struct. 16 968 Google Scholar
[2]	Fu Y F, Yuan C Q, Bai X Q 2017 Biosurf. Biotribol. 3 11 Google Scholar
[3]	Gu Y Q, Zhao G, Zheng J X, Li Z Y, Liu W B, Muhammad F K 2014 Ocean Eng. 81 50 Google Scholar
[4]	李芳, 赵刚, 刘维新, 张殊, 毕红时 2015 64 034703 Google Scholar Li F, Zhao G, Liu W X, Zhang S, Bi H S 2015 Acta Phys. Sin. 64 034703 Google Scholar
[5]	康晓宣, 胡建新, 林昭武, 潘定一 2023 力学学报 55 1087 Google Scholar Kang X X, Hu J X, Lin Z W, Pan D Y 2023 Acta Mech. Sinica. 55 1087 Google Scholar
[6]	史同雨 2020 硕士学位论文(大连: 大连海事大学) Shi T Y 2020 M. S. Thesis (Dalian: Dalian Maritime University
[7]	Wang H W, Wang K Y, Liu G H 2022 Ocean Eng. 258 111833 Google Scholar
[8]	赵超, 吕明利, 贾文广 2022 船舶工程 44 69 Google Scholar Zhao C, Lyu M L, Jia W G 2022 Ship Eng. 44 69 Google Scholar
[9]	詹杰民, 陆尚平, 李熠华, 李雨田, 胡文清 2023 海洋工程 41 1 Google Scholar Zhan J M, Lu S P, Li Y H, Li Y T, Hu W Q 2023 Ocean Eng. 41 1 Google Scholar
[10]	张晨远, 张智嘉, 丛巍巍, 魏浩, 张松松 2023 化学通报 86 863 Google Scholar Zhang C Y, Zhang Z J, Cong W W, Wei H, Zhang S S 2023 Chem. Bull. 86 863 Google Scholar
[11]	Moaven K, Rad M, Taeibi-Rahni M 2013 Exp. Therm. Fluid. Sci. 51 239 Google Scholar
[12]	Gao J, Zhang K, Li H, Lang C, Zhang L X 2023 Prog. Org. Coat. 183 107769 Google Scholar
[13]	Chen H W, Zhang X, Che D, Zhang D Y, Li X, Li Y Y 2014 Adv. Mech. Eng. 2014 425701 Google Scholar
[14]	Luo Y, Zhang D, Liu Y, Li Y, Ng E Y K 2015 J. Mech. Med. Biol. 15 1550084 Google Scholar
[15]	Shen X, Ceccio S L, Perlin M 2006 Exp. Fluids 41 415 Google Scholar
[16]	Zhao X J, Zong Z 2022 Ocean Eng. 251 111032 Google Scholar
[17]	Tanaka T, Oishi Y, Park H J, Tasaka Y, Murai Y, Kawakita C 2023 Ocean Eng. 272 113807 Google Scholar
[18]	Maryami R, Javadpoor M, Farahat S 2016 Heat Mass Transfer 52 2593 Google Scholar
[19]	Bidkar R A, Leblanc L, Kulkarni A J, Bahadur V, Ceccio S L, Perlin M 2014 Phys. Fluids 26 085108 Google Scholar
[20]	Mail M, Moosmann M, Häger P, Barthlott W 2019 Phil. Trans. R. Soc. A 377 20190126 Google Scholar
[21]	Wang F C, Qian J H, Fan J C, Li J C, Xu H Y, Wu H A 2022 Sci. China Phys. Mech. 65 264601 Google Scholar
[22]	石小燕, 曾丹苓, 蔡治勇 2005 热科学与技术 4 195 Google Scholar Shi X Y, Zeng D L, Cai Z Y 2005 J. Therm. Sci. Technol. 4 195 Google Scholar
[23]	Weijs J H, Snoeijer J H, Lohse D 2012 Phys. Rev. Lett. 108 104501 Google Scholar
[24]	Cao B Y, Chen M, Guo Z Y 2006 Phys. Rev. E 74 066311 Google Scholar
[25]	Stukowski A 2010 Model. Simul. Mater. Sci. Eng. 18 015012 Google Scholar
[26]	Zhang Z Q, Liu H L, Liu Z, Zhang Z, Cheng G G, Wang X D, Ding J N 2019 Appl. Surf. Sci. 475 857 Google Scholar
[27]	刘汉伦, 张忠强, 郝茂磊, 程广贵, 丁建宁 2018 气体物理 3 32 Google Scholar Liu H L, Zhang Z Q, Hao M L, Cheng G G, Ding J N 2018 Phys. Gases 3 32 Google Scholar
[28]	Ceccio S L 2010 Annu. Rev. Fluid Mech. 42 183 Google Scholar
[29]	Kitagawa A, Denissenko P, Murai Y 2019 Exp. Therm. Fluid Sci. 104 141 Google Scholar
[30]	邢赫威, 陈占秀, 杨历, 苏瑶, 李源华, 呼和仓 2024 73 094701 Google Scholar Xing H W, Chen Z X, Yang L, Su Y, Li Y H, Huhe C 2024 Acta Phys. Sin. 73 094701 Google Scholar
[31]	Hu H B, Wang D Z, Ren F, Bao L Y, Priezjev N V, Wen J 2018 Int. J. Multiphase Flow 104 166 Google Scholar
[32]	吕鹏宇, 薛亚辉, 段慧玲 2016 力学进展 46 179 Google Scholar Lyu P Y, Xue Y H, Duan H L 2016 Adv. Mech. 46 179 Google Scholar
[33]	García-Magariño A, Lopez-Gavilan P, Sor S, Terroba F 2023 J. Mar. Sci. Eng. 11 1315 Google Scholar
[34]	Tretyakov N, Müller M 2013 Soft Matter 9 3613 Google Scholar
[35]	He Y Y, Fu Y H, Wang H, Yang J 2021 Tribol. Int. 162 107144 Google Scholar
[36]	Tang S N, Zhu Y, Yuan S Q 2023 J. Bionic Eng. 20 2797 Google Scholar
[37]	He Y Y, Fu Y H, Wang H, Yang J 2022 J. Manuf. Process. 75 1089 Google Scholar

Cited By

图 1 (a)两相Couette流流动系统模型示意图; (b)纳米通道和粗糙结构模型图

Figure 1. (a) Schematic diagram of a two-phase Couette flow system model; (b) diagram of the nanochannel and rough structure model

DownLoad: Full-Size Img PowerPoint

图 2 不同剪切速度下的液体原子(a)速度轮廓图和(b)密度分布图; (c)稳态气泡形貌图

Figure 2. Influence of shear velocity on (a) velocity profile and (b) density profile of liquid atoms; (c) steady-state bubble morphology.

DownLoad: Full-Size Img PowerPoint

图 3 (a)边界滑移速度随剪切速度的变化; (b)剪切应力随剪切速度的变化

Figure 3. (a) Plot of boundary slip velocity as a function of shear velocity; (b) plot of shear stress as a function of shear velocity.

DownLoad: Full-Size Img PowerPoint

图 4 (a)不同固-气相互作用强度下的液体原子速度轮廓图; (b)边界滑移速度随固-气相互作用强度的变化

Figure 4. (a) Velocity profiles of liquid atoms at different solid-gas interaction strength; (b) plot of boundary slip velocity as a function of solid-gas interaction strength.

DownLoad: Full-Size Img PowerPoint

图 5 不同固-气相互作用强度下的(a)稳态气泡形貌图和(b)液体原子密度分布图

Figure 5. (a) Steady-state bubble morphology and (b) density profiles of liquid atoms under different solid-gas interaction strength.

DownLoad: Full-Size Img PowerPoint

图 6 剪切速度为40 m/s时, (a)不同粗糙面积分数下的液体原子速度轮廓图; (b)边界滑移速度随粗糙面积分数的变化

Figure 6. When the shear velocity is 40 m/s, (a) velocity profile of liquid atoms at different rough area fraction; (b) plot of boundary slip velocity as a function of rough area fraction.

DownLoad: Full-Size Img PowerPoint

图 7 剪切速度为40 m/s时, 不同粗糙面积分数下的(a)稳态气泡形貌图和(b)液体原子密度分布图

Figure 7. When the shear velocity is 40 m/s, (a) steady-state bubble morphology and (b) density profiles of liquid atoms under different rough area fraction.

DownLoad: Full-Size Img PowerPoint

图 8 剪切速度为40 m/s时, (a)不同肋高下的液体原子速度轮廓图; (b)边界滑移速度随肋高的变化

Figure 8. When the shear velocity is 40 m/s, (a) velocity profiles of liquid atoms at different rib heights; (b) plot of boundary slip velocity as a function of rib height.

DownLoad: Full-Size Img PowerPoint

图 9 剪切速度为40 m/s时, 不同肋高下的(a)稳态气泡形貌图和(b)液体原子密度分布图

Figure 9. When the shear velocity is 40 m/s, (a) steady-state bubble morphology and (b) density profiles of liquid atoms under different rib heights.

DownLoad: Full-Size Img PowerPoint

图 10 剪切速度为40 m/s时, (a)不同气体浓度下的液体原子速度轮廓图; (b)边界滑移速度随气体浓度的变化

Figure 10. When the shear velocity is 40 m/s, (a) velocity profiles of liquid atoms at different gas concentrations; (b) plot of boundary slip velocity as a function of gas concentration.

DownLoad: Full-Size Img PowerPoint

图 11 剪切速度为40 m/s时, 不同气体浓度下的(a)稳态气泡形貌图和(b)液体原子密度分布图

Figure 11. When the shear velocity is 40 m/s, (a) steady-state bubble morphology and (b) density profiles of liquid atoms under different gas concentration.

DownLoad: Full-Size Img PowerPoint

表 1 三相相互作用势能参数

Table 1. Potential energy parameter of three-phase interaction.

两相类型 ε/(kcal·mol^–1) σ/Å

固-液 0.41712825 3.4
固-气 0.5958975 4.2
气-液 0.238359 4.488

DownLoad: CSV

表 2 不同肋间距对应的粗糙面积分数

Table 2. Rough area fraction corresponding to different rib spacing.

肋间距b/nm

1.2 1.4 1.6 1.8 2.0 2.2

粗糙面积
分数 f 0.5 0.4545 0.4167 0.3846 0.3571 0.3333

DownLoad: CSV

map

[1]	Sindagi S, Vijayakumar R 2020 Ships Offshore Struct. 16 968 Google Scholar
[2]	Fu Y F, Yuan C Q, Bai X Q 2017 Biosurf. Biotribol. 3 11 Google Scholar
[3]	Gu Y Q, Zhao G, Zheng J X, Li Z Y, Liu W B, Muhammad F K 2014 Ocean Eng. 81 50 Google Scholar
[4]	李芳, 赵刚, 刘维新, 张殊, 毕红时 2015 64 034703 Google Scholar Li F, Zhao G, Liu W X, Zhang S, Bi H S 2015 Acta Phys. Sin. 64 034703 Google Scholar
[5]	康晓宣, 胡建新, 林昭武, 潘定一 2023 力学学报 55 1087 Google Scholar Kang X X, Hu J X, Lin Z W, Pan D Y 2023 Acta Mech. Sinica. 55 1087 Google Scholar
[6]	史同雨 2020 硕士学位论文(大连: 大连海事大学) Shi T Y 2020 M. S. Thesis (Dalian: Dalian Maritime University
[7]	Wang H W, Wang K Y, Liu G H 2022 Ocean Eng. 258 111833 Google Scholar
[8]	赵超, 吕明利, 贾文广 2022 船舶工程 44 69 Google Scholar Zhao C, Lyu M L, Jia W G 2022 Ship Eng. 44 69 Google Scholar
[9]	詹杰民, 陆尚平, 李熠华, 李雨田, 胡文清 2023 海洋工程 41 1 Google Scholar Zhan J M, Lu S P, Li Y H, Li Y T, Hu W Q 2023 Ocean Eng. 41 1 Google Scholar
[10]	张晨远, 张智嘉, 丛巍巍, 魏浩, 张松松 2023 化学通报 86 863 Google Scholar Zhang C Y, Zhang Z J, Cong W W, Wei H, Zhang S S 2023 Chem. Bull. 86 863 Google Scholar
[11]	Moaven K, Rad M, Taeibi-Rahni M 2013 Exp. Therm. Fluid. Sci. 51 239 Google Scholar
[12]	Gao J, Zhang K, Li H, Lang C, Zhang L X 2023 Prog. Org. Coat. 183 107769 Google Scholar
[13]	Chen H W, Zhang X, Che D, Zhang D Y, Li X, Li Y Y 2014 Adv. Mech. Eng. 2014 425701 Google Scholar
[14]	Luo Y, Zhang D, Liu Y, Li Y, Ng E Y K 2015 J. Mech. Med. Biol. 15 1550084 Google Scholar
[15]	Shen X, Ceccio S L, Perlin M 2006 Exp. Fluids 41 415 Google Scholar
[16]	Zhao X J, Zong Z 2022 Ocean Eng. 251 111032 Google Scholar
[17]	Tanaka T, Oishi Y, Park H J, Tasaka Y, Murai Y, Kawakita C 2023 Ocean Eng. 272 113807 Google Scholar
[18]	Maryami R, Javadpoor M, Farahat S 2016 Heat Mass Transfer 52 2593 Google Scholar
[19]	Bidkar R A, Leblanc L, Kulkarni A J, Bahadur V, Ceccio S L, Perlin M 2014 Phys. Fluids 26 085108 Google Scholar
[20]	Mail M, Moosmann M, Häger P, Barthlott W 2019 Phil. Trans. R. Soc. A 377 20190126 Google Scholar
[21]	Wang F C, Qian J H, Fan J C, Li J C, Xu H Y, Wu H A 2022 Sci. China Phys. Mech. 65 264601 Google Scholar
[22]	石小燕, 曾丹苓, 蔡治勇 2005 热科学与技术 4 195 Google Scholar Shi X Y, Zeng D L, Cai Z Y 2005 J. Therm. Sci. Technol. 4 195 Google Scholar
[23]	Weijs J H, Snoeijer J H, Lohse D 2012 Phys. Rev. Lett. 108 104501 Google Scholar
[24]	Cao B Y, Chen M, Guo Z Y 2006 Phys. Rev. E 74 066311 Google Scholar
[25]	Stukowski A 2010 Model. Simul. Mater. Sci. Eng. 18 015012 Google Scholar
[26]	Zhang Z Q, Liu H L, Liu Z, Zhang Z, Cheng G G, Wang X D, Ding J N 2019 Appl. Surf. Sci. 475 857 Google Scholar
[27]	刘汉伦, 张忠强, 郝茂磊, 程广贵, 丁建宁 2018 气体物理 3 32 Google Scholar Liu H L, Zhang Z Q, Hao M L, Cheng G G, Ding J N 2018 Phys. Gases 3 32 Google Scholar
[28]	Ceccio S L 2010 Annu. Rev. Fluid Mech. 42 183 Google Scholar
[29]	Kitagawa A, Denissenko P, Murai Y 2019 Exp. Therm. Fluid Sci. 104 141 Google Scholar
[30]	邢赫威, 陈占秀, 杨历, 苏瑶, 李源华, 呼和仓 2024 73 094701 Google Scholar Xing H W, Chen Z X, Yang L, Su Y, Li Y H, Huhe C 2024 Acta Phys. Sin. 73 094701 Google Scholar
[31]	Hu H B, Wang D Z, Ren F, Bao L Y, Priezjev N V, Wen J 2018 Int. J. Multiphase Flow 104 166 Google Scholar
[32]	吕鹏宇, 薛亚辉, 段慧玲 2016 力学进展 46 179 Google Scholar Lyu P Y, Xue Y H, Duan H L 2016 Adv. Mech. 46 179 Google Scholar
[33]	García-Magariño A, Lopez-Gavilan P, Sor S, Terroba F 2023 J. Mar. Sci. Eng. 11 1315 Google Scholar
[34]	Tretyakov N, Müller M 2013 Soft Matter 9 3613 Google Scholar
[35]	He Y Y, Fu Y H, Wang H, Yang J 2021 Tribol. Int. 162 107144 Google Scholar
[36]	Tang S N, Zhu Y, Yuan S Q 2023 J. Bionic Eng. 20 2797 Google Scholar
[37]	He Y Y, Fu Y H, Wang H, Yang J 2022 J. Manuf. Process. 75 1089 Google Scholar

[1]	Chen Jing-Jing, Zhao Hong-Po, Wang Kui, Zhan Hui-Min, Luo Ze-Yu. Molecular dynamics simulation of mechanical strengthening properties of SiC substrate covered with multilayer graphene. Acta Physica Sinica, 2024, 73(10): 109601. doi: 10.7498/aps.73.20232031
[2]	Zhang Yu-Hang, Li Xiao-Bao, Zhan Chun-Xiao, Wang Mei-Qin, Pu Yu-Xue. Molecular dynamics simulation study on mechanical properties of Janus MoSSe monolayer. Acta Physica Sinica, 2023, 72(4): 046201. doi: 10.7498/aps.72.20221815
[3]	Wang Xiao-Feng, Tao Gang, Xu Ning, Wang Peng, Li Zhao, Wen Peng. Molecular dynamics analysis of shock wave-induced nanobubble collapse in water. Acta Physica Sinica, 2021, 70(13): 134702. doi: 10.7498/aps.70.20210058
[4]	Diwu Min-Jie, Hu Xiao-Mian. Molecular dynamics simulation of shock-induced isostructural phase transition in single crystal Ce. Acta Physica Sinica, 2020, 69(11): 116202. doi: 10.7498/aps.69.20200323
[5]	Mei Tao, Chen Zhan-Xiu, Yang Li, Zhu Hong-Man, Miao Rui-Can. Molecular dynamics study of interface thermal resistance in asymmetric nanochannel. Acta Physica Sinica, 2020, 69(22): 224701. doi: 10.7498/aps.69.20200491
[6]	Li Jie-Jie, Lu Bin-Bin, Xian Yue-Hui, Hu Guo-Ming, Xia Re. Characterization of nanoporous silver mechanical properties by molecular dynamics simulation. Acta Physica Sinica, 2018, 67(5): 056101. doi: 10.7498/aps.67.20172193
[7]	Dong Qi-Qi, Hu Hai-Bao, Chen Shao-Qiang, He Qiang, Bao Lu-Yao. Molecular dynamics simulation of freezing process of water droplets impinging on cold surface. Acta Physica Sinica, 2018, 67(5): 054702. doi: 10.7498/aps.67.20172174
[8]	Zhang Bao-Ling, Song Xiao-Yong, Hou Qing, Wang Jun. Molecular dynamics study on the phase transition of high density helium. Acta Physica Sinica, 2015, 64(1): 016202. doi: 10.7498/aps.64.016202
[9]	Wang Cheng-Long, Wang Qing-Yu, Zhang Yue, Li Zhong-Yu, Hong Bing, Su Zhe, Dong Liang. Molecular dynamics study of cascade damage at SiC/C interface. Acta Physica Sinica, 2014, 63(15): 153402. doi: 10.7498/aps.63.153402
[10]	Chang Xu. Ripples of multilayer graphenes：a molecular dynamics study. Acta Physica Sinica, 2014, 63(8): 086102. doi: 10.7498/aps.63.086102
[11]	Zhou Hua-Guang, Lin Xin, Wang Meng, Huang Wei-Dong. Calculation of crystal-melt interfacial free energy of Cu by molecular dynamics simulation. Acta Physica Sinica, 2013, 62(5): 056803. doi: 10.7498/aps.62.056803
[12]	Wei Qi, E Wen-Ji. Thermodynamic analyses of dewetting instability in thin films. Acta Physica Sinica, 2012, 61(16): 160508. doi: 10.7498/aps.61.160508
[13]	Ma Ying. Variable charge molecular dynamics simulation of vitreous silica. Acta Physica Sinica, 2011, 60(2): 026101. doi: 10.7498/aps.60.026101
[14]	Zhang Liang, Fu Wei-Ji, Zhang Li-Feng, Wu Hai-Yan, Huang Hong. On the evolution of Couette flow energy. Acta Physica Sinica, 2010, 59(3): 1437-1448. doi: 10.7498/aps.59.1437
[15]	Shao Jian-Li, Wang Pei, Qin Cheng-Sen, Zhou Hong-Qiang. Shock-induced phase transformations of iron studied with molecular dynamics. Acta Physica Sinica, 2007, 56(9): 5389-5393. doi: 10.7498/aps.56.5389
[16]	Zhou Nai-Gen, Zhou Lang. Conditions for formation of misfit dislocation in epitaxial films — a molecular dynamics study. Acta Physica Sinica, 2005, 54(7): 3278-3283. doi: 10.7498/aps.54.3278
[17]	Luo Jin, Zhu Wen-Jun, Lin Li-Bin, He Hong-Liang, Jing Fu-Qian. Molecular dynamics simulation of void growth in single crystal copper under uniaxial impacting. Acta Physica Sinica, 2005, 54(6): 2791-2798. doi: 10.7498/aps.54.2791
[18]	Wang Hai-Long, Wang Xiu-Xi, Liang Hai-Yi. Molecular dynamics simulation of strain effects on surface melting for metal Cu. Acta Physica Sinica, 2005, 54(10): 4836-4841. doi: 10.7498/aps.54.4836
[19]	Liang Hai-Ge, Wang Xiu-Xi, Wu Heng-An, Wang Yu and. . Acta Physica Sinica, 2002, 51(10): 2308-2314. doi: 10.7498/aps.51.2308
[20]	Wu Heng-An, Ni Xiang-Gui, Wang Yu, Wang Xiu-Xi. . Acta Physica Sinica, 2002, 51(7): 1412-1415. doi: 10.7498/aps.51.1412

Catalog

Vol. 73, Issue 15 - 2024-08-05

Metrics

Abstract views: 1395
PDF Downloads: 49
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板