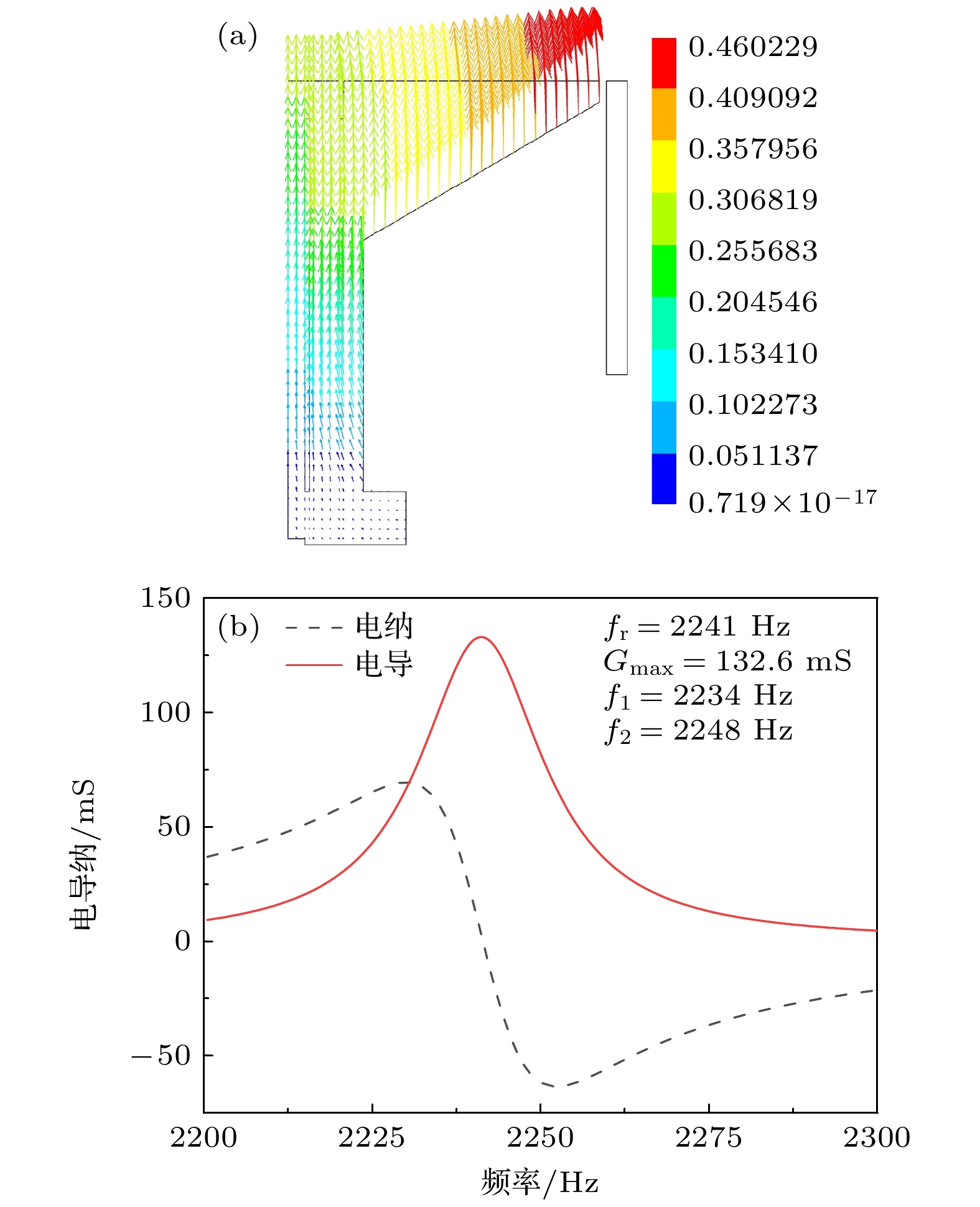
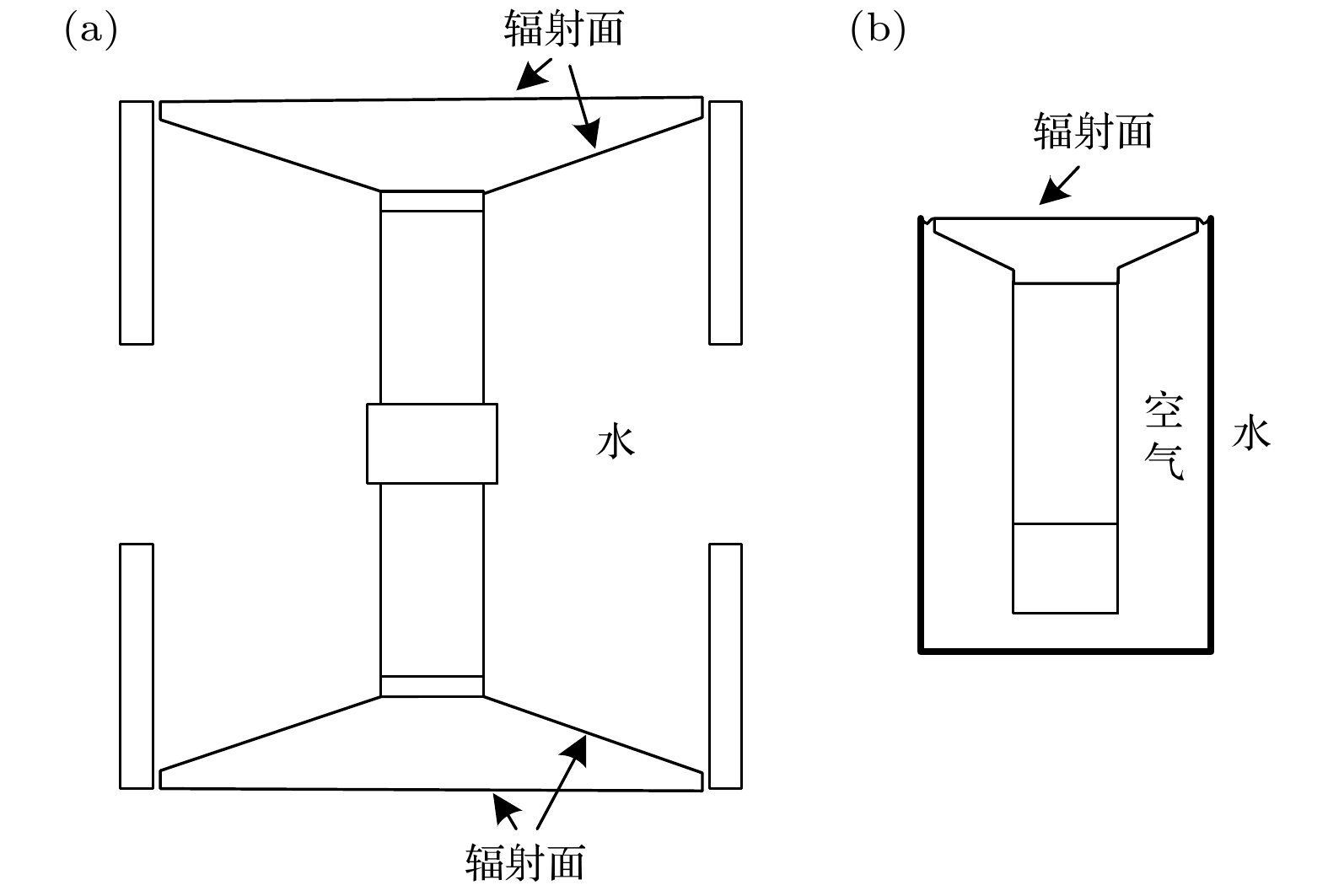
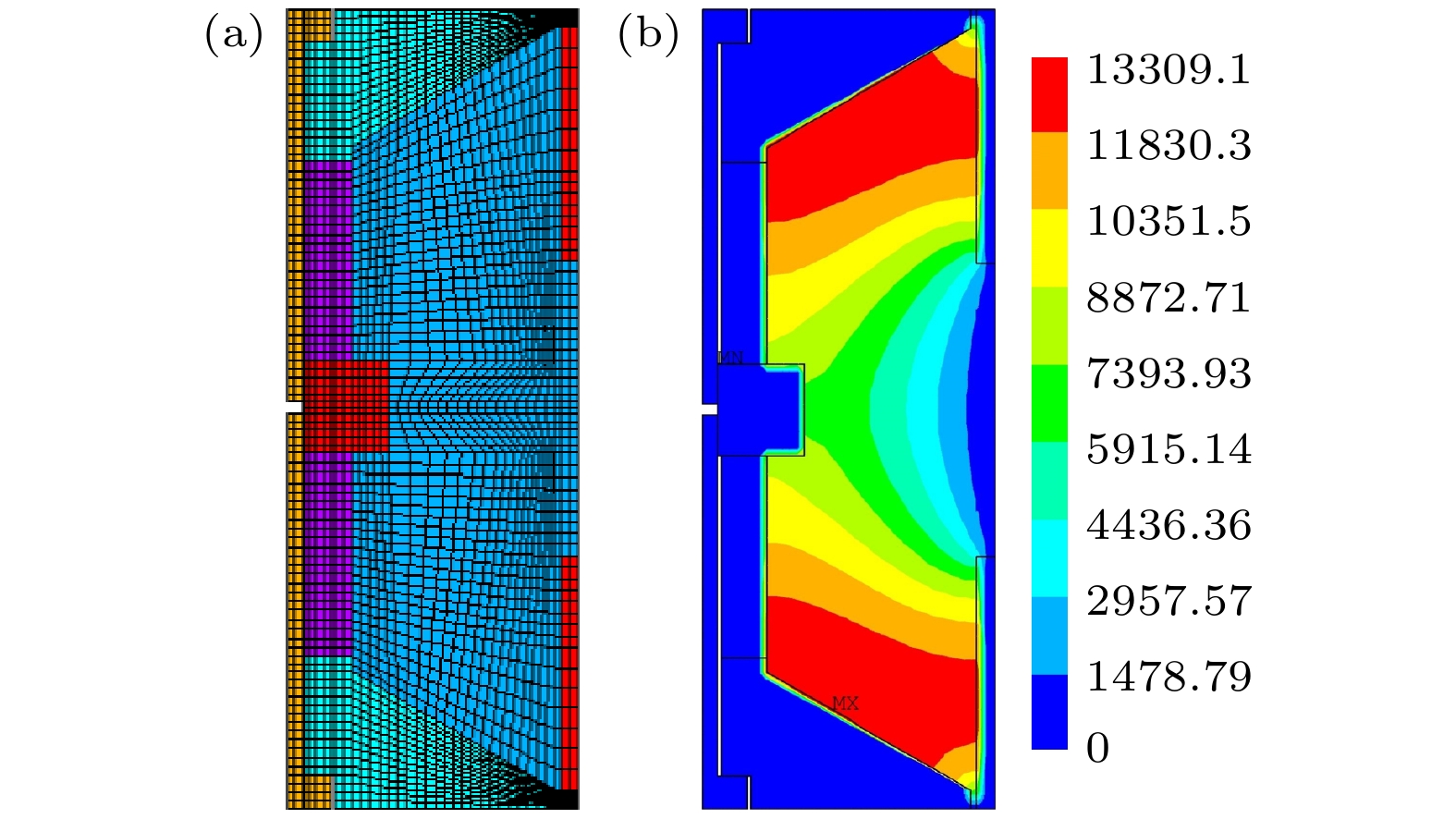
-
Janus-Helmholtz transducer has the characteristics of high-power and broadband transmission due to the coupling between the longitudinal resonance of the driver and the liquid cavity resonance of Helmholtz resonator. Traditional view holds that the low frequency resonance peak in the transmitting voltage response curve in water is fluid cavity mode of Helmholtz resonator while the high frequency resonance is the longitudinal mode of Janus transducer. However, in the past few decades, a large number of experimental studies have found that this conclusion is questionable. This work is to distinguish the two resonances in the response curve and the two vibration modes of Janus-Helmholtz transducer. Based on the Janus-Helmholtz transducer prototype reported in the literature, the resonance frequencies of the vibration modes of Janus-Helmholtz transducer are studied theoretically. The structure dimensions and material parameters of the prototype are listed in detail. The test results and simulation results of conductivity are also presented. The longitudinal resonance of the driver is determined by using the equivalent circuit method and finite element analysis. Radiation masses of both Janus transducer and typical longitudinal vibration transducer are also calculated to analyze the phenomenon of the sharp decrease of longitudinal resonance frequency in water. Acoustic modal analysis by using ANSYS software is performed to investigate the resonance frequency of complex Helmholtz resonator in Janus-Helmholtz transducer. Correction length on the vent introduced by external fluid sound radiation is used to obtain the accurate Helmholtz resonance frequency. The sound pressure distribution of Helmholtz resonator obtained through finite element method is investigated, and the correct equivalent formula for solving the Helmholtz resonance frequency is obtained. The results reveal that the first resonance in the response curve is driver resonance while the second one is Helmholtz resonance, which is contrary to the traditional view. The considerable reduction of driver resonance frequency in water is mainly due to the large radiation mass on the four large radiation surfaces of the Janus transducer, which also causes the sharp response of driver resonance. In Janus-Helmholtz transducer, there are two Helmholtz resonators with the same size, instead of only one resonator in the traditional view. The two resonance frequencies solved by the method proposed in this work are in good agreement with the test and simulation results. These conclusions play an important role in correctly understanding the structure and characteristics of Janus-Helmholtz transducer at source, as well as provide technical support for structural optimization and innovation, thus improving the acoustic emission properties of the transducer.
-
Keywords:
- Janus-Helmholtz transducer /
- resonance frequency /
- equivalent circuit method /
- radiation mass
[1] Decarpigny J N, Hamonic B, Wilson O B 1991 IEEE J. Ocean. Eng. 16 107
Google Scholar
[2] Le Gall Y, Boucher D, Lurton X, Bruneau A M 1994 Proceedings of OCEANS'94 Brest, France, September 13–16, 1994 p284
[3] Le Gall Y 1994 J. Phys. IV 4 343 (in French
Google Scholar
[4] Le Gall Y, Boucher D, Lurton X, Bruneau A M 1993 Proceedings of OCEANS'93 Victoria, BC, Canada, October 18–21, 1993 p278
[5] Le Gall Y 1999 Proceedings of Sonar Transducers'99 Birmingham, UK, April, 1999 p103
[6] Ker S, Le Gall Y, Marsset T, Leon P 2008 70th EAGE Conference and Exhibition incorporating SPE EUROPEC Rome, Italy, June 9–12, 2008 cp-40-00440
[7] Ker S, Marsset B, Garziglia S, Le Gonidec Y, Gibert D, Voisset M, Adamy J 2010 Geophys. J. Int. 182 1524
Google Scholar
[8] Marsset T, Marsset B, Ker S, Thomas Y, Le Gall Y 2010 Deep-Sea Res. I: Oceanogr. Res. Pap. 57 628
Google Scholar
[9] 张振雨, 王艳, 陈光华 2016 声学技术 35 479
Google Scholar
Zhang Z Y, Wang Y, Chen G H 2016 Tech. Acoust. 35 479
Google Scholar
[10] 伊子旭, 莫喜平, 柴勇, 张运强, 崔斌 2017 中国声学学会2017年全国声学学术会议 哈尔滨, 9月21日—23日, 2017 p803
Yi Z X, Mo X P, Chai Y, Zhang Y Q, Cui B 2017 National Acoustics Academic Conference of the Chinese Acoustic Society Harbin, China, September 21–23, 2017 p803
[11] 李世平, 莫喜平, 柴勇, 张运强, 崔斌 2015 中国声学学会水声学分会2015年学术会议 武汉, 6月5—8日, 2015 p193
Li S P, Mo X P, Chai Y, Zhang Y Q, Cui B 2015 Academic Conference of the Underwater Acoustics Branch of the Chinese Acoustic Society Wuhan, China, June 5–8, 2015 p193
[12] 桑永杰, 蓝宇 2013 哈尔滨工程大学学报 34 1261
Google Scholar
Sang Y J, Lan Y 2013 J. Harbin Eng. Univ. 34 1261
Google Scholar
[13] 桑永杰, 蓝宇, 吴彤, 丁玥文 2017 声学学报 42 397
Google Scholar
Sang Y J, Lan Y, Wu T, Ding Y W 2017 Acta Acoust. 42 397
Google Scholar
[14] 桑永杰, 蓝宇 2015 哈尔滨工程大学学报 36 906
Google Scholar
Sang Y J, Lan Y 2015 J. Harbin Eng. Univ. 36 906
Google Scholar
[15] Moffett M B, Powers J M, Jevnager M D 1998 J. Acoust. Soc. Am. 103 3353
Google Scholar
[16] Chen H, Tang Y N, Gu Z Q 2015 Radar ECM 35 60 [陈浩, 唐永宁, 顾郑强 2015 雷达与对抗 35 60]
Google Scholar
Chen H, Tang Y N, Gu Z Q 2015 Radar ECM35 60 Google Scholar
[17] Butler S C 2002 Proceedings of SPIE San Diego, CA, July 11, 2002 p510
[18] Butler J L, Sherman C H 2016 Transducers and Arrays for Underwater Sound (New York: Springer) pp220–225
[19] 林书玉 2004 超声换能器的原理及设计(北京: 科学出版社) 第98—111页
Lin S Y 2004 The Principle and Design of Ultrasonic Transducers (Beijing: Science Press) pp98–111
[20] 莫喜平 2007 声学技术 26 1279
Google Scholar
Mo X P 2007 Tech. Acoust. 26 1279
Google Scholar
[21] 莫喜平 2018 应用声学 37 671
Google Scholar
Mo X P 2018 J. Appl. Acoust. 37 671
Google Scholar
-
图 1 换能器样机的结构示意图及照片 (a) 结构示意图; (b) 照片
Figure 1. Structure diagram and photo of the prototype: (a) Structure diagram; (b) photo.
图 2 换能器的1/2模型及其尺寸标识
Figure 2. Half transducer and its dimensions.
图 3 电导测试及仿真结果
Figure 3. Test and simulation results of conductivity.
图 4 换能器空气中的有限元分析结果 (a) 模态分析结果; (b) 电导纳曲线
Figure 4. Modal analysis results and admittance curves of the Janus-Helmholtz transducer in air: (a) Modal analysis result; (b) admittance curves.
图 5 水中1/2 Janus换能器的机电等效图
Figure 5. Electromechanical equivalent circuit of the half Janus transducer in water.
图 6 基于四端网络等效电路理论计算得到的水中电导纳曲线
Figure 6. Theoretical result of admittance in water using four-terminal network equivalent circuit.
图 7 空气中Janus换能器的等效图 (a)等效电路图; (b)机电等效图
Figure 7. Equivalent circuit of the Janus transducer in air: (a) Electrical equivalent circuit; (b) electromechanical equivalent circuit.
图 8 水中Janus换能器的机电等效图
Figure 8. Electromechanical equivalent circuit of the Janus transducer in water.
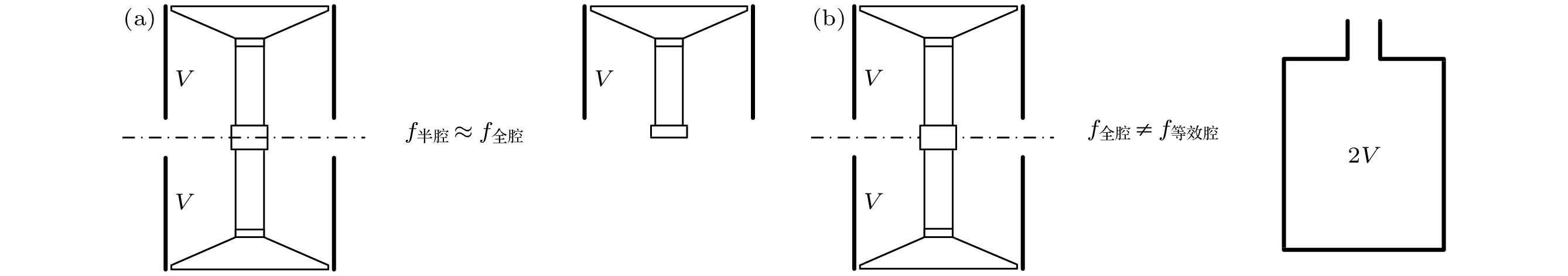
图 9 Janus-Helmholtz换能器与典型纵振动换能器的结构对比 (a) Janus-Helmholtz换能器结构示意图; (b) 典型纵振动换能器结构示意图
Figure 9. Structural comparison between Janus-Helmholtz transducer and typical longitudinal vibration transducer: (a) Structure diagram of Janus-Helmholtz transducer; (b) structure diagram of typical longitudinal vibration transducer.
图 10 Janus换能器和典型纵振动换能器辐射质量的比较
Figure 10. Radiation mass comparison between Janus transducer and typical longitudinal transducer.
图 11 腔体充液换能器的有限元模型与模态分析结果 (a)有限元模型; (b) 模态分析结果
Figure 11. Finite element model and acoustic modal analysis result of the Helmholtz resonator: (a) Finite element model; (b) acoustic modal analysis results.
图 12 辐射口修正长度后的有限元模型与模态分析结果 (a) 有限元模型; (b) 模态分析结果
Figure 12. Finite element model with radiation mass and analysis results of the Helmholtz resonator: (a) Finite element model; (b) acoustic modal analysis results.
图 13 求解Helmholtz液腔谐振频率的两种等效方法 (a) 正确的等效方法; (b) 错误的等效方法
Figure 13. Two equivalents for solving the Helmholtz resonance frequency: (a) Correct equivalent; (b) incorrect equivalent.
图 14 腔体长度不等的Janus-Helmholtz换能器结构示意图及谐波响应仿真结果 (a) 结构示意图; (b) 谐波响应仿真结果
Figure 14. Janus-Helmholtz transducer withunequal length housings and harmonic analysis simulation results: (a) Structure diagram; (b) harmonic analysis simulation results.
表 1 换能器的尺寸(单位: m)
Table 1. Dimensions of the transducer (Unit: m).
$ {r_1} $ $ {r_2} $ $ {r_3} $ $ {r_4} $ $ {r_5} $ $ {t_1} $ $ {t_2} $ $ {t_3} $ $ {t_4} $ $ {t_5} $ $ {l_1} $ $ {l_2} $ 0.05 0.035 0.145 0.148 0.158 0.025 0.11 0.008 0.065 0.01 0.08 0.138 
DownLoad: CSV
表 3 压电晶堆PZT-4的材料属性
Table 3. Material properties of PZT-4 used in the transducer.
$ c_{{11}}^{\text{E}}/{\text{GPa}} $ $ c_{12}^{\text{E}}/{\text{GPa}} $ $ c_{13}^{\text{E}}/{\text{GPa}} $ $ c_{33}^{\text{E}}/{\text{GPa}} $ $ c_{{44}}^{\text{E}}/{\text{GPa}} $ $ {e_{31}}/({\text{C}}{\cdot}{{\text{m}}^{{{ - 2}}}}) $ $ {e_{33}}/({\text{C}}{\cdot}{{\text{m}}^{{{ - 2}}}}) $ $ {e_{15}}/({\text{C}} {\cdot} {{\text{m}}^{{{ - 2}}}}) $ $ {\varepsilon _{33}} $ $ {\varepsilon _{11}} $ 139 77.8 74.3 115 25.6 –5.2 15.1 12.7 35 30
DownLoad: CSV
表 2 换能器金属部分的材料属性
Table 2. Material properties of metal used in the transducer.
换能器部件 材质 $ Y/{\text{GPa}} $ $ \rho /({\text{kg}}{\cdot}{{\text{m}}^{{{ - 3}}}}) $ $ c/({\text{m}}{\cdot}{{\mathrm{s}}^{{{ - 1}}}}) $ 中间质量块 不锈钢 193 7900 4940 辐射盖板、腔体 硬铝 71 2700 5150
DownLoad: CSV
表 4 几种纵振动换能器空气中和水中谐振频率的比较
Table 4. Comparison of resonance frequencies of several typical longitudinal vibration transducers in air and water.
DownLoad: CSV
-
[1] Decarpigny J N, Hamonic B, Wilson O B 1991 IEEE J. Ocean. Eng. 16 107
Google Scholar
[2] Le Gall Y, Boucher D, Lurton X, Bruneau A M 1994 Proceedings of OCEANS'94 Brest, France, September 13–16, 1994 p284
[3] Le Gall Y 1994 J. Phys. IV 4 343 (in French
Google Scholar
[4] Le Gall Y, Boucher D, Lurton X, Bruneau A M 1993 Proceedings of OCEANS'93 Victoria, BC, Canada, October 18–21, 1993 p278
[5] Le Gall Y 1999 Proceedings of Sonar Transducers'99 Birmingham, UK, April, 1999 p103
[6] Ker S, Le Gall Y, Marsset T, Leon P 2008 70th EAGE Conference and Exhibition incorporating SPE EUROPEC Rome, Italy, June 9–12, 2008 cp-40-00440
[7] Ker S, Marsset B, Garziglia S, Le Gonidec Y, Gibert D, Voisset M, Adamy J 2010 Geophys. J. Int. 182 1524
Google Scholar
[8] Marsset T, Marsset B, Ker S, Thomas Y, Le Gall Y 2010 Deep-Sea Res. I: Oceanogr. Res. Pap. 57 628
Google Scholar
[9] 张振雨, 王艳, 陈光华 2016 声学技术 35 479
Google Scholar
Zhang Z Y, Wang Y, Chen G H 2016 Tech. Acoust. 35 479
Google Scholar
[10] 伊子旭, 莫喜平, 柴勇, 张运强, 崔斌 2017 中国声学学会2017年全国声学学术会议 哈尔滨, 9月21日—23日, 2017 p803
Yi Z X, Mo X P, Chai Y, Zhang Y Q, Cui B 2017 National Acoustics Academic Conference of the Chinese Acoustic Society Harbin, China, September 21–23, 2017 p803
[11] 李世平, 莫喜平, 柴勇, 张运强, 崔斌 2015 中国声学学会水声学分会2015年学术会议 武汉, 6月5—8日, 2015 p193
Li S P, Mo X P, Chai Y, Zhang Y Q, Cui B 2015 Academic Conference of the Underwater Acoustics Branch of the Chinese Acoustic Society Wuhan, China, June 5–8, 2015 p193
[12] 桑永杰, 蓝宇 2013 哈尔滨工程大学学报 34 1261
Google Scholar
Sang Y J, Lan Y 2013 J. Harbin Eng. Univ. 34 1261
Google Scholar
[13] 桑永杰, 蓝宇, 吴彤, 丁玥文 2017 声学学报 42 397
Google Scholar
Sang Y J, Lan Y, Wu T, Ding Y W 2017 Acta Acoust. 42 397
Google Scholar
[14] 桑永杰, 蓝宇 2015 哈尔滨工程大学学报 36 906
Google Scholar
Sang Y J, Lan Y 2015 J. Harbin Eng. Univ. 36 906
Google Scholar
[15] Moffett M B, Powers J M, Jevnager M D 1998 J. Acoust. Soc. Am. 103 3353
Google Scholar
[16] Chen H, Tang Y N, Gu Z Q 2015 Radar ECM 35 60 [陈浩, 唐永宁, 顾郑强 2015 雷达与对抗 35 60]
Google Scholar
Chen H, Tang Y N, Gu Z Q 2015 Radar ECM35 60 Google Scholar
[17] Butler S C 2002 Proceedings of SPIE San Diego, CA, July 11, 2002 p510
[18] Butler J L, Sherman C H 2016 Transducers and Arrays for Underwater Sound (New York: Springer) pp220–225
[19] 林书玉 2004 超声换能器的原理及设计(北京: 科学出版社) 第98—111页
Lin S Y 2004 The Principle and Design of Ultrasonic Transducers (Beijing: Science Press) pp98–111
[20] 莫喜平 2007 声学技术 26 1279
Google Scholar
Mo X P 2007 Tech. Acoust. 26 1279
Google Scholar
[21] 莫喜平 2018 应用声学 37 671
Google Scholar
Mo X P 2018 J. Appl. Acoust. 37 671
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 2869
- PDF Downloads: 98
- Cited By: 0
