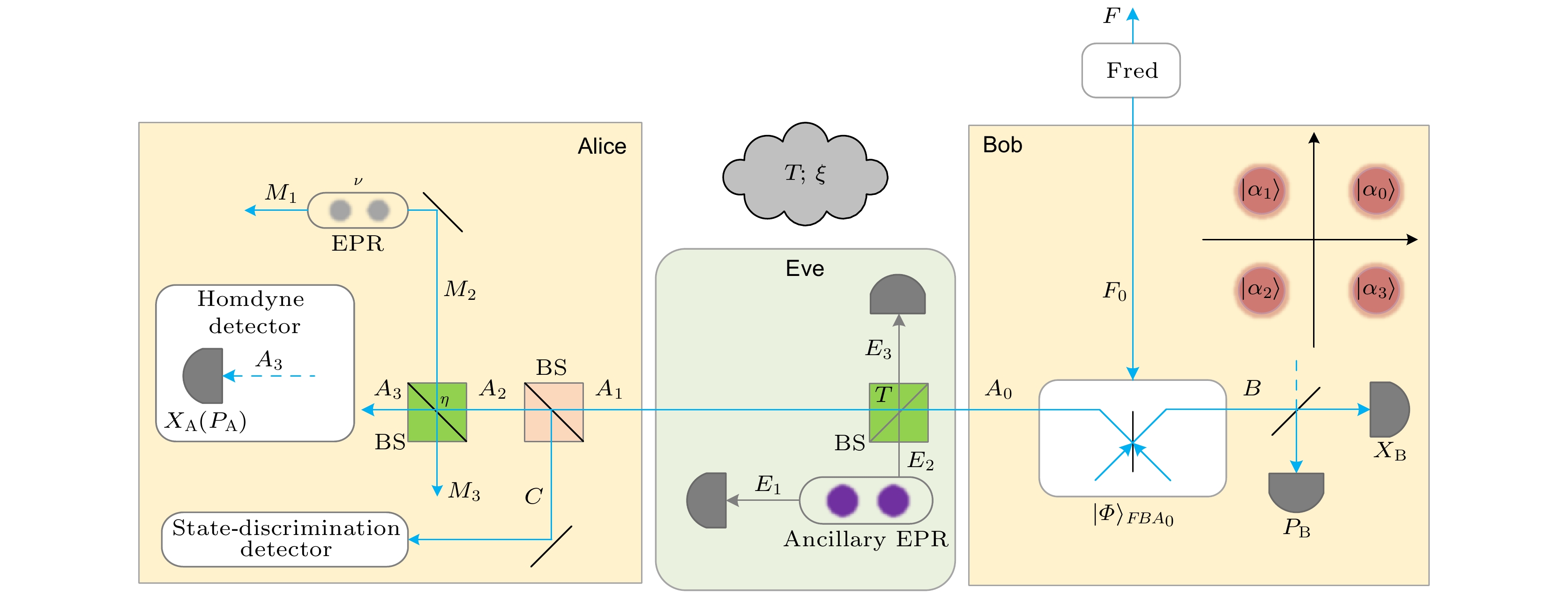
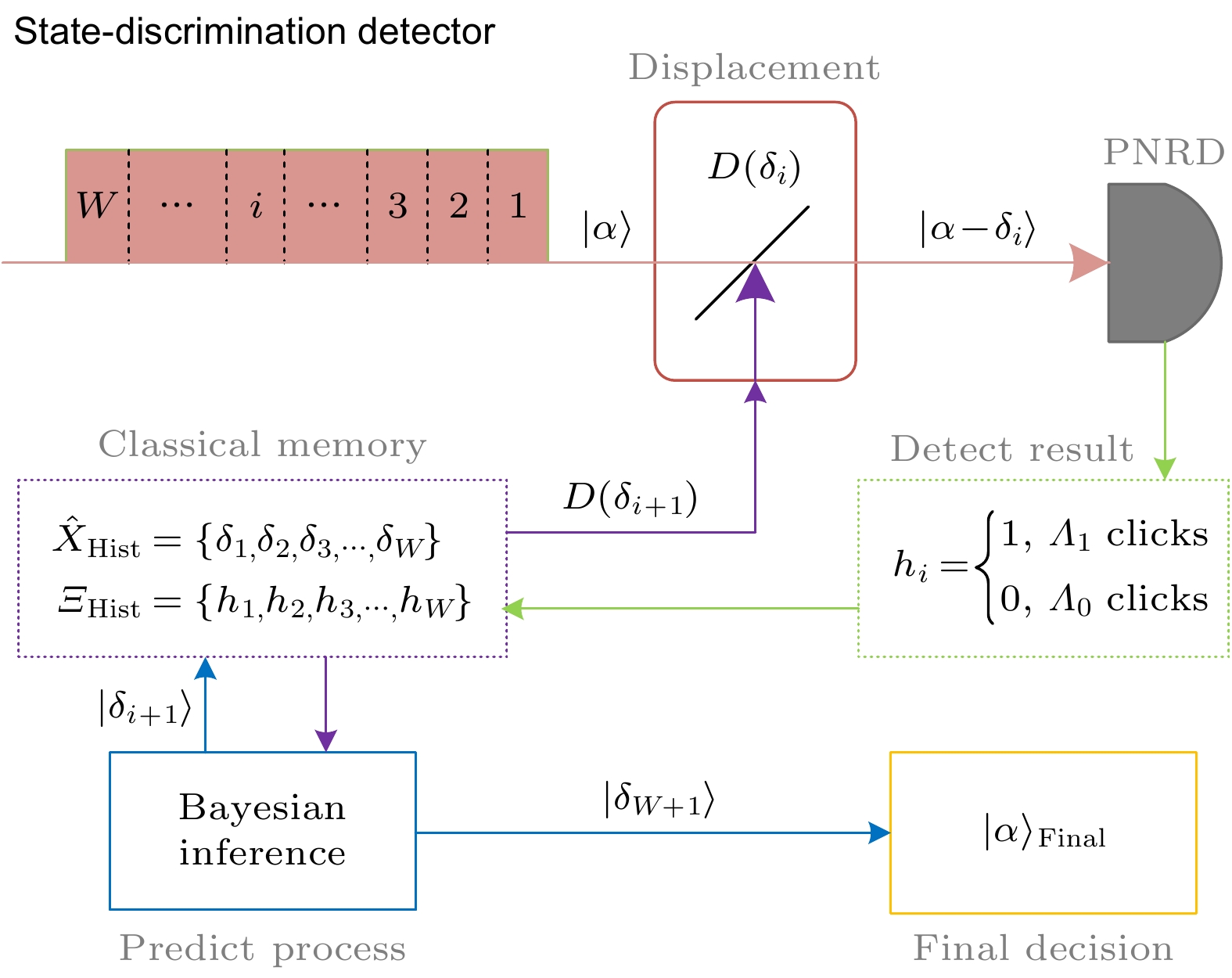
-
Plug-and-play discrete modulation continuous variable quantum key distribution can generate local oscillator light locally without using two independent lasers, and both signal light and local oscillator are generated from the same laser, which can effectively ensure the practical security of the system and have a completely identical frequency characteristic. In addition, this scheme has good compatibility with efficient error correction codes, and can achieve high reconciliation efficiency even at low signal-to-noise ratio. However, there exists large excess noise in the plug-and-play configuration based on the untrusted source model, which seriously limits the maximum transmission distance of the discrete modulation scheme. To solve this problem, we propose a plug-and-play discrete modulation continuous variable quantum key distribution based on non-Gaussian state-discrimination detection. That is to say, a non-Gaussian state-discrimination detector is deployed at the receiver. With adaptive measurement method and Bayesian inference, four non-orthogonal coherent states which are based on four-state discrete modulation can be unconditionally distinguished on condition that the error probability is lower than the standard quantum limit. We analyze the security of the proposed protocol by considering both asymptotic limit and finite-size effect. Simulation results show that the secret key rate and maximum transmission distance are significantly enhanced by using no-Gaussian state-discrimination detection even under the influence of the untrusted source noise compared with the original plug-and-play discrete modulation continuous variable quantum key distribution. These results indicate that the proposed scheme can effectively reduce the negative influence of the untrust source noise on the performance of the plug-and-play discrete modulation continuous variable quantum key distribution protocol. The proposed protocol can not only ensure the practical security of the system, but also achieve more efficient and longer transmission distance quantum key distribution.
-
Keywords:
- plug-and-play /
- discrete modulation /
- continuous variable quantum key distribution /
- non-Gaussian state-discrimination detection
[1] Xu F, Ma X, Zhang Q, Lo H K, Pan J W 2020 Rev. Mod. Phys. 92 025002
Google Scholar
[2] Pirandola S, Andersen U L, Banchi L, et al. 2020 Adv. Opt. Photon. 12 1012
Google Scholar
[3] Liu H, Jiang C, Zhu H T, et al. 2021 Phys. Rev. Lett. 126 250502
Google Scholar
[4] Lo H K, Chau H F 1999 Science 283 2050
Google Scholar
[5] Shor P W, Preskill J 2000 Phys. Rev. Lett. 85 441
Google Scholar
[6] Yin J, Li Y H, Liao S K, et al. 2020 Nature 582 501
Google Scholar
[7] Fang X T, Zeng P, Liu H, et al. 2020 Nat. Photonics 14 422
Google Scholar
[8] Chen J P, Zhang C, Liu Y, et al. 2021 Nat. Photonics 15 570
Google Scholar
[9] Laudenbach F, Pacher C, Fung C H F, Poppe A, Peev M, Schrenk B, Hentschel M, Walther P, Hübel H 2018 Adv. Quantum Technol. 1 1800011
Google Scholar
[10] Wu X D, Wang Y J, Huang D, Guo Y 2020 Front. Phys. 15 31601
Google Scholar
[11] Grosshans F, Grangier P 2002 Phys. Rev. Lett. 88 057902
Google Scholar
[12] 钟海, 叶炜, 吴晓东, 郭迎 2021 70 020301
Google Scholar
Zhong H, Ye W, Wu X D, Guo Y 2021 Acta Phys. Sin. 70 020301
Google Scholar
[13] Grosshans F, Assche G V, Wenger J, Brouri R, Cerf N J, Grangier P 2003 Nature (London) 421 238
[14] Huang D, Huang P, Lin D, Zeng G 2016 Sci. Rep. 6 19201
Google Scholar
[15] Jouguet P, Kunz-Jacques S, Leverrier A, Grangier P, Diamanti E 2013 Nat. Photonics 7 378
Google Scholar
[16] Huang D, Lin D, Wang C, Liu W, Fang S, Peng J, Huang P, Zeng G 2015 Opt. Express 23 17511
Google Scholar
[17] Zhang Y, Chen Z, Pirandola S, Wang X, Zhou C, Chu B, Zhao Y, Xu B, Yu S, Guo H 2020 Phys. Rev. Lett. 125 010502
Google Scholar
[18] Huang D, Huang P, Li H, Wang T, Zhou Y, Zeng G 2016 Opt. Lett. 41 3511
Google Scholar
[19] Ma X C, Sun S H, Jiang M S, Liang L M 2013 Phys. Rev. A 88 022339
Google Scholar
[20] Ma X C, Sun S H, Jiang M S, Liang L M 2013 Phys. Rev. A 87 052309
Google Scholar
[21] Jouguet P, Kunz-Jacques S, Diamanti E 2013 Phys. Rev. A 87 062313
Google Scholar
[22] Qin H, Kumar R, Alléaume R 2016 Phys. Rev. A 94 012325
Google Scholar
[23] Qi B, Lougovski P, Pooser R, Grice W, Bobrek M 2015 Phys. Rev. X 5 041009
Google Scholar
[24] Soh D B S, Brif C, Coles P J, Lütkenhaus N, Camacho R M, Urayama J, Sarovar M 2015 Phys. Rev. X 5 041010
Google Scholar
[25] Huang D, Lin D K, Huang P, Zeng G H 2015 Opt. Lett. 40 3695
Google Scholar
[26] Marie A, Alléaume R 2017 Phys. Rev. A 95 012316
Google Scholar
[27] Wang T, Huang P, Zhou Y, Liu W, Zeng G 2018 Phys. Rev. A 97 012310
Google Scholar
[28] Wu X, Wang Y, Guo Y, Zhong H, Huang D 2021 Phys. Rev. A 103 032604
Google Scholar
[29] Huang D, Huang P, Wang T, Li H, Zhou Y, Zeng G 2016 Phys. Rev. A 94 032305
Google Scholar
[30] Silberhorn C, Ralph T C, Lütkenhaus N, Leuchs G 2002 Phys. Rev. Lett. 89 167901
Google Scholar
[31] Leverrier A, Grangier P 2009 Phys. Rev. Lett. 102 180504
Google Scholar
[32] Becerra F E, Fan J, Baumgartner G, Goldhar J, Kosloski J T, Migdall A 2013 Nat. Photonics 7 147
Google Scholar
[33] Becerra F E, Fan J, Migdall A 2013 Nat. Commun. 4 2028
Google Scholar
[34] Becerra F E, Fan J, Baumgartner G, Polyakov S V, Goldhar J, Kosloski J T, Migdall A 2011 Phys. Rev. A 84 062324
Google Scholar
[35] Helstrom C W 1976 Quantum Detection and Estimation Theory (Mathematics in Science and Engineering) (Vol. 123) (New York: Academic)
[36] Liao Q, Guo Y, Huang D, Huang P, Zeng G 2018 New J. Phys. 20 023015
Google Scholar
[37] Shen Y, Peng X, Yang J, Guo H 2011 Phys. Rev. A 83 052304
Google Scholar
[38] Wu X D, Wang Y J, Zhong H, Liao Q, Guo Y 2019 Front. Phys. 14 41501
Google Scholar
[39] Wu X, Wang Y, Zhong H, Ye W, Huang D, Guo Y 2020 Quantum Inf. Process. 19 234
Google Scholar
[40] Navascués M, Acín A 2005 Phys. Rev. Lett. 94 020505
Google Scholar
[41] García-Patrón R, Cerf N J 2006 Phys. Rev. Lett. 97 190503
Google Scholar
[42] Pirandola S, Braunstein S L, Lloyd S 2008 Phys. Rev. Lett. 101 200504
Google Scholar
[43] Renner R, Cirac J I 2009 Phys. Rev. Lett. 102 110504
Google Scholar
[44] Leverrier A, Grosshans F, Grangier P 2010 Phys. Rev. A 81 062343
Google Scholar
[45] Pirandola S, Laurenza R, Ottaviani C, Banchi L 2017 Nat. Commun. 8 15043
Google Scholar
-
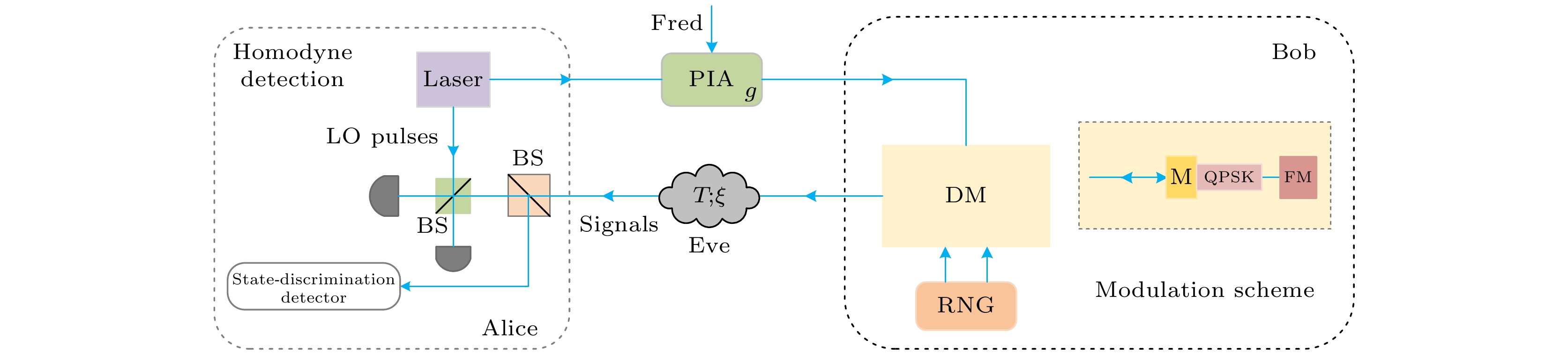
图 1 基于非高斯态区分探测的往返式离散调制CV-QKD制备-测量方案图. DM为离散调制, RNG为随机数发生器, M为调制器, QPSK为正交相移键控, PIA为相位非敏感放大器, FM为法拉第镜, BS为分束器, LO为本振光,
$ T $ 表示非可信信道的透过率,$ \xi $ 表示信道过噪声, g表示相位非敏感放大器的增益参数Figure 1. Prepare-and-measure version of plug-and-play discrete modulation CV-QKD protocol based on non-Gaussian state-discrimination detection. DM, discrete modulation; RNG, random number generator; M, modulator; QPSK, quadrature phase shift keying; PIA, phase insensitive amplifier; FM, Faraday mirror; BS, beam splitter; LO, local oscillator;
$ T $ , transmission efficiency;$ \xi $ , channel excess noise; g, gain parameters of phase insensitive amplifier.
图 2 基于非高斯态区分探测的往返式离散调制CV-QKD纠缠模型原理图
Figure 2. Schematic diagram of the entanglement-based (EB) model of plug-and-play discrete modulation CV-QKD protocol based on state-discrimination detection.
图 3 非高斯态区分探测器原理图. PNRD为光子数分辨探测器
Figure 3. Schematic diagram of non-Gaussian state discrimination detector. PNRD, photon-number-resolving detector.
图 4 不同增益参数
$g$ 下基于非高斯态区分探测的往返式离散调制CV-QKD方案的渐近密钥率和传输距离的关系Figure 4. The relationship between the asymptotic secret key rate of plug-and-play discrete modulation CV-QKD protocol based on non-Gaussian state-discrimination detection and the transmission distance under different gain
$g$ .
图 5 在实际信源(
$g = 1.005$ )与不同传输距离$L$ 下, 基于非高斯态区分探测的往返式离散调制CV-QKD方案的渐近密钥率与协商效率的关系Figure 5. The relationship between the asymptotic secret key rate of plug-and-play discrete modulation CV-QKD protocol based on non-Gaussian state-discrimination detection and the reconciliation efficiency under practical source(
$g = 1.005$ ) and different transmission distance$L$ .
图 6 不同传输距离下L下, 基于非高斯态区分探测的往返式离散调制CV-QKD方案的信噪比与增益参数g (不同的信源条件)的关系
Figure 6. The relationship between the signal-to-noise ratio of plug-and-play discrete modulation CV-QKD protocol based on non-Gaussian state-discrimination detection and the gain g (different source conditions) under different transmission distance L.
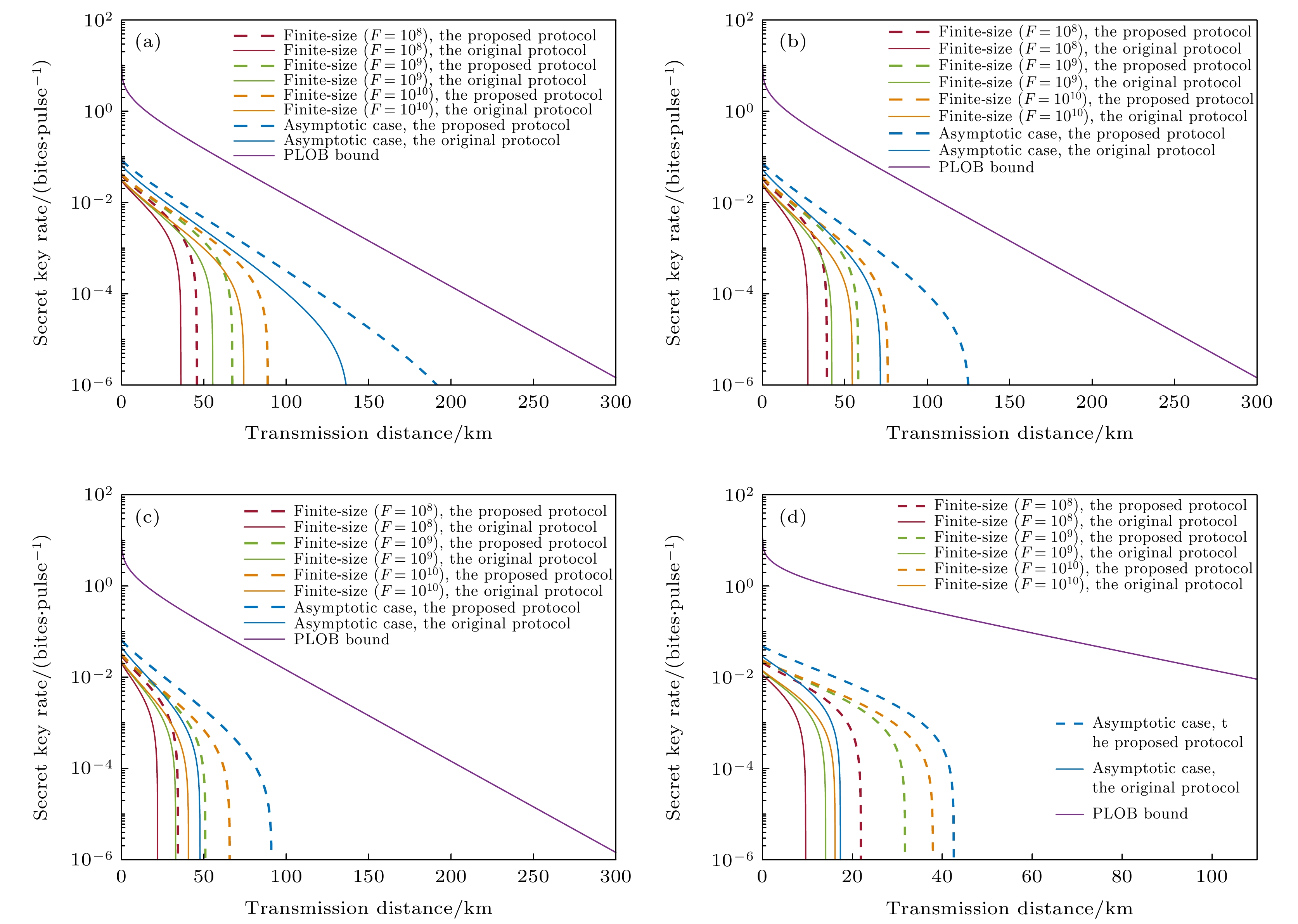
图 7 在不同的有效数据总长度
$F$ 下基于非高斯态区分探测的往返式离散调制CV-QKD方案有限长密钥率与传输距离的关系 (a)$g = 1$ ; (b)$g = 1.003$ ; (c)$g = 1.005$ ; (d)$g = 1.01$ Figure 7. The relationship between the finite-size secret key rate of plug-and-play discrete modulation CV-QKD protocol based on non-Gaussian state-discrimination detection and the transmission distance under different total exchanged signals
$F$ : (a)$g = 1$ ; (b)$g = 1.003$ ; (c)$g = 1.005$ ; (d)$g = 1.01$ . -
[1] Xu F, Ma X, Zhang Q, Lo H K, Pan J W 2020 Rev. Mod. Phys. 92 025002
Google Scholar
[2] Pirandola S, Andersen U L, Banchi L, et al. 2020 Adv. Opt. Photon. 12 1012
Google Scholar
[3] Liu H, Jiang C, Zhu H T, et al. 2021 Phys. Rev. Lett. 126 250502
Google Scholar
[4] Lo H K, Chau H F 1999 Science 283 2050
Google Scholar
[5] Shor P W, Preskill J 2000 Phys. Rev. Lett. 85 441
Google Scholar
[6] Yin J, Li Y H, Liao S K, et al. 2020 Nature 582 501
Google Scholar
[7] Fang X T, Zeng P, Liu H, et al. 2020 Nat. Photonics 14 422
Google Scholar
[8] Chen J P, Zhang C, Liu Y, et al. 2021 Nat. Photonics 15 570
Google Scholar
[9] Laudenbach F, Pacher C, Fung C H F, Poppe A, Peev M, Schrenk B, Hentschel M, Walther P, Hübel H 2018 Adv. Quantum Technol. 1 1800011
Google Scholar
[10] Wu X D, Wang Y J, Huang D, Guo Y 2020 Front. Phys. 15 31601
Google Scholar
[11] Grosshans F, Grangier P 2002 Phys. Rev. Lett. 88 057902
Google Scholar
[12] 钟海, 叶炜, 吴晓东, 郭迎 2021 70 020301
Google Scholar
Zhong H, Ye W, Wu X D, Guo Y 2021 Acta Phys. Sin. 70 020301
Google Scholar
[13] Grosshans F, Assche G V, Wenger J, Brouri R, Cerf N J, Grangier P 2003 Nature (London) 421 238
[14] Huang D, Huang P, Lin D, Zeng G 2016 Sci. Rep. 6 19201
Google Scholar
[15] Jouguet P, Kunz-Jacques S, Leverrier A, Grangier P, Diamanti E 2013 Nat. Photonics 7 378
Google Scholar
[16] Huang D, Lin D, Wang C, Liu W, Fang S, Peng J, Huang P, Zeng G 2015 Opt. Express 23 17511
Google Scholar
[17] Zhang Y, Chen Z, Pirandola S, Wang X, Zhou C, Chu B, Zhao Y, Xu B, Yu S, Guo H 2020 Phys. Rev. Lett. 125 010502
Google Scholar
[18] Huang D, Huang P, Li H, Wang T, Zhou Y, Zeng G 2016 Opt. Lett. 41 3511
Google Scholar
[19] Ma X C, Sun S H, Jiang M S, Liang L M 2013 Phys. Rev. A 88 022339
Google Scholar
[20] Ma X C, Sun S H, Jiang M S, Liang L M 2013 Phys. Rev. A 87 052309
Google Scholar
[21] Jouguet P, Kunz-Jacques S, Diamanti E 2013 Phys. Rev. A 87 062313
Google Scholar
[22] Qin H, Kumar R, Alléaume R 2016 Phys. Rev. A 94 012325
Google Scholar
[23] Qi B, Lougovski P, Pooser R, Grice W, Bobrek M 2015 Phys. Rev. X 5 041009
Google Scholar
[24] Soh D B S, Brif C, Coles P J, Lütkenhaus N, Camacho R M, Urayama J, Sarovar M 2015 Phys. Rev. X 5 041010
Google Scholar
[25] Huang D, Lin D K, Huang P, Zeng G H 2015 Opt. Lett. 40 3695
Google Scholar
[26] Marie A, Alléaume R 2017 Phys. Rev. A 95 012316
Google Scholar
[27] Wang T, Huang P, Zhou Y, Liu W, Zeng G 2018 Phys. Rev. A 97 012310
Google Scholar
[28] Wu X, Wang Y, Guo Y, Zhong H, Huang D 2021 Phys. Rev. A 103 032604
Google Scholar
[29] Huang D, Huang P, Wang T, Li H, Zhou Y, Zeng G 2016 Phys. Rev. A 94 032305
Google Scholar
[30] Silberhorn C, Ralph T C, Lütkenhaus N, Leuchs G 2002 Phys. Rev. Lett. 89 167901
Google Scholar
[31] Leverrier A, Grangier P 2009 Phys. Rev. Lett. 102 180504
Google Scholar
[32] Becerra F E, Fan J, Baumgartner G, Goldhar J, Kosloski J T, Migdall A 2013 Nat. Photonics 7 147
Google Scholar
[33] Becerra F E, Fan J, Migdall A 2013 Nat. Commun. 4 2028
Google Scholar
[34] Becerra F E, Fan J, Baumgartner G, Polyakov S V, Goldhar J, Kosloski J T, Migdall A 2011 Phys. Rev. A 84 062324
Google Scholar
[35] Helstrom C W 1976 Quantum Detection and Estimation Theory (Mathematics in Science and Engineering) (Vol. 123) (New York: Academic)
[36] Liao Q, Guo Y, Huang D, Huang P, Zeng G 2018 New J. Phys. 20 023015
Google Scholar
[37] Shen Y, Peng X, Yang J, Guo H 2011 Phys. Rev. A 83 052304
Google Scholar
[38] Wu X D, Wang Y J, Zhong H, Liao Q, Guo Y 2019 Front. Phys. 14 41501
Google Scholar
[39] Wu X, Wang Y, Zhong H, Ye W, Huang D, Guo Y 2020 Quantum Inf. Process. 19 234
Google Scholar
[40] Navascués M, Acín A 2005 Phys. Rev. Lett. 94 020505
Google Scholar
[41] García-Patrón R, Cerf N J 2006 Phys. Rev. Lett. 97 190503
Google Scholar
[42] Pirandola S, Braunstein S L, Lloyd S 2008 Phys. Rev. Lett. 101 200504
Google Scholar
[43] Renner R, Cirac J I 2009 Phys. Rev. Lett. 102 110504
Google Scholar
[44] Leverrier A, Grosshans F, Grangier P 2010 Phys. Rev. A 81 062343
Google Scholar
[45] Pirandola S, Laurenza R, Ottaviani C, Banchi L 2017 Nat. Commun. 8 15043
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 3780
- PDF Downloads: 74
- Cited By: 0
