-
As is well known, large-scale type-I edge localized modes (ELMs) may pose serious risks to machine components in future large fusion devices. The resonant magnetic perturbation (RMP), generated by magnetic coils external to the plasma, can either suppress or mitigate ELMs, as has been shown in recent experiments on several present-day fusion devices. Understanding the ELM control with RMP may involve various physics. This work focuses on the understanding of the roles played by three key physical quantities: the edge safety factor, the RMP coil current, and the particle drift kinetic effects resulting from thermal and fusion-born α-particles. Full toroidal computations are performed by using the MARS-F/K codes. The results show that the plasma response based figures-of-merit i.e. the pitch resonant radial field component near the plasma edge and the plasma displacement near the X-point of the separatrix,consistently yield the same periodic amplification as
$ q_{95} $ varies. The number of peaks, y, is positively correlated with the toroidal number n, i.e.$y \approx n\Delta {q_{95}}$ with$\Delta {q_{95}} = 3.5$ . The peak window in$ q_{95} $ occurs when a new resonant surface passes through a specific region of the plasma edge. Two-dimensional parameter scans, for the edge safety factor and the coil phasing between the upper and lower rows of coils, yield a linear relationship between the optimal/worst current phase difference and$ q_{95} $ , which can be well fitted by a simple analytic model. The optimal value of coil current amplitude is sensitive to n. Compared with the same current amplitude assumed for the two/three rows of coils, the optimal current amplitude can increase the${\xi _{\text{X}}}$ but does not change the prediction of the relative toroidal phase difference. More advanced response model, including kinetic resonances between the RMP perturbation and drift motions of thermal particles and fusion-born alphas, shows that the modification of kinetic effects should be considered in order to better describe the plasma response to RMP fields in high-β plasmas. The fluid response model with a strong parallel sound wave damping (${\kappa _\parallel } = 1.5$ ) can well predict the plasma response for the ‘DEMO-like’ equilibria. For low β plasma, the kinetic response is consistent with the fluid response, whether a strong parallel sound wave damping exists or not.-
Keywords:
- resonant magnetic perturbation /
- plasma response /
- coil current optimization /
- drift kinetic effects
[1] Suttrop W 2000 Plasma Phys. Contr. F. 42 A1
Google Scholar
[2] Loarte A, Lipschultz B, Kukushkin A S, Matthews G F, Stangeby P C, Asakura N, Counsell G F, Federici G, Kallenbach A, Krieger K, Mahdavi A, Philipps V, Reiter D, Roth J, Strachan J, Whyte D, Doerner R, Eich T, Fundamenski W, Herrmann A, Fenstermacher M, Ghendrih P, Groth M, Kirschner A, Konoshima S, LaBombard B, Lang P, Leonard A W, Monier-Garbet P, Neu R, Pacher H, Pegourie B, Pitts R A, Takamura S, Terry J, Tsitrone E, Group D P T 2007 Nucl. Fusion 47 S203
Google Scholar
[3] Ryter F, Fuchs J, Schneider W, Sips A, Stäbler A, Stober J, Team A U 2008 J. Phys. Conf. Ser. 123 012035
Google Scholar
[4] Loarte A, Saibene G, Sartori R, Becoulet M, Horton L, Eich T, Herrmann A, Laux M, Matthews G, Jachmich S, Asakura N, Chankin A, Leonard A, Porter G, Federici G, Shimada M, Sugihara M, Janeschitz G 2003 J. Nucl. Mater 313 962
Google Scholar
[5] Wenninger R P, Bernert M, Eich T, Fable E, Federici G, Kallenbach A, Loarte A, Lowry C, McDonald D, Neu R, Pütterich T, Schneider P, Sieglin B, Strohmayer G, Reimold F, Wischmeier M 2014 Nucl. Fusion 54 114003
Google Scholar
[6] Liang Y, Koslowski H R, Thomas P R, Nardon E, Alper B, Andrew P, Andrew Y, Arnoux G, Baranov Y, Becoulet M, Beurskens M, Biewer T, Bigi M, Crombe K, De La Luna E, de Vries P, Fundamenski W, Gerasimov S, Giroud C, Gryaznevich M P, Hawkes N, Hotchin S, Howell D, Jachmich S, Kiptily V, Moreira L, Parail V, Pinches S D, Rachlew E, Zimmermann O 2007 Phys. Rev. Lett. 98 265004
Google Scholar
[7] Suttrop W, Eich T, Fuchs J C, Gunter S, Janzer A, Herrmann A, Kallenbach A, Lang P T, Lunt T, Maraschek M, McDermott R M, Mlynek A, Putterich T, Rott M, Vierle T, Wolfrum E, Yu Q, Zammuto I, Zohm H, Team A U 2011 Phys. Rev. Lett. 106 225004
Google Scholar
[8] Evans T E, Moyer R A, Thomas P R, Watkins J G, Osborne T H, Boedo J A, Doyle E J, Fenstermacher M E, Finken K H, Groebner R J, Groth M, Harris J H, La Haye R J, Lasnier C J, Masuzaki S, Ohyabu N, Pretty D G, Rhodes T L, Reimerdes H, Rudakov D L, Schaffer M J, Wang G, Zeng L 2004 Phys. Rev. Lett. 92 235003
Google Scholar
[9] Jeon Y M, Park J K, Yoon S W, Ko W H, Lee S G, Lee K D, Yun G S, Nam Y U, Kim W C, Kwak J G, Lee K S, Kim H K, Yang H L, Team K 2012 Phys. Rev. Lett. 109 035004
Google Scholar
[10] Kirk A, Suttrop W, Chapman I T, Liu Y, Scannell R, Thornton A J, Orte L B, Cahyna P, Eich T, Fischer R, Fuchs C, Ham C, Harrison J R, Jakubowski M W, Kurzan B, Pamela S, Peterka M, Ryan D, Saarelma S, Sieglin B, Valovic M, Willensdorfer M 2015 Nucl. Fusion 55 043011
Google Scholar
[11] Liang Y, Koslowski H R, Thomas P R, Nardon E, Alper B, Andrew P, Andrew Y, Arnoux G, Baranov Y, Bécoulet M, Beurskens M, Biewer T, Bigi M, Crombe K, De La Luna E, de Vries P, Fundamenski W, Gerasimov S, Giroud C, Gryaznevich M P, Hawkes N, Hotchin S, Howell D, Jachmich S, Kiptily V, Moreira L, Parail V, Pinches S D, Rachlew E, Zimmermann O 2007 Physical Review Letters 98 265004
[12] Sun Y, Liang Y, Liu Y Q, Gu S, Yang X, Guo W, Shi T, Jia M, Wang L, Lyu B, Zhou C, Liu A, Zang Q, Liu H, Chu N, Wang H H, Zhang T, Qian J, Xu L, He K, Chen D, Shen B, Gong X, Ji X, Wang S, Qi M, Song Y, Yuan Q, Sheng Z, Gao G, Fu P, Wan B 2016 Phys. Rev. Lett. 117 115001
Google Scholar
[13] Wan B N, Liang Y, Gong X Z, Xiang N, Xu G S, Sun Y, Wang L, Qian J P, Liu H Q, Zeng L, Zhang L, Zhang X J, Ding B J, Zang Q, Lyu B, Garofalo A M, Ekedahl A, Li M H, Ding F, Ding S Y, Du H F, Kong D F, Yu Y, Yang Y, Luo Z P, Huang J, Zhang T, Zhang Y, Li G Q, Xia T Y 2019 Nucl. Fusion 59 112003
Google Scholar
[14] Duan X R, Liu Y, Xu M, Yan L W, Xu Y, Song X M, Dong J Q, Ding X T, Chen L Y, Lu B, Liu D Q, Rao J, Xuan W M, Yang Q W, Zheng G Y, Zou X L, Liu Y Q, Zhong W L, Zhao K J, Ji X Q, Mao W C, Wang Q M, Li Q, Cao J Y, Cao Z, Lei G J, Zhang J H, Li X D, Bai X Y, Cheng J, Chen W, Cui Z Y, Delpech L, Diamond P H, Dong Y B, Ekedahl A, Hoang T, Huang Y, Ida K, Itoh K, Itoh S I, Isobe M, Inagaki S, Mazon D, Morita S, Peysson Y, Shi Z B, Wang X G, Xiao G L, Yu D L, Yu L M, Zhang Y P, Zhou Y, Cui C H, Feng B B, Huang M, Li Y G, Li B, Li G S, Li H J, Li Q, Peng J F, Wang Y Q, Yuan B S, Liu Y 2017 Nucl. Fusion 57 102013
Google Scholar
[15] Ding Y, Chen Z, Chen Z, Yang Z, Wang N, Hu Q, Rao B, Chen J, Cheng Z, Gao L, Jiang Z, Wang L, Wang Z, Zhang X, Zheng W, Zhang M, Zhuang G, Yu Q, Liang Y, Yu K, Hu X, Pan Y, Gentle K W, Team J T 2018 Plasma Sci. Technol. 20 125101
Google Scholar
[16] Haskey S R, Lanctot M J, Liu Y Q, Hanson J M, Blackwell B D, Nazikian R 2014 Plasma Phys. Contr. F. 56 035005
Google Scholar
[17] Schaffer M J, Menard J E, Aldan M P, Bialek J M, Evans T E, Moyer R A 2008 Nucl. Fusion 48 024004
Google Scholar
[18] Liu Y Q, Kirk A, Sun Y, Cahyna P, Chapman I T, Denner P, Fishpool G, Garofalo A M, Harrison J R, Nardon E 2012 Plasma Phys. Contr. F. 54 124013
Google Scholar
[19] Liu Y, Li L, Loarte A, Pinches S D, Polevoi A 2021 Nucl. Fusion 61 106029
Google Scholar
[20] 苏春燕, 牟茂淋, 陈少永, 郭文平, 唐昌建 2021 70 095207
Google Scholar
Su C Y, Mou M L, Chen S Y, Guo W P, Tang C J 2021 Acta Phys. Sin. 70 095207
Google Scholar
[21] Zhou L, Liu Y, Liu Y, Yang X 2016 Plasma Phys. Contr. F. 58 115003
Google Scholar
[22] 陈撷宇, 牟茂淋, 苏春燕, 陈少永, 唐昌建 2020 69 195201
Google Scholar
Chen X Y, Mou M L, Su C Y, Chen S Y, Tang C J 2020 Acta Phys. Sin. 69 195201
Google Scholar
[23] 李春雨, 郝广周, 刘钺强, 王炼, 刘艺慧子 2022 71 075202
Google Scholar
Li C Y, Hao G Z, Liu Y Q, Wang L, Liu Y H Z 2022 Acta Phys. Sin. 71 075202
Google Scholar
[24] Liu Y, Chu M S, Chapman I T, Hender T C 2008 Phys. Plasmas 15 112503
Google Scholar
[25] Liu Y, Kirk A, Nardon E 2010 Phys. Plasmas 17 122502
Google Scholar
[26] Sun Y, Liang Y, Liu Y Q, Gu S, Yang X, Guo W, Shi T, Jia M, Wang L, Lyu B, Zhou C, Liu A, Zang Q, Liu H, Chu N, Wang H H, Zhang T, Qian J, Xu L, He K, Chen D, Shen B, Gong X, Ji X, Wang S, Qi M, Song Y, Yuan Q, Sheng Z, Gao G, Fu P, Wan B 2016 Physical Review Letters 117 115001
[27] Liu Y Q, Bondeson A, Fransson C M, Lennartson B, Breitholtz C 2000 Phys. Plasmas 7 3681
Google Scholar
[28] Liu Y, Kirk A, Gribov Y, Gryaznevich M P, Hender T C, Nardon E 2011 Nucl. Fusion 51 083002
Google Scholar
[29] Wang Z R, Lanctot M J, Liu Y Q, Park J K, Menard J E 2015 Phys. Rev. Lett. 114 145005
Google Scholar
[30] Li L, Liu Y Q, Kirk A, Wang N, Liang Y, Ryan D, Suttrop W, Dunne M, Fischer R, Fuchs J C, Kurzan B, Piovesan P, Willensdorfer M, Zhong F C 2016 Nucl. Fusion 56 126007
Google Scholar
[31] Yang X, Sun Y, Liu Y, Gu S, Liu Y, Wang H, Zhou L, Guo W 2016 Plasma Phys. Contr. F. 58 114006
Google Scholar
[32] Liu Y, Kirk A, Li L, In Y, Nazikian R, Sun Y, Suttrop W, Lyons B, Ryan D, Wang S, Yang X, Zhou L 2017 Phys. Plasmas 24 056111
Google Scholar
[33] Bondeson A, Iacono R 1989 Phys. Fluids B Plasma Phys. 1 1431
Google Scholar
[34] Liu Y, Chapman I T, Saarelma S, Gryaznevich M P, Hender T C, Howell D F 2009 Plasma Phys. Contr. F. 51 115005
Google Scholar
[35] Liu Y, Ryan D, Kirk A, Li L, Suttrop W, Dunne M, Fischer R, Fuchs J C, Kurzan B, Piovesan P, Willensdorfer M 2016 Nucl. Fusion 56 056015
Google Scholar
[36] Wang Z, Park J-K, Menard J E, Liu Y, Kaye S M, Gerhardt S 2018 Nucl. Fusion 58 016015
Google Scholar
[37] Hu Q M, Nazikian R, Grierson B A, Logan N C, Paz-Soldan C, Yu Q 2020 Nucl. Fusion 60 076001
Google Scholar
[38] Wang S X, Liu H Q, Jie Y X, Ding W X, Zeng L, Sun Y W, Lian H, Zhu X, Zou Z Y, Lyu B, Li Y Y, Zang Q, Du H F, Zhou C, Liu A D, Zhang T, Gao W, Gao X 2018 Nucl. Fusion 58 112013
Google Scholar
[39] Zhou L, Liu Y, Wenninger R, Liu Y, Wang S, Yang X 2018 Nucl. Fusion 58 076025
Google Scholar
[40] Li L, Liu Y Q, Wang N, Kirk A, Koslowski H R, Liang Y, Loarte A, Ryan D, Zhong F C 2017 Plasma Phys. Contr. F. 59 044005
Google Scholar
[41] Wenninger R, Kembleton R, Bachmann C, Biel W, Bolzonella T, Ciattaglia S, Cismondi F, Coleman M, Donné A J H, Eich T, Fable E, Federici G, Franke T, Lux H, Maviglia F, Meszaros B, Pütterich T, Saarelma S, Snickers A, Villone F, Vincenzi P, Wolff D, Zohm H 2017 Nucl. Fusion 57 016011
Google Scholar
[42] Lütjens H, Bondeson A, Sauter O 1996 Comput. Phys. Commun. 97 219
Google Scholar
[43] Liu Y, Akers R, Chapman I T, Gribov Y, Hao G Z, Huijsmans G T A, Kirk A, Loarte A, Pinches S D, Reinke M, Ryan D, Sun Y, Wang Z R 2015 Nucl. Fusion 55 063027
Google Scholar
[44] Fenstermacher M E, Evans T E, Osborne T H, Schaffer M J, Aldan M P, deGrassie J S, Gohil P, Joseph I, Moyer R A, Snyder P B, Groebner R J, Jakubowski M, Leonard A W, Schmitz O, Team D 2008 Phys. Plasmas 15 056122
Google Scholar
[45] Gu S, Wan B, Sun Y, Chu N, Liu Y Q, Shi T, Wang H H, Jia M, He K 2019 Nucl. Fusion 59 126042
Google Scholar
[46] Nazikian R, Petty C C, Bortolon A, Chen X, Eldon D, Evans T E, Grierson B A, Ferraro N M, Haskey S R, Knolker M, Lasnier C, Logan N C, Moyer R A, Orlov D, Osborne T H, Paz-Soldan C, Turco F, Wang H Q, Weisberg D B 2018 Nucl. Fusion 58 106010
Google Scholar
[47] Hu Q M, Nazikian R, Logan N C, Park J K, Paz-Soldan C, Yang S M, Grierson B A, In Y, Jeon Y M, Kim M, Kim S K, Orlov D M, Park G Y, Yu Q 2021 Phys. Plasmas 28 052505
Google Scholar
[48] Federici G, Bachmann C, Barucca L, Baylard C, Biel W, Boccaccini L V, Bustreo C, Ciattaglia S, Cismondi F, Corato V, Day C, Diegele E, Franke T, Gaio E, Gliss C, Haertl T, Ibarra A, Holden J, Keech G, Kembleton R, Loving A, Maviglia F, Morris J, Meszaros B, Moscato I, Pintsuk G, Siccinio M, Taylor N, Tran M Q, Vorpahl C, Walden H, You J H 2019 Nucl. Fusion 59 066013
Google Scholar
[49] Zhou L, Liu Y, Hu H, Siccinio M, Maviglia F, Zohm H, Pigatto L, Wang Y, Li L, Hao G Z, Yang X, Zhang H, Duan P, Chen L 2023 Plasma Phys. Contr. F. 65 035008
Google Scholar
[50] Hu B, Betti R, Manickam J 2006 Phys. Plasmas 13 112505
Google Scholar
[51] Zhou L, Liu Y, Siccinio M, Fable E, Wu T, Kurki-Suonio T, Varje J, Liu D 2021 Plasma Phys. Contr. F. 63 065007
Google Scholar
-
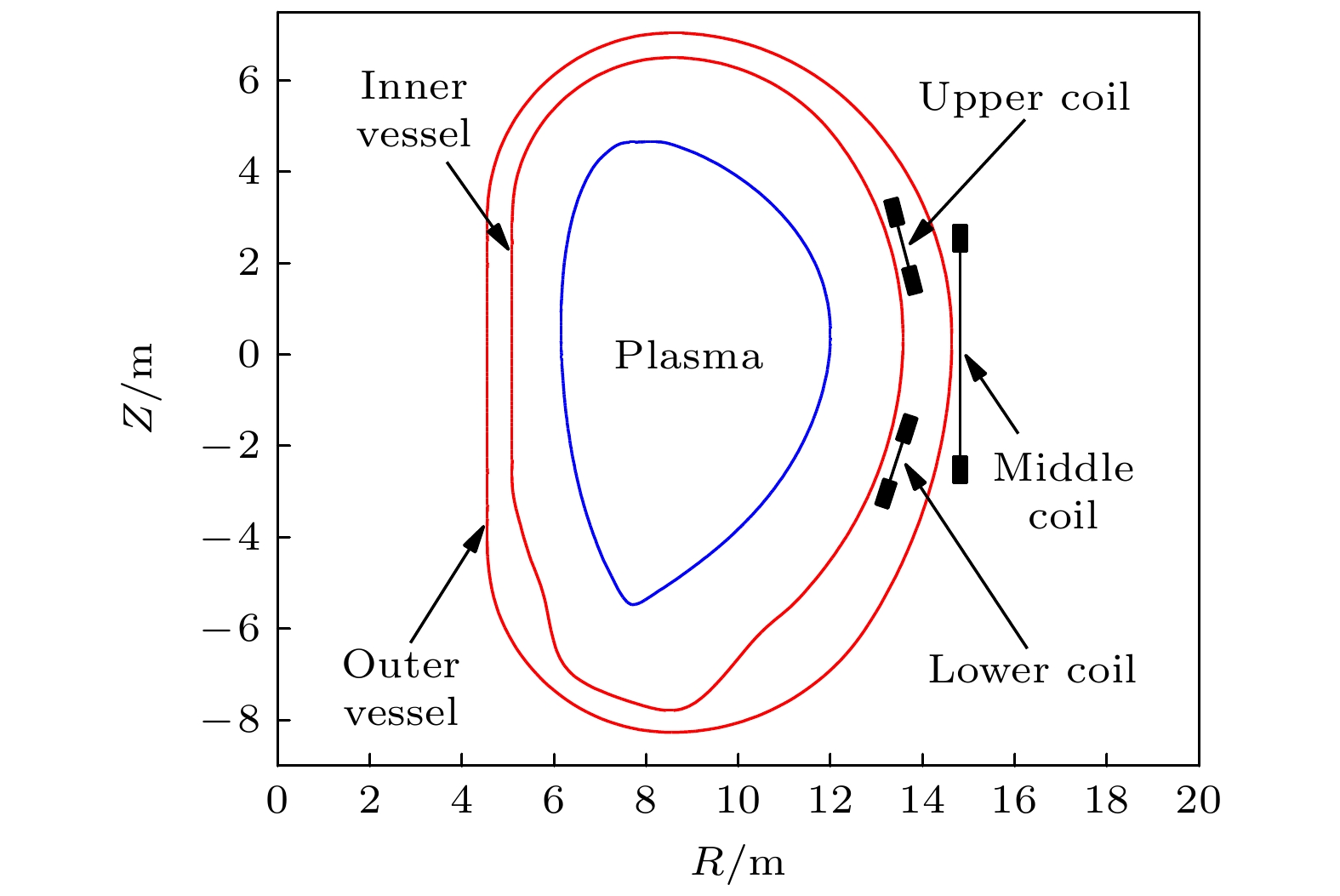
图 1 等离子体平衡的径向剖面 (a)在固定的磁场下扫描等离子体电流时安全因子剖面的变化; (b)由
${{B_0^2} \mathord{\left/ {\vphantom {{B_0^2} {{\mu _0}}}} \right. } {{\mu _0}}}$ 归一化的压强剖面; (c)磁轴处归一化为1的密度剖面; (d)由磁轴处阿尔芬频率${\omega _A} = {{{B_0}} \mathord{\left/ {\vphantom {{{B_0}} {\left[ {{R_0}\left( {{\mu _0}{\rho _0}} \right)} \right]}}} \right. } {\left[ {{R_0}\left( {{\mu _0}{\rho _0}} \right)} \right]}}$ 归一化的旋转频率剖面Figure 1. Plasma equilibrium radial profiles: (a) Variation of the equilibrium safety factor profile while scanning the plasma current at fixed field; (b) the plasma pressure normalized by
${{B_0^2} \mathord{\left/ {\vphantom {{B_0^2} {{\mu _0}}}} \right. } {{\mu _0}}}$ ; (c) the plasma density normalized to unity at the magnetic axis; (d) the (assumed) plasma toroidal rotation frequencies normalized by the on-axis Alfven frequency${\omega _A} = {{{B_0}} \mathord{\left/ {\vphantom {{{B_0}} {\left[ {{R_0}\left( {{\mu _0}{\rho _0}} \right)} \right]}}} \right. } {\left[ {{R_0}\left( {{\mu _0}{\rho _0}} \right)} \right]}}$ .
图 2 等离子体的边界(蓝线)、真空双壁(红线)和RMP线圈的几何位形(黑线)
Figure 2. Plasma boundary (bule line), double wall shapes (red lines) and RMP coil geometry (black lines).
图 3 在环向模数 (a)
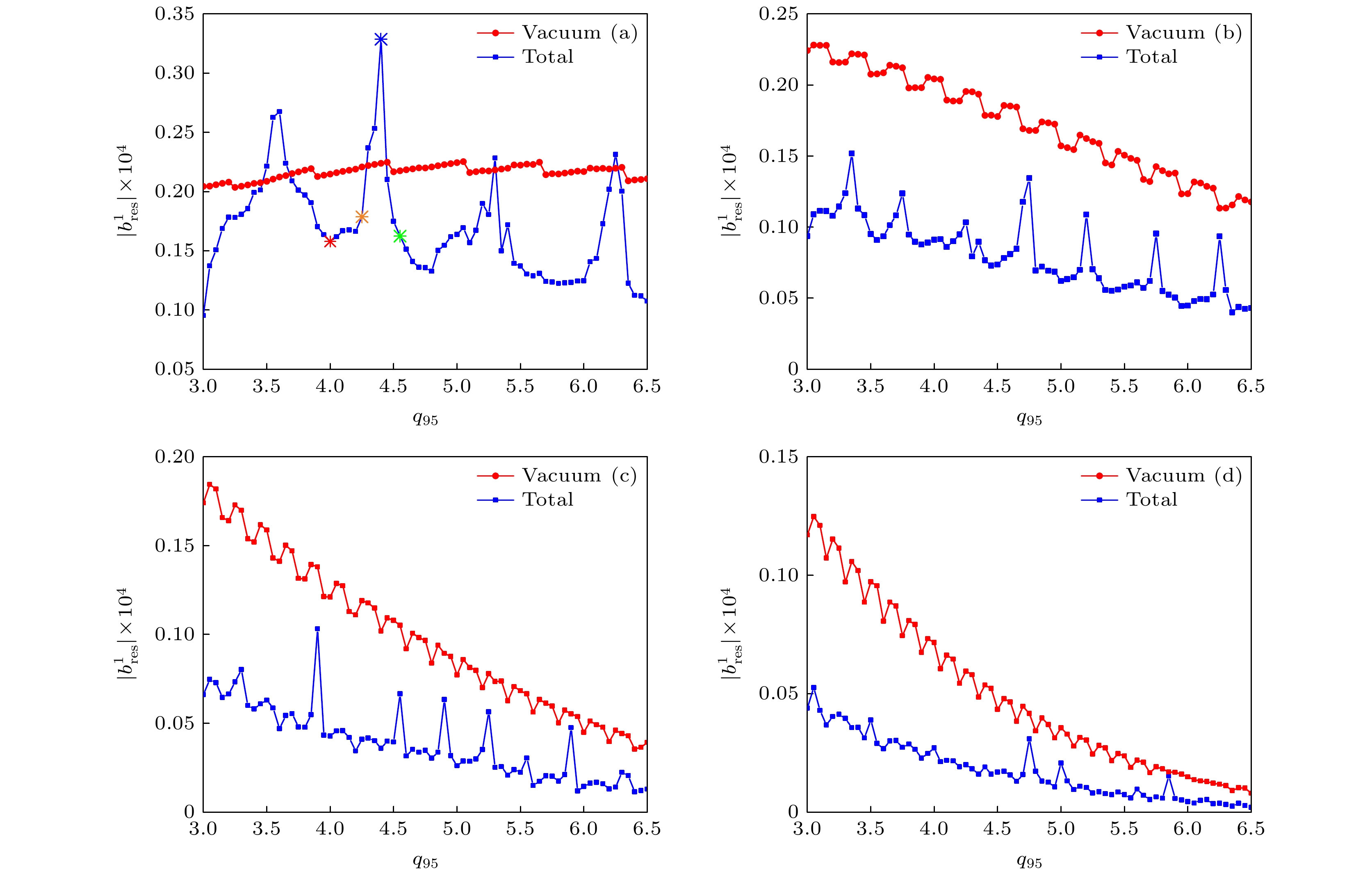
$n = 1$ , (b)$n = 2$ , (c)$n = 3$ 和(d)$n = 4$ 线圈位形下, 中线圈在最外层有理面处产生的径向扰动场幅值$ \left| {b_{{\text{res}}}^{\text{1}}} \right| $ 随安全因子${q_{95}}$ 的变化. 中线圈的极向宽度和电流分别为$ \Delta {\theta ^{\text{M}}} = 4{{\text{5}}^{\circ}} $ 和$ {I^{\text{M}}} = 90{\text{ kAt}} $ Figure 3. Comparison of the computed amplitude of the outermost pitch resonant radial field components
$ \left| {b_{{\text{res}}}^{\text{1}}} \right| $ , between the vacuum field (solid) and the total field including the plasma response (dashed), versus the edge safety factor q95, for the (a) n = 1, (b) n = 2, (c) n = 3 and (d) n = 4 coil configurations, respectively, using the middle row of RMP coils with the poloidal width$ \Delta {\theta ^{\text{M}}} = 4{{\text{5}}^{\circ}} $ and current$ {I^{\text{M}}} = 90{\text{ kAt}} $ .
图 4 在环向模数 (a)
$n = 1$ , (b)$n = 2$ , (c)$n = 3$ 和(d)$n = 4$ 线圈位形下, 中线圈在X点附近产生的扰动位移幅值$\left| {{\xi _X}} \right|$ 随安全因子${q_{95}}$ 的变化, 中线圈的极向宽度和电流分别为$ \Delta {\theta ^{\text{M}}} = 4{{\text{5}}^{\circ}} $ 和$ {I^{\text{M}}} = 90{\text{ kAt}} $ Figure 4. The computed amplitude of the plasma surface displacement near the X-point
$\left| {{\xi _X}} \right|$ versus the edge safety factor${q_{95}}$ , using the middle row of RMP coils with the poloidal width$ \Delta {\theta ^{\text{M}}} = 4{{\text{5}}^{\circ}} $ and current$ {I^{\text{M}}} = 90{\text{ kAt}} $ , for the (a)$n = 1$ , (b)$n = 2$ , (c)$n = 3$ and(d)$n = 4$ coil configurations, respectively.
图 5 在环向模数
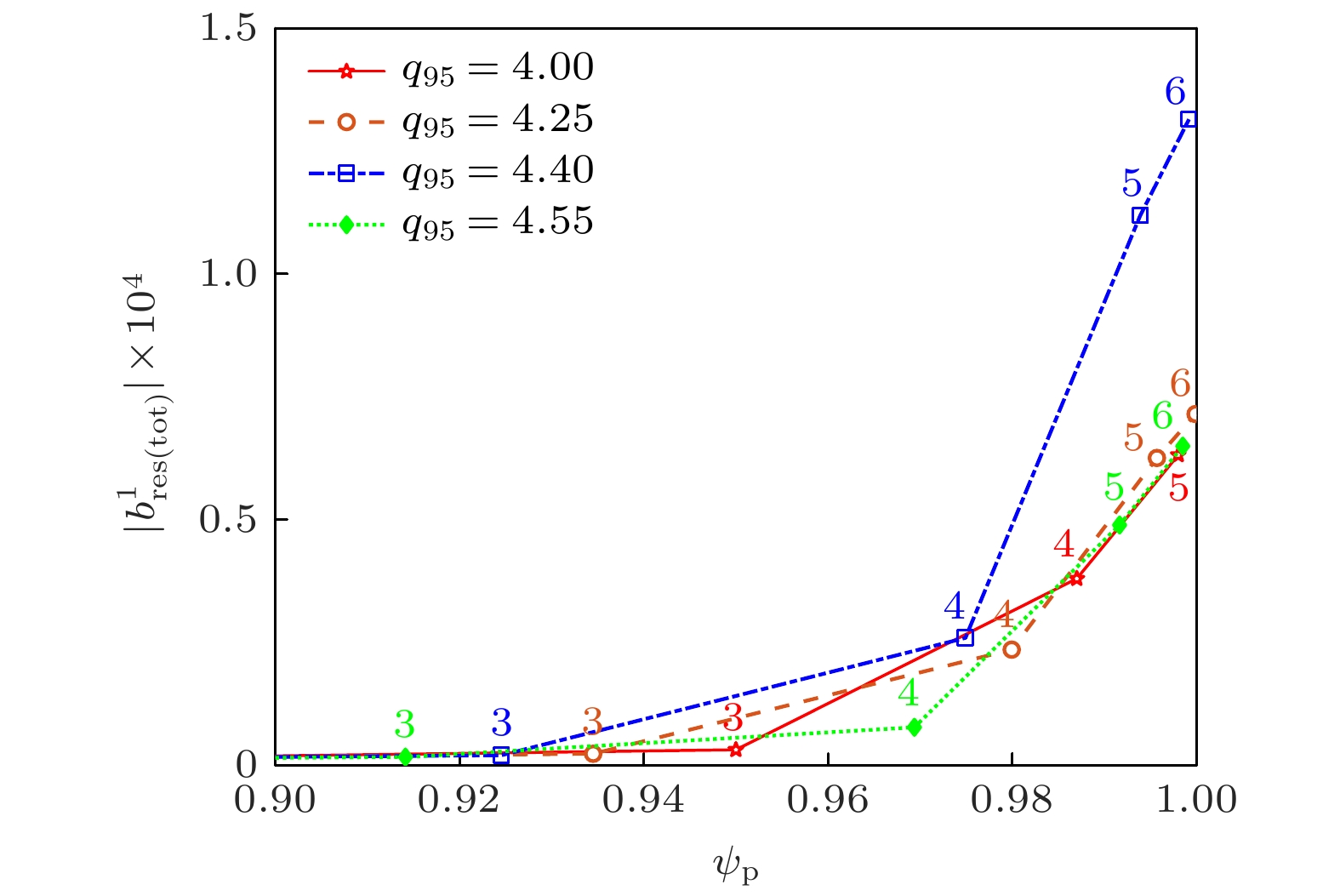
$n = 1$ 线圈位形下,$ {q_{95}} = 4 $ , 4.25, 4.4和4.55时有理面处相应总径向扰动场$b_{{\text{res(tot)}}}^1$ 的对比. 红线(${q_{95}} = 4$ )和蓝线(${q_{95}} = 4.4$ )分别对应最外层有理面处总径向扰动场或X点附近扰动位移的谷值和峰值, 如图3(a)或图4(a)中红点和蓝点所示Figure 5. Comparison of the resonant field amplitude at the corresponding rational surfaces among
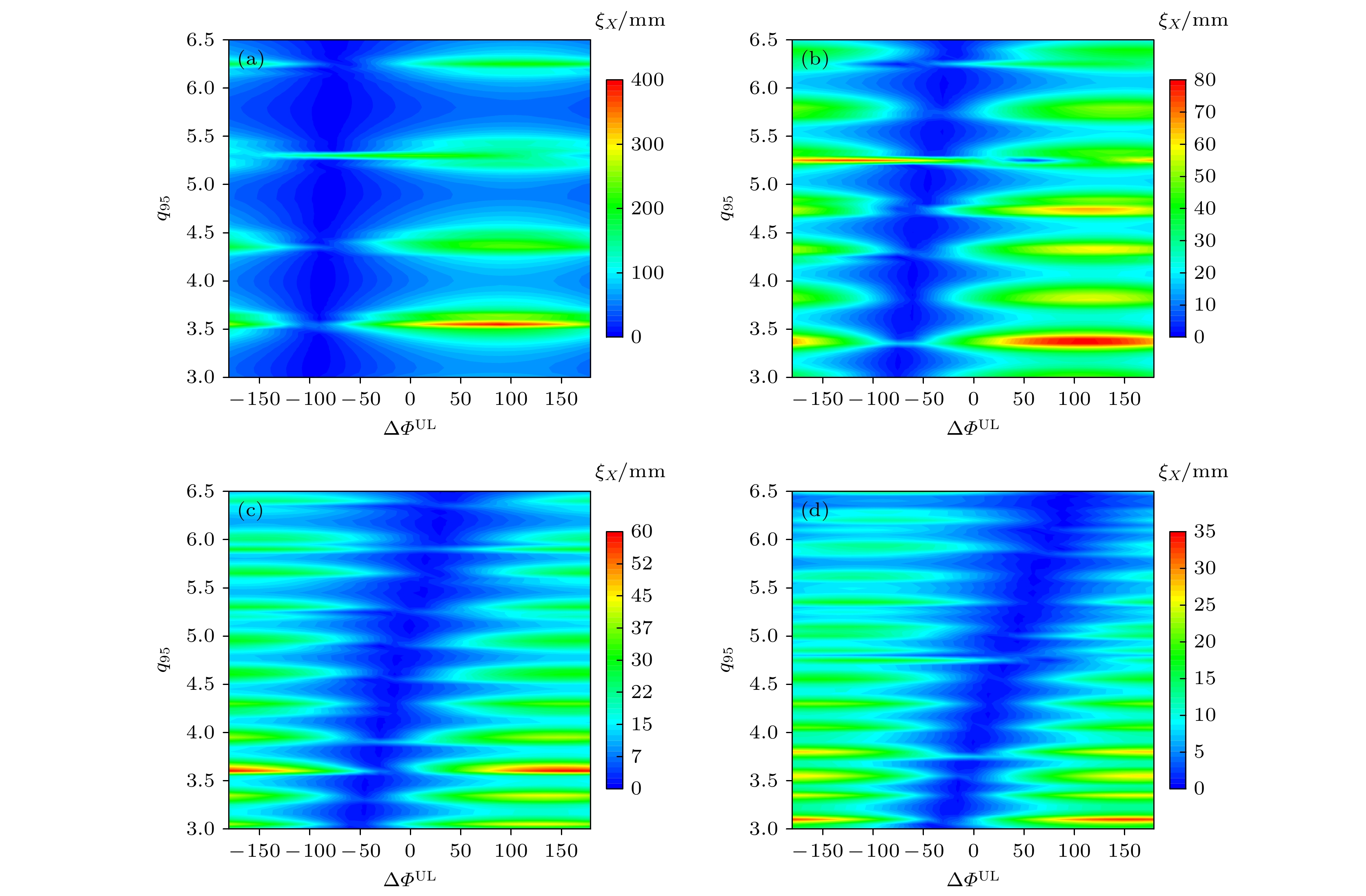
$ {q_{95}} =4, {\text{ }}4.25, {\text{ }}4.4, $ $ {\text{ }}4.55 $ with$n = 1$ . The red line (${q_{95}} = 4$ ) and blue line (${q_{95}} = 4.4$ ) indicate the valley and peak values of the amplitude of the outermost pitch resonant radial field components in Fig. 3(a) or the plasma surface displacement near the X-point in Fig. 4(a), respectively.
图 6 最外侧有理面处归一化的(a)(c)(e)(g)真空径向场和(b)(d)(f)(h)总径向场(包括等离子体响应)随上下两组线圈电流相位差
$ \Delta {\varPhi ^{{\text{UL}}}} = {\varPhi ^{\text{U}}} - {\varPhi ^{\text{L}}} $ 和安全因子${q_{95}}$ 的变化. 环向模数分别为(a)(b)n = 1, (c)(d)n = 2, (e)(f)n = 3和(g)(h)n = 4. 上下两组线圈的极向位置分别为$\theta _{\text{c}}^{\text{U}} = + {20^{\circ}}$ 和$\theta _{\text{c}}^{\text{L}} = - {20^{\circ}}$ , 极向宽度和电流分别为$\Delta {\theta ^{\text{U}}} = \Delta {\theta ^{\text{L}}} = {\text{1}}{{\text{5}}^{\circ}}$ 和$ {I^{\text{U}}} = {I^{\text{L}}} = 90{\text{ kAt}} $ Figure 6. Comparison of the amplitude of the outermost pitch resonant radial field component in 2 D parameter space (
$\Delta \varPhi , {q_{95}}$ ), between the vacuum radial field (left panel) and the total radial field including the plasma response (right panel), for the toroidal number (a)(b) n = 1, (c)(d) n = 2, (e)(f) n = 3, and (g)(h) n = 4, respectively, using upper and lower rows of coils located at$\theta _{\text{c}}^{\text{U}} = + {20^{\circ}}$ and$\theta _{\text{c}}^{\text{L}} = - {20^{\circ}}$ with poloidal width$\Delta {\theta ^{\text{U}}} = \Delta {\theta ^{\text{L}}} = {\text{1}}{{\text{5}}^{\circ}}$ and current$ {I^{\text{U}}} = {I^{\text{L}}} = 90{\text{ kAt}} $ . The field amplitude is linearly scaled to the range of [0, 1] for each q95 value.
图 7 X点附近扰动位移随上下两组线圈电流相位差
$ \Delta {\varPhi ^{{\text{UL}}}} = {\varPhi ^{\text{U}}} - {\varPhi ^{\text{L}}} $ 和安全因子${q_{95}}$ 的变化. 环向模数分别为(a) n = 1, (b) n = 2, (c) n = 3和(d) n = 4.上下两组线圈的极向位置分别为$\theta _{\text{c}}^{\text{U}} = + {20^{\circ}}$ 和$\theta _{\text{c}}^{\text{L}} = - {20^{\circ}}$ , 极向宽度和电流分别为$\Delta {\theta ^{\text{U}}} = \Delta {\theta ^{\text{L}}} = {\text{1}}{{\text{5}}^{\circ}}$ 和$ {I^{\text{U}}} = {I^{\text{L}}} = 90{\text{ kAt}} $ Figure 7. Amplitude of the plasma surface displacement near the X-point, with 2D parameter scan in toroidal phase difference
$ \Delta {\varPhi ^{{\text{UL}}}} = {\varPhi ^{\text{U}}} - {\varPhi ^{\text{L}}} $ (vertical axis) between the two offmidplane rows and q95 (horizontal axis), for the toroidal number (a) n = 1, (b) n = 2, (c) n = 3, and (d) n = 4, respectively, using upper and lower rows of coils located at$\theta _{\text{c}}^{\text{U}} = + {20^{\circ}}$ and$\theta _{\text{c}}^{\text{L}} = - {20^{\circ}}$ with poloidal width$\Delta {\theta ^{\text{U}}} = \Delta {\theta ^{\text{L}}} = {\text{1}}{{\text{5}}^{\circ}}$ and current$ {I^{\text{U}}} = {I^{\text{L}}} = 90{\text{ kAt}} $ .
图 8 在环向模数 (a)
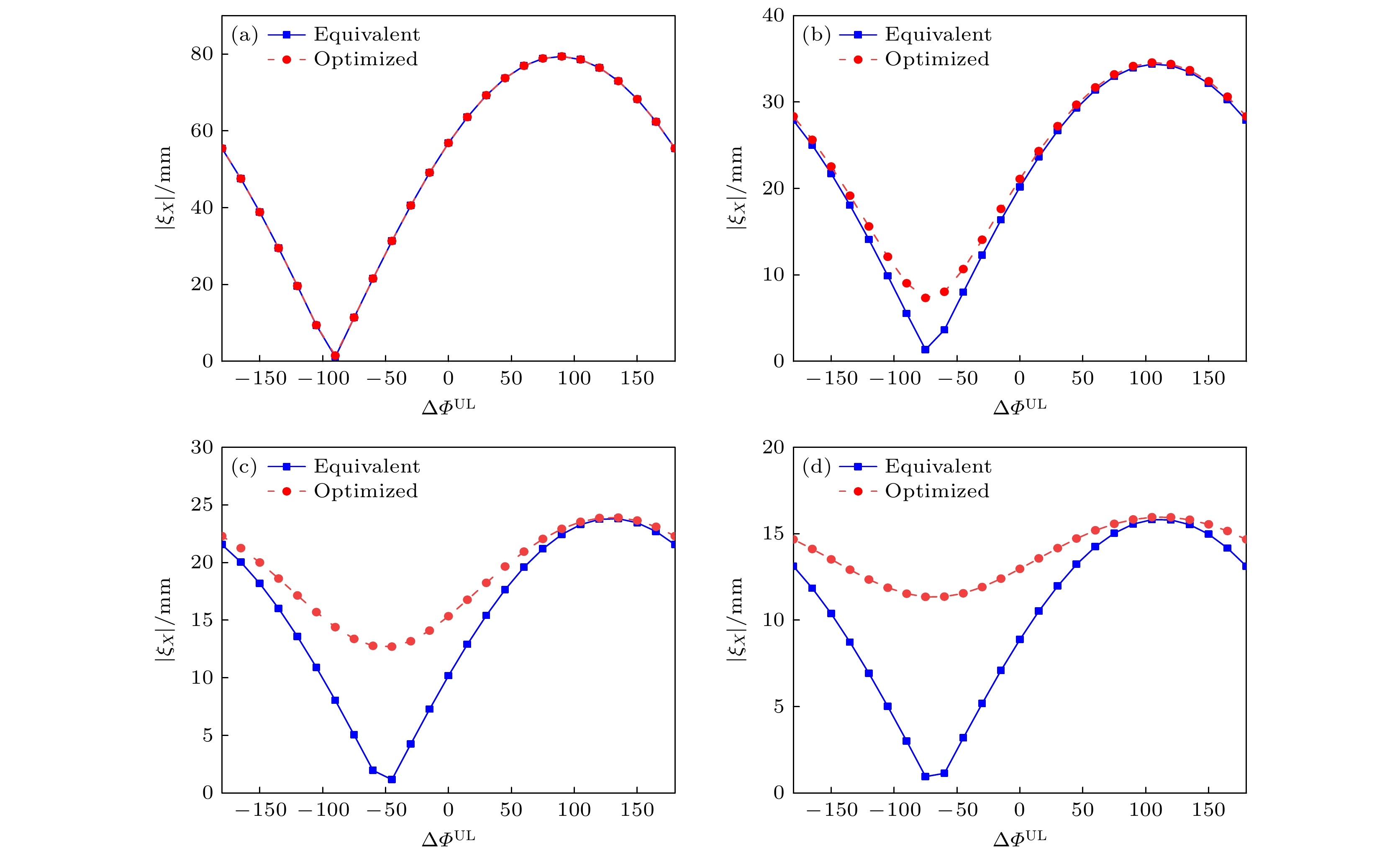
$n = 1$ , (b)$n = 2$ , (c)$n = 3$ 和(d)$n = 4$ 线圈位形下, 比较线圈电流幅值相等和优化两种情况下X点附近扰动位移随上下两组线圈电流相位差$ \Delta {\varPhi ^{{\text{UL}}}} = {\varPhi ^{\text{U}}} - {\varPhi ^{\text{L}}} $ 的变化. 上下两组线圈的极向位置分别为$\theta _{\text{c}}^{\text{U}} = + {20^{\circ}}$ 和$\theta _{\text{c}}^{\text{L}} = - {20^{\circ}}$ , 极向宽度为$\Delta {\theta ^{\text{U}}} = \Delta {\theta ^{\text{L}}} = {\text{1}}{{\text{5}}^{\circ}}$ , 两组线圈的总电流固定为180 kAtFigure 8. Comparison of the amplitude of the plasma surface displacement near the X-point between the equal coil current and optimized coil current, versus the toroidal phase difference
$ \Delta {\varPhi ^{{\text{UL}}}} = {\varPhi ^{\text{U}}} - {\varPhi ^{\text{L}}} $ between the upper and lower rows of coils located at$\theta _{\text{c}}^{\text{U}} = + {20^{\circ}}$ and$\theta _{\text{c}}^{\text{L}} = - {20^{\circ}}$ with poloidal width$\Delta {\theta ^{\text{U}}} = \Delta {\theta ^{\text{L}}} = {\text{1}}{{\text{5}}^{\circ}}$ , for the toroidal number (a) n = 1, (b) n = 2, (c) n = 3, and (d) n = 4, respectively. The total current of the two rows of coils is fixed at 180 kAt.
图 9 在环向模数
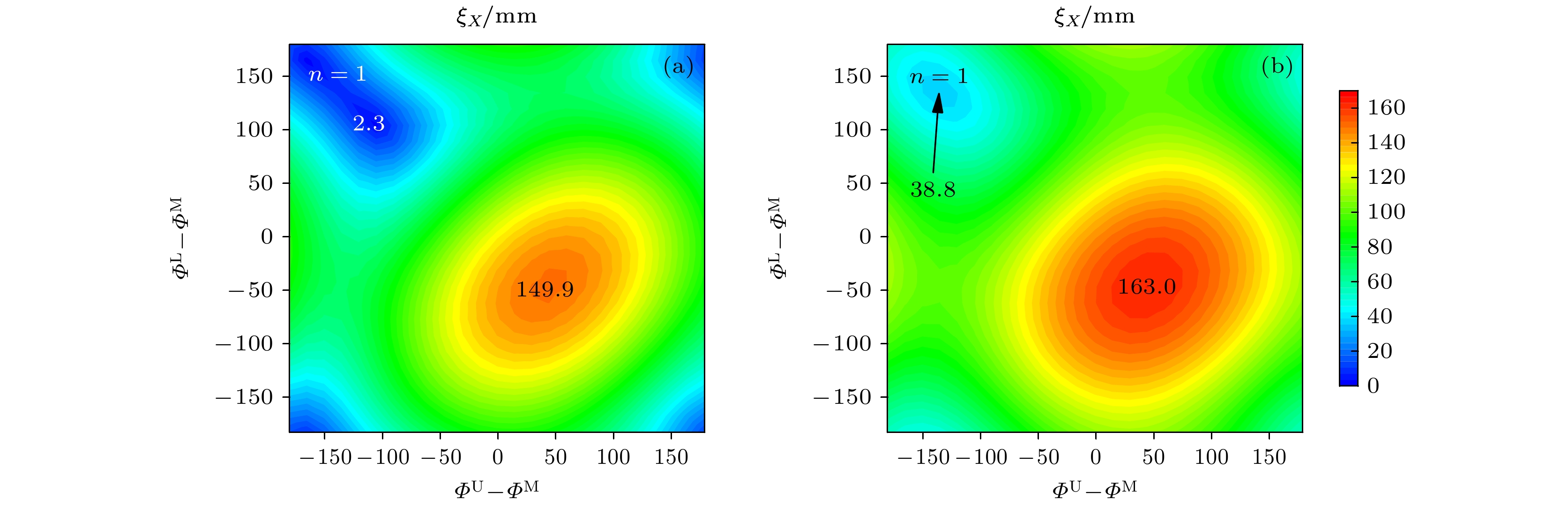
$n = 1$ 线圈位形下, 比较线圈电流幅值(a)相等和(b)优化两种条件下, X点附近扰动位移随上、下两组线圈与中线圈电流相位差($ {\varPhi ^{\text{U}}} - {\varPhi ^{\text{M}}} $ ,$ {\varPhi ^{\text{L}}} - {\varPhi ^{\text{M}}} $ )的变化. 上中下三组线圈的极向位置分别为$\theta _{\text{c}}^{\text{U}} = + {20^{\circ}}$ ,$\theta _{\text{c}}^{\text{M}} = {0^{\circ}}$ 和$\theta _{\text{c}}^{\text{L}} = - {20^{\circ}}$ , 极向宽度为$\Delta {\theta ^{\text{U}}} = \Delta {\theta ^{\text{L}}} = {\text{1}}{{\text{5}}^{\circ}}$ 和$\Delta {\theta ^{\text{M}}} = 4{{\text{5}}^{\circ}}$ , 三组线圈的总电流固定为270 kAtFigure 9. Comparison of the amplitude of the plasma surface displacement near the X-point between the (a) equal coil current and (b) optimized coil current for the toroidal number n=1. The relative toroidal phasing, (
$ {\varPhi ^{\text{U}}} - {\varPhi ^{\text{M}}} $ ,$ {\varPhi ^{\text{L}}} - {\varPhi ^{\text{M}}} $ ), of the upper and lower rows of coil currents with respect to the middle row, is scanned in the 2 D parameter space. The coils located at$\theta _{\text{c}}^{\text{U}} = + {20^{\circ}}$ ,$\theta _{\text{c}}^{\text{M}} = {0^{\circ}}$ and$\theta _{\text{c}}^{\text{L}} = - {20^{\circ}}$ with poloidal width$\Delta {\theta ^{\text{U}}} = \Delta {\theta ^{\text{L}}} = {\text{1}}{{\text{5}}^{\circ}}$ and$\Delta {\theta ^{\text{M}}} = 4{{\text{5}}^{\circ}}$ . The total current of the three rows of coils is fixed at 270 kAt
图 10 在强平行声波阻尼(
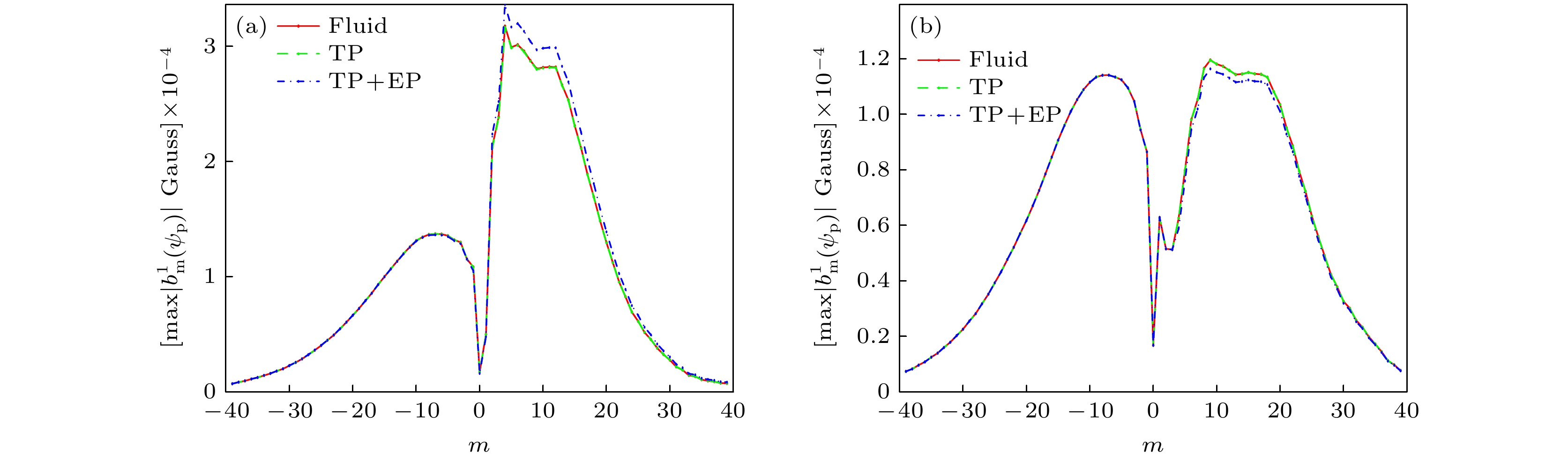
${\kappa _\parallel } = 1.5$ )情况下, 比较流体响应、包含背景粒子的动理学响应(TP)以及包含背景粒子和聚变产生α粒子的动理学响应(TP+EP)对应的n = 1总径向扰动场沿小半径的最大幅值. 对于(a)${q_{95}} = 3.27$ 和(b)${q_{95}} = 6.54$ , 共振谐波分别为m = 1—5和m = 2—10, 其他m为非共振谐波Figure 10. The maximal amplitude (along the minor radius) of all the poloidal Fourier harmonics of the n=1 total radial field. The resonant harmonics are m = 1—5 for (a)
${q_{95}} = 3.27$ and m = 2—10 for (b)${q_{95}} = 6.54$ , and the remaining harmonics are nonresonant. Compared are the response fields obtained assuming the fluid model (fluid), and the MHD-kinetic hybrid model including the non-adiabatic contributions from thermal particles (TP), or both thermal particles and energetic particles (i.e. fusion-born alphas) (EP+TP). A strong parallel sound wave damping model is assumed for both plasmas (${\kappa _\parallel } = 1.5$ ).
图 11 在无平行声波阻尼(
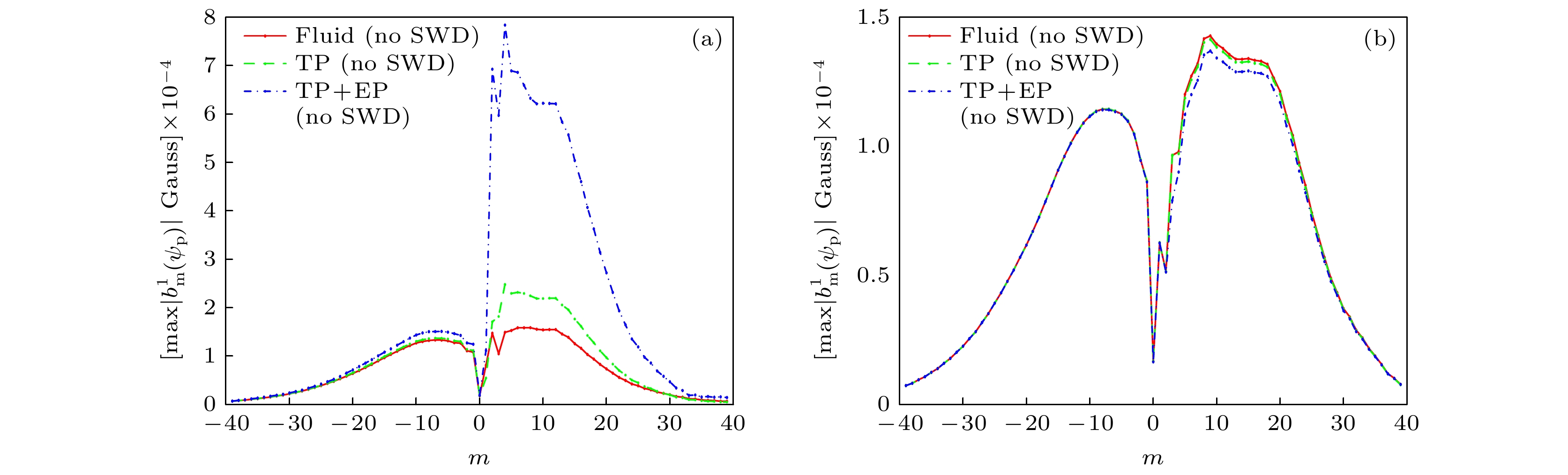
${\kappa _\parallel } = 0$ )情况下, 比较流体响应(Fluid)、包含背景粒子的动理学响应(TP)以及包含背景粒子和聚变产生α粒子的动理学响应(TP+EP)对应的n=1总径向扰动场沿小半径的最大振幅. 对于(a)${q_{95}} = 3.27$ 和(b)${q_{95}} = 6.54$ , 共振谐波分别为m = 1—5和m = 2—10, 其他m为非共振谐波Figure 11. The maximal amplitude (along the minor radius) of all the poloidal Fourier harmonics of the n=1 total radial field. The resonant harmonics are m = 1—5 for (a)
${q_{95}} = 3.27$ and m = 2—10 for (b)${q_{95}} = 6.54$ , and the remaining harmonics are nonresonant. Compared are the response fields obtained assuming the fluid model, and the MHD-kinetic hybrid model including the non-adiabatic contributions from thermal particles (TP), or both thermal particles and energetic particles (i.e. fusion-born alphas) (EP+TP). The parallel sound wave damping model is eliminated for both plasmas (${\kappa _\parallel } = 0$ ).
图 12 在无平行声波阻尼(
${\kappa _\parallel } = 0$ )情况下, 比较DEMO平衡(${q_{95}} = 3.27$ )的(a)流体响应、(b)包含背景粒子的动理学响应以及(c)包含背景粒子和聚变产生α粒子的动理学响应对应的n = 1等离子体径向位移沿法向分量的振幅Figure 12. Comparison of the amplitude of the normal component of the computed plasma radial displacement, due to the plasma response to the applied n = 1 RMP field for
${q_{95}} = 3.27$ , assuming (a) the fluid model, and the MHD-kinetic hybrid model including non-adiabatic contributions from (b) thermal particles, and (c) both fusion-born alphas and thermal particles. The parallel sound wave damping model is eliminated (${\kappa _\parallel } = 0$ ).表 1 在流体响应和动理学响应计算过程中扫描参数的相关信息
Table 1. Parameter information used to compute fluid response and kinetic response.
流体响应 动理学响应(TP, TP+EP) $n = 1—4$ $n = 1$ ${\kappa _\parallel } = 1.5$ ${\kappa _\parallel } = 1.5$ ${\kappa _\parallel } = 0$ 中线圈 上下两组线圈 上中下三组线圈 中线圈 ${q_{95} } = 3.0—6.5$ ${q_{95}} = 3.27$ $\begin{gathered}{q_{95}} = 3.27\left( {{\beta _{\text{N}}} = 2.69} \right)\\{q_{95}} = 6.54\left( {{\beta _{\text{N}}} = 1.35} \right)\end{gathered}$ — $\begin{gathered} \Delta {\varPhi ^{ {\text{UL} } } } = {\varPhi ^{\text{U} } } - {\varPhi ^{\text{L} } } \\ = - {180^{\circ} }—{180^{\circ} } \end{gathered}$ $\begin{gathered}{\varPhi ^{\text{U} } } - {\varPhi ^{\text{M} } } = - {180^{\circ} }—{180^{\circ} }\\{\varPhi ^{\text{L} } } - {\varPhi ^{\text{M} } } = - {180^{\circ} }—{180^{\circ} }\end{gathered}$ — $ {I^{\text{M}}} = 90{\text{ kAt}} $ $ \begin{gathered}{I^{\text{U}}} = {I^{\text{L}}} = 90{\text{ kAt}}\\ {I^{\text{U}}} + {I^{\text{L}}} = 180{\text{ kAt}} \end{gathered}$ $\begin{gathered} {I^{\text{U}}} = {I^{\text{M}}} = {I^{\text{L}}} = 90{\text{ kAt}}\\{I^{\text{U}}} + {I^{\text{M}}} + {I^{\text{L}}} = 270{\text{ kAt}} \end{gathered}$ $ {I^{\text{M}}} = 90{\text{ kAt}} $ 
DownLoad: CSV
表 2 不同环向模数下, 电流为90 kAt时单组上/下线圈在X点附近产生扰动位移的幅值和相位
Table 2. Amplitude and phase of the plasma surface displacement near the X-point for different toroidal number, using only upper/lower row of coils with 90 kAt current.
单组上线圈 单组下线圈 $n$ $\left| {{\xi _X}} \right|$/mm $\varPhi /\left( ^\circ \right)$ $\left| {{\xi _X}} \right|$/mm $\varPhi /\left( ^\circ \right)$ 1 39.98 $ - 155.8$ 39.41 $ - 67.3$ 2 16.19 $ - 156.4$ 11.16 $ - 48.1$ 3 3.46 $ - 107.0$ 11.09 $22.4$ 4 6.90 $149.4$ 1.20 $ - 98.8$
DownLoad: CSV
表 3 上下两组线圈电流幅值和相位差的优化结果
Table 3. Optimization results of the current amplitude and phase difference between the upper and lower rows of coils.
$n$ ${I^{\text{U}}}/{\text{kAt}}$ ${I^{\text{L}}}/{\text{kAt}}$ $ ({\varPhi ^{\text{U}}} - {\varPhi ^{\text{L}}})/(^\circ ) $ 1 90.64 89.36 $88.5$ 2 106.56 73.44 $108.3$ 3 42.80 137.20 $ 129.4 $ 4 153.34 26.66 $ 111.8 $
DownLoad: CSV
表 4 不同环向模数下, 电流为90 kAt时单组中线圈在X点附近产生扰动位移的幅值和相位
Table 4. Amplitude and phase of the plasma surface displacement near the X-point for different toroidal number, using only middle row of coils with 90 kAt current.
$n$ $\left| {{\xi _X}} \right|$/mm $\varPhi {\text{/(}}^\circ )$ 1 $70.57$ $ - 110.8$ 2 5.52 $ - 102.5$ 3 12.30 $ - 39.0$ 4 6.33 $ - 178.8$
DownLoad: CSV
表 5 上中下三组线圈电流幅值和相位差的优化结果
Table 5. Optimization results of the current amplitude and phasing with three rows of coils.
$n$ ${I^{\text{L}}}/{\text{kAt}}$ ${I^{\text{M}}}/{\text{kAt}}$ ${I^{\text{U}}}/{\text{kAt}}$ $({\varPhi ^{\text{L} } } - {\varPhi ^{\text{M} } }) $$ /(^\circ )$ $({\varPhi ^{\text{U} } } - {\varPhi ^{\text{M} } }) $$ /(^\circ )$ 1 70.95 127.05 72.00 $ - 43.5$ $45.0$ 2 91.68 45.33 132.99 $ - 54.4 $ $53.9$ 3 111.52 123.69 34.79 $ - 61.4$ $68.0$ 4 22.44 118.44 129.12 $ - 80.0$ $31.8$
DownLoad: CSV
-
[1] Suttrop W 2000 Plasma Phys. Contr. F. 42 A1
Google Scholar
[2] Loarte A, Lipschultz B, Kukushkin A S, Matthews G F, Stangeby P C, Asakura N, Counsell G F, Federici G, Kallenbach A, Krieger K, Mahdavi A, Philipps V, Reiter D, Roth J, Strachan J, Whyte D, Doerner R, Eich T, Fundamenski W, Herrmann A, Fenstermacher M, Ghendrih P, Groth M, Kirschner A, Konoshima S, LaBombard B, Lang P, Leonard A W, Monier-Garbet P, Neu R, Pacher H, Pegourie B, Pitts R A, Takamura S, Terry J, Tsitrone E, Group D P T 2007 Nucl. Fusion 47 S203
Google Scholar
[3] Ryter F, Fuchs J, Schneider W, Sips A, Stäbler A, Stober J, Team A U 2008 J. Phys. Conf. Ser. 123 012035
Google Scholar
[4] Loarte A, Saibene G, Sartori R, Becoulet M, Horton L, Eich T, Herrmann A, Laux M, Matthews G, Jachmich S, Asakura N, Chankin A, Leonard A, Porter G, Federici G, Shimada M, Sugihara M, Janeschitz G 2003 J. Nucl. Mater 313 962
Google Scholar
[5] Wenninger R P, Bernert M, Eich T, Fable E, Federici G, Kallenbach A, Loarte A, Lowry C, McDonald D, Neu R, Pütterich T, Schneider P, Sieglin B, Strohmayer G, Reimold F, Wischmeier M 2014 Nucl. Fusion 54 114003
Google Scholar
[6] Liang Y, Koslowski H R, Thomas P R, Nardon E, Alper B, Andrew P, Andrew Y, Arnoux G, Baranov Y, Becoulet M, Beurskens M, Biewer T, Bigi M, Crombe K, De La Luna E, de Vries P, Fundamenski W, Gerasimov S, Giroud C, Gryaznevich M P, Hawkes N, Hotchin S, Howell D, Jachmich S, Kiptily V, Moreira L, Parail V, Pinches S D, Rachlew E, Zimmermann O 2007 Phys. Rev. Lett. 98 265004
Google Scholar
[7] Suttrop W, Eich T, Fuchs J C, Gunter S, Janzer A, Herrmann A, Kallenbach A, Lang P T, Lunt T, Maraschek M, McDermott R M, Mlynek A, Putterich T, Rott M, Vierle T, Wolfrum E, Yu Q, Zammuto I, Zohm H, Team A U 2011 Phys. Rev. Lett. 106 225004
Google Scholar
[8] Evans T E, Moyer R A, Thomas P R, Watkins J G, Osborne T H, Boedo J A, Doyle E J, Fenstermacher M E, Finken K H, Groebner R J, Groth M, Harris J H, La Haye R J, Lasnier C J, Masuzaki S, Ohyabu N, Pretty D G, Rhodes T L, Reimerdes H, Rudakov D L, Schaffer M J, Wang G, Zeng L 2004 Phys. Rev. Lett. 92 235003
Google Scholar
[9] Jeon Y M, Park J K, Yoon S W, Ko W H, Lee S G, Lee K D, Yun G S, Nam Y U, Kim W C, Kwak J G, Lee K S, Kim H K, Yang H L, Team K 2012 Phys. Rev. Lett. 109 035004
Google Scholar
[10] Kirk A, Suttrop W, Chapman I T, Liu Y, Scannell R, Thornton A J, Orte L B, Cahyna P, Eich T, Fischer R, Fuchs C, Ham C, Harrison J R, Jakubowski M W, Kurzan B, Pamela S, Peterka M, Ryan D, Saarelma S, Sieglin B, Valovic M, Willensdorfer M 2015 Nucl. Fusion 55 043011
Google Scholar
[11] Liang Y, Koslowski H R, Thomas P R, Nardon E, Alper B, Andrew P, Andrew Y, Arnoux G, Baranov Y, Bécoulet M, Beurskens M, Biewer T, Bigi M, Crombe K, De La Luna E, de Vries P, Fundamenski W, Gerasimov S, Giroud C, Gryaznevich M P, Hawkes N, Hotchin S, Howell D, Jachmich S, Kiptily V, Moreira L, Parail V, Pinches S D, Rachlew E, Zimmermann O 2007 Physical Review Letters 98 265004
[12] Sun Y, Liang Y, Liu Y Q, Gu S, Yang X, Guo W, Shi T, Jia M, Wang L, Lyu B, Zhou C, Liu A, Zang Q, Liu H, Chu N, Wang H H, Zhang T, Qian J, Xu L, He K, Chen D, Shen B, Gong X, Ji X, Wang S, Qi M, Song Y, Yuan Q, Sheng Z, Gao G, Fu P, Wan B 2016 Phys. Rev. Lett. 117 115001
Google Scholar
[13] Wan B N, Liang Y, Gong X Z, Xiang N, Xu G S, Sun Y, Wang L, Qian J P, Liu H Q, Zeng L, Zhang L, Zhang X J, Ding B J, Zang Q, Lyu B, Garofalo A M, Ekedahl A, Li M H, Ding F, Ding S Y, Du H F, Kong D F, Yu Y, Yang Y, Luo Z P, Huang J, Zhang T, Zhang Y, Li G Q, Xia T Y 2019 Nucl. Fusion 59 112003
Google Scholar
[14] Duan X R, Liu Y, Xu M, Yan L W, Xu Y, Song X M, Dong J Q, Ding X T, Chen L Y, Lu B, Liu D Q, Rao J, Xuan W M, Yang Q W, Zheng G Y, Zou X L, Liu Y Q, Zhong W L, Zhao K J, Ji X Q, Mao W C, Wang Q M, Li Q, Cao J Y, Cao Z, Lei G J, Zhang J H, Li X D, Bai X Y, Cheng J, Chen W, Cui Z Y, Delpech L, Diamond P H, Dong Y B, Ekedahl A, Hoang T, Huang Y, Ida K, Itoh K, Itoh S I, Isobe M, Inagaki S, Mazon D, Morita S, Peysson Y, Shi Z B, Wang X G, Xiao G L, Yu D L, Yu L M, Zhang Y P, Zhou Y, Cui C H, Feng B B, Huang M, Li Y G, Li B, Li G S, Li H J, Li Q, Peng J F, Wang Y Q, Yuan B S, Liu Y 2017 Nucl. Fusion 57 102013
Google Scholar
[15] Ding Y, Chen Z, Chen Z, Yang Z, Wang N, Hu Q, Rao B, Chen J, Cheng Z, Gao L, Jiang Z, Wang L, Wang Z, Zhang X, Zheng W, Zhang M, Zhuang G, Yu Q, Liang Y, Yu K, Hu X, Pan Y, Gentle K W, Team J T 2018 Plasma Sci. Technol. 20 125101
Google Scholar
[16] Haskey S R, Lanctot M J, Liu Y Q, Hanson J M, Blackwell B D, Nazikian R 2014 Plasma Phys. Contr. F. 56 035005
Google Scholar
[17] Schaffer M J, Menard J E, Aldan M P, Bialek J M, Evans T E, Moyer R A 2008 Nucl. Fusion 48 024004
Google Scholar
[18] Liu Y Q, Kirk A, Sun Y, Cahyna P, Chapman I T, Denner P, Fishpool G, Garofalo A M, Harrison J R, Nardon E 2012 Plasma Phys. Contr. F. 54 124013
Google Scholar
[19] Liu Y, Li L, Loarte A, Pinches S D, Polevoi A 2021 Nucl. Fusion 61 106029
Google Scholar
[20] 苏春燕, 牟茂淋, 陈少永, 郭文平, 唐昌建 2021 70 095207
Google Scholar
Su C Y, Mou M L, Chen S Y, Guo W P, Tang C J 2021 Acta Phys. Sin. 70 095207
Google Scholar
[21] Zhou L, Liu Y, Liu Y, Yang X 2016 Plasma Phys. Contr. F. 58 115003
Google Scholar
[22] 陈撷宇, 牟茂淋, 苏春燕, 陈少永, 唐昌建 2020 69 195201
Google Scholar
Chen X Y, Mou M L, Su C Y, Chen S Y, Tang C J 2020 Acta Phys. Sin. 69 195201
Google Scholar
[23] 李春雨, 郝广周, 刘钺强, 王炼, 刘艺慧子 2022 71 075202
Google Scholar
Li C Y, Hao G Z, Liu Y Q, Wang L, Liu Y H Z 2022 Acta Phys. Sin. 71 075202
Google Scholar
[24] Liu Y, Chu M S, Chapman I T, Hender T C 2008 Phys. Plasmas 15 112503
Google Scholar
[25] Liu Y, Kirk A, Nardon E 2010 Phys. Plasmas 17 122502
Google Scholar
[26] Sun Y, Liang Y, Liu Y Q, Gu S, Yang X, Guo W, Shi T, Jia M, Wang L, Lyu B, Zhou C, Liu A, Zang Q, Liu H, Chu N, Wang H H, Zhang T, Qian J, Xu L, He K, Chen D, Shen B, Gong X, Ji X, Wang S, Qi M, Song Y, Yuan Q, Sheng Z, Gao G, Fu P, Wan B 2016 Physical Review Letters 117 115001
[27] Liu Y Q, Bondeson A, Fransson C M, Lennartson B, Breitholtz C 2000 Phys. Plasmas 7 3681
Google Scholar
[28] Liu Y, Kirk A, Gribov Y, Gryaznevich M P, Hender T C, Nardon E 2011 Nucl. Fusion 51 083002
Google Scholar
[29] Wang Z R, Lanctot M J, Liu Y Q, Park J K, Menard J E 2015 Phys. Rev. Lett. 114 145005
Google Scholar
[30] Li L, Liu Y Q, Kirk A, Wang N, Liang Y, Ryan D, Suttrop W, Dunne M, Fischer R, Fuchs J C, Kurzan B, Piovesan P, Willensdorfer M, Zhong F C 2016 Nucl. Fusion 56 126007
Google Scholar
[31] Yang X, Sun Y, Liu Y, Gu S, Liu Y, Wang H, Zhou L, Guo W 2016 Plasma Phys. Contr. F. 58 114006
Google Scholar
[32] Liu Y, Kirk A, Li L, In Y, Nazikian R, Sun Y, Suttrop W, Lyons B, Ryan D, Wang S, Yang X, Zhou L 2017 Phys. Plasmas 24 056111
Google Scholar
[33] Bondeson A, Iacono R 1989 Phys. Fluids B Plasma Phys. 1 1431
Google Scholar
[34] Liu Y, Chapman I T, Saarelma S, Gryaznevich M P, Hender T C, Howell D F 2009 Plasma Phys. Contr. F. 51 115005
Google Scholar
[35] Liu Y, Ryan D, Kirk A, Li L, Suttrop W, Dunne M, Fischer R, Fuchs J C, Kurzan B, Piovesan P, Willensdorfer M 2016 Nucl. Fusion 56 056015
Google Scholar
[36] Wang Z, Park J-K, Menard J E, Liu Y, Kaye S M, Gerhardt S 2018 Nucl. Fusion 58 016015
Google Scholar
[37] Hu Q M, Nazikian R, Grierson B A, Logan N C, Paz-Soldan C, Yu Q 2020 Nucl. Fusion 60 076001
Google Scholar
[38] Wang S X, Liu H Q, Jie Y X, Ding W X, Zeng L, Sun Y W, Lian H, Zhu X, Zou Z Y, Lyu B, Li Y Y, Zang Q, Du H F, Zhou C, Liu A D, Zhang T, Gao W, Gao X 2018 Nucl. Fusion 58 112013
Google Scholar
[39] Zhou L, Liu Y, Wenninger R, Liu Y, Wang S, Yang X 2018 Nucl. Fusion 58 076025
Google Scholar
[40] Li L, Liu Y Q, Wang N, Kirk A, Koslowski H R, Liang Y, Loarte A, Ryan D, Zhong F C 2017 Plasma Phys. Contr. F. 59 044005
Google Scholar
[41] Wenninger R, Kembleton R, Bachmann C, Biel W, Bolzonella T, Ciattaglia S, Cismondi F, Coleman M, Donné A J H, Eich T, Fable E, Federici G, Franke T, Lux H, Maviglia F, Meszaros B, Pütterich T, Saarelma S, Snickers A, Villone F, Vincenzi P, Wolff D, Zohm H 2017 Nucl. Fusion 57 016011
Google Scholar
[42] Lütjens H, Bondeson A, Sauter O 1996 Comput. Phys. Commun. 97 219
Google Scholar
[43] Liu Y, Akers R, Chapman I T, Gribov Y, Hao G Z, Huijsmans G T A, Kirk A, Loarte A, Pinches S D, Reinke M, Ryan D, Sun Y, Wang Z R 2015 Nucl. Fusion 55 063027
Google Scholar
[44] Fenstermacher M E, Evans T E, Osborne T H, Schaffer M J, Aldan M P, deGrassie J S, Gohil P, Joseph I, Moyer R A, Snyder P B, Groebner R J, Jakubowski M, Leonard A W, Schmitz O, Team D 2008 Phys. Plasmas 15 056122
Google Scholar
[45] Gu S, Wan B, Sun Y, Chu N, Liu Y Q, Shi T, Wang H H, Jia M, He K 2019 Nucl. Fusion 59 126042
Google Scholar
[46] Nazikian R, Petty C C, Bortolon A, Chen X, Eldon D, Evans T E, Grierson B A, Ferraro N M, Haskey S R, Knolker M, Lasnier C, Logan N C, Moyer R A, Orlov D, Osborne T H, Paz-Soldan C, Turco F, Wang H Q, Weisberg D B 2018 Nucl. Fusion 58 106010
Google Scholar
[47] Hu Q M, Nazikian R, Logan N C, Park J K, Paz-Soldan C, Yang S M, Grierson B A, In Y, Jeon Y M, Kim M, Kim S K, Orlov D M, Park G Y, Yu Q 2021 Phys. Plasmas 28 052505
Google Scholar
[48] Federici G, Bachmann C, Barucca L, Baylard C, Biel W, Boccaccini L V, Bustreo C, Ciattaglia S, Cismondi F, Corato V, Day C, Diegele E, Franke T, Gaio E, Gliss C, Haertl T, Ibarra A, Holden J, Keech G, Kembleton R, Loving A, Maviglia F, Morris J, Meszaros B, Moscato I, Pintsuk G, Siccinio M, Taylor N, Tran M Q, Vorpahl C, Walden H, You J H 2019 Nucl. Fusion 59 066013
Google Scholar
[49] Zhou L, Liu Y, Hu H, Siccinio M, Maviglia F, Zohm H, Pigatto L, Wang Y, Li L, Hao G Z, Yang X, Zhang H, Duan P, Chen L 2023 Plasma Phys. Contr. F. 65 035008
Google Scholar
[50] Hu B, Betti R, Manickam J 2006 Phys. Plasmas 13 112505
Google Scholar
[51] Zhou L, Liu Y, Siccinio M, Fable E, Wu T, Kurki-Suonio T, Varje J, Liu D 2021 Plasma Phys. Contr. F. 63 065007
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 3708
- PDF Downloads: 72
- Cited By: 0
