-
锯齿振荡是托卡马克装置上常见的磁流体不稳定性之一. 先进实验超导托卡马克(experimental advanced superconducting Tokamak, EAST)装置放电中观察到外加共振磁扰动(resonant magnetic perturbation, RMP)会影响锯齿振荡行为. 本文研究了环向模数n = 2的RMP对锯齿周期的影响. 通过RMP线圈相位差扫描实验发现, 当RMP上下线圈(n = 2)相位差
$ \Delta {\phi }_{{\rm{U}}{\rm{L}}} $ 发生变化时, 扰动场的谱型发生变化, 锯齿周期和崩塌幅度也随之发生明显的改变. 单流体电阻磁流体力学 (MARS-F)程序分析表明, 在相同实验条件下, 当RMP上下线圈(n = 2)相位差为$\Delta {\phi }_{{\rm{U}}{\rm{L}}}=270^\circ$ 时, 等离子体芯部区域的响应最强, 此时锯齿周期和幅度最小. 锯齿行为的改变可能与RMP引起的快离子损失相关. 该研究利用RMP上下线圈(n = 2)实现对锯齿行为的有效调控, 有利于未来EAST发展高比压放电条件下的大锯齿自动控制技术.-
关键词:
- 先进实验超导托卡马克 /
- 共振磁扰动 /
- 锯齿振荡
Sawtooth oscillation is one of the most important magneto-hydrodynamic (MHD) instabilities in Tokamak plasma, which can result in the periodic relaxation of the temperature and density of the core plasma when the safety factor on the magnetic axis (q0) is lower than unity. Owing to the periodic relaxation of the plasma core parameters, sawtooth oscillations are beneficial to avoiding impurity accumulation in plasma core. However, the large sawtooth crash may trigger off other MHD instabilities, like tearing modes (TMs) or neoclassical tearing modes (NTMs), which is a matter of concern for the plasma stability. Therefore, it is essential to control sawtooth oscillations for ensuring safe operation in the future Tokamaks such as ITER. The resonant magnetic perturbation (RMP) is widely used to control edge-localized modes (ELMs) and divertor heat flux in Tokamak. The application of RMP has also been found to affect the sawtooth behaviors. This paper studies the influence of RMP coils at n = 2 on sawtooth behaviors in experimental advanced superconducting Tokamak (EAST), where n is the toroidal mode number of the applied RMP. It is found that the phase difference between upper RMP coil and lower RMP coil ($ \Delta {\phi }_{{\rm{U}}{\rm{L}}}\left(^\circ\right)={\phi }_{{\rm{U}}}\left(^\circ\right)-{\phi }_{{\rm{L}}}\left(^\circ\right) $ ) is a notable parameter of affecting sawtooth behavior. The experiments for scanning the phase difference$ \Delta {\phi }_{{\rm{U}}{\rm{L}}} $ are carried out. When the phase difference$ \Delta {\phi }_{{\rm{U}}{\rm{L}}} $ of RMP at n = 2 is changed, the sawtooth period and amplitude become subsequently different. The minimum sawtooth period and amplitude appear at$\Delta {\phi }_{{\rm{U}}{\rm{L}}}=270^\circ$ . At the same time, neutron yields measured by neutron diagnostic system have the same trend as sawtooth behavior during RMP phase difference scanning. The plasma response to RMP at n = 2 is analyzed by using the MARS-F code. The results show that the plasma responses much strongly at the$\Delta {\phi }_{{\rm{U}}{\rm{L}}}=270^\circ$ . The loss of fast ion, caused by RMP coils, is possibly stronger at the$\Delta {\phi }_{{\rm{U}}{\rm{L}}}=270^\circ$ than that at other phase difference$ \Delta {\phi }_{{\rm{U}}{\rm{L}}}. $ The loss of fast ion can reduces its stabilization effect on sawtooth behavior, which results in the reduction of the sawtooth period and amplitude. Further research is needed to optimize the sawtooth control method with RMP to make it compatible with plasma performance.-
Keywords:
- experimental advanced superconducting Tokamak /
- resonant magnetic perturbation /
- sawtooth oscillations
[1] Porcelli F, Boucher D, Rosenbluth M N 1996 Plasma Phys. Controlled Fusion 38 2163
Google Scholar
[2] Goniche M, Dumont R J, Bobkov V, et al. 2017 Plasma Phys. Controlled Fusion 59 055001
Google Scholar
[3] Bando T, Wakatsuki T, Honda M, Isayama A, Shinohara K, Inoue S, Yoshida M, Matsunaga G, Takechi M, Oyama N, Ide S 2021 Plasma Phys. Controlled Fusion 63 085009
Google Scholar
[4] Wang J Q, Xiao C J, Wang X G, Ji X Q, Liu Y 2012 Plasma Phys. Controlled Fusion 54 122001
Google Scholar
[5] Chao Y, Xu L Q, Hu L Q, Yuan Y, Zhang Y K, Lü B, Zhong G Q, Liu Y, Liu H Q, Du H F 2019 AIP Adv. 9 015226
Google Scholar
[6] Li J C, Gong X Y, Dong J Q, Wang J, Yin L 2016 Chin. Phys. B 25 045201
Google Scholar
[7] Lerche E, Lennholm M, Carvalho I S, Jacquet P, Mantsinen M, Dumortier P, Van Eester D, Graves J P, Card P, Noble C 2020 Nucl. Fusion 60 126037
Google Scholar
[8] Sun Y W, Ma Q, Jia M N, et al. 2021 Nucl. Fusion 61 106037
Google Scholar
[9] Bonfiglio D, Veranda M, Cappello S, Chacón L, Escande D F 2017 Plasma Phys. Controlled Fusion 59 014032
Google Scholar
[10] Li J C, Ding Y H, Yu Q Q, Wang N C, Li D, Zhang X Q, Han D L, Chen Z P, Yang Z J, Zhou S, Yan W, Liang Y F, Zhang X L, Lin X D, Sun H B, Gao X, Li J G 2020 Nucl. Fusion 60 126002
Google Scholar
[11] Kim G, Yun G S, Woo M 2019 Plasma Phys. Controlled Fusion 61 055001
Google Scholar
[12] Yuan Y, Hu L Q, Xu L Q, Wang X G, Wang X J, Xu H D, Luo Z P, Chen K Y, Lin S Y, Duan Y M, Chang P X, Zhao H L, He K Y, Liang Y F 2016 Phys. Plasmas 23 062503
Google Scholar
[13] Sun Y W, Liang Y, Qian J P, Shen B, Wan B 2015 Plasma Phys. Controlled Fusion 57 045003
Google Scholar
[14] Mao F Y, Wang N C, Huang Z, Ren Z K, Zhou S, Shen C S, Zhang X Y, He Y, Zhang Q, Jia R, Zhao C X, Li Y B, Hu B, Li D, Bala A A, Chen Z P, Chen Z Y, Yang Z J, Liang Y F, Ding Y H, Pan Y 2022 Plasma Sci. Technol. 24 124002
Google Scholar
[15] Chen L, Zonca F 2016 Rev. Mod. Phys. 88 015008
Google Scholar
[16] Qian J P, Lao L L, Liu H Q, Ding W X, Zeng L, Luo Z P, Ren Q L, Huang Y, Huang J, Brower D L, Hanada K, Chen D L, Sun Y W, Shen B, Gong X Z, Xiao B J, Wan B N 2017 Nucl. Fusion 57 036008
Google Scholar
[17] Liu H Q, Qian J P, Jie Y X, Ding W X, Brower D L, Zou Z Y, Li W M, Lian H, Wang S X, Yang Y, Zeng L, Lan T, Yao Y, Hu L Q, Zhang X D, Wan B N 2016 Rev. Sci. Instrum. 87 11D903
Google Scholar
[18] Zhong G Q, Cao H R, Hu L Q, Zhou R J, Xiao M, Li K, Pu N, Huang J, Liu G Z, Lin S Y, Lyu B, Liu H Q, Zhang X J 2016 Plasma Phys. Controlled Fusion 58 075013
Google Scholar
[19] Lyu B, Chen J, Hu R J, Delgado-Aparicio L F, Wang F D, Bitter M, Hill K W, Pablant N, Lee S G, Ye M Y, Shi Y J, Wan B N 2018 Rev. Sci. Instrum. 89 10F112
Google Scholar
[20] Zhong G Q, Hu L Q, Pu N, Zhou R J, Xiao M, Cao H R, Zhu Y B, Li K, Fan T S, Peng X Y, Du T F, Ge L J, Huang J, Xu G S, Wan B N 2016 Rev. Sci. Instrum. 87 11D820
Google Scholar
[21] Chapman I T, Pinches S D, Graves J P, et al. 2007 Plasma Phys. Controlled Fusion 49 B385
Google Scholar
[22] Chen W, Ding X T, Liu Y, Yang Q W, Ji X Q, Isobe M, Yuan G L, Zhang Y P, Zhou Y, Song X Y, Dong Y B, Li W, Zhou J, Lei G J, Cao J Y, Deng W, Song X M, Duan X R 2010 Nucl. Fusion. 50 084008
Google Scholar
[23] Ding Y H, Chen Z Y, Chen Z P, et al. 2018 Plasma Sci. Technol. 20 125101
Google Scholar
[24] 苏春燕, 牟茂淋, 陈少永, 郭文平, 唐昌建 2021 70 095207
Google Scholar
Su C Y, Mou M L, Chen S Y, Guo W P, Tang C J 2021 Acta Phys. Sin. 70 095207
Google Scholar
[25] He K Y, Sun Y W, Wan B N, Gu S, Jia M N, Hu Y 2021 Nucl. Fusion 61 016009
Google Scholar
[26] Hao G Z, Li C Y, Liu Y Q, Chen H T, Wang S, Bai X, Dong G Q, He H D, Zhao Y F, Miao Y T, Zhou L N, Xu J Q, Zhang N, Chen Q, Sun T F, Ji X Q, Liu Y, Zhong W L, Xu M, Duan X R 2021 Nucl. Fusion 61 126031
Google Scholar
[27] 李春雨, 郝广周, 刘钺强, 王炼, 刘艺慧子 2022 71 075202
Google Scholar
Li C Y, Hao G Z, Liu Y Q, Wang L, Liu Y H Z 2022 Acta Phys. Sin. 71 075202
Google Scholar
[28] Liu Y Q, Kirk A, Nardon E 2010 Phys. Plasmas 17 122502
Google Scholar
[29] Ren J, Sun Y W, Wang H H, Gu S, Qian J P, Shi T H, Shen B, Liu Y Q, Guo W F, Chu N, He K Y, Jia M N, Wang Y, Sheng Z C, Luo Z P, Zeng L, Gong X Z, Liang Y F, Wan B N 2021 Nucl. Fusion. 61 056007
Google Scholar
[30] Jia M N, Loarte A, Sun Y W, et al. 2021 Nucl. Fusion. 61 106023
Google Scholar
-
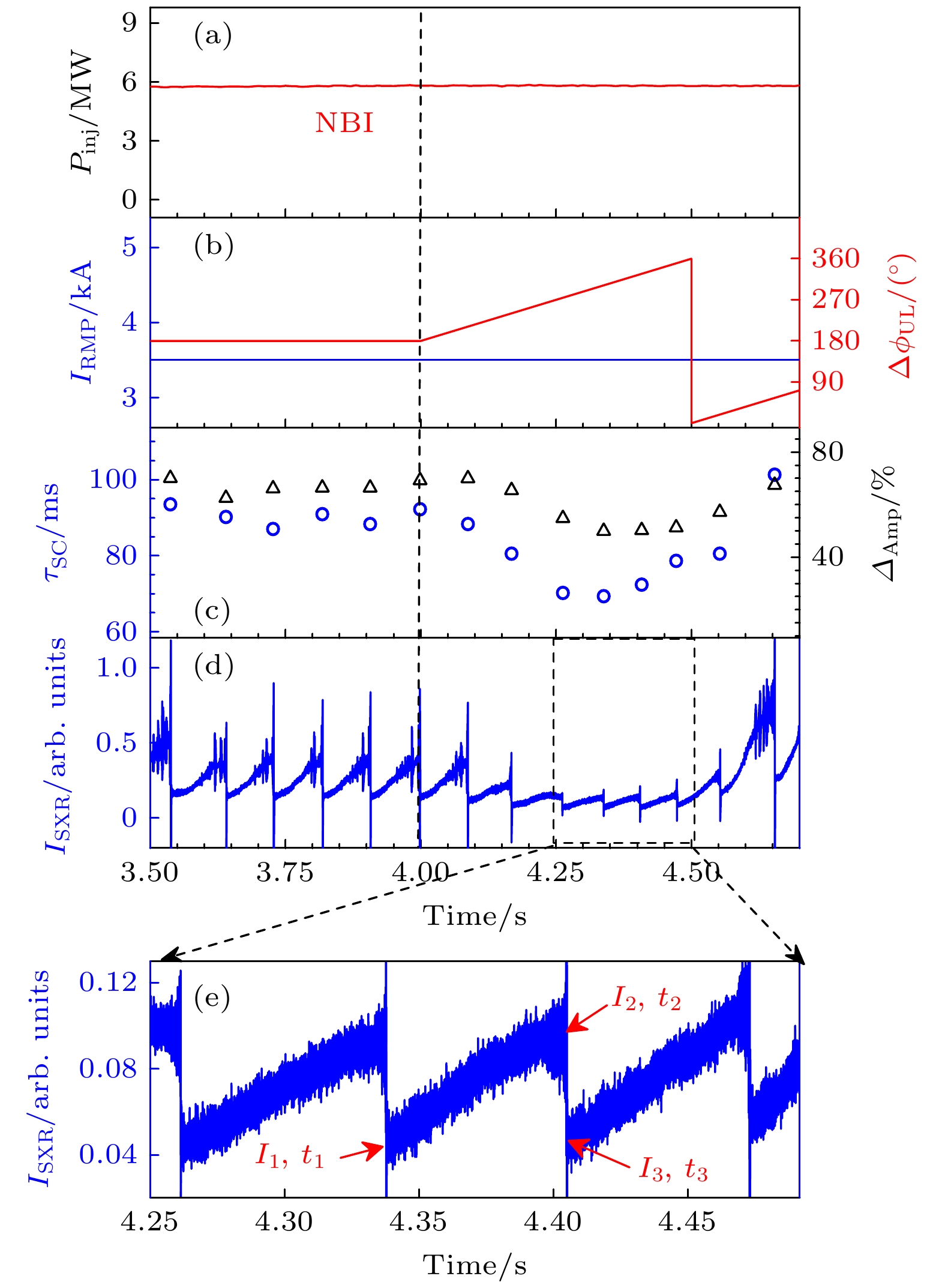
图 2 EAST #116507炮放电中等离子体主要参数的演化 (a)中性束注入功率; (b) n = 2的RMP电流(蓝色)及其上下线圈相位差(红色); (c)锯齿周期(蓝色圆圈)和崩塌幅度(黑色三角); (d)芯部SXR辐射信号; (e)图(d)的局部放大图
Fig. 2. Evolution of plasma parameters for EAST shot #116507: (a) NBI heating power; (b) n = 2 RMP coil current amplitude (blue) and the phase difference between the upper and lower coil
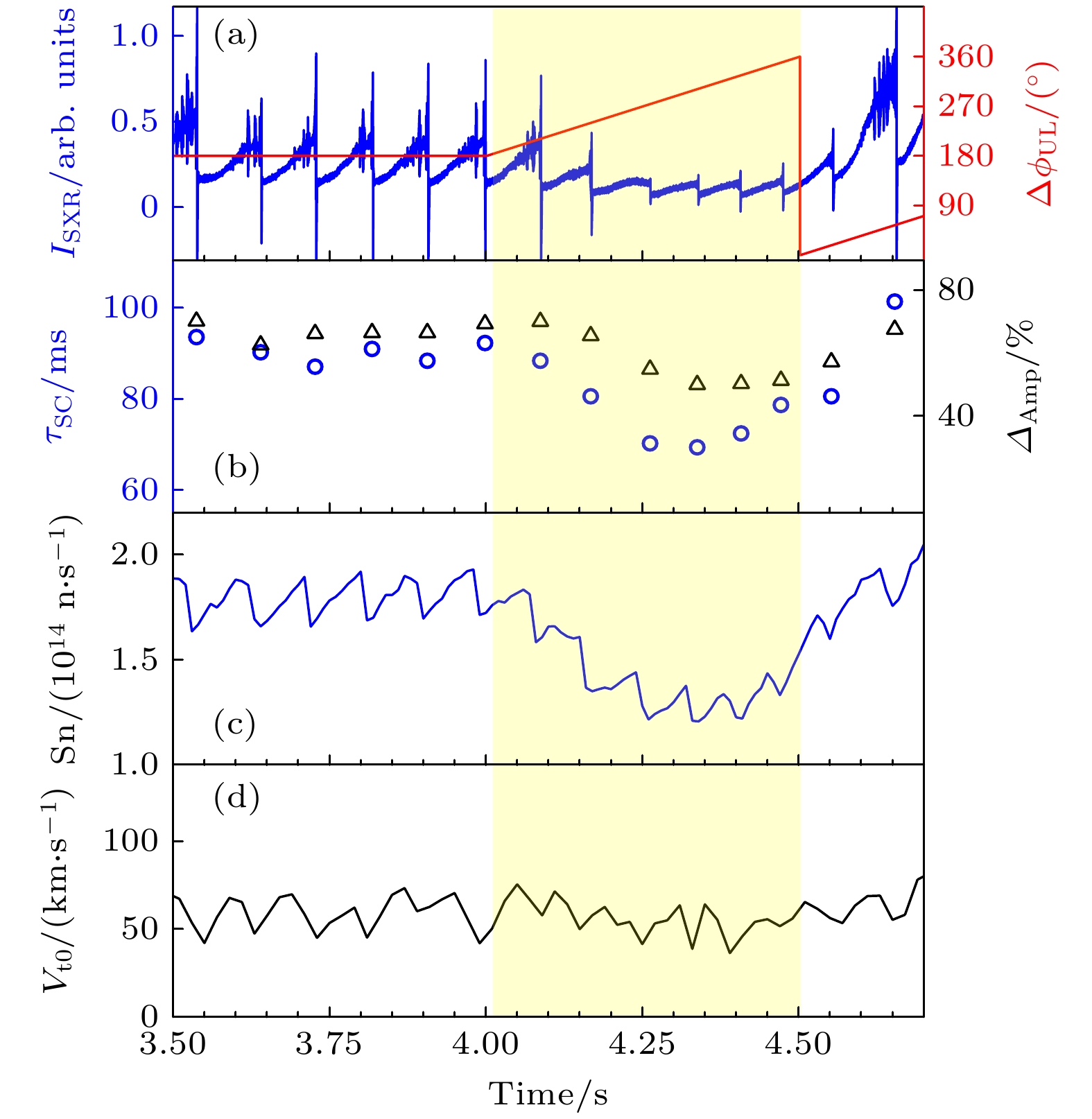
$\Delta {\phi }_{{\rm{U}}{\rm{L}}}$ (red); (c) sawtooth period (blue circles) and amplitude (black triangles); (d) core SXR emission; (e) partial enlargement of panel (d).
图 3 n = 2的RMP线圈不同相位差下的芯部SXR辐射信号 (a) #116507; (b) #116510; (c) #116505; (d) #116508
Fig. 3. Core SXR emission of various phase difference of RMP at n = 2: (a) #116507; (b) #116510; (c) #116505; (d) #116508.
图 5 EAST #116507炮放电中等离子体参数的演变 (a)芯部SXR辐射信号(蓝色)和RMP上下线圈相位差(红色); (b)锯齿周期(蓝色圆圈)和崩塌幅度(黑色三角); (c)中子产额; (d)等离子体芯部旋转速度
Fig. 5. Evolution of plasma parameters of EAST shot #116507: (a) Core SXR emission (blue) and the phase difference between the upper and lower coil
$\Delta {\phi }_{{\rm{U}}{\rm{L}}}$ of RMP at n = 2 (red); (b) sawtooth period (blue circles) and amplitude (black triangles); (c) neutron yield; (d) toroidal rotation velocity of plasma core.
图 6 IRMP = 3.5 kA时, EAST 四炮等离子体参数的演变(a)等离子体电流; (b)中性束注入功率; (c)等离子体密度; (d) n = 2 的RMP上下线圈相位差; (e)中子产额
Fig. 6. Evolution of plasma parameters of four shot with EAST at IRMP = 3.5 kA: (a) Plasma current; (b) NBI heating power; (c) electron density; (d) phase difference between the upper and lower coil
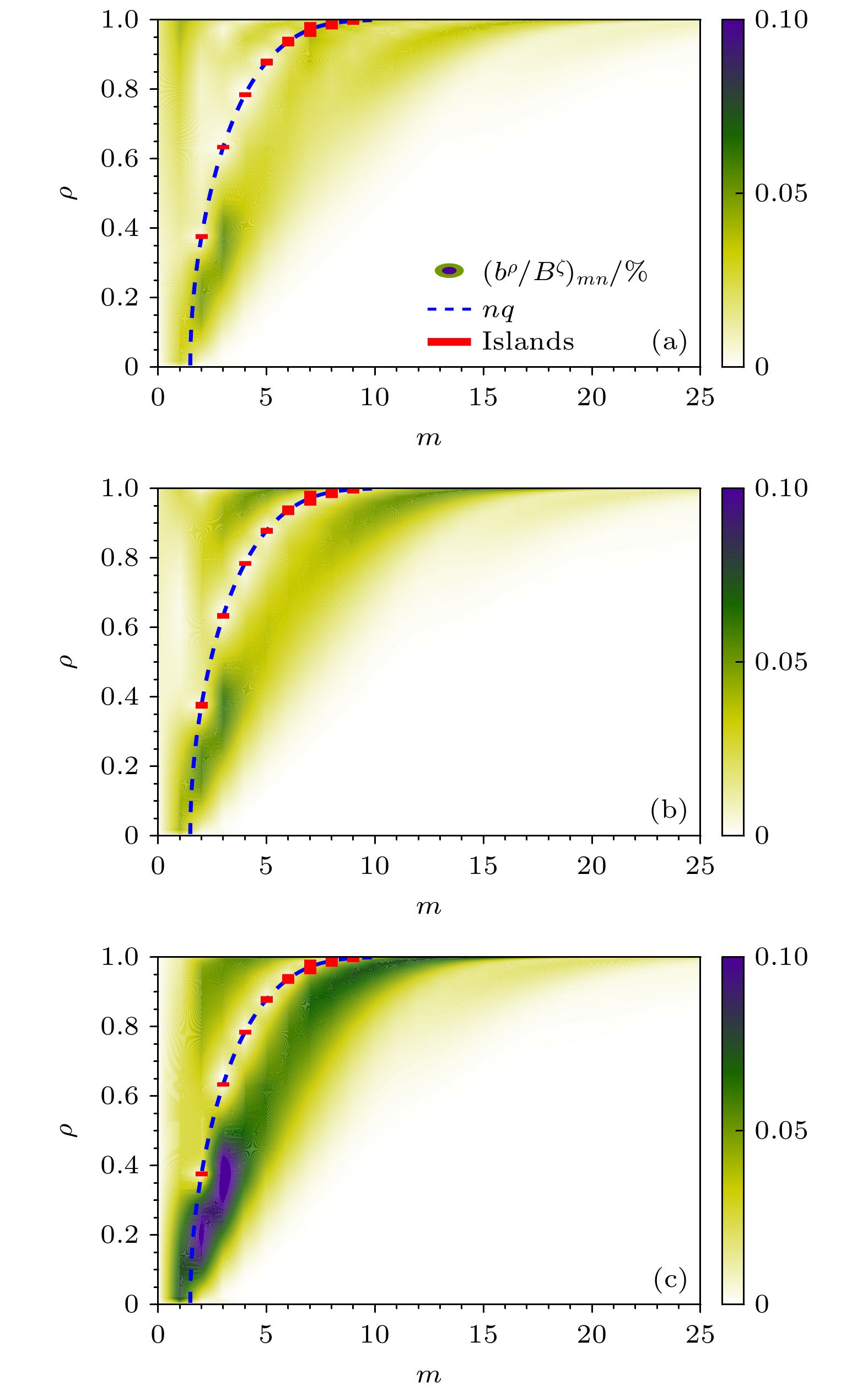
$\Delta {\phi }_{{\rm{U}}{\rm{L}}}$ of RMP at n = 2; (e) neutron yield.
图 7 (a), (c) EAST #116507炮放电芯部SXR辐射信号; (b), (d) 图(a), (c)对应时间段的SXR频谱图
Fig. 7. (a), (c) Core SXR signal of discharge #116507; (b), (d) spectrum of SXR for the time corresponding to panels (a) and (c).
图 8 EAST #116507炮放电中n = 2的扰动场在不同相位差下的谱型对比 (a)
$\Delta {\phi }_{{\rm{U}}{\rm{L}}}=0^\circ$ ; (b)$\Delta {\phi }_{{\rm{U}}{\rm{L}}}=180^\circ$ ; (c)$\Delta {\phi }_{{\rm{U}}{\rm{L}}}= 270^\circ$ Fig. 8. Comparison of n = 2 RMP spectrum for different phase difference of discharge #116507: (a)
$\Delta {\phi }_{{\rm{U}}{\rm{L}}}=0^\circ$ ; (b)$\Delta {\phi }_{{\rm{U}}{\rm{L}}}= $ $ 180^\circ$ ; (c)${\Delta }{\phi }_{{\rm{U}}{\rm{L}}}=270^\circ$ . -
[1] Porcelli F, Boucher D, Rosenbluth M N 1996 Plasma Phys. Controlled Fusion 38 2163
Google Scholar
[2] Goniche M, Dumont R J, Bobkov V, et al. 2017 Plasma Phys. Controlled Fusion 59 055001
Google Scholar
[3] Bando T, Wakatsuki T, Honda M, Isayama A, Shinohara K, Inoue S, Yoshida M, Matsunaga G, Takechi M, Oyama N, Ide S 2021 Plasma Phys. Controlled Fusion 63 085009
Google Scholar
[4] Wang J Q, Xiao C J, Wang X G, Ji X Q, Liu Y 2012 Plasma Phys. Controlled Fusion 54 122001
Google Scholar
[5] Chao Y, Xu L Q, Hu L Q, Yuan Y, Zhang Y K, Lü B, Zhong G Q, Liu Y, Liu H Q, Du H F 2019 AIP Adv. 9 015226
Google Scholar
[6] Li J C, Gong X Y, Dong J Q, Wang J, Yin L 2016 Chin. Phys. B 25 045201
Google Scholar
[7] Lerche E, Lennholm M, Carvalho I S, Jacquet P, Mantsinen M, Dumortier P, Van Eester D, Graves J P, Card P, Noble C 2020 Nucl. Fusion 60 126037
Google Scholar
[8] Sun Y W, Ma Q, Jia M N, et al. 2021 Nucl. Fusion 61 106037
Google Scholar
[9] Bonfiglio D, Veranda M, Cappello S, Chacón L, Escande D F 2017 Plasma Phys. Controlled Fusion 59 014032
Google Scholar
[10] Li J C, Ding Y H, Yu Q Q, Wang N C, Li D, Zhang X Q, Han D L, Chen Z P, Yang Z J, Zhou S, Yan W, Liang Y F, Zhang X L, Lin X D, Sun H B, Gao X, Li J G 2020 Nucl. Fusion 60 126002
Google Scholar
[11] Kim G, Yun G S, Woo M 2019 Plasma Phys. Controlled Fusion 61 055001
Google Scholar
[12] Yuan Y, Hu L Q, Xu L Q, Wang X G, Wang X J, Xu H D, Luo Z P, Chen K Y, Lin S Y, Duan Y M, Chang P X, Zhao H L, He K Y, Liang Y F 2016 Phys. Plasmas 23 062503
Google Scholar
[13] Sun Y W, Liang Y, Qian J P, Shen B, Wan B 2015 Plasma Phys. Controlled Fusion 57 045003
Google Scholar
[14] Mao F Y, Wang N C, Huang Z, Ren Z K, Zhou S, Shen C S, Zhang X Y, He Y, Zhang Q, Jia R, Zhao C X, Li Y B, Hu B, Li D, Bala A A, Chen Z P, Chen Z Y, Yang Z J, Liang Y F, Ding Y H, Pan Y 2022 Plasma Sci. Technol. 24 124002
Google Scholar
[15] Chen L, Zonca F 2016 Rev. Mod. Phys. 88 015008
Google Scholar
[16] Qian J P, Lao L L, Liu H Q, Ding W X, Zeng L, Luo Z P, Ren Q L, Huang Y, Huang J, Brower D L, Hanada K, Chen D L, Sun Y W, Shen B, Gong X Z, Xiao B J, Wan B N 2017 Nucl. Fusion 57 036008
Google Scholar
[17] Liu H Q, Qian J P, Jie Y X, Ding W X, Brower D L, Zou Z Y, Li W M, Lian H, Wang S X, Yang Y, Zeng L, Lan T, Yao Y, Hu L Q, Zhang X D, Wan B N 2016 Rev. Sci. Instrum. 87 11D903
Google Scholar
[18] Zhong G Q, Cao H R, Hu L Q, Zhou R J, Xiao M, Li K, Pu N, Huang J, Liu G Z, Lin S Y, Lyu B, Liu H Q, Zhang X J 2016 Plasma Phys. Controlled Fusion 58 075013
Google Scholar
[19] Lyu B, Chen J, Hu R J, Delgado-Aparicio L F, Wang F D, Bitter M, Hill K W, Pablant N, Lee S G, Ye M Y, Shi Y J, Wan B N 2018 Rev. Sci. Instrum. 89 10F112
Google Scholar
[20] Zhong G Q, Hu L Q, Pu N, Zhou R J, Xiao M, Cao H R, Zhu Y B, Li K, Fan T S, Peng X Y, Du T F, Ge L J, Huang J, Xu G S, Wan B N 2016 Rev. Sci. Instrum. 87 11D820
Google Scholar
[21] Chapman I T, Pinches S D, Graves J P, et al. 2007 Plasma Phys. Controlled Fusion 49 B385
Google Scholar
[22] Chen W, Ding X T, Liu Y, Yang Q W, Ji X Q, Isobe M, Yuan G L, Zhang Y P, Zhou Y, Song X Y, Dong Y B, Li W, Zhou J, Lei G J, Cao J Y, Deng W, Song X M, Duan X R 2010 Nucl. Fusion. 50 084008
Google Scholar
[23] Ding Y H, Chen Z Y, Chen Z P, et al. 2018 Plasma Sci. Technol. 20 125101
Google Scholar
[24] 苏春燕, 牟茂淋, 陈少永, 郭文平, 唐昌建 2021 70 095207
Google Scholar
Su C Y, Mou M L, Chen S Y, Guo W P, Tang C J 2021 Acta Phys. Sin. 70 095207
Google Scholar
[25] He K Y, Sun Y W, Wan B N, Gu S, Jia M N, Hu Y 2021 Nucl. Fusion 61 016009
Google Scholar
[26] Hao G Z, Li C Y, Liu Y Q, Chen H T, Wang S, Bai X, Dong G Q, He H D, Zhao Y F, Miao Y T, Zhou L N, Xu J Q, Zhang N, Chen Q, Sun T F, Ji X Q, Liu Y, Zhong W L, Xu M, Duan X R 2021 Nucl. Fusion 61 126031
Google Scholar
[27] 李春雨, 郝广周, 刘钺强, 王炼, 刘艺慧子 2022 71 075202
Google Scholar
Li C Y, Hao G Z, Liu Y Q, Wang L, Liu Y H Z 2022 Acta Phys. Sin. 71 075202
Google Scholar
[28] Liu Y Q, Kirk A, Nardon E 2010 Phys. Plasmas 17 122502
Google Scholar
[29] Ren J, Sun Y W, Wang H H, Gu S, Qian J P, Shi T H, Shen B, Liu Y Q, Guo W F, Chu N, He K Y, Jia M N, Wang Y, Sheng Z C, Luo Z P, Zeng L, Gong X Z, Liang Y F, Wan B N 2021 Nucl. Fusion. 61 056007
Google Scholar
[30] Jia M N, Loarte A, Sun Y W, et al. 2021 Nucl. Fusion. 61 106023
Google Scholar
下载:
计量
- 文章访问数: 3431
- PDF下载量: 111
- 被引次数: 0
