Elastic wave propagation characteristics in unsaturated double-porosity medium under capillary pressure

Shi Zhi-Qi; He Xiao; Liu Lin; Chen De-Hua; Wang Xiu-Ming

doi:10.7498/aps.72.20222063

Abstract

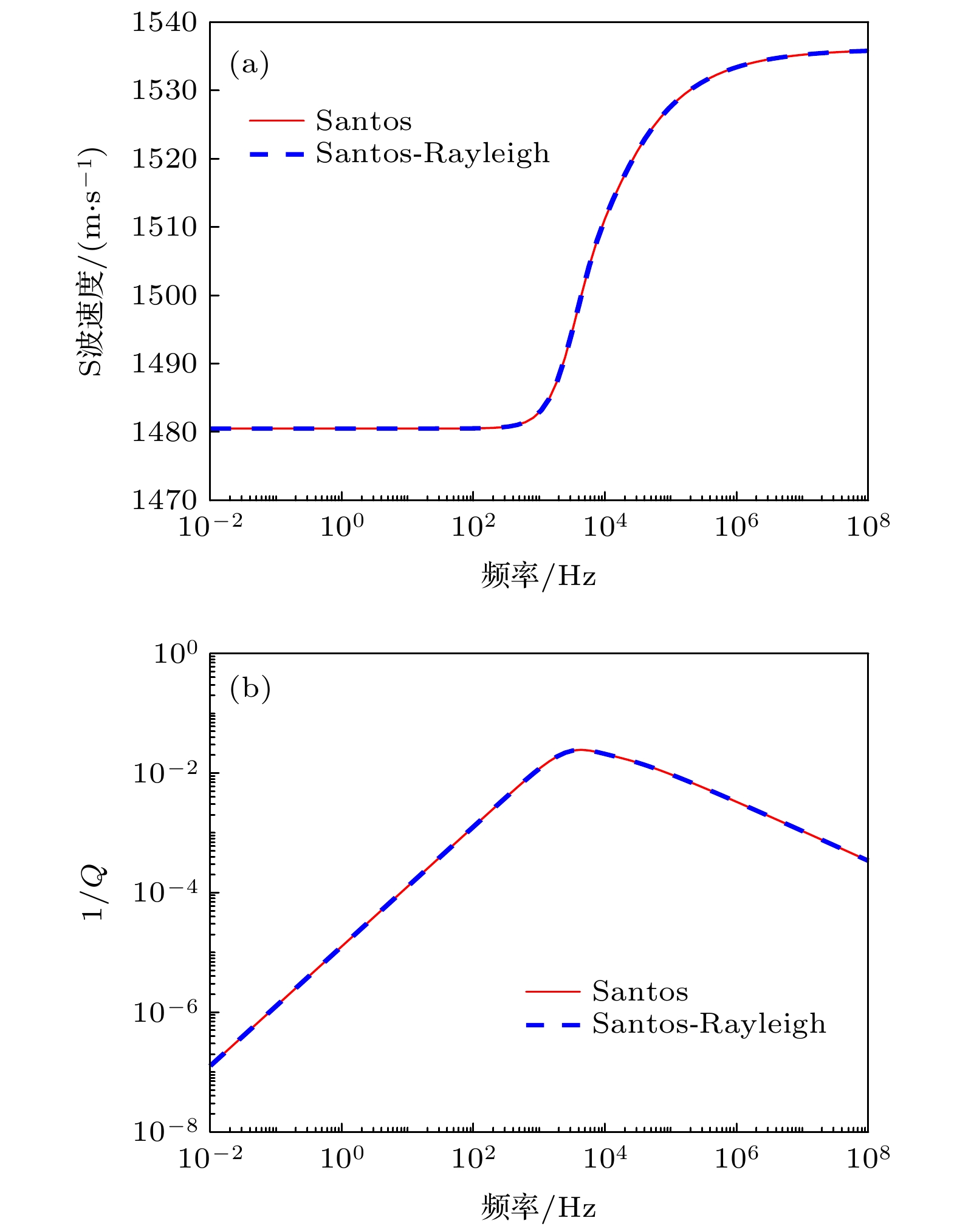
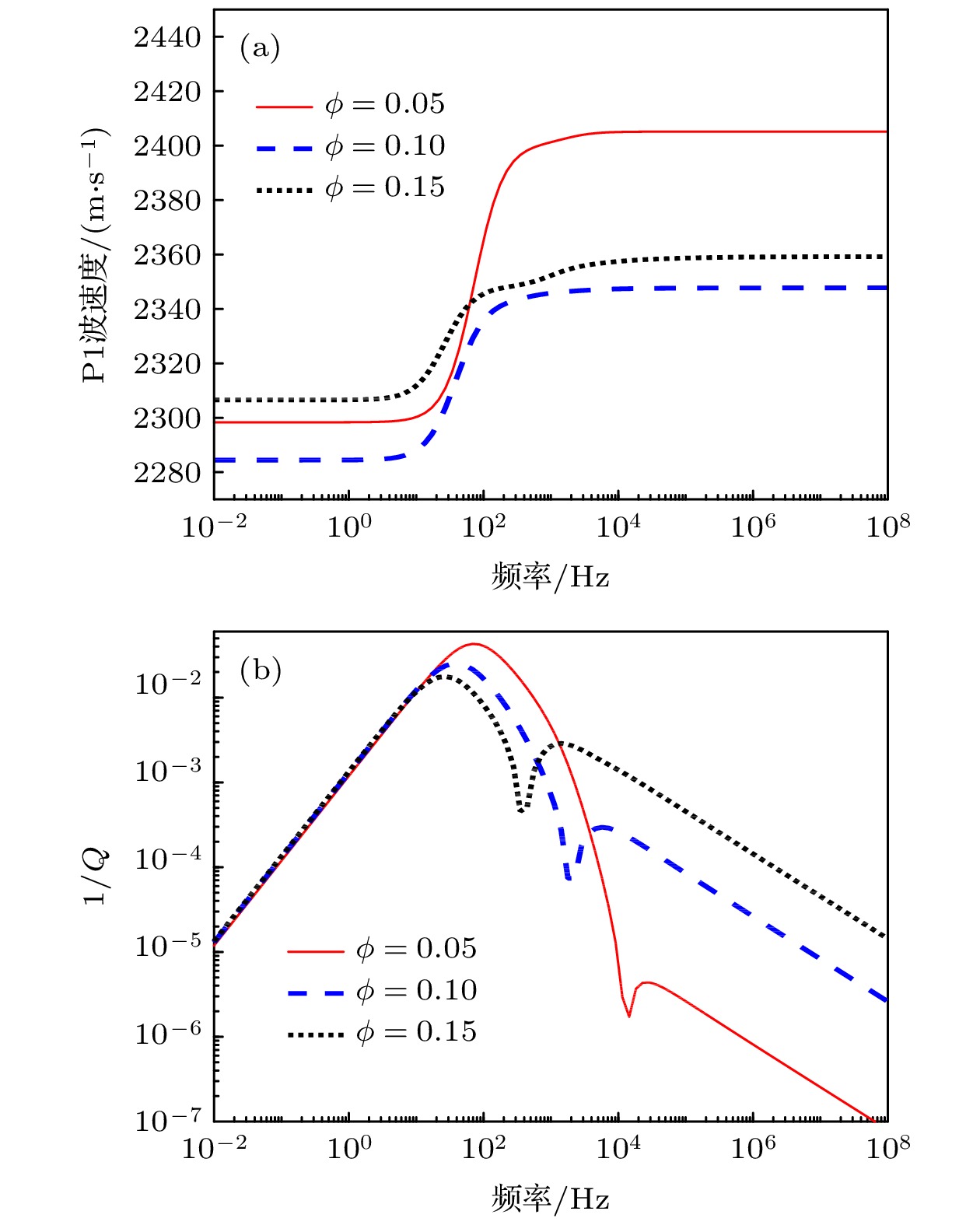
Rock pores often contain two-phase or multi-phase fluids, so it is important to understand how the wave-induced fluid pressure diffusion affects dispersion and attenuation of elastic waves for resource exploration. To describe the propagation of elastic wave in a double-porosity medium saturated by two-phase fluids, a wave propagation model, including both global and local flow mechanisms and considering the effect of capillary pressure, is derived. The dispersion and attenuation characteristics of three longitudinal waves (P1, P2, P3) and one transverse wave (S wave) are investigated by analyzing a plane wave, and the effects of physical parameters, such as inclusion radius, water saturation, permeability and porosity, on the propagation characteristics of P1 wave are investigated. Theoretical analysis shows that the model derived in this work can be degenerated into the Biot model under specific conditions. According to the numerical simulation results, due to the coupling of global flow and local flow, the P1 wave velocity may decrease below the Gassmann-Wood limit. The physical explanation of this phenomenon is as follows: when considering the effect of capillary pressure, the coupling effect of global flow and local flow will break the basic assumption that rock is undrained. The relationship between physical parameters of porous medium and the dispersion and attenuation characteristics of elastic wave is complicated and nonlinear. Compared with Santos model, elastic modulus predicted by Santos-Rayleigh model is in good agreement with the experimental data in the low frequency band, which proves that this model has good reliability in modeling the velocity field of seismic exploration.

Keywords:

Authors and contacts

1.
State Key Laboratory of Acoustics, Institute of Acoustics, Chinese Academy of Sciences, Beijing 100190, China
2.
University of Chinese Academy of Sciences, Beijing 100049, China
3.
Beijing Engineering and Technology Research Center for Deep Drilling Exploration and Measurement, Institute of Acoustic, Chinese Academy of Sciences, Beijing 100190, China

Corresponding author: He Xiao, hex@mail.ioa.ac.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12174421, 42074174).

FullText HTML : translate this paragraph

References

[1]	Müller T M, Gurevich B, Lebedev M 2010 Geophysics 75 75A147 Google Scholar
[2]	Biot M A 1956 J. Acoust. Soc. Am. 28 168 Google Scholar
[3]	Biot M A 1962 J. Appl. Phys. 33 1482 Google Scholar
[4]	巴晶 2013 岩石物理学进展与评述 (北京: 清华大学出版社) 第151页 Ba J 2013 Progress and Review of Rock Physics (Beijing: Tsinghua University Press) p151 (in Chinese)
[5]	Santos J E, Douglas J, Corberó J, Lovera O M 1990 J. Acoust. Soc. Am. 87 1439 Google Scholar
[6]	Santos J E, Corberó J M, Douglas J 1990 J. Acoust. Soc. Am. 87 1428 Google Scholar
[7]	Lo W C, Sposito G, Majer E 2005 Water Resour. Res. 41 W02025 Google Scholar
[8]	王婷, 崔志文, 刘金霞, 王克协 2018 67 114301 Google Scholar Wang T, Cui Z W, Liu J X, Wang K X 2018 Acta Phys. Sin. 67 114301 Google Scholar
[9]	Mavko G, Nur A 1975 J. Geophys. Res. 80 1444 Google Scholar
[10]	White J E 1975 Geophysics 40 224 Google Scholar
[11]	Dutta N C, Odé H 1979 Geophysics 44 1777 Google Scholar
[12]	Wang Y, Zhao L, Cao C, Yao Q, Yang Z, Cao H, Geng J 2022 Geophysics 87 MR247 Google Scholar
[13]	Johnson D L 2001 J. Acoust. Soc. Am. 110 682 Google Scholar
[14]	Tserkovnyak Y, Johnson D L 2003 J. Acoust. Soc. Am. 114 2596 Google Scholar
[15]	Qi Q, Müller T M, Gurevich B, Lopes S, Lebedev M, Caspari E 2014 Geophysics 79 WB35 Google Scholar
[16]	Ciz R, Shapiro S A 2007 Geophysics 72 A75 Google Scholar
[17]	Müller T M, Gurevich B 2005 J. Acoust. Soc. Am. 117 2732 Google Scholar
[18]	Dvorkin J, Nur A 1993 Geophysics 58 524 Google Scholar
[19]	Pride S R, Berryman, Harris J M 2004 J. Geophys. Res. 109 B01201 Google Scholar
[20]	巴晶, Carcione J M, 曹宏, 杜启振, 袁振宇, 卢明辉 2012 地球 55 219 Google Scholar Ba J, Carcione J M, Cao H, Du Q Z, Yuan Z Y, Lu M H 2012 Chin. J. Geophys. 55 219 Google Scholar
[21]	Sun W T, Ba J, Carcione J M 2016 Geophys. J. Int. 205 22 Google Scholar
[22]	Ba J, Xu W, Fu L Y, Carcione J M, Zhang L 2017 J. Geophys. Res. Solid Earth 122 1949 Google Scholar
[23]	Zhang L, Ba J, Carcione J M, Wu C F 2022 J. Geophys. Res. Solid Earth 127 e2021JB023809 Google Scholar
[24]	李红星, 张嘉辉, 樊嘉伟, 陶春辉, 肖昆, 黄光南, 盛书中, 宫猛 2022 71 089101 Google Scholar Li H X, Zhang J H, Fan J W, Tao C H, Xiao K, Huang G N, Sheng S Z, Gong M 2022 Acta Phys. Sin. 71 089101 Google Scholar
[25]	Murphy W M 1984 J. Geophys. Res. Solid Earth 89 11549 Google Scholar
[26]	Batzle M L, Han D H, Hofmann R 2006 Geophysics 71 N1 Google Scholar
[27]	Chapman S, Borgomano J V M, Quintal B, Benson S M, Fortin J 2021 J. Geophys. Res. Solid Earth 126 e2021JB021643 Google Scholar
[28]	Ravazzoli C L, Santos J E, Carcione J M 2003 J. Acoust. Soc. Am. 113 1801 Google Scholar
[29]	赵海波 2007 博士学位论文 (北京: 中国科学院研究生院) Zhao H B 2007 Ph. D. Dissertation (Beijing: Graduate University of Chinese Academy of Sciences) (in Chinese)
[30]	Carcione J M, Cavallini F, Santos J E, Ravazzoli C L, Gauzellino P M 2004 Wave Motion 39 227 Google Scholar

Cited By

图 1 一种骨架和两种流体组成的非饱和双重孔隙介质示意图. 红色虚线箭头和黑色实线箭头分别表示波传播方向上的全局流矢量和嵌入体径向的局域流矢量

Figure 1. Schematic diagram of unsaturated double-porosity medium composed of one type skeleton and two types of fluids. The velocity vectors of global flow and local flow are indicated by red dashed and black solid arrows respectively.

DownLoad: Full-Size Img PowerPoint

图 2 Santos模型和Santos-Rayleigh模型预测的P1波速度与衰减随频率变化曲线对比　(a) P1波相速度; (b) P1波逆品质因子

Figure 2. Comparison of P1 wave velocity and attenuation as the function of frequencies predicted by Santos and Santos-Rayleigh models: (a) Phase velocity of P1 wave; (b) dissipation factor of P1 wave.

DownLoad: Full-Size Img PowerPoint

图 3 Santos模型和Santos-Rayleigh模型预测的P2和P3波速度与衰减随频率变化曲线对比　(a) P2, P3波相速度; (b) P2, P3波逆品质因子

Figure 3. Comparison of P2 and P3 wave velocities and attenuations as the function of frequencies predicted by Santos and Santos-Rayleigh models: (a) Phase velocity of P2 and P3 wave; (b) dissipation factor of P2 and P3 wave.

DownLoad: Full-Size Img PowerPoint

图 4 Santos模型和Santos-Rayleigh模型预测的S波速度与衰减随频率变化曲线对比　(a) S波相速度; (b) S波逆品质因子

Figure 4. Comparison of S wave velocity and attenuation as the function of frequencies predicted by Santos and Santos-Rayleigh models: (a) Phase velocity of S wave; (b) dissipation factor of S wave.

DownLoad: Full-Size Img PowerPoint

图 5 不同嵌入体半径下Santos-Rayleigh模型P1波速度与衰减随频率变化曲线　(a) P1波相速度; (b) P1波逆品质因子

Figure 5. P1 wave velocity and attenuation predicted by Santos-Rayleigh model with different radii of inclusion: (a) Phase velocity of P1 wave; (b) dissipation factor of P1 wave.

DownLoad: Full-Size Img PowerPoint

图 6 不同含水饱和度下Santos-Rayleigh模型P1波速度与衰减随频率变化曲线　(a) P1波相速度; (b) P1波逆品质因子

Figure 6. P1 wave velocity and attenuation predicted by Santos-Rayleigh model with different water saturations: (a) Phase velocity of P1 wave; (b) dissipation factor of P1 wave.

DownLoad: Full-Size Img PowerPoint

图 7 不同渗透率下Santos-Rayleigh模型P1波速度与衰减随频率变化曲线　(a) P1波相速度; (b) P1波逆品质因子

Figure 7. P1 wave velocity and attenuation predicted by Santos-Rayleigh model with different permeabilities: (a) Phase velocity of P1 wave; (b) dissipation factor of P1 wave.

DownLoad: Full-Size Img PowerPoint

图 8 不同孔隙度下Santos-Rayleigh模型P1波速度与衰减随频率变化曲线　(a) P1波相速度; (b) P1波逆品质因子

Figure 8. P1 wave velocity and attenuation predicted by Santos-Rayleigh model with different porosities: (a) Phase velocity of P1 wave; (b) dissipation factor of P1 wave.

DownLoad: Full-Size Img PowerPoint

图 9 Santos-Rayleigh模型与实际数据对比

Figure 9. Comparison of measured data with Santos-Rayleigh model.

DownLoad: Full-Size Img PowerPoint

表 1 非饱和孔隙介质物性参数表

Table 1. Physical parameters of unsaturated porous media model.

参数	值
固体基质体积模量/GPa	36
固体基质密度/(kg ·m^–3)	2650
骨架体积模量/GPa	6.21
骨架剪切模量/GPa	4.55
孔隙度	0.33
渗透率/m²	4.935×10^–12
润湿相流体体积模量/GPa	2.223
润湿相流体密度/(kg·m^–3)	1000
润湿相流体黏度/(Pa·s)	0.001
非润湿相流体体积模量/GPa	0.022
非润湿相流体密度/(kg·m^–3)	100
非润湿相流体黏度/(Pa·s)	15×10^–6

DownLoad: CSV

表 2 Berea砂岩及流体物性参数表

Table 2. Physical parameters of Berea sandstone and fluids.

参数	值
固体基质体积模量/GPa	30
固体基质密度/(kg·m^–3)	2600
骨架体积模量/GPa	11.7
骨架剪切模量/GPa	11.1
孔隙度	0.196
渗透率/m²	0.266×10^–12
地层水体积模量/GPa	2.23
地层水密度/(kg·m^–3)	997.67
地层水黏度/(Pa·s)	0.00091
二氧化碳体积模量/GPa	1.17×10^–3
二氧化碳密度/(kg·m^–3)	17.21
二氧化碳黏度/(Pa·s)	1.5×10^–5

DownLoad: CSV

map

[1]	Müller T M, Gurevich B, Lebedev M 2010 Geophysics 75 75A147 Google Scholar
[2]	Biot M A 1956 J. Acoust. Soc. Am. 28 168 Google Scholar
[3]	Biot M A 1962 J. Appl. Phys. 33 1482 Google Scholar
[4]	巴晶 2013 岩石物理学进展与评述 (北京: 清华大学出版社) 第151页 Ba J 2013 Progress and Review of Rock Physics (Beijing: Tsinghua University Press) p151 (in Chinese)
[5]	Santos J E, Douglas J, Corberó J, Lovera O M 1990 J. Acoust. Soc. Am. 87 1439 Google Scholar
[6]	Santos J E, Corberó J M, Douglas J 1990 J. Acoust. Soc. Am. 87 1428 Google Scholar
[7]	Lo W C, Sposito G, Majer E 2005 Water Resour. Res. 41 W02025 Google Scholar
[8]	王婷, 崔志文, 刘金霞, 王克协 2018 67 114301 Google Scholar Wang T, Cui Z W, Liu J X, Wang K X 2018 Acta Phys. Sin. 67 114301 Google Scholar
[9]	Mavko G, Nur A 1975 J. Geophys. Res. 80 1444 Google Scholar
[10]	White J E 1975 Geophysics 40 224 Google Scholar
[11]	Dutta N C, Odé H 1979 Geophysics 44 1777 Google Scholar
[12]	Wang Y, Zhao L, Cao C, Yao Q, Yang Z, Cao H, Geng J 2022 Geophysics 87 MR247 Google Scholar
[13]	Johnson D L 2001 J. Acoust. Soc. Am. 110 682 Google Scholar
[14]	Tserkovnyak Y, Johnson D L 2003 J. Acoust. Soc. Am. 114 2596 Google Scholar
[15]	Qi Q, Müller T M, Gurevich B, Lopes S, Lebedev M, Caspari E 2014 Geophysics 79 WB35 Google Scholar
[16]	Ciz R, Shapiro S A 2007 Geophysics 72 A75 Google Scholar
[17]	Müller T M, Gurevich B 2005 J. Acoust. Soc. Am. 117 2732 Google Scholar
[18]	Dvorkin J, Nur A 1993 Geophysics 58 524 Google Scholar
[19]	Pride S R, Berryman, Harris J M 2004 J. Geophys. Res. 109 B01201 Google Scholar
[20]	巴晶, Carcione J M, 曹宏, 杜启振, 袁振宇, 卢明辉 2012 地球 55 219 Google Scholar Ba J, Carcione J M, Cao H, Du Q Z, Yuan Z Y, Lu M H 2012 Chin. J. Geophys. 55 219 Google Scholar
[21]	Sun W T, Ba J, Carcione J M 2016 Geophys. J. Int. 205 22 Google Scholar
[22]	Ba J, Xu W, Fu L Y, Carcione J M, Zhang L 2017 J. Geophys. Res. Solid Earth 122 1949 Google Scholar
[23]	Zhang L, Ba J, Carcione J M, Wu C F 2022 J. Geophys. Res. Solid Earth 127 e2021JB023809 Google Scholar
[24]	李红星, 张嘉辉, 樊嘉伟, 陶春辉, 肖昆, 黄光南, 盛书中, 宫猛 2022 71 089101 Google Scholar Li H X, Zhang J H, Fan J W, Tao C H, Xiao K, Huang G N, Sheng S Z, Gong M 2022 Acta Phys. Sin. 71 089101 Google Scholar
[25]	Murphy W M 1984 J. Geophys. Res. Solid Earth 89 11549 Google Scholar
[26]	Batzle M L, Han D H, Hofmann R 2006 Geophysics 71 N1 Google Scholar
[27]	Chapman S, Borgomano J V M, Quintal B, Benson S M, Fortin J 2021 J. Geophys. Res. Solid Earth 126 e2021JB021643 Google Scholar
[28]	Ravazzoli C L, Santos J E, Carcione J M 2003 J. Acoust. Soc. Am. 113 1801 Google Scholar
[29]	赵海波 2007 博士学位论文 (北京: 中国科学院研究生院) Zhao H B 2007 Ph. D. Dissertation (Beijing: Graduate University of Chinese Academy of Sciences) (in Chinese)
[30]	Carcione J M, Cavallini F, Santos J E, Ravazzoli C L, Gauzellino P M 2004 Wave Motion 39 227 Google Scholar

[1]	Wan Cheng-Liang, Pan Yu-Zhou, Zhu Li-Ping, Li Peng-Fei, Zhang Hao-Wen, Zhao Zhuo-Yan, Yuan Hua, Fan Xu-Hong, Sun Wen-Sheng, Du Zhan-Hui, Chen Qian, Cui Ying, Liao Tian-Fa, Wei Xiao-Hui, Wang Tian-Qi, Chen Xi-Meng, Li Gong-Ping, Reinhold Schuch, Zhang Hong-Qiang. Production and measurement of MeV proton microbeams in atmospheric environment based on glass capillary. Acta Physica Sinica, 2024, 73(10): 104101. doi: 10.7498/aps.73.20240301
[2]	Shi Zhi-Qi, He Xiao, Liu Lin, Chen De-Hua. Simulation and analysis of elastic waves in partially saturated double-porosity media based on finite difference method. Acta Physica Sinica, 2024, 73(10): 100201. doi: 10.7498/aps.73.20240227
[3]	Zhu Xin-Zhe, Li Bo-Yuan, Liu Feng, Li Jian-Long, Bi Ze-Wu, Lu Lin, Yuan Xiao-Hui, Yan Wen-Chao, Chen Min, Chen Li-Ming, Sheng Zheng-Ming, Zhang Jie. Experimental study on capillary discharge for laser plasma wake acceleration. Acta Physica Sinica, 2022, 71(9): 095202. doi: 10.7498/aps.71.20212435
[4]	Li Hong-Xing, Zhang Jia-Hui, Fan Jia-Wei, Tao Chun-Hui, Xiao Kun, Huang Guang-Nan, Sheng Shu-Zhong, Gong Meng. Wave propagation theory of multi-scale wave induced flow in unsaturated porous medium. Acta Physica Sinica, 2022, 71(8): 089101. doi: 10.7498/aps.71.20211463
[5]	Su Na-Na, Han Qing-Bang, Jiang Jian. Guided circumferential wave propagation characteristics for porous cylinder immersed in infinite fluid. Acta Physica Sinica, 2019, 68(8): 084301. doi: 10.7498/aps.68.20182300
[6]	Wang Ting, Cui Zhi-Wen, Liu Jin-Xia, Wang Ke-Xie. Propagation of elastic waves in saturated porous medium containing a small amount of bubbly fluid. Acta Physica Sinica, 2018, 67(11): 114301. doi: 10.7498/aps.67.20180209
[7]	Zhou Hong-Wei, Wang Lin-Wei, Xu Sheng-Hua, Sun Zhi-Wei. Capillary-driven flow in tubes connected to the containers under microgravity condition. Acta Physica Sinica, 2015, 64(12): 124703. doi: 10.7498/aps.64.124703
[8]	Song Yong-Jia, Hu Heng-Shan. Variation of effective elastic moduli of a solid with transverse isotropy due to aligned inhomogeneities. Acta Physica Sinica, 2014, 63(1): 016202. doi: 10.7498/aps.63.016202
[9]	Yun Mei-Juan, Zheng Wei, Li Yun-Bao, Li Yu. Fractal analysis of Herschel-Bulkley fluid flow in a capillary. Acta Physica Sinica, 2012, 61(16): 164701. doi: 10.7498/aps.61.164701
[10]	Gu Yu-Qiu, Ma Zhan-Nan, Zheng Wu-Di, Wang Xiao-Fang, Wu Yu-Chi, Zhu Bin, Dong Ke-Gong, Cao Lei-Feng, He Ying-Ling, Liu Hong-Jie, Hong Wei, Zhou Wei-Min, Zhao Zong-Qing, Zhang Bao-Han, Jiao Chun-Ye, Wen Xian-Lun, Zang Hua-Ping, Yu Jin-Qing, Wei Lai. Density measurement and MHD simulation ofgas-filled capillary discharge waveguide. Acta Physica Sinica, 2011, 60(9): 095202. doi: 10.7498/aps.60.095202
[11]	Cui Zhi-Wen, Liu Jin-Xia, Wang Chun-Xia, Wang Ke-Xie. Elastic waves in Maxwell fluid-saturated porous media with the squirt flow mechanism. Acta Physica Sinica, 2010, 59(12): 8655-8661. doi: 10.7498/aps.59.8655
[12]	Guo Tie-Ying, Lou Shu-Qin, Li Hong-Lei, Jian Shui-Sheng. Capillary drawing for fabrication of photonic crystal fibers: theoretical calculation and experiments. Acta Physica Sinica, 2009, 58(7): 4724-4730. doi: 10.7498/aps.58.4724
[13]	Cao Shi-Ying, Wang Ying, Zhang Zhi-Gang, Chai Lu, Wang Qing-Yue, Yang Jian-Jun, Zhu Xiao-Nong. Spectrum evolution of filamentation restricted by capillary in high pressure gas. Acta Physica Sinica, 2006, 55(9): 4734-4738. doi: 10.7498/aps.55.4734
[14]	Wei Zhong-Chao, Dai Qiao-Feng, Wang He-Zhou. Spectral properties of fcc-like cylindrical colloidal crystals. Acta Physica Sinica, 2006, 55(2): 733-736. doi: 10.7498/aps.55.733
[15]	Sun Jiao, Zhang Jia-Liang, Wang De-Zhen, Ma Teng-Cai. A novel cold plasma jet generated by capillary atmospheric dielectric barrier discharge. Acta Physica Sinica, 2006, 55(1): 344-350. doi: 10.7498/aps.55.344
[16]	Guan Wei, Hu Heng-Shan, Chu Zhao-Tan. Formulation of the acoustically-induced electromagnetic field in a porous formation in terms of Hertz vectors and simulation of the borehole electromagnetic field excited by an acoustic multipole source. Acta Physica Sinica, 2006, 55(1): 267-274. doi: 10.7498/aps.55.267
[17]	Zhao Yong-Peng, Cheng Yuan-Li, Wang Qi, Hayashi Yasushi, Hotta Eiki. The lasing time of soft x-ray laser pumped by capillary discharge. Acta Physica Sinica, 2005, 54(6): 2731-2734. doi: 10.7498/aps.54.2731
[18]	Cheng Yuan-Li, Luan Bo-Han, Wu Yin-Chu, Zhao Yong-Peng, Wang Qi, Zheng Wu-Di, Peng Hui-Min, Yang Da-Wei. Effect of pre-pulses on capillary discharge soft x-ray laser. Acta Physica Sinica, 2005, 54(10): 4979-4984. doi: 10.7498/aps.54.4979
[19]	Hu Heng-Shan. Acoustic head wave on the borehole wall in a porous formation and the causes for its accompanying electromagnetic field. Acta Physica Sinica, 2003, 52(8): 1954-1959. doi: 10.7498/aps.52.1954
[20]	Du Qi-Zhen, Yang Hui-Zhu. . Acta Physica Sinica, 2002, 51(9): 2101-2108. doi: 10.7498/aps.51.2101

Catalog

Vol. 72, Issue 6 - 2023-03-20

Metrics

Abstract views: 3734
PDF Downloads: 90
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板