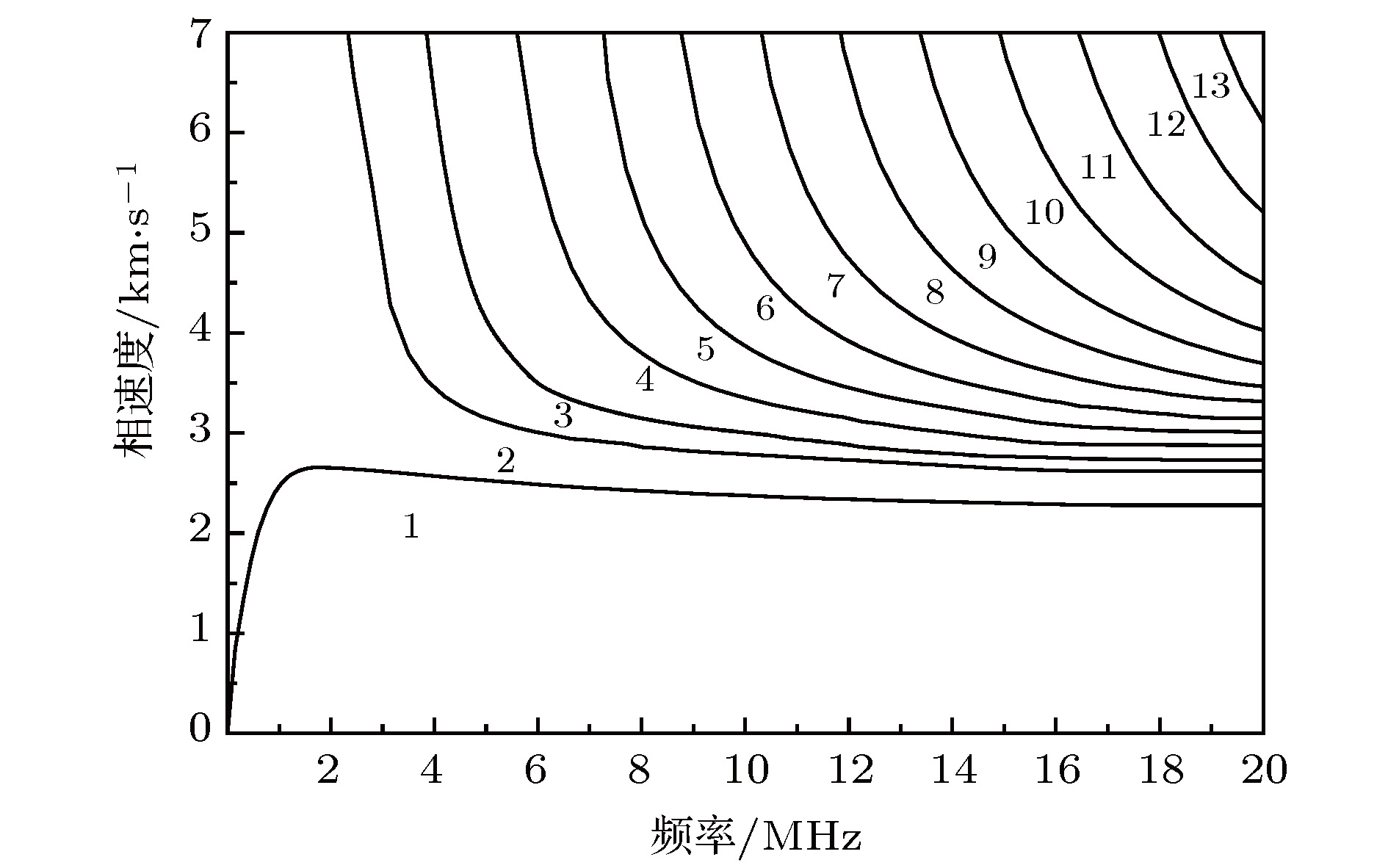
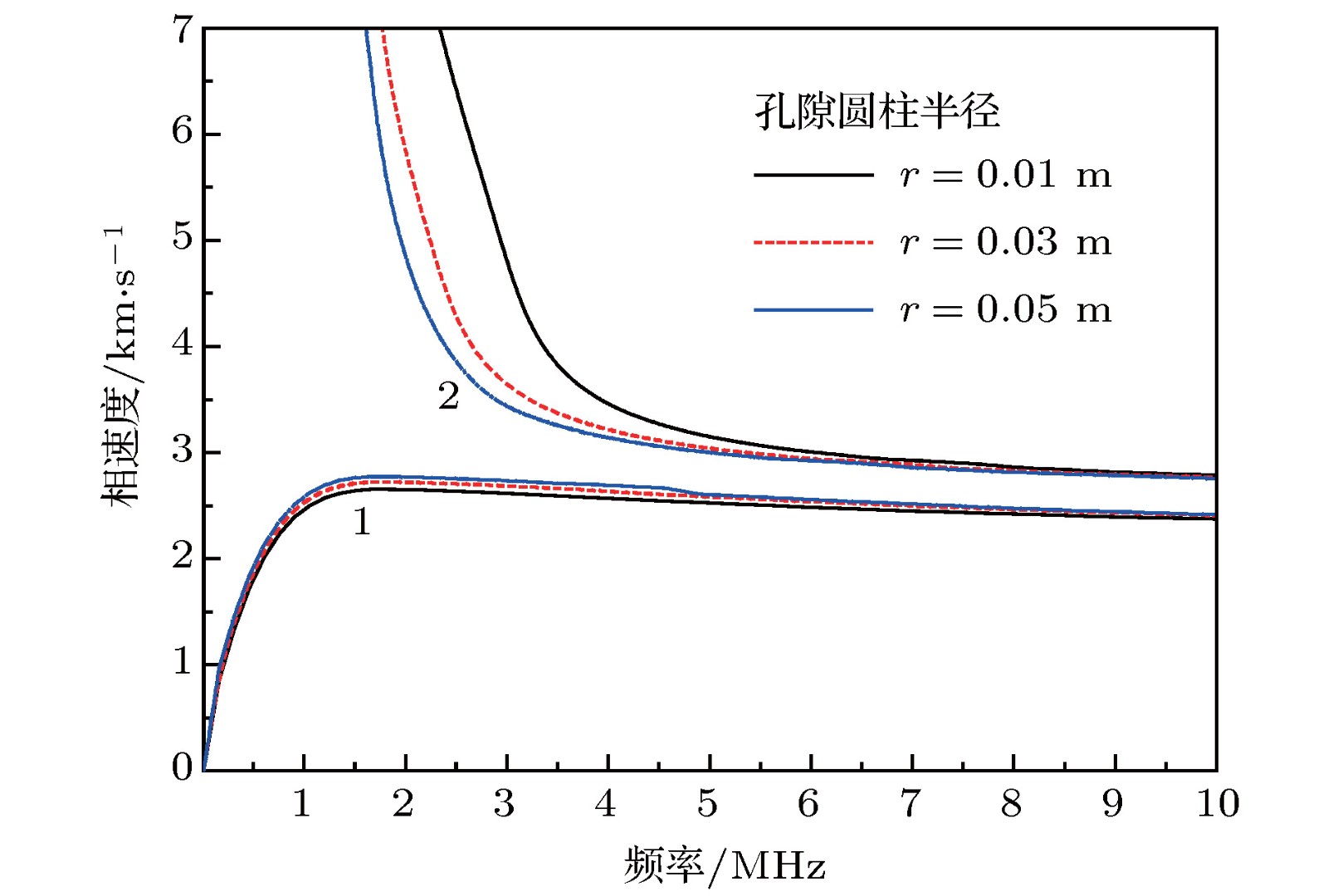
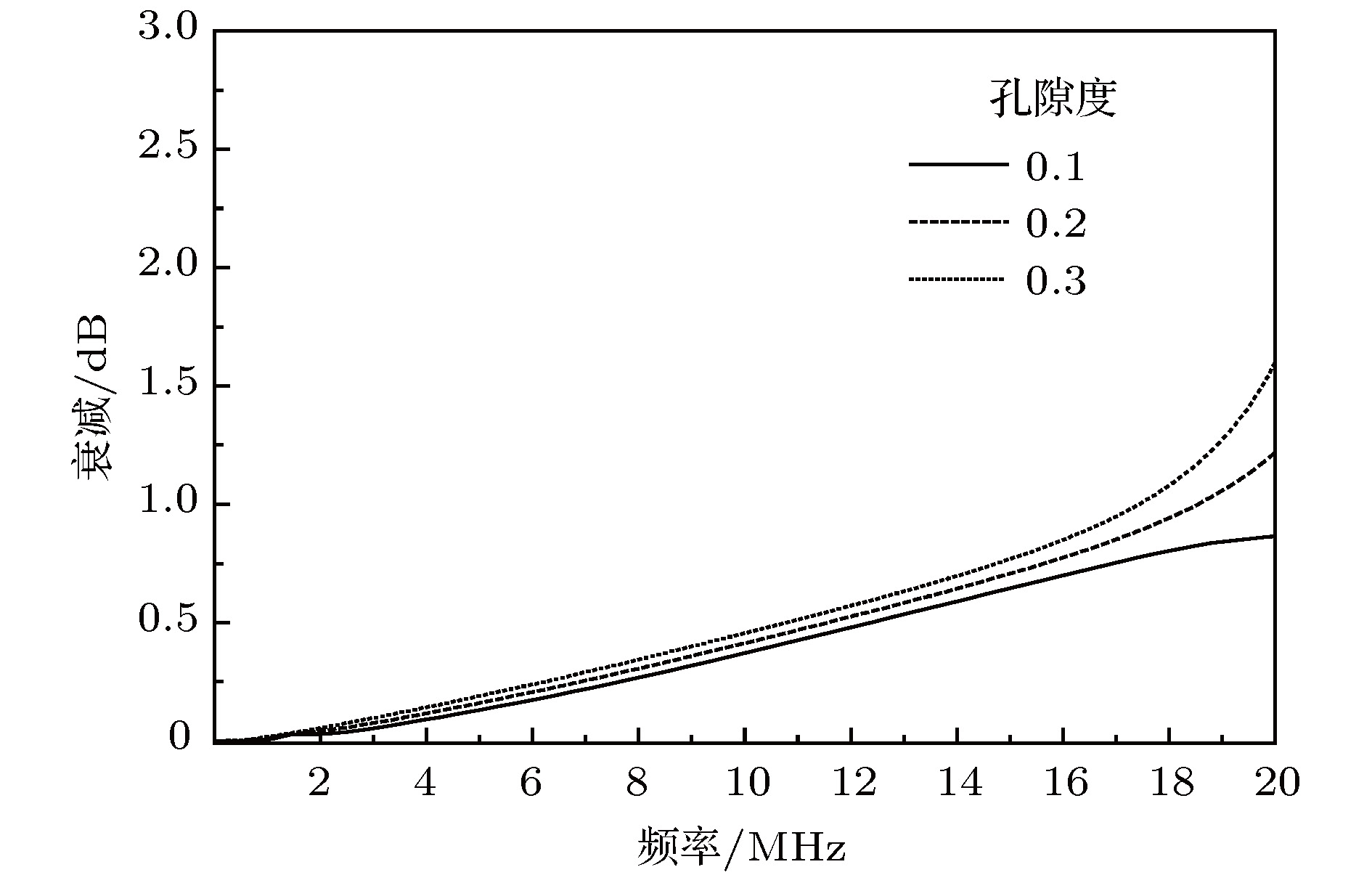
-
Underground water, gas and oil all exist in the fractured or porous strata. Waves that propagate through porous cylinder immersed in infinite fluid are of considerable interest in the estimation of porous parameter, such as an underwater concrete column may present pore characteristics after a long time water immersion. Compared with longitudinal guided wave, circumferential guided wave has its advantages in the ultrasonic nondestructive inspection of porous cylinder. In order to investigate the propagation characteristics of guided waves in a porous cylinder immersed in infinite fluid and analyze the effects of the porous medium parameters on the dispersion characteristic, a model of porous cylinder surrounded by fluid is built. Based on the elastic-dynamic theory and modified liquid-saturated porous theory, the characteristic equation of guided wave is established, and the dispersion curves are obtained numerically. The effects of cylindrical radius and pore parameters on the propagation characteristics of guided waves are discussed; the attenuation characteristics of guided waves are also analyzed; the time domain waveforms of the guided circumferential waves are obtained by numerical inversion, and the influence of porous parameters on waveforms is simulated. It is found that the dispersion curves are similar to that of elastic cylinder in the fluid, there exist multiple mode guided waves and approximate shear velocity of medium for higher modes, and higher order modes are more affected by the radius, but it does not change the tendency of curve. The phase velocity decreases with porosity increasing at the same frequency and the effect of porosity on higher order modes is greater than that on mode 1; due to the dissipation in the medium, the attenuation increases porosity increasing. It can be seen from the transient responses that the wave packets move backward and the displacement amplitude decreases with the porosity increasing. The characteristics of the inversed transient response are in good agreement with theoretical dispersion and attenuation. The results show that the propagation of guided circumferential wave is affected by the pore parameters, especially for porosity, which can provide a theoretical reference for the non-destructive evaluation of the porous cylinder surrounded by infinite fluid.
-
Keywords:
- guided circumferential waves /
- porous media /
- dispersion
[1] 韩庆邦, 徐杉, 谢祖峰, 葛蕤, 王茜, 赵胜永, 朱昌平 2013 62 194301
Google Scholar
Han Q B, Xu B, Xie Z F, Ge R, Wang X, Zhao S Y, Zhu C P 2013 Acta Phys. Sin. 62 194301
Google Scholar
[2] Edelman I, Wilmanski K 2002 Continuum Mech. Thermodyan. 14 25
Google Scholar
[3] Yan S G, Xie F L, Li C Z, Zhang B X 2016 Appl. Geophys. 13 333
Google Scholar
[4] Ding H R, Liu J X, Cui Z W 2017 Chin. Phys. B 26 124301
Google Scholar
[5] Han Q B, Qi L H, Shan M L, Yin, C, Zhu C P 2017 Ultrasonics 81 73
Google Scholar
[6] 董庆德, 王克协, 许吉庆 1985 地球 28 208
Google Scholar
Dong Q D, Wang K X, Xu J Q 1985 Chin. J. Geophys. 28 208
Google Scholar
[7] Zhang S G, Hu W X 2008 Chin. Phys. Lett. 25 4314
Google Scholar
[8] 郭文杰, 李天匀, 朱翔, 屈凯旸 2018 67 084302
Google Scholar
Guo W J, Li T Y, Zhu X, Qu K S 2018 Acta Phys. Sin. 67 084302
Google Scholar
[9] Valle C, Qu J, Jacobs L J 1999 Int. J. Eng. Sci. 37 1369
Google Scholar
[10] Yang W, Fung T C, Chian K S, Chong C K 2007 J. Biomech. 40 481
Google Scholar
[11] 高广健, 邓明晰, 李明亮, 刘畅 2015 64 224301
Google Scholar
Gao G J, Deng M X, Li M L, Liu C 2015 Acta Phys. Sin. 64 224301
Google Scholar
[12] 许洲琛, 韩庆邦, 童紫薇, 齐立华, 王鹏, 单鸣雷, 朱昌平 2018 声学学报 43 52
Xu Z C, Han Q B, Tong Z W, Qi L H, Wang P, Shan M L, Zhu C P 2018 Acta Acust. 43 52
[13] Biot M A 1956 J. Acoust. Soc. Am. 28 167
[14] Ogushwitz P R 1985 J. Acoust. Soc. Am. 77 441
Google Scholar
[15] Johnson D L 1987 J. Fluid Mech. 176 379
Google Scholar
[16] Zheng P, Ding B Y, Zhao S X 2014 Acta Mech. Sin. 30 206
Google Scholar
[17] Biot M A 1957 J. Appl. Mech. 15 594
[18] Butt H S U, Xue P, Jiang T Z, Wang B 2015 Int. J. Mech. Sci. 91 46
Google Scholar
[19] Biot M A 1962 J. Appl. Phys. 33 1482
Google Scholar
[20] 邵广周, 李庆春, 梁志强 2007 地球 50 915
Google Scholar
Shao G Z, Li Q C, Liang Z Q 2007 Chin. J. Geophys. 50 915
Google Scholar
[21] Feng S, Johnson D L 1983 J. Acoust. Soc. Am. 74 906
Google Scholar
[22] Deresiewicz H, Skalak R 1963 Bull. Seismol. Soc. Am. 53 783
[23] 崔寒茵, 师芳芳, 籍顺心, 张碧星 2010 声学学报 35 446
Cui H Y, Shi F F, Ji S X, Zhang B X 2010 Acta Acust. 35 446
[24] 王婷, 崔志文, 刘金霞, 王克协 2018 67 114301
Google Scholar
Wang T, Cui Z W, Liu J X, Wang K X 2018 Acta Phys. Sin. 67 114301
Google Scholar
-
图 1 无限流体中孔隙介质圆柱的示意图
Figure 1. Schematic of porous medium cylinder in an infinite fluid.
图 2 无限流体中孔隙介质圆柱周向导波的相速度频散曲线
Figure 2. The dispersion curves of porous cylinder in infinite fluid.
图 3 无限流体中孔隙介质圆柱周向导波群速度频散曲线的前6阶模态
Figure 3. The first sixth order mode of dispersion curves of porous cylinder in infinite fluid.
图 4 无限流体中弹性圆柱和孔隙介质圆柱周向导波的频散曲线对比
Figure 4. The dispersion curves of circumferential guide waves between an elastic cylinder and a porous cylinder in infinite fluid.
图 5 不同圆柱半径下的导波频散曲线对比
Figure 5. Dispersion curves for different cylinder radius.
图 6 不同孔隙度下的导波频散曲线对比
Figure 6. Dispersion curves versus porosity.
图 7 不同孔隙度时周向导波的衰减曲线对比
Figure 7. Attenuation curves of circumferential waves with different porosity.
图 8 无限流体中孔隙介质圆柱的激发与检测点示意图
Figure 8. Schematic of excitation and detection points of porous medium cylinder in an infinite fluid.
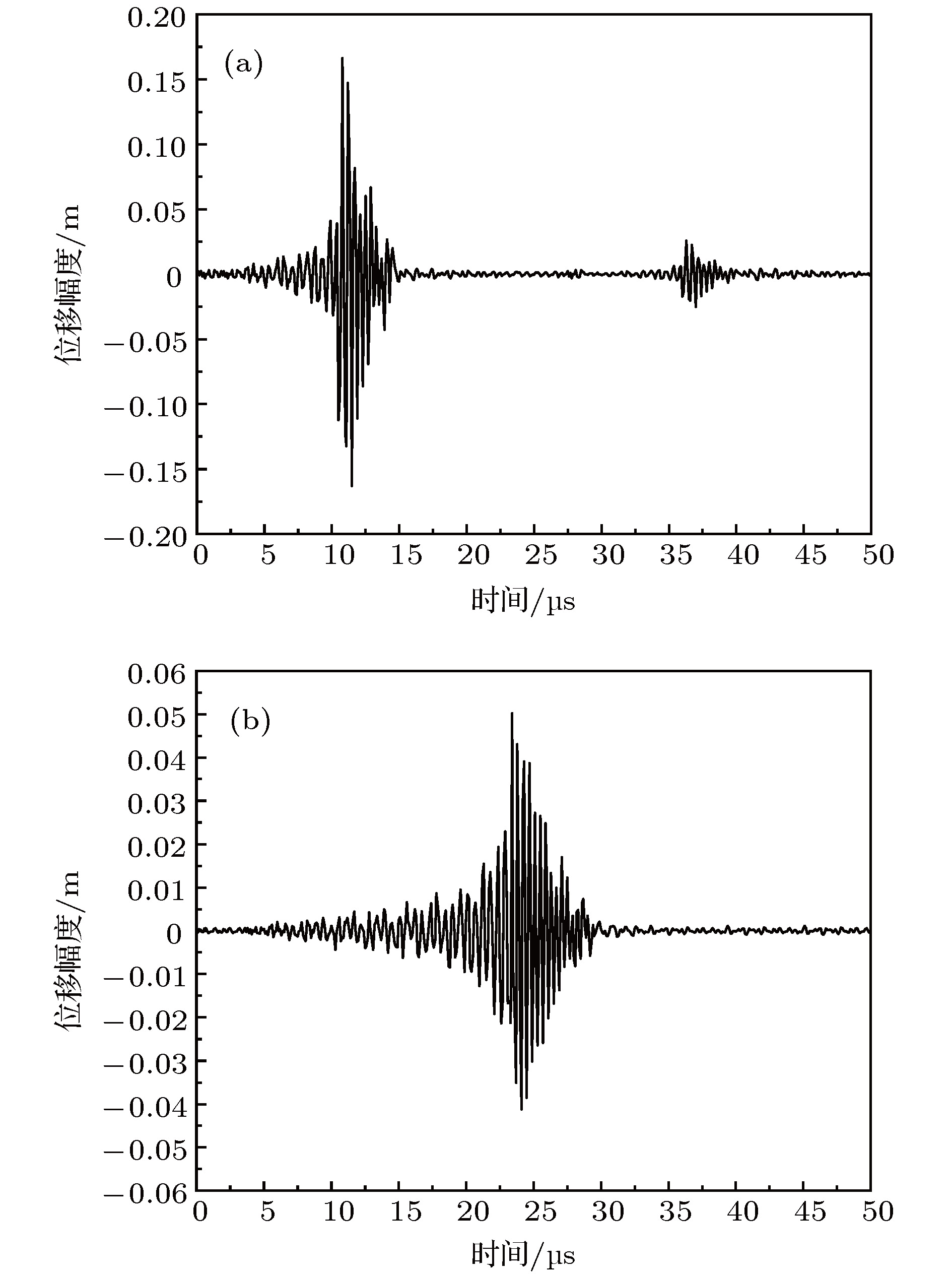
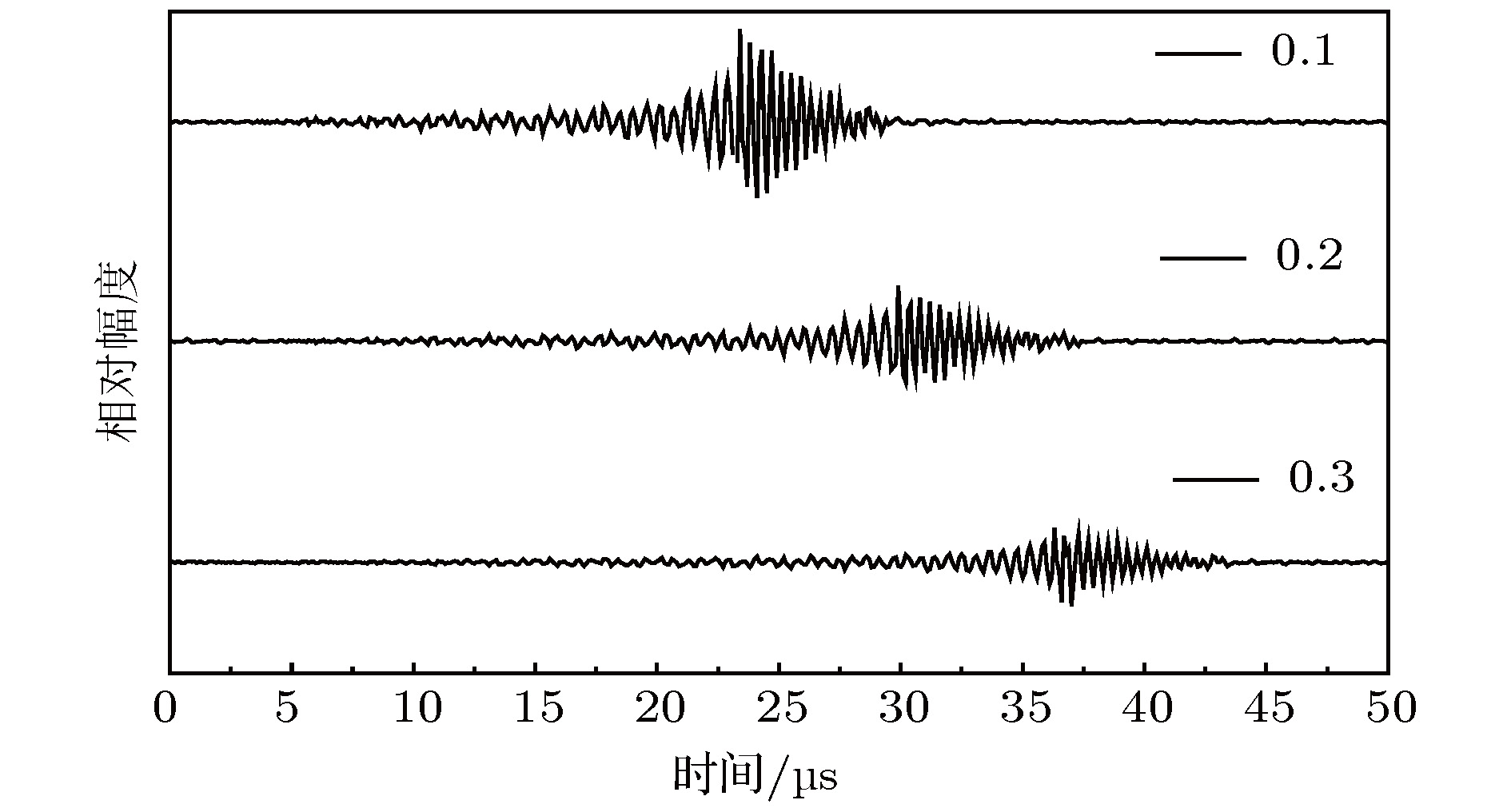
图 9 孔隙度为0.1时, 不同检测点处的径向位移时域波形的对比 (a)检测点在
$\theta = {\text{π}}/2$ 位置; (b)检测点在$\theta = {\text{π}}$ 位置Figure 9. Time-domain waveform of radial displacement at different detection points when the porosity is 0.1: (a) Detection point at
$\theta = {\text{π}}/2$ ; (b) detection point at$\theta = {\text{π}}$ .
图 10 孔隙度为0.1,0.2,0.3时, 检测点在
$\theta = {\text{π}}$ 位置处径向位移时域波形的对比Figure 10. Time-domain waveform of radial displacement of detection point at
$\theta = {\text{π}}$ when the porosity is 0.1, 0.2, 0.3.表 1 模型材料参数表
Table 1. Material parameters.
介质 纵波波速/m·s–1 横波波速/m·s–1 密度/kg·m–3 弹性圆柱 5370 3100 2700 孔隙介质 固体基质 5370 3100 2700 孔隙流体 1483 998 外部流体 1483 998 
DownLoad: CSV
表 2 孔隙介质圆柱参数表
Table 2. Material parameters of porous medium.
孔隙度β/% 孔隙弯曲度a∞ 静态渗透率κ0/m2 黏滞系数n/kg·s–1·m–1 10 5.5 10–12 0.001 固体颗粒体积模量Ks/GPa 流体体积模量Kf/GPa 固体骨架体积模量Kb/GPa 固体骨架剪切模量N/GPa 43.33 2.19 33.70 20.86
DownLoad: CSV
表 3 不同孔隙度时孔隙介质圆柱中体声波的波速
Table 3. Velocity of body sound waves in porous media with different porosity.
孔隙度 快纵波波速/km·s–1 慢纵波波速/km·s–1 横波波速/km·s–1 0.1 4.9690 0.3694 2.8712 0.2 4.4811 0.6748 2.5794 0.3 3.8662 0.8579 2.2006
DownLoad: CSV
-
[1] 韩庆邦, 徐杉, 谢祖峰, 葛蕤, 王茜, 赵胜永, 朱昌平 2013 62 194301
Google Scholar
Han Q B, Xu B, Xie Z F, Ge R, Wang X, Zhao S Y, Zhu C P 2013 Acta Phys. Sin. 62 194301
Google Scholar
[2] Edelman I, Wilmanski K 2002 Continuum Mech. Thermodyan. 14 25
Google Scholar
[3] Yan S G, Xie F L, Li C Z, Zhang B X 2016 Appl. Geophys. 13 333
Google Scholar
[4] Ding H R, Liu J X, Cui Z W 2017 Chin. Phys. B 26 124301
Google Scholar
[5] Han Q B, Qi L H, Shan M L, Yin, C, Zhu C P 2017 Ultrasonics 81 73
Google Scholar
[6] 董庆德, 王克协, 许吉庆 1985 地球 28 208
Google Scholar
Dong Q D, Wang K X, Xu J Q 1985 Chin. J. Geophys. 28 208
Google Scholar
[7] Zhang S G, Hu W X 2008 Chin. Phys. Lett. 25 4314
Google Scholar
[8] 郭文杰, 李天匀, 朱翔, 屈凯旸 2018 67 084302
Google Scholar
Guo W J, Li T Y, Zhu X, Qu K S 2018 Acta Phys. Sin. 67 084302
Google Scholar
[9] Valle C, Qu J, Jacobs L J 1999 Int. J. Eng. Sci. 37 1369
Google Scholar
[10] Yang W, Fung T C, Chian K S, Chong C K 2007 J. Biomech. 40 481
Google Scholar
[11] 高广健, 邓明晰, 李明亮, 刘畅 2015 64 224301
Google Scholar
Gao G J, Deng M X, Li M L, Liu C 2015 Acta Phys. Sin. 64 224301
Google Scholar
[12] 许洲琛, 韩庆邦, 童紫薇, 齐立华, 王鹏, 单鸣雷, 朱昌平 2018 声学学报 43 52
Xu Z C, Han Q B, Tong Z W, Qi L H, Wang P, Shan M L, Zhu C P 2018 Acta Acust. 43 52
[13] Biot M A 1956 J. Acoust. Soc. Am. 28 167
[14] Ogushwitz P R 1985 J. Acoust. Soc. Am. 77 441
Google Scholar
[15] Johnson D L 1987 J. Fluid Mech. 176 379
Google Scholar
[16] Zheng P, Ding B Y, Zhao S X 2014 Acta Mech. Sin. 30 206
Google Scholar
[17] Biot M A 1957 J. Appl. Mech. 15 594
[18] Butt H S U, Xue P, Jiang T Z, Wang B 2015 Int. J. Mech. Sci. 91 46
Google Scholar
[19] Biot M A 1962 J. Appl. Phys. 33 1482
Google Scholar
[20] 邵广周, 李庆春, 梁志强 2007 地球 50 915
Google Scholar
Shao G Z, Li Q C, Liang Z Q 2007 Chin. J. Geophys. 50 915
Google Scholar
[21] Feng S, Johnson D L 1983 J. Acoust. Soc. Am. 74 906
Google Scholar
[22] Deresiewicz H, Skalak R 1963 Bull. Seismol. Soc. Am. 53 783
[23] 崔寒茵, 师芳芳, 籍顺心, 张碧星 2010 声学学报 35 446
Cui H Y, Shi F F, Ji S X, Zhang B X 2010 Acta Acust. 35 446
[24] 王婷, 崔志文, 刘金霞, 王克协 2018 67 114301
Google Scholar
Wang T, Cui Z W, Liu J X, Wang K X 2018 Acta Phys. Sin. 67 114301
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 7756
- PDF Downloads: 62
- Cited By: 0
