-
Based on the quantum mechanics, the physical observables are represented by Hermitian linear operators. Derived from the conservation of energy, these Hermitian operators exhibit real eigenvalues. However, when a closed system described by an effective Hamiltonian is coupled with the surrounding environment, the dynamics of the system itself becomes non-Hermitian dynamic. In general, the eigenvalues of an open optical non-Hermitian system are complex. Parity-time symmetric structure is the system composed of complex potentials, which is neither parity symmetric nor time reversal symmetric alone but is symmetric after operations of parity inversion and time reversal have been combined. The eigenvalue of the parity-time symmetric Hamiltonian can be found to be real, despite the non-Hermitian nature of the system. One of the most attractive properties of non-Hermitian system is the exceptional point, which is degenerate at which two or more eigenvalues and eigenstates of a non-Hermitian physical system coalesce. The unique topological features of EPs, forming a self-intersecting Riemann surface, have given rise to several exotic physical properties. As a kind of phase singularity in a physical system, exceptional point of non-Hermitian system gives rise to a plethora of counterintuitive phenomenon, such as the loss-induced transmission enhancement, unidirectional reflection and asymmetric state transfer. Especially, the eigenvectors of exceptional point are self-orthogonal and an inherent chirality can be determined because of the missing dimension. Chirality lies at the heart of the most fascinating and fundamental phenomena in modern physics, and how to impose a strong chirality and a switchable direction of light propagation in an optical system by steering it to an exceptional point is an interesting research topic. In this work, a non-Hermitian system is constructed based on the special metamaterial resonator of split-ring resonator, in which the sign of coupling coefficient can be flexibly controlled. Especially, the chiral inversion at an exceptional point of non-Hermitian system is observed experimentally. This sign of coupling coefficient controlled exceptional point not only paves a new way for studying the fundamental non-Hermitian physics in an open system, but also holds great potential in the applied photonic devices such as the efficient chiral mode converter and chiral antennas.
-
Keywords:
- non-Hermitian system /
- metamaterial resonator /
- exceptional point /
- chirality
[1] Berry M V 2004 Czech. J. Phys. 54 1039
Google Scholar
[2] El-Ganainy R, Makris K G, Khajavikhan M, Musslimani Z H, Rotter S, Christodoulides D N 2018 Nat. Phys. 14 11
Google Scholar
[3] Bender C M, Boettcher S 1998 Phys. Rev. Lett. 80 5243
Google Scholar
[4] Heiss W D 2012 J. Phys. A:Math. Theor. 45 444016
Google Scholar
[5] Zeng C, Guo Z, Zhu K, Fan C, Li G, Jiang J, Li Y, Jiang H, Yang Y, Sun Y, Chen H 2022 Chin. Phys. B 31 010307
Google Scholar
[6] Guo A, Salamo G J, Duchesne D, et al. 2009 Phys. Rev. Lett. 103 093902
Google Scholar
[7] Feng L, El-Ganainy R, Ge L 2017 Nat. Photonics 11 752
Google Scholar
[8] Özdemir K, Rotter S, Nori F, Yang L 2019 Nat. Mater. 18 783
Google Scholar
[9] Miri M, Alù A 2019 Science 363 eaar7709
Google Scholar
[10] Shi C, Dubois M, Chen Y, Cheng L, Ramezani H, Wang Y, Zhang X 2016 Nat. Commun. 7 11110
Google Scholar
[11] Ding K, Ma G, Xiao M, Zhang Z Q, Chan C T 2016 Phys. Rev. X 6 021007
[12] Tang W, Jiang X, Ding K, Xiao Y X, Zhang Z Q, Chan C T, Ma G 2020 Science 370 1077
Google Scholar
[13] Assawaworrarit S, Yu X, Fan S 2017 Nature 546 387
Google Scholar
[14] Choi Y, Hahn C, Yoon J W, Song S H 2018 Nat. Commun. 9 2182
Google Scholar
[15] Xiao Z, Li H, Kottos T, Alù A 2019 Phys. Rev. Lett. 123 213901
Google Scholar
[16] Peng B, Özdemir K, Rotterh S, Yilmaz H, Liertzer M, Monifi F, Bender C, Nori F, Yang L 2014 Science 346 328
Google Scholar
[17] Lin Z, Ramezani H, Eichelkraut T, Kottos T, Cao H, Christodoulides D N 2011 Phys. Rev. Lett. 106 213901
Google Scholar
[18] Song J, Yang F, Guo Z, Wu X, Zhu K, Jiang J, Sun Y, Li Y, Jiang H, Chen H 2021 Phys. Rev. Appl. 15 014009
Google Scholar
[19] Shu X, Li A, Hu G, Wang J, Alù A, Chen L 2022 Nat. Commun. 13 2123
Google Scholar
[20] Schumer A, Liu Y, Leshin J, Ding L, et al. 2022 Science 375 884
Google Scholar
[21] Hodaei H, Hassan A U, Wittek S, et al. 2017 Nature 548 187
Google Scholar
[22] Chen W J, Özdemir K, Zhao G M, Wiersig J, Yang L 2017 Nature 548 192
Google Scholar
[23] Guo Z, Zhang T, Song J, Jiang H, Chen H 2021 Photonics Res. 9 574
Google Scholar
[24] Guo Z, Jiang J, Jiang H, et al. 2021 Phys. Rev. Res. 3 013122
Google Scholar
[25] Doppler J, Mailybaev A, Böhm J, et al. 2016 Nature 537 76
Google Scholar
[26] Liu Q, Li S, Wang B, Ke S, Qin C, Wang K, Liu W, Gao D, Berini P, Lu P 2020 Phys. Rev. Lett. 124 153903
Google Scholar
[27] Song Q H, Odeh M, Zúñiga-Pérez J, Kanté B, and Genevet P 2021 Science 373 1133
Google Scholar
[28] Dembowski C, Dietz B, Gräf H D, Harney H L, Heine A, Heiss W D, Richter A 2003 Phys. Rev. Lett. 90 034101
Google Scholar
[29] Cao Q T, Wang H M, Dong C H, Jing H, Liu R S, Chen X, Ge L, Gong Q H, Xiao Y F 2017 Phys. Rev. Lett. 118 033901
Google Scholar
[30] Chen H, Liu T, Luan H, et al. 2020 Nat. Phys. 16 571
Google Scholar
[31] Wang C Q, Sweeney W R, Stone A D, Yang L 2021 Science 373 1261
Google Scholar
[32] Keil R, Poli C, Heinrich M, Arkinstall J, Weihs G, Schomerus H, Szameit A 2016 Phys. Rev. Lett. 116 213901
Google Scholar
[33] Fu N, Fu Z, Zhang H, Liao Q, Zhao D, Ke S 2020 Opt. Quantum Electron. 52 61
Google Scholar
[34] Ke S, Wang B, Qin C, Long H, Wang K, Lu P 2016 J. Lightwave Technol. 34 5258
Google Scholar
[35] Guo Z, Jiang H, Chen H 2022 J. Phys. D:Appl. Phys. 55 083001
Google Scholar
[36] 梁浩, 李剑生, 郭云胜 2014 63 144101
Liang H, Li J S, Guo Y S 2014 Acta Phys. Sin. 63 144101
[37] 张萌徕, 覃赵福, 陈卓 2021 70 054206
Google Scholar
Zhang M L, Qin Z F, Chen Z 2021 Acta Phys. Sin. 70 054206
Google Scholar
[38] Pendry J B, Holden A J, Robbins D J, Stewart W J 1999 IEEE Trans. Microwave Theory Tech. 47 2075
Google Scholar
[39] Pendry J B 2000 Phys. Rev. Lett. 85 3966
Google Scholar
[40] Shamonina E 2008 Phys. Status Solidi B 245 1471
Google Scholar
[41] Jiang J, Guo Z, Ding Y, Sun Y, Li Y, Jiang H, Chen H 2018 Opt. Express 26 12891
Google Scholar
[42] Guo Z, Jiang H, Sun Y, Li Y, Chen H 2018 Opt. Lett. 43 5142
Google Scholar
[43] Jiang J, Ren J, Guo Z, Zhu W, Long Y, Jiang H, Chen H 2020 Phys. Rev. B 101 165427
Google Scholar
[44] Liu H, Genov D A, Wu D M, Liu Y M, Liu Z W, Sun C, Zhu S N, Zhang X 2007 Phys. Rev. B 76 073101
Google Scholar
[45] Grbic A, Eleftheriades G V 2004 Phys. Rev. Lett. 92 117403
Google Scholar
[46] Guo Z, Jiang H, Zhu K, Sun Y, Li Y, Chen H 2018 Phys. Rev. Appl. 10 064048
Google Scholar
[47] Guo Z, Long Y, Jiang H, et al. 2021 Adv. Photonics 3 036001
[48] Guo Z, Jiang H, Li Y, et al. 2018 Opt. Express 26 627
Google Scholar
[49] Li Y, Sun Y, Zhu W, et al. 2018 Nat. Commun. 9 4598
Google Scholar
[50] Hadad Y, Soric J C, Khanikaev A B, Alù A 2018 Nat. Electron. 1 178
Google Scholar
-
图 1 两个共振原子近场耦合组成的二阶非厄米系统
Figure 1. A second-order non-Hermitian system composed of two coupled resonant atoms with near-field coupling.
图 2 在耦合系数
$ \kappa $ 和本征损耗因子${\varGamma _2}$ 构成的参数空间内, 非厄米系统本征频率 (a)实部和(b)虚部的黎曼表面Figure 2. The Riemannian surface of the (a) real part and (b)imaginary part of the eigenfrequency of the non-Hermitian system in the parameter space composed of the coupling coefficient
$ \kappa $ and the intrinsic loss factor${\varGamma _2}$ 
图 3 开口谐振环不同相对转角下的耦合情况 (a)开口谐振环相对夹角为
$ \theta ={180}^{\circ} $ 时的负耦合; (b)开口谐振环相对夹角为$ \theta = {0^\circ} $ 的正耦合Figure 3. The coupling of the split-ring resonator under different relative rotation angles: (a) The negative coupling when the relative rotation angle of the split-ring resonators is
$ \theta = {180^\circ} $ ; (b) the positive coupling when the relative rotation angle of the split-ring resonators is$ \theta = {0^\circ} $ .
图 4 开口谐振环相对夹角为 (a)
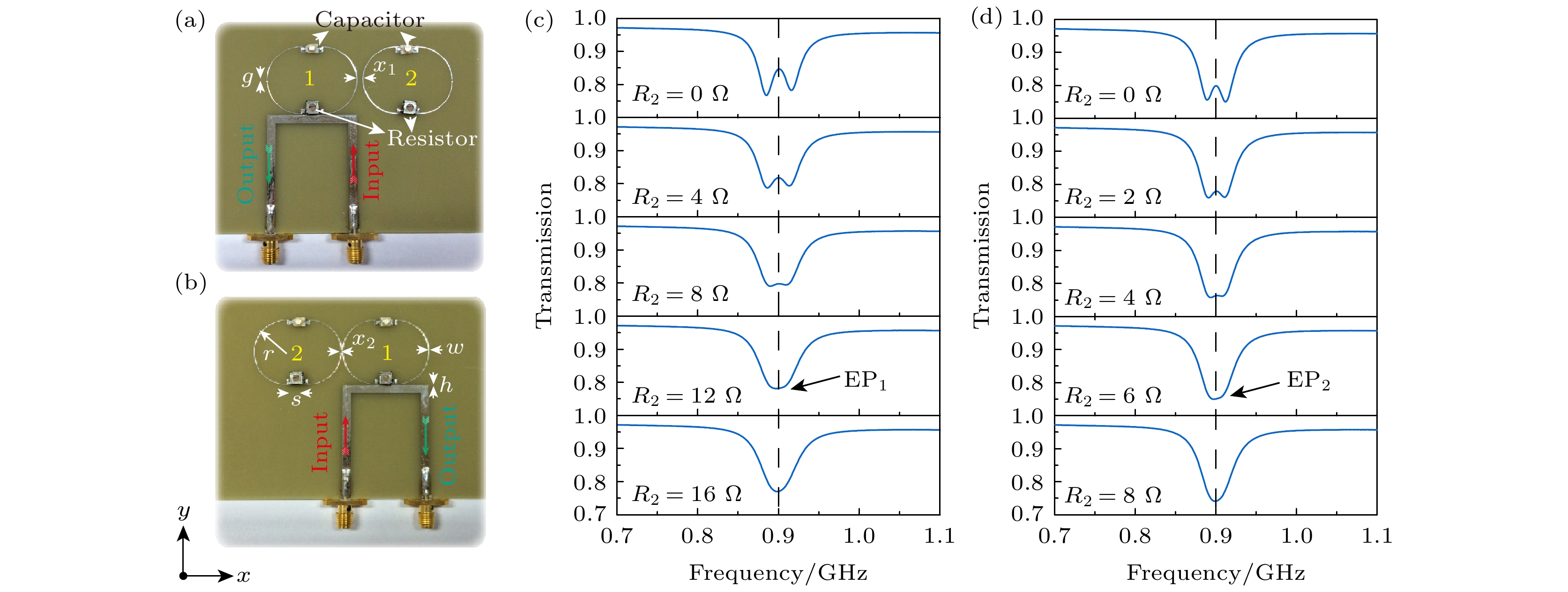
$\theta = {180^\circ}$ 和(b)$ \theta = {0^\circ} $ 时构造的非厄米系统实验样品图; (c)$ \theta = {180^\circ} $ 和(d)$ \theta = {0^\circ} $ 对应的非厄米系统中, 调节集总电阻的阻值$ {R_2} $ 时测得的透射谱Figure 4. Sample photos of the non-Hermitian system with relative rotation angle between split-ring resonators is (a)
$ \theta = {180^\circ} $ and (b)$ \theta = {0^\circ} $ ; The corresponding transmittance spectrum of the non-Hermitian system with (c)$ \theta = {180^\circ} $ and (d)$ \theta = {0^\circ} $ as a function of lumped resistance$ {R_2} $ .
图 5 实验观测当
${\varGamma _2}$ 变化时, 开口谐振环相对夹角为 (a)$\theta = {180^\circ}$ 和(b)$\theta = {0^\circ}$ 构成的非厄米系统的本征频率实部(上图)和虚部(下图)Figure 5. Experimental measured the eigenfrequeies of the non-Hermitian system as a function of
${\varGamma _2}$ when the relative rotation angle between split-ring resonators is (a)$\theta = {180^\circ}$ and(b)$\theta = {0^\circ}$ . The upper and lower rows denote the real part and imaginary part, respectively.
图 6 实验测得开口谐振环相对夹角为
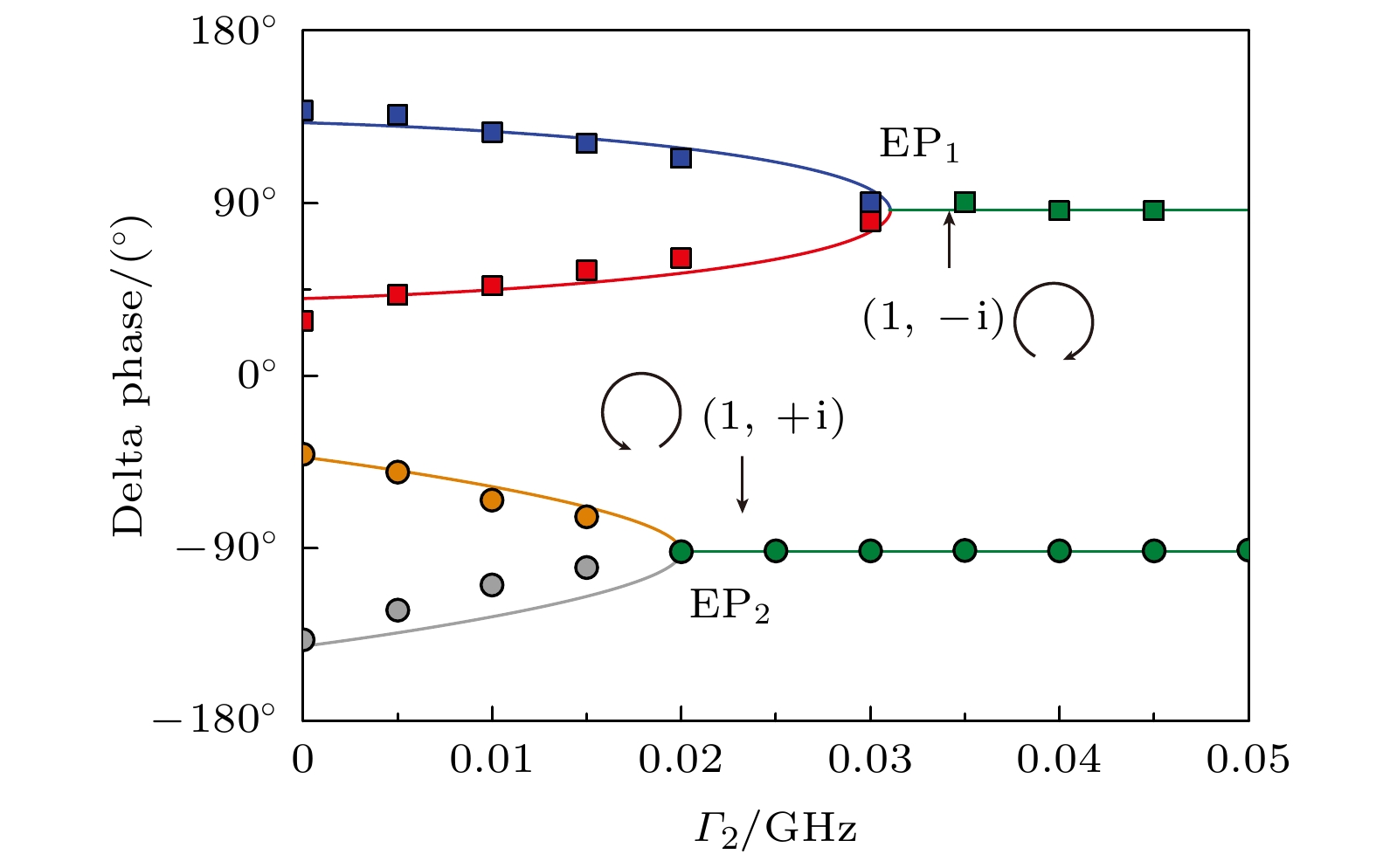
$ \theta = {180^\circ} $ (上图)和$ \theta = {0^\circ} $ (下图)构成的非厄米系统中, 不同的本征损耗因子$ {\varGamma _2} $ 两个振子的相位差$ \Delta \varphi = {\varphi _1} - {\varphi _2} $ Figure 6. Measured phase difference between two resonant atoms
$ \Delta \varphi = {\varphi _1} - {\varphi _2} $ for different intrinsic loss factor$ {\varGamma _2} $ in the non-Hermitian systems exhibiting the intrinsic chirality of EP. The upper and lower figures denote the relative rotation angle between split-ring resonators is$ \theta = {180^\circ} $ and$ \theta = {0^\circ} $ , respectively.
图 7 全场数值仿真得到的开口谐振环相对夹角为 (a)
$\theta = $ $ {180^\circ}$ 和(b)$\theta = {0^\circ}$ 构成的非厄米系统中, EP位置手性态的场分布仿真演化图Figure 7. Full-wave numerical simulated evolution of field distribution for the non-Hermitian system with the relative rotation angle between split-ring resonators is (a)
$\theta = {180^\circ}$ and (b)$\theta = {0^\circ}$ -
[1] Berry M V 2004 Czech. J. Phys. 54 1039
Google Scholar
[2] El-Ganainy R, Makris K G, Khajavikhan M, Musslimani Z H, Rotter S, Christodoulides D N 2018 Nat. Phys. 14 11
Google Scholar
[3] Bender C M, Boettcher S 1998 Phys. Rev. Lett. 80 5243
Google Scholar
[4] Heiss W D 2012 J. Phys. A:Math. Theor. 45 444016
Google Scholar
[5] Zeng C, Guo Z, Zhu K, Fan C, Li G, Jiang J, Li Y, Jiang H, Yang Y, Sun Y, Chen H 2022 Chin. Phys. B 31 010307
Google Scholar
[6] Guo A, Salamo G J, Duchesne D, et al. 2009 Phys. Rev. Lett. 103 093902
Google Scholar
[7] Feng L, El-Ganainy R, Ge L 2017 Nat. Photonics 11 752
Google Scholar
[8] Özdemir K, Rotter S, Nori F, Yang L 2019 Nat. Mater. 18 783
Google Scholar
[9] Miri M, Alù A 2019 Science 363 eaar7709
Google Scholar
[10] Shi C, Dubois M, Chen Y, Cheng L, Ramezani H, Wang Y, Zhang X 2016 Nat. Commun. 7 11110
Google Scholar
[11] Ding K, Ma G, Xiao M, Zhang Z Q, Chan C T 2016 Phys. Rev. X 6 021007
[12] Tang W, Jiang X, Ding K, Xiao Y X, Zhang Z Q, Chan C T, Ma G 2020 Science 370 1077
Google Scholar
[13] Assawaworrarit S, Yu X, Fan S 2017 Nature 546 387
Google Scholar
[14] Choi Y, Hahn C, Yoon J W, Song S H 2018 Nat. Commun. 9 2182
Google Scholar
[15] Xiao Z, Li H, Kottos T, Alù A 2019 Phys. Rev. Lett. 123 213901
Google Scholar
[16] Peng B, Özdemir K, Rotterh S, Yilmaz H, Liertzer M, Monifi F, Bender C, Nori F, Yang L 2014 Science 346 328
Google Scholar
[17] Lin Z, Ramezani H, Eichelkraut T, Kottos T, Cao H, Christodoulides D N 2011 Phys. Rev. Lett. 106 213901
Google Scholar
[18] Song J, Yang F, Guo Z, Wu X, Zhu K, Jiang J, Sun Y, Li Y, Jiang H, Chen H 2021 Phys. Rev. Appl. 15 014009
Google Scholar
[19] Shu X, Li A, Hu G, Wang J, Alù A, Chen L 2022 Nat. Commun. 13 2123
Google Scholar
[20] Schumer A, Liu Y, Leshin J, Ding L, et al. 2022 Science 375 884
Google Scholar
[21] Hodaei H, Hassan A U, Wittek S, et al. 2017 Nature 548 187
Google Scholar
[22] Chen W J, Özdemir K, Zhao G M, Wiersig J, Yang L 2017 Nature 548 192
Google Scholar
[23] Guo Z, Zhang T, Song J, Jiang H, Chen H 2021 Photonics Res. 9 574
Google Scholar
[24] Guo Z, Jiang J, Jiang H, et al. 2021 Phys. Rev. Res. 3 013122
Google Scholar
[25] Doppler J, Mailybaev A, Böhm J, et al. 2016 Nature 537 76
Google Scholar
[26] Liu Q, Li S, Wang B, Ke S, Qin C, Wang K, Liu W, Gao D, Berini P, Lu P 2020 Phys. Rev. Lett. 124 153903
Google Scholar
[27] Song Q H, Odeh M, Zúñiga-Pérez J, Kanté B, and Genevet P 2021 Science 373 1133
Google Scholar
[28] Dembowski C, Dietz B, Gräf H D, Harney H L, Heine A, Heiss W D, Richter A 2003 Phys. Rev. Lett. 90 034101
Google Scholar
[29] Cao Q T, Wang H M, Dong C H, Jing H, Liu R S, Chen X, Ge L, Gong Q H, Xiao Y F 2017 Phys. Rev. Lett. 118 033901
Google Scholar
[30] Chen H, Liu T, Luan H, et al. 2020 Nat. Phys. 16 571
Google Scholar
[31] Wang C Q, Sweeney W R, Stone A D, Yang L 2021 Science 373 1261
Google Scholar
[32] Keil R, Poli C, Heinrich M, Arkinstall J, Weihs G, Schomerus H, Szameit A 2016 Phys. Rev. Lett. 116 213901
Google Scholar
[33] Fu N, Fu Z, Zhang H, Liao Q, Zhao D, Ke S 2020 Opt. Quantum Electron. 52 61
Google Scholar
[34] Ke S, Wang B, Qin C, Long H, Wang K, Lu P 2016 J. Lightwave Technol. 34 5258
Google Scholar
[35] Guo Z, Jiang H, Chen H 2022 J. Phys. D:Appl. Phys. 55 083001
Google Scholar
[36] 梁浩, 李剑生, 郭云胜 2014 63 144101
Liang H, Li J S, Guo Y S 2014 Acta Phys. Sin. 63 144101
[37] 张萌徕, 覃赵福, 陈卓 2021 70 054206
Google Scholar
Zhang M L, Qin Z F, Chen Z 2021 Acta Phys. Sin. 70 054206
Google Scholar
[38] Pendry J B, Holden A J, Robbins D J, Stewart W J 1999 IEEE Trans. Microwave Theory Tech. 47 2075
Google Scholar
[39] Pendry J B 2000 Phys. Rev. Lett. 85 3966
Google Scholar
[40] Shamonina E 2008 Phys. Status Solidi B 245 1471
Google Scholar
[41] Jiang J, Guo Z, Ding Y, Sun Y, Li Y, Jiang H, Chen H 2018 Opt. Express 26 12891
Google Scholar
[42] Guo Z, Jiang H, Sun Y, Li Y, Chen H 2018 Opt. Lett. 43 5142
Google Scholar
[43] Jiang J, Ren J, Guo Z, Zhu W, Long Y, Jiang H, Chen H 2020 Phys. Rev. B 101 165427
Google Scholar
[44] Liu H, Genov D A, Wu D M, Liu Y M, Liu Z W, Sun C, Zhu S N, Zhang X 2007 Phys. Rev. B 76 073101
Google Scholar
[45] Grbic A, Eleftheriades G V 2004 Phys. Rev. Lett. 92 117403
Google Scholar
[46] Guo Z, Jiang H, Zhu K, Sun Y, Li Y, Chen H 2018 Phys. Rev. Appl. 10 064048
Google Scholar
[47] Guo Z, Long Y, Jiang H, et al. 2021 Adv. Photonics 3 036001
[48] Guo Z, Jiang H, Li Y, et al. 2018 Opt. Express 26 627
Google Scholar
[49] Li Y, Sun Y, Zhu W, et al. 2018 Nat. Commun. 9 4598
Google Scholar
[50] Hadad Y, Soric J C, Khanikaev A B, Alù A 2018 Nat. Electron. 1 178
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 6662
- PDF Downloads: 478
- Cited By: 0
