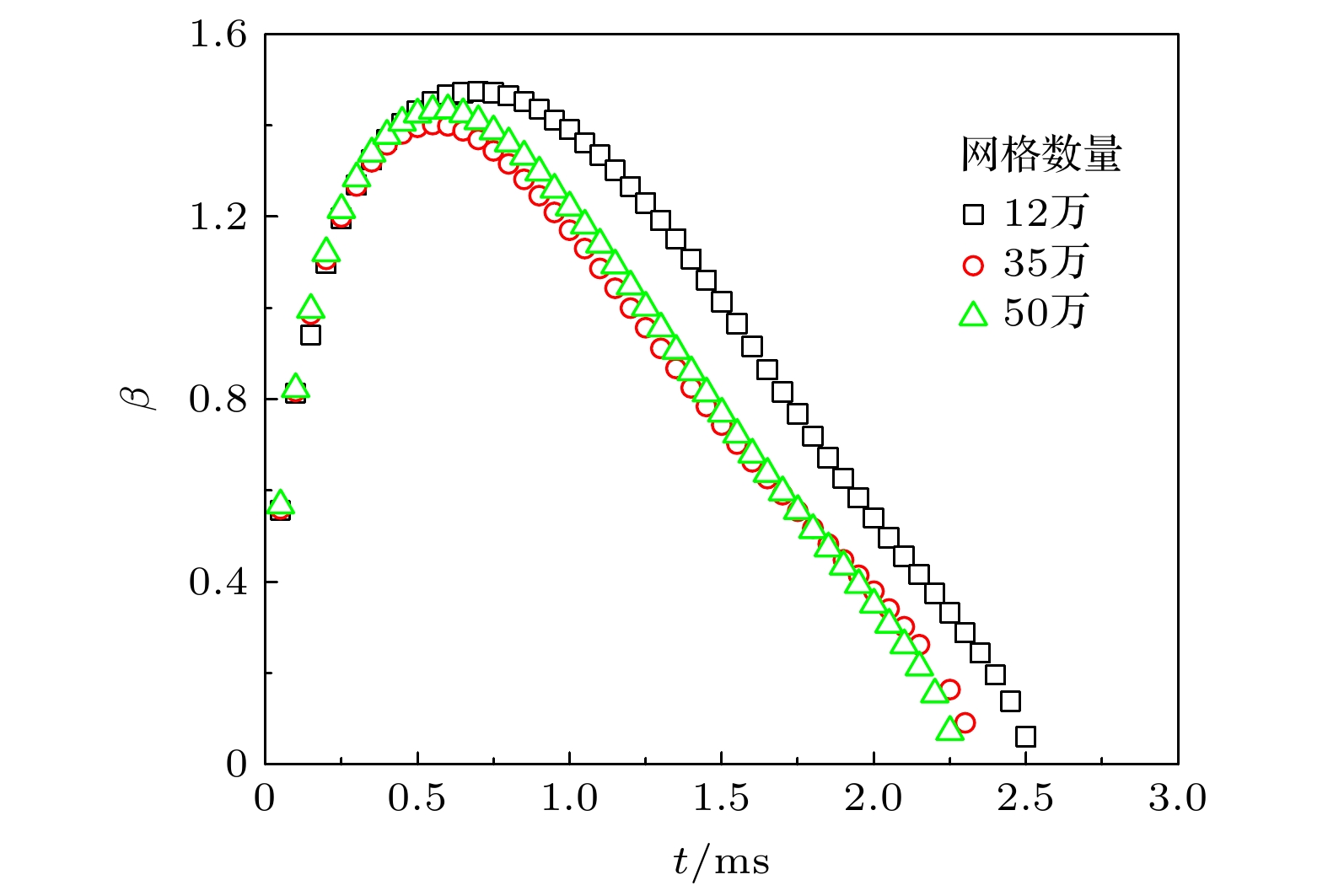
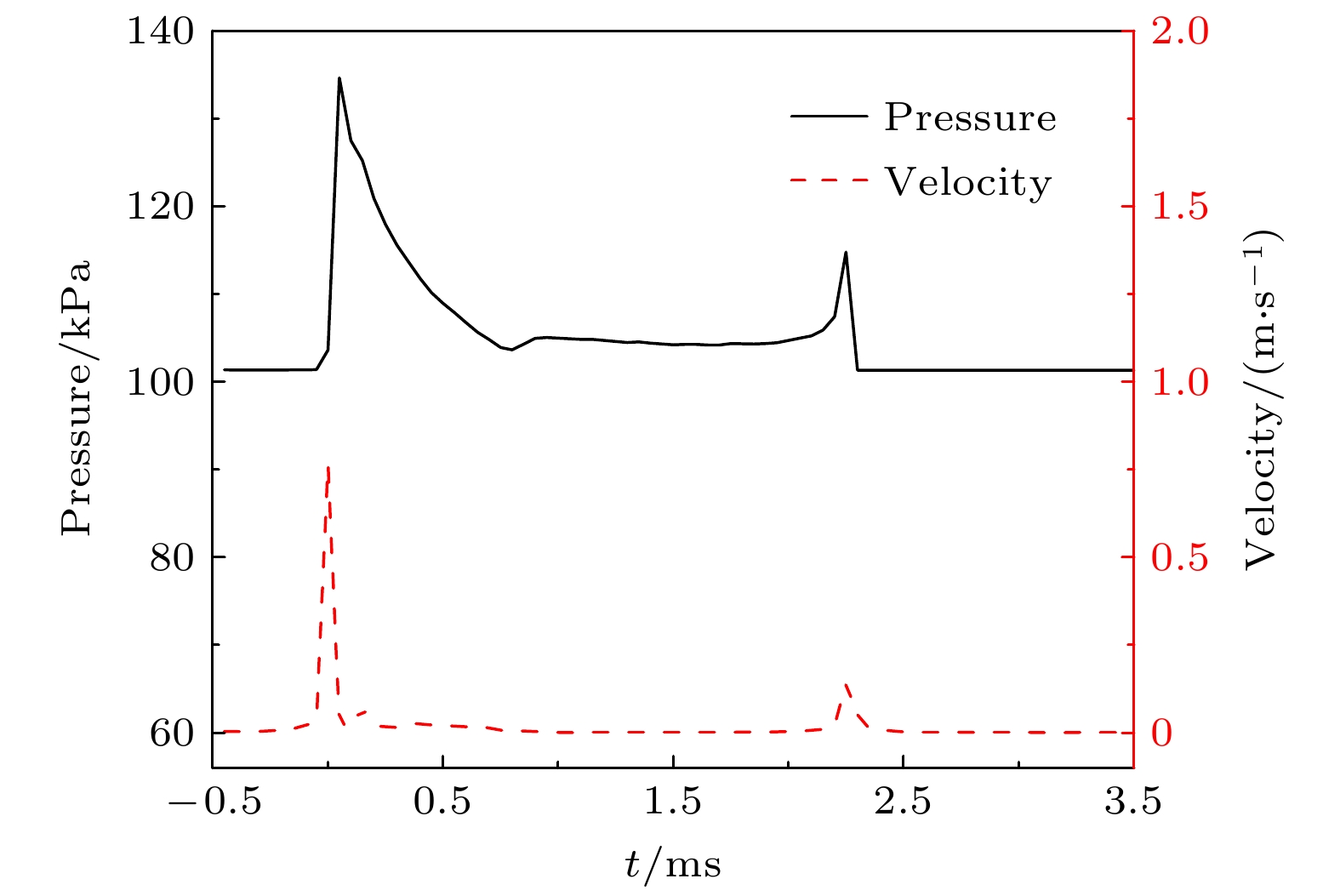
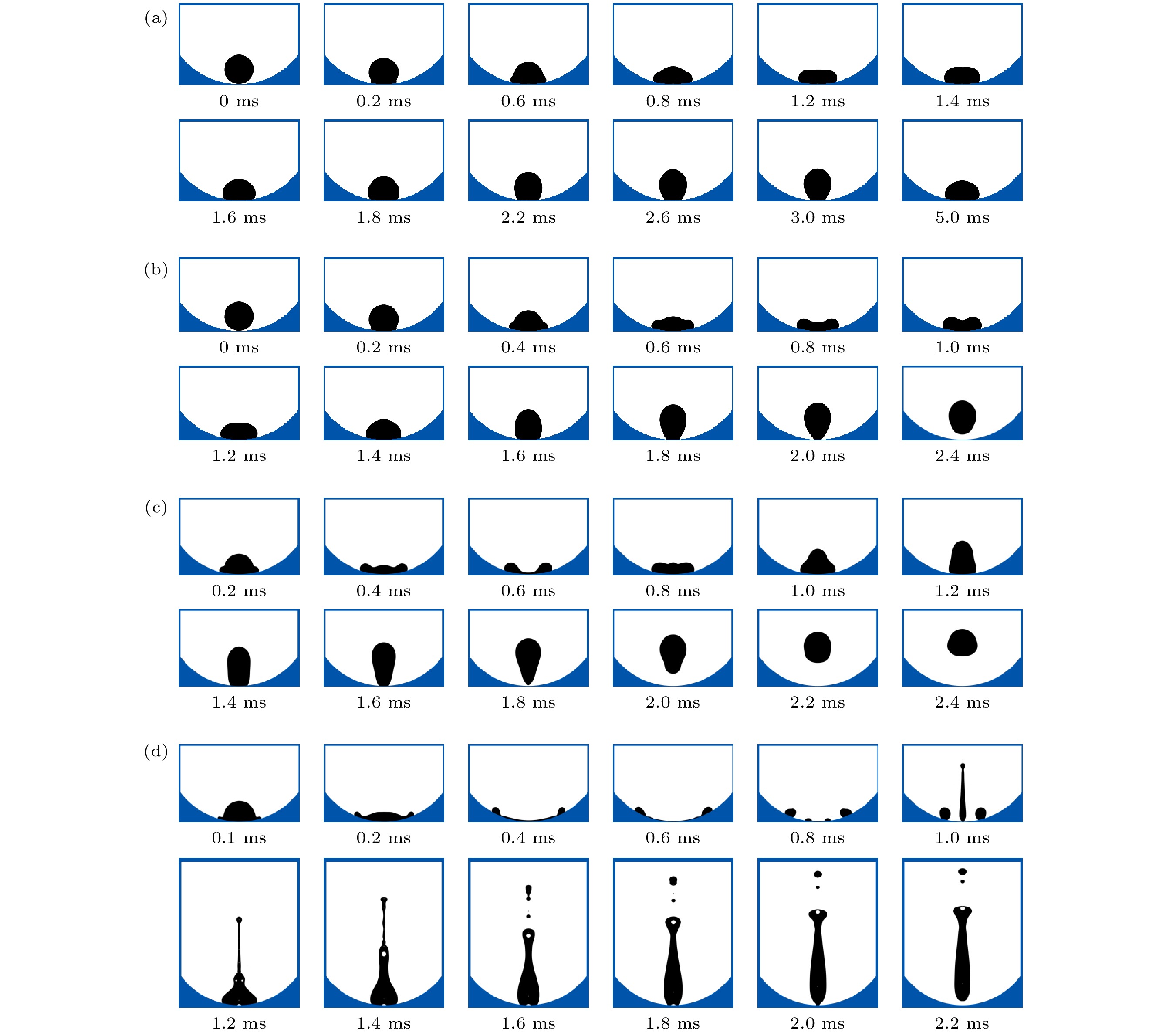
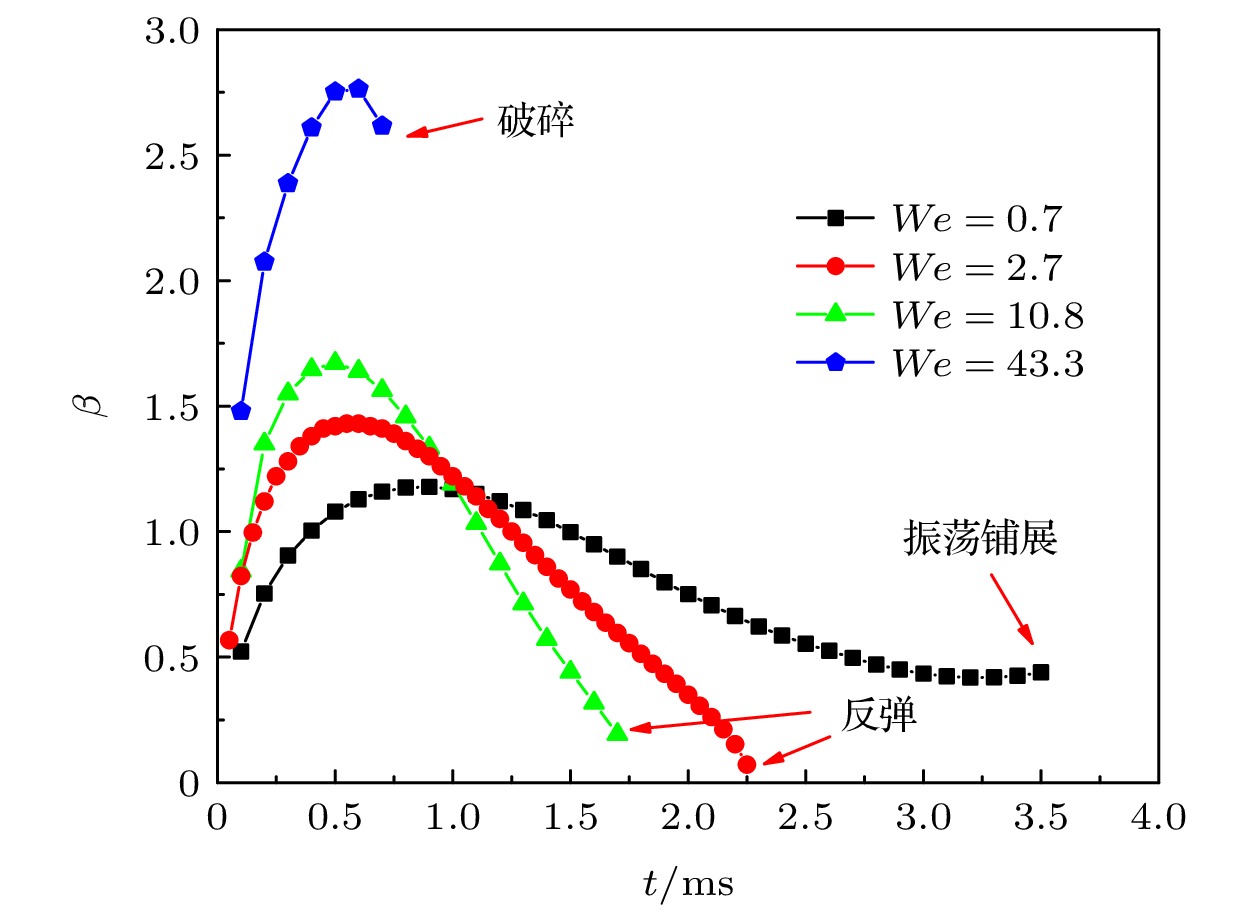
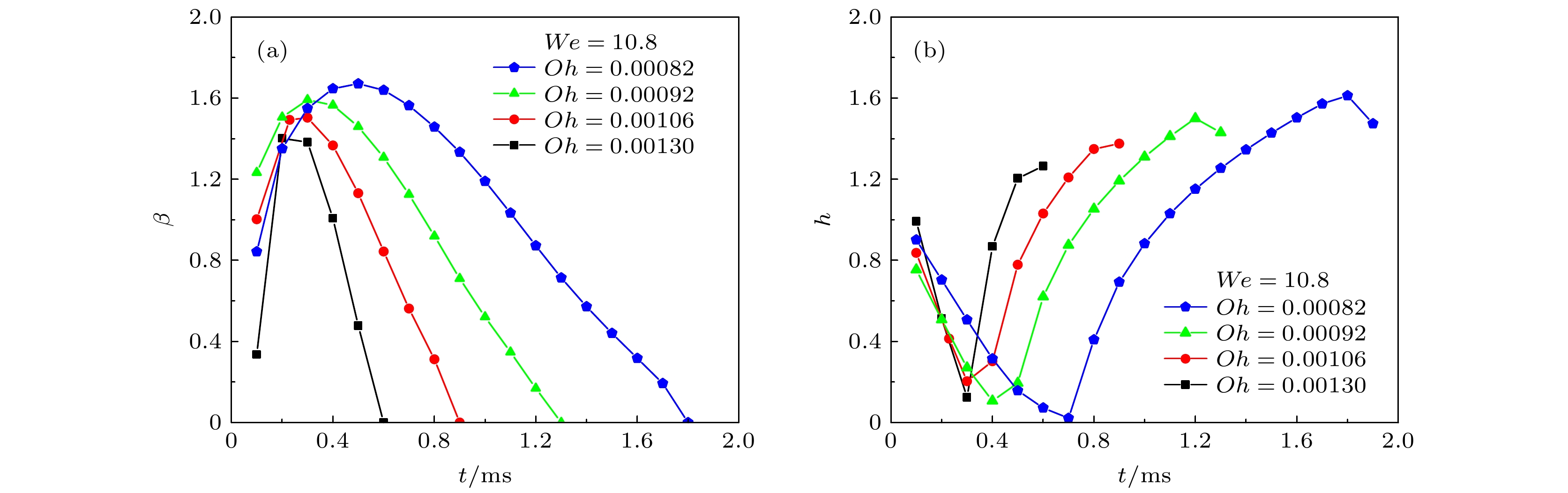
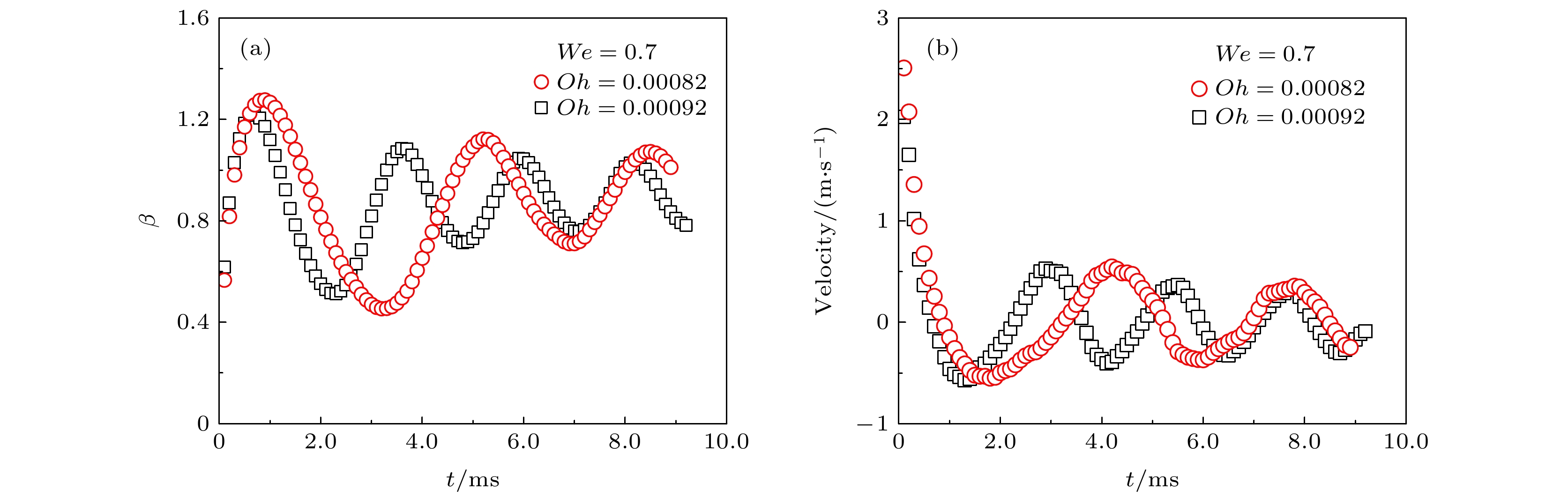
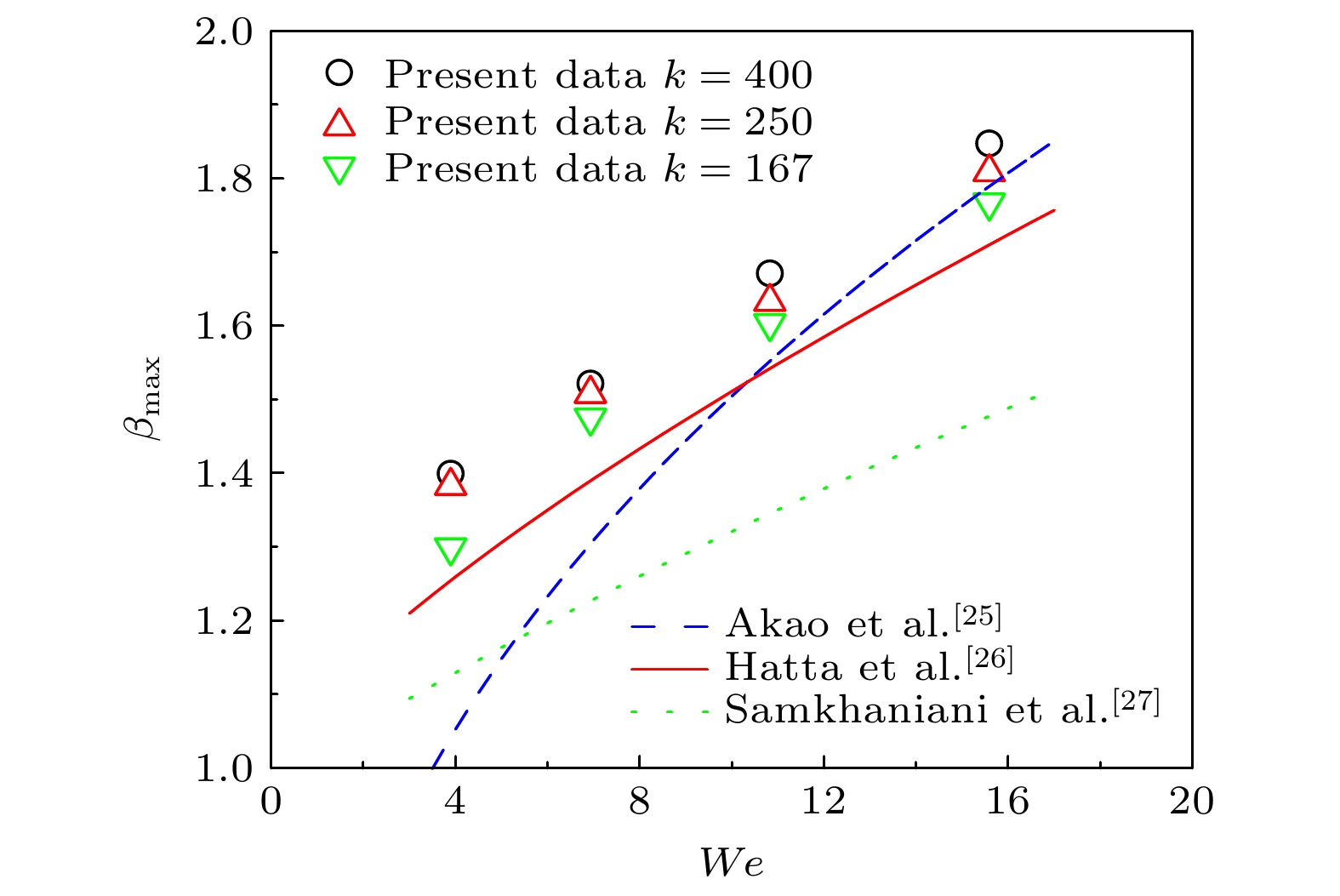
-
In order to reveal the mechanism of reaction between aluminum droplet and curved wall, a numerical calculation model based on the volume of fluid method of aluminum droplet impacting curved wall is established. By analyzing the influence law of Weber number, Ohnesorge number and wall curvature on the process of droplet impacting the wall, the spreading characteristics and flow mechanism of droplet on curved surface are studied. The results show that the flow characteristics of aluminum droplets after impacting the wall are affected not only by inertial force, surface tension, and viscous force, but also by the structure of the wall. The behavior patterns of the droplets contain adhesion, rebound and splash under different Weber numbers. Because energy dissipation is produced in both spreading process and retracting process, the retracting speed of droplet is always less than its spreading speed. During the flow of the droplet, there are two pressure peaks and velocity peaks at the contact point, while the two peaks appear respectively at the moment when the droplet impacts the wall and when the droplet is about to rebound. In the behavioral mode of rebound, as Ohnesorge number increases, the maximum spreading diameter of the droplet gradually decreases, and the contact time is shorter. In the behavioral mode of adhesion, the spreading radius of the droplets is of oscillatory decay. Within the same period, the maximum spreading coefficient of the larger-Ohnesorge number droplets is smaller, and the decay rate is faster and the oscillation period is shorter. With the increase of wall curvature, the maximum spreading coefficient of droplet increases and that on the plane is the minimum. Based on the calculation results, the empirical formula is revised. Compared with the previous formula, it can well predict the maximum spreading coefficient on the curved surface, whose average error is within 3%. Further, according to the conservation of energy, theoretical models which predict the maximum spreading coefficients when droplets impact a curved and plate wall are also established. Compared with the scenario on the plane, the spreading coefficient of droplet on the curved surface is related to not only the motion parameters of droplet and the wettability of wall surface, but also the ratio of wall curvature to droplet curvature. More importantly, the new theoretical model takes into account the coupling effects of Weber number, Reynolds number, curvature ratio and contact angle, so it has stronger applicability and better robustness. The research results in this work will provide the theoretical basis for practical engineering application.
-
Keywords:
- aluminum droplet /
- curved surface /
- volume of fluid method /
- prediction model
[1] Yi H, Qi L, Luo J, Zhang D, Li H, Hou X 2018 Int. J. Mach. Tools Manuf. 130–131 1
[2] 李涛 2020 博士学位论文 (济南: 山东大学)
Li T 2020 Ph. D. Dissertation (Jinan: Shandong University) (in Chinese)
[3] Rioboo R, Tropea C, Marengo M 2001 Atomization Sprays 11 155
Google Scholar
[4] Rioboo R, Marengo M, Tropea C 2002 Exp. Fluids 33 112
Google Scholar
[5] Mundo C, Sommerfeld M, Tropea C 1994 Int. J. Multiphase Flow 21 151
[6] Stanton D W, Rutland C J 1996 SAE Trans. 105 960628
[7] Xu H T, Liu Y C, He P, Wang H Q 1998 J. Fluids Eng. 120 593
Google Scholar
[8] Attané P, Girard F, Morin V 2007 Phys. Fluids 19 12101
Google Scholar
[9] 宋云超 2013 博士学位论文 (北京: 北京交通大学)
Song Y C 2013 Ph. D. Dissertation (Beijing: Beijing Jiaotong University) (in Chinese)
[10] 李大树 2015 博士学位论文 (北京: 中国石油大学)
Li D S 2015 Ph. D. Dissertation (Beijing: China University of Petroleum) (in Chinese)
[11] 陈石, 王辉, 沈胜强, 梁刚涛 2013 62 204702
Google Scholar
Chen S, Wang H, Shen S Q, Liang G T 2013 Acta Phys. Sin. 62 204702
Google Scholar
[12] 梁刚涛, 郭亚丽, 沈胜强 2013 62 024705
Google Scholar
Liang G T, Guo Y L, Shen S Q 2013 Acta Phys. Sin. 62 024705
Google Scholar
[13] 黄虎, 洪宁, 梁宏, 施保昌, 柴振华 2013 62 084702
Google Scholar
Huang H, Hong N, Liang H, Shi B C, Chai Z H 2013 Acta Phys. Sin. 62 084702
Google Scholar
[14] 武冠杰 2019 博士学位论文 (西安: 西北工业大学)
Wu G J 2019 Ph. D. Dissertation (Xi’an: Northwestern Polytechnical University) (in Chinese)
[15] Pasandideh-Fard M, Chandra S, Mostaghimi J 2002 Int. J. Heat Mass Transfer 45 2229
Google Scholar
[16] 尚超, 阳倦成, 张杰, 倪明玖 2019 力学学报 51 380
Google Scholar
Shang C, Yang Q C, Zhang J, Ni M J 2019 J. Theor. Appl. Mech. 51 380
Google Scholar
[17] Dou Y, Luo J, Qi L, Lian H, Huang J 2021 J. Mater. Process. Technol. 297 117268
Google Scholar
[18] Li C, Wu G, Li M, Hu C, Wei J 2020 Aerosp. Sci. Technol. 97 105639
Google Scholar
[19] Assael M J, Kakosimos K, Banish R M, Brillo J, Egry I, Brooks R, Quested P N, Mills K C, Nagashima A, Sato Y, Wakeham W A 2006 J. Phys. Chem. Ref. Data 35 285
Google Scholar
[20] Sarou-Kanian V, Millot F, Rifflet J C 2003 Int. J. Thermophys. 24 277
Google Scholar
[21] Wu G J, Ren Q B, Fu Y, Liu Y M, Hu C B 2018 J. Solid Rocket Technol. 41 677
[22] Brackbill J U, Kothe D B, Zemach C 1992 J. Comput. Phys. 100 335
Google Scholar
[23] Balla M, Kumar Tripathi M, Sahu K C 2019 Phys. Rev. E 99 23107
Google Scholar
[24] 唐鹏博, 王关晴, 王路, 石中玉, 李源, 徐江荣 2020 69 024702
Google Scholar
Tang P B, Wang G Q, Wang L, Shi Z Y, Li Y, Xu J R 2020 Acta Phys. Sin. 69 024702
Google Scholar
[25] Akao F, Araki K, Mori S, Moriyama A 1980 Trans. ISIJ 20 737
Google Scholar
[26] Hatta N, Fujimoto H, Takuda H, Kinoshita K, Takahashi O 1995 ISIJ Int. 35 50
Google Scholar
[27] Samkhaniani N, Stroh A, Holzinger M, Marschall H, Frohnapfel B, Wörner M 2021 Int. J. Heat Mass Transfer 180 121777
Google Scholar
[28] Chandra S, Avedisian C T 1991 Proc. R. Soc. London, Ser. A 432 13
Google Scholar
[29] Pasandideh-Fard M, Qiao Y M, Chandra S, Mostaghimi J 1996 Phys. Fluids 8 650
Google Scholar
-
图 1 物理模型
Figure 1. Schematic diagram of physical model.
图 2 网格无关性验证
Figure 2. Verification of grid independence.
图 4 铝液滴撞击曲面过程的压力与速度分布 (a) 压力云图; (b) 速度云图
Figure 4. Pressure and velocity distribution of aluminum droplet impinging on concave surface: (a) Pressure contours; (b) velocity contours.
图 5 固-液接触点的压力与速度变化规律
Figure 5. Time evolution of the pressure and velocity of solid-liquid contact point.
图 6 不同We下铝液滴在曲面上的铺展行为 (a) We = 0.7; (b) We = 2.7; (c) We = 10.8; (d) We = 43.3
Figure 6. Spreading behavior of droplets on the surface at different impact We: (a) We = 0.7; (b) We = 2.7; (c) We = 10.8; (d) We = 43.3
图 7 不同We下铝液滴铺展系数随时间的变化
Figure 7. Time evolution of spreading coefficient of aluminum droplets under different We.
图 8 铝液滴的铺展系数与中心高度系数随时间的变化规律 (a)铺展系数; (b)中心高度系数
Figure 8. Time evolution of the spreading and center height coefficient of aluminum droplets of different sizes: (a) Spreading coefficient; (b) center height coefficient.
图 9 铝液滴铺展系数和铺展速度随时间的变化规律 (a)铺展系数; (b)铺展速度
Figure 9. Time evolution of the spreading coefficient and spreading velocity of droplets of different sizes: (a) Spreading coefficient; (b) spreading velocity.
图 10 不同液滴温度下铺展系数随时间的变化规律
Figure 10. Time evolution of the spreading coefficient of droplets of different temperatures.
图 11 铝液滴最大铺展系数随温度的变化规律
Figure 11. Variation of the maximum spreading coefficient of aluminum droplets with temperature.
图 12 铝液滴撞击不同曲率壁面时铺展系数与中心高度系数的变化规律 (a) 铺展系数; (b)中心高度系数
Figure 12. Time evolution of spreading and center height coefficient of aluminum droplet on different curvature surface: (a) Spreading coefficient; (b) center height coefficient.
图 13 铝液滴撞击曲面时最大铺展系数随We的变化规律
Figure 13. Variation of the maximum spreading coefficient with Weber number when aluminum droplet impinges on surface.
图 14 修正的预测模型
Figure 14. Modified prediction formula.
图 15 铝液滴撞壁过程的形态变化 (a) 初始时刻; (b) 最大铺展时刻
Figure 15. Deformation process of a droplet colliding with the wall: (a) The initial moment; (b) maximum spreading moment.
表 1 主要物性参数
Table 1. Main physical properties.
参数 数值 单位 温度 1000 K 压力 101325 Pa 液滴密度 2357 kg/m3 液滴黏度 1.178×10–3 Pa/s 表面张力 0.871 N/m 接触角 161 (°) 
DownLoad: CSV
表 2 铝液滴物性参数随温度的变化
Table 2. Physical parameters properties of aluminum droplets at different temperatures.
温度/K 密度/
(kg·m–3)黏度/
(10–3 Pa·s–1)表面张力/
(N·m–1)1200 2294 0.865 0.834 1400 2232 0.694 0.797 1600 2170 0.589 0.760 1800 2108 0.518 0.723 2000 2046 0.467 0.686
DownLoad: CSV
表 3 铺展过程的特征参数
Table 3. Characteristic parameters of the spreading process.
k $ {\beta }_{\mathrm{m}\mathrm{a}\mathrm{x}} $ $ {h}_{\mathrm{m}\mathrm{i}\mathrm{n}} $ t1/ms t2/ms tmax/ms 0 1.394 0.192 0.6 0.9 2.3 167 1.411 0.242 0.6 0.8 2.2 250 1.418 0.257 0.6 0.8 2.2 400 1.433 0.281 0.6 0.8 2.3
DownLoad: CSV
表 4 预测模型的相对误差
Table 4. Relative error of prediction model
k ε Re We Relative error/% Hatta模型 Samkhaniani模型 Eq. (7) Eq.(32) 167 0.083 2401 3.54 3.45 33.91 1.27 24.54 167 0.083 3201 6.30 5.80 54.13 0.36 18.45 167 0.083 4001 9.84 3.83 74.51 1.18 18.42 167 0.083 4801 14.17 3.23 87.61 1.06 16.95 250 0.125 2401 3.54 9.29 29.48 2.83 20.53 250 0.125 3201 6.30 7.84 50.37 0.29 15.69 250 0.125 4001 9.84 5.54 68.87 1.49 14.75 250 0.125 4801 14.17 5.46 85.67 1.28 15.92 400 0.2 2401 3.54 10.35 24.34 1.59 16.06 400 0.2 3201 6.30 8.67 49.43 1.836 15.33 400 0.2 4001 9.84 7.73 67.43 1.60 14.19 400 0.2 4801 14.17 7.45 79.43 0.97 12.52
DownLoad: CSV
-
[1] Yi H, Qi L, Luo J, Zhang D, Li H, Hou X 2018 Int. J. Mach. Tools Manuf. 130–131 1
[2] 李涛 2020 博士学位论文 (济南: 山东大学)
Li T 2020 Ph. D. Dissertation (Jinan: Shandong University) (in Chinese)
[3] Rioboo R, Tropea C, Marengo M 2001 Atomization Sprays 11 155
Google Scholar
[4] Rioboo R, Marengo M, Tropea C 2002 Exp. Fluids 33 112
Google Scholar
[5] Mundo C, Sommerfeld M, Tropea C 1994 Int. J. Multiphase Flow 21 151
[6] Stanton D W, Rutland C J 1996 SAE Trans. 105 960628
[7] Xu H T, Liu Y C, He P, Wang H Q 1998 J. Fluids Eng. 120 593
Google Scholar
[8] Attané P, Girard F, Morin V 2007 Phys. Fluids 19 12101
Google Scholar
[9] 宋云超 2013 博士学位论文 (北京: 北京交通大学)
Song Y C 2013 Ph. D. Dissertation (Beijing: Beijing Jiaotong University) (in Chinese)
[10] 李大树 2015 博士学位论文 (北京: 中国石油大学)
Li D S 2015 Ph. D. Dissertation (Beijing: China University of Petroleum) (in Chinese)
[11] 陈石, 王辉, 沈胜强, 梁刚涛 2013 62 204702
Google Scholar
Chen S, Wang H, Shen S Q, Liang G T 2013 Acta Phys. Sin. 62 204702
Google Scholar
[12] 梁刚涛, 郭亚丽, 沈胜强 2013 62 024705
Google Scholar
Liang G T, Guo Y L, Shen S Q 2013 Acta Phys. Sin. 62 024705
Google Scholar
[13] 黄虎, 洪宁, 梁宏, 施保昌, 柴振华 2013 62 084702
Google Scholar
Huang H, Hong N, Liang H, Shi B C, Chai Z H 2013 Acta Phys. Sin. 62 084702
Google Scholar
[14] 武冠杰 2019 博士学位论文 (西安: 西北工业大学)
Wu G J 2019 Ph. D. Dissertation (Xi’an: Northwestern Polytechnical University) (in Chinese)
[15] Pasandideh-Fard M, Chandra S, Mostaghimi J 2002 Int. J. Heat Mass Transfer 45 2229
Google Scholar
[16] 尚超, 阳倦成, 张杰, 倪明玖 2019 力学学报 51 380
Google Scholar
Shang C, Yang Q C, Zhang J, Ni M J 2019 J. Theor. Appl. Mech. 51 380
Google Scholar
[17] Dou Y, Luo J, Qi L, Lian H, Huang J 2021 J. Mater. Process. Technol. 297 117268
Google Scholar
[18] Li C, Wu G, Li M, Hu C, Wei J 2020 Aerosp. Sci. Technol. 97 105639
Google Scholar
[19] Assael M J, Kakosimos K, Banish R M, Brillo J, Egry I, Brooks R, Quested P N, Mills K C, Nagashima A, Sato Y, Wakeham W A 2006 J. Phys. Chem. Ref. Data 35 285
Google Scholar
[20] Sarou-Kanian V, Millot F, Rifflet J C 2003 Int. J. Thermophys. 24 277
Google Scholar
[21] Wu G J, Ren Q B, Fu Y, Liu Y M, Hu C B 2018 J. Solid Rocket Technol. 41 677
[22] Brackbill J U, Kothe D B, Zemach C 1992 J. Comput. Phys. 100 335
Google Scholar
[23] Balla M, Kumar Tripathi M, Sahu K C 2019 Phys. Rev. E 99 23107
Google Scholar
[24] 唐鹏博, 王关晴, 王路, 石中玉, 李源, 徐江荣 2020 69 024702
Google Scholar
Tang P B, Wang G Q, Wang L, Shi Z Y, Li Y, Xu J R 2020 Acta Phys. Sin. 69 024702
Google Scholar
[25] Akao F, Araki K, Mori S, Moriyama A 1980 Trans. ISIJ 20 737
Google Scholar
[26] Hatta N, Fujimoto H, Takuda H, Kinoshita K, Takahashi O 1995 ISIJ Int. 35 50
Google Scholar
[27] Samkhaniani N, Stroh A, Holzinger M, Marschall H, Frohnapfel B, Wörner M 2021 Int. J. Heat Mass Transfer 180 121777
Google Scholar
[28] Chandra S, Avedisian C T 1991 Proc. R. Soc. London, Ser. A 432 13
Google Scholar
[29] Pasandideh-Fard M, Qiao Y M, Chandra S, Mostaghimi J 1996 Phys. Fluids 8 650
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4962
- PDF Downloads: 93
- Cited By: 0
