-
The effect of interorbital hopping on orbital selective Mottness in a two-band correlation system is investigated by using the dynamical mean-field theory with the Lanczos method as impurity solver. The phase diagrams of the two-orbital Hubbard model with non-local interorbital hopping (t12) , where the orbital selective Mott phases (OSMP) region is expanded by the increasing of the interorbital hopping. We compare the results obtained by self-consistent relations of Bethe lattice and squate lattice based on DMFT procedure, and the general OSMP physics of Bethe lattice is consistent with that of the square lattice while the critical points of two kinds of lattices are different. We extend the method to the study of half-filled Ba2CuO4–δ materials. By comparing with the band structure obtained from the density functional theory (DFT), it is found that the isotropic inter-orbital hopping has a great influence on the bandstructure. The DFT bandstructure in DMFT is considered, and the phase diagram of the material is obtained. The half-filled Ba2CuO4–δ should be orbital-selective Mott compound according to our results.
[1] Anisimov V I, Nekrasov I A, Kondakov D E, Rice T M, Sigrist M 2002 Eur. Phys. J. B 25 191
[2] Zhong Z, Hansmann P 2017 Phys. Rev. X 7 011023
[3] Huang J, Yu R, Xu Z, Zhu J X, Jiang Q, Wang M, Wu H, Chen T, Denlinger J D, Mo S K, Hashimoto M, Gu G, Dai P, Chu J H, Lu D, Si Q, Birgeneau R J, Yi M 2021 arXiv: 2010.13913
[4] Oleś A M 1983 Phys. Rev. B 28 327
Google Scholar
[5] Koga A, Imai Y, Kawakami N 2002 Phys. Rev. B 66 165107
Google Scholar
[6] Koga A, Kawakami N, Rice T M, Sigrist M 2005 Phys. Rev. B 72 045128
Google Scholar
[7] Kubo K 2007 Phys. Rev. B 75 224509
Google Scholar
[8] Georges A, Kotliar G, Krauth W, Rozenberg M J 1996 Rev. Mod. Phys. 68 13
Google Scholar
[9] Held K, Keller G, Eyert V, Vollhardt D, Anisimov V I 2001 Phys. Rev. Lett. 86 5345
Google Scholar
[10] Kotliar G, Savrasov S Y, Haule K, Oudovenko V S, Parcollet O, Marianetti C A 2006 Rev. Mod. Phys. 78 865
Google Scholar
[11] Zhao J Z, Zhuang J N, Deng X Y, Bi Y, Cai L C, Fang Z, Dai X 2012 Chin. Phys. B 21 057106
Google Scholar
[12] Liebsch A 2005 Phys. Rev. Lett. 95 116402
Google Scholar
[13] Peters R, Kawakami N, Pruschke T 2011 Phys. Rev. B 83 125110
Google Scholar
[14] Hirsch J E 1983 Phys. Rev. Lett. 51 1900
Google Scholar
[15] Hirsch J E 1985 Phys. Rev. B 31 4403
Google Scholar
[16] Chang C C, Zhang S 2008 Phys. Rev. B 78 165101
Google Scholar
[17] Tocchio L F, Becca F, Sorella S 2016 Phys. Rev. B 94 195126
Google Scholar
[18] Hanke W, Hirsch J E, 1982 Phys. Rev. B 25 6748
Google Scholar
[19] Shankar R 1994 Rev. Mod. Phys. 66 129
Google Scholar
[20] Hille C, Kugler F B, Eckhardt C J, He Y Y, Kauch A, Honerkamp C, Toschi A, Andergassen S 2020 Phys. Rev. Research 2 033372
Google Scholar
[21] Kotliar G, Ruckenstein A E 1986 Phys. Rev. Lett. 57 1362
Google Scholar
[22] Lechermann F, A Georges, Kotliar G, Parcollet O 2007 Phys. Rev. B 76 155102
Google Scholar
[23] Yu R, Si Q 2012 Phys. Rev. B 86 085104
Google Scholar
[24] Imada M, Fujimori A, Tokura Y 1998 Rev. Mod. Phys. 70 1039
Google Scholar
[25] Lee P A, Nagaosa N, Wen X G 2006 Rev. Mod. Phys. 78 17
Google Scholar
[26] Rohringer G, Hafermann H, Toschi A, Katanin A A, Antipov A E, Katsnelson M I, Lichtenstein A I, Rubtsov A N, Held K 2018 Rev. Mod. Phys. 90 025003
Google Scholar
[27] Song Y, Zou L J 2005 Phys. Rev. B 72 085114
Google Scholar
[28] Song Y, Zou L J 2009 Eur. Phys. J. B 72 59
Google Scholar
[29] Li W M, Zhao J F, Cao L P, Hu Z, Huang Q Z, Wang X C, Y. Liu, Zhao G Q, Zhang J, Liu Q Q, Yu R Z, Long Y W, Wu H, Lin H J, Chen C T, Li Z, Gong Z Z, Guguchia Z, Kim J S, Stewart G R, Uemura Y J, Uchida S, Jin C Q 2019 PNAS 116 12156
Google Scholar
[30] Liu K, Lu Z, Xiang T 2019 Phys. Rev. Mater. 3 044802
Google Scholar
[31] Li Y, Du S, Weng Z, Liu Z 2020 Phys. Rev. Mater. 4 044801
Google Scholar
[32] Wang Z, Zhou S, Chen W, Zhang F 2020 Phys. Rev. B 101 180509
Google Scholar
[33] Maier T, Berlijn T, Scalapino D J 2019 Phys. Rev. B 99 224515
Google Scholar
[34] Jiang K, Le C, Li Y, Qin S, Wang Z, Zhang F, Hu J 2021 Phys. Rev. B 103 045108
Google Scholar
[35] Jin H, Pickett W E, Lee K 2021 Phys. Rev. B 104 054516
Google Scholar
[36] Ni Y, Quan Y M, Liu J, Song Y, Zou L J 2021 Phys. Rev. B 103 214510
Google Scholar
[37] Niu Y, Sun J, Ni Y, Liu J, Song Y, Feng S 2019 Phys. Rev. B 100 075158
Google Scholar
[38] Dagotto E 1994 Rev. Mod. Phys. 66 763
Google Scholar
[39] Caffarel M, Krauth W 1994 Phys. Rev. Lett. 72 1545
Google Scholar
[40] Capone M, de’Medici L, Georges A 2007 Phys. Rev. B 76 245116
Google Scholar
[41] Perroni C A, Ishida H, Liebsch A 2007 Phys. Rev. B 75 045125
Google Scholar
[42] Georges A, de’Medici L, Mravlje J 2013 Annu. Rev. Condens. Matter Phys. 4 137
Google Scholar
[43] Koga A, Kawakami N, Rice T M, Sigrist M 2004 Phys. Rev. Lett. 92 216402
Google Scholar
[44] Costi T A, Liebsch A 2007 Phys. Rev. Lett. 99 236404
Google Scholar
[45] de'Medici L, Mravlje J, Georges A 2011 Phys. Rev. Lett. 107 256401
Google Scholar
[46] 孙健, 刘洋, 宋筠 2015 64 247101
Google Scholar
Sun J, Liu Y, Song Y 2015 Acta Phys. Sin. 64 247101
Google Scholar
[47] de’Medici L 2011 Phys. Rev. B 83 205112
Google Scholar
-
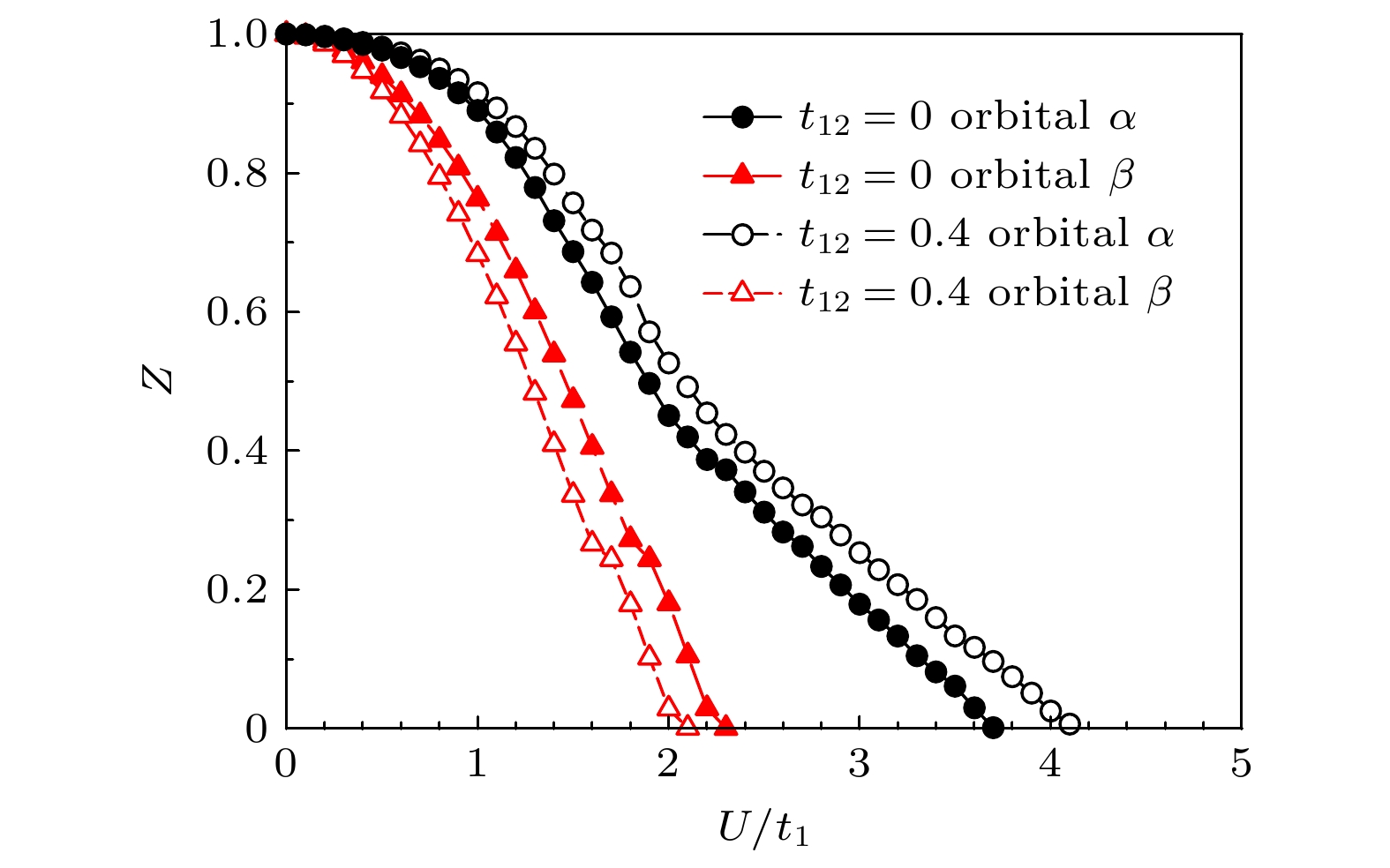
图 1 不同轨道间跃迁系数下两个有效轨道的准粒子权重
$Z$ 随相互作用U 的变化曲线. 轨道α 和轨道β分别用黑色圆圈和红色三角标识, 实心标志和空心标志分别代表$t_{12}=0$ 和$t_{12}=0.4$ 的情况. 体系中的洪特耦合作用$ J_{\rm{H}}=0.25 U$ , 两轨道带宽比$t_2/t_1=0.4$ , 所选用的能量单位为宽带跃迁系数$t_1$ Figure 1. The interaction dependencies of the quasiparticle weight Z of the effective orbital α (black circles) and β (red triangles) with interorbital hopping
$t_{12}=0.4$ (hollow symbols) and without interorbital hopping$t_{12}=0$ (solid symbols) when$J_{\rm{H}}=0.25 U$ and$t_2/t_1=0.4$ . The energy is in unit$t_1$ 
图 2 两个有效轨道的态密度随相互作用U 增加的演化图像, 体系中的轨道间跃迁
$t_{12}=0.4$ , 带宽比$t_2/t_1=0.4$ 以及相互作用强度$J_{\rm{H}}=0.25 U.$ 左边的图像为α轨道的结果, 右边图像为β轨道的结果, 计算中选取的能量展宽因子为$\eta=0.05$ Figure 2. Evolution of the orbital-resolved spectral density
$A(\omega)$ with the increasing Coulomb interaction U when$t_{12}=0.4$ ,$t_2/t_1=0.4$ and$J_{\rm{H}}=0.25 U.$ Left panels show the results of effective orbital α and right panels are for effective orbital β. The energy broadening factor in our calculation takes$\eta=0.05$ 
图 3 轨道间跃迁系数不同时, 相互作用U和带宽比
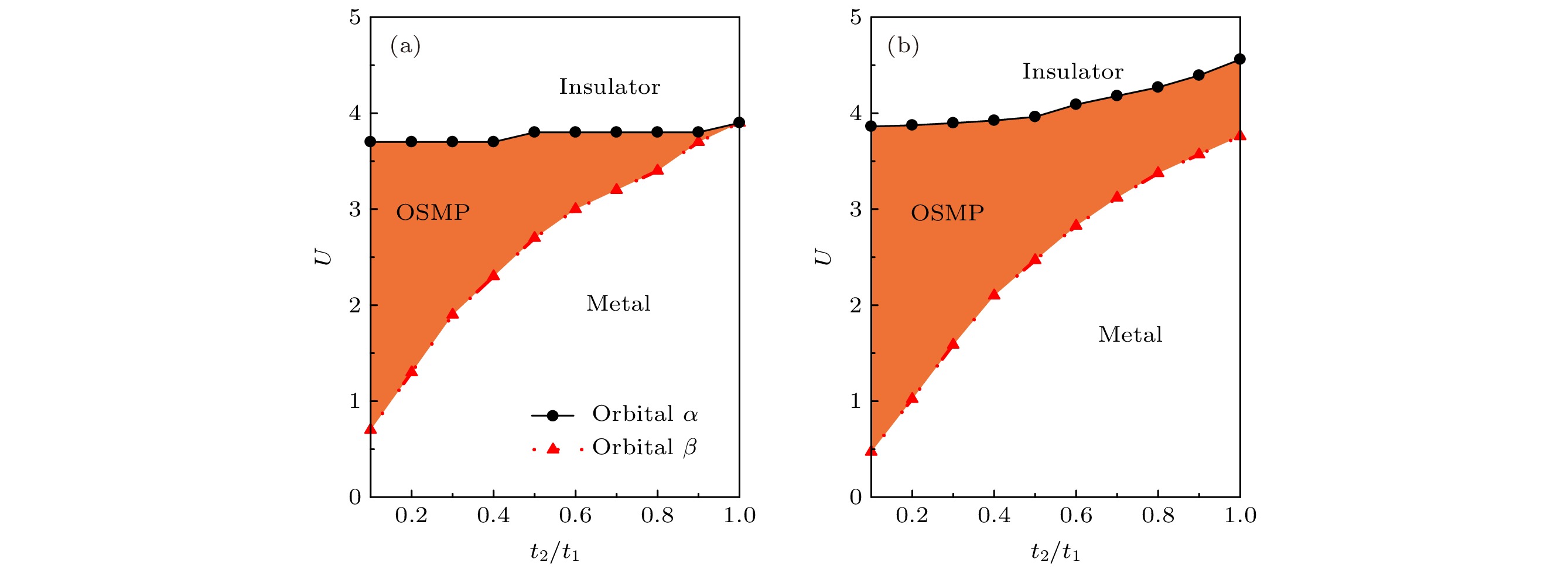
$t_2/t_1$ 影响下的双轨道体系相图 (a) 轨道间跃迁$t_{12}=0$ ; (b) 轨道间跃迁$t_{12}=0.4$ . 体系的洪特耦合$J_{\rm{H}}=0.25 U$ . 黑色圆圈和红色三角分别代表轨道α和β 的MIT 相变点Figure 3. Phase diagrams in the plane of interaction U and hopping integral
$t_2/t_1$ of the effective two-orbital Hubbard model with different interorbital hopping, when$J_{\rm{H}}=0.25 U$ : (a)$t_{12}=0$ ; (b)$t_{12}=0.4$ . The black circles (red triangles) denote the critical points of MIT for effective orbital α(β)
图 4 DMFT结合正方晶格能带结构所得到的结果 (a)轨道间跃迁系数不同时, 准粒子权重Z 随相互作用U 的变化曲线, 体系的洪特耦合
$J_{\rm{H}}$ = 0.25U, 带宽比$t_2$ /$t_1$ = 0.4; (b)$t_{12}=0.4$ 以及$J_{\rm{H}}=0.25 U$ 时, 相互作用U 和带宽比$t_2/t_1$ 影响下的有效轨道模型相图Figure 4. DMFT results of the square lattice: (a) The interaction dependencies of the quasiparticle weight Z with different interorbital hoppings when
$J_{\rm{H}}$ = 0.25U and$t_2$ /$t_1$ = 0.4; (b) phase diagrams in the plane of interaction U and hopping integral$t_2/t_1$ of the effective two-orbital Hubbard model when$t_{12}=0.4$ and$J_{\rm{H}}=0.25 U$ 
图 5 (a)
$J_{\rm{H}}=0.25 U$ 时有效轨道的准粒子权重随相互作用强度的变化曲线. 不同相互作用强度U下体系各有效轨道的态密度图像 (b)$U=1.0$ eV; (c)$U=2.2$ eV; (d)$U=2.5$ eV. 当相互作用2.1 eV$\leqslant U < $ 2.3 eV时体系处于OSMPFigure 5. (a) Quasiparticle weight Z as a function of interaction U when
$J_{\rm{H}}=0.25 U$ . The orbital-resolved spectral density$A(\omega)$ with different intraorbital interaction: (b)$U=1.0$ eV; (c)$U=2.2$ eV; (d)$U=2.5$ eV for the two effective orbitals. An OSMP occurs in a narrow interaction region with 2.1 eV$\leqslant U < $ 2.3 eV
图 6 相互作用U和洪特耦合
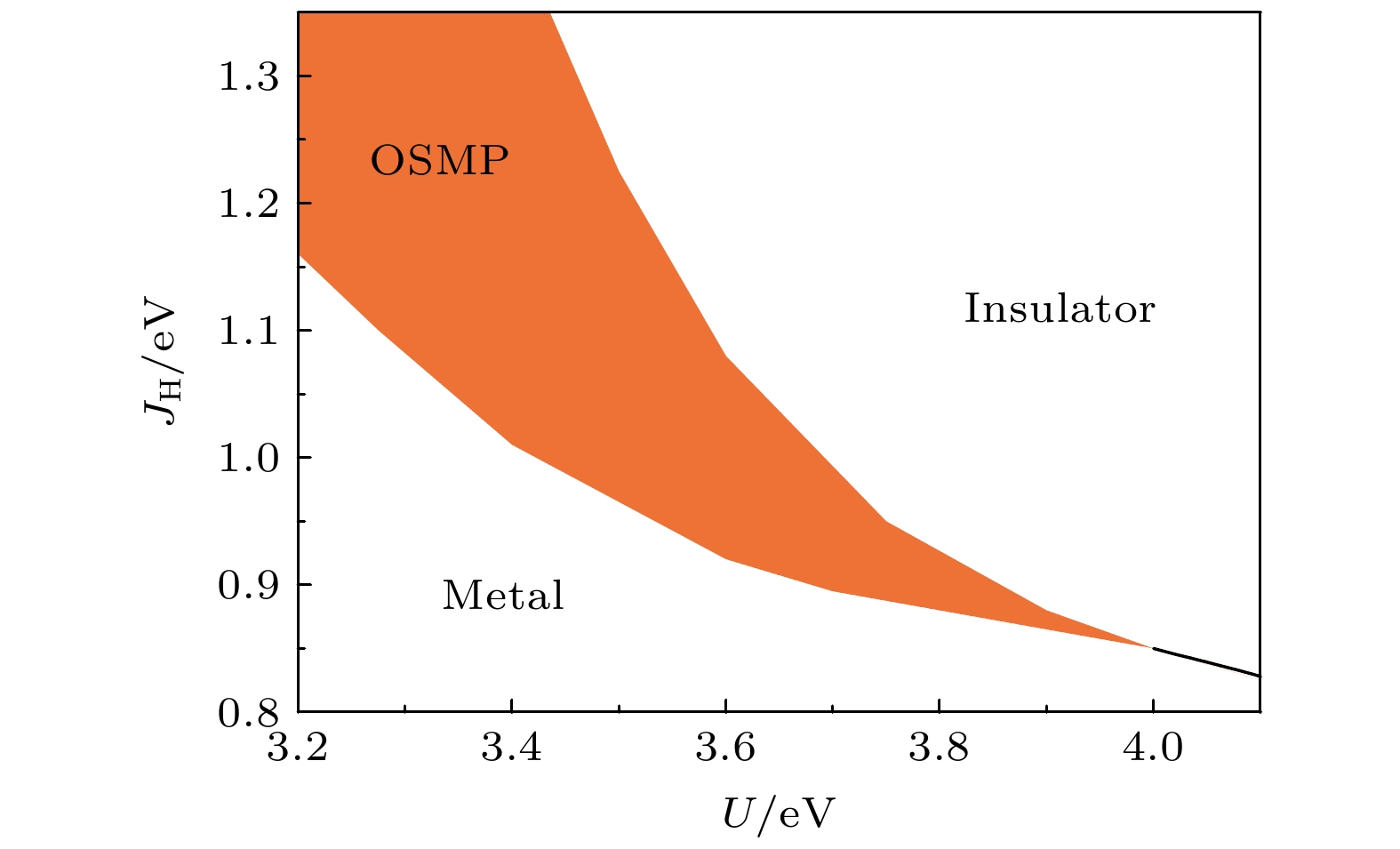
$J_{\rm{H}}$ 影响下体系的相图. 随着相互作用U的增加以及洪特耦合$J_{\rm{H}}$ 的减小, OSMP区域逐渐缩小, 大约在$J_{\rm{H}}=0.34$ eV以及$U=2.7$ eV时体系中的OSMT消失Figure 6. The phase diagram of the effective two-orbital Hubbard model with interaction U and Hund's rule coupling
$J_{\rm{H}}$ . The region of OSMP becomes narrower with the decreasing$J_{\rm{H}}$ and increasing U, and OSMT vanishes around$J_{\rm{H}}=0.34$ eV and$U=2.7$ eV
图 7 由不同模型得到的半满Ba2CuO
$_{4-\delta}$ 体系的能带图 (a)$t_{1}$ =0.504 eV,$t_{2}$ =0.196 eV,$t_{12}=-0.302$ eV,$\mu_1=-0.222$ eV 和$\mu_2=0.661$ eV的紧束缚模型所得到的能带图; (b) 根据DFT模拟的双带模型能带图像[33]Figure 7. Bandstructure for half-filling Ba2CuO3.5 with different model: (a) Tight binding model with
$t_{1}$ =0.504 eV,$t_{2}$ =0.196 eV,$t_{12}=-0.302$ eV,$\mu_1=-0.222$ eV and$\mu_2=0.661$ eV; (b) two-orbital model according to DFT results[33].
表 1 半满Ba2CuO4–δ紧束缚哈密顿量中的参数(单位为eV)[33]
Table 1. Model parameters of the TB Hamiltonian of Ba2CuO4–δ at half-filling (unit: eV)[33]
on-site energy (ε) 1st hopping (t) 2nd hopping ($t^\prime$) 3rd hopping ($t^{\prime\prime}$) Orbital ${\rm{d}}_{x^2-y^2}$ –0.222 0.504 –0.067 0.130 Orbital ${\rm{d}}_{3 z^2-r^2}$ 0.661 0.196 0.026 0.029 inter-orbital 0 –0.302 0 –0.051 
DownLoad: CSV
-
[1] Anisimov V I, Nekrasov I A, Kondakov D E, Rice T M, Sigrist M 2002 Eur. Phys. J. B 25 191
[2] Zhong Z, Hansmann P 2017 Phys. Rev. X 7 011023
[3] Huang J, Yu R, Xu Z, Zhu J X, Jiang Q, Wang M, Wu H, Chen T, Denlinger J D, Mo S K, Hashimoto M, Gu G, Dai P, Chu J H, Lu D, Si Q, Birgeneau R J, Yi M 2021 arXiv: 2010.13913
[4] Oleś A M 1983 Phys. Rev. B 28 327
Google Scholar
[5] Koga A, Imai Y, Kawakami N 2002 Phys. Rev. B 66 165107
Google Scholar
[6] Koga A, Kawakami N, Rice T M, Sigrist M 2005 Phys. Rev. B 72 045128
Google Scholar
[7] Kubo K 2007 Phys. Rev. B 75 224509
Google Scholar
[8] Georges A, Kotliar G, Krauth W, Rozenberg M J 1996 Rev. Mod. Phys. 68 13
Google Scholar
[9] Held K, Keller G, Eyert V, Vollhardt D, Anisimov V I 2001 Phys. Rev. Lett. 86 5345
Google Scholar
[10] Kotliar G, Savrasov S Y, Haule K, Oudovenko V S, Parcollet O, Marianetti C A 2006 Rev. Mod. Phys. 78 865
Google Scholar
[11] Zhao J Z, Zhuang J N, Deng X Y, Bi Y, Cai L C, Fang Z, Dai X 2012 Chin. Phys. B 21 057106
Google Scholar
[12] Liebsch A 2005 Phys. Rev. Lett. 95 116402
Google Scholar
[13] Peters R, Kawakami N, Pruschke T 2011 Phys. Rev. B 83 125110
Google Scholar
[14] Hirsch J E 1983 Phys. Rev. Lett. 51 1900
Google Scholar
[15] Hirsch J E 1985 Phys. Rev. B 31 4403
Google Scholar
[16] Chang C C, Zhang S 2008 Phys. Rev. B 78 165101
Google Scholar
[17] Tocchio L F, Becca F, Sorella S 2016 Phys. Rev. B 94 195126
Google Scholar
[18] Hanke W, Hirsch J E, 1982 Phys. Rev. B 25 6748
Google Scholar
[19] Shankar R 1994 Rev. Mod. Phys. 66 129
Google Scholar
[20] Hille C, Kugler F B, Eckhardt C J, He Y Y, Kauch A, Honerkamp C, Toschi A, Andergassen S 2020 Phys. Rev. Research 2 033372
Google Scholar
[21] Kotliar G, Ruckenstein A E 1986 Phys. Rev. Lett. 57 1362
Google Scholar
[22] Lechermann F, A Georges, Kotliar G, Parcollet O 2007 Phys. Rev. B 76 155102
Google Scholar
[23] Yu R, Si Q 2012 Phys. Rev. B 86 085104
Google Scholar
[24] Imada M, Fujimori A, Tokura Y 1998 Rev. Mod. Phys. 70 1039
Google Scholar
[25] Lee P A, Nagaosa N, Wen X G 2006 Rev. Mod. Phys. 78 17
Google Scholar
[26] Rohringer G, Hafermann H, Toschi A, Katanin A A, Antipov A E, Katsnelson M I, Lichtenstein A I, Rubtsov A N, Held K 2018 Rev. Mod. Phys. 90 025003
Google Scholar
[27] Song Y, Zou L J 2005 Phys. Rev. B 72 085114
Google Scholar
[28] Song Y, Zou L J 2009 Eur. Phys. J. B 72 59
Google Scholar
[29] Li W M, Zhao J F, Cao L P, Hu Z, Huang Q Z, Wang X C, Y. Liu, Zhao G Q, Zhang J, Liu Q Q, Yu R Z, Long Y W, Wu H, Lin H J, Chen C T, Li Z, Gong Z Z, Guguchia Z, Kim J S, Stewart G R, Uemura Y J, Uchida S, Jin C Q 2019 PNAS 116 12156
Google Scholar
[30] Liu K, Lu Z, Xiang T 2019 Phys. Rev. Mater. 3 044802
Google Scholar
[31] Li Y, Du S, Weng Z, Liu Z 2020 Phys. Rev. Mater. 4 044801
Google Scholar
[32] Wang Z, Zhou S, Chen W, Zhang F 2020 Phys. Rev. B 101 180509
Google Scholar
[33] Maier T, Berlijn T, Scalapino D J 2019 Phys. Rev. B 99 224515
Google Scholar
[34] Jiang K, Le C, Li Y, Qin S, Wang Z, Zhang F, Hu J 2021 Phys. Rev. B 103 045108
Google Scholar
[35] Jin H, Pickett W E, Lee K 2021 Phys. Rev. B 104 054516
Google Scholar
[36] Ni Y, Quan Y M, Liu J, Song Y, Zou L J 2021 Phys. Rev. B 103 214510
Google Scholar
[37] Niu Y, Sun J, Ni Y, Liu J, Song Y, Feng S 2019 Phys. Rev. B 100 075158
Google Scholar
[38] Dagotto E 1994 Rev. Mod. Phys. 66 763
Google Scholar
[39] Caffarel M, Krauth W 1994 Phys. Rev. Lett. 72 1545
Google Scholar
[40] Capone M, de’Medici L, Georges A 2007 Phys. Rev. B 76 245116
Google Scholar
[41] Perroni C A, Ishida H, Liebsch A 2007 Phys. Rev. B 75 045125
Google Scholar
[42] Georges A, de’Medici L, Mravlje J 2013 Annu. Rev. Condens. Matter Phys. 4 137
Google Scholar
[43] Koga A, Kawakami N, Rice T M, Sigrist M 2004 Phys. Rev. Lett. 92 216402
Google Scholar
[44] Costi T A, Liebsch A 2007 Phys. Rev. Lett. 99 236404
Google Scholar
[45] de'Medici L, Mravlje J, Georges A 2011 Phys. Rev. Lett. 107 256401
Google Scholar
[46] 孙健, 刘洋, 宋筠 2015 64 247101
Google Scholar
Sun J, Liu Y, Song Y 2015 Acta Phys. Sin. 64 247101
Google Scholar
[47] de’Medici L 2011 Phys. Rev. B 83 205112
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 5794
- PDF Downloads: 219
- Cited By: 0
