Dependence of spallstrength on temperature, grain size and strain rate in pure ductile metals

Zhang Feng-Guo; Zhao Fu-Qi; Liu Jun; He An-Min; Wang Pei

doi:10.7498/aps.71.20210702

Abstract

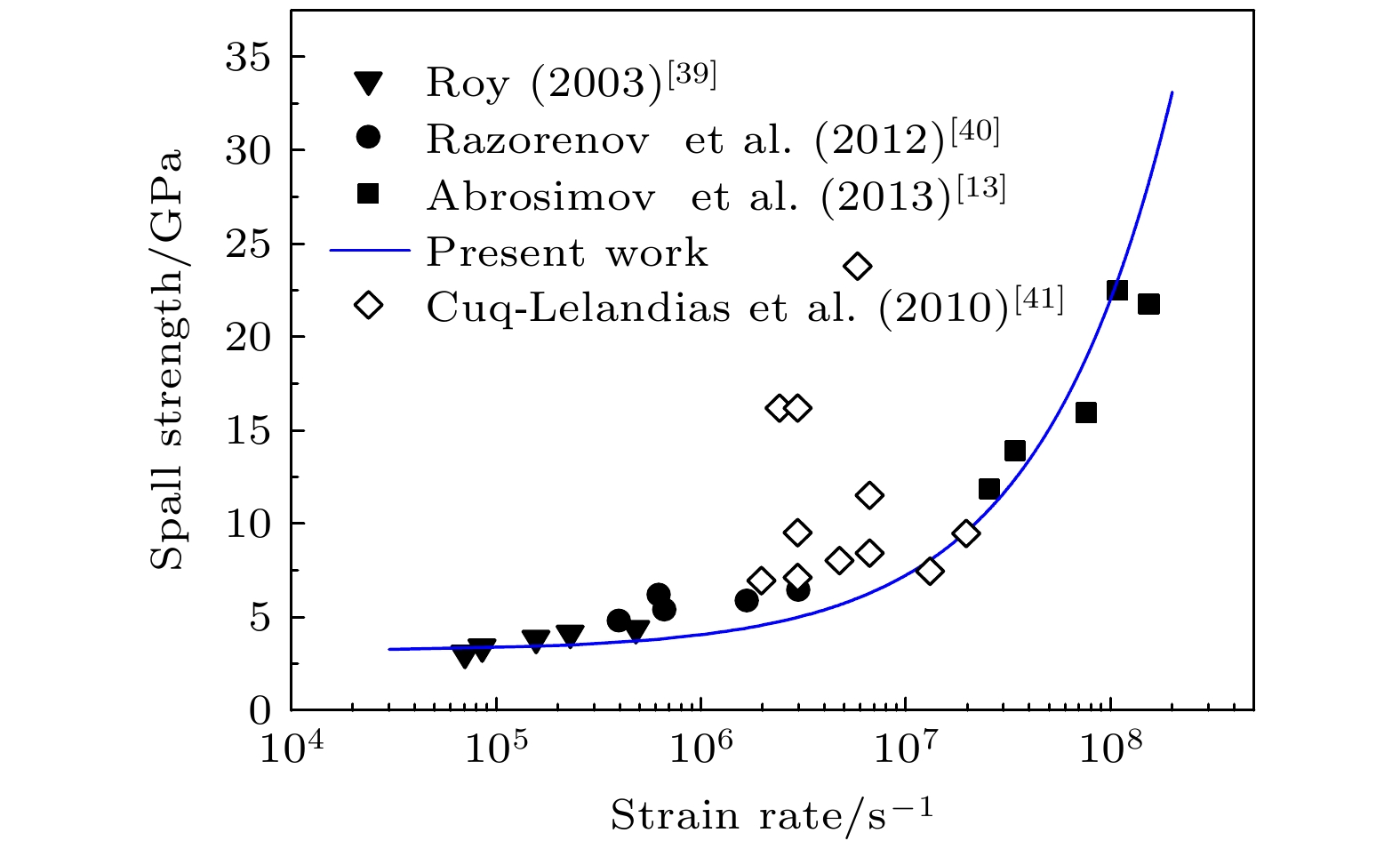
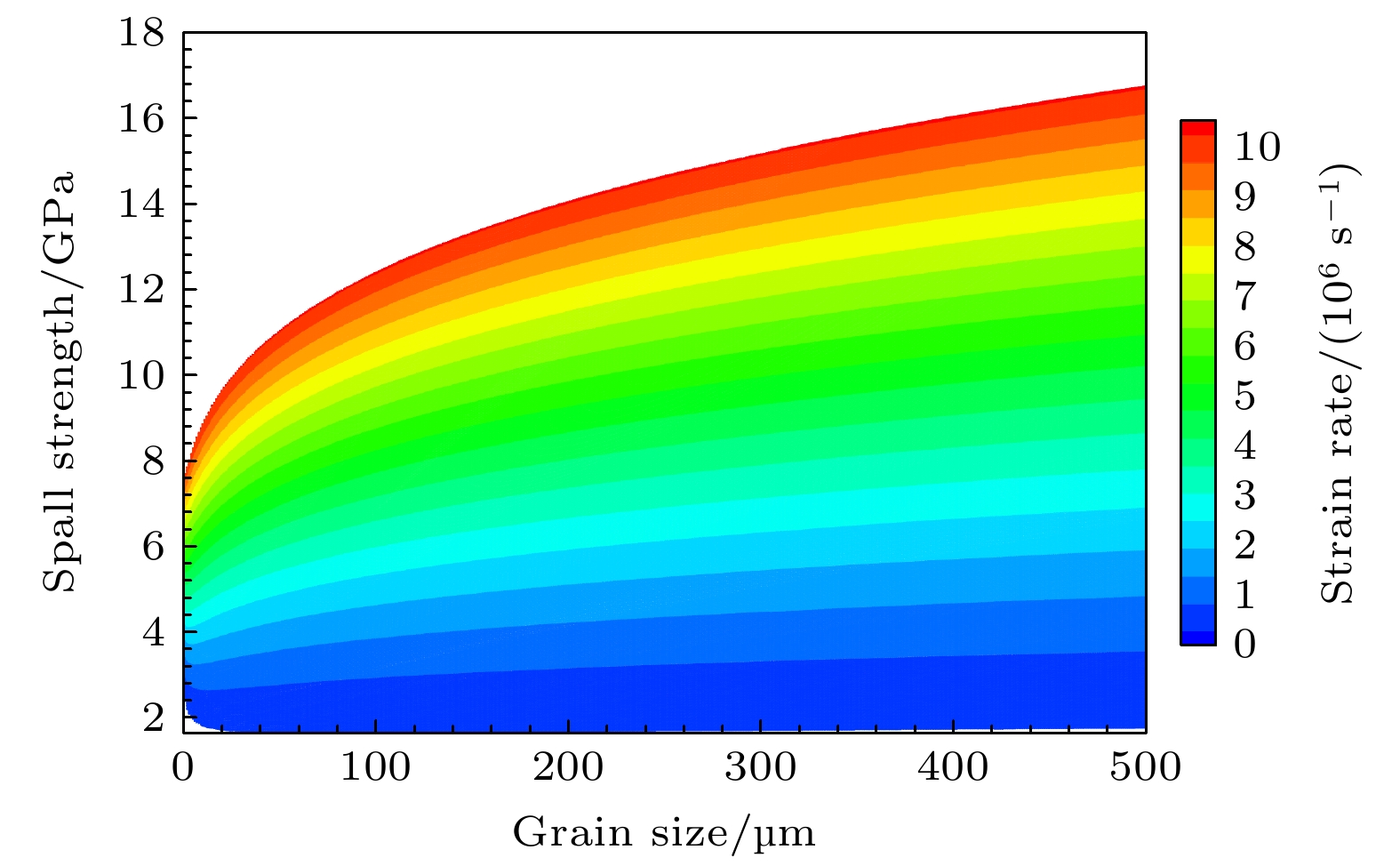
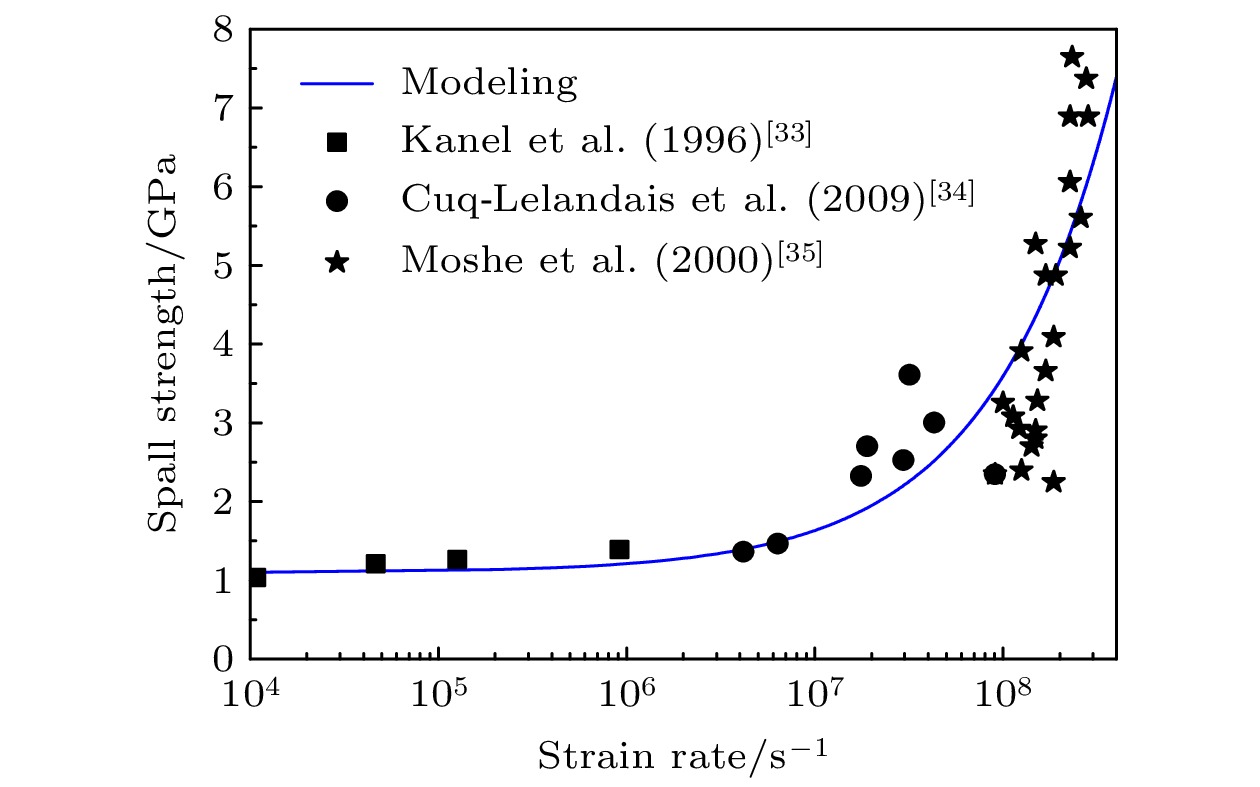
When a shockwave, which can be generated by high velocity impact or explosive detonation, reflects from the free surface of a metal, it usually creates tensile stress inside the metal. While the tensile stress is large enough, voids nucleation, growth and coalescence happen inside the metal, causing the metal to spall. As one of the main contents of the spallation damage research, the spallation strength, which is often characterized by features of the free surface velocity history measured in spallation experiments, represents the maximum tensile stress that the material can withstand, and is actually a complex interaction among several competing mechanisms. Optimizing the spallation strengths of metals is important for their applications in the aerospace, automotive, and defense industries, and can be achieved by using the advanced manufacturing strategies, if we can know better the meaning and present analytic model of the spallation strength of metal. A large number of experiments show that the spallation strength of ductile metal is strongly dependent on the tensile strain rate, grain size and temperature of material. Based on the analysis of early spallation evolution and influence of grain size and temperature on the material, a simple analytic model of spallation strength is presented in this paper, which takes into account the effects of strain rate, grain size and temperature in materials. The applicability of this model is verified by comparing the calculated results from the model with the experimental results of spall strength of typical ductile metals such as high purity aluminum, copper, and tantalum.

Keywords:

Authors and contacts

Institute of Applied Physics and Computational Mathematics, Beijing 100088, China

Corresponding author: Zhang Feng-Guo, zhang_fengguo@iapcm.ac.cn

Funds: Project supported by the Science Challenge Project, China (Grant No. TZ2018001)

FullText HTML

References

[1]	Novikov S A 1967 J. App. Mech. Tech. Phys. 3 109
[2]	Stepanov G V 1976 Problemy Prochnosti 8 66
[3]	Romanchenko V I, Sepanov G V 1980 J. App. Mech. Tech. Phys. 21 141
[4]	Kanel G I 2010 Int. J. Frac. 163 173 Google Scholar
[5]	Turley W D, Fensin S J, Hixson R S, Jones D R, La Lone B M, Stevens G D, Thomas S A, Veeser L R 2018 J. App. Phys. 123 55102 Google Scholar
[6]	Mallick D D, Zhao M, Parker J, Kannan V, Bosworth B T, Sagapuram D, Foster M A, Ramesh K T 2019 Exp. Mech. 59 1 Google Scholar
[7]	Zurek A, Thissell W, Johnson J N, Tonks D, Hixson R. 1996 J. Mater. Process. Technol. 60 261 Google Scholar
[8]	谢普初, 汪小松, 胡昌明, 胡建波, 张凤国, 王永刚 2020 69 034601 Google Scholar Xie P C, Wang X S, Hu C M, Hu J B, Zhang F G, Wang Y G 2020 Acta Phys. Sin. 69 034601 Google Scholar
[9]	Eftis J, Nemes J A, Randles P 1991 Int. J. Plast. 7 15 Google Scholar
[10]	Tonks D L, Thissell W R, Schwartz D S 2003 Shock Compression of Condensed Matter (New York: Melville) p507
[11]	Kanel G, Razorenov S, Bogatch A, Utkin A, Grady D 1997 Int. J. Impact Eng. 20 467 Google Scholar
[12]	Antoun T, Seaman L, Curran D R, Kanel G I, Razorenov S V, Utkin A V 2003 Spall Fracture (New York: Springer-Verlag) p130
[13]	Abrosimov S A, Bazhulin A P, Voronov V V, Geras’kin A A, Krasyuk I K, Pashinin P P, Semenov A Yu, Stuchebryukhov I A, Khishchenko K V, Fortov V E 2013 Quantum Electron. 43 246 Google Scholar
[14]	Remington T P, Hahn E N, Zhao S, Flanagan R, Mertens J C E, Sabbaghianrad S, Langdon T G, Wehrenberg C E, Maddox B R, Swift D C, Remington B A, Chawla N, Meyers M A 2018 Acta Mater. 158 313 Google Scholar
[15]	Zaretsky E B, Kanel G I 2013 J. Appl. Phys. 114 083511
[16]	Garkushin G V, Kanel G I, Savinykh A S, Razorenov S V 2016 Int. J. Fract. 197 1 Google Scholar
[17]	Zaretsky E B, Kanel G I 2012 J. Appl. Phys. 112 073504 Google Scholar
[18]	Bogach A A, Kanel G I, Razorenov S V, Utkin A V, Protasova S G, Sursaeva V G 1998 Phys. Solid State 40 1676 Google Scholar
[19]	Trivedi P B, Asay J R, Gupta Y M, Field D P 2007 J. Appl. Phys. 102 083513 Google Scholar
[20]	Pedrazas N A, Worthington D L, Dalton D A, Sherek P A, Steucka S P, Quevedo H J, Bernstein A C, Taleff E M, Ditmire T 2012 Mater. Sci. Eng., A 536 117 Google Scholar
[21]	Chen X, Asay J R, Dwivedi S K, Field D P 2006 J. Appl. Phys. 99 023528 Google Scholar
[22]	Escobedo J P, Dennis-Koller D, Cerreta E K, Patterson B M, Bronkhorst C A, Hansen B L, Tonks D L, Lebensohn R A 2011 J. Appl. Phys. 110 033513 Google Scholar
[23]	Chen T, Jiang Z X, Peng H, He H L, Wang L L, Wang Y G 2015 Strain 51 190 Google Scholar
[24]	Wilkerson J W, Ramesh K T 2016 Phys. Rev. Lett. 117 215503 Google Scholar
[25]	Nguyen T, Luscher D J, Wilkerson J W 2020 J. Mech. Phys. Solids 137 103875 Google Scholar
[26]	Seaman L, Curran D R, Shockey D A 1976 J. App. Phys. 47 4814 Google Scholar
[27]	Johnson J N 1981 J. App. Phys. 52 2812 Google Scholar
[28]	Czarnota C, Jacques N, Mercier S, Molinari A 2008 J. Mech. Phys. Solids 56 1624 Google Scholar
[29]	Wilkerson J W 2017 Int. J. Plast. 95 21 Google Scholar
[30]	Wright T W, Ramesh K T 2008 J. Mech. Phys. Solids 56 336 Google Scholar
[31]	张凤国, 王裴, 王昆, 周洪强, 赵福祺 2020 防护工程 42 33 Google Scholar Zhang F G, Wang P, Wang K, Zhou H Q, Zhao F Q 2020 Protective Engineering 42 33 Google Scholar
[32]	Wu X Y, Ramesh K T, Wright T W 2003 J. Mech. Phys. Solids 51 1 Google Scholar
[33]	Kanel G I, Razorenov S V, Bogatch A, Utkin A V, Fortov V E, Grady D E 1996 J. App. Phys. 79 8310 Google Scholar
[34]	Cuq-Lelandais J P, Boustie M, Berthe L, De Rességuier T, Combis P, Colombier J P, Nivard M, Claverie A 2009 J. Phys. D: Appl. Phys. 42 065402 Google Scholar
[35]	Moshe E, Eliezer S, Henis Z, Werdiger M, Dekel E, Horovitz Y, Maman S 2000 App. Phys. Lett. 76 1555 Google Scholar
[36]	Moshe E, Eliezer S, Deke E, Schwart A J 1998 J. App. Phys. 83 4004 Google Scholar
[37]	Kanel G I, Fortov V E, Razorenov S V 2007 Phys. Usp. 50 771 Google Scholar
[38]	Bachmann H, Baumung K, Kanel G I, Karov H U, Licht V, Rusch D, Singer J, Stoltz O 1993 Proc. 9th Int. Conf. High Power Particle Beams (Vol. 2) (Springfield, VA: NTIS) p963
[39]	Roy G 2003 Ph. D. Dissertation (Poitiers: University of Poitiers)
[40]	Razorenov S V, Kanel G I, Garkushin G V, Ignatova O N 2012 Phys. Solid State 54 790 Google Scholar
[41]	Cuq-Lelandais J P, Boustie M, Soulard L, Berthe L, De Rességuier T, Combis P, Bontaz-Carion J, Lescoute E 2010 EPJ Web Conferences 10 00014 Google Scholar
[42]	张凤国, 周洪强 2013 62 164601 Google Scholar Zhang F G, Zhou H Q 2013 Acta Phys. Sin. 62 164601 Google Scholar
[43]	Hall E O 1951 Proc. Phys. Soc. London, Ser. B 64 747 Google Scholar
[44]	Petch N J 1953 J. Iron Steel Inst. 174 25
[45]	Zerilli F J, Armstrong R W 1990 J. App. Phys. 68 1580 Google Scholar
[46]	Steinberg D J, Cochran S G, Guinan M W 1980 J. Appl. Phys. 51 1498 Google Scholar
[47]	李茂生, 陈栋泉 2001 高压 15 24 Google Scholar Li M S, Chen D Q 2001 Chin. J. High Pressure Phys. 15 24 Google Scholar

Cited By

图 1 金属铝材料层裂强度与加载拉伸应变率的关系

Figure 1. Spall strength in both experimental and calculated results of commercial pure aluminum.

DownLoad: Full-Size Img PowerPoint

图 2 高纯金属铜层裂强度与加载拉伸应变率的关系

Figure 2. Spall strength in both experimental and calculated results of pure copper.

DownLoad: Full-Size Img PowerPoint

图 3 金属钽材料层裂强度与加载拉伸应变率的关系

Figure 3. Spall strength in both experimental and calculated results of pure tantalum.

DownLoad: Full-Size Img PowerPoint

图 4 晶粒尺寸对纯钽金属层裂强度的影响

Figure 4. Experimental data and numerical results show there is a correlation between spall strength and grain size for pure tantalum.

DownLoad: Full-Size Img PowerPoint

图 5 晶粒尺寸、应变率对纯钽金属层裂强度的影响

Figure 5. Spall strength vs. grain size and tensile strain rate for pure tantalum.

DownLoad: Full-Size Img PowerPoint

图 6 初始温度对纯铝材料层裂强度的影响

Figure 6. Temperature dependence of the spall strength for pure aluminum.

DownLoad: Full-Size Img PowerPoint

图 7 初始温度、应变率对层裂强度的影响

Figure 7. Spall strength vs. temperature and tensile strain rate for pure aluminum.

DownLoad: Full-Size Img PowerPoint

表 1 材料参数以及层裂强度模型参数

Table 1. Material parameters and parameters of spall strength model.

Material	密度$ {\rho }_{0} $/(kg·m^–3)	屈服强度$ {Y}_{0} $/GPa	剪切模量$ G $/GPa	体积声速$ {C}_{0}$/(m·s^–1)	模型参数$ {N}_{0} $/m^–3
Aluminum	2760	0.26	26.5	5240	3.18 × 10¹⁷
Copper	8924	0.15	48.4	3910	7.67 × 10¹⁵
Tantalum	16690	0.77	69.0	3410	1.01 × 10¹⁶

DownLoad: CSV

map

[1]	Novikov S A 1967 J. App. Mech. Tech. Phys. 3 109
[2]	Stepanov G V 1976 Problemy Prochnosti 8 66
[3]	Romanchenko V I, Sepanov G V 1980 J. App. Mech. Tech. Phys. 21 141
[4]	Kanel G I 2010 Int. J. Frac. 163 173 Google Scholar
[5]	Turley W D, Fensin S J, Hixson R S, Jones D R, La Lone B M, Stevens G D, Thomas S A, Veeser L R 2018 J. App. Phys. 123 55102 Google Scholar
[6]	Mallick D D, Zhao M, Parker J, Kannan V, Bosworth B T, Sagapuram D, Foster M A, Ramesh K T 2019 Exp. Mech. 59 1 Google Scholar
[7]	Zurek A, Thissell W, Johnson J N, Tonks D, Hixson R. 1996 J. Mater. Process. Technol. 60 261 Google Scholar
[8]	谢普初, 汪小松, 胡昌明, 胡建波, 张凤国, 王永刚 2020 69 034601 Google Scholar Xie P C, Wang X S, Hu C M, Hu J B, Zhang F G, Wang Y G 2020 Acta Phys. Sin. 69 034601 Google Scholar
[9]	Eftis J, Nemes J A, Randles P 1991 Int. J. Plast. 7 15 Google Scholar
[10]	Tonks D L, Thissell W R, Schwartz D S 2003 Shock Compression of Condensed Matter (New York: Melville) p507
[11]	Kanel G, Razorenov S, Bogatch A, Utkin A, Grady D 1997 Int. J. Impact Eng. 20 467 Google Scholar
[12]	Antoun T, Seaman L, Curran D R, Kanel G I, Razorenov S V, Utkin A V 2003 Spall Fracture (New York: Springer-Verlag) p130
[13]	Abrosimov S A, Bazhulin A P, Voronov V V, Geras’kin A A, Krasyuk I K, Pashinin P P, Semenov A Yu, Stuchebryukhov I A, Khishchenko K V, Fortov V E 2013 Quantum Electron. 43 246 Google Scholar
[14]	Remington T P, Hahn E N, Zhao S, Flanagan R, Mertens J C E, Sabbaghianrad S, Langdon T G, Wehrenberg C E, Maddox B R, Swift D C, Remington B A, Chawla N, Meyers M A 2018 Acta Mater. 158 313 Google Scholar
[15]	Zaretsky E B, Kanel G I 2013 J. Appl. Phys. 114 083511
[16]	Garkushin G V, Kanel G I, Savinykh A S, Razorenov S V 2016 Int. J. Fract. 197 1 Google Scholar
[17]	Zaretsky E B, Kanel G I 2012 J. Appl. Phys. 112 073504 Google Scholar
[18]	Bogach A A, Kanel G I, Razorenov S V, Utkin A V, Protasova S G, Sursaeva V G 1998 Phys. Solid State 40 1676 Google Scholar
[19]	Trivedi P B, Asay J R, Gupta Y M, Field D P 2007 J. Appl. Phys. 102 083513 Google Scholar
[20]	Pedrazas N A, Worthington D L, Dalton D A, Sherek P A, Steucka S P, Quevedo H J, Bernstein A C, Taleff E M, Ditmire T 2012 Mater. Sci. Eng., A 536 117 Google Scholar
[21]	Chen X, Asay J R, Dwivedi S K, Field D P 2006 J. Appl. Phys. 99 023528 Google Scholar
[22]	Escobedo J P, Dennis-Koller D, Cerreta E K, Patterson B M, Bronkhorst C A, Hansen B L, Tonks D L, Lebensohn R A 2011 J. Appl. Phys. 110 033513 Google Scholar
[23]	Chen T, Jiang Z X, Peng H, He H L, Wang L L, Wang Y G 2015 Strain 51 190 Google Scholar
[24]	Wilkerson J W, Ramesh K T 2016 Phys. Rev. Lett. 117 215503 Google Scholar
[25]	Nguyen T, Luscher D J, Wilkerson J W 2020 J. Mech. Phys. Solids 137 103875 Google Scholar
[26]	Seaman L, Curran D R, Shockey D A 1976 J. App. Phys. 47 4814 Google Scholar
[27]	Johnson J N 1981 J. App. Phys. 52 2812 Google Scholar
[28]	Czarnota C, Jacques N, Mercier S, Molinari A 2008 J. Mech. Phys. Solids 56 1624 Google Scholar
[29]	Wilkerson J W 2017 Int. J. Plast. 95 21 Google Scholar
[30]	Wright T W, Ramesh K T 2008 J. Mech. Phys. Solids 56 336 Google Scholar
[31]	张凤国, 王裴, 王昆, 周洪强, 赵福祺 2020 防护工程 42 33 Google Scholar Zhang F G, Wang P, Wang K, Zhou H Q, Zhao F Q 2020 Protective Engineering 42 33 Google Scholar
[32]	Wu X Y, Ramesh K T, Wright T W 2003 J. Mech. Phys. Solids 51 1 Google Scholar
[33]	Kanel G I, Razorenov S V, Bogatch A, Utkin A V, Fortov V E, Grady D E 1996 J. App. Phys. 79 8310 Google Scholar
[34]	Cuq-Lelandais J P, Boustie M, Berthe L, De Rességuier T, Combis P, Colombier J P, Nivard M, Claverie A 2009 J. Phys. D: Appl. Phys. 42 065402 Google Scholar
[35]	Moshe E, Eliezer S, Henis Z, Werdiger M, Dekel E, Horovitz Y, Maman S 2000 App. Phys. Lett. 76 1555 Google Scholar
[36]	Moshe E, Eliezer S, Deke E, Schwart A J 1998 J. App. Phys. 83 4004 Google Scholar
[37]	Kanel G I, Fortov V E, Razorenov S V 2007 Phys. Usp. 50 771 Google Scholar
[38]	Bachmann H, Baumung K, Kanel G I, Karov H U, Licht V, Rusch D, Singer J, Stoltz O 1993 Proc. 9th Int. Conf. High Power Particle Beams (Vol. 2) (Springfield, VA: NTIS) p963
[39]	Roy G 2003 Ph. D. Dissertation (Poitiers: University of Poitiers)
[40]	Razorenov S V, Kanel G I, Garkushin G V, Ignatova O N 2012 Phys. Solid State 54 790 Google Scholar
[41]	Cuq-Lelandais J P, Boustie M, Soulard L, Berthe L, De Rességuier T, Combis P, Bontaz-Carion J, Lescoute E 2010 EPJ Web Conferences 10 00014 Google Scholar
[42]	张凤国, 周洪强 2013 62 164601 Google Scholar Zhang F G, Zhou H Q 2013 Acta Phys. Sin. 62 164601 Google Scholar
[43]	Hall E O 1951 Proc. Phys. Soc. London, Ser. B 64 747 Google Scholar
[44]	Petch N J 1953 J. Iron Steel Inst. 174 25
[45]	Zerilli F J, Armstrong R W 1990 J. App. Phys. 68 1580 Google Scholar
[46]	Steinberg D J, Cochran S G, Guinan M W 1980 J. Appl. Phys. 51 1498 Google Scholar
[47]	李茂生, 陈栋泉 2001 高压 15 24 Google Scholar Li M S, Chen D Q 2001 Chin. J. High Pressure Phys. 15 24 Google Scholar

[1]	Liu Yu, Tian Qiang, Wang Xin-Yan, Guan Xue-Fei. Efficient grain size evaluation based on single direction measurement of ultrasonic backscattering coefficient. Acta Physica Sinica, 2024, 73(7): 074301. doi: 10.7498/aps.73.20231959
[2]	. Spall strength dependence on temperature, grain size and strain rate in pure ductile metals. Acta Physica Sinica, 2021, (): . doi: 10.7498/aps.70.20210702
[3]	Li Shan, Li Xiong-Bing, Song Yong-Feng, Chen Chao. Ultrasonic sacttering unified theory for polycrystal material with grain sizes distribution. Acta Physica Sinica, 2018, 67(23): 234301. doi: 10.7498/aps.67.20181751
[4]	Wang Peng, Xu Jian-Gang, Zhang Yun-Guang, Song Hai-Yang. Molecular dynamics simulation of effect of grain on mechanical properties of nano-polycrystal -Fe. Acta Physica Sinica, 2016, 65(23): 236201. doi: 10.7498/aps.65.236201
[5]	Liu Ying-Guang, Zhang Shi-Bing, Han Zhong-He, Zhao Yu-Jin. Influence of grain size on the thermal conduction of nanocrystalline copper. Acta Physica Sinica, 2016, 65(10): 104401. doi: 10.7498/aps.65.104401
[6]	Deng Chun-Yu, Hou Shang-Lin, Lei Jing-Li, Wang Dao-Bin, Li Xiao-Xiao. Simultaneous measurement on strain and temperature via guided acoustic-wave Brillouin scattering in single mode fibers. Acta Physica Sinica, 2016, 65(24): 240702. doi: 10.7498/aps.65.240702
[7]	Liu Jun-Chi, Li Hong-Wen, Wang Jian-Li, Liu Xin-Yue, Ma Xin-Xue. A temperature and emissivity separation algorithm based on maximum entropy estimation of alpha spectrum's scaling and translation. Acta Physica Sinica, 2015, 64(17): 175205. doi: 10.7498/aps.64.175205
[8]	Cao Ye, Pei Yong-Wei, Tong Zheng-Rong. Simultaneous measurement of temperature and bending-curvature using a single local micro-structured longperiod fiber grating. Acta Physica Sinica, 2014, 63(2): 024206. doi: 10.7498/aps.63.024206
[9]	Sun Pei, Li Jian-Jun, Deng Jun, Han Jun, Ma Ling-Yun, Liu Tao. Temperature window of the (Al0.1Ga0.9)0.5In0.5P growth by MOCVD. Acta Physica Sinica, 2013, 62(2): 026801. doi: 10.7498/aps.62.026801
[10]	Jiang Zhong-Ying, Zhang Guo-Liang, Ma Jing, Zhu Tao. Lipid exhange between membranes: effects of temperature and ionic strength. Acta Physica Sinica, 2013, 62(1): 018701. doi: 10.7498/aps.62.018701
[11]	Sun Zhan-Feng, He Hong-Liang, Li Ping, Li Qing-Zhong. The spall strength and shock compressive damage of AD95 ceramics. Acta Physica Sinica, 2012, 61(9): 096201. doi: 10.7498/aps.61.096201
[12]	Wang Ying-Long, Zhang Peng-Cheng, Liu Hong-Rang, Liu Bao-Ting, Fu Guang-Sheng. Effects of grain size and substrate stress of ferroelectric film on the physical properties. Acta Physica Sinica, 2011, 60(7): 077702. doi: 10.7498/aps.60.077702
[13]	Mao Chao-Liang, Dong Xian-Lin, Wang Gen-Shui, Yao Chun-Hua, Cao Fei, Cao Sheng, Yang Li-Hui, Wang Yong-Ling. Grain size dependence of the dielectric properties of Ba0.70Sr0.30TiO3 ceramics and its mechanisms. Acta Physica Sinica, 2009, 58(8): 5784-5789. doi: 10.7498/aps.58.5784
[14]	Wang Hao, Liu Guo-Quan, Luan Jun-Hua, Yue Jing-Chao, Qin Xiang-Ge. Study on the relation among grain edge length, grain size and topology with Monte Carlo simulation. Acta Physica Sinica, 2009, 58(13): 132-S136. doi: 10.7498/aps.58.132
[15]	Wang Yong-Gang, Chen Deng-Ping, He Hong-Liang, Wang Li-Li, Jing Fu-Qian. Temperature dependence of dynamic yield strength and spall strength for LY12 aluminum alloy under shock loading. Acta Physica Sinica, 2006, 55(8): 4202-4207. doi: 10.7498/aps.55.4202
[16]	Yu Bo-Lin, Qi Qiong, Tang Xin-Feng, Zhang Qing-Jie. Effect of grain size on thermoelectric properties of CoSb3 compound. Acta Physica Sinica, 2005, 54(12): 5763-5768. doi: 10.7498/aps.54.5763
[17]	Peng Jian-Xiang, Jing Fu-Qian, Wang Li-Li, Li Da-Hong. Pressure and temperature dependence of shear modulus and yield strength for aluminum, copper and tungsten under shock compression. Acta Physica Sinica, 2005, 54(5): 2194-2197. doi: 10.7498/aps.54.2194
[18]	Feng Wei-Cun, Gao Ru-Wei, Han Guang-Bing, Zhu Ming-Gang, Li Wei. Exchange-coupling interaction and effective anisotropy of NdFeB nanocomposite permanent magnetic materials. Acta Physica Sinica, 2004, 53(9): 3171-3176. doi: 10.7498/aps.53.3171
[19]	Li Mei-Juan, Hu Hai-Yun, Xing Xiu-San. The relationship between fatigue life and grain size of polycrystalline metals. Acta Physica Sinica, 2003, 52(8): 2092-2095. doi: 10.7498/aps.52.2092
[20]	Teng Jiao, Cai Jian-Wang, Xiong Xiao-Tao, Lai Wu-Yan, Zhu Feng-Wu. Ｔｈｅｉｎｆｌｕｅｎｃｅｏｆ(Ｎｉ0 81 Ｆｅ0 1 9)1 -ｘＣｒｘｓｅｅｄｌａｙｅｒｏｎｔｈｅｅｘｃｈａｎｇｅｂｉａｓｏｆＮｉＦｅＦｅＭｎｂｉｌａｙｅｒｓ. Acta Physica Sinica, 2002, 51(12): 2849-2853. doi: 10.7498/aps.51.2849

Catalog

Vol. 71, Issue 3 - 2022-02-05

Metrics

Abstract views: 5396
PDF Downloads: 104
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板