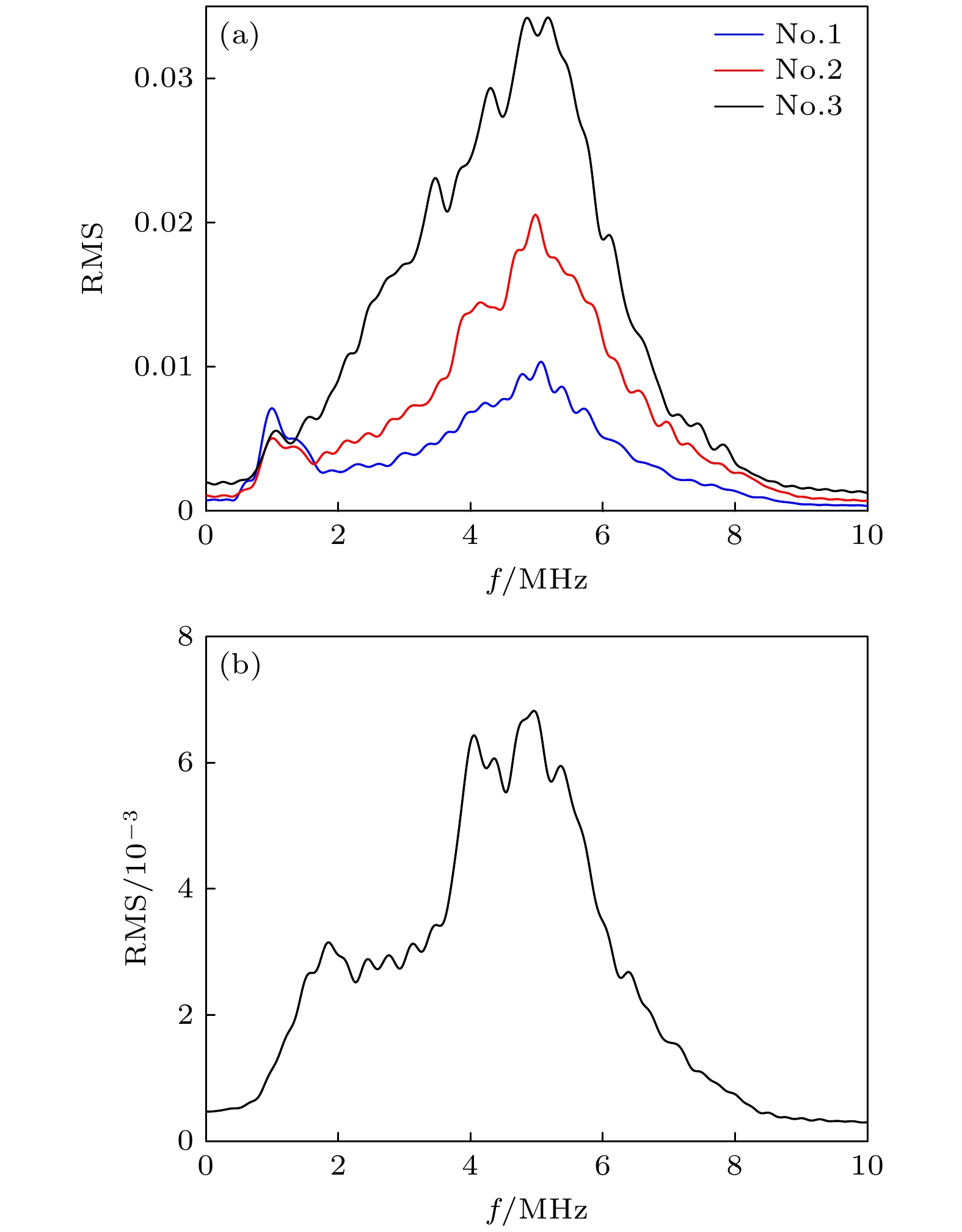
-
镍基高温合金GH4742具有优异的机械性能, 而晶粒尺寸是影响其性能的关键因素. 基于物理模型的超声背散射法可以实现晶粒尺寸高效和准确的评价, 但受限于复杂模型或多角度声束测量. 因此, 本文提出了一种只需单向测量的背散射系数法, 且无需考虑测量系统等无关因素的影响. 基于独立散射模型, 推导了只与材料相关的背散射系数; 利用空间相关函数描述了晶粒尺寸与背散射系数的关系; 采用参考信号剔除干扰因素的影响, 实现实验背散射系数的快速提取. 制备三组不同晶粒尺寸的GH4742试块进行相控阵超声实验和平均晶粒尺寸评价, 并与金相法结果进行对比. 结果表明本文方法得到的晶粒度与金相法结果最大相对误差为–22.7%, 最小相对误差为–3.7%.
GH4742 nickel-based superalloy exhibits excellent mechanical properties, and grain size is a key factor affecting its performance. A physical model-based ultrasonic backscattering method makes grain size measurement accurate and efficient. Nevertheless, it is constrained by complex models or multiple measurements taken from various beam angles. As a result, a backscattering coefficient method that requires only a single measurement for grain size evaluation is proposed. In contrast to the existing methods, the proposed method solely focuses on the backscattering coefficient component of the backscattering signal. It effectively eliminates the influence of unrelated factors, such as the measurement system and the acoustic field, through the utilization of reference signals. The independent scattering model is employed to derive the backscattering coefficient, which solely pertains to the material itself. The relationship between grain size and backscattering coefficient is described by using a spatial correlation function. To consider the irrelevant factors, an experimental measurement method is developed by using the reference signals. Through numerical calculation and analysis, it has been observed that the backscattering coefficient is closely related to the frequency. When the product of the wavenumber and the grain size is significantly greater than 1 ( $ ka\gg 1 $ ), a Stochastic scattering limit is reached. Conversely, when$ ka\ll 1 $ , a Rayleigh scattering limit is observed. Furthermore, the backscattering coefficient is directly proportional to the grain size. As a general trend, larger grain sizes result in higher backscattering coefficient.Three sets of GH4742 specimens with different grain sizes are prepared for phased array ultrasound experiments. It can be observed that the experimental backscattering coefficients, root mean square (RMS) values, and the amplitude trend of time domain signal are consistent. To perform grain size inversion, the backscattering coefficients in the effective bandwidth range of the probe are selected. By utilizing the least-square method, the theoretical backscattering coefficient is employed to fit the curves of the experimental backscattering coefficients. The evaluation results are compared with those obtained by metallographic analysis. The results show that the grain sizes obtained by the proposed method have a maximum relative error of –22.7% and a minimum relative error of –3.7%. -
Keywords:
- ultrasonic evaluation /
- backscattering coefficient /
- GH4742 /
- grain size
[1] Cruzado A, Lucarini S, Lorca J, Segurado J 2018 Int. J. Fatigue 113 236
Google Scholar
[2] Lehto P, Remes H, Saukkonen T, Hänninen H, Romanoff J 2014 Mater. Sci. Eng. A 592 28
Google Scholar
[3] 秦鹤勇, 李振团, 赵光普, 张文云, 张晓敏 2023 材料研究学报 37 502
Google Scholar
Qin H Y, Li Z T, Zhao G P, Zhang W Y, Zhang X M 2023 Chin. J. Mater. Res. 37 502
Google Scholar
[4] He K, Chen N F, Wang C J, Wei L S, Chen J K 2018 Cryst. Res. Technol. 53 1700157
Google Scholar
[5] Toth L S, Biswas S, Gu C, Beausir B 2013 Mater. Charact. 84 67
Google Scholar
[6] Aghaie-Khafri M, Honarvar F, Zanganeh S 2012 J. Nondest. Eval. 31 191
Google Scholar
[7] Homa L, Cherry M, Wertz J 2021 Inverse Probl. 37 065004
Google Scholar
[8] 金士杰, 王志诚, 田鑫, 孙旭, 林莉 2023 航空学报 44 231
Google Scholar
Jin S J, Wang Z C, Tian X, Sun X, Lin L 2023 Acta Aeronaut. Astronaut. Sin. 44 231
Google Scholar
[9] 朱琦, 许多, 张元军, 李玉娟, 王文, 张海燕 2022 71 244301
Google Scholar
Zhu Q, Xu D, Zhang Y J, Li Y J, Wang W, Zhang H Y 2022 Acta Phys. Sin. 71 244301
Google Scholar
[10] Liu Y, Tian Q, Guan X F 2021 NDT&E Int. 122 102479
Google Scholar
[11] 李珊, 李雄兵, 宋永锋, 陈超 2018 67 234301
Google Scholar
Li S, Li X B, Song Y F, Chen C 2018 Acta Phys. Sin. 67 234301
Google Scholar
[12] 东蕊, 刘成成, 蔡勋兵, 邵留磊, 李博艺, 他得安 2019 68 184301
Google Scholar
Dong R, Liu C C, Cai X B, Shao L L, Li B Y, Ta D A 2019 Acta Phys. Sin. 68 184301
Google Scholar
[13] Yu L X, Guo Y M, Margetan F J, Thompson R B 2001 Rev. Prog. Quant. Nondestruct. Eval. 20 1330
Google Scholar
[14] Zhang F Z, Wang C S, Wu Y S, Zhou L Z, Tian Q 2022 Mater. Sci. Eng. A 832 142488
Google Scholar
[15] Margetan F J, Yu L X, Thompson R B 2005 Rev. Prog. Quant. Nondestruct. Eval. 24 1300
Google Scholar
[16] Rokhlin S I, Sha G F, Li J, Pilchak A L 2021 Ultrasonics 115 106433
Google Scholar
[17] Liu Y, Tian Q, Yu P, He J J, Guan X F 2022 NDT E Int. 129 102634
Google Scholar
[18] Ghoshal G, Turner J A, Weaver R L 2007 J. Acoust. Soc. Am. 122 2009
Google Scholar
[19] Arguelles A P, Kube C M, Hu P, Turner J A 2016 T J. Acoust. Soc. Am. 140 1570
Google Scholar
[20] Wydra A, Chertov A, Maev R G, Kube C M, Du H L, Turner J A 2015 Res. Nondestruct. Eval. 26 225
Google Scholar
[21] Rose J H 1991 Rev. Prog. Quant. Nondestruct. Eval. 10B 1715
Google Scholar
[22] Margetan F J, Gray T, Thompson R B 1991 Rev. Prog. Quant. Nondestruct. Eval. 10B 1721
Google Scholar
[23] Kube C M, Turner J A 2015 Wave Motion 27 182
Google Scholar
[24] Weaver R L 1990 J. Mech. Phys. Solids 38 55
Google Scholar
[25] Stanke F E 1986 J. Acoust. Soc. Am. 80 1479
Google Scholar
[26] 杜金辉, 吕旭东, 董建新, 孙文儒, 毕中南, 赵光普, 邓群, 崔传勇, 马惠萍, 张北江 2019 金属学报 55 1115
Google Scholar
Du J H, Lü X D, Dong J X, Sun W R, Bi Z N, Zhao G P, Deng Q, Cui C Y, Ma H P, Zhang B J 2019 Acta Metallurg. Sin. 55 1115
Google Scholar
[27] Haldipur P, Margetan F J, Thompson R B 2004 Rev. Prog. Quant. Nondestructive Eval. 23 1061
Google Scholar
-
图 1 所提出方法基本思路图
Fig. 1. Overall development diagram of the backscattering coefficient quantification of grain size.
图 3 瑞利散射极限和随机散射极限下归一化背散射幅值与归一化频率关系
Fig. 3. Normalized backscattering amplitude versus normalized frequency within the Rayleigh limits and stochastic limits.
图 4 不同频率下背散射幅值与晶粒尺寸关系 (a) 0—100 MHz; (b) 0—20 MHz. 其他参数: $ {c}_{11}=250\;{\mathrm{G}}{\mathrm{P}}{\mathrm{a}} $, $ {c}_{12}= $$ 160\;{\mathrm{G}}{\mathrm{P}}{\mathrm{a}} $, $ {c}_{44}=118\;{\mathrm{G}}{\mathrm{P}}{\mathrm{a}} $, $ \rho =8240\;{\mathrm{k}}{\mathrm{g}}/{{\mathrm{m}}}^{3} $
Fig. 4. Backscattering amplitude versus grain sizes in different frequencies: (a) 0–100 MHz; (b) 0–20 MHz, where $ {c}_{11} = $$ 250\;{\mathrm{G}}{\mathrm{P}}{\mathrm{a}} $, $ {c}_{12} = 160\;{\mathrm{G}}{\mathrm{P}}{\mathrm{a}} $, $ {c}_{44} = 118\;{\mathrm{G}}{\mathrm{P}}{\mathrm{a}} $, $ \rho = 8240\;{\mathrm{k}}{\mathrm{g}}/{{\mathrm{m}}}^{3} $
图 6 不同试块的时域背散射信号对比
Fig. 6. Comparison of backscatter signals of different test blocks in time domain.
图 7 不同试块的背散射信号均方根对比 (a) 被测GH4742试块; (b) 参考试块
Fig. 7. Measured backscatter RMS spectra of different test blocks: (a) GH4742 blocks; (b) reference block.
图 8 背散射系数实验测量曲线及其拟合结果 (a) No.1; (b) No.2; (c) No.3
Fig. 8. Experimental measurement curve of backscattering coefficient and its fitting results: (a) No.1; (b) No.2; (c) No.3.
图 9 所有试块的金相图 (a) No.1; (b) No.2; (c) No.3
Fig. 9. Micrographs of all test blocks: (a) No.1; (b) No.2; (c) No.3.
表 1 GH4742化学组成成分
Table 1. Chemical composition of GH4742.
成分 Ti Cr Mo Co Nb Mn Fe C Al B Ni 质量分数% 2.52 14.0 5.12 9.95 2.6 0.2 0.5 0.06 2.54 0.01 Bal. 
下载: 导出CSV
表 2 晶粒尺寸测量结果对比
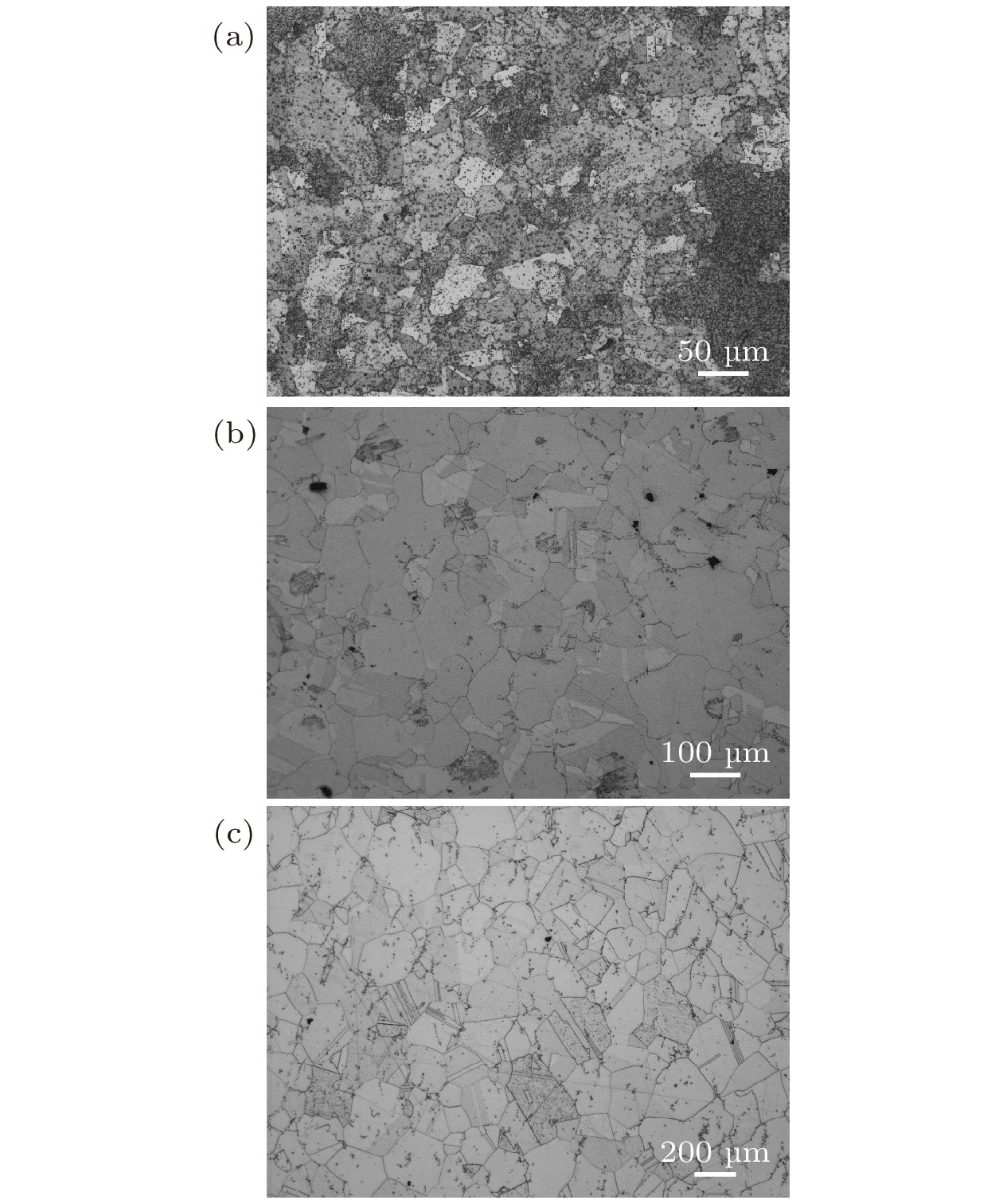
Table 2. Comparison of grain size measured by micrographs and backscattering coefficient.
试块 金相法 金相法晶粒度 背散射
系数法背散射系数
法晶粒度No.1 55 μm 5.4 58 μm 5.2 No.2 77 μm 4.4 103 μm 3.6 No.3 163 μm 2.2 200 μm 1.7
下载: 导出CSV
-
[1] Cruzado A, Lucarini S, Lorca J, Segurado J 2018 Int. J. Fatigue 113 236
Google Scholar
[2] Lehto P, Remes H, Saukkonen T, Hänninen H, Romanoff J 2014 Mater. Sci. Eng. A 592 28
Google Scholar
[3] 秦鹤勇, 李振团, 赵光普, 张文云, 张晓敏 2023 材料研究学报 37 502
Google Scholar
Qin H Y, Li Z T, Zhao G P, Zhang W Y, Zhang X M 2023 Chin. J. Mater. Res. 37 502
Google Scholar
[4] He K, Chen N F, Wang C J, Wei L S, Chen J K 2018 Cryst. Res. Technol. 53 1700157
Google Scholar
[5] Toth L S, Biswas S, Gu C, Beausir B 2013 Mater. Charact. 84 67
Google Scholar
[6] Aghaie-Khafri M, Honarvar F, Zanganeh S 2012 J. Nondest. Eval. 31 191
Google Scholar
[7] Homa L, Cherry M, Wertz J 2021 Inverse Probl. 37 065004
Google Scholar
[8] 金士杰, 王志诚, 田鑫, 孙旭, 林莉 2023 航空学报 44 231
Google Scholar
Jin S J, Wang Z C, Tian X, Sun X, Lin L 2023 Acta Aeronaut. Astronaut. Sin. 44 231
Google Scholar
[9] 朱琦, 许多, 张元军, 李玉娟, 王文, 张海燕 2022 71 244301
Google Scholar
Zhu Q, Xu D, Zhang Y J, Li Y J, Wang W, Zhang H Y 2022 Acta Phys. Sin. 71 244301
Google Scholar
[10] Liu Y, Tian Q, Guan X F 2021 NDT&E Int. 122 102479
Google Scholar
[11] 李珊, 李雄兵, 宋永锋, 陈超 2018 67 234301
Google Scholar
Li S, Li X B, Song Y F, Chen C 2018 Acta Phys. Sin. 67 234301
Google Scholar
[12] 东蕊, 刘成成, 蔡勋兵, 邵留磊, 李博艺, 他得安 2019 68 184301
Google Scholar
Dong R, Liu C C, Cai X B, Shao L L, Li B Y, Ta D A 2019 Acta Phys. Sin. 68 184301
Google Scholar
[13] Yu L X, Guo Y M, Margetan F J, Thompson R B 2001 Rev. Prog. Quant. Nondestruct. Eval. 20 1330
Google Scholar
[14] Zhang F Z, Wang C S, Wu Y S, Zhou L Z, Tian Q 2022 Mater. Sci. Eng. A 832 142488
Google Scholar
[15] Margetan F J, Yu L X, Thompson R B 2005 Rev. Prog. Quant. Nondestruct. Eval. 24 1300
Google Scholar
[16] Rokhlin S I, Sha G F, Li J, Pilchak A L 2021 Ultrasonics 115 106433
Google Scholar
[17] Liu Y, Tian Q, Yu P, He J J, Guan X F 2022 NDT E Int. 129 102634
Google Scholar
[18] Ghoshal G, Turner J A, Weaver R L 2007 J. Acoust. Soc. Am. 122 2009
Google Scholar
[19] Arguelles A P, Kube C M, Hu P, Turner J A 2016 T J. Acoust. Soc. Am. 140 1570
Google Scholar
[20] Wydra A, Chertov A, Maev R G, Kube C M, Du H L, Turner J A 2015 Res. Nondestruct. Eval. 26 225
Google Scholar
[21] Rose J H 1991 Rev. Prog. Quant. Nondestruct. Eval. 10B 1715
Google Scholar
[22] Margetan F J, Gray T, Thompson R B 1991 Rev. Prog. Quant. Nondestruct. Eval. 10B 1721
Google Scholar
[23] Kube C M, Turner J A 2015 Wave Motion 27 182
Google Scholar
[24] Weaver R L 1990 J. Mech. Phys. Solids 38 55
Google Scholar
[25] Stanke F E 1986 J. Acoust. Soc. Am. 80 1479
Google Scholar
[26] 杜金辉, 吕旭东, 董建新, 孙文儒, 毕中南, 赵光普, 邓群, 崔传勇, 马惠萍, 张北江 2019 金属学报 55 1115
Google Scholar
Du J H, Lü X D, Dong J X, Sun W R, Bi Z N, Zhao G P, Deng Q, Cui C Y, Ma H P, Zhang B J 2019 Acta Metallurg. Sin. 55 1115
Google Scholar
[27] Haldipur P, Margetan F J, Thompson R B 2004 Rev. Prog. Quant. Nondestructive Eval. 23 1061
Google Scholar
下载:
计量
- 文章访问数: 1940
- PDF下载量: 125
- 被引次数: 0
