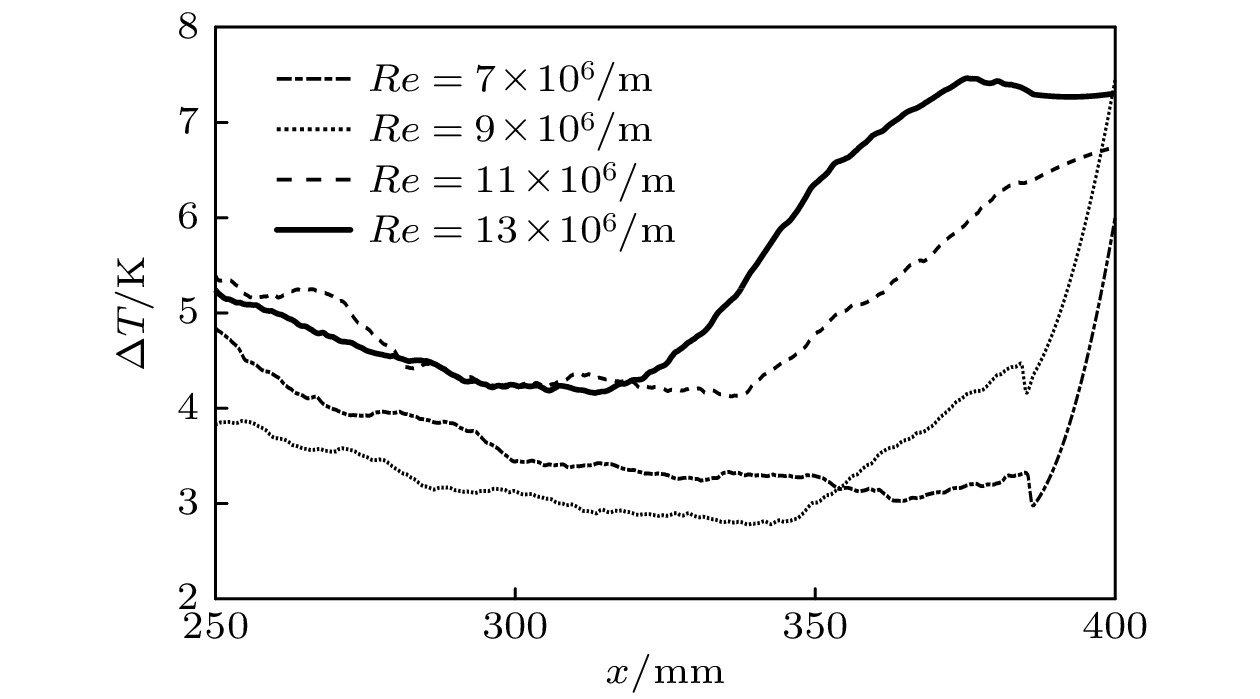
-
In this work, the cross-flow instability on the surface of a blunt elliptical cone with a long-short-axis ratio of 4∶1 is studied experimentally in the Mach 6 hypersonic quiet wind tunnel. Comprehensive use of temperature sensitive paint (TSP) technology, nano-tracer-based planar laser scattering (NPLS) technology and Kulite sensor pressure test to measure the temperature distribution in the cross-flow area on the surface of the model, boundary layer flow structure and model surface pressure are tested. The mechanism of boundary layer transition in the cross-flow control area on the surface of the elliptical cone is studied, and the influence law of incoming flow unit Reynolds number and angle of attack on boundary layer transition is obtained, and some conclusions are obtained below. In the wind tunnel noise mode, the transition of the boundary layer in the cross-flow area between the surface center line and the leading edge of the elliptical cone model with a length-to-short-axis ratio of 4∶1 is controlled by the traveling waves, and no footprint of the steady vortex is found. The characteristic frequency of the traveling wave is about 20 kHz. When the unit Reynolds number of the incoming flow increases, the transition position will be advanced, and the frequency and amplitude of the traveling wave will increase. Within a certain angle of attack, the transition position of the upwind boundary layer is delayed, and the characteristic frequency of the traveling wave does not change much but the energy is weakened. When the angle of attack continues to increase, the transition phenomenon disappears.
-
Keywords:
- hypersonic /
- elliptical cone /
- crossflow instabilities /
- boundary layer transition
[1] Kimmel R L, Klein M A, Schwoerke S N 1997 J. Spacecr. Rockets 34 409
Google Scholar
[2] Porter K M, Poggie J, Kimmel R L 2017 47th AIAA Fluid Dynamics Conference Denver, Colorado, USA, June 5−9, 2017 p3132
[3] Kimmel R L, Adamczak D W, Hartley D, Alesi H, Frost M, Pietsch R, Shannon J, Sylvester T 2018 J. Spacecr. Rockets 55 1303
Google Scholar
[4] Tufts M P, Borg M, Gosse R, Kimmel R L 2018 Applied Aerodynamics Conference Atlanta, Georgia, USA, June 25−29, 2018 p3807
[5] Choudhari M, Chang C L, Li F, Berger K, Candler G, Kimmel R 2009 39th AIAA Fluid Dynamics Conference San Antonio, Texas, USA, June 22−25, 2009 p4056
[6] Paredes P, Theofilis V 2015 J. Fluids Struct. 53 36
Google Scholar
[7] Yates H, Juliano T J, Matlis E H, Tufts M W 2018 2018 AIAA Aerospace Sciences Meeting Kissimmee, Florida, USA, January 8−12, 2018 p1076
[8] Saric W S, Reed H L 2003 41st Aerospace Sciences Meeting and Exhibit Reno, Nevada, USA, January 6−9, 2003 p771
[9] Choudhari M, Li F, Paredes P 2020 AIAA Scitech 2020 Forum Orlando, Florida, USA, January 6−10, 2020 p828
[10] Paredes P, Gosse R, Theofilis V, Kimmel R L 2016 J. Fluids Mech. 804 442
Google Scholar
[11] Saric W S, Reed H L, White E B 2003 Annu. Rev. Fluids Mech. 35 413
[12] Reed H L, Saric W S, Arnal D 1996 Annu. Rev. Fluids Mech. 28 389
Google Scholar
[13] Bippes H 1999 Prog. Aerosp. Sci. 35 363
Google Scholar
[14] White E B, Saric W S 2005 J. Fluids Mech. 525 275
Google Scholar
[15] Reed H L, Saric W S 2002 40th Aerospace Sciences Meeting and Exhibit Reno, Nevada, USA, January 14−17, 2002 p147
[16] Kimmel R L, Poggie J J, Schwoerke S N 1999 AIAA. J. 37 1080
Google Scholar
[17] Dinzl D J, Candler G V 2017 AIAA. J. 55 1769
[18] Moyes A J, Kocian T S, Mullen D, Reed H L 2018 J. Spacecr. Rockets 55 1341
Google Scholar
[19] Berridge D, Chou A, Ward C, Steen L, Gilbert P, Juliano T, Schneider S, Gronvall J 2010 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition Orlando, Florida, USA, January 4−7, 2010 p1061
[20] Juliano T J, Schneider S P 2010 40th Fluid Dynamics Conference and Exhibit Chicago, Illinois, USA, June 28− July 1, 2010 p5004
[21] Borg M P, Kimmel R L 2016 54th AIAA Aerospace Sciences Meeting San Diego, California, USA, January 4−8, 2016 p354
[22] Borg M P, Kimmel R L, Stanfiled S 2012 42nd AIAA Fluid Dynamics Conference and Exhibit New Orleans, Louisiana, USA, June 25−28, 2012 p2821
[23] Borg M P, Kimmel R, Stanfield S 2011 41st AIAA Fluid Dynamics Conference and Exhibit Honolulu, Hawaii, USA, June 27−30, 2011 p3247
[24] Borg M P, Kimmel R, Stanfield S 2013 43rd Fluid Dynamics Conference San Diego, California, June 24−27, 2013 p2737
[25] Borg M P, Kimmel R, Stanfield S 2015 J. Spacecr. Rockets 52 664
Google Scholar
[26] Juliano T J, Borg M P, Schneider S P 2015 AIAA. J. 53 832
Google Scholar
[27] Juliano T J, Paquin L, Borg M P 2016 54th AIAA Aerospace Sciences Meeting San Diego, California, USA, January 4−8, 2016 p595
[28] Juliano T J, Paquin L, Borg M P 2019 AIAA. J. 57 2001
Google Scholar
[29] Borg M P, Kimmel R L, Hofferth J W, Bowersox R D, Mai C L 2015 53rd AIAA Aerospace Sciences Meeting Kissimmee, Florida, USA, January 5−9, 2015 p278
[30] Hembling E, Wirth J, Semper M 2021 AIAA Scitech 2021 Forum January 11−15, 19−21, 2021 p5
[31] Juliano T J, Jewell J S, Kimmel R 2019 J. Spacecr. Rockets 56 1045
Google Scholar
[32] Juliano T J, Jewell J S, Kimmel R 2021 J. Spacecr. Rockets 58 265
Google Scholar
[33] Li F, Choudhari M, Chang C L, White J, Kimmel R, Adamczak D, Borg M, Stanfifield S, Smith M 2012 42nd AIAA Fluid Dynamics Conference and Exhibit New Orleans, Louisiana, USA, June 25−28, 2012 p2961
[34] [35] Niu H, Yi S, Liu X, Lu X, Gang D 2020 Chin. J. Aeronaut. 33 1889
Google Scholar
[36] 刘小林, 易仕和, 牛海波, 陆小革, 赵鑫海 2018 67 174701
Google Scholar
Liu X L, Yi S H, Niu H B, Lu X G, Zhao X H 2018 Acta Phys. Sin. 67 174701
Google Scholar
[37] Zhao Y, Yi S, Tian L, Cheng Z 2009 Sci. China Ser. D 52 3640
Google Scholar
-
图 1 高超声速静风洞照片
Figure 1. Picture of hypersonic static wind tunnel.
图 2 模型示意图
Figure 2. Model diagram.
图 3 标定曲线
Figure 3. Calibration curve.
图 4 NPLS技术原理图
Figure 4. Schematic diagram of NPLS technique.
图 5 传感器分布示意图
Figure 5. Distribution diagram of sensors.
图 6 温升分布云图(0°, Re = 1.3 × 107/m)
Figure 6. Contour of temperature rise distribution (0°, Re = 1.3 × 107/m).
图 7 时间相关的NPLS图像
Figure 7. NPLS images with time correlation.
图 8 温升曲线
Figure 8. Temperature rise curve.
图 9 功率谱计算结果 (0°, Re = 1.3 × 107/m) (a) 风洞运行时; (b) 底噪
Figure 9. Power spectrum calculation results (0°, Re = 1.3 × 107/m): (a) During wind tunnel operation; (b) noise of background.
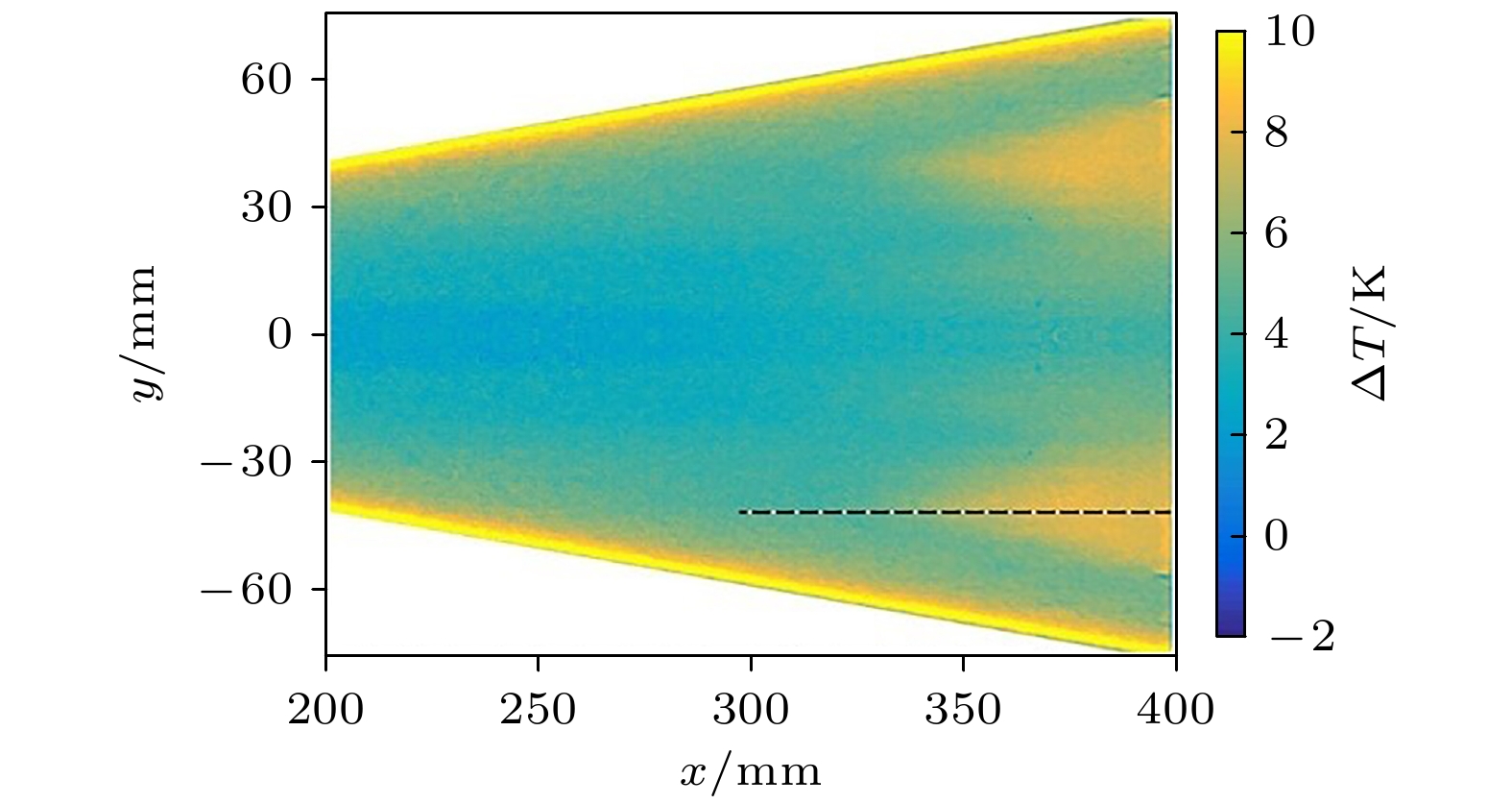
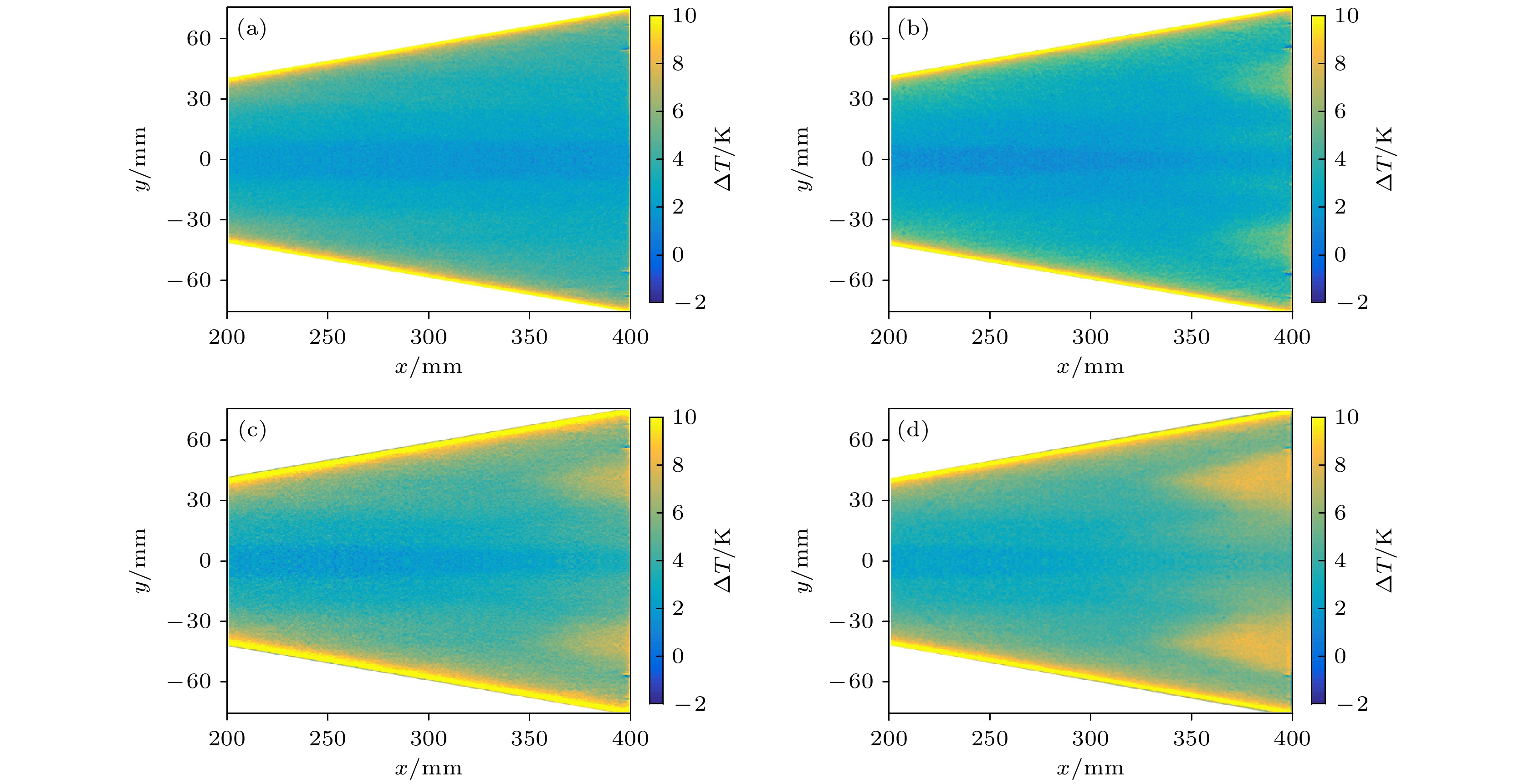
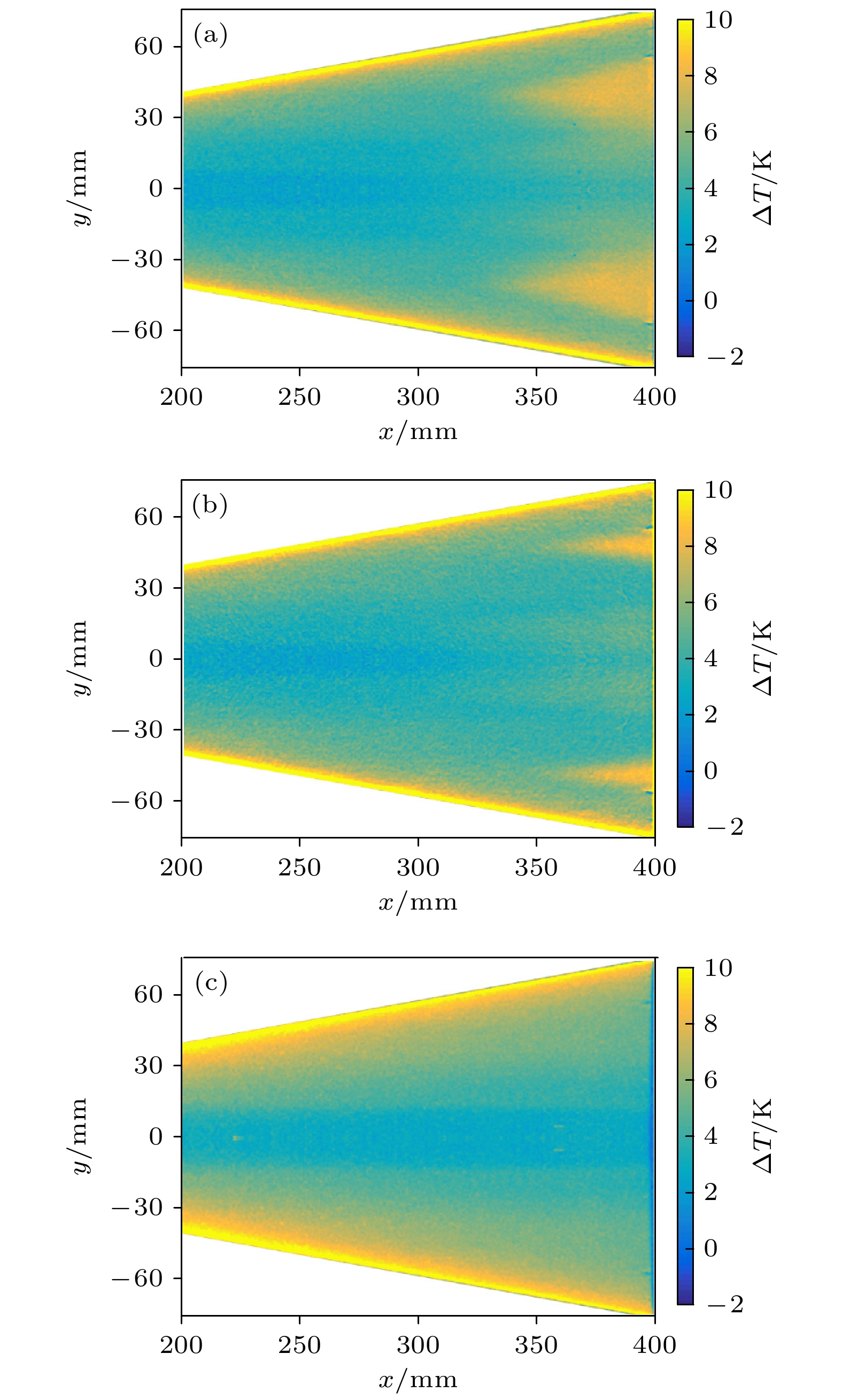
图 10 0°攻角不同雷诺数条件下TSP图像 (a)
${Re}_{\rm{\infty }} = 7\times {10}^{6}\;{\rm{m}}^{-1}$ ; (b)$ {Re}_{\rm{\infty }} = 9\times {10}^{6}\;{\rm{m}}^{-1} $ ; (c)$ {Re}_{\rm{\infty }} = 1.1\times {10}^{7}\;{\rm{m}}^{-1} $ ; (d)$ {Re}_{\rm{\infty }} = 1.3\times {10}^{7}\;{\rm{m}}^{-1} $ Figure 10. TSP images of angle of attack of 0° at different Reynolds numbers: (a)
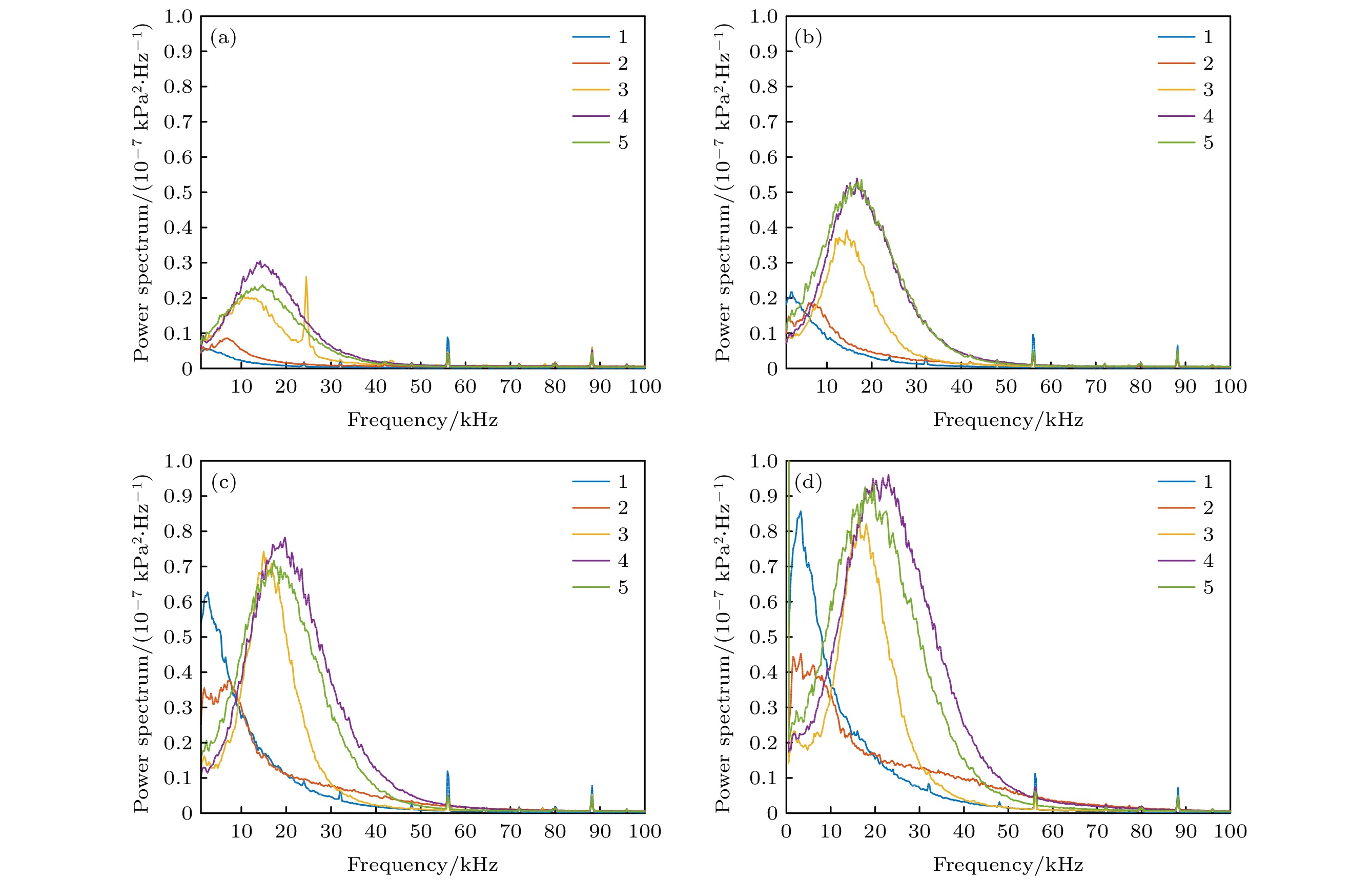
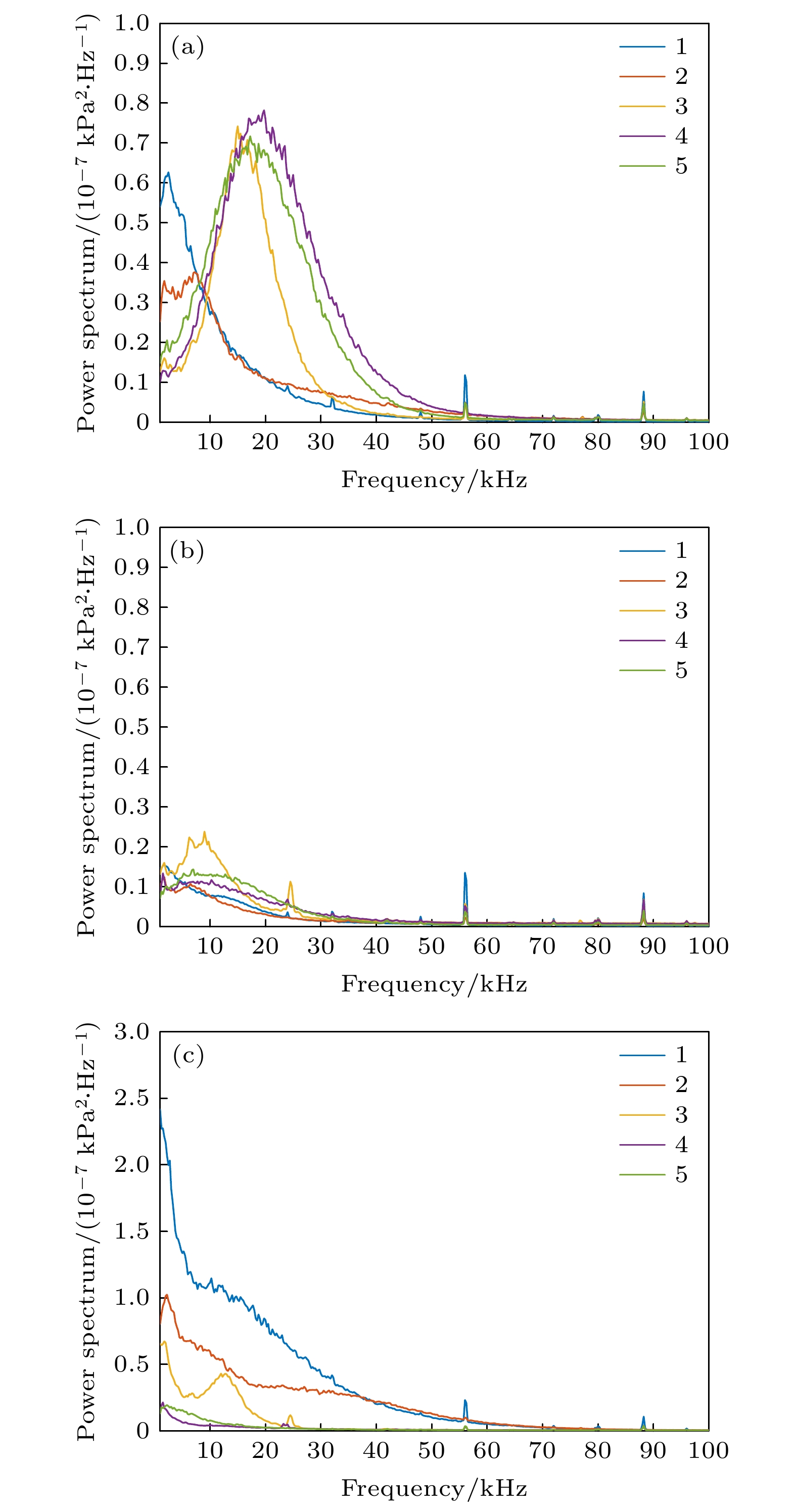
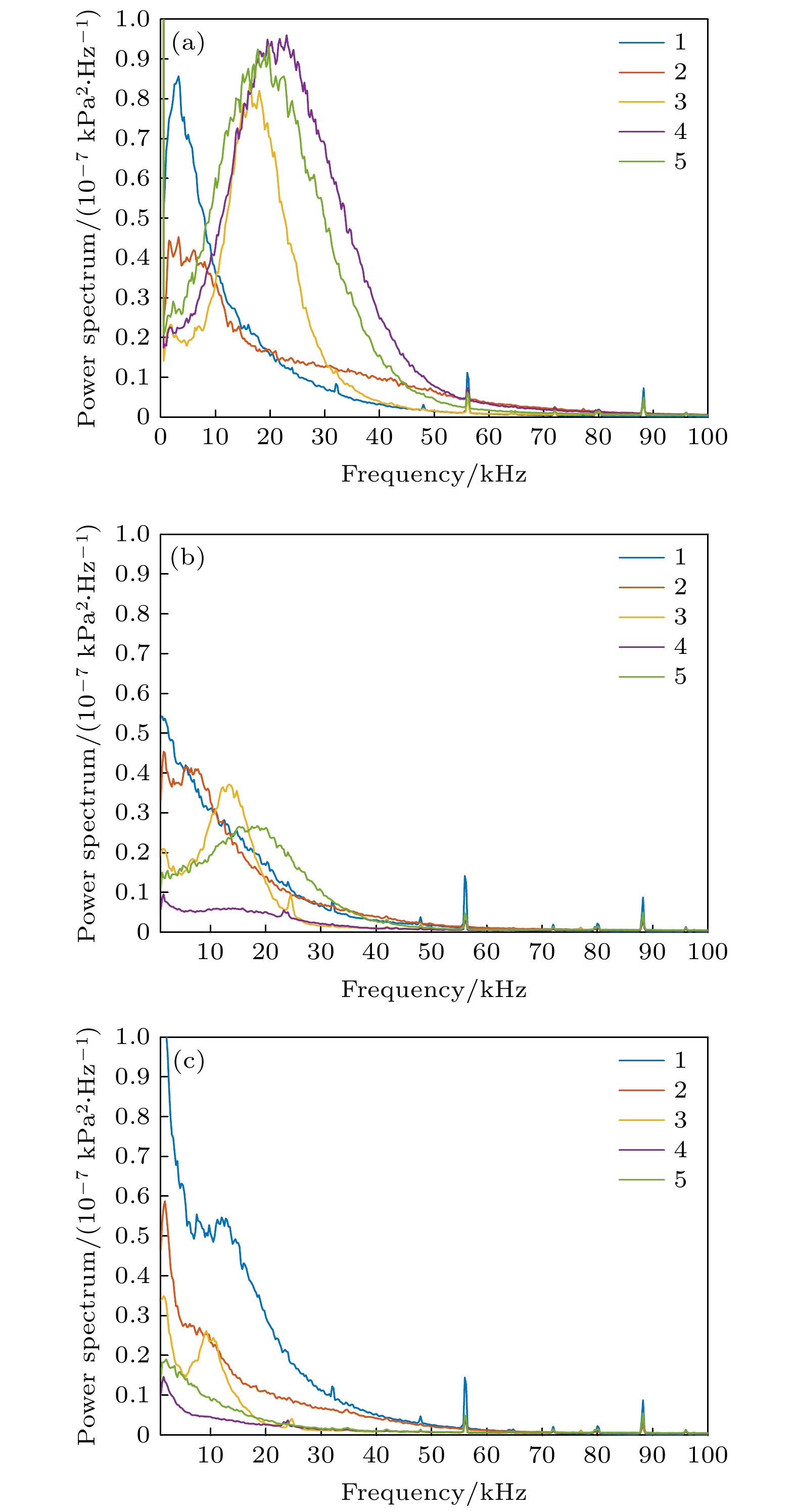
$ {Re}_{\rm{\infty }} = 7\times {10}^{6}\;{\rm{m}}^{-1} $ ; (b)$ {Re}_{\rm{\infty }} = 9\times {10}^{6}\;{\rm{m}}^{-1} $ ; (c)$ {Re}_{\rm{\infty }} = 1.1\times \;{10}^{7}{\rm{m}}^{-1} $ ; (d)$ {Re}_{\rm{\infty }} = 1.3\times {10}^{7}\;{\rm{m}}^{-1} $ .
图 11 不同雷诺数条件下温升曲线
Figure 11. Temperature rise curves at different Reynolds numbers.
图 12 0°攻角、不同雷诺数条件下功率谱结果 (a)
$ {Re}_{\rm{\infty }} = 7\times {10}^{6}\;{\rm{m}}^{-1} $ ; (b)$ {Re}_{\rm{\infty }} = 9\times {10}^{6}\;{\rm{m}}^{-1} $ ; (c)$ {Re}_{\rm{\infty }} = 1.1\times {10}^{7}\;{\rm{m}}^{-1} $ ; (d)$ {Re}_{\rm{\infty }} = 1.3\times {10}^{7}\;{\rm{m}}^{-1} $ Figure 12. Power spectrum calculation results of angle of attack of 0° at different Reynolds numbers: (a)
$ {Re}_{\rm{\infty }} = 7\times {10}^{6}\;{\rm{m}}^{-1} $ ; (b)$ {Re}_{\rm{\infty }} = 9\times {10}^{6}\;{\rm{m}}^{-1} $ ; (c)$ {Re}_{\rm{\infty }} = 1.1\times {10}^{7}\;{\rm{m}}^{-1} $ ; (d)$ {Re}_{\rm{\infty }} = 1.3\times {10}^{7}\;{\rm{m}}^{-1}$ 
图 13 不同攻角TSP图像(
${Re}_{\rm{\infty }} = 11\times {10}^{6}\;{\rm{m}}^{-1}$ ) (a) 0°; (b) 2°; (c) 5°Figure 13. TSP images at different angles of attack (
${Re}_{\rm{\infty }} = $ $ 11\times {10}^{6}\;{\rm{m}}^{-1}$ ) : (a) 0°; (b) 2°; (c) 5°.
图 14 不同攻角TSP图像(
${Re}_{\rm{\infty }} = 13\times {10}^{6}\;{\rm{m}}^{-1}$ ) (a) 0°; (b) 2°; (c) 5°Figure 14. TSP images at different attack angles (
${Re}_{\rm{\infty }} = $ $ 13\times {10}^{6}\;{\rm{m}}^{-1}$ ): (a) 0°; (b) 2°; (c) 5°.
图 15 不同攻角功率谱结果(
${Re}_{\rm{\infty }} = 11\times {10}^{6}\;{\rm{m}}^{-1}$ ) (a) 0°; (b) 2°; (c) 5°Figure 15. Power spectrum calculation results at different angles of attack (
${Re}_{\rm{\infty }} = 11\times {10}^{6}\;{\rm{m}}^{-1}$ ) : (a) 0°; (b) 2°; (c) 5°.
图 16 不同攻角功率谱结果(
${Re}_{\rm{\infty }} = 13\times {10}^{6}\;{\rm{m}}^{-1}$ ) (a) 0°; (b) 2°; (c) 5°Figure 16. Power spectrum calculation results at different angles of attack (
${Re}_{\rm{\infty }} = 13\times {10}^{6}\;{\rm{m}}^{-1}$ ) : (a) 0°; (b) 2°; (c) 5°.表 1 传感器测点位置
Table 1. Sensor measuring point position.
Sensor (x, y)/mm Sensor (x, y)/mm 1 (360, 0) 2 (360, 12) 3 (360, 24) 4 (360, 36) 5 (360, 48) 
DownLoad: CSV
-
[1] Kimmel R L, Klein M A, Schwoerke S N 1997 J. Spacecr. Rockets 34 409
Google Scholar
[2] Porter K M, Poggie J, Kimmel R L 2017 47th AIAA Fluid Dynamics Conference Denver, Colorado, USA, June 5−9, 2017 p3132
[3] Kimmel R L, Adamczak D W, Hartley D, Alesi H, Frost M, Pietsch R, Shannon J, Sylvester T 2018 J. Spacecr. Rockets 55 1303
Google Scholar
[4] Tufts M P, Borg M, Gosse R, Kimmel R L 2018 Applied Aerodynamics Conference Atlanta, Georgia, USA, June 25−29, 2018 p3807
[5] Choudhari M, Chang C L, Li F, Berger K, Candler G, Kimmel R 2009 39th AIAA Fluid Dynamics Conference San Antonio, Texas, USA, June 22−25, 2009 p4056
[6] Paredes P, Theofilis V 2015 J. Fluids Struct. 53 36
Google Scholar
[7] Yates H, Juliano T J, Matlis E H, Tufts M W 2018 2018 AIAA Aerospace Sciences Meeting Kissimmee, Florida, USA, January 8−12, 2018 p1076
[8] Saric W S, Reed H L 2003 41st Aerospace Sciences Meeting and Exhibit Reno, Nevada, USA, January 6−9, 2003 p771
[9] Choudhari M, Li F, Paredes P 2020 AIAA Scitech 2020 Forum Orlando, Florida, USA, January 6−10, 2020 p828
[10] Paredes P, Gosse R, Theofilis V, Kimmel R L 2016 J. Fluids Mech. 804 442
Google Scholar
[11] Saric W S, Reed H L, White E B 2003 Annu. Rev. Fluids Mech. 35 413
[12] Reed H L, Saric W S, Arnal D 1996 Annu. Rev. Fluids Mech. 28 389
Google Scholar
[13] Bippes H 1999 Prog. Aerosp. Sci. 35 363
Google Scholar
[14] White E B, Saric W S 2005 J. Fluids Mech. 525 275
Google Scholar
[15] Reed H L, Saric W S 2002 40th Aerospace Sciences Meeting and Exhibit Reno, Nevada, USA, January 14−17, 2002 p147
[16] Kimmel R L, Poggie J J, Schwoerke S N 1999 AIAA. J. 37 1080
Google Scholar
[17] Dinzl D J, Candler G V 2017 AIAA. J. 55 1769
[18] Moyes A J, Kocian T S, Mullen D, Reed H L 2018 J. Spacecr. Rockets 55 1341
Google Scholar
[19] Berridge D, Chou A, Ward C, Steen L, Gilbert P, Juliano T, Schneider S, Gronvall J 2010 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition Orlando, Florida, USA, January 4−7, 2010 p1061
[20] Juliano T J, Schneider S P 2010 40th Fluid Dynamics Conference and Exhibit Chicago, Illinois, USA, June 28− July 1, 2010 p5004
[21] Borg M P, Kimmel R L 2016 54th AIAA Aerospace Sciences Meeting San Diego, California, USA, January 4−8, 2016 p354
[22] Borg M P, Kimmel R L, Stanfiled S 2012 42nd AIAA Fluid Dynamics Conference and Exhibit New Orleans, Louisiana, USA, June 25−28, 2012 p2821
[23] Borg M P, Kimmel R, Stanfield S 2011 41st AIAA Fluid Dynamics Conference and Exhibit Honolulu, Hawaii, USA, June 27−30, 2011 p3247
[24] Borg M P, Kimmel R, Stanfield S 2013 43rd Fluid Dynamics Conference San Diego, California, June 24−27, 2013 p2737
[25] Borg M P, Kimmel R, Stanfield S 2015 J. Spacecr. Rockets 52 664
Google Scholar
[26] Juliano T J, Borg M P, Schneider S P 2015 AIAA. J. 53 832
Google Scholar
[27] Juliano T J, Paquin L, Borg M P 2016 54th AIAA Aerospace Sciences Meeting San Diego, California, USA, January 4−8, 2016 p595
[28] Juliano T J, Paquin L, Borg M P 2019 AIAA. J. 57 2001
Google Scholar
[29] Borg M P, Kimmel R L, Hofferth J W, Bowersox R D, Mai C L 2015 53rd AIAA Aerospace Sciences Meeting Kissimmee, Florida, USA, January 5−9, 2015 p278
[30] Hembling E, Wirth J, Semper M 2021 AIAA Scitech 2021 Forum January 11−15, 19−21, 2021 p5
[31] Juliano T J, Jewell J S, Kimmel R 2019 J. Spacecr. Rockets 56 1045
Google Scholar
[32] Juliano T J, Jewell J S, Kimmel R 2021 J. Spacecr. Rockets 58 265
Google Scholar
[33] Li F, Choudhari M, Chang C L, White J, Kimmel R, Adamczak D, Borg M, Stanfifield S, Smith M 2012 42nd AIAA Fluid Dynamics Conference and Exhibit New Orleans, Louisiana, USA, June 25−28, 2012 p2961
[34] [35] Niu H, Yi S, Liu X, Lu X, Gang D 2020 Chin. J. Aeronaut. 33 1889
Google Scholar
[36] 刘小林, 易仕和, 牛海波, 陆小革, 赵鑫海 2018 67 174701
Google Scholar
Liu X L, Yi S H, Niu H B, Lu X G, Zhao X H 2018 Acta Phys. Sin. 67 174701
Google Scholar
[37] Zhao Y, Yi S, Tian L, Cheng Z 2009 Sci. China Ser. D 52 3640
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 3869
- PDF Downloads: 64
- Cited By: 0
