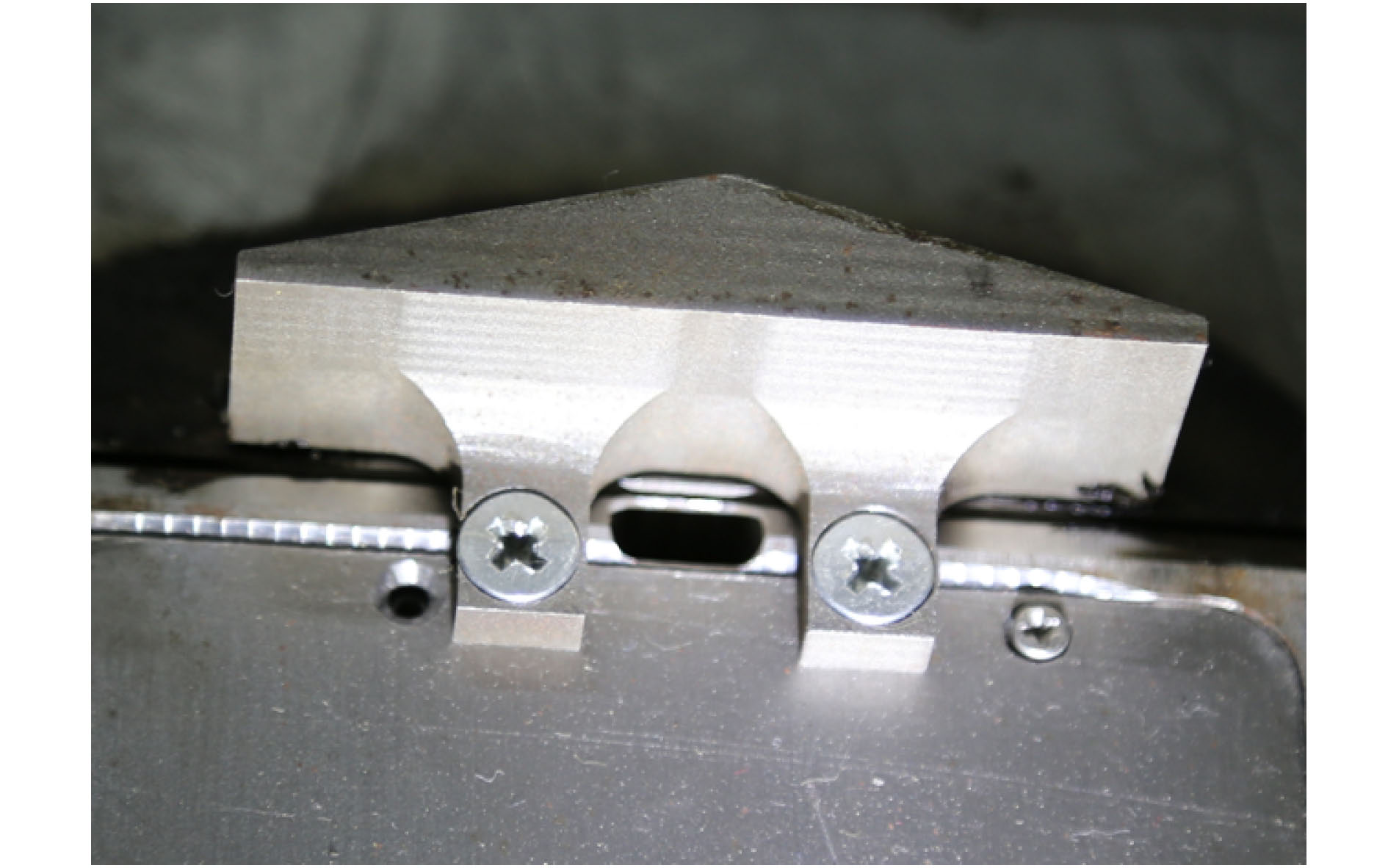
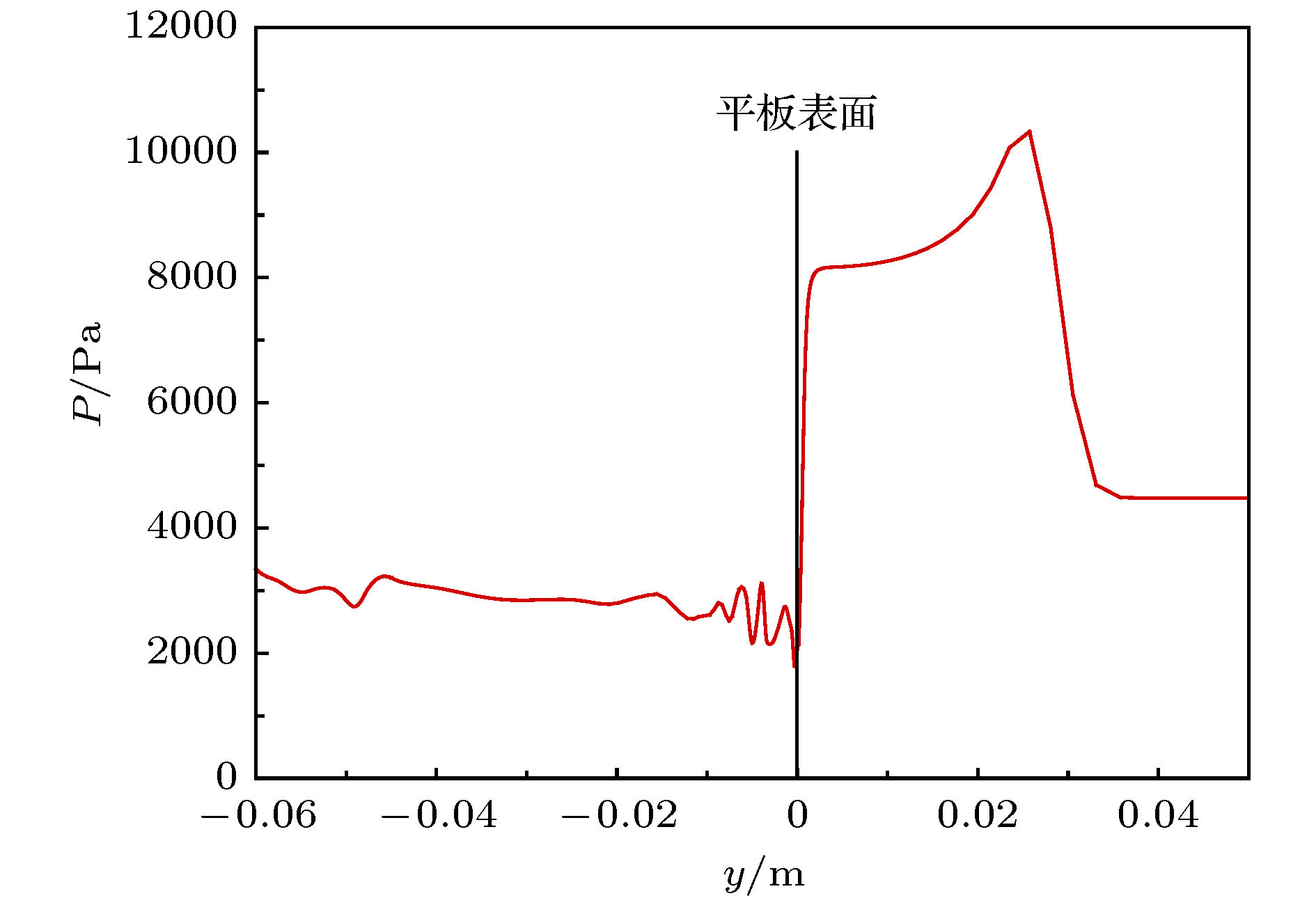
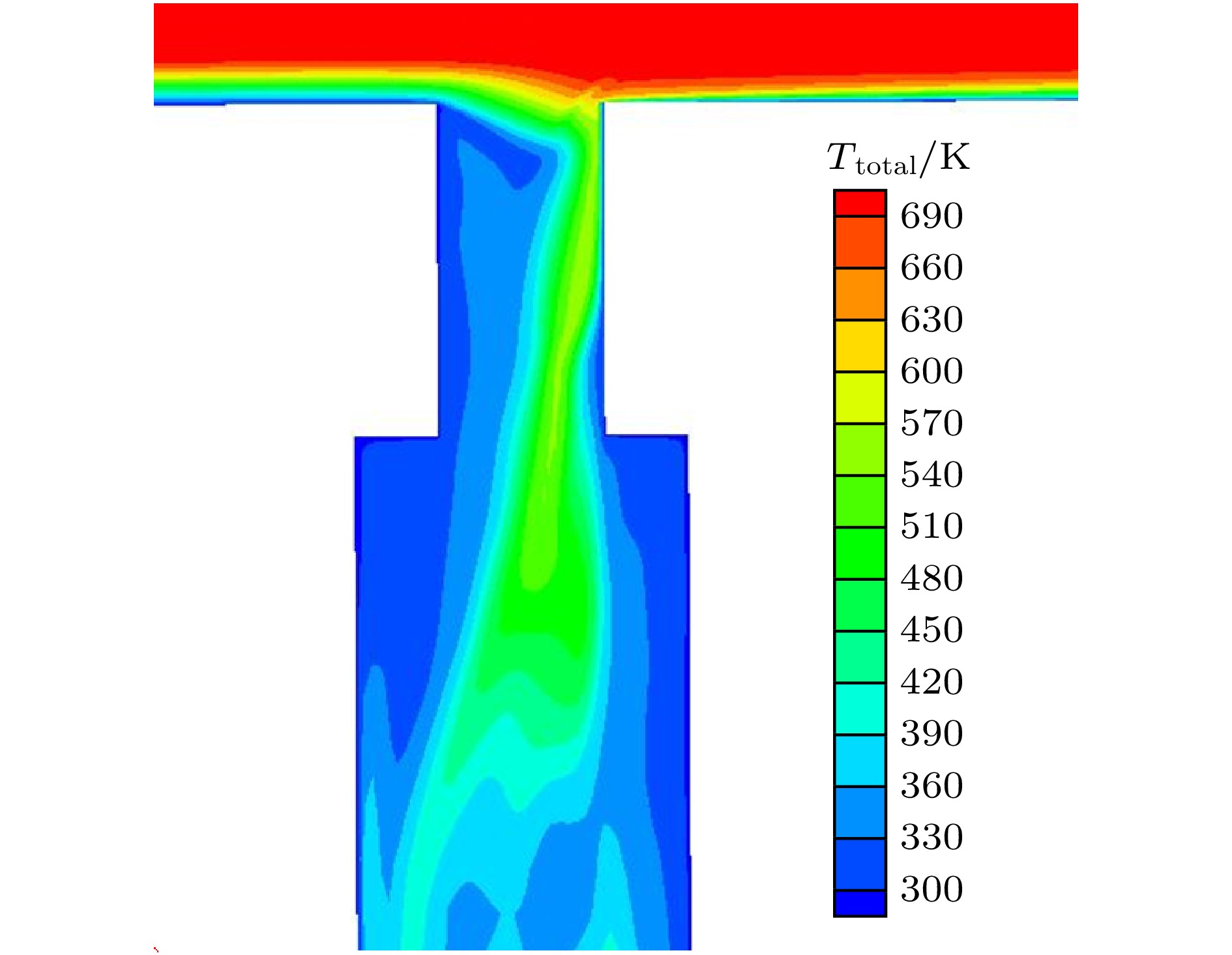
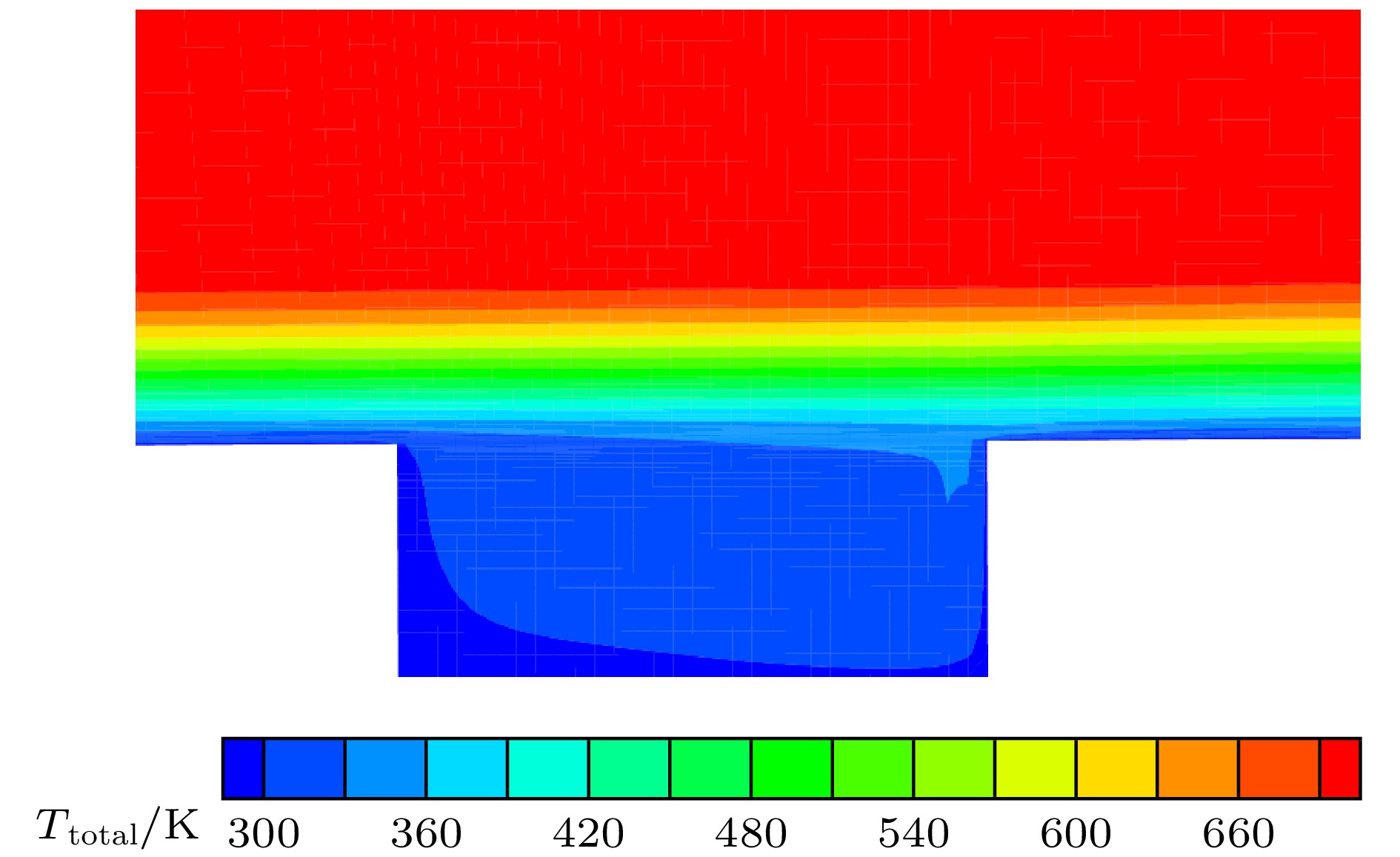
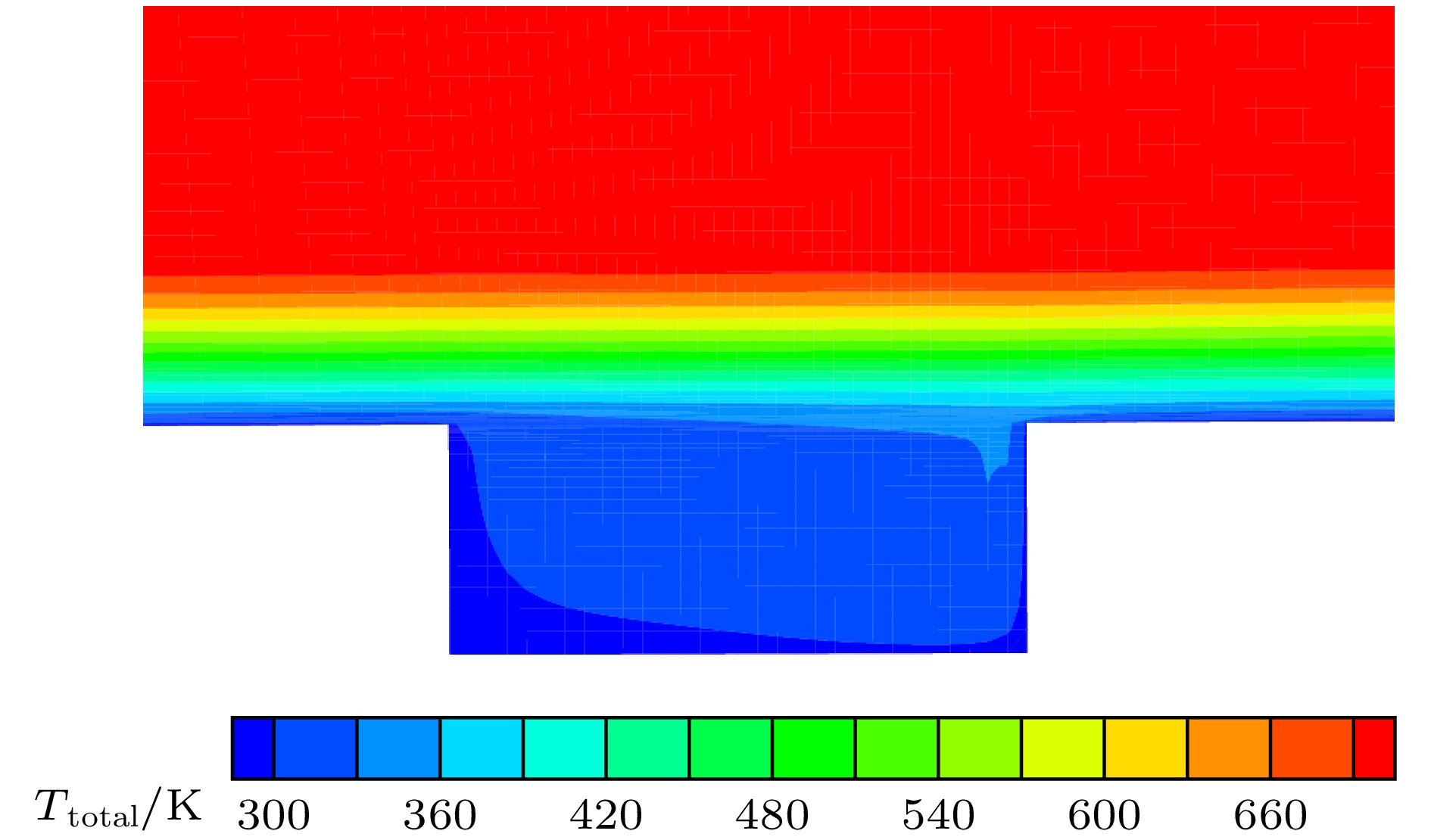
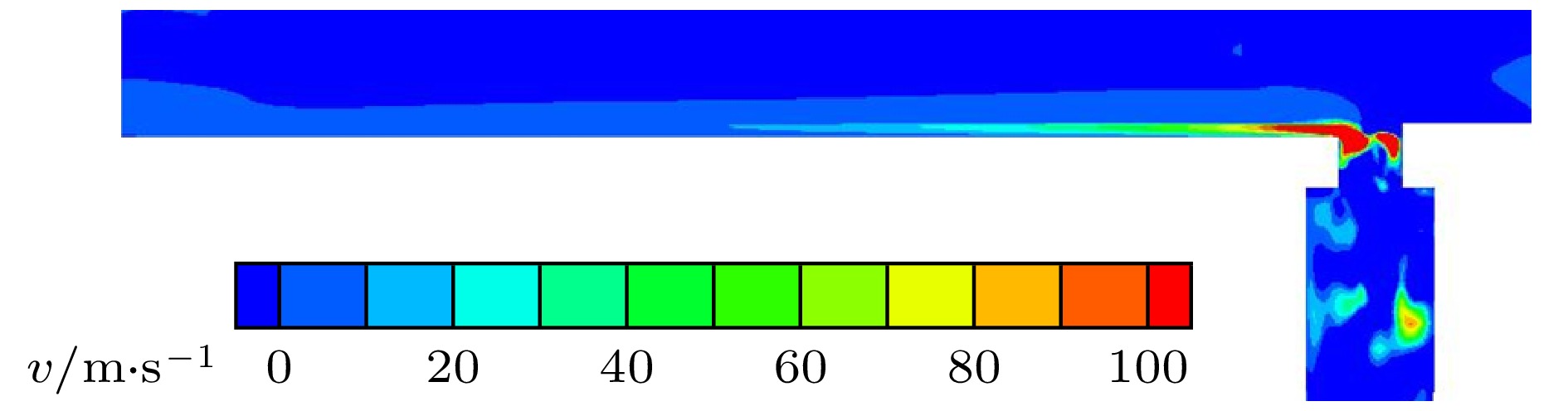
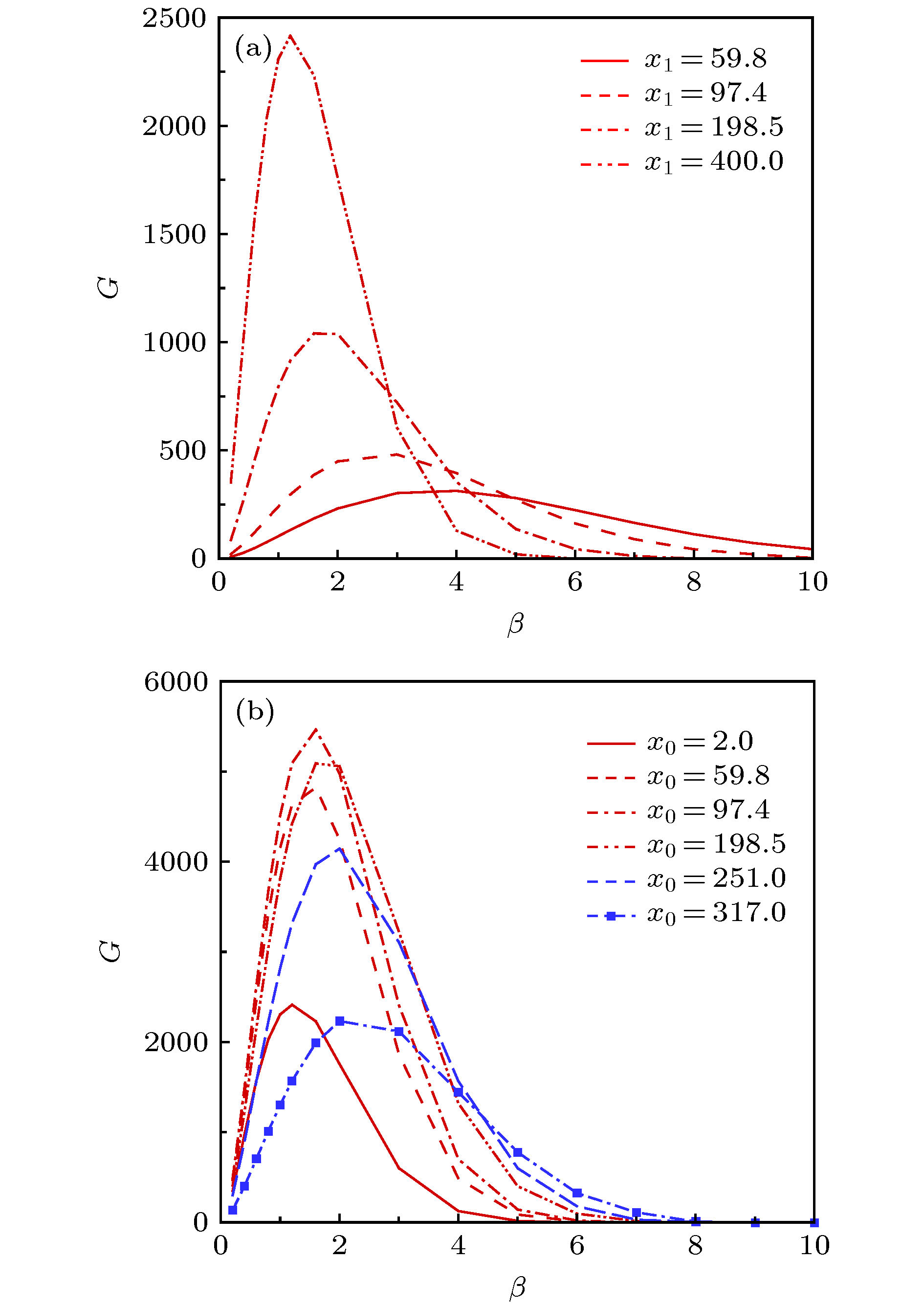
-
Experiments are carried out on the Φ2 m Shock Tunnel (FD-14A) at the China Aerodynamics Research and Development Center to study the effect of the transverse groove with/without discharge hole on the hypersonic blunt flat-plate boundary layer transition, and the preliminary computational and theoretical research are carried out. The inflow Mach number of the test is 6, the unit Reynolds number is 3.3 × 107/m, the leading edge radius of the flat-plate is 1 mm, and the angle of attack is –4°. Three different sets of two-dimensional transverse grooves are arranged at 110 mm away from the leading edge of the flat-plate. The width and depth of the grooves are, respectively, 2.5 mm and 1 mm for groove 1, 3.75 mm and 1.5 mm for groove 2, and 5 mm and 2 mm for groove 3, at the same time, both ends of the groove 1 can open the discharge hole (the discharge hole has a size of 2.5 mm × 5.0 mm and a width the same as that of groove 1). The discharge hole is denoted as the groove 4, and the smooth flat-plate when the groove is not included is denoted as groove 5 or the flat. The Φ2-mm-diameter cylindrical heat flux sensor is used to measure the heat flux distributions of the center line of the flat-plate under different conditions, and thus we can judge the transition of the boundary layer. The measurement results show that the smooth plate starts to transit at x ≈ 340 mm, and the transition is nearly completed at x ≈ 425 mm. The groove causes the transition position of the boundary layer of the plate to advance, and as the width and depth of the groove increase, the promoting effect on the transition is enhanced, and the transition position moves upstream. After the groove 1 is added to the discharge hole (groove 4), the heat flux distribution and the transition position are substantially the same as those of the smooth plate. After the boundary layer flow completely transits into turbulent flow, the difference in heat flux for each case is small, which indicates that the grooves of different specifications affect only the heat flux distribution in the transition process, but have little effect on the heat flux of the turbulent wall after the transition. The computational fluid dynamic results show that the discharge holes cause passive suction, and the test results show that the suction effect of the discharge holes at both ends of the groove counteracts the effect of the groove on the transition of the center line boundary layer, but it may be just a coincidence, and further research is needed. The linear stability theory (LST) and the optimal perturbation method are used to analyze the flow instability mechanism of the smooth blunt plate. The LST results show that there is no first mode instability nor second mode instability in the blunt plate flow. The modal instability mechanism cannot explain the observed transition in the test. The optimal disturbance calculation shows that the blunt plate flow suffers strong non-modal instability, which can qualitatively explain the observed transition phenomenon.
-
Keywords:
- shock tunnel /
- transverse groove /
- discharge hole /
- boundary layer transition
[1] 陈坚强, 涂国华, 张毅锋, 徐国亮, 袁先旭, 陈诚 2017 空气动力学学报 35 311
Google Scholar
Chen J Q, Tu G H, Zhang Y F, Xu G L, Yuan X X, Chen C 2017 Acta Aerodyn. Sin. 35 311
Google Scholar
[2] 杨武兵, 沈清, 朱德华, 禹旻, 刘智勇 2018 空气动力学学报 36 183
Google Scholar
Yang W B, Shen Q, Zhu D H, Yu M, Liu Z Y 2018 Acta Aerodyn. Sin. 36 183
Google Scholar
[3] 刘向宏, 赖光伟, 吴杰 2018 空气动力学学报 36 197
Liu X H, Lai G W, Wu J 2018 Acta Aerodyn. Sin. 36 197
[4] Hahn M 1969 AIAA J. 7 1092
Google Scholar
[5] Soltani S, Hillier R 1994 AIAA Paper 1994−0766
[6] Netterfield M P, Hillier R 1989 AIAA Paper 1989−1842
[7] Nestler D E 1981 AIAA Paper 1981−0335
[8] Everhart J L, Alter S J, Merski N R, Wood W A, Prabhu R K 2006 AIAA Paper 2006−0185
[9] Everhart J L, Greene F A 2009 AIAA Paper 2009−1400
[10] Everhart J L 2008 AIAA Paper 2008−1283
[11] Everhart J L 2011 AIAA Paper 2011−3480
[12] Horvath T J, Berry S A, Merski N R, Berger K T, Buck G M, Liechty D S 2006 AIAA Paper 2006−2919
[13] Ohmichi Y, Suzuki K 2011 AIAA Paper 2011−3966
[14] Xiao L H, Xiao Z X, Duan Z W, Fu S 2015 Int. J. Heat Fluid Flow 51 138
Google Scholar
[15] Beguet S, Perraud J, Forte M, Brazier J P 2017 J. Aircr. 14 794
[16] Fedorov A V, Khokhlov A P 2002 Theor. Comput. Fluid Dyn. 15 231
Google Scholar
[17] 江贤洋, 李存标 2017 实验流体力学 31 1
Google Scholar
Jiang X Y, Li C B 2017 J. Exper. Fluid Mech. 31 1
Google Scholar
[18] Collier F S 1993 AIAA Paper. 1993−2987
[19] 朱自强, 鞠胜军, 吴宗成 2016 航空学报 37 2065
Google Scholar
Zhu Z Q, Ju S J, Wu Z C 2016 Acta Aeronaut. Astronaut. Sin. 37 2065
Google Scholar
[20] 张驰宇, 肖志祥, 邓一菊 2014 中国科学: 物理学 力学 天文学 44 944
Google Scholar
Zhang C Y, Xiao Z X, Deng Y J 2014 Sci. China-Phys. Mech. Astron. 44 944
Google Scholar
[21] 邓双国, 额日其太, 聂俊杰 2011 实验流体力学 25 30
Google Scholar
Deng S G, ERIQITAI, Nie J J 2011 J. Exper. Fluid Mech. 25 30
Google Scholar
[22] 段会申, 刘沛清, 陈建中, 佟增军 2010 空气动力学学报 28 676
Google Scholar
Duan H S, Liu P Q, Chen J Z, Tong Z J 2010 Acta Aerodyn. Sin. 28 676
Google Scholar
[23] Joslin R D 1998 Annu. Rev. Fluid Mech. 30 1
Google Scholar
[24] Denning R M, Allen J E, Armstrong F W 1997 J. Aeronaut. 101 187
[25] Wilson R A L, Jones R I 1996 Aerosp. Eng. 16 21
[26] Macmanus D G, Eaton J A 2000 J. Fluid Mech. 417 47
Google Scholar
[27] Macmanus D G, Eaton J A. 1998 AIAA J. 36 1553
Google Scholar
[28] 赵耕夫 2001 力学学报 33 519
Google Scholar
Zhao G F 2001 Chin. J. Theor. Appl. Mech. 33 519
Google Scholar
[29] Wang X W, Zhong X L 2004 AIAA Paper 2004−254
[30] 李强, 刘济春, 孔荣宗 2017 电子测量与仪器学报 31 623
Google Scholar
Li Q, Liu J Q, Kong R Z 2017 J. of Electr. Measur. Instr. 31 623
Google Scholar
[31] 李强, 江涛, 陈苏宇, 常雨, 赵磊, 张扣立 2019 航空学报 40 122740
Li Q, Jiang T, Chen S Y, Chang Y, Zhao L, Zhang K L 2019 Acta Aeronaut. Astronaut. Sin. 40 122740
[32] 李强, 张扣立, 庄宇, 赵金山 2017 宇航学报 38 758
Li Q, Zhang K L, Zhuang Y, Zhao J S 2017 J. Astronaut. 38 758
[33] Li Q, Nie L, Zhong K L, Li Y, Chen S Y, Zhu G Y 2019 Chin. J. Aeronaut. 32 1215
Google Scholar
[34] Paredes P, Choudhari M M, Li F, Chang C L 2016 AIAA Paper 2016−3050
-
图 1 Φ2 mm柱状热流传感器
Figure 1. The Φ2-mm-diameter cylindrical heat flux sensors.
图 2 平板凹槽测点分布示意图
Figure 2. Schematic diagram of measuring point distribution.
图 3 不同尺寸凹槽对比图
Figure 3. Comparison chart of different size grooves.
图 4 凹槽4泄流孔出口
Figure 4. The discharge hole outlet of Groove 4.
图 5 平板中心线热流测量结果与计算结果
Figure 5. The heat flux measurement and CFD results of the flat centerline.
图 6 泄流孔中心轴线压力分布
Figure 6. Pressure distribution at the center axis of the discharge hole.
图 7 z = 97.5 mm剖面总温分布图
Figure 7. Total temperature distribution of z = 97.5 mm profile
图 8 凹槽1中心对称面总温分布图
Figure 8. Total temperature distribution of the symmetry plane of Groove 1.
图 9 凹槽4中心对称面总温分布图
Figure 9. Total temperature distribution of the symmetry plane of Groove 4.
图 10 x = 110 mm剖面横向速度分布
Figure 10. Transverse velocity distribution of the profile x = 110 mm.
图 11 –4°攻角平板边界层第二模态不稳定区
Figure 11. The unstable zone of Mack second-mode waves for the cases of –4° attack angle.
图 12 固定入口x0 (a)和出口x1 (b)时不同计算域的最优能量增益
Figure 12. Dependence of the optimal energy gain on the computational domain for fixed inlet location (a) and outlet lo-cation (b)
-
[1] 陈坚强, 涂国华, 张毅锋, 徐国亮, 袁先旭, 陈诚 2017 空气动力学学报 35 311
Google Scholar
Chen J Q, Tu G H, Zhang Y F, Xu G L, Yuan X X, Chen C 2017 Acta Aerodyn. Sin. 35 311
Google Scholar
[2] 杨武兵, 沈清, 朱德华, 禹旻, 刘智勇 2018 空气动力学学报 36 183
Google Scholar
Yang W B, Shen Q, Zhu D H, Yu M, Liu Z Y 2018 Acta Aerodyn. Sin. 36 183
Google Scholar
[3] 刘向宏, 赖光伟, 吴杰 2018 空气动力学学报 36 197
Liu X H, Lai G W, Wu J 2018 Acta Aerodyn. Sin. 36 197
[4] Hahn M 1969 AIAA J. 7 1092
Google Scholar
[5] Soltani S, Hillier R 1994 AIAA Paper 1994−0766
[6] Netterfield M P, Hillier R 1989 AIAA Paper 1989−1842
[7] Nestler D E 1981 AIAA Paper 1981−0335
[8] Everhart J L, Alter S J, Merski N R, Wood W A, Prabhu R K 2006 AIAA Paper 2006−0185
[9] Everhart J L, Greene F A 2009 AIAA Paper 2009−1400
[10] Everhart J L 2008 AIAA Paper 2008−1283
[11] Everhart J L 2011 AIAA Paper 2011−3480
[12] Horvath T J, Berry S A, Merski N R, Berger K T, Buck G M, Liechty D S 2006 AIAA Paper 2006−2919
[13] Ohmichi Y, Suzuki K 2011 AIAA Paper 2011−3966
[14] Xiao L H, Xiao Z X, Duan Z W, Fu S 2015 Int. J. Heat Fluid Flow 51 138
Google Scholar
[15] Beguet S, Perraud J, Forte M, Brazier J P 2017 J. Aircr. 14 794
[16] Fedorov A V, Khokhlov A P 2002 Theor. Comput. Fluid Dyn. 15 231
Google Scholar
[17] 江贤洋, 李存标 2017 实验流体力学 31 1
Google Scholar
Jiang X Y, Li C B 2017 J. Exper. Fluid Mech. 31 1
Google Scholar
[18] Collier F S 1993 AIAA Paper. 1993−2987
[19] 朱自强, 鞠胜军, 吴宗成 2016 航空学报 37 2065
Google Scholar
Zhu Z Q, Ju S J, Wu Z C 2016 Acta Aeronaut. Astronaut. Sin. 37 2065
Google Scholar
[20] 张驰宇, 肖志祥, 邓一菊 2014 中国科学: 物理学 力学 天文学 44 944
Google Scholar
Zhang C Y, Xiao Z X, Deng Y J 2014 Sci. China-Phys. Mech. Astron. 44 944
Google Scholar
[21] 邓双国, 额日其太, 聂俊杰 2011 实验流体力学 25 30
Google Scholar
Deng S G, ERIQITAI, Nie J J 2011 J. Exper. Fluid Mech. 25 30
Google Scholar
[22] 段会申, 刘沛清, 陈建中, 佟增军 2010 空气动力学学报 28 676
Google Scholar
Duan H S, Liu P Q, Chen J Z, Tong Z J 2010 Acta Aerodyn. Sin. 28 676
Google Scholar
[23] Joslin R D 1998 Annu. Rev. Fluid Mech. 30 1
Google Scholar
[24] Denning R M, Allen J E, Armstrong F W 1997 J. Aeronaut. 101 187
[25] Wilson R A L, Jones R I 1996 Aerosp. Eng. 16 21
[26] Macmanus D G, Eaton J A 2000 J. Fluid Mech. 417 47
Google Scholar
[27] Macmanus D G, Eaton J A. 1998 AIAA J. 36 1553
Google Scholar
[28] 赵耕夫 2001 力学学报 33 519
Google Scholar
Zhao G F 2001 Chin. J. Theor. Appl. Mech. 33 519
Google Scholar
[29] Wang X W, Zhong X L 2004 AIAA Paper 2004−254
[30] 李强, 刘济春, 孔荣宗 2017 电子测量与仪器学报 31 623
Google Scholar
Li Q, Liu J Q, Kong R Z 2017 J. of Electr. Measur. Instr. 31 623
Google Scholar
[31] 李强, 江涛, 陈苏宇, 常雨, 赵磊, 张扣立 2019 航空学报 40 122740
Li Q, Jiang T, Chen S Y, Chang Y, Zhao L, Zhang K L 2019 Acta Aeronaut. Astronaut. Sin. 40 122740
[32] 李强, 张扣立, 庄宇, 赵金山 2017 宇航学报 38 758
Li Q, Zhang K L, Zhuang Y, Zhao J S 2017 J. Astronaut. 38 758
[33] Li Q, Nie L, Zhong K L, Li Y, Chen S Y, Zhu G Y 2019 Chin. J. Aeronaut. 32 1215
Google Scholar
[34] Paredes P, Choudhari M M, Li F, Chang C L 2016 AIAA Paper 2016−3050
DownLoad:
Catalog
Metrics
- Abstract views: 7875
- PDF Downloads: 76
- Cited By: 0
