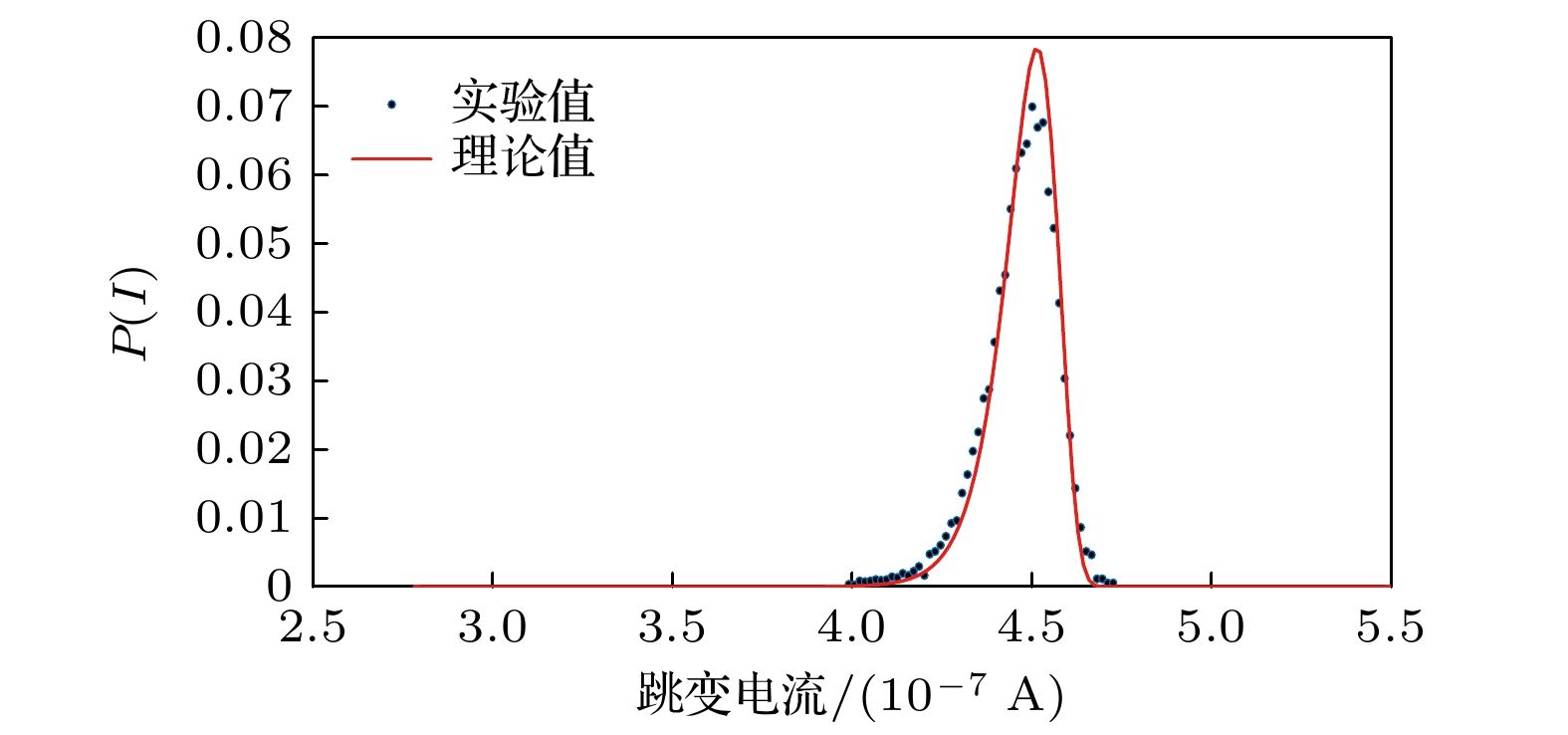
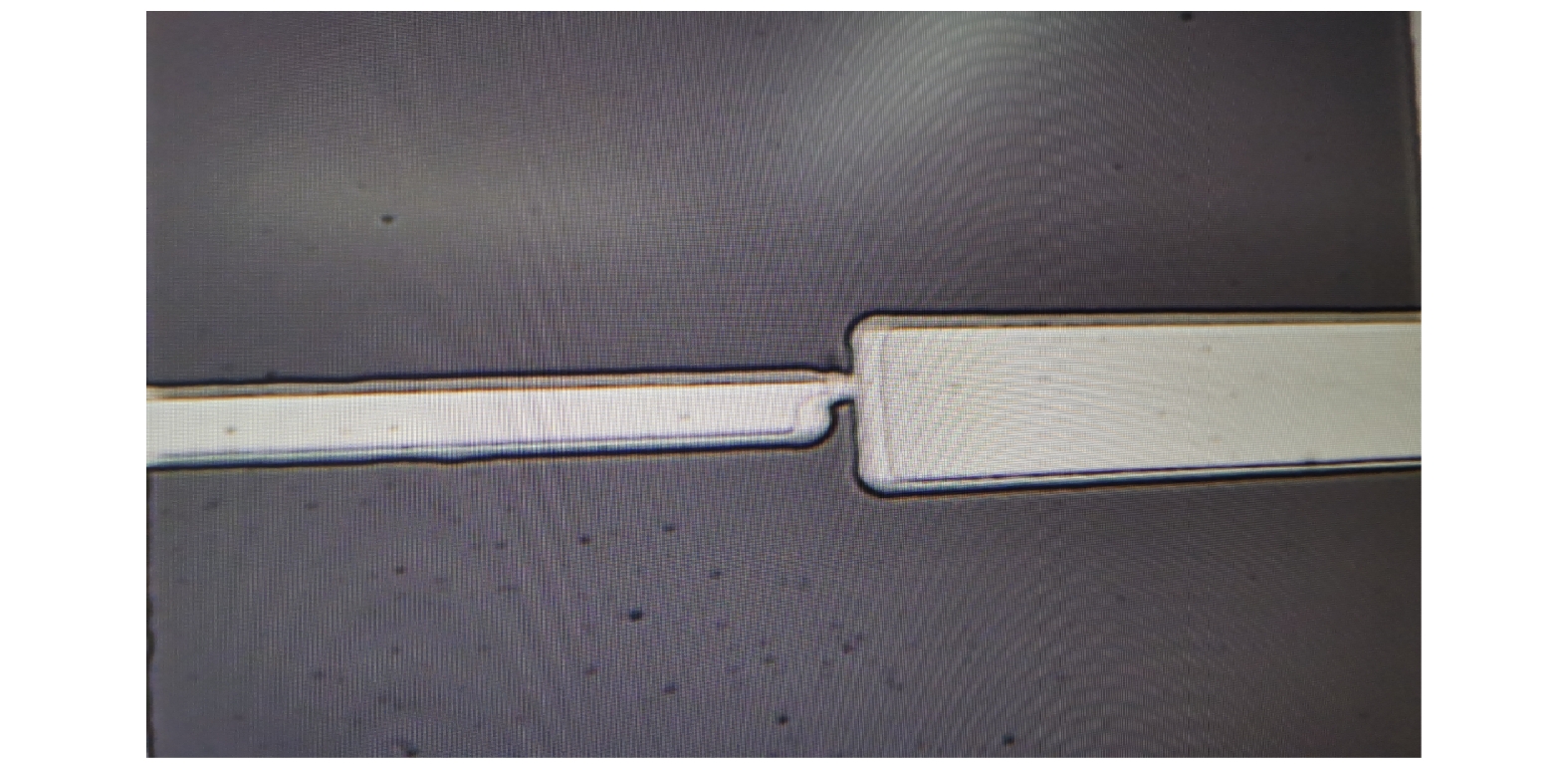
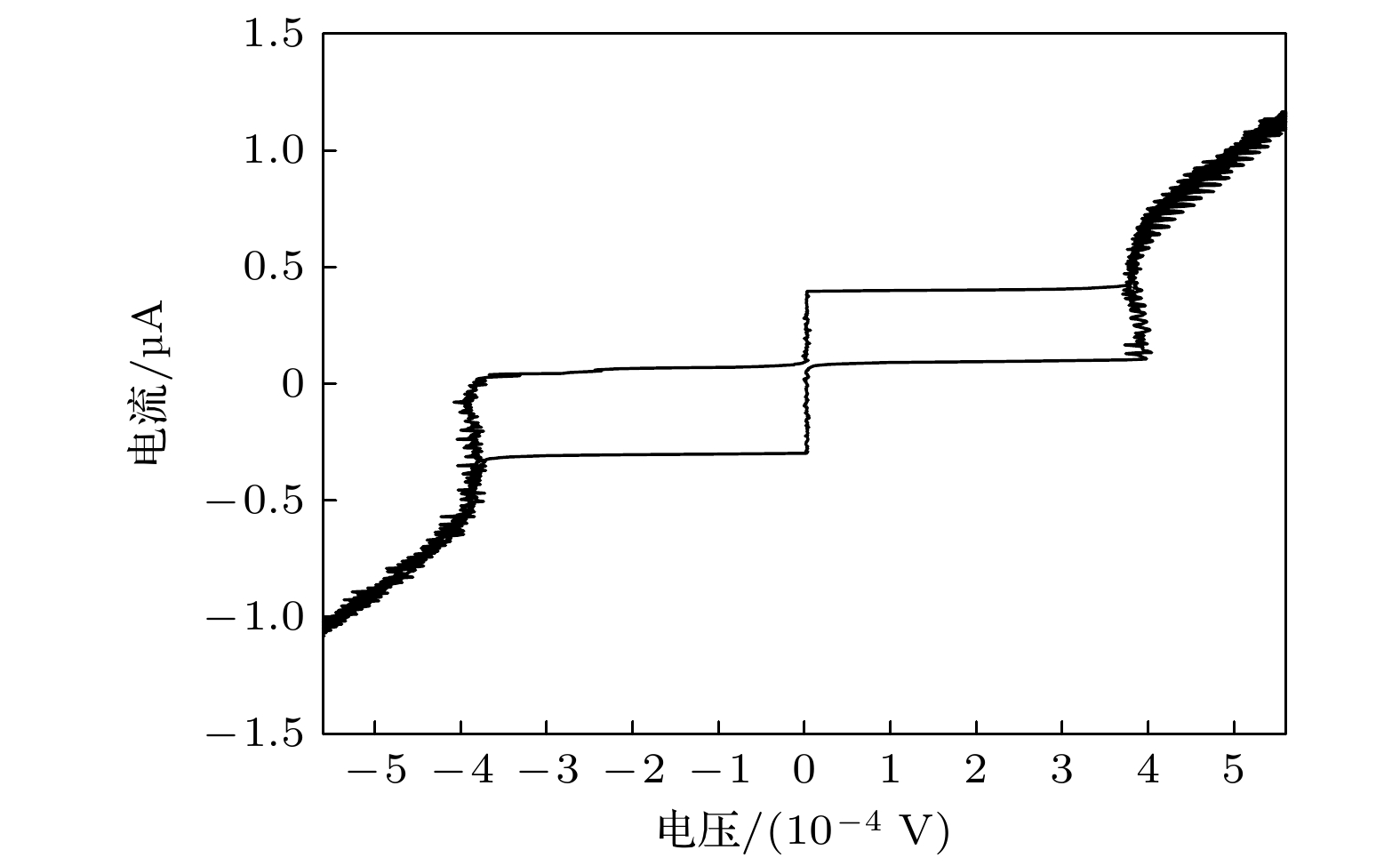
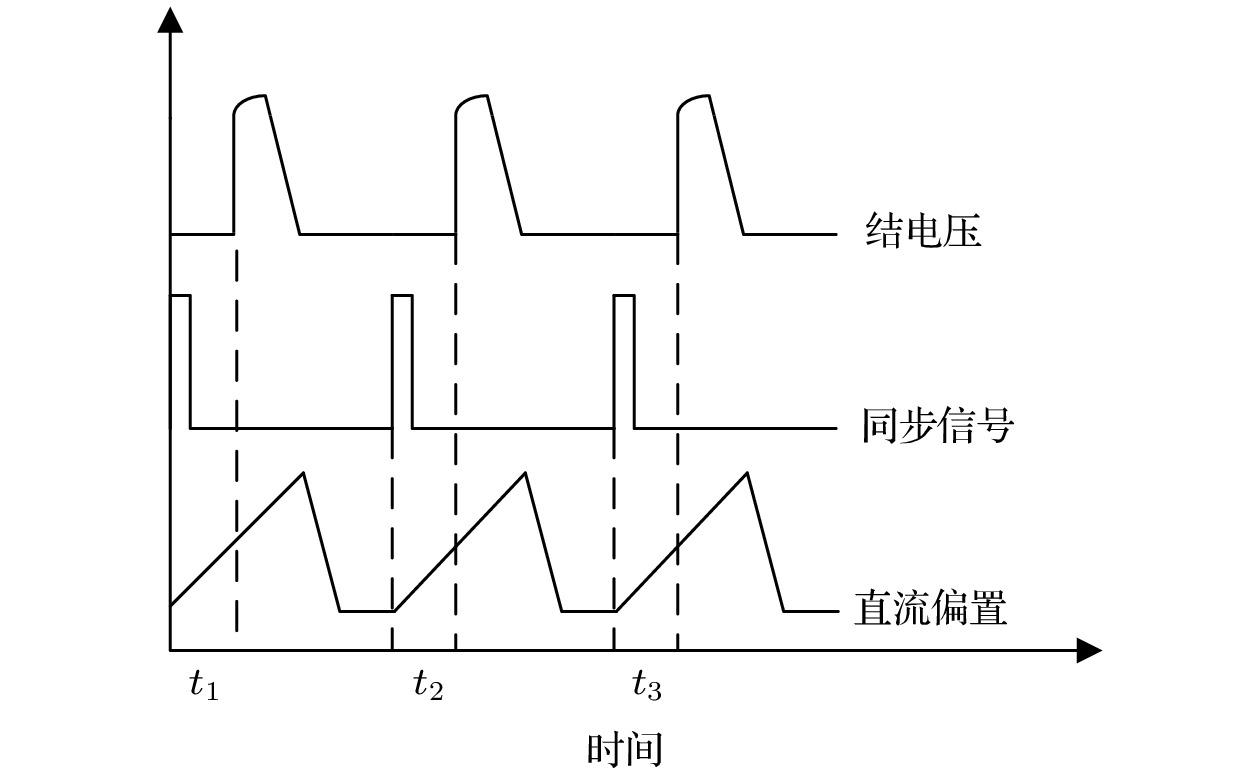
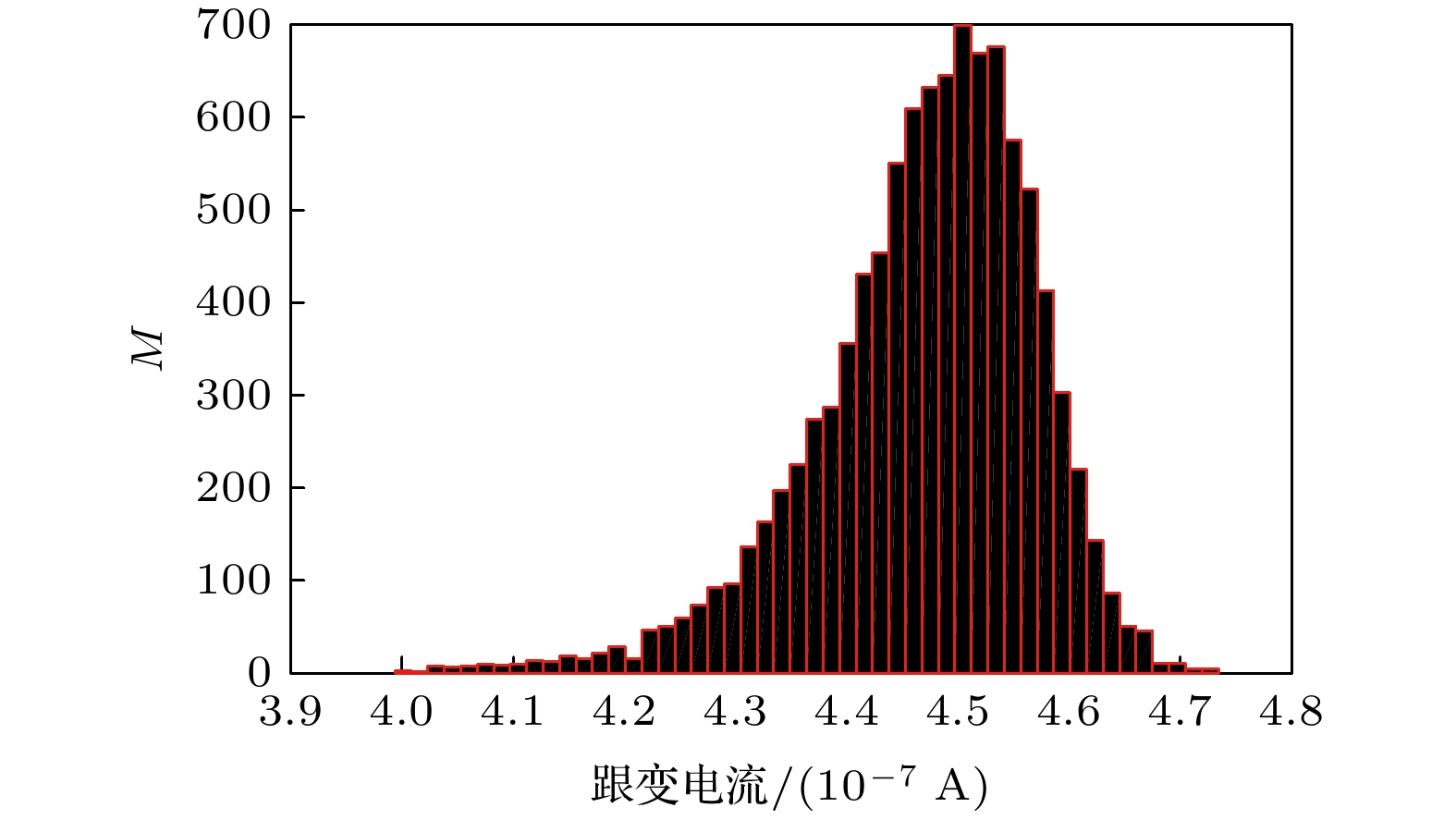
-
Superconducting Josephson junctions are the key devices for superconducting quantum computation and microwave single photon detection. It is important to fabricate the Josephson junctions with designable parameters. Different from the typical methods to calibrate the parameters of the Josephson junctions,, e.g., by using the microwave drivings and measuring the ratio of hysteresis current to critical one, in this paper we achieve the calibrations with the low frequency current biases. First, we measure the I-V characteristic curves of the fabricated Al/AlOx/Al junctions. Second, we measure the statistical distributions of the jump currents of the Josephson junction samples driven by the low frequency (@71.3 Hz) biased currents at an extremely low temperature of 50 mK. These two sets of experimental data are utilized to estimate the typical parameters of the Josephson junction, i.e., junction capacitance, critical current, and the damping coefficient, which are difficult to be directly measured in the usual experiments. The critical current and capacitance of the Josephson junction are estimated by fitting the statistical distribution of the measured jump currents with the relevant theoretical model of the "particle" escape from the potential driven by the thermal excitations and quantum tunnelings. With the calibrated critical current of the junction, the relation between
$I/I_{\rm{c}}$ and${\rm{d}}\varphi/{\rm{d}}\tau,\,\tau=\omega_{\rm{c}}t$ (with$\omega_{\rm{c}}$ being the plasmon frequency) is obtained from the measured$I\text-V$ curve. Using the standard resistively capacitance shunted junction model to fit such a relation, the damping coefficient of the junction can be estimated. With the estimated critical current, capacitance, and damping coefficient, the resistance$R_n$ of the junction at the working temperature is calibrated consequently. It is shown that our estimated results are in good agreement with that predicted by the famous Ambgaokar-Baratoff formula. Physically, the method demonstrated here possesses two advantages. First, it is relatively insensitive to the noise during the measurement of the junction's I-V characteristic curve, compared with the usual method to calibrate damping coefficient by measuring the ratio of hysteresis current to critical current. Second, only the low frequency driving is required to measure the jump current of the junction for estimating the damping coefficient. The microwave driving is not necessary. Hopefully, the present work is useful for the on-demand designs of the Josephson junctions for various applications.-
Keywords:
- Josephson junction /
- resistively capacitance shunted junction model /
- jump current /
- parameter estimation
[1] Barends R, Kelly J, Megrant A, Veitia A, Sank D, Jeffrey E, White T C, Mutus J, Fowler A G, Campbell B, Chen Y, Chen Z, Chiaro B, Dunsworth A, Neill C, Malley P O, Roushan P, Vainsencher A, Wenner J, Korotkov A N, Cleland A N, Martinis J M 2014 Nature 508 500
Google Scholar
[2] 李春光, 王佳, 吴云, 王旭, 孙亮, 董慧, 高波, 李浩, 尤立星, 林志荣, 任浩, 李婧, 张文, 贺青, 王轶文, 韦联福, 孙汉聪, 王华兵, 李劲劲, 屈继峰 2021 70 018501
Google Scholar
Li C G, Wang J, Wu Y, Wang X, Sun L, Dong H, Gao B, Li H, You L X, Lin Z R, Ren H, Li J, Zhang W, He Q, Wang Y W, Wei L F, Sun H C, Wang H B, Li J J, Qu J F 2021 Acta Phys. Sin. 70 018501
Google Scholar
[3] Arute F, Arya K, Babbush R, Bacon D, Bardin J, Barends R, Biswas R, Boixo S, Brandao F, Buell D, Burkett B, Chen Y, Chen Z J, Chiaro B, Collins R, Courtney W, Dunsworth A, Farhi E, Foxen B, Fowler A, Gidney C, Giustina M, Graff B, Guerin K, Habegger S, Harrigan M, Hartmann M, Ho A, Hoffmann M, Huang T, Humble T, Isakov S, Jeffrey E, Zhang J, Kafri D, Kechedzhi K, Kelly J, Klimov P, Knysh S, Korotkov A, Kostritsa F, Landhuis D, Lindmark M, Lucero E, Lyakh D, Mandrà S, McClean J, McEwen M, Megrant A, Mi X, Michielsen K, Mohseni M, Mutus J, Naaman O, Neeley M, Neill C, Niu M Y, Ostby E, Petukhov A, Platt J, Quintana C, Rieffel E, Roushan P, Rubin N, Sank D, Satzinger K, Smelyanskiy V, Sung K, Trevithick M, Vainsencher A, Villalonga B, Yao T J, Yeh P, Zalcman A, Neven H, Martinis J 2019 Nature 574 505
Google Scholar
[4] Zhong H S, Wang H, Deng Y H, Chen M C, Peng L C, Luo Y H, Qin J, Wu D, Ding X, Hu Y, Hu P, Yang X Y, Zhang W J, Li H, Li Y X, Jiang X, Gan L, Yang G W, You L X, Wang Z, Li L, Liu N L, Lu C Y, Pan J W 2020 Science 370 1460
[5] Sathyamoorthy S R, Stace T M, Johansson G 2016 C. R. Phys. 17 756
Google Scholar
[6] Chen Y F, Hover D, Sendelbach S, Maurer L, Merkel S T, Pritchett E J, Wilhelm F K, McDermott R 2011 Phys. Rev. Lett. 370 1460
[7] 张裕恒, 李玉芝 2009 超导物理 (卷3) (合肥: 中国科学技术大学出版社) 第342−484页
Zhang Y H, Li Y Z 2009 Superconductor Physics (Vol. 3) (Hefei: China University of science and Technology Press) pp342−484 (in Chinese)
[8] 郑东宁 2021 70 018502
Zheng D L 2021 Acta Phys. Sin. 70 018502
[9] Clarke J, Wilhelm F K 2008 Nature 453 1031
Google Scholar
[10] Sun G Z, Wang Y W, Cao J Y, Chen J, Ji Z M, Kang L, Xu W W, Yu Y, Han S Y, Wu P H 2008 Phys. Rev. B 77 104531
Google Scholar
[11] Stewart W C 1968 Appl. Phys. Lett. 12 277
Google Scholar
[12] Makhlin Y, Sch?n G, Shnirman A 2001 Rev. Mod. Phys. 73 357
Google Scholar
[13] Stoutimore M J A, Rossolenko A N, Bolginov V V, Oboznov V A, Rusanov A Y, Baranov D S, Pugach N, Frolov S M, Ryazanov V V, Van Harlingen D J 2018 Phys. Rev. Lett. 121 17
[14] 崔大健, 林德华, 于海峰, 彭智慧, 朱晓波, 郑东宁, 景秀年, 吕力, 赵士平 2008 09 5933
Google Scholar
Cui D J, Lin D H, Yu H F, Peng Z H, Zhu X B, Zheng D N, Jing X N, Lu L, Zhao S P 2008 Acta Phys. Sin. 09 5933
Google Scholar
[15] Kramers H A 1940 Physica 7 284
Google Scholar
[16] Caldeira A O, Leggett A J 1981 Phys. Rev. Lett. 46 211
Google Scholar
[17] Trees B R, Saranathan V, Stroud D 2005 Phys. Rev. E 71 016215
Google Scholar
[18] 陈伟 2019 博士学位论文 (南京: 南京大学)
Chen W 2019 Ph. D. Dissertation (Nanjing: Nanjing University) (in Chinese)
[19] 曹俊宇, 孙国柱, 王轶文, 陈健, 吴培亨 2007 低温 03 196
Cao J Y, Sun G Z, Wang Y W, Chen J, Wu P H 2007 Chin. J. Low Temp. 2007 03 196 (in Chinese)
[20] Ambegaokar V, Baratoff A 1963 Phys. Rev. Lett. 10 486
Google Scholar
-
图 1 电流偏置下的约瑟夫森结势能曲线
Figure 1. Potential of a current-biased Josephson junction.
图 2 约瑟夫森结制备流程图
Figure 2. Preparation process of a Josephson junction.
图 3 约瑟夫森结样品
Figure 3. Josephson junction sample.
图 4 约瑟夫森结I-V特性曲线
Figure 4. Measured I-V characteristic curve of the fabricated Josephson junction.
图 5 约瑟夫森结跳变电流实验测量的时序图
Figure 5. Time sequence diagram for the junction jump current measurements.
图 6 约瑟夫森结跳变电流及其次数统计
Figure 6. Josephson junction jump currents and their statistical distributions.
图 7 结跳变电流的归一化统计分布: 理论拟合(红实线)与实验数据(点状线)
Figure 7. Statistical distributions of the junction jump currents: theoretical simulations (red soild line), and measurement data (dot line).
图 8 约瑟夫森结的等效I-V曲线
Figure 8. Effective I-V curve of the measured Josephson junction
图 9 斜率K随阻尼参数变化
$\beta_{\rm c}$ 关系Figure 9. Relationship between the parameters
$\beta_{\rm c}$ and K.表 1 不同参数取值对实验数据拟合的偏差度分析
Table 1. Deviations from the data simulated by using the different theoretical parameters.
$I_{\rm c}/(10^{-7}{\rm A})$ C/fF 峰值位置
偏差(误差/
实验值)峰值高度
偏差(误差/
实验值)半高宽偏差
(误差/实验值)$ 5.56 $ $ 23.3 $ 0.06% 9.75% 3.52% $ 5.57 $ $ 23.3 $ 0.27% 9.75% 3.52% $ 5.55 $ $ 23.3 $ 0.15% 9.75% 3.52% $ 5.56 $ $ 23.4 $ 0.17% 9.74% 3.53% $ 5.56 $ $ 23.2 $ 0.31% 9.76% 3.52% 
DownLoad: CSV
-
[1] Barends R, Kelly J, Megrant A, Veitia A, Sank D, Jeffrey E, White T C, Mutus J, Fowler A G, Campbell B, Chen Y, Chen Z, Chiaro B, Dunsworth A, Neill C, Malley P O, Roushan P, Vainsencher A, Wenner J, Korotkov A N, Cleland A N, Martinis J M 2014 Nature 508 500
Google Scholar
[2] 李春光, 王佳, 吴云, 王旭, 孙亮, 董慧, 高波, 李浩, 尤立星, 林志荣, 任浩, 李婧, 张文, 贺青, 王轶文, 韦联福, 孙汉聪, 王华兵, 李劲劲, 屈继峰 2021 70 018501
Google Scholar
Li C G, Wang J, Wu Y, Wang X, Sun L, Dong H, Gao B, Li H, You L X, Lin Z R, Ren H, Li J, Zhang W, He Q, Wang Y W, Wei L F, Sun H C, Wang H B, Li J J, Qu J F 2021 Acta Phys. Sin. 70 018501
Google Scholar
[3] Arute F, Arya K, Babbush R, Bacon D, Bardin J, Barends R, Biswas R, Boixo S, Brandao F, Buell D, Burkett B, Chen Y, Chen Z J, Chiaro B, Collins R, Courtney W, Dunsworth A, Farhi E, Foxen B, Fowler A, Gidney C, Giustina M, Graff B, Guerin K, Habegger S, Harrigan M, Hartmann M, Ho A, Hoffmann M, Huang T, Humble T, Isakov S, Jeffrey E, Zhang J, Kafri D, Kechedzhi K, Kelly J, Klimov P, Knysh S, Korotkov A, Kostritsa F, Landhuis D, Lindmark M, Lucero E, Lyakh D, Mandrà S, McClean J, McEwen M, Megrant A, Mi X, Michielsen K, Mohseni M, Mutus J, Naaman O, Neeley M, Neill C, Niu M Y, Ostby E, Petukhov A, Platt J, Quintana C, Rieffel E, Roushan P, Rubin N, Sank D, Satzinger K, Smelyanskiy V, Sung K, Trevithick M, Vainsencher A, Villalonga B, Yao T J, Yeh P, Zalcman A, Neven H, Martinis J 2019 Nature 574 505
Google Scholar
[4] Zhong H S, Wang H, Deng Y H, Chen M C, Peng L C, Luo Y H, Qin J, Wu D, Ding X, Hu Y, Hu P, Yang X Y, Zhang W J, Li H, Li Y X, Jiang X, Gan L, Yang G W, You L X, Wang Z, Li L, Liu N L, Lu C Y, Pan J W 2020 Science 370 1460
[5] Sathyamoorthy S R, Stace T M, Johansson G 2016 C. R. Phys. 17 756
Google Scholar
[6] Chen Y F, Hover D, Sendelbach S, Maurer L, Merkel S T, Pritchett E J, Wilhelm F K, McDermott R 2011 Phys. Rev. Lett. 370 1460
[7] 张裕恒, 李玉芝 2009 超导物理 (卷3) (合肥: 中国科学技术大学出版社) 第342−484页
Zhang Y H, Li Y Z 2009 Superconductor Physics (Vol. 3) (Hefei: China University of science and Technology Press) pp342−484 (in Chinese)
[8] 郑东宁 2021 70 018502
Zheng D L 2021 Acta Phys. Sin. 70 018502
[9] Clarke J, Wilhelm F K 2008 Nature 453 1031
Google Scholar
[10] Sun G Z, Wang Y W, Cao J Y, Chen J, Ji Z M, Kang L, Xu W W, Yu Y, Han S Y, Wu P H 2008 Phys. Rev. B 77 104531
Google Scholar
[11] Stewart W C 1968 Appl. Phys. Lett. 12 277
Google Scholar
[12] Makhlin Y, Sch?n G, Shnirman A 2001 Rev. Mod. Phys. 73 357
Google Scholar
[13] Stoutimore M J A, Rossolenko A N, Bolginov V V, Oboznov V A, Rusanov A Y, Baranov D S, Pugach N, Frolov S M, Ryazanov V V, Van Harlingen D J 2018 Phys. Rev. Lett. 121 17
[14] 崔大健, 林德华, 于海峰, 彭智慧, 朱晓波, 郑东宁, 景秀年, 吕力, 赵士平 2008 09 5933
Google Scholar
Cui D J, Lin D H, Yu H F, Peng Z H, Zhu X B, Zheng D N, Jing X N, Lu L, Zhao S P 2008 Acta Phys. Sin. 09 5933
Google Scholar
[15] Kramers H A 1940 Physica 7 284
Google Scholar
[16] Caldeira A O, Leggett A J 1981 Phys. Rev. Lett. 46 211
Google Scholar
[17] Trees B R, Saranathan V, Stroud D 2005 Phys. Rev. E 71 016215
Google Scholar
[18] 陈伟 2019 博士学位论文 (南京: 南京大学)
Chen W 2019 Ph. D. Dissertation (Nanjing: Nanjing University) (in Chinese)
[19] 曹俊宇, 孙国柱, 王轶文, 陈健, 吴培亨 2007 低温 03 196
Cao J Y, Sun G Z, Wang Y W, Chen J, Wu P H 2007 Chin. J. Low Temp. 2007 03 196 (in Chinese)
[20] Ambegaokar V, Baratoff A 1963 Phys. Rev. Lett. 10 486
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 6853
- PDF Downloads: 244
- Cited By: 0
