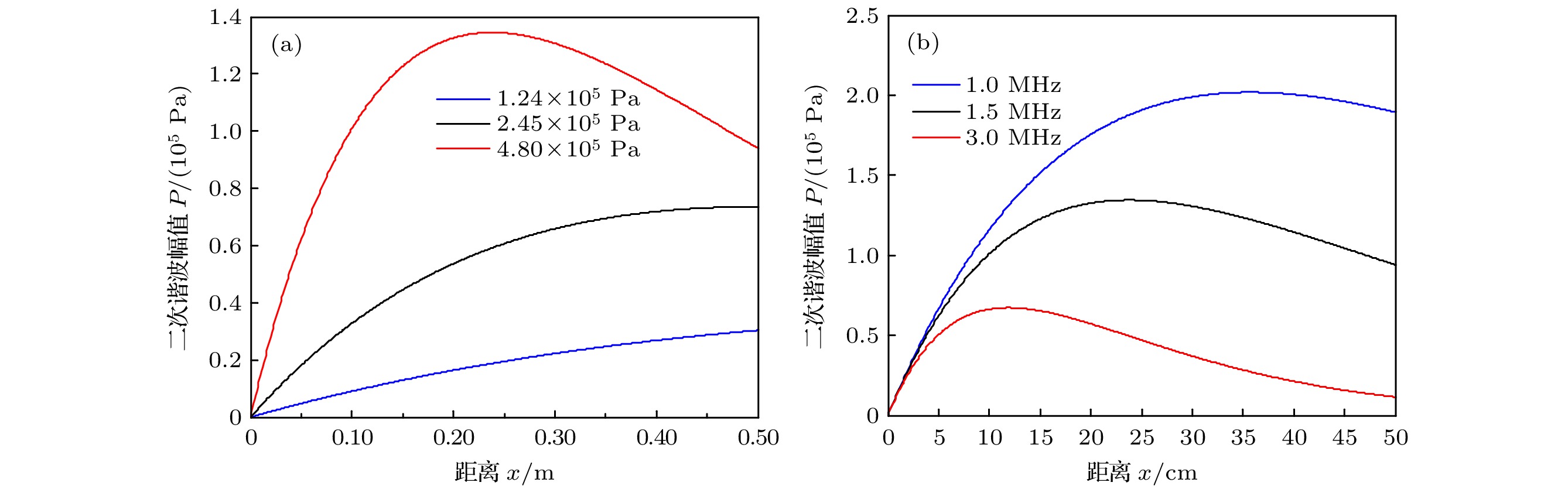
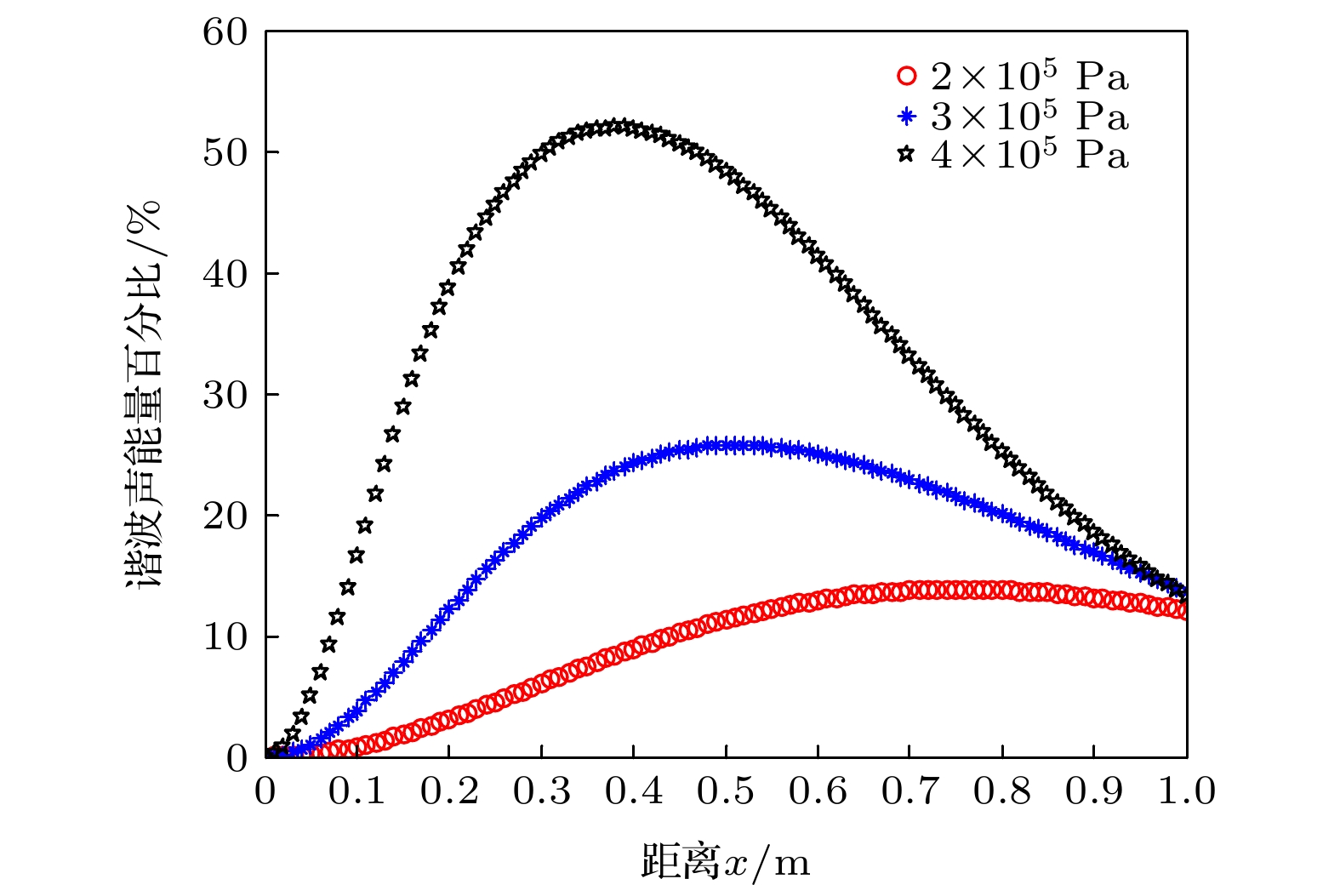
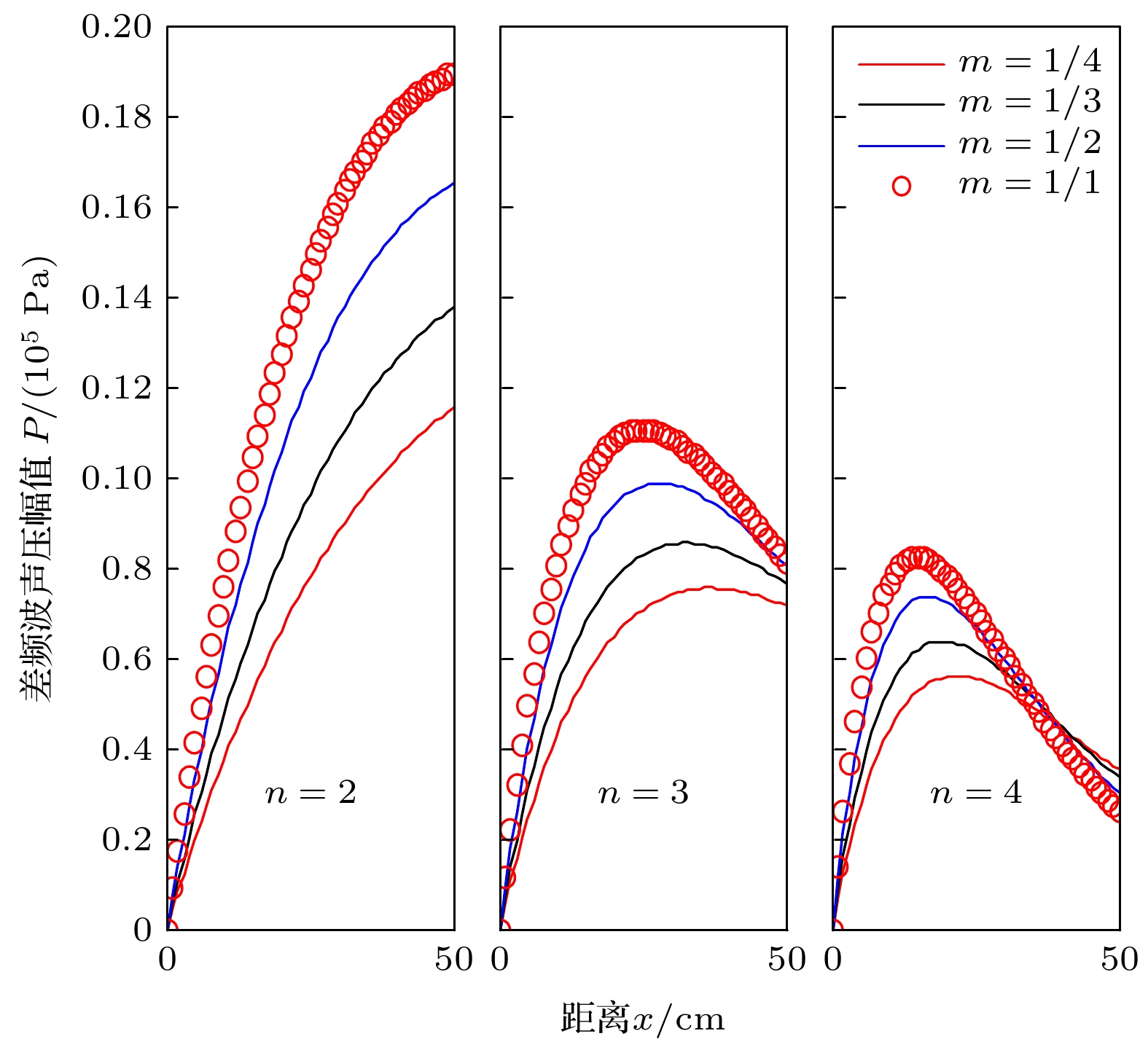
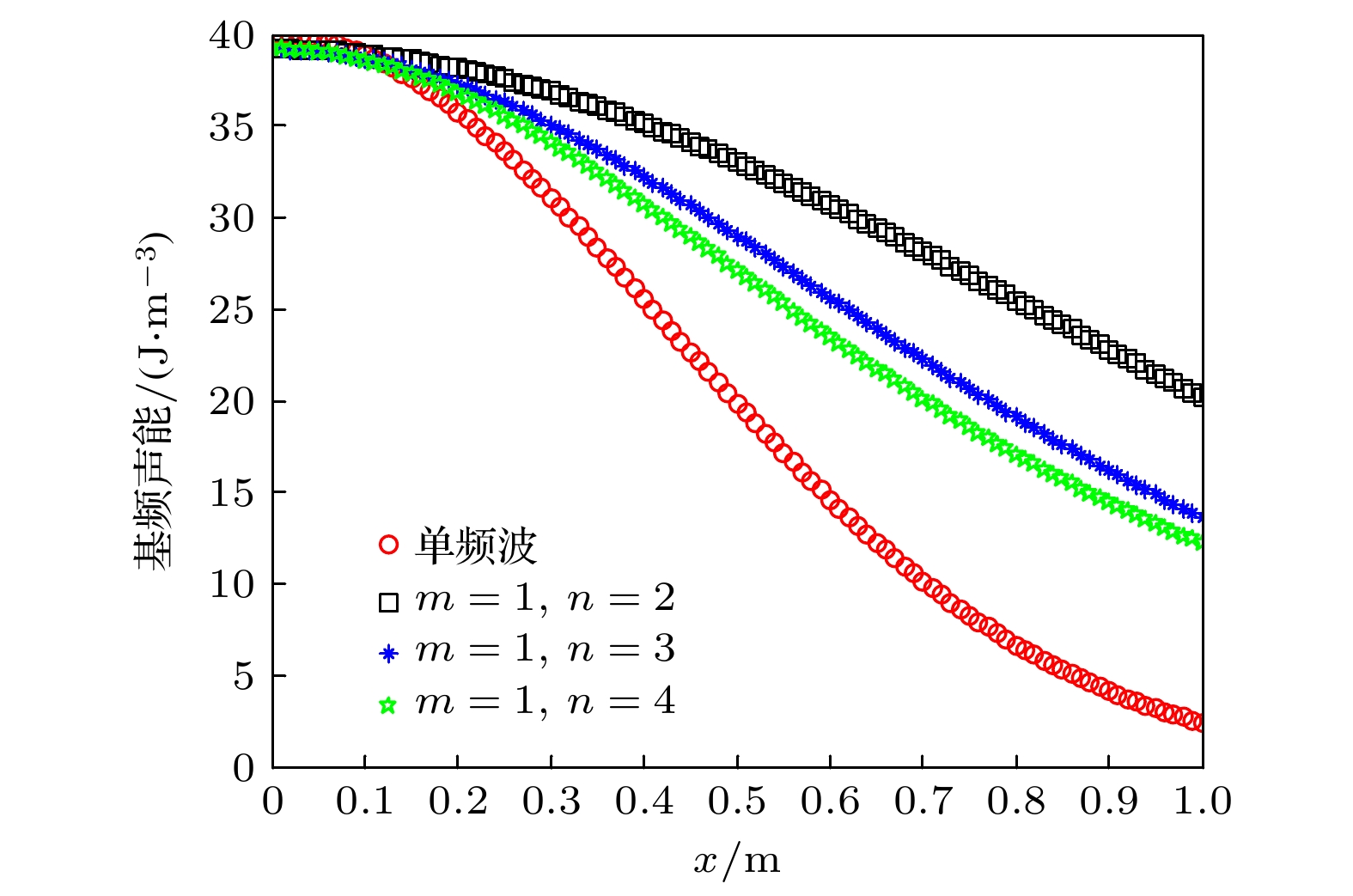
-
The formula for the nonlinear propagation of harmonics is obtained by using the generalized Navier-Stokes equations and the modified equations of state, considering the presence of heat transfer and fluid viscidity. The quantitative relationship among the harmonic pressure, initial sound pressure amplitude, frequency and the media property is obtained by approximately solving the single-frequency acoustic equation. In this paper, the hamonics’ distributions and propagations in the radiation field of single- and double-frequency sound source with different driving pressures and frequencies are discussed. It is found that new harmonics constantly appear in the sound field, and each-order harmonic of excitation gradually increases and then weakens with the increase of distance. The amplitude of harmonic pressure increases with the increase of the driving acoustic pressure near the sound source, but decreases with the increase of the frequency. Compared with the single-frequency field, the dual-frequency field has a large propagation distance, a very uniform acoustic energy distribution, and a large harmonic content in the far-field when the input total sound energy is constant. The physical mechanism is that the higher driving frequency causes a faster acoustic loss, a slower harmonic accumulation, and a smaller sound propagation distance. The higher driving pressure causes the much fundamental sound energy to be transferred, the more harmonics to be generated, the fundamental wave to be attenuated faster, and the negative effect of sound pressure on far-field sound energy to be increased. Through the analysis, it is found that the multi-frequency sound source can increase the propagation distance of sound, and improve the uniformity of sound energy distribution.
-
Keywords:
- nonlinear effect /
- wave equations /
- acoustic parametrics
[1] Westervelt P J 1957 J. Acoust. Soc. Am. 29 199
Google Scholar
[2] Westervelt P J 1957 J. Acoust. Soc. Am. 29 934
Google Scholar
[3] Ingard U, Pridmo-Brown D C 1955 J. Acoust. Soc. Am. 27 1002
[4] Ingard U, Pridmo-Brown D C 1956 J. Acoust. Soc. Am. 28 367
Google Scholar
[5] 钱祖文 1981 30 1479
Google Scholar
Qian Z W 1981 Acta Phys. Sin. 30 1479
Google Scholar
[6] 钱祖文 1981 30 1559
Google Scholar
Qian Z W 1981 Acta Phys. Sin. 30 1559
Google Scholar
[7] 钱祖文 1988 37 221
Google Scholar
Qian Z W 1988 Acta Phys. Sin. 37 221
Google Scholar
[8] Westervelt P J 1963 J. Acoust. Soc. Am. 35 535
Google Scholar
[9] 钱祖文 1976 25 472
Google Scholar
Qian Z W 1976 Acta Phys. Sin. 25 472
Google Scholar
[10] 钱祖文 1999 物理 28 593
Google Scholar
Qian Z W 1999 J. Phys. 28 593
Google Scholar
[11] Wang X, Chen W Z, Liang S D, Zhao T Y, Liang J F 2017 Phys. Rev. E 95 033118
Google Scholar
[12] Wang X, Chen W Z, Yang J, Liang S D 2018 J. Appl. Phys. 123 214904
Google Scholar
[13] 陈伟中 2018 应用声学 37 675
Google Scholar
Chen W Z 2018 Appl. Acoust. 37 675
Google Scholar
[14] Ashokumar M 2011 Ultrason. Sonochem. 18 864
Google Scholar
[15] Wijngaarden L V 1972 Ann. Rev. Fluid Mech. 4 369
Google Scholar
[16] Commander K W, Prosperetti A 1989 J. Acoust. Soc. Am. 85 732
Google Scholar
[17] Vanhille C, Cleofé C P 2011 Ultrason. Sonochem. 18 679
Google Scholar
[18] Thiessen R J, Cheviakov A F 2019 Commun. Nonliear Sci. Numer. Simul. 73 244
Google Scholar
[19] Zhang H H 2020 J. Acoustic Soc. Am. 147 399
Google Scholar
[20] 王勇, 林书玉, 张小丽 2014 63 034301
Google Scholar
Wang Y, Lin S Y, Zhang X L 2014 Acta Phys. Sin. 63 034301
Google Scholar
[21] 钱祖文 2009 非线性声学(北京: 科学出版社) 第14页
Qian Z W 2009 Nonliear Acoustics (Beijing: Science Press) p14 (in Chinese)
[22] 陈海霞, 林书玉 2020 69 134301
Google Scholar
Chen H X, Lin S Y 2020 Acta Phys. Sin. 69 134301
Google Scholar
[23] 杜功焕, 朱哲民, 龚秀芬 2001 声学基础 (南京: 南京大学出版社) 第495页
Du G H, Zhu Z M, Gong X F 2001 Fundamentals of Sound (Nanjing: Nanjing University Press) p495 (in Chinese)
-
图 1 各阶谐波的声压分布曲线
Figure 1. The harmonic curve of acoustics.
图 2 二次谐波曲线 (a)不同声压下的二次谐波; (b)不同频率下的二次谐波
Figure 2. The distribution of second harmonic: (a) Different acoustic pressure; (b) different acoustic frequency.
图 3 谐波声能与总能量比分布
Figure 3. Distributions of the ratio of the hamonic and total energies.
图 4 和频波声压分布曲线
Figure 4. The acoustic pressure curves of sum frequency.
图 5 差频波声压分布曲线
Figure 5. The acoustic pressure curves of difference frequency.
图 6 谐波声能与总能量比分布
Figure 6. The ratio of the hamonic and total energies.
图 7 基频声能量的分布
Figure 7. The distribution of fundamental energy.
图 8 基频声能量与总声能量比分布
Figure 8. The ratio of the fundamental and total energies.
-
[1] Westervelt P J 1957 J. Acoust. Soc. Am. 29 199
Google Scholar
[2] Westervelt P J 1957 J. Acoust. Soc. Am. 29 934
Google Scholar
[3] Ingard U, Pridmo-Brown D C 1955 J. Acoust. Soc. Am. 27 1002
[4] Ingard U, Pridmo-Brown D C 1956 J. Acoust. Soc. Am. 28 367
Google Scholar
[5] 钱祖文 1981 30 1479
Google Scholar
Qian Z W 1981 Acta Phys. Sin. 30 1479
Google Scholar
[6] 钱祖文 1981 30 1559
Google Scholar
Qian Z W 1981 Acta Phys. Sin. 30 1559
Google Scholar
[7] 钱祖文 1988 37 221
Google Scholar
Qian Z W 1988 Acta Phys. Sin. 37 221
Google Scholar
[8] Westervelt P J 1963 J. Acoust. Soc. Am. 35 535
Google Scholar
[9] 钱祖文 1976 25 472
Google Scholar
Qian Z W 1976 Acta Phys. Sin. 25 472
Google Scholar
[10] 钱祖文 1999 物理 28 593
Google Scholar
Qian Z W 1999 J. Phys. 28 593
Google Scholar
[11] Wang X, Chen W Z, Liang S D, Zhao T Y, Liang J F 2017 Phys. Rev. E 95 033118
Google Scholar
[12] Wang X, Chen W Z, Yang J, Liang S D 2018 J. Appl. Phys. 123 214904
Google Scholar
[13] 陈伟中 2018 应用声学 37 675
Google Scholar
Chen W Z 2018 Appl. Acoust. 37 675
Google Scholar
[14] Ashokumar M 2011 Ultrason. Sonochem. 18 864
Google Scholar
[15] Wijngaarden L V 1972 Ann. Rev. Fluid Mech. 4 369
Google Scholar
[16] Commander K W, Prosperetti A 1989 J. Acoust. Soc. Am. 85 732
Google Scholar
[17] Vanhille C, Cleofé C P 2011 Ultrason. Sonochem. 18 679
Google Scholar
[18] Thiessen R J, Cheviakov A F 2019 Commun. Nonliear Sci. Numer. Simul. 73 244
Google Scholar
[19] Zhang H H 2020 J. Acoustic Soc. Am. 147 399
Google Scholar
[20] 王勇, 林书玉, 张小丽 2014 63 034301
Google Scholar
Wang Y, Lin S Y, Zhang X L 2014 Acta Phys. Sin. 63 034301
Google Scholar
[21] 钱祖文 2009 非线性声学(北京: 科学出版社) 第14页
Qian Z W 2009 Nonliear Acoustics (Beijing: Science Press) p14 (in Chinese)
[22] 陈海霞, 林书玉 2020 69 134301
Google Scholar
Chen H X, Lin S Y 2020 Acta Phys. Sin. 69 134301
Google Scholar
[23] 杜功焕, 朱哲民, 龚秀芬 2001 声学基础 (南京: 南京大学出版社) 第495页
Du G H, Zhu Z M, Gong X F 2001 Fundamentals of Sound (Nanjing: Nanjing University Press) p495 (in Chinese)
DownLoad:
Catalog
Metrics
- Abstract views: 5472
- PDF Downloads: 103
- Cited By: 0
