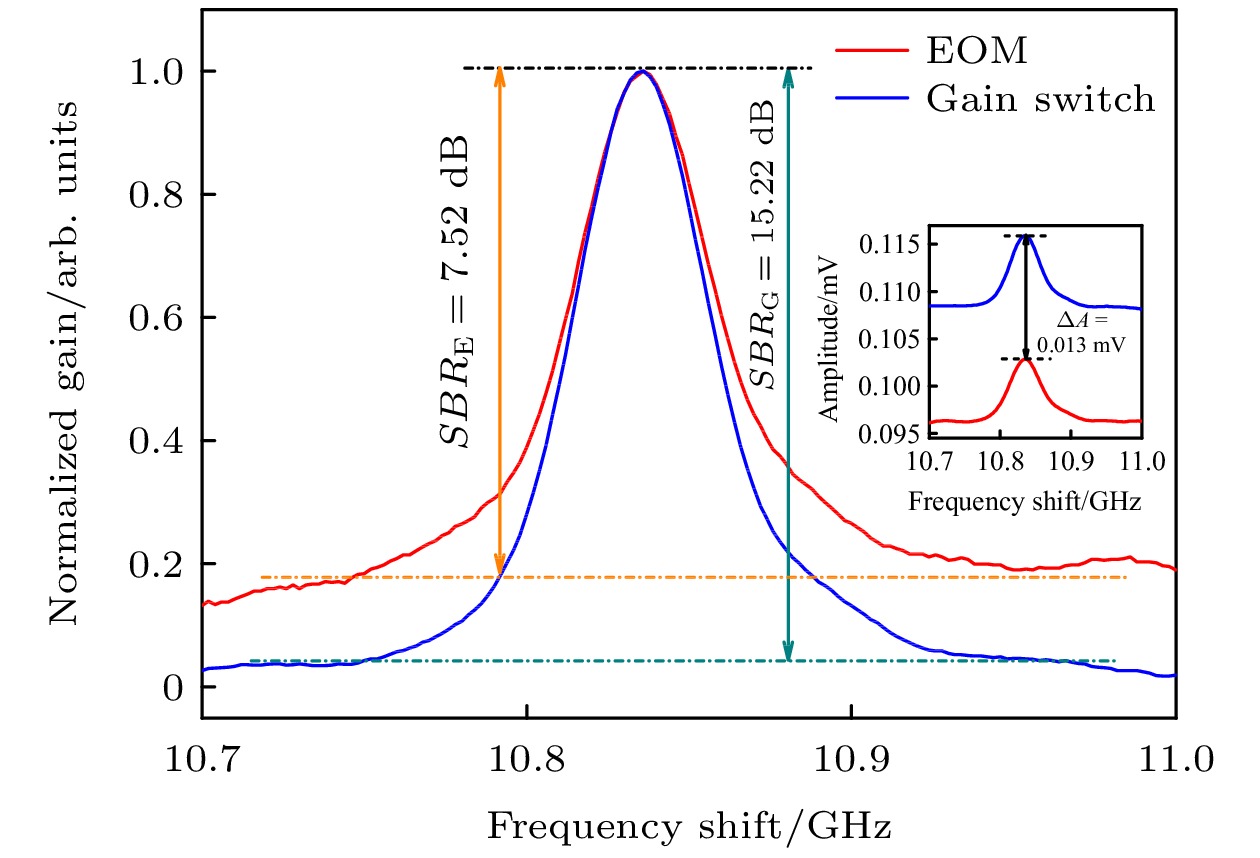
-
Nowadays, distributed dynamic sensing technology based on stimulated Brillouin scattering has been widely employed in civil structure health monitoring, disaster warning, national defense, etc. In this paper, we propose and experimentally demonstrate a novel Brillouin optical correlation-domain analysis based-on gain-switch modulation and dual-slope assisted method for achieving high-accuracy large-range dynamic strain measurement. In single-slope assisted chaotic Brillouin sensing, the measurement accuracy of dynamic strain is deteriorated by the inherent characteristics of time delay signature and power stochastic fluctuations. First, the mechanism behind the acoustic field deterioration and principle of background noise suppression are analyzed theoretically. Then, the chaotic continuous pump light is modulated into pulsed light with a higher extinct ratio of 48.6 dB, where the electro-optical modulator is replaced by a gain switch. And thus, the noise peaks, induced by the secondary peaks and irregular basal oscillations of chaotic auto-correlation curve, are greatly restrained. Comparing with the electro-optical modulator-based system, the signal-to-noise ratio of stimulated Brillouin acoustic field is increased by 3.31 dB in simulation and the signal-to-background ratio of Brillouin gain spectrum is doubled in experiment. Consequently, the measurement accuracy of dynamic strain is improved from ± 40.2 με to ± 23.1 με and the relative error decreases from ± 5.0% to ± 2.9% in single-slope assisted system based-on gain switch modulation. In addition, a dual-slope assisted method is inspired to eliminate the detrimental effect caused by the intrinsic power fluctuations of chaotic laser. A verification experiment is pre-conducted that the dynamic strain could be correctly interrogated although a wide range pump power variation has been manually applied. The measurement accuracy is ultimately enhanced to ± 8.1 με and the relative error is ± 1% correspondingly, implying a higher stability. The dynamic range of this proposed system is retained at 800 με, which is approximately 5 times as large as the dynamic range of the traditional dual-slope assisted configurations. The 4-cm spatial resolution along 30-m FUT is also investigated and consistent with the result obtained previously. A larger measurement range and a higher vibration frequency would be further explored by using the multi-slope assisted method and piezoelectric ceramic oscillator respectively. The superior slope-assisted chaotic Brillouin optical correlation-domain analysis will provide a new solution for the accurate positioning and real-time monitoring of dynamic parameters in modern industry.
-
Keywords:
- chaotic laser /
- dynamic strain measurement /
- slope assisted /
- Brillouin optical correlation-domain analysis
[1] Bao X Y, Chen L 2011 Sensors 11 4152
Google Scholar
[2] 刘铁根, 于哲, 江俊峰, 刘琨, 张学智, 丁振扬, 王双, 胡浩丰, 韩群, 张红霞, 李志宏 2017 66 070705
Google Scholar
Liu T G, Yu Z, Jiang J F, Liu K, Zhang X Z, Ding Z Y, Wang S, Hu H F, Han Q, Zhang H X, Li Z H 2017 Acta Phys. Sin. 66 070705
Google Scholar
[3] 饶云江 2017 66 074207
Google Scholar
Rao Y J 2017 Acta Phys. Sin. 66 074207
Google Scholar
[4] 胡鑫鑫, 王亚辉, 赵乐, 张倩, 张明江, 张建忠, 乔丽君, 王涛, 高少华 2021 中国激光 48 0100001
Google Scholar
Hu X X, Wang Y H, Zhao L, Zhang Q, Zhang M J, Zhang J Z, Qiao L J, Wang T, Gao S H 2021 Chin. J. Lasers 48 0100001
Google Scholar
[5] 朱涛, 郑华, 张敬栋 2020 应用科学学报 38 197
Google Scholar
Zhu T, Zheng H, Zhang J D 2020 J. Appl. Sci. 38 197
Google Scholar
[6] 周登望, 王本章, 巴德欣, 徐金龙, 徐鹏柏, 姜桃飞, 张东昱, 李惠, 董永康 2018 光学学报 38 0328005
Google Scholar
Zhou D W, Wang B Z, Ba D X, Xu J L, Xu P B, Jiang T F, Zhang D Y, Li H, Dong Y K 2018 Acta Opt. Sin. 38 0328005
Google Scholar
[7] Voskoboinik A, Yilmaz O, Willner A, Tur M 2011 Opt. Express 19 B842
Google Scholar
[8] Peled Y, Motil A, Tur M 2012 Opt. Express 20 8584
Google Scholar
[9] Zhou D W, Dong Y K, Wang B Z, Pang C, Ba D X, Zhang H Y, Lu Z W, Li H, Bao X Y 2018 Light Sci. Appl. 7 32
Google Scholar
[10] Zhang C Y, Kishi M, Hotate K 2015 Appl. Phys. Express 8 042501
Google Scholar
[11] Wang B, Fan X Y, Fu Y X, He Z Y 2018 Opt. Express 26 6916
Google Scholar
[12] Bernini R, Minardo A, Zeni L 2009 Opt. Lett. 34 2613
Google Scholar
[13] Peled Y, Motil A, Yaron L, Tur M 2011 Opt. Express 19 19845
Google Scholar
[14] Yang G Y, Fan X Y, He Z Y 2017 J. Lightwave Technol. 35 4451
Google Scholar
[15] Yang G Y, Fan X Y, Wang B, He Z Y 2018 Opt. Express 26 32599
Google Scholar
[16] Motil A, Danon O, Peled Y, Tur M 2014 IEEE Photonics Technol. Lett. 26 797
Google Scholar
[17] Ba D X, Wang B Z, Zhou D W, Yin M J, Dong Y K, Li H, Lu Z W, Fan Z G 2016 Opt. Express 24 9781
Google Scholar
[18] Zheng H, Feng D Q, Zhang J D, Zhu T, Bai Y Z, Qu D R, Huang X B, Qiu F 2019 Opt. Lett. 44 1245
Google Scholar
[19] Wang B, Fan X, Fu Y X, He Z 2019 J. Lightwave Technol. 37 4573
Google Scholar
[20] Wang Y H, Zhao L, Zhang M J, Zhang J Z, Qiao L J, Wang T, Gao S H, Zhang Q, Wang Y C 2020 Opt. Lett. 45 1822
Google Scholar
[21] Zhao L, Wang Y H, Hu X X, Zhang M J, Zhang J Z, Qiao L J, Wang T, Gao S H, Himika A A 2020 Opt. Express 28 18189
Google Scholar
[22] Jeong J H, Lee K, Song K Y, Jeong J M, Lee S B 2011 Opt. Express 19 18721
Google Scholar
[23] Zhang J Z, Wang Y H, Zhang M J, Zhang Q, Li M W, Wu C Y, Qiao L J, Wang Y C 2018 Opt. Express 26 17597
Google Scholar
[24] Bai Q, Xue B, Gu H, Wang D, Wang Y , Zhang M J, Jin B Q, Wang Y C 2019 IEEE Photon. Technol. Lett. 31 283
[25] Wang Y H, Zhang M J, Zhang J Z, Qiao L J, Wang T, Zhang Q, Zhao L, Wang Y C 2019 J. Lightwave Technol. 37 3706
Google Scholar
[26] 张倩, 王亚辉, 张明江, 张建忠, 乔丽君, 王涛, 赵乐 2019 68 104208
Google Scholar
Zhang Q, Wang Y H, Zhang M J, Zhang J Z, Qiao L J, Wang T, Zhao L 2019 Acta Phys. Sin. 68 104208
Google Scholar
-
图 1 斜坡辅助式混沌布里渊光相关域分析动态应变传感技术实验装置图. 插图A为相同输出功率下混沌激光器与分布式反馈半导体激光器的输出信号时序, 插图B为混沌激光自相关曲线和多阶相关峰示意图, 插图C为连续混沌激光被调制为脉冲混沌激光
Figure 1. Experimental setup of SA-CBOCDA for dynamic strain sensing. Inset A, the time series of chaotic laser and that of DFB-LD under the same output power. Inset B, the autocorrelation curve of chaotic laser and the schematic diagram of multiple order correlation peaks. Inset C, the continuous chaotic laser being amplitude-modulated into pulse chaos.
图 2 不同调制方式及作用效果 (a)不同调制方式下脉冲混沌光时序及消光比; (b)不同调制方式下仿真的混沌受激布里渊声波场强度及信噪比
Figure 2. Different modulation methods and the corresponding effects: (a) The time series of pulse chaos and ERs of these under different modulation modes; (b) simulation results of chaotic SBS acoustic field amplitude and the corresponding SNR.
图 3 不同调制方式下的归一化混沌布里渊增益谱及其信号背景噪声比; 插图为测得的原始布里渊增益谱
Figure 3. Normalized chaotic BGSs and SBR analysis of these, under different modulation modes. Inset view: the measured original BGSs, corresponding to the main view.
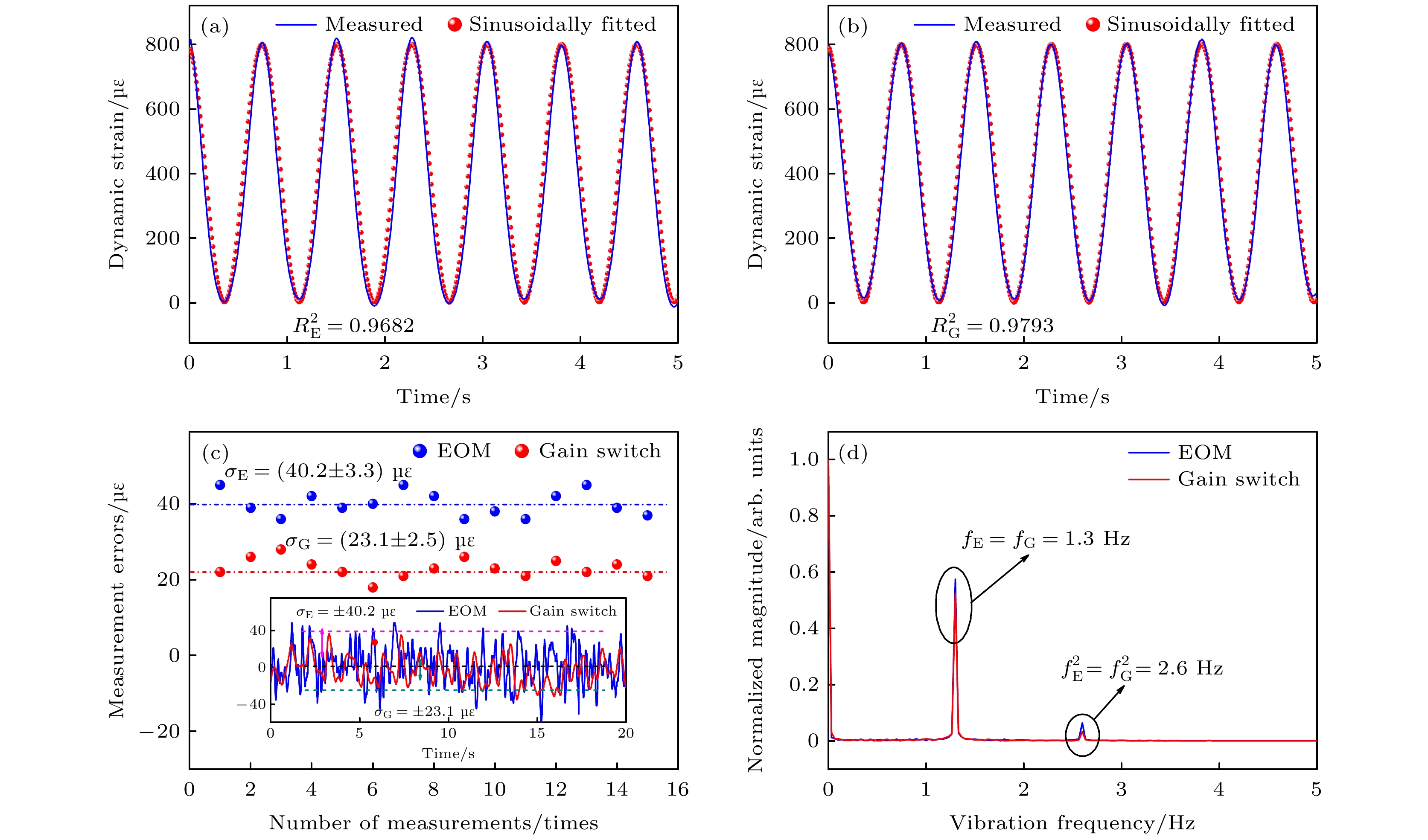
图 4 不同调制方式下的动态应变测量结果 (a) EOM调制系统动态应变测量时序及正弦拟合曲线; (b) GS调制系统动态应变测量时序及正弦拟合曲线; (c)两种调制方式下的动态应变测量误差, 插图为单次测量典型结果; (d)两种调制方式下的动态应变频率
Figure 4. The results of dynamic strain measurement under different modulation modes: (a) Time series and sine fitting curve of dynamic strain in EOM modulation system; (b) time series and sine fitting curve of dynamic strain in GS modulation system; (c) measurement error of dynamic strain under two modulation modes, inset view is the typical result of a single measurement; (d) vibration frequency under two modulation modes.
图 5 双斜坡辅助技术原理示意图 (a)布里渊增益比的理论分析; (b)布里渊增益比实验标定的理论模型
Figure 5. Principle of DSA method: (a) theoretical analysis of
${R_{\rm{B}}}$ ; (b) theoretical model for experimentally calibrating${R_{\rm{B}}}$ .
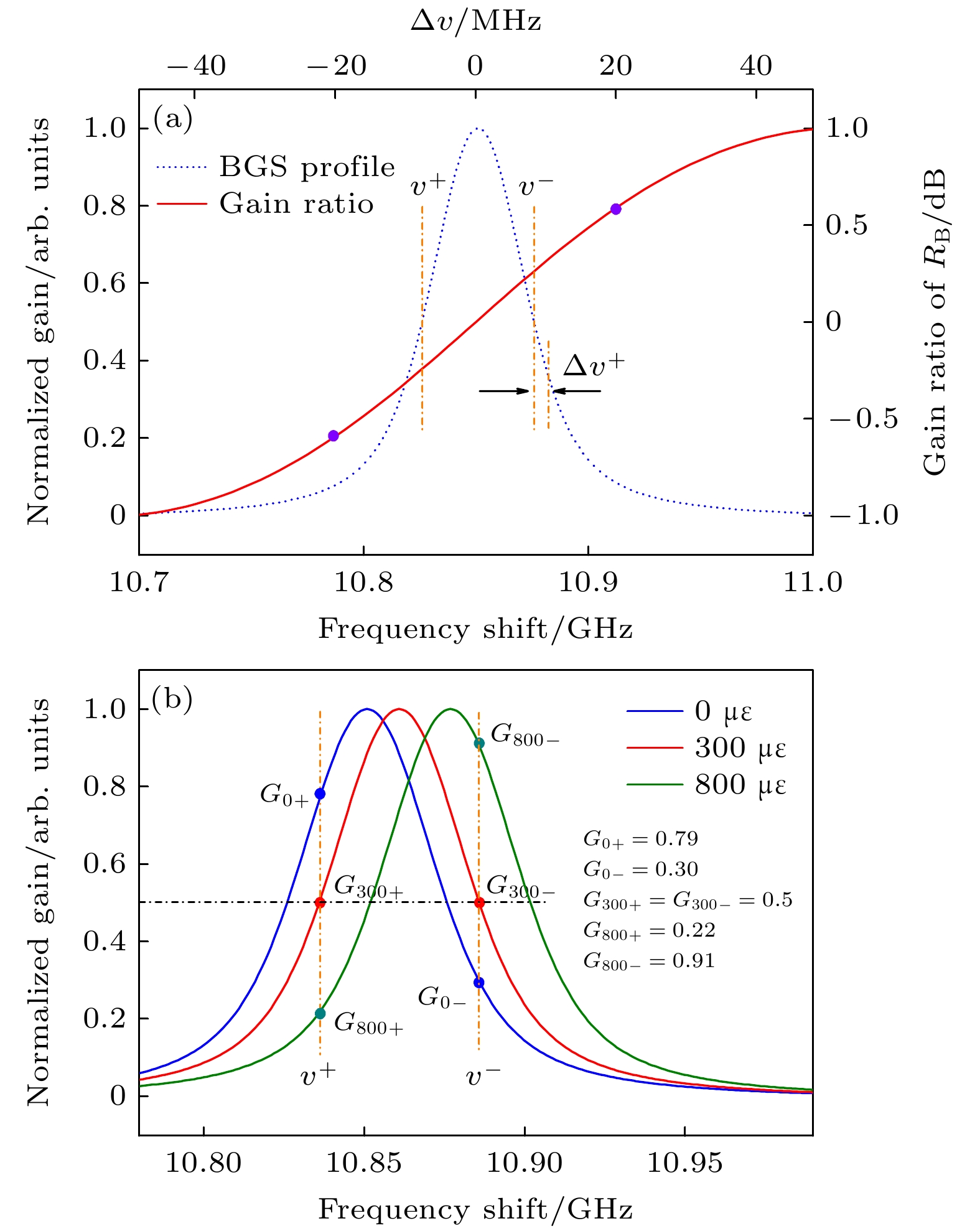
图 6 双斜坡辅助系统的增益比-应变转换系数 (a)不同大小准静态应变对应的增益比曲线; (b)根据线性拟合曲线计算的转换系数
Figure 6. Estimate of the conversion coefficient,
${C_{\rm{s}}}$ , between the strain value and${R_{\rm{B}}}$ in DSA system: (a) The${R_{\rm{B}}}$ curves under different quasi-static strain; (b) final coefficient calculated from the linear fitted curve.
图 7 双斜坡辅助技术消除泵浦功率波动影响的验证实验 (a)手动施加泵浦光功率波动时利用单斜坡辅助技术测得的动态应变响应时序; (b)双斜坡辅助技术解调的动态应变大小
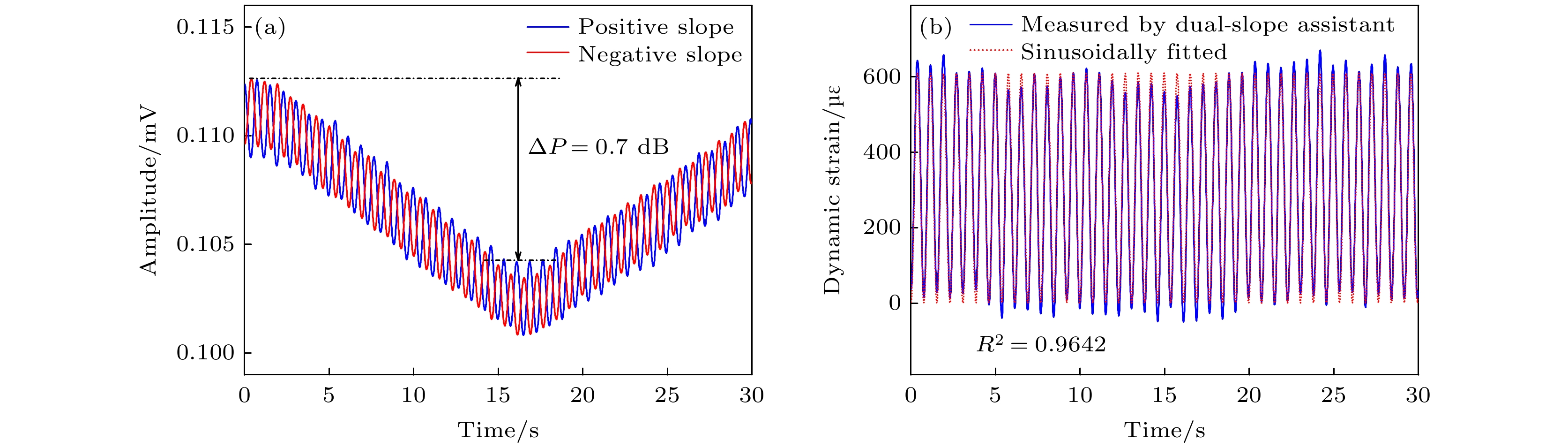
Figure 7. The verification experiment of pump-power-independent measurement by using DSA method: (a) Measured Brillouin amplitude using SSA method when a dynamic strain is applied with a manual change of pump power; (b) measured dynamic strain using DSA method.
图 8 施加0−800 με时的双斜坡辅助系统动态应变测量结果 (a)动态应变测量时序及正弦拟合曲线; (b)动态应变测量误差, 插图为单次测量典型结果; (c)动态应变振动频率
Figure 8. Measurement results of DSA system when a dynamic strain of 0−800 με is applied: (a) Measured time trace and sine-fitted curve; (b) measurement error, inset view is the typical result of a single measurement; (c) vibration frequency.
-
[1] Bao X Y, Chen L 2011 Sensors 11 4152
Google Scholar
[2] 刘铁根, 于哲, 江俊峰, 刘琨, 张学智, 丁振扬, 王双, 胡浩丰, 韩群, 张红霞, 李志宏 2017 66 070705
Google Scholar
Liu T G, Yu Z, Jiang J F, Liu K, Zhang X Z, Ding Z Y, Wang S, Hu H F, Han Q, Zhang H X, Li Z H 2017 Acta Phys. Sin. 66 070705
Google Scholar
[3] 饶云江 2017 66 074207
Google Scholar
Rao Y J 2017 Acta Phys. Sin. 66 074207
Google Scholar
[4] 胡鑫鑫, 王亚辉, 赵乐, 张倩, 张明江, 张建忠, 乔丽君, 王涛, 高少华 2021 中国激光 48 0100001
Google Scholar
Hu X X, Wang Y H, Zhao L, Zhang Q, Zhang M J, Zhang J Z, Qiao L J, Wang T, Gao S H 2021 Chin. J. Lasers 48 0100001
Google Scholar
[5] 朱涛, 郑华, 张敬栋 2020 应用科学学报 38 197
Google Scholar
Zhu T, Zheng H, Zhang J D 2020 J. Appl. Sci. 38 197
Google Scholar
[6] 周登望, 王本章, 巴德欣, 徐金龙, 徐鹏柏, 姜桃飞, 张东昱, 李惠, 董永康 2018 光学学报 38 0328005
Google Scholar
Zhou D W, Wang B Z, Ba D X, Xu J L, Xu P B, Jiang T F, Zhang D Y, Li H, Dong Y K 2018 Acta Opt. Sin. 38 0328005
Google Scholar
[7] Voskoboinik A, Yilmaz O, Willner A, Tur M 2011 Opt. Express 19 B842
Google Scholar
[8] Peled Y, Motil A, Tur M 2012 Opt. Express 20 8584
Google Scholar
[9] Zhou D W, Dong Y K, Wang B Z, Pang C, Ba D X, Zhang H Y, Lu Z W, Li H, Bao X Y 2018 Light Sci. Appl. 7 32
Google Scholar
[10] Zhang C Y, Kishi M, Hotate K 2015 Appl. Phys. Express 8 042501
Google Scholar
[11] Wang B, Fan X Y, Fu Y X, He Z Y 2018 Opt. Express 26 6916
Google Scholar
[12] Bernini R, Minardo A, Zeni L 2009 Opt. Lett. 34 2613
Google Scholar
[13] Peled Y, Motil A, Yaron L, Tur M 2011 Opt. Express 19 19845
Google Scholar
[14] Yang G Y, Fan X Y, He Z Y 2017 J. Lightwave Technol. 35 4451
Google Scholar
[15] Yang G Y, Fan X Y, Wang B, He Z Y 2018 Opt. Express 26 32599
Google Scholar
[16] Motil A, Danon O, Peled Y, Tur M 2014 IEEE Photonics Technol. Lett. 26 797
Google Scholar
[17] Ba D X, Wang B Z, Zhou D W, Yin M J, Dong Y K, Li H, Lu Z W, Fan Z G 2016 Opt. Express 24 9781
Google Scholar
[18] Zheng H, Feng D Q, Zhang J D, Zhu T, Bai Y Z, Qu D R, Huang X B, Qiu F 2019 Opt. Lett. 44 1245
Google Scholar
[19] Wang B, Fan X, Fu Y X, He Z 2019 J. Lightwave Technol. 37 4573
Google Scholar
[20] Wang Y H, Zhao L, Zhang M J, Zhang J Z, Qiao L J, Wang T, Gao S H, Zhang Q, Wang Y C 2020 Opt. Lett. 45 1822
Google Scholar
[21] Zhao L, Wang Y H, Hu X X, Zhang M J, Zhang J Z, Qiao L J, Wang T, Gao S H, Himika A A 2020 Opt. Express 28 18189
Google Scholar
[22] Jeong J H, Lee K, Song K Y, Jeong J M, Lee S B 2011 Opt. Express 19 18721
Google Scholar
[23] Zhang J Z, Wang Y H, Zhang M J, Zhang Q, Li M W, Wu C Y, Qiao L J, Wang Y C 2018 Opt. Express 26 17597
Google Scholar
[24] Bai Q, Xue B, Gu H, Wang D, Wang Y , Zhang M J, Jin B Q, Wang Y C 2019 IEEE Photon. Technol. Lett. 31 283
[25] Wang Y H, Zhang M J, Zhang J Z, Qiao L J, Wang T, Zhang Q, Zhao L, Wang Y C 2019 J. Lightwave Technol. 37 3706
Google Scholar
[26] 张倩, 王亚辉, 张明江, 张建忠, 乔丽君, 王涛, 赵乐 2019 68 104208
Google Scholar
Zhang Q, Wang Y H, Zhang M J, Zhang J Z, Qiao L J, Wang T, Zhao L 2019 Acta Phys. Sin. 68 104208
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 5298
- PDF Downloads: 86
- Cited By: 0
