-
In the face of the historical change of international measurement system, the classical physics based physical standard corresponding to many measurement parameters develops toward "natural standard", namely quantum standard. In order to further improve the reproducibility and accuracy of vacuum value, the latest research uses quantum technology to realize the measurement and characterization of vacuum value. In this method, Fabry- Perot cavity is used to accurately measure the refractive index of the gas. The density can be calculated by the refractive index and inversed to obtain the corresponding vacuum value. The measurement of the gas refractive index is the key to the accuracy of the vacuum value. The macroscopic permittivity of nonpolar gases is related to the microscopic polarization parameters of atoms through quantum dynamics. In recent years, with the rapid development of ab initio theory and methods on the electromagnetic and thermodynamic properties of monatomic molecules, the calculation accuracy of relevant parameters was constantly improved, which can further reduce the measurement uncertainty of the above methods. In this paper, the theoretical value of helium refractive index is calculated accurately based on the first principle with known pressure and temperature. The relationship between gas pressure and refractive index is obtained, and the relative uncertainty of the theoretical value of refractive index is 6.27 × 10–12. Then, the refractive index of helium in a range of 102–105 Pa is measured by the vacuum measuring device which is based on Fabry-Perot cavity, and the uncertainty of measurement is 9.59 × 10–8. Finally, the discrepancy between the theoretical and measured values of helium refractive index is compared and analyzed. It can be concluded that the the uncertainty of helium refractive index measurement originates from the deformation of the cavity caused by helium permeation. Therefore, solving the problem of helium permeation is the key to establishing a new vacuum standard. In this paper, the change of cavity length caused by helium penetration in the cavity is corrected. The refractive index coefficient is corrected at various pressure points in a vacuum range of 103–105 Pa, and its pressure-dependent expression is obtained The variation of cavity length caused by gas pressure is further quantified. The relationship between the change of cavity caused by gas pressure and that caused by the refractive index is obtained. The correction parameter of cavity length is calculated to be 3.12 × 10–2. In the future experiment of helium refractive index measurement by means of Fabry-Perot cavity, the refractive index correction coefficient at each pressure point given in this paper can be used to correct the refractive index measurement results, thereby eliminating the influence of helium penetration on the refractive index measurement, and obtaining the gas pressure with high accuracy.
-
Keywords:
- vacuum metrology /
- Fabry-Perot cavity /
- refractive index of gas /
- helium permeability
[1] Gibney E 2017 Nature 550 312
Google Scholar
[2] 李得天, 成永军, 习振华 2018 宇航计测技术 38 1
Google Scholar
Li D T, Cheng Y J, Xi Z H 2018 Journal of Astronautic Metrology and Measurement 38 1
Google Scholar
[3] Egan P F, Stone J A, Scherschligt J K, Harvey A H 2019 J. Vac. Sci. Technol. A 37 031603
Google Scholar
[4] Silander I, Hausmaninger T, Zelan M, Axner O 2018 J. Vac. Sci. Technol. A 36 03E105
[5] Egan P F, Stone J A 2011 Appl. Opt. 50 3076
Google Scholar
[6] Hendricks J H, Ricker J E, Stone J A, Egan P F, Scace G E, Strouse G F, Olson D A, Gerty D 2015 XXI IMEKO World Congress “Measurement in Research and Industry” Prague, Czech Republic, August 30–September 4, 2015 p1636
[7] Egan P, Stone J, Ricker J, Hendricks J 2016 2016 Conference on Precision Electromagnetic Measurements Ottawa, Canada, July 10–15, 2016 p1
[8] Zelan M, Silander I, Hausmaninger T, Axner O 2017 arXiv: 1704.01185
[9] Takei Y, Arai K, Yoshida H 2020 Measurement 151 107090
Google Scholar
[10] Axner O, Silander I, Hansmaninger T, Zelan M 2017 arXiv: 1704.01187
[11] 贾文杰, 习振华, 范栋, 董猛, 吴成耀, 成永军 2020 光学学报 40 2212005
Google Scholar
Jia W J, Xi Z H, Fan D, Dong M, Wu C Y, Cheng Y J 2020 Acta Opt. Sin. 40 2212005
Google Scholar
[12] 许玉蓉, 刘洋洋, 王进, 孙羽, 习振华, 李得天, 胡水明 2020 69 15
Xu Y R, Liu Y Y, Wang J, Sun Y, Xi Z H, Li D T, Hu S M 2020 Acta Phys. Sin. 69 15
[13] Bhatia A K, Drachman R J 1998 Phys. Rev. A. 58 4470
Google Scholar
[14] Hurly J J, Moldover M R 2000 Res. Natl. Inst. Stand. Technol. 105 667
Google Scholar
[15] Koch H, Hättig C, Larsen H, Olsen J, Jorgensen P, Fernandez B, Rizzo A 1999 J. Chem. Phys. 111 10108
Google Scholar
[16] Łach G, Jeziorski B, Szalewicz K 2004 Phys. Rev. Lett. 92 233001
Google Scholar
[17] Puchalski M, Piszczatowski K, Komasa J, Jeziorski B, Szalewicz K 2016 Phys. Rev. A 93 032515
Google Scholar
[18] Cencek W, Drzybytek M, Komasa J, Mehl J B, Jeziorski B 2012 J. Chem. Phys. 136 224303
Google Scholar
[19] Bich E, Hellmann R, Vogel E 2007 Mol. Phys. 105 3035
Google Scholar
[20] Rizzo K A, Hättig C, Fernández B, Koch H 2002 J. Chem. Phys. 117 2609
Google Scholar
[21] Bruch L W, Weinhold F 2002 J. Chem. Phys. 117 3243
Google Scholar
[22] Mohr P J, Newell D B, Taylor B N, Tiesinga E 2018 Metrologia 55 125
Google Scholar
[23] Acdiaj S, Yang Y C, Jousten K, Rubin T 2018 J. Chem. Phys. 148 116101
Google Scholar
-
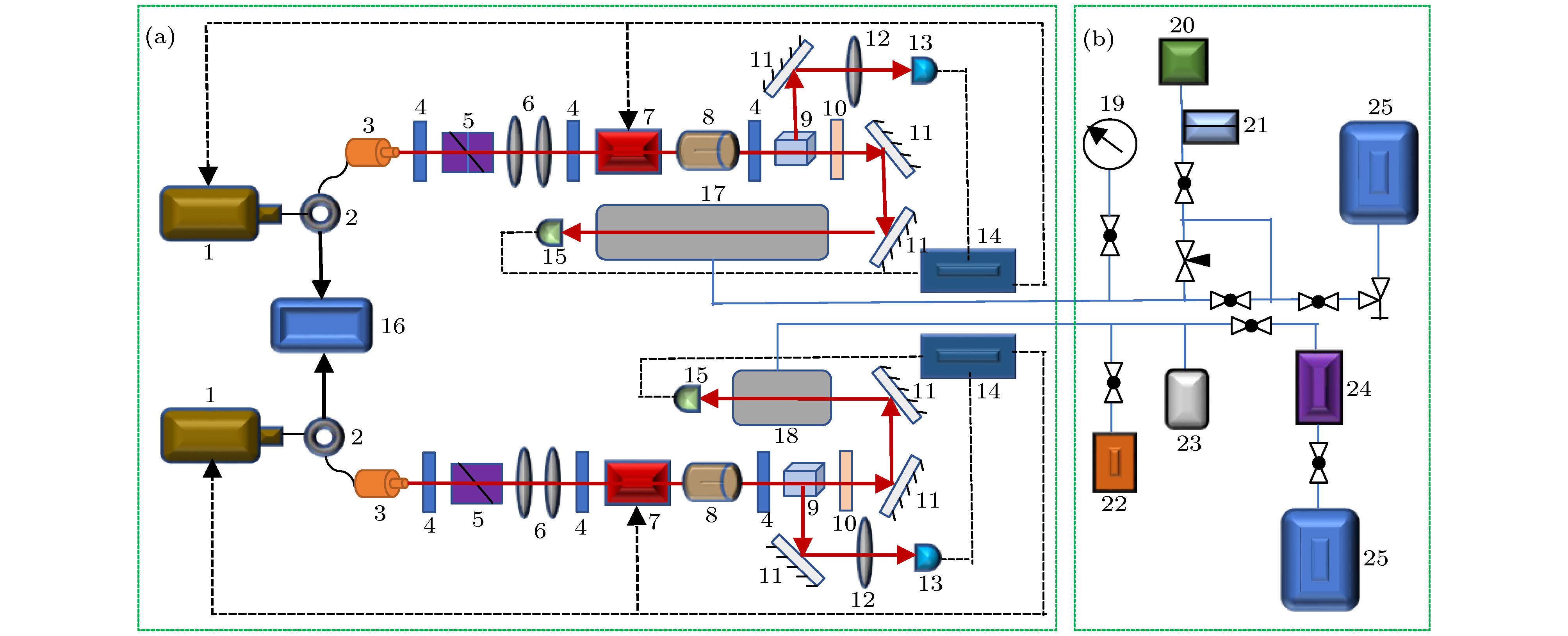
图 1 基于F-P激光谐振腔的真空测量装置结构图 (a)部分为光路图;(b)部分为气路图 (1. 激光器; 2. 分束光纤; 3. 激光准直器; 4. 1/2波片; 5. 格兰棱镜; 6.透镜组; 7.电光调制器; 8. 光隔离器; 9. 偏振分光棱镜; 10. 1/4波片; 11. 高反镜; 12. 透镜; 13. 光电放大探测器; 14. PDH锁频装置; 15. 光电探测器; 16. 频率计; 17. 检测腔; 18. 参考腔; 19. 电容薄膜真空计; 20. 气瓶; 21. 冷阱; 22. 离子泵; 23. 电离规; 24. 分子泵; 25. 机械泵. 红色实线为光路; 黑色实线为光纤; 黑色虚线表示反馈作用; 蓝色实线为气路.)
Figure 1. Structure diagram of vacuum measuring device based on F-P cavity.
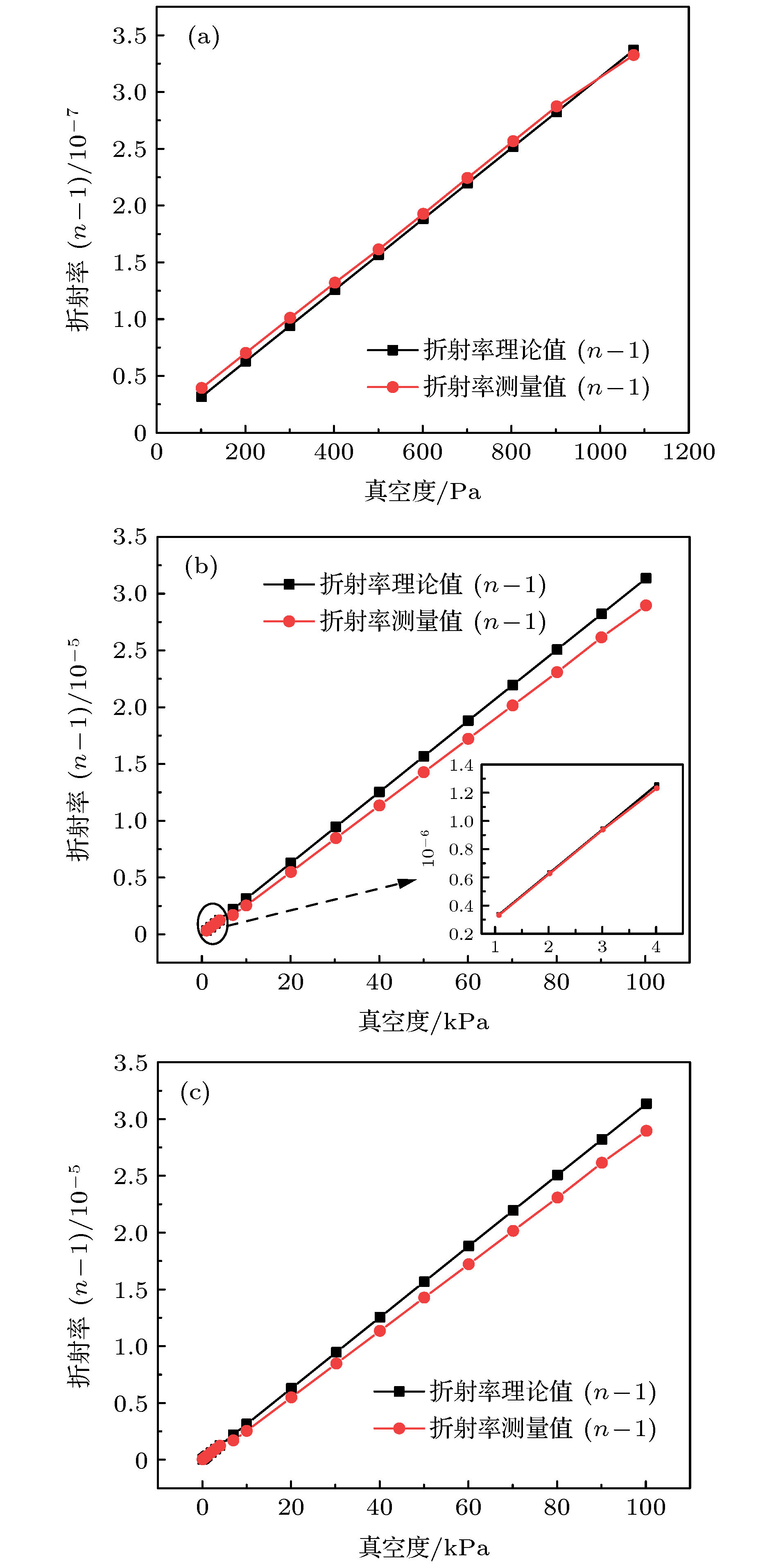
图 2 (a)102−103 Pa范围内折射率理论值与测量值对比图; (b) 103−105 Pa范围内折射率理论值与测量值对比图; (c) 102−105 Pa范围内折射率理论值与测量值总对比图
Figure 2. (a) Comparison between theoretical and measured values of refractive index in the range of 102−103 Pa; (b) comparison between theoretical and measured values of refractive index in the range of 103−104 Pa; (c) total comparison between theoretical and measured values of refractive index in the range of 102−105 Pa.
表 1 He极化率的展开系数(原子单位制)[17]
Table 1. Cofficients in the expansion of the polarizability of Helium.
系数 值 A0 1.3837295330(1) A2 3.2036661813(3) × 105 A4 8.803569264(2) × 1010 A6 2.6219915496(7) × 1016 
DownLoad: CSV
表 2 折射率计算结果
Table 2. Calculation results of refractive index.
序号 真空度 p/Pa 折射率 n – 1 序号 真空度 p/Pa 折射率 n – 1 1 101 3.17726 × 10–8 13 4015 1.25716 × 10–6 2 201 6.29408 × 10–8 14 7031 2.20149 × 10–6 3 301 9.42498 × 10–8 15 10036 3.14235 × 10–6 4 402 1.25916 × 10–7 16 20086 6.28879 × 10–6 5 500 1.56736 × 10–7 17 30252 9.47123 × 10–6 6 601 1.88312 × 10–7 18 40070 1.25445 × 10–5 7 701 2.19523 × 10–7 19 50035 1.56690 × 10–5 8 804 2.51819 × 10–7 20 60098 1.88127 × 10–5 9 901 2.82417 × 10–7 21 70122 2.19495 × 10–5 10 1075 3.36856 × 10–7 22 80111 2.50751 × 10–5 11 2020 6.32721 × 10–7 23 90131 2.82100 × 10–5 12 3012 9.43113 × 10–7 24 100166 3.13494 × 10–5
DownLoad: CSV
表 3 折射率测量结果
Table 3. Text results of refractive index.
序号 真空度 p/Pa 折射率n – 1 序号 真空度 p/Pa 折射率n – 1 1 101 3.93406 × 10–8 13 4015 1.23185 × 10–6 2 201 7.02328 × 10–8 14 7031 1.69882 × 10–6 3 301 1.01155 × 10–7 15 10036 2.53943 × 10–6 4 402 1.32115 × 10–7 16 20086 5.48760 × 10–6 5 500 1.61436 × 10–7 17 30252 8.46879 × 10–6 6 601 1.92653 × 10–7 18 40070 1.13490 × 10–5 7 701 2.24327 × 10–7 19 50035 1.42741 × 10–5 8 804 2.56659 × 10–7 20 60098 1.72200 × 10–5 9 901 2.87352 × 10–7 21 70122 2.01575 × 10–5 10 1075 3.32603 × 10–7 22 80111 2.30841 × 10–5 11 2020 6.27737 × 10–7 23 90131 2.61473 × 10–5 12 3012 9.38649 × 10–7 24 100166 2.89609 × 10–5
DownLoad: CSV
表 4 折射率修正系数
Table 4. Refractive index correction coefficient.
序号 真空度 p/Pa 修正系数 φ(p) 序号 真空度 p/Pa 修正系数 φ(p) 1 1075 4.25267 × 10–9 13 40070 1.19548 × 10–6 2 2020 4.98394 × 10–9 14 50053 1.39494 × 10–6 3 3012 4.46381 × 10–9 15 60098 1.59267 × 10–6 4 4015 2.53114 × 10–8 16 70122 1.79205 × 10–6 5 7031 5.02669 × 10–7 17 80111 1.99100 × 10–6 6 10036 6.02924 × 10–7 18 90131 2.06270 × 10–6 7 20086 8.01189 × 10–7 19 100166 2.38851 × 10–6 8 30252 1.00244 × 10–6
DownLoad: CSV
-
[1] Gibney E 2017 Nature 550 312
Google Scholar
[2] 李得天, 成永军, 习振华 2018 宇航计测技术 38 1
Google Scholar
Li D T, Cheng Y J, Xi Z H 2018 Journal of Astronautic Metrology and Measurement 38 1
Google Scholar
[3] Egan P F, Stone J A, Scherschligt J K, Harvey A H 2019 J. Vac. Sci. Technol. A 37 031603
Google Scholar
[4] Silander I, Hausmaninger T, Zelan M, Axner O 2018 J. Vac. Sci. Technol. A 36 03E105
[5] Egan P F, Stone J A 2011 Appl. Opt. 50 3076
Google Scholar
[6] Hendricks J H, Ricker J E, Stone J A, Egan P F, Scace G E, Strouse G F, Olson D A, Gerty D 2015 XXI IMEKO World Congress “Measurement in Research and Industry” Prague, Czech Republic, August 30–September 4, 2015 p1636
[7] Egan P, Stone J, Ricker J, Hendricks J 2016 2016 Conference on Precision Electromagnetic Measurements Ottawa, Canada, July 10–15, 2016 p1
[8] Zelan M, Silander I, Hausmaninger T, Axner O 2017 arXiv: 1704.01185
[9] Takei Y, Arai K, Yoshida H 2020 Measurement 151 107090
Google Scholar
[10] Axner O, Silander I, Hansmaninger T, Zelan M 2017 arXiv: 1704.01187
[11] 贾文杰, 习振华, 范栋, 董猛, 吴成耀, 成永军 2020 光学学报 40 2212005
Google Scholar
Jia W J, Xi Z H, Fan D, Dong M, Wu C Y, Cheng Y J 2020 Acta Opt. Sin. 40 2212005
Google Scholar
[12] 许玉蓉, 刘洋洋, 王进, 孙羽, 习振华, 李得天, 胡水明 2020 69 15
Xu Y R, Liu Y Y, Wang J, Sun Y, Xi Z H, Li D T, Hu S M 2020 Acta Phys. Sin. 69 15
[13] Bhatia A K, Drachman R J 1998 Phys. Rev. A. 58 4470
Google Scholar
[14] Hurly J J, Moldover M R 2000 Res. Natl. Inst. Stand. Technol. 105 667
Google Scholar
[15] Koch H, Hättig C, Larsen H, Olsen J, Jorgensen P, Fernandez B, Rizzo A 1999 J. Chem. Phys. 111 10108
Google Scholar
[16] Łach G, Jeziorski B, Szalewicz K 2004 Phys. Rev. Lett. 92 233001
Google Scholar
[17] Puchalski M, Piszczatowski K, Komasa J, Jeziorski B, Szalewicz K 2016 Phys. Rev. A 93 032515
Google Scholar
[18] Cencek W, Drzybytek M, Komasa J, Mehl J B, Jeziorski B 2012 J. Chem. Phys. 136 224303
Google Scholar
[19] Bich E, Hellmann R, Vogel E 2007 Mol. Phys. 105 3035
Google Scholar
[20] Rizzo K A, Hättig C, Fernández B, Koch H 2002 J. Chem. Phys. 117 2609
Google Scholar
[21] Bruch L W, Weinhold F 2002 J. Chem. Phys. 117 3243
Google Scholar
[22] Mohr P J, Newell D B, Taylor B N, Tiesinga E 2018 Metrologia 55 125
Google Scholar
[23] Acdiaj S, Yang Y C, Jousten K, Rubin T 2018 J. Chem. Phys. 148 116101
Google Scholar
-
[1] Liu Yang-Yang, Hu Chang-Le, Sun Yu, Wang Jin, Hu Shui-Ming. Measurement of gas pressure by double-cavity comparison refractive index method. Acta Physica Sinica, 2022, 71(8): 080601. doi: 10.7498/aps.71.20212234 [2] Xu Zi-Qiang, Wu Xiao-Qing, Xu Man-Man, Bi Cui-Cui, Han Yong, Shao Shi-Yong. Estimation of ${\boldsymbol{C_n^2}}$ profile of troposphere over the sea. Acta Physica Sinica, 2021, 70(24): 244204. doi: 10.7498/aps.70.20211201[3] Zhang Xiang-Yu, Liu Hui-Gang, Kang Ming, Liu Bo, Liu Hai-Tao. Metal-dielectric-metal multilayer structure with tunable Fabry-Perot resonance for highly sensitive refractive index sensing. Acta Physica Sinica, 2021, 70(14): 140702. doi: 10.7498/aps.70.20202058 [4] Xu Yu-Rong, Liu Yang-Yang, Wang Jin, Sun Yu, Xi Zhen-Hua, Li De-Tian, Hu Shui-Ming. Vacuum metrology based on refractive index of gas. Acta Physica Sinica, 2020, 69(15): 150601. doi: 10.7498/aps.69.20200706 [5] Qi Yun-Ping, Zhang Xue-Wei, Zhou Pei-Yang, Hu Bing-Bing, Wang Xiang-Xian. Refractive index sensor and filter of metal-insulator-metal waveguide based on ring resonator embedded by cross structure. Acta Physica Sinica, 2018, 67(19): 197301. doi: 10.7498/aps.67.20180758 [6] Zhang Chen, Cao Xiang-Yu, Gao Jun, Li Si-Jia, Zheng Yue-Jun. Design of a broadband and high-gain shared-aperture fabry-perot resonator magneto-electric microstrip antenna. Acta Physica Sinica, 2016, 65(13): 134205. doi: 10.7498/aps.65.134205 [7] Cong Li-Li, Fu Qiang, Cao Xiang-Yu, Gao Jun, Song Tao, Li Wen-Qiang, Zhao Yi, Zheng Yue-Jun. A novel circularly polarized patch antenna with low radar cross section and high-gain. Acta Physica Sinica, 2015, 64(22): 224219. doi: 10.7498/aps.64.224219 [8] Chen Ying, Fan Hui-Qing, Lu Bo. Tamm state of semi-infinite photonic crystal based on surface defect cavity with porous silicon and its refractive index sensing mechanism. Acta Physica Sinica, 2014, 63(24): 244207. doi: 10.7498/aps.63.244207 [9] Liu Xiao-Bo, Shi Hong-Yu, Chen Bo, Jiang Yan-Sheng, Xu Zhuo, Zhang An-Xue. Studies on the mechanism of refractive index gradient surface. Acta Physica Sinica, 2014, 63(21): 214201. doi: 10.7498/aps.63.214201 [10] Zhou Wen-Fei, Ye Xiao-Ling, Xu Bo, Zhang Shi-Zhu, Wang Zhan-Guo. Study on properties of the H1 photonic crystal slab cavity using the effective index perturbation method. Acta Physica Sinica, 2012, 61(5): 054202. doi: 10.7498/aps.61.054202 [11] Zeng Zhi-Wen, Liu Hai-Tao, Zhang Si-Wen. Design of extraordinary-optical-transimission refractive-index sensor of subwavelength metallic slit array based on a Fabry-Perot model. Acta Physica Sinica, 2012, 61(20): 200701. doi: 10.7498/aps.61.200701 [12] Yue Hong-Wei, Wang Zheng, Fan Bin, Song Feng-Bin, You Feng, Zhao Xin-Jie, He Ming, Fang Lan, Yan Shao-Lin. Millimeter wavelength coherent emission from high temperature superconducting bicrystal Josephson junction array. Acta Physica Sinica, 2010, 59(8): 5755-5758. doi: 10.7498/aps.59.5755 [13] Wang Zheng, Zhao Xin-Jie, He Ming, Zhou Tie-Ge, Yue Hong-Wei, Yan Shao-Lin. Simulations of impedance matching and phase locking of Josephson junction arrays embedded in a Fabry-Perot resonator. Acta Physica Sinica, 2010, 59(5): 3481-3487. doi: 10.7498/aps.59.3481 [14] Yue Hong-Wei, Yan Shao-Lin, Zhou Tie-Ge, Xie Qing-Lian, You Feng, Wang Zheng, He Ming, Zhao Xin-Jie, Fang Lan, Yang Yang, Wang Fu-Yin, Tao Wei-Wei. Millimeter wave irradiation characteristics of high temperature superconductor bicrystal Josephson junction embedded in a Fabry-Perot resonator. Acta Physica Sinica, 2010, 59(2): 1282-1287. doi: 10.7498/aps.59.1282 [15] Tang Shi-Wei, Zhu Wei-Ren, Zhao Xiao-Peng. Multiband negative index metamaterials at optical frequencies. Acta Physica Sinica, 2009, 58(5): 3220-3223. doi: 10.7498/aps.58.3220 [16] Zeng Ran, Xu Jing-Ping, Yang Ya-Ping, Liu Shu-Tian. Influence of negative refractive material on Casimir effect. Acta Physica Sinica, 2007, 56(11): 6446-6450. doi: 10.7498/aps.56.6446 [17] Shen Zi-Cai, Shen Jian, Liu Shi-Jie, Kong Wei-Jin, Shao Jian-Da, Fan Zheng-Xiu. Discussion on the stratified merit of graded index coatings. Acta Physica Sinica, 2007, 56(3): 1325-1328. doi: 10.7498/aps.56.1325 [18] Zhuang Fei, Shen Jian-Qi, Ye Jun. Controlling the photonic bandgap structures via manipulation of refractive index of electromagnetically induced transparency vapor. Acta Physica Sinica, 2007, 56(1): 541-545. doi: 10.7498/aps.56.541 [19] Shen Zi-Cai, Kong Wei-Jin, Liu Shi-Jie, Shen Jian, Shao Jian-Da, Fan Zheng-Xiu. Refractive index analysis of graded index coatings prepared by glancing angle deposition. Acta Physica Sinica, 2006, 55(10): 5157-5160. doi: 10.7498/aps.55.5157 [20] YIN ZONG-MIN, ZHU SONG-LAI. IMAGE PROPERTIES OF TAPERED GRADIENT-INDEX FIBER. Acta Physica Sinica, 1981, 30(12): 1603-1608. doi: 10.7498/aps.30.1603
DownLoad:
Catalog
Metrics
- Abstract views: 5722
- PDF Downloads: 80
- Cited By: 0
