Topological properties and orbital magnetism in twisted graphene systems

Liu Jian-Peng; Dai Xi

doi:10.7498/aps.69.20200506

Abstract

We review and discuss the electronic structures, topological properties and orbital magnetism in twisted bilayer (TBG) and multilayer graphene systems. Moiré pattern is formed in twisted bilayer graphene due to the mutual twist of the two graphene layers. The moiré potential induced by the twist can generate opposite pseudo magnetic fields in the Moiré supercell, which are coupled with the Dirac fermions and generate two sets of pseudo Landau levels with opposite Chern numbers $\pm1$. The two flat bands for each valley each spin of TBG are equivalent to the two zeroth pseudo Landau levels with opposite Chern numbers and opposite sublattice polarizations. Such a pseudo-Landau-level representation has significant implications on the quantum anomalous Hall states observed at integer fillings of the flat bands in TBG at the magic angle. The origin of the magic angle can also be naturally explained by using the pseudo-Landau-level picture. We further discuss twisted multilayer graphene systems, and show that topological flat bands generally exist in the twisted multilayer graphene systems. These topological flat bands have nonzero valley Chern numbers, which can be described by a succinct formula under certain approxmations. These topological flat bands in twisted bilayer and multilayer graphene systems are associated with orbital magnetism. A valley polarized state in the twist graphene system is an orbital magnetic state with nontrivial current-loop pattern in the moiré supercell. The experimentally observed correlated insulating states at $\pm 1/2$ fillings and at charge neutrality point of magic-angle TBG can be valley polarized states, which are associated with compensating current loops and induce staggered orbital magnetizations on the moiré length scale. If $C_{2z}$ symmetry is broken due to the alignment of hexagonal boron nitride substrate, then a valley-polarized ground state would be a moiré orbital ferromagnetic state, which exhibits not only (quantum) anomalous Hall effect, but also novel magneto-optical and nonlinear optical responses.

Keywords:

Authors and contacts

Liu Jian-Peng^1,2,
Dai Xi²

1.
School of Physical Science and Technology, Shanghai Tech University, Shanghai 201210, China
2.
Department of Physics, Hong Kong University of Science and Technology, Hong Kong, China

Corresponding author: Liu Jian-Peng, liujp@shanghaitech.edu.cn ; Dai Xi, daix@ust.hk

Funds: Project supported by the Hong Kong Research Grants Council Project, China (Grant No. GRF16300918

FullText HTML : translate this paragraph

References

[1]	Lopes dos Santos J M B, Peres N M R, Castro Neto A H 2007 Phys. Rev. Lett. 99 256802 Google Scholar
[2]	Mele E J 2010 Phys. Rev. B 81 161405 Google Scholar
[3]	Trambly de Laissardiere G, Mayou D, Magaud L 2010 Nano Lett. 10 804 Google Scholar
[4]	Bistritzer R, MacDonald A H 2011 Proc. Natl. Acad. Sci. 108 12233 Google Scholar
[5]	Lopes dos Santos J M B, Peres N M R, Castro Neto A H 2012 Phys. Rev. B 86 155449 Google Scholar
[6]	San-Jose P, González J, Guinea F 2012 Phys. Rev. Lett. 108 216802 Google Scholar
[7]	Cao Y, Fatemi V, Demir A, et al. 2018 Nature 556 80 Google Scholar
[8]	Cao Y, Fatemi V, Fang S, Watanabe K, Taniguchi T, Kaxiras E, Jarillo-Herrero P 2018 Nature 556 43 Google Scholar
[9]	Yankowitz M, Chen S, Polshyn H, Zhang Y, Watanabe K, Taniguchi T, Graf D, Young A F, Dean C R 2019 Science 363 1059 Google Scholar
[10]	Sharpe A L, Fox E J, Barnard A W, Finney J, Watanabe K, Taniguchi T, Kastner M A, Goldhaber-Gordon D 2019 Science 365 605 Google Scholar
[11]	Serlin M, Tschirhart C, Polshyn H, Zhang Y, Zhu J, Watanabe K, Taniguchi T, Balents L, Young A 2019 Science 367 900
[12]	Stepanov P, Das I, Lu X, Fahimniya A, Watanabe K, Taniguchi T, Koppens F H, Lischner J, Levitov L, Efetov D K 2019 arXiv preprint arXiv: 1911.09198
[13]	Po H C, Zou L, Vishwanath A, Senthil T 2018 Phys. Rev. X 8 031089
[14]	Koshino M, Yuan N F Q, Koretsune T, Ochi M, Kuroki K, Fu L 2018 Phys. Rev. X 8 031087
[15]	Kang J, Vafek O 2018 Phys. Rev. X 8 031088
[16]	Isobe H, Yuan N F Q, Fu L 2018 Phys. Rev. X 8 041041
[17]	Xu X Y, Law K T, Lee P A 2018 Phys. Rev. B 98 121406 Google Scholar
[18]	Huang T, Zhang L, Ma T 2019 Sci. Bull. 64 310 Google Scholar
[19]	Liu C C, Zhang L D, Chen W Q, Yang F 2018 Phys. Rev. Lett. 121 217001 Google Scholar
[20]	Rademaker L, Mellado P 2018 Phys. Rev. B 98 235158 Google Scholar
[21]	Venderbos J W F, Fernandes R M 2018 Phys. Rev. B 98 245103 Google Scholar
[22]	Kang J, Vafek O 2019 Phys. Rev. Lett. 122 246401 Google Scholar
[23]	Xie M, MacDonald A H 2020 Phys. Rev. Lett. 124 097601 Google Scholar
[24]	Jian C M, Xu C 2018 arXiv preprint arXiv: 1810.03610
[25]	Bultinck N, Chatterjee S, Zaletel M P 2020 Phys. Rev. Lett. 124 166601 Google Scholar
[26]	Zhang Y H, Mao D, Senthil T 2019 Phys. Rev. Res. 1 033126 Google Scholar
[27]	Liu S, Khalaf E, Lee J Y, Vishwanath A 2019 arXiv preprint arXiv: 1905.07409
[28]	Wu F, Das Sarma S 2020 Phys. Rev. Lett. 124 046403 Google Scholar
[29]	Chatterjee S, Bultinck N, Zaletel M P 2020 Phys. Rev. B 101 165141 Google Scholar
[30]	Alavirad Y, Sau J D 2019 arXiv preprint arXiv: 1907.13633
[31]	Cécile R, Dong Z H, Zhang Y H, Senthil T 2020 Phys. Rev. Lett. 124 187601 Google Scholar
[32]	Bultinck N, Khalaf E, Liu S, Chatterjee S, Vishwanath A, Zaletel M P 2019 arXiv preprent, arXiv: 1911.02045
[33]	Angeli M, Tosatti E, Fabrizio M 2019 Phys. Rev. X 9 041010
[34]	Zhang Y, Jiang K, Wang Z, Zhang F 2020 arXiv preprint arXiv: 2001.02476
[35]	Lu C, Zhang Y, Zhang Y, Zhang M, Liu C C, Gu Z C, Chen W Q, Yang F 2020 arXiv preprint arXiv: 2003.09513
[36]	Po H C, Zou L, Senthil T, Vishwanath A 2019 Phys. Rev. B 99 195455 Google Scholar
[37]	Song Z, Wang Z, Shi W, Li G, Fang C, Bernevig B A 2019 Phys. Rev. Lett. 123 036401 Google Scholar
[38]	Yuan N F Q, Fu L 2018 Phys. Rev. B 98 045103 Google Scholar
[39]	Zou L, Po H C, Vishwanath A, Senthil T 2018 Phys. Rev. B 98 085435 Google Scholar
[40]	Liu J, Dai X 2019 arXiv preprint arXiv: 1911.03760
[41]	Shen C, Chu Y, Wu Q, Li N, Wang S, Zhao Y, Tang J, Liu J, Tian J, Watanabe K, Taniguchi T, Yang R, Meng Z Y, Shi D, Yazyev O V, Zhang G 2020 Nat. Phys. 16 520 Google Scholar
[42]	Liu X, Hao Z, Khalaf E, Lee J Y, Watanabe K, Taniguchi T, Vishwanath A, Kim P 2019 arXiv preprint arXiv: 1903.08130
[43]	Cao Y, Rodan-Legrain D, Rubies-Bigorda O, Park J M, Watanabe K, Taniguchi T, Jarillo-Herrero P 2020 Nature 583 215 Google Scholar
[44]	Chen G, Sharpe A L, Fox E J, et al. 2020 Nature 579 56 Google Scholar
[45]	Chen G, Sharpe A L, Gallagher P, Rosen I T, Fox E J, Jiang L, Lyu B, Li H, Watanabe K, Taniguchi T, Jung J, Shi Z, GoldhaberGordon D, Zhang Y, Wang F 2019 Nature 572 215 Google Scholar
[46]	Chen G, Jiang L, Wu S, et al. 2019 Nat. Phys. 15 237 Google Scholar
[47]	Zhang Y H, Mao D, Cao Y, Jarillo-Herrero P, Senthil T 2019 Phys. Rev. B 99 075127 Google Scholar
[48]	Liu J, Ma Z, Gao J, Dai X 2019 Phys. Rev. X 9 031021
[49]	Lee J Y, Khalaf E, Liu S, Liu X, Hao Z, Kim P, Vishwanath A 2019 Nat. Commun. 10 5333 Google Scholar
[50]	Chebrolu N R, Chittari B L, Jung J 2019 Phys. Rev. B 99 235417 Google Scholar
[51]	Cea T, Walet N R, Guinea F 2019 Nano Lett. 19 8683 Google Scholar
[52]	Koshino M 2019 Phys. Rev. B 99 235406 Google Scholar
[53]	Ma Z, Li S, Zheng Y W, Xiao M M, Jiang H, Gao J H, Xie X 2019 arXiv preprint arXiv: 1905.00622
[54]	Haddadi F, Wu Q, Kruchkov A J, Yazyev O V 2020 Nano Lett. 20 2410 Google Scholar
[55]	Li S Y, Zhang Y, Ren Y N, Liu J, Dai X, He L 2019 arXiv preprint arXiv: 1912.13133
[56]	Liu J, Dai X 2020 npj Comput. Mater. 6 57 Google Scholar
[57]	de Gail R, Goerbig M O, Guinea F, Montambaux G, Castro Neto A H 2011 Phys. Rev. B 84 045436 Google Scholar
[58]	Uchida K, Furuya S, Iwata J I, Oshiyama A 2014 Phys. Rev. B 90 155451 Google Scholar
[59]	Liu J, Liu J, Dai X 2019 Phys. Rev. B 99 155415 Google Scholar
[60]	Jung J, Raoux A, Qiao Z, MacDonald A H 2014 Phys. Rev. B 89 205414 Google Scholar
[61]	Moon P, Koshino M 2014 Phys. Rev. B 90 155406 Google Scholar
[62]	Zhang Y H, Mao D, Senthil T 2019 arXiv preprint arXiv: 1901.08209
[63]	Ahn J, Park S, Yang B J 2019 Phys. Rev. X 9 021013
[64]	Yu R, Qi X L, Bernevig A, Fang Z, Dai X 2011 Phys. Rev. B 84 075119 Google Scholar
[65]	Soluyanov A A, Vanderbilt D 2011 Phys. Rev. B 83 235401 Google Scholar
[66]	Kane C L, Mele E J 2005 Phys. Rev. Lett. 95 146802 Google Scholar
[67]	Kane C L, Mele E J 2005 Phys. Rev. Lett. 95 226801 Google Scholar
[68]	Fu L, Kane C L 2007 Phys. Rev. B 76 045302 Google Scholar
[69]	Castro Neto A H, Guinea F, Peres N M R, Novoselov K S, Geim A K 2009 Rev. Mod. Phys. 81 109 Google Scholar
[70]	Zhang Y H, Senthil T 2019 Phys. Rev. B 99 205150 Google Scholar
[71]	Chittari B L, Chen G, Zhang Y, Wang F, Jung J 2019 Phys. Rev. Lett. 122 016401 Google Scholar
[72]	McCann E, Koshino M 2013 Rep. Prog. Phys. 76 056503 Google Scholar
[73]	Thonhauser T, Ceresoli D, Vanderbilt D, Resta R 2005 Phys. Rev. Lett. 95 137205 Google Scholar
[74]	Ceresoli D, Thonhauser T, Vanderbilt D, Resta R 2006 Phys. Rev. B 74 024408 Google Scholar
[75]	Shi J, Vignale G, Xiao D, Niu Q 2007 Phys. Rev. Lett. 99 197202 Google Scholar
[76]	Choi Y, Kemmer J, Peng Y, et al. 2019 Nat. Phys. 15 1174 Google Scholar
[77]	Jiang Y, Lai X, Watanabe K, Taniguchi T, Haule K, Mao J, Andrei E Y 2019 Nature 573 91 Google Scholar
[78]	Nagaosa N, Sinova J, Onoda S, MacDonald A, Ong N 2010 Rev. Mod. Phys. 82 1539 Google Scholar
[79]	Fukumura T, Toyosaki H, Ueno K, Nakano M, Yamasaki T, Kawasaki M 2007 Jpn. J. Appl. Phys. 46 L642 Google Scholar
[80]	Sarma S D, Pinczuk A 2008 Perspectives in Quantum Hall Effects: Novel Quantum Liquids in Low-dimensional Semiconductor Structures (Hoboken: John Wiley & Sons)
[81]	Wu F, Lovorn T, Tutuc E, Martin I, MacDonald A H 2019 Phys. Rev. Lett. 122 086402 Google Scholar
[82]	Wu F, Lovorn T, Tutuc E, MacDonald A H 2018 Phys. Rev. Lett. 121 026402 Google Scholar
[83]	Wang L, Shih E M, Ghiotto A, et al. 2019 arXiv preprint arXiv: 1910.12147
[84]	Regan E C, Wang D, Jin C, et al. 2020 Nature 579 359 Google Scholar
[85]	An L, Cai X, Huang M, Wu Z, Lin J, Ying Z, Ye Z, Feng X, Wang N 2019 arXiv preprint arXiv: 1907.03966
[86]	Zhang Y, Yuan N F, Fu L 2019 arXiv preprint arXiv: 1910.14061
[87]	Lian B, Liu Z, Zhang Y, Wang J 2020 Phys. Rev. Lett. 124 126402 Google Scholar
[88]	Hejazi K, Luo Z X, Balents L 2020 Proc. Natl. Acad. Sci. 117 10721 Google Scholar

Cited By

图 1 (a)转角双层石墨烯的摩尔条纹示意图, 插图展示两层石墨烯在不同区域层间距离的褶皱起伏; (b) 转角双层-双层石墨烯体系的示意图; (c)转角石墨烯体系的摩尔布里渊区示意图

Figure 1. (a) Schematic illustration of the moiré pattern in twisted bilayer graphene, the inserted shows the wrinkles of the graphene for different layer distances; (b) schematic illustration of twisted double bilayer graphene system; (c) moiré Brillouin zone of twisted graphene systems.

DownLoad: Full-Size Img PowerPoint

图 2 (a)转角双层石墨烯在魔角时的能带; (b)转角双层石墨烯中K谷两条平带的威尔逊圈 (以$2{\text{π}}$为单位)

Figure 2. (a) Band structures of twisted bilayer graphene at the magic angle; (b)Wilson loops of the two flat bands from the K valley of twisted bilayer graphene (in $2{\text{π}}$).

DownLoad: Full-Size Img PowerPoint

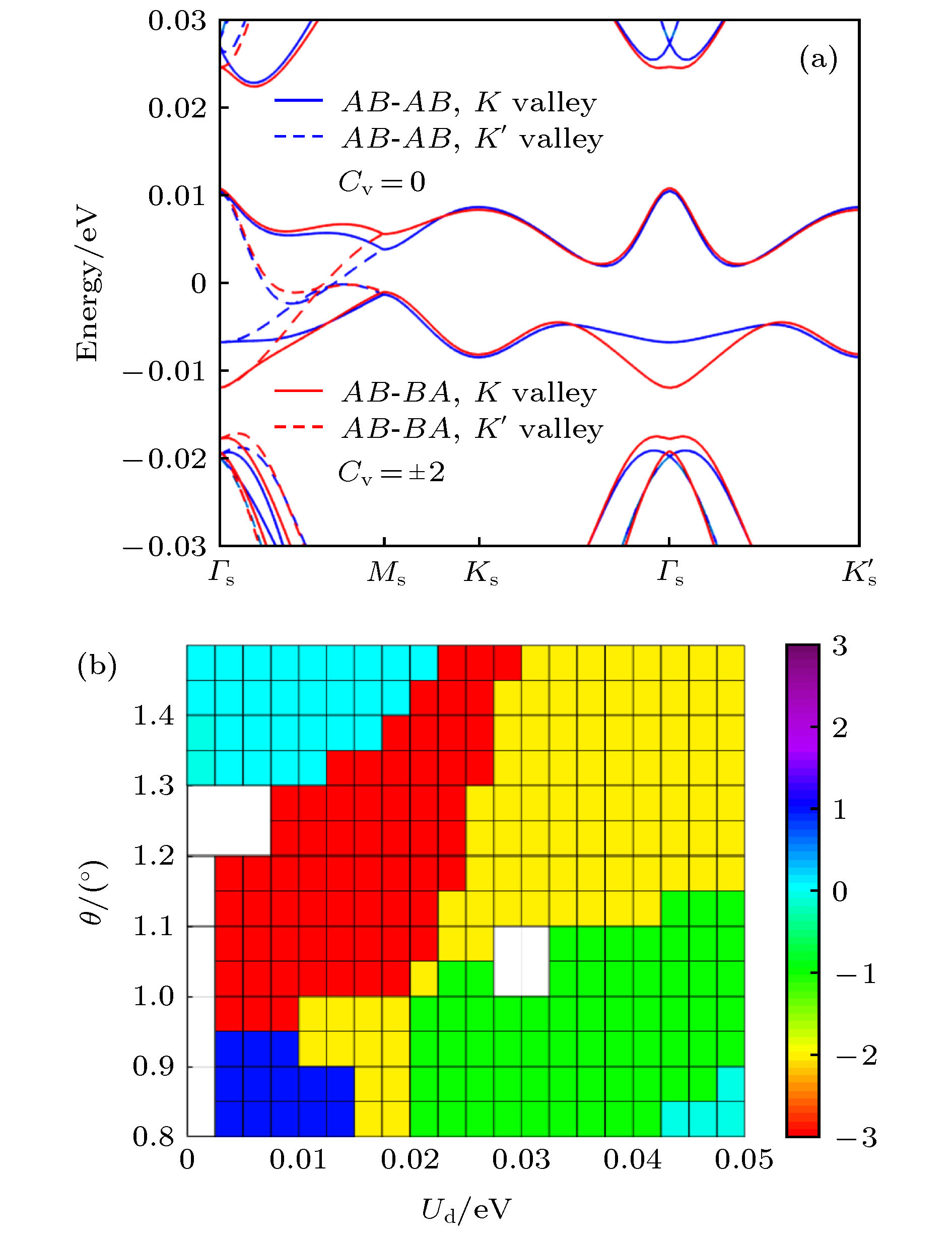
图 3 (a) 转角双层-双层石墨烯在$1.24 ^{\circ}$时的能带; (b) AB-AB堆垛的转角双层-双层石墨烯在$1.24 ^{\circ}$时K谷第一个导带陈数随转角$\theta$和垂直偏压$U_{\rm{d}}$的变化

Figure 3. (a) Band structures of twisted double bilayer graphene at $\theta=1.24^{\circ}$; (b) the Chern number of the first conduction band for the K valley of AB-AB stacked twist-ed double bilayer graphene vs. the twist angle $\theta$ and vertical potential drop $U_{\rm{d}}$.

DownLoad: Full-Size Img PowerPoint

图 4 魔角双层石墨烯平带对应的实空间电流密度分布　(a) K谷, $\varDelta_{\rm M}=0$; (b) $K'$谷, $\varDelta_{\rm M}=0$; (c) K谷, $\varDelta_{\rm M}=15\, {\rm{meV}}$; (d) $K'$谷, $\varDelta_{\rm M}=15\, {\rm{meV}}$, 图中黑色箭头代表电流方向, 颜色编码表示电流诱导的磁场强度, 单位为T

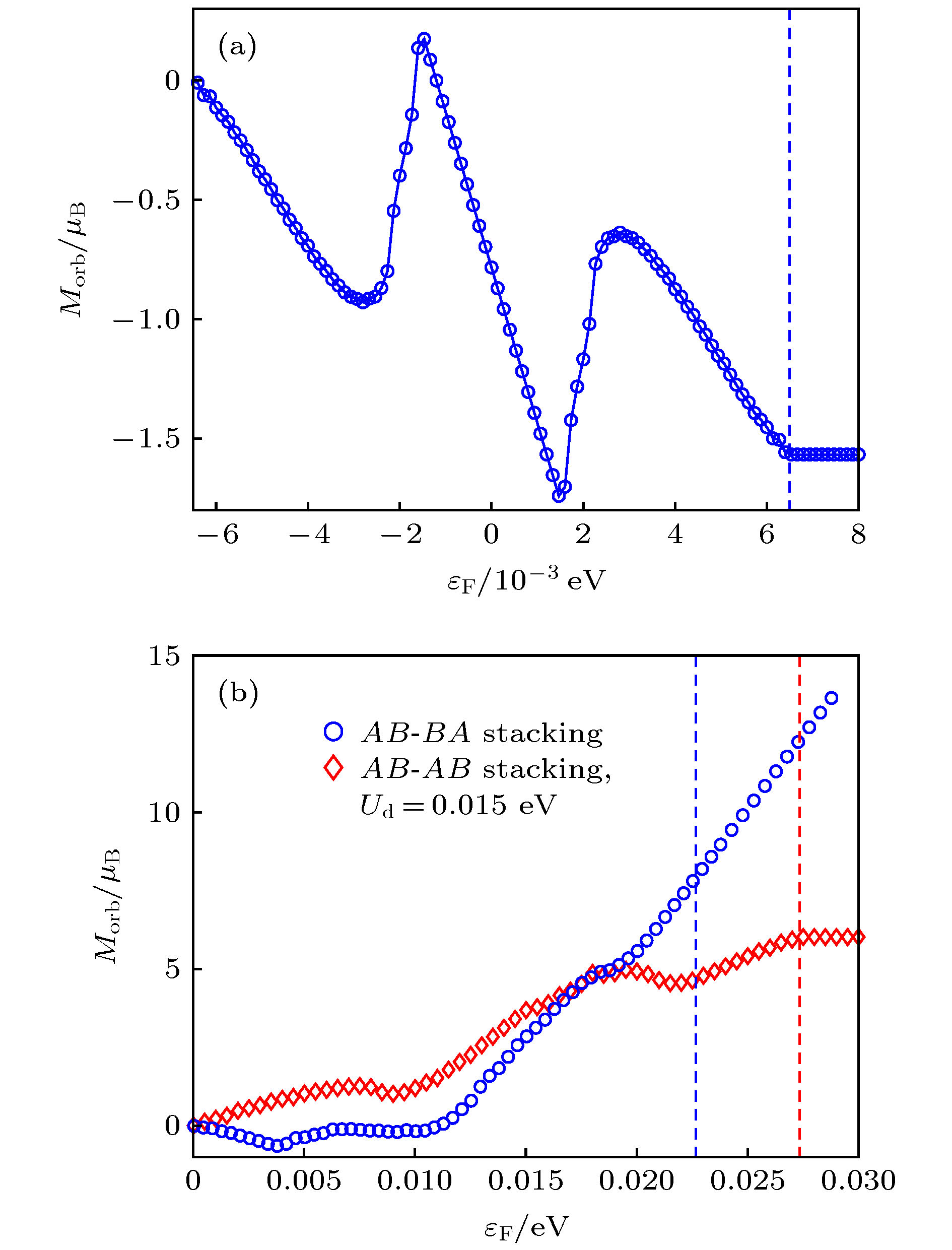
Figure 4. Real-space current-density distribution contributed by the flat bands of magic-angle twisted bilayer graphene: (a) K valley, $\varDelta_{\rm M}=0$; (b) $K'$ valley, $\varDelta_{\rm M}=0$; (c) K valley, $\varDelta_{\rm M}=15\, {\rm{meV}}$; (d) $K'$ valley, $\varDelta_{\rm M}=15\, {\rm{meV}}$. The black arrows indicate the dire-ctions of the current density, and the color coding indicates the magnetic field induced by the current in unites of Tesla.

DownLoad: Full-Size Img PowerPoint

图 5 (a)转角双层石墨烯在魔角时K谷平带贡献的轨道磁矩($M_ {\rm {orb}}$)随化学势 ($\varepsilon_{\rm{F}}$)的变化; (b)转角双层-双层石墨烯在$\theta=1.24^ {\circ}$时K谷平带贡献的轨道磁矩随化学势的变化

Figure 5. (a) The orbital magnetization contributed by the flat bands of the K valley of twisted bilayer graphene at the magic angle; (b) the orbital magnetization contributed by the flat bands of K valley for twisted double bilayer graphene at $\theta=1.24^{\circ}$.

DownLoad: Full-Size Img PowerPoint

表 1 由赝朗道能级图像推算出的魔角

Table 1. Magic angles derived from the pseudo Landau-level picture.

n 1 2 3 4 5

文献[4] 1.05° 0.50° 0.35° 0.24° 0.20°
(7)式 1.05° 0.52° 0.35° 0.26° 0.21°

DownLoad: CSV

map

[1]	Lopes dos Santos J M B, Peres N M R, Castro Neto A H 2007 Phys. Rev. Lett. 99 256802 Google Scholar
[2]	Mele E J 2010 Phys. Rev. B 81 161405 Google Scholar
[3]	Trambly de Laissardiere G, Mayou D, Magaud L 2010 Nano Lett. 10 804 Google Scholar
[4]	Bistritzer R, MacDonald A H 2011 Proc. Natl. Acad. Sci. 108 12233 Google Scholar
[5]	Lopes dos Santos J M B, Peres N M R, Castro Neto A H 2012 Phys. Rev. B 86 155449 Google Scholar
[6]	San-Jose P, González J, Guinea F 2012 Phys. Rev. Lett. 108 216802 Google Scholar
[7]	Cao Y, Fatemi V, Demir A, et al. 2018 Nature 556 80 Google Scholar
[8]	Cao Y, Fatemi V, Fang S, Watanabe K, Taniguchi T, Kaxiras E, Jarillo-Herrero P 2018 Nature 556 43 Google Scholar
[9]	Yankowitz M, Chen S, Polshyn H, Zhang Y, Watanabe K, Taniguchi T, Graf D, Young A F, Dean C R 2019 Science 363 1059 Google Scholar
[10]	Sharpe A L, Fox E J, Barnard A W, Finney J, Watanabe K, Taniguchi T, Kastner M A, Goldhaber-Gordon D 2019 Science 365 605 Google Scholar
[11]	Serlin M, Tschirhart C, Polshyn H, Zhang Y, Zhu J, Watanabe K, Taniguchi T, Balents L, Young A 2019 Science 367 900
[12]	Stepanov P, Das I, Lu X, Fahimniya A, Watanabe K, Taniguchi T, Koppens F H, Lischner J, Levitov L, Efetov D K 2019 arXiv preprint arXiv: 1911.09198
[13]	Po H C, Zou L, Vishwanath A, Senthil T 2018 Phys. Rev. X 8 031089
[14]	Koshino M, Yuan N F Q, Koretsune T, Ochi M, Kuroki K, Fu L 2018 Phys. Rev. X 8 031087
[15]	Kang J, Vafek O 2018 Phys. Rev. X 8 031088
[16]	Isobe H, Yuan N F Q, Fu L 2018 Phys. Rev. X 8 041041
[17]	Xu X Y, Law K T, Lee P A 2018 Phys. Rev. B 98 121406 Google Scholar
[18]	Huang T, Zhang L, Ma T 2019 Sci. Bull. 64 310 Google Scholar
[19]	Liu C C, Zhang L D, Chen W Q, Yang F 2018 Phys. Rev. Lett. 121 217001 Google Scholar
[20]	Rademaker L, Mellado P 2018 Phys. Rev. B 98 235158 Google Scholar
[21]	Venderbos J W F, Fernandes R M 2018 Phys. Rev. B 98 245103 Google Scholar
[22]	Kang J, Vafek O 2019 Phys. Rev. Lett. 122 246401 Google Scholar
[23]	Xie M, MacDonald A H 2020 Phys. Rev. Lett. 124 097601 Google Scholar
[24]	Jian C M, Xu C 2018 arXiv preprint arXiv: 1810.03610
[25]	Bultinck N, Chatterjee S, Zaletel M P 2020 Phys. Rev. Lett. 124 166601 Google Scholar
[26]	Zhang Y H, Mao D, Senthil T 2019 Phys. Rev. Res. 1 033126 Google Scholar
[27]	Liu S, Khalaf E, Lee J Y, Vishwanath A 2019 arXiv preprint arXiv: 1905.07409
[28]	Wu F, Das Sarma S 2020 Phys. Rev. Lett. 124 046403 Google Scholar
[29]	Chatterjee S, Bultinck N, Zaletel M P 2020 Phys. Rev. B 101 165141 Google Scholar
[30]	Alavirad Y, Sau J D 2019 arXiv preprint arXiv: 1907.13633
[31]	Cécile R, Dong Z H, Zhang Y H, Senthil T 2020 Phys. Rev. Lett. 124 187601 Google Scholar
[32]	Bultinck N, Khalaf E, Liu S, Chatterjee S, Vishwanath A, Zaletel M P 2019 arXiv preprent, arXiv: 1911.02045
[33]	Angeli M, Tosatti E, Fabrizio M 2019 Phys. Rev. X 9 041010
[34]	Zhang Y, Jiang K, Wang Z, Zhang F 2020 arXiv preprint arXiv: 2001.02476
[35]	Lu C, Zhang Y, Zhang Y, Zhang M, Liu C C, Gu Z C, Chen W Q, Yang F 2020 arXiv preprint arXiv: 2003.09513
[36]	Po H C, Zou L, Senthil T, Vishwanath A 2019 Phys. Rev. B 99 195455 Google Scholar
[37]	Song Z, Wang Z, Shi W, Li G, Fang C, Bernevig B A 2019 Phys. Rev. Lett. 123 036401 Google Scholar
[38]	Yuan N F Q, Fu L 2018 Phys. Rev. B 98 045103 Google Scholar
[39]	Zou L, Po H C, Vishwanath A, Senthil T 2018 Phys. Rev. B 98 085435 Google Scholar
[40]	Liu J, Dai X 2019 arXiv preprint arXiv: 1911.03760
[41]	Shen C, Chu Y, Wu Q, Li N, Wang S, Zhao Y, Tang J, Liu J, Tian J, Watanabe K, Taniguchi T, Yang R, Meng Z Y, Shi D, Yazyev O V, Zhang G 2020 Nat. Phys. 16 520 Google Scholar
[42]	Liu X, Hao Z, Khalaf E, Lee J Y, Watanabe K, Taniguchi T, Vishwanath A, Kim P 2019 arXiv preprint arXiv: 1903.08130
[43]	Cao Y, Rodan-Legrain D, Rubies-Bigorda O, Park J M, Watanabe K, Taniguchi T, Jarillo-Herrero P 2020 Nature 583 215 Google Scholar
[44]	Chen G, Sharpe A L, Fox E J, et al. 2020 Nature 579 56 Google Scholar
[45]	Chen G, Sharpe A L, Gallagher P, Rosen I T, Fox E J, Jiang L, Lyu B, Li H, Watanabe K, Taniguchi T, Jung J, Shi Z, GoldhaberGordon D, Zhang Y, Wang F 2019 Nature 572 215 Google Scholar
[46]	Chen G, Jiang L, Wu S, et al. 2019 Nat. Phys. 15 237 Google Scholar
[47]	Zhang Y H, Mao D, Cao Y, Jarillo-Herrero P, Senthil T 2019 Phys. Rev. B 99 075127 Google Scholar
[48]	Liu J, Ma Z, Gao J, Dai X 2019 Phys. Rev. X 9 031021
[49]	Lee J Y, Khalaf E, Liu S, Liu X, Hao Z, Kim P, Vishwanath A 2019 Nat. Commun. 10 5333 Google Scholar
[50]	Chebrolu N R, Chittari B L, Jung J 2019 Phys. Rev. B 99 235417 Google Scholar
[51]	Cea T, Walet N R, Guinea F 2019 Nano Lett. 19 8683 Google Scholar
[52]	Koshino M 2019 Phys. Rev. B 99 235406 Google Scholar
[53]	Ma Z, Li S, Zheng Y W, Xiao M M, Jiang H, Gao J H, Xie X 2019 arXiv preprint arXiv: 1905.00622
[54]	Haddadi F, Wu Q, Kruchkov A J, Yazyev O V 2020 Nano Lett. 20 2410 Google Scholar
[55]	Li S Y, Zhang Y, Ren Y N, Liu J, Dai X, He L 2019 arXiv preprint arXiv: 1912.13133
[56]	Liu J, Dai X 2020 npj Comput. Mater. 6 57 Google Scholar
[57]	de Gail R, Goerbig M O, Guinea F, Montambaux G, Castro Neto A H 2011 Phys. Rev. B 84 045436 Google Scholar
[58]	Uchida K, Furuya S, Iwata J I, Oshiyama A 2014 Phys. Rev. B 90 155451 Google Scholar
[59]	Liu J, Liu J, Dai X 2019 Phys. Rev. B 99 155415 Google Scholar
[60]	Jung J, Raoux A, Qiao Z, MacDonald A H 2014 Phys. Rev. B 89 205414 Google Scholar
[61]	Moon P, Koshino M 2014 Phys. Rev. B 90 155406 Google Scholar
[62]	Zhang Y H, Mao D, Senthil T 2019 arXiv preprint arXiv: 1901.08209
[63]	Ahn J, Park S, Yang B J 2019 Phys. Rev. X 9 021013
[64]	Yu R, Qi X L, Bernevig A, Fang Z, Dai X 2011 Phys. Rev. B 84 075119 Google Scholar
[65]	Soluyanov A A, Vanderbilt D 2011 Phys. Rev. B 83 235401 Google Scholar
[66]	Kane C L, Mele E J 2005 Phys. Rev. Lett. 95 146802 Google Scholar
[67]	Kane C L, Mele E J 2005 Phys. Rev. Lett. 95 226801 Google Scholar
[68]	Fu L, Kane C L 2007 Phys. Rev. B 76 045302 Google Scholar
[69]	Castro Neto A H, Guinea F, Peres N M R, Novoselov K S, Geim A K 2009 Rev. Mod. Phys. 81 109 Google Scholar
[70]	Zhang Y H, Senthil T 2019 Phys. Rev. B 99 205150 Google Scholar
[71]	Chittari B L, Chen G, Zhang Y, Wang F, Jung J 2019 Phys. Rev. Lett. 122 016401 Google Scholar
[72]	McCann E, Koshino M 2013 Rep. Prog. Phys. 76 056503 Google Scholar
[73]	Thonhauser T, Ceresoli D, Vanderbilt D, Resta R 2005 Phys. Rev. Lett. 95 137205 Google Scholar
[74]	Ceresoli D, Thonhauser T, Vanderbilt D, Resta R 2006 Phys. Rev. B 74 024408 Google Scholar
[75]	Shi J, Vignale G, Xiao D, Niu Q 2007 Phys. Rev. Lett. 99 197202 Google Scholar
[76]	Choi Y, Kemmer J, Peng Y, et al. 2019 Nat. Phys. 15 1174 Google Scholar
[77]	Jiang Y, Lai X, Watanabe K, Taniguchi T, Haule K, Mao J, Andrei E Y 2019 Nature 573 91 Google Scholar
[78]	Nagaosa N, Sinova J, Onoda S, MacDonald A, Ong N 2010 Rev. Mod. Phys. 82 1539 Google Scholar
[79]	Fukumura T, Toyosaki H, Ueno K, Nakano M, Yamasaki T, Kawasaki M 2007 Jpn. J. Appl. Phys. 46 L642 Google Scholar
[80]	Sarma S D, Pinczuk A 2008 Perspectives in Quantum Hall Effects: Novel Quantum Liquids in Low-dimensional Semiconductor Structures (Hoboken: John Wiley & Sons)
[81]	Wu F, Lovorn T, Tutuc E, Martin I, MacDonald A H 2019 Phys. Rev. Lett. 122 086402 Google Scholar
[82]	Wu F, Lovorn T, Tutuc E, MacDonald A H 2018 Phys. Rev. Lett. 121 026402 Google Scholar
[83]	Wang L, Shih E M, Ghiotto A, et al. 2019 arXiv preprint arXiv: 1910.12147
[84]	Regan E C, Wang D, Jin C, et al. 2020 Nature 579 359 Google Scholar
[85]	An L, Cai X, Huang M, Wu Z, Lin J, Ying Z, Ye Z, Feng X, Wang N 2019 arXiv preprint arXiv: 1907.03966
[86]	Zhang Y, Yuan N F, Fu L 2019 arXiv preprint arXiv: 1910.14061
[87]	Lian B, Liu Z, Zhang Y, Wang J 2020 Phys. Rev. Lett. 124 126402 Google Scholar
[88]	Hejazi K, Luo Z X, Balents L 2020 Proc. Natl. Acad. Sci. 117 10721 Google Scholar

[1]	Qin Zhi-Jie, Zhang Hui-Qing, Zhang Guang-Ping, Ren Jun-Feng, Wang Chuan-Kui, Hu Gui-Chao, Qiu Shuai. Theoretical study of introducing spin into nonmagnetic graphene-based single-molecule junction by edge modifications. Acta Physica Sinica, 2023, 72(13): 138504. doi: 10.7498/aps.72.20230267
[2]	Wang Huan, He Chun-Juan, Xu Sheng, Wang Yi-Yan, Zeng Xiang-Yu, Lin Jun-Fa, Wang Xiao-Yan, Gong Jing, Ma Xiao-Ping, Han Kun, Wang Yi-Ting, Xia Tian-Long. Single crystal growth of topological semimetals and magnetic topological materials. Acta Physica Sinica, 2023, 72(3): 038103. doi: 10.7498/aps.72.20221574
[3]	Liu Yi-Jun, Chen Yi-Wei, Zhu Yu-Jian, Huang Yan, An Dong-Dong, Li Qing-Xin, Gan Qi-Kang, Zhu Wang, Song Jun-Wei, Wang Kai-Yuan, Wei Ling-Nan, Zong Qi-Jun, Liu Shuo-Han, Li Shi-Wei, Liu Zhi, Zhang Qi, Xu Ying-Hai, Cao Xin-Yu, Yang Ao, Wang Hao-Lin, Yang Bing, Andy Shen, Yu Ge-Liang, Wang Lei. Isospin polarized Chern insulator state of C = 4 in twisted double bilayer graphene. Acta Physica Sinica, 2023, 72(14): 147303. doi: 10.7498/aps.72.20230497
[4]	Bai Zhan-Bin, Wang Rui, Zhou Ya-Zhou, Wu Tian-Ru, Ge Jian-Lei, Li Jing, Qin Yu-Yuan, Fei Fu-Cong, Cao Lu, Wang Xue-Feng, Wang Xin-Ran, Zhang Shuai, Sun Li-Ling, Song You, Song Feng-Qi. Selective enhancement of Kane Mele-type spin-orbit interaction in graphene. Acta Physica Sinica, 2022, 71(6): 067202. doi: 10.7498/aps.71.20211815
[5]	Zheng Yu-Qiang, Wang Shi-Yong. Delocalized magnetism in low-dimensional graphene system. Acta Physica Sinica, 2022, 71(18): 188101. doi: 10.7498/aps.71.20220895
[6]	. Mimicing the Kane-Mele type spin orbit interaction by spin-flexual phonon coupling in graphene devices. Acta Physica Sinica, 2021, (): . doi: 10.7498/aps.70.20211815
[7]	Cai Xiao-Xiao, Luo Guo-Yu, Li Zhi-Qiang, He Yan. Optical conductivity of twisted bilayer graphene under heterostrain. Acta Physica Sinica, 2021, 70(18): 187301. doi: 10.7498/aps.70.20210110
[8]	Liu Yu-Ting, He Wen-Yu, Liu Jun-Wei, Shao Qi-Ming. Berry curvature-induced emerging magnetic response in two-dimensional materials. Acta Physica Sinica, 2021, 70(12): 127303. doi: 10.7498/aps.70.20202132
[9]	Xu Xian-Da, Zhao Lei, Sun Wei-Feng. First-principles on the energy band mechanism for modifying conduction property of graphene nanomeshes. Acta Physica Sinica, 2020, 69(4): 047101. doi: 10.7498/aps.69.20190657
[10]	Xi Xiang, Ye Kang-Ping, Wu Rui-Xin. Influence of bias magnetic field direction on band structure of magnetic photonic crystal and its role in constructing topological edge states. Acta Physica Sinica, 2020, 69(15): 154102. doi: 10.7498/aps.69.20200198
[11]	Lü Xin-Yu, Li Zhi-Qiang. Topological properties of graphene moiré superlattice systems and recent optical studies. Acta Physica Sinica, 2019, 68(22): 220303. doi: 10.7498/aps.68.20191317
[12]	Zhang Hua-Lin, Sun Lin, Wang Ding. Electromagnetic properties of zigzag graphene nanoribbons with single-row line defect. Acta Physica Sinica, 2016, 65(1): 016101. doi: 10.7498/aps.65.016101
[13]	Chen Dong-Hai, Yang Mou, Duan Hou-Jian, Wang Rui-Qiang. Electronic transport properties of graphene pn junctions with spin-orbit coupling. Acta Physica Sinica, 2015, 64(9): 097201. doi: 10.7498/aps.64.097201
[14]	Xu Lei, Dai Zhen-Hong, Sui Peng-Fei, Wang Wei-Tian, Sun Yu-Ming. Electronic and magnetic properties of fluorinated graphene sheets with divacancy substitutional doping. Acta Physica Sinica, 2014, 63(18): 186101. doi: 10.7498/aps.63.186101
[15]	Gao Tan-Hua. Magnetic and electronic properties of fluorographene sheet with foreign atom substitutions. Acta Physica Sinica, 2014, 63(4): 046102. doi: 10.7498/aps.63.046102
[16]	Jin Feng, Zhang Zhen-Hua, Wang Cheng-Zhi, Deng Xiao-Qing, Fan Zhi-Qiang. Twisting effects on energy band structures and transmission behaviors of graphene nanoribbons. Acta Physica Sinica, 2013, 62(3): 036103. doi: 10.7498/aps.62.036103
[17]	Lin Qi, Chen Yu-Hang, Wu Jian-Bao, Kong Zong-Min. Effect of N-doping on band structure and transport property of zigzag graphene nanoribbons. Acta Physica Sinica, 2011, 60(9): 097103. doi: 10.7498/aps.60.097103
[18]	Gao Shuang-Hong, Ren Zhao-Yu, Guo Ping, Zheng Ji-Ming, Du Gong-He, Wan Li-Juan, Zheng Lin-Lin. Magnetic properties and excited states of thegraphene quantum dots. Acta Physica Sinica, 2011, 60(4): 047105. doi: 10.7498/aps.60.047105
[19]	Wang Xue-Mei, Liu Hong. Band structures of zigzag graphene nanoribbons. Acta Physica Sinica, 2011, 60(4): 047102. doi: 10.7498/aps.60.047102
[20]	Wang Yong-Long, Pan Hong-Zhe, Xu Ming, Chen Li, Sun Yuan-Yuan. Electronic structure and magnetism of single-layer trigonal graphene quantum dots with zigzag edges. Acta Physica Sinica, 2010, 59(9): 6443-6449. doi: 10.7498/aps.59.6443

Catalog

Vol. 69, Issue 14 - 2020-07-20

Metrics

Abstract views: 16851
PDF Downloads: 1415
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板