-
Twisted bilayer graphene (TBG) is a two-dimensional material composed of two layers stacked at a certain angle. When the twisted angle decreases, the lattice mismatch between two layers produces moiré pattern at a long wavelength which significantly modifies the low-energy band structure. In particular, when the twisted angle is close to the so-called “magic angle”, two moiré flat bands are formed near a charge neutral point due to the strong interlayer coupling. These flat bands with high density of states are essential in realizing superconductivity and correlated insulating states. More recently, the magic angle TBG combining an hBN system has exhibited spin-valley polarization when 3/4 of flat bands are filled, thereby providing an ideal platform to achieve quantum anomalous Hall states. Whether it is TBG system or TBG-hBN system, the flat band becomes a crucial condition for discovering so rich physical connotations. Besides the twisted angle, the strain gives an alternative way to modulate flat bands. It has been reported that applying heterostrain in magic angle TBG can makes flat moiré band tunable; strain can also generate flat bands in non-magic angle TBG. Moreover, the reconstruction of TBG due to the strain gives rise to a serial of novel physical phenomena such as topological protected soliton and photonic crystal. Another reason for studying strain effect is that the strain is ubiquitous in the fabrication progress. The strain can also be controlled via piezoelectric substrate which makes possible the in situ modulation of correlated states, topology and quantum effect. Our work is to study the heterostrain effect in TBG band structure and optical conductivity by using a continuum model. Although the resulting conduction band and valence bands keep connected through Dirac points protected by the C2 symmetry, their separation increases significantly when heterostrain is applied while the Dirac point is also shifted. The optical conductivity is characterized by a series of peaks associated with van Hove singularities, and the peak energies are systematically shifted with the strain amplitude. These changes show that the heterostrain exerts a great influence on electron property of TBG. -
Keywords:
- twisted bilayer graphene /
- heterostrain /
- optical conductivity
[1] 卢晓波, 张广宇 2015 64 077305
Google Scholar
Lu X B, Zhang G Y 2015 Acta Phys. Sin. 64 077305
Google Scholar
[2] Cao Y, Fatemi V, Demir A, Fang S, Tomarken S L, Luo J Y, Sanchez-Yamagishi J D, Watanabe K, Taniguchi T, Kaxiras E 2018 Nature 556 80
Google Scholar
[3] Cao Y, Fatemi V, Fang S, Watanabe K, Taniguchi T, Kaxiras E, Jarillo-Herrero P 2018 Nature 556 43
Google Scholar
[4] Xia L, Kennes D M, Tancogne-Dejean N, Altarelli M, Rubio A 2019 Nano Lett. 19 4934
Google Scholar
[5] Zhang Y H, Mao D, Cao Y, Jarillo-Herrero P, Senthil T 2019 Phys. Rev. B 99 075127
Google Scholar
[6] Zhang Y H, Mao M, Senthil T 2019 Phys. Rev. Research 1 033126
Google Scholar
[7] Sharpe A L, Fox E J, Barnard A W, Finney J, Watanabe K, Taniguchi T, Kastner M A, Goldhaber-Gordon D 2019 Science 365 605
Google Scholar
[8] Serlin M, Tschirhart C L, Polshyn H, Zhang Y, Zhu J, Watanabe K, Taniguchi T, Balents L, Young A F 2020 Science 367 900
Google Scholar
[9] Huder L, Artaud A, Quang T L, Laissardière G T D, Jansen A G M, Lapertot G, Chapelier C, Renard V T 2018 Phys. Rev. Lett. 120 156405
Google Scholar
[10] Bi Z, Yuan N F Q, Fu L 2019 Phys. Rev. B 100 035448
Google Scholar
[11] Alden J S, Tsen A W, Huang P Y, Hovden R, Brown L, Park J, Muller D A, McEuen P L 2013 PNAS 110 11256
Google Scholar
[12] Sunku S S, Ni G X, Jiang B Y, Yoo H, Sternbach A, McLeod A S, Stauber T, Xiong L, Taniguchi T, Watanabe K, Kim P, M. Fogler M M, Basov D N 2018 Science 362 1153
Google Scholar
[13] Wen L, Li Z Q, He Y 2021 Chin. Phys. B 30 017303
Google Scholar
[14] Kerelsky A, McGilly L, Kennes D M, Xian L, Yankowitz M, Chen S, Watanabe K, Taniguchi T, Hone J, Dean C 2019 Nature 572 95
Google Scholar
[15] Lopes dos Santos J M B, Peres N M R, Castro Neto A H 2007 Phys. Rev. Lett. 99 256802
Google Scholar
[16] Koshino M, Yuan N F Q, Koretsune T, Ochi M, Kuroki K, Fu L 2018 Phys. Rev. X 8 031087
[17] McCann E, Koshino M 2013 Rep. Prog. Phys. 76 056503
Google Scholar
[18] Moon P, Koshino M 2013 Phys. Rev. B 87 205404
Google Scholar
[19] Po H C, Zou L J, Vishwanath A, Senthil T 2018 Phys. Rev. X 8 031089
[20] 吕新宇, 李志强 2019 68 220303
Google Scholar
Lü X Y, Li Z Q 2019 Acta Phys. Sin. 68 220303
Google Scholar
-
图 1 TBG在转角为5°时的结构示意图, 其莫尔周期结构清晰可见
Figure 1. Schematic of TBG structure at θ = 5°, the resulted moiré pattern can be clearly seen.
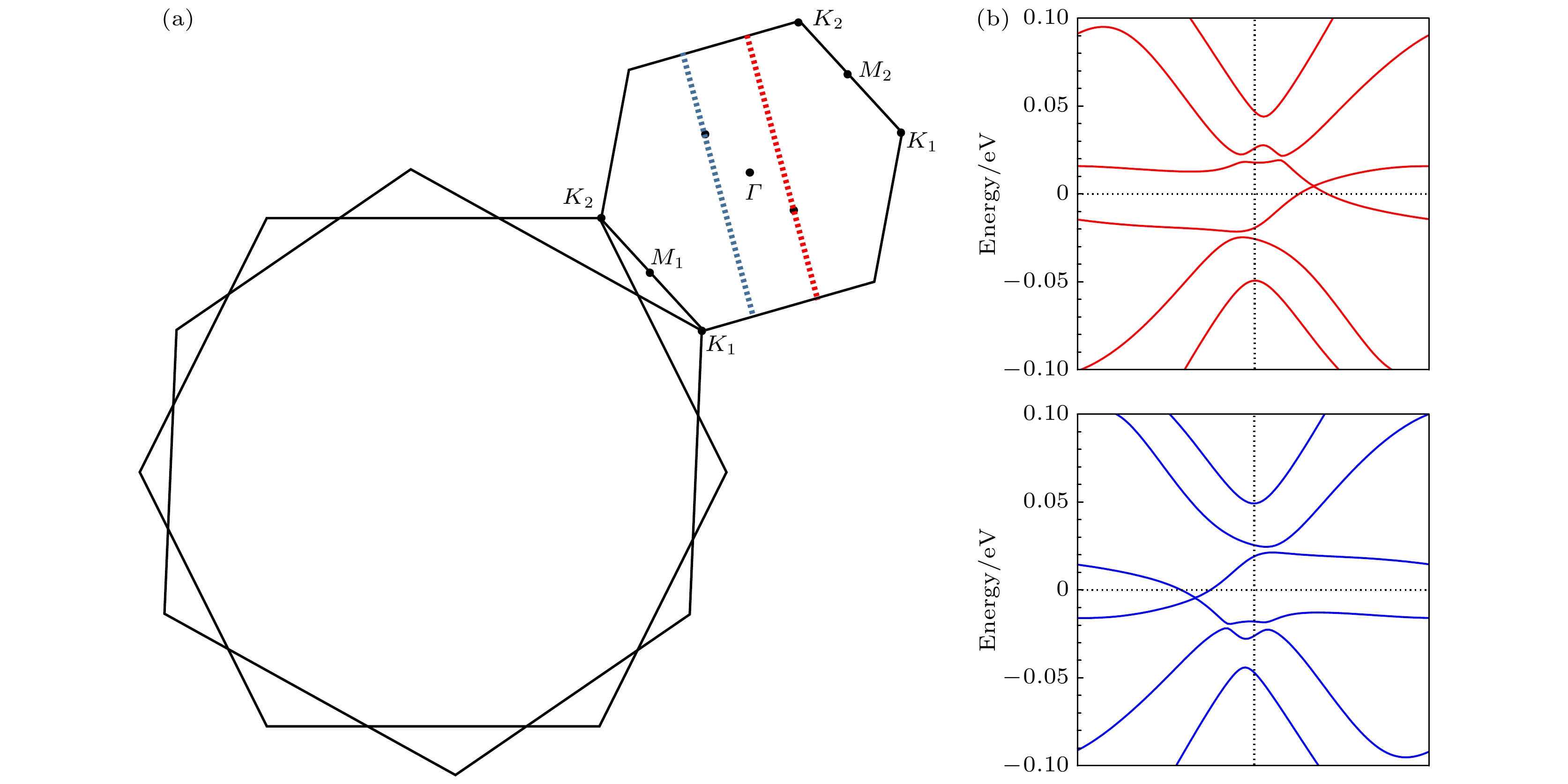
图 2 (a) TBG莫尔布里渊区示意图: 两个大正六边形代表上下两层单层石墨烯的第一布里渊区, 小正六边形为转角形成的莫尔布里渊区; (b) 偏移后的狄拉克点附近能带: 红色和蓝色曲线分别代表图(a)中莫尔布里渊区同色虚线路径的能带, 虚线路径中的黑点代表狄拉克点的位置
Figure 2. (a) Schematic of TBG moiré Brillouin zone: The two large regular hexagons represent the first Brillouin zone of the upper and lower graphene layers, the small regular hexagons refer to the moiré Brillouin zone. (b) The band structures near the shifted Dirac points: Red and blue curve lines represent the band structures follow the same colored dashed lines path in the panel (a) respectively, the Dirac points are marked by the black dots in dash lines.
图 3 TBG的能带 (a)—(c) TBG在转角为1.05°, 应变大小分别为0%, 3%和6%时的能带; (d)—(f) TBG在转角为1.47°, 应变大小分别为0%, 3%和6%时的能带
Figure 3. Band structures of TBG: (a)−(c) The band structures with 0%, 0.3%, 0.6% uniaxial heterostrain at twisted angle θ = 1.05°, respectively; (d)−(f) the band structures with 0%, 0.3%, 0.6% uniaxial heterostrain of at twisted angle θ = 1.47°, respectively.
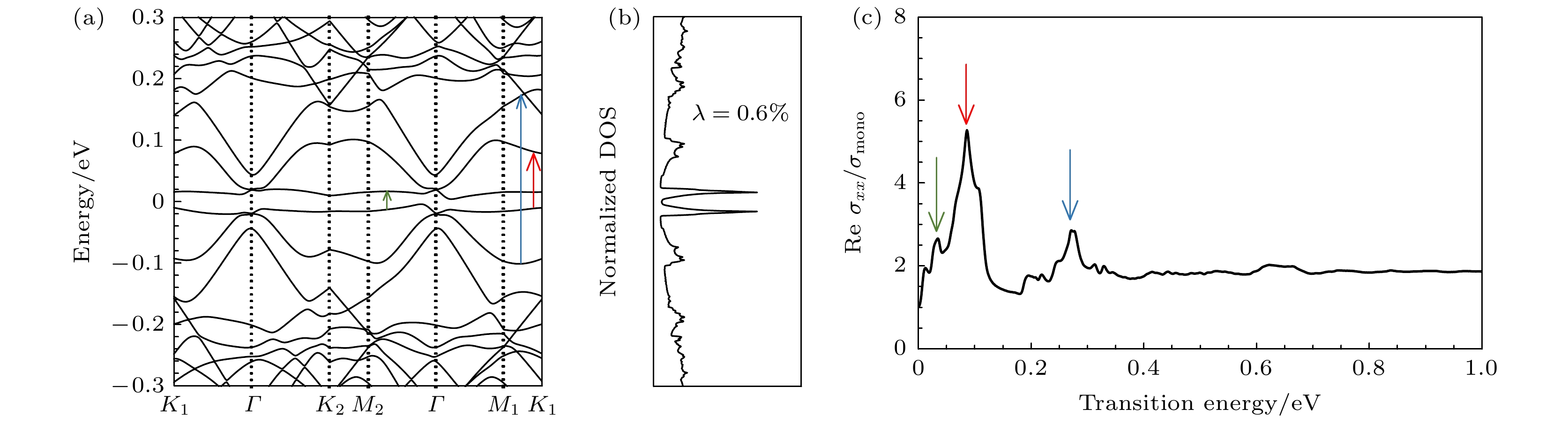
图 4 TBG在转角为1.05°、施加0.6%大小的应变时的能带(a)、态密度(b)以及光电导(c), 图(c)中绿色、红色与蓝色箭头对应的吸收峰分别对应于图(a)中的同色箭头代表的带间跃迁
Figure 4. (a) Band structure, (b) density of states and (c) corresponding optical conductivity of TBG with 0.6% uniaxial heterostrain at 1.05°. The green, red and blue arrows in panel (c) correspond to the interband transition marked with arrows of the same color in panel (a).
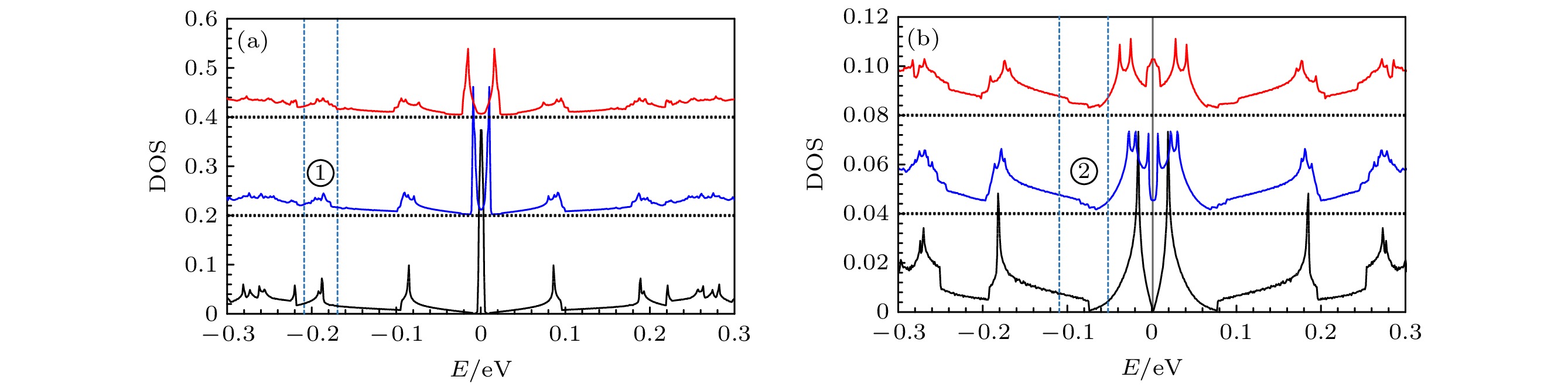
图 5 TBG的态密度 (a) TBG在转角为1.05°, 应变大小分别为0% (黑色), 0.3% (蓝色), 0.6% (红色)时的态密度; (b) TBG在转角为1.47°, 应变大小分别为0% (黑色), 0.3% (蓝色), 0.6% (红色)时的态密度; 蓝色虚线表示正文中所讨论的①, ②区域的边界
Figure 5. (a) Density of states (DOS) of TBG with 0% (black curve), 0.3% (blue curve) and 0.6% (red curve) uniaxial heterostrain at 1.05°; (b) DOS of TBG with 0% (black curve), 0.3% (blue curve) and 0.6% (red curve) uniaxial heterostrain at 1.47°. Blue dash lines in panel (a) and (b) represent the boundary of ①, ② region, respectively.
图 6 TBG的光电导 (a) TBG在转角为1.05°, 应变大小分别为0% (黑色), 0.3% (蓝色), 0.6% (红色)时的光电导; (b) TBG在转角为1.47°, 应变大小分别为0% (黑色), 0.3% (蓝色), 0.6% (红色)时的光电导; 蓝色虚线表示正文中所讨论的①, ②区域的边界
Figure 6. (a) Optical conductivity of TBG with 0% (black curve), 0.3% (blue curve) and 0.6% (red curve) uniaxial heterostrain at 1.05°; (b) optical conductivity of TBG with 0% (black curve), 0.3% (blue curve) and 0.6% (red curve) uniaxial heterostrain at 1.47°. Blue dash lines in panel (a) and (b) represent the boundary of ①, ② region, respectively.
-
[1] 卢晓波, 张广宇 2015 64 077305
Google Scholar
Lu X B, Zhang G Y 2015 Acta Phys. Sin. 64 077305
Google Scholar
[2] Cao Y, Fatemi V, Demir A, Fang S, Tomarken S L, Luo J Y, Sanchez-Yamagishi J D, Watanabe K, Taniguchi T, Kaxiras E 2018 Nature 556 80
Google Scholar
[3] Cao Y, Fatemi V, Fang S, Watanabe K, Taniguchi T, Kaxiras E, Jarillo-Herrero P 2018 Nature 556 43
Google Scholar
[4] Xia L, Kennes D M, Tancogne-Dejean N, Altarelli M, Rubio A 2019 Nano Lett. 19 4934
Google Scholar
[5] Zhang Y H, Mao D, Cao Y, Jarillo-Herrero P, Senthil T 2019 Phys. Rev. B 99 075127
Google Scholar
[6] Zhang Y H, Mao M, Senthil T 2019 Phys. Rev. Research 1 033126
Google Scholar
[7] Sharpe A L, Fox E J, Barnard A W, Finney J, Watanabe K, Taniguchi T, Kastner M A, Goldhaber-Gordon D 2019 Science 365 605
Google Scholar
[8] Serlin M, Tschirhart C L, Polshyn H, Zhang Y, Zhu J, Watanabe K, Taniguchi T, Balents L, Young A F 2020 Science 367 900
Google Scholar
[9] Huder L, Artaud A, Quang T L, Laissardière G T D, Jansen A G M, Lapertot G, Chapelier C, Renard V T 2018 Phys. Rev. Lett. 120 156405
Google Scholar
[10] Bi Z, Yuan N F Q, Fu L 2019 Phys. Rev. B 100 035448
Google Scholar
[11] Alden J S, Tsen A W, Huang P Y, Hovden R, Brown L, Park J, Muller D A, McEuen P L 2013 PNAS 110 11256
Google Scholar
[12] Sunku S S, Ni G X, Jiang B Y, Yoo H, Sternbach A, McLeod A S, Stauber T, Xiong L, Taniguchi T, Watanabe K, Kim P, M. Fogler M M, Basov D N 2018 Science 362 1153
Google Scholar
[13] Wen L, Li Z Q, He Y 2021 Chin. Phys. B 30 017303
Google Scholar
[14] Kerelsky A, McGilly L, Kennes D M, Xian L, Yankowitz M, Chen S, Watanabe K, Taniguchi T, Hone J, Dean C 2019 Nature 572 95
Google Scholar
[15] Lopes dos Santos J M B, Peres N M R, Castro Neto A H 2007 Phys. Rev. Lett. 99 256802
Google Scholar
[16] Koshino M, Yuan N F Q, Koretsune T, Ochi M, Kuroki K, Fu L 2018 Phys. Rev. X 8 031087
[17] McCann E, Koshino M 2013 Rep. Prog. Phys. 76 056503
Google Scholar
[18] Moon P, Koshino M 2013 Phys. Rev. B 87 205404
Google Scholar
[19] Po H C, Zou L J, Vishwanath A, Senthil T 2018 Phys. Rev. X 8 031089
[20] 吕新宇, 李志强 2019 68 220303
Google Scholar
Lü X Y, Li Z Q 2019 Acta Phys. Sin. 68 220303
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 6633
- PDF Downloads: 278
- Cited By: 0
