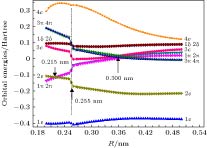
-
The potential energy curves (PECs) of the low-lying electronic states of TiAl are calculated with the complete active space self-consistent field (CASSCF) method combined with the N-electron valence perturbation theory (NEVPT2) approximation. The complete active space is mainly composed of the (3s23p1) valence orbital of Al and (3d24s2) valence orbital of Ti. Moreover, the valence splitting all-electron basis set def2-nZVPP (n = T, Q) proposed by Karlsruhe group is used in the calculation. On the basis of confirming that the ground state of TiAl is a quadruple state, the PECs of the ground state and the lowest two excited states of TiAl are obtained in a range of nuclear distance R of 0.200–0.500 nm, and the electronic states are identified. It is found that there is a “break” of the electronic structure near R = 0.255 nm. In the R > 0.255 nm region, the ground state and the two excited states are X4Δ, A4Π and B4Γ respectively; in the R < 0.255 nm region, the ground state is still X4Δ, but the two excited states become A'4Φ and B'4Π, and the degeneracy of the excited state tends to be eliminated. Based on the PECs of TiAl obtained by the dynamic correlation correction with NEVPT2, the characteristic parameters of three low-lying quadruple electronic states (such as equilibrium nuclear distance, binding energy, adiabatic excitation energy) and transition dipole moment, are obtained, and these parameters are used to explain the reason why the electronic transition spectrum of TiAl is not observed experimentally. The characteristic of “break” in the electronic state structure also provides a meaningful reference for analyzing and understanding the brittleness of TiAl alloy at room temperature.
-
Keywords:
- TiAl /
- excited state /
- potential energy curve /
- complet active space self-consistent field /
- N electronic valence perturbation theory approximation
[1] Lewandowski J J, Seifi M 2016 Annu. Rev. Mater. Res. 46 151
Google Scholar
[2] Hug G, Loiseau A, Lasalmonie A 1986 Philos. Mag. A 54 47
Google Scholar
[3] Hong T, Watsonyang T, Guo X, Freeman A, Oguchi T, Xu J 1990 Phys. Rev. B 41 12462
Google Scholar
[4] Hall E L, Huang S C 1989 J. Mater. Res. 4 595
Google Scholar
[5] Hussain A, Hayat S S, Choudhry M A 2011 Physica B 406 1961
Google Scholar
[6] Yuan X, Yin S, Lian Y, Yan P Y, Xu H F, Yan B 2019 Chin. Phys. B 28 043101
Google Scholar
[7] Zhao H Y, Ma H M, Wang J, Liu Y 2016 Chin. Phys. Lett. 33 108105
Google Scholar
[8] Tang F D, Du Q H, Petrovic C, Zhang W, He M Q, Zhang L Y 2019 Chin. Phys. B 28 037104
Google Scholar
[9] Wan M J, Jin C G, Yu Y, Huang D H, Shao J X 2017 Chin. Phys. B 26 033101
Google Scholar
[10] Chen G, Peng Y, Zheng G, Qi Z, Wand M, Yu H, Dong C, Liu C T 2016 Nat. Mater. 15 876
Google Scholar
[11] 李兴华, 杨绍利 2011 材料导报 25 94
Li X H, Yang S L 2011 Mater. Reports 25 94
[12] 宋成粉, 樊沁娜, 李蔚, 刘永利, 张林 2011 60 063104
Google Scholar
Song C F, Fan X N, Li W, Liu Y L, Zhang L 2011 Acta Phys. Sin. 60 063104
Google Scholar
[13] 闫蕴琪, 张振祺 2000 材料导报 14 31
Google Scholar
Yan Y Q, Zhang Z Q 2000 Mater. Reports 14 31
Google Scholar
[14] Behm J M, Morse M D 1994 J. Chem. Phys. 101 6500
Google Scholar
[15] Behm J M, Brugh D J, Morse M D 1994 J. Chem. Phys. 101 6487
Google Scholar
[16] Behm J M, Arrington C A, Langenberg J D, Morse M D 1993 J. Chem. Phys. 99 6394
Google Scholar
[17] 宋庆功, 秦国顺, 杨宝宝, 蒋清杰, 胡雪兰 2016 65 046102
Google Scholar
Song Q G, Qin G S, Yang B B, Jiang Q J, Hu X L 2016 Acta Phys. Sin. 65 046102
Google Scholar
[18] Zope R R, Mishin Y 2003 Phys. Rev. B 68 024102
Google Scholar
[19] Hu H, Ren Y S, Wu X Z, Liu W G, Luo J J 2019 Int. J. Mod. Phys. B 33 1950097
Google Scholar
[20] Jeong B, Kim J, Lee T, Kim S W, Ryu S 2018 Sci. Rep. 8 15200
Google Scholar
[21] Ouyang Y, Wang J, Liu F, Liu Y, Du Y, He Y 2009 J. Mol. Struc. Theochem 905 106
Google Scholar
[22] Neese F 2012 Comput. Mol. Sci. 2 73
Google Scholar
[23] Gerard H, Davidson E R, Eisenstein O 2002 Mol. Phys. 100 533
Google Scholar
[24] Larsson S 2011 Int. J. Quantum Chem. 111 3424
Google Scholar
[25] Zhang S D, Wand M X, Wand Z F, Xu K, Dong J P 2017 J. Phys. Soc. Jpn. 86 074301
Google Scholar
[26] Weigend F, Ahlrichs R 2005 Phys. Chem. Chem. Phys. 7 3297
Google Scholar
[27] Barysz M 2016 J. Chem. Theory Comput. 12 1614
Google Scholar
[28] NIST Standard Reference Database 78 https://dx.doi.org/10.18434/T4W30F [2019-9-4]
[29] Smith E B 2014 Basic Physical Chemistry: The Route to Understanding (London: Imperial College Press)
-
图 1 SA-CAS (7, 10)对TiAl最低6条电子态势能曲线的扫描计算, 其中采用的基组分别是(a) def2-TZVP; (b) def2-TZVPP, (c) def2-QZVPP
Figure 1. The lowest 6 potential energy curves of TiAl calculated by SA-CAS (7, 10) with basis set of (a) def2-TZVP; (b) def2-TZVPP; (c) def2-QZVPP.
图 2 活动基轨道能量随核间距的变化
Figure 2. Variation of CAS orbital energy with nuclear distance.
图 3 活动基轨道上电子占居数随核间距的变化
Figure 3. Variation of occupied electrons in the CAS orbital with nuclear distance.
图 4 TiAl最低四重电子态的标识(SA-CAS计算结果)
Figure 4. Identification of the lowest quadruple electronic state of TiAl (SA-CAS calculation results).
图 5 TiAl电子态势能曲线的SC-NEVPT2动态相关修正计算
Figure 5. Dynamic correlation correction calculation of SC-NEVPT2 for TiAl electronic potential energy curve.
表 1 CAS (7, 10)/def2-TZVP计算的活动基分子轨道(MO14−MO23)系数(Eh = 2625.5 kJ/mol)
Table 1. Coefficients of the CAS orbital (MO14−MO23) calculated by CAS (7, 10)/def2-TZVP.
MO No. 14 15 16 17 18 19 20 21 22 23 Energy/Eh –0.4035 –0.1838 0.0029 –0.0159 –0.0159 0.0743 0.0743 0.0439 0.1714 0.1714 Number of occupied electron 1.957 1.772 0.669 0.595 0.595 0.494 0.494 0.222 0.099 0.099 Symbol σ σ σ π π δ δ σ π π Ti s σ 12.1 47.5 7.7 0 0 0 0 13.4 0 0 Ti pz σ 7.5 2.6 1.2 0 0 0 0 38.2 0 0 Ti px π 0 0 0 4.9 0 0 0 0 0 0 Ti py 0 0 0 1.8 0 0 0 0 0 0 Ti dz2 σ 7 1.7 86.1 0 0 0 0 10.4 0 0 Ti dxz π 0 0 0 31.4 11.4 0 0 0 34.6 31.2 Ti dyz 0 0 0 11.4 31.4 0 0 0 31.2 34.6 Ti dx2y2 δ 0 0 0 0 0 82.6 17.2 0 0 0 Ti dxy 0 0 0 0 0 17.2 82.6 0 0 0 Al s σ 72.9 7.8 1.2 0 0 0 0 0 0 0 Al pz σ 0.5 39.2 3.2 0 0 0 0 36.6 0 0 Al px π 0 0 0 34.4 12.5 0 0 0 16.7 15.1 Al py 0 0 0 12.5 34.4 0 0 0 15.1 16.7 
DownLoad: CSV
表 2 两组
${\text{π}}$ 轨道的组成分析Table 2. Composition analysis of two π orbits
Orbital R = 0.200 nm R = 0.240 nm R = 0.280 nm R = 0.490 nm ${\rm{(1{\text{π}})(2{\text{π}})}}$ $\begin{aligned}& {\rm{Ti(}}3{{\rm{p}}_x}, {\rm{ }}3{{\rm{p}}_y}{\rm{) }}7{\rm{\% }} \\& {\rm{Ti(}}3{{\rm{d}}_{xz}}, 3{{\rm{d}}_{yz}}{\rm{) }}60{\rm{\% }} \\ &{\rm{Al(}}3{{\rm{p}}_x}, 3{{\rm{p}}_y}{\rm{) }}28{\rm{\% }}\end{aligned} $ $\begin{aligned}& {\rm{Ti(3}}{{\rm{p}}_x}, {\rm{ 3}}{{\rm{p}}_y}{\rm{) }}7{\rm{\% }} \\& {\rm{Ti(3}}{{\rm{d}}_{xz}}, 3{{\rm{d}}_{yz}}{\rm{) }}5{\rm{7\% }} \\& {\rm{Al(3}}{{\rm{p}}_x}, 3{{\rm{p}}_y}{\rm{) }}3{\rm{2\% }}\end{aligned} $ $\begin{aligned}& {\rm{Ti(3}}{{\rm{p}}_x}, {\rm{ }}3{{\rm{p}}_y}{\rm{) 3\% }} \\ &{\rm{Ti(3}}{{\rm{d}}_{xz}}, 3{{\rm{d}}_{yz}}{\rm{) 73\% }} \\& {\rm{Al(3}}{{\rm{p}}_x}, 3{{\rm{p}}_y}{\rm{) 21\% }}\end{aligned} $ ${\rm{Ti}}(3{{\rm{d}}_{xz}}, 3{{\rm{d}}_{yz}}){\rm{ }}1{\rm{00}}\% $ ${\rm{(3{\text{π}})(4{\text{π}})}}$ $\begin{aligned} &{\rm{Ti(3}}{{\rm{d}}_{xz}}{\rm{, 3}}{{\rm{d}}_{yz}}{\rm{) 52\% }} \\ &{\rm{Al(3}}{{\rm{p}}_x}{\rm{, 3}}{{\rm{p}}_y}{\rm{) 36\% }}\end{aligned} $ $\begin{aligned} &{\rm{Ti(3}}{{\rm{d}}_{xz}}, 3{{\rm{d}}_{yz}}{\rm{) 52\% }} \\& {\rm{Al(3}}{{\rm{p}}_x}, 3{{\rm{p}}_y}{\rm{) 40\% }}\end{aligned} $ $\begin{aligned}& {\rm{Ti(3}}{{\rm{p}}_x}, {\rm{ }}3{{\rm{p}}_y}{\rm{) 12\% }} \\ &{\rm{Ti(3}}{{\rm{d}}_{xz}}, 3{{\rm{d}}_{yz}}{\rm{) 34\% }} \\ &{\rm{Al(3}}{{\rm{p}}_x}, 3{{\rm{p}}_y}{\rm{) 52\% }}\end{aligned} $ ${\rm{Al(3}}{{\rm{p}}_x}, 3{{\rm{p}}_y}{\rm{) 99\% }}$
DownLoad: CSV
表 3 R = 0.490 nm处活动基分子轨道MO14-MO23组成
Table 3. Composition of CAS orbitals MO14-MO23 at R = 0.490 nm.
Orbital No 12 13 14 15 16 17 Energy/Hartree –1.79778 –1.7976 –0.37486 –0.21513 0.02001 0.03822 Occupied electron 2.00000 2.0000 1.91976 1.89900 0.62463 0.55980 Ti s 0 0 2.8 94.5 0 0 Ti pz 0 99.8 0.5 0 0 0 Ti px 55.7 0.1 0 0 0 0 Ti py 44.3 0 0 0 0 0 Ti dxz 0 0 0 0 55.8 43.6 Ti dyz 0 0 0 0 44.1 55.2 Al s 0 0 95.8 2.8 0 0 Orbital No 18 19 20 21 22 23 Energy/Hartree –0.00791 –0.00682 0.0885 0.08979 0.05651 0.1227 Occupied electron 0.51786 0.51750 0.41058 0.40645 0.10159 0.04283 Ti pz 0 0 0 0.0 91.4 5.3 Ti dx2y2 0 0 1.4 98.6 0 0 Ti dxy 0 0 98.4 1.4 0 0 Al pz 0 0 0 0 7 92.7 Al px 55.3 43.3 0 0 0 0 Al py 43.7 54.8 0 0 0 0
DownLoad: CSV
表 4 基态及最低激发态的组态及跃迁偶极矩
Table 4. Configuration and transition dipole moment of the ground state and the lowest excited state
R/nm state Main configuration Excitation energy/cm–1 Transition dipole moment T2/Debye2 Possible quartet state Idetified state 0.285 Ground state ${{\rm{\text{σ} }}^{\rm{2}}}{{\rm{\text{σ} }}^{\rm{2}}}{{\text{π}}^{\rm{2}}}{{\rm{\text{δ} }}^{\rm{1}}}{{\text{π}}^{\rm{0}}}$ 0 4Δ X4Δ 1st excited state ${{\rm{\text{σ} }}^{\rm{2}}}{{\rm{\text{σ} }}^{\rm{2}}}{{\text{π}}^{\rm{2}}}{{\rm{\text{δ} }}^{\rm{0}}}{{\text{π}}^{\rm{1}}}$ 3212 0.034 4Π A4Π 2nd excited state ${{\rm{\text{σ} }}^{\rm{2}}}{{\rm{\text{σ} }}^{\rm{2}}}{{\text{π}}^{\rm{1}}}{{\rm{\text{δ} }}^{\rm{1}}}{{\text{π}}^{\rm{1}}}$ 3462 0 4Σ, 4Δ(2), 4Γ B4Γ 0.240 Ground state ${{\rm{\text{σ} }}^{\rm{2}}}{{\rm{\text{σ} }}^{\rm{2}}}{{\text{π}}^{\rm{2}}}{{\rm{\text{δ} }}^{\rm{1}}}$ 0 4Δ X4Δ 1st excited state ${{\rm{\text{σ} }}^{\rm{2}}}{{\rm{\text{σ} }}^{\rm{1}}}{{\text{π}}^{\rm{3}}}{{\rm{\text{δ} }}^{\rm{1}}}$ 4140 0.00824 4Π, 4Φ A'4Φ 2nd excited state ${{\rm{\text{σ} }}^{\rm{2}}}{{\rm{\text{σ} }}^{\rm{1}}}{{\text{π}}^{\rm{3}}}{{\rm{\text{δ} }}^{\rm{1}}}$ 4727 0.00869 4Π, 4Φ B'4Π 3rd excited state ${{\rm{\text{σ} }}^{\rm{2}}}{{\rm{\text{σ} }}^{\rm{1}}}{{\text{π}}^{\rm{3}}}{{\rm{\text{δ} }}^{\rm{1}}}$ 5074 0.00551 4Π, 4Φ B'4Π
DownLoad: CSV
表 5 TiAl最低3个四重态的结构参数
Table 5. Structural parameters of the lowest three quadruple states of TiAl.
State Re/nm De/cm–1 CAS NEVPT2 CAS NEVPT2 X4Δ 0.288 0.266 3016 8151 A4Π 0.320 $\left\{\begin{aligned}& {0.248} \\ & {0.296} \end{aligned} \right.$ 796 $\left\{\begin{aligned}& {3845} \\ & {3406} \end{aligned} \right.$ B4Γ 0.324 $\left\{\begin{aligned}& {0.248} \\ & {0.306} \end{aligned} \right.$ 711 $\left\{\begin{aligned}& {2884} \\ & {3406} \end{aligned} \right.$
DownLoad: CSV
-
[1] Lewandowski J J, Seifi M 2016 Annu. Rev. Mater. Res. 46 151
Google Scholar
[2] Hug G, Loiseau A, Lasalmonie A 1986 Philos. Mag. A 54 47
Google Scholar
[3] Hong T, Watsonyang T, Guo X, Freeman A, Oguchi T, Xu J 1990 Phys. Rev. B 41 12462
Google Scholar
[4] Hall E L, Huang S C 1989 J. Mater. Res. 4 595
Google Scholar
[5] Hussain A, Hayat S S, Choudhry M A 2011 Physica B 406 1961
Google Scholar
[6] Yuan X, Yin S, Lian Y, Yan P Y, Xu H F, Yan B 2019 Chin. Phys. B 28 043101
Google Scholar
[7] Zhao H Y, Ma H M, Wang J, Liu Y 2016 Chin. Phys. Lett. 33 108105
Google Scholar
[8] Tang F D, Du Q H, Petrovic C, Zhang W, He M Q, Zhang L Y 2019 Chin. Phys. B 28 037104
Google Scholar
[9] Wan M J, Jin C G, Yu Y, Huang D H, Shao J X 2017 Chin. Phys. B 26 033101
Google Scholar
[10] Chen G, Peng Y, Zheng G, Qi Z, Wand M, Yu H, Dong C, Liu C T 2016 Nat. Mater. 15 876
Google Scholar
[11] 李兴华, 杨绍利 2011 材料导报 25 94
Li X H, Yang S L 2011 Mater. Reports 25 94
[12] 宋成粉, 樊沁娜, 李蔚, 刘永利, 张林 2011 60 063104
Google Scholar
Song C F, Fan X N, Li W, Liu Y L, Zhang L 2011 Acta Phys. Sin. 60 063104
Google Scholar
[13] 闫蕴琪, 张振祺 2000 材料导报 14 31
Google Scholar
Yan Y Q, Zhang Z Q 2000 Mater. Reports 14 31
Google Scholar
[14] Behm J M, Morse M D 1994 J. Chem. Phys. 101 6500
Google Scholar
[15] Behm J M, Brugh D J, Morse M D 1994 J. Chem. Phys. 101 6487
Google Scholar
[16] Behm J M, Arrington C A, Langenberg J D, Morse M D 1993 J. Chem. Phys. 99 6394
Google Scholar
[17] 宋庆功, 秦国顺, 杨宝宝, 蒋清杰, 胡雪兰 2016 65 046102
Google Scholar
Song Q G, Qin G S, Yang B B, Jiang Q J, Hu X L 2016 Acta Phys. Sin. 65 046102
Google Scholar
[18] Zope R R, Mishin Y 2003 Phys. Rev. B 68 024102
Google Scholar
[19] Hu H, Ren Y S, Wu X Z, Liu W G, Luo J J 2019 Int. J. Mod. Phys. B 33 1950097
Google Scholar
[20] Jeong B, Kim J, Lee T, Kim S W, Ryu S 2018 Sci. Rep. 8 15200
Google Scholar
[21] Ouyang Y, Wang J, Liu F, Liu Y, Du Y, He Y 2009 J. Mol. Struc. Theochem 905 106
Google Scholar
[22] Neese F 2012 Comput. Mol. Sci. 2 73
Google Scholar
[23] Gerard H, Davidson E R, Eisenstein O 2002 Mol. Phys. 100 533
Google Scholar
[24] Larsson S 2011 Int. J. Quantum Chem. 111 3424
Google Scholar
[25] Zhang S D, Wand M X, Wand Z F, Xu K, Dong J P 2017 J. Phys. Soc. Jpn. 86 074301
Google Scholar
[26] Weigend F, Ahlrichs R 2005 Phys. Chem. Chem. Phys. 7 3297
Google Scholar
[27] Barysz M 2016 J. Chem. Theory Comput. 12 1614
Google Scholar
[28] NIST Standard Reference Database 78 https://dx.doi.org/10.18434/T4W30F [2019-9-4]
[29] Smith E B 2014 Basic Physical Chemistry: The Route to Understanding (London: Imperial College Press)
DownLoad:
Catalog
Metrics
- Abstract views: 7483
- PDF Downloads: 80
- Cited By: 0
