Source boundary parameter of Monte Carlo inversion technology based on virtual source principle

Tian Zi-Ning; Ouyang Xiao-Ping; Chen Wei; Wang Xue-Mei; Deng Ning; Liu Wen-Biao; Tian Yan-Jie

doi:10.7498/aps.68.20191095

Abstract

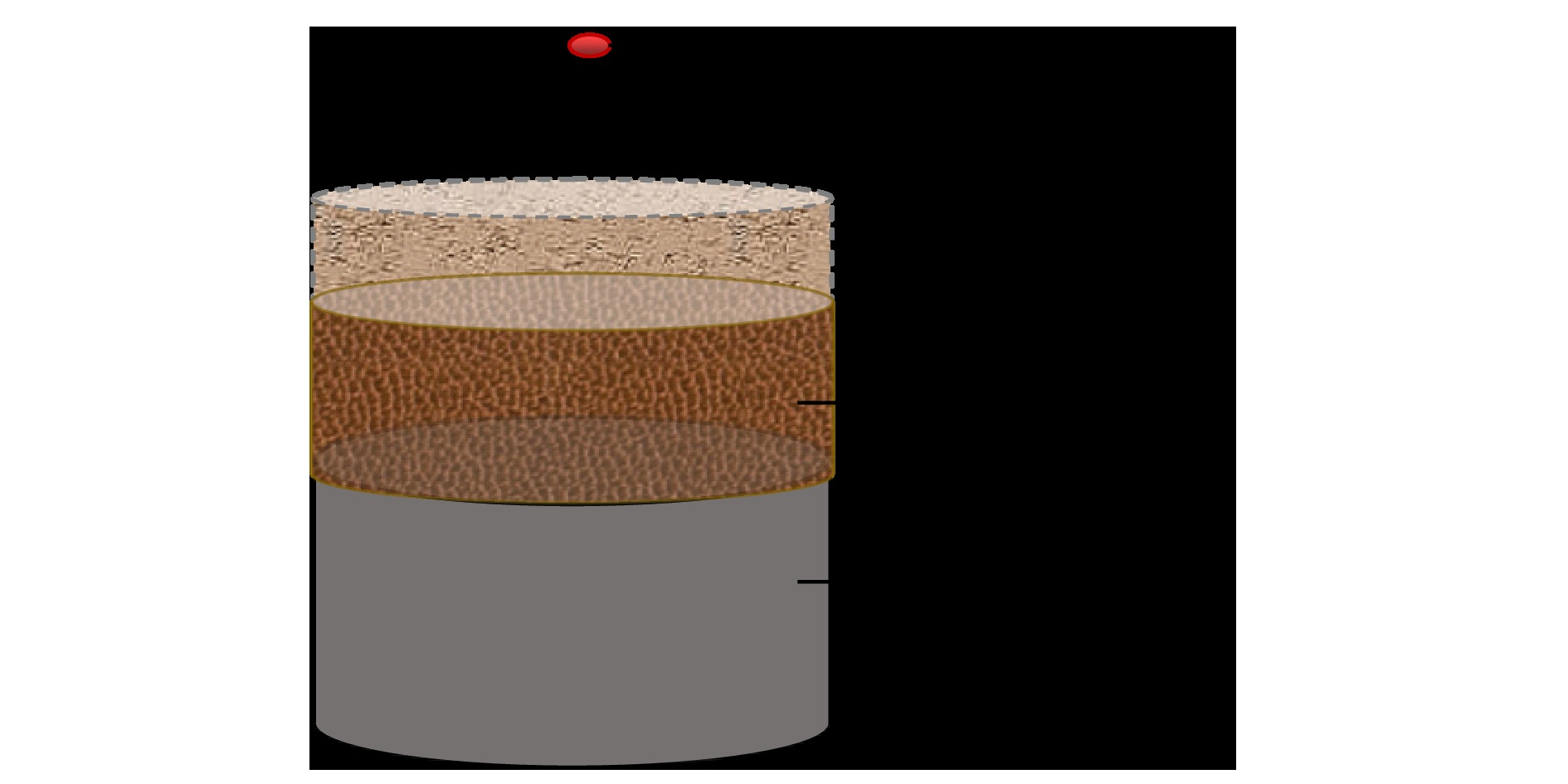
In the in situ γ spectrometer based measurement of " hot particular”, " radioactive collection point” and " radioactive collection area”, only the position of the pollution source can be located roughly, but its boundary parameters such as the thickness of pollution source cannot be given. In this paper, the application of virtual technology to the scanning of γ spectrometer is studied. We convert γ spectrometer measurement objects into a four-layer theoretical model, which are attenuation thickness + radioactive hot area + attenuation thickness + disturb source. Then, the source item layer is virtualized into a point source by using virtual technology. So, the theoretical model is further simplified. Then the detection efficiency and peak/valley ratio parameter of source term are simulated by Monte Carlo method. Finally, the source term parameters are retrieved by using the least square method, and thus establishing the theoretical method and procedure of inversion calculation of source boundary parameters. In this paper, the theoretical and experimental results are shown to be consistent with each other. So, this method is verified to be correct and practicable. Currently, the method can accurately determine the depth distribution parameters of radioactive contamination area for uniformly distributed radio nuclides. In conclusion, the technical achievements can be used to accurately determine the boundary range of the radioactive hot zone, thus realizing the purpose of reducing the waste disposal capacity during the treatment. At the same time, the determination of the inert layer thickness parameters of the target nuclear warhead of Nuclear Test Ban Treaty has a significant reference value.

Keywords:

Authors and contacts

Northwest Institute of Nuclear Technology, Xi’an 710024, China

Corresponding author: Tian Zi-Ning, tzn1019@126.com

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 11405134)

FullText HTML : translate this paragraph

References

[1]	熊宗华, 亢武, 龚建, 胡广春, 向永春, 裴永全 2003 52 1 Google Scholar Xiong Z H, Kang W, Gong J, Hu G C, Xiang Y C, Pei Y Q 2003 Atca Phys. Sin. 52 1 Google Scholar
[2]	Kováik A, Sy′kora I, Povinec P P 2013 J. Radioanal. Nucl. Chem. 298 665 Google Scholar
[3]	Peyres V, García-Torao E 2007 Nucl. Instr. Meth. Phys. Res. A 580 296 Google Scholar
[4]	田自宁, 欧阳晓平, 殷经鹏, 张洋, 杨文静 2013 原子能科学技术 47 1411 Google Scholar Tian Z N, Ouyang X P, Yin J P, Zhang Y, Yang W J 2013 Atomic Energy Sci. Technol. 47 1411 Google Scholar
[5]	Elanique A, Marzocchi O, Leone D, Hegenbart L, Breustedt B, Oufni L 2012 Appl. Radiat. Isot. 70 538 Google Scholar
[6]	Budjá D, Heisel M, Maneschg W, Simgen H 2009 Appl. Radiat. Isot. 67 706 Google Scholar
[7]	Gasparro J, Hult M, Johnston P N, Tagziria H 2008 Nucl. Instr. Meth. Phys. Res. A 594 196 Google Scholar
[8]	Huya N Q, Binhb D Q, An V X 2007 Nucl. Instr. Meth. Phys. Res. A 573 384 Google Scholar
[9]	Huy N Q 2010 Nucl. Instr. Meth. Phys. Res. A 621 390 Google Scholar
[10]	Luís R, Bento J, Carvalhal G, Nogueira P, Silva L, Teles P, Vaz P 2010 Nucl. Instr. Meth. Phys. Res. A 623 1014 Google Scholar
[11]	Mohammadi M A, Abdi M R, Kamali M, Mostajaboddavati M, Zare M R 2011 Appl. Radiat. Isot. 69 521 Google Scholar
[12]	Presler O, German U, Pelled O, Alfassi Z B 2004 Appl. Radiat. Isot. 60 213 Google Scholar
[13]	Mahling S, Orion I, Alfassi Z B 2006 Nucl. Instr. Meth. Phys. Res. A 557 544 Google Scholar
[14]	熊文彬, 仇春华, 段天英, 刘浩杰, 潘君艳, 陈海涛, 刘进辉 2011 原子能科学技术 45 999 Xiong W B, Qiu C H, Duan T Y, Liu H J, Pan J Y, Chen H T, Liu J H 2011 Atomic Energy Sci. Technol. 45 999
[15]	Noteal A 1971 Nucl. Instr. Meth. 91 513 Google Scholar
[16]	田自宁, 欧阳晓平, 曾鸣, 成智威 2013 62 162902 Google Scholar Tian Z N, Ouyang X P, Zeng M, Cheng Z W 2013 Acta Phys. Sin. 62 162902 Google Scholar
[17]	田自宁, 陈伟, 韩斌, 田言杰, 刘文彪, 冯天成, 欧阳晓平 2016 65 062901 Google Scholar Tian Z N, Chen W, Han B, Tian Y J, Liu W B, Feng T C, Ouyang X P 2016 Acta Phys. Sin. 65 062901 Google Scholar

Cited By

图 1 源边界参数反演理论模型

Figure 1. The inversion theory model of source boundary parameters.

DownLoad: Full-Size Img PowerPoint

图 2 探测模式1

Figure 2. The detection mode 1.

DownLoad: Full-Size Img PowerPoint

图 3 探测模式2

Figure 3. The detection mode 2.

DownLoad: Full-Size Img PowerPoint

图 4 探测模式1的MCNP程序计算模型

Figure 4. Calculation model of MCNP procedure for detection mode 1.

DownLoad: Full-Size Img PowerPoint

图 5 探测模式2的MCNP程序计算模型

Figure 5. Calculation model of MCNP procedure for detection mode 2.

DownLoad: Full-Size Img PowerPoint

表 1 探测模式1实验能谱峰计数及处理结果

Table 1. Energy peak count of experimental spectrum and process results for detection mode 1.

测量对象	测量时长t/10⁵ s	N₂₄₁ (54—57 keV)	N₂₄₁ (59.54 keV)	N₂₃₉ (51.62, 129 keV)	A/10⁴ Bq
测量对象	测量时长t/10⁵ s	N₂₄₁ (54—57 keV)	N₂₄₁ (59.54 keV)	N₂₃₉ (51.62, 129 keV)	²⁴¹Am	²³⁹Pu
探测模式1	3.16	4325136	25339979	—	—	—
²³⁹Pu体源	2.00	—	75248200	239711, 52717	4.56	18.7

DownLoad: CSV

表 2 探测模式2实验能谱峰计数及处理结果

Table 2. Energy peak count of experimental spectrum and process results for detection mode 2.

测量对象	测量时长 t/10⁵s	N₂₄₁ (26.4 keV)	N₂₄₁ (54—57 keV)	N₂₄₁ (59.54 keV)	A/× 10⁴ Bq
测量对象	测量时长 t/10⁵s	N₂₄₁ (26.4 keV)	N₂₄₁ (54—57 keV)	N₂₄₁ (59.54 keV)	26.4 keV	59.54 keV
探测模式2	4.00	240050	9531180	65964536	8.16	8.91
²⁴¹Am点源	0.565	4160024	—	65331456	7.74	8.38
²⁴¹Am体源	0.800	45346	—	2745773	0.423	0.532

DownLoad: CSV

表 3 等效虚拟点源探测效率及峰谷比

Table 3. The detection efficiency and peak/valley of equivalent virtual point source.

h/cm	${\varepsilon _{241}}(h)$/10^–3	${\varepsilon _{239}}(h)$/10^–3	A₂₄₁/10⁴ Bq	A₂₃₉/10⁵ Bq	Q	${N}/{ { {N_{\rm v}} } }(h)$
–1.25	24.3	24.6	0.921	0.497	5.4	8.05
–1.60	17.9	19.5	1.24	0.629	5.0	6.99
–2.00	12.9	15.1	1.73	0.810	4.7	6.12
–2.40	9.45	11.9	2.36	1.03	4.4	5.44
–2.80	7.00	9.45	3.19	1.30	4.1	4.92
–3.20	5.24	7.60	4.26	1.61	3.8	4.51
–3.60	3.97	6.16	5.62	1.98	3.5	4.18
–3.80	3.47	5.56	6.45	2.20	3.4	4.04
–4.00	3.03	5.04	7.37	2.43	3.3	3.90

DownLoad: CSV

表 4 不同组合下等效虚拟点的均方偏差计算数据

Table 4. The mean square deviation calculation data of equivalent virtual point at different combination.

w	h/cm	$\varepsilon (h)$/10^–3	${\varepsilon ^*}(h)$/10^–3	${N}/{ { {N_{\rm v}} } }(h)$	X₂/10^–3	X₃/10^–3	X₁	$\sigma (X)$
0.10	–1.25	24.3	24.6	8.05	—	—	—	—
0.90	–3.20	5.24	7.60	4.51	7.15	9.30	4.87	0.177
0.90	–3.60	3.97	6.16	4.18	6.00	8.01	4.56	0.308
0.90	–3.80	3.47	5.56	4.04	5.55	7.47	4.44	0.385
0.90	–4.00	3.03	5.04	3.90	5.16	6.99	4.31	0.458
0.10	–1.60	17.9	19.5	6.99	—	—	—	—
0.90	–3.20	5.24	7.60	4.51	6.51	8.79	4.76	0.217
0.90	–3.60	3.97	6.16	4.18	5.36	7.50	4.46	0.396
0.90	–3.80	3.47	5.56	4.04	4.91	6.96	4.33	0.479
0.90	–4.00	3.03	5.04	3.90	4.52	6.48	4.21	0.554
0.10	–2.00	12.9	15.1	6.12	—	—	—	—
0.90	–3.20	5.24	7.60	4.51	6.01	8.35	4.67	0.277
0.90	–3.60	3.97	6.16	4.18	4.86	7.06	4.37	0.474
0.90	–3.80	3.47	5.56	4.04	4.41	6.52	4.24	0.559
0.90	–4.00	3.03	5.04	3.90	4.02	6.04	4.12	0.635
0.10	–2.40	9.45	11.9	5.44	—	—	—	—
0.90	–3.20	5.24	7.60	4.51	5.66	8.03	4.61	0.327
0.90	–3.60	3.97	6.16	4.18	4.52	6.74	4.30	0.530
0.90	–3.80	3.47	5.56	4.04	4.06	6.20	4.18	0.616
0.90	–4.00	3.03	5.04	3.90	3.67	5.72	4.05	0.693

DownLoad: CSV

表 5 不同组合下等效虚拟点的均方偏差计算数据

Table 5. The mean square deviation calculation data of equivalent virtual point at different combination.

h/cm	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$
–1.25	0.10	—	0.20	—	0.30	—	0.40	—	0.50	—
–3.20	0.90	0.177	0.80	0.355	0.70	0.664	0.60	0.988	0.50	1.32
–3.60	0.90	0.308	0.80	0.223	0.70	0.508	0.60	0.848	0.50	1.20
–3.80	0.90	0.385	0.80	0.201	0.70	0.448	0.60	0.792	0.50	1.15
–4.00	0.90	0.458	0.80	0.210	0.70	0.399	0.60	0.744	0.50	1.11
–1.60	0.10	—	0.20	—	0.30	—	0.40	—	0.50	—
–3.20	0.90	0.217	0.80	0.195	0.70	0.360	0.60	0.568	0.50	0.784
–3.60	0.90	0.396	0.80	0.210	0.70	0.234	0.60	0.435	0.50	0.669
–3.80	0.90	0.479	0.80	0.262	0.70	0.203	0.60	0.384	0.50	0.622
–4.00	0.90	0.554	0.80	0.318	0.70	0.196	0.60	0.343	0.50	0.583
–2.00	0.10	—	0.20	—	0.30	—	0.40	—	0.50	—
–3.20	0.90	0.277	0.80	0.187	0.70	0.177	0.60	0.256	0.50	0.371
–3.60	0.90	0.474	0.80	0.328	0.70	0.210	0.60	0.181	0.50	0.272
–3.80	0.90	0.559	0.80	0.399	0.70	0.257	0.60	0.178	0.50	0.238
–4.00	0.90	0.635	0.80	0.465	0.70	0.307	0.60	0.193	0.50	0.215
–2.40	0.10	—	0.20	—	0.30	—	0.40	—	0.50	—
–3.20	0.90	0.327	0.80	0.264	0.70	0.209	0.60	0.173	0.50	0.167
–3.60	0.90	0.530	0.80	0.435	0.70	0.344	0.60	0.261	0.50	0.195
–3.80	0.90	0.616	0.80	0.510	0.70	0.407	0.60	0.309	0.50	0.225
–4.00	0.90	0.693	0.80	0.577	0.70	0.464	0.60	0.356	0.50	0.257

DownLoad: CSV

h/cm	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$
–1.25	0.60	—	0.70	—	0.80	—	0.9	—
–3.20	0.40	1.65	0.30	1.98	0.20	2.31	0.10	2.64
–3.60	0.40	1.55	0.30	1.90	0.20	2.26	0.10	2.61
–3.80	0.40	1.51	0.30	1.88	0.20	2.24	0.10	2.60
–4.00	0.40	1.48	0.30	1.85	0.20	2.22	0.10	2.60
–1.60	0.60	—	0.70	—	0.80	—	0.90	—
–3.20	0.40	1.00	0.30	1.23	0.20	1.45	0.10	1.67
–3.60	0.40	0.910	0.30	1.16	0.20	1.40	0.10	1.65
–3.80	0.40	0.872	0.30	1.13	0.20	1.38	0.10	1.64
–4.00	0.40	0.839	0.30	1.10	0.20	1.37	0.10	1.63
–2.00	0.60	—	0.70	—	0.80	—	0.90	—
–3.20	0.40	0.498	0.30	0.630	0.20	0.763	0.10	0.898
–3.60	0.40	0.410	0.30	0.561	0.20	0.716	0.10	0.875
–3.80	0.40	0.375	0.30	0.533	0.20	0.698	0.10	0.865
–4.00	0.40	0.347	0.30	0.509	0.20	0.681	0.10	0.857
–2.40	0.60	—	0.70	—	0.80	—	0.90	—
–3.20	0.40	0.194	0.30	0.244	0.20	0.305	0.10	0.372
–3.60	0.40	0.169	0.30	0.199	0.20	0.267	0.10	0.351
–3.80	0.40	0.174	0.30	0.186	0.20	0.252	0.10	0.342
–4.00	0.40	0.186	0.30	0.178	0.20	0.240	0.10	0.335

DownLoad: CSV

表 6 体源参数的反演计算数据

Table 6. The inversion data of volume source parameters.

h_V/cm	体源厚度/cm	$\varepsilon ({h_{\rm{V}}})$ /10^–3	${\varepsilon ^*}({h_{\rm{V}}})$ /10^–2	${N}/{ { {N_{\rm v}} } }({h_{\rm{V} } })$	$\sigma (X)$
–2.80	0.80	4.54	0.689	4.69	0.444
–2.80	1.2	4.60	0.696	4.74	0.431
–2.80	1.6	4.70	0.705	4.81	0.414
–2.45	0.80	5.68	0.818	5.03	0.231
–2.45	1.2	5.76	0.826	5.07	0.217
–2.45	1.6	5.89	0.837	5.16	0.200
–2.45	2.0	6.05	0.851	5.26	0.181
–2.45	2.5	6.31	0.874	5.41	0.159
–2.45	3.0	6.65	0.903	5.63	0.163
–2.45	4.0	7.57	0.981	6.24	0.289
–2.45	4.9	8.78	1.08	7.12	0.539
–2.00	0.80	7.62	1.03	5.58	0.188
–2.00	1.2	7.75	1.04	5.64	0.212
–2.00	1.6	7.93	1.05	5.75	0.248
–2.00	2.0	8.17	1.07	5.88	0.295
–2.00	2.5	8.55	1.10	6.11	0.373
–2.00	3.0	9.03	1.14	6.41	0.474
–2.00	4.0	10.4	1.25	7.35	0.769
–1.50	0.80	10.7	1.33	6.43	0.744
–1.50	1.2	10.9	1.35	6.54	0.781
–1.50	1.6	11.2	1.37	6.68	0.834
–1.50	2.0	11.5	1.40	6.90	0.905
–1.50	3.0	12.9	1.50	7.79	1.18
–0.50	0.80	22.0	2.34	10.1	2.81

DownLoad: CSV

表 7 等效虚拟点源探测效率、峰谷比及活度比

Table 7. The detection efficiency, peak/valley and acvitiy ratio of equivalent virtual point source.

h/cm	${\varepsilon _{26.4\;{\rm{keV}}}}(h)$/10^–3	${\varepsilon _{59.54\;{\rm{keV}}}}(h)$/10^–2	A_{26.4 keV}/10⁴ Bq	A_{59.54 keV}/10⁴ Bq	A_{59.54 keV}/A_{26.4 keV}	${N}/{ { {N_{\rm v}} } }(h)$
0.80	48.200	14.4	0.0519	0.318	6.10	17.0
0.40	13.500	8.79	0.1850	0.523	2.80	12.0
0	4.060	5.56	0.6150	0.826	1.30	9.3
–0.20	2.260	4.48	1.1100	1.030	0.90	8.4
–0.40	1.270	3.64	1.9700	1.260	0.60	7.7
–0.60	0.717	2.98	3.4900	1.540	0.40	7.1
–0.80	0.409	2.45	6.1200	1.880	0.30	6.6

DownLoad: CSV

表 9 体源参数的反演计算数据

Table 9. The inversion data of volume source parameters.

h_V/cm	体源厚度/cm	${\varepsilon ^*}({h_{\rm{V}}})$/ 10^–2	$\varepsilon ({h_{\rm{V}}})$/ 10^–3	${N}/{ { {N_{\rm v}} } }({h_{\rm{V} } })$	$\sigma (X)$	h_V/cm	体源厚度/cm	${\varepsilon ^*}({h_{\rm{V}}})$/ 10^–2	$\varepsilon ({h_{\rm{V}}})$/ 10^–3	${N}/{ { {N_{\rm v}} } }({h_{\rm{V} } })$	$\sigma (X)$
0.75	1.00	3.54	10.4	11.90	1.5900	0.15	2.20	2.45	5.00	9.34	0.2310
0.75	0.60	3.47	8.06	11.40	1.0100	0.15	1.60	2.31	2.69	8.54	0.3470
0.75	0.30	3.44	7.23	11.20	0.8060	0.15	1.00	2.21	1.72	8.07	0.5920
0.25	2.00	2.59	5.49	9.61	0.3530	0.15	0.40	2.17	1.32	7.86	0.6920
0.25	1.90	2.56	4.91	9.44	0.2110	0	2.50	2.26	4.40	9.00	0.0900
0.25	1.80	2.54	4.42	9.29	0.0890	0	2.45	2.24	4.14	8.90	0.0470
0.25	1.70	2.51	4.00	9.14	0.0220	0	2.40	2.23	3.90	8.83	0.0640
0.25	1.60	2.49	3.63	9.03	0.1090	0	2.00	2.13	2.52	8.30	0.3950
0.25	1.50	2.47	3.32	8.92	0.1870	0	1.50	2.04	1.60	7.83	0.6260
0.25	1.30	2.43	2.81	8.72	0.3130	0	1.00	1.97	1.13	7.54	0.7470
0.25	0.80	2.37	2.04	8.38	0.5080	–0.25	1.10	1.65	0.602	6.86	0.8910
						–0.25	0.50	1.61	0.457	6.70	0.9300

DownLoad: CSV

表 8 不同组合下等效虚拟点的均方偏差计算数据

Table 8. The mean square deviation calculation data of equivalent virtual point at different combination.

h/cm	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$
0.80	0.10	—	0.20	—	0.30	—	0.40	—	0.50	—
–0.40	0.90	2.414	0.80	1.783	0.70	2.807	0.60	3.832	0.50	4.86
–0.60	0.90	2.115	0.80	1.512	0.70	2.569	0.60	3.627	0.50	4.69
–0.80	0.90	1.951	0.80	1.362	0.70	2.433	0.60	3.510	0.50	4.59
0.40	0.10	—	0.20	—	0.30	—	0.40	—	0.50	—
–0.40	0.90	0.466	0.80	0.291	0.70	0.529	0.60	0.780	0.50	1.03
–0.60	0.90	0.177	0.80	0.119	0.70	0.286	0.60	0.567	0.50	0.857
–0.80	0.90	0.185	0.80	0.245	0.70	0.175	0.60	0.447	0.50	0.753
0	0.10	—	0.20	—	0.30	—	0.40	—	0.50	—
–0.40	0.90	0.190	0.80	0.160	0.70	0.168	0.60	0.235	0.50	0.327
–0.60	0.90	0.431	0.80	0.346	0.70	0.214	0.60	0.123	0.50	0.165
–0.80	0.90	0.620	0.80	0.512	0.70	0.350	0.60	0.197	0.50	0.112

DownLoad: CSV

h/cm	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$	w	$\sigma (X)$
0.80	0.60	—	0.70	—	0.80	–	0.90	—
–0.40	0.40	5.88	0.30	6.91	0.20	7.93	0.10	8.96
–0.60	0.40	5.75	0.30	6.81	0.20	7.87	0.10	8.92
–0.80	0.40	5.67	0.30	6.75	0.20	7.83	0.10	8.90
0.40	0.60	–	0.70	—	0.80	–	0.90	—
–0.40	0.40	1.29	0.30	1.55	0.20	1.80	0.10	2.06
–0.60	0.40	1.15	0.30	1.44	0.20	1.73	0.10	2.03
–0.80	0.40	1.06	0.30	1.38	0.20	1.69	0.10	2.01
0	0.60	—	0.70	—	0.80	—	0.90	—
–0.40	0.40	0.427	0.30	0.532	0.20	0.639	0.10	0.747
–0.60	0.40	0.287	0.30	0.425	0.20	0.567	0.10	0.711
–0.80	0.40	0.207	0.30	0.361	0.20	0.523	0.10	0.689

DownLoad: CSV

map

[1]	熊宗华, 亢武, 龚建, 胡广春, 向永春, 裴永全 2003 52 1 Google Scholar Xiong Z H, Kang W, Gong J, Hu G C, Xiang Y C, Pei Y Q 2003 Atca Phys. Sin. 52 1 Google Scholar
[2]	Kováik A, Sy′kora I, Povinec P P 2013 J. Radioanal. Nucl. Chem. 298 665 Google Scholar
[3]	Peyres V, García-Torao E 2007 Nucl. Instr. Meth. Phys. Res. A 580 296 Google Scholar
[4]	田自宁, 欧阳晓平, 殷经鹏, 张洋, 杨文静 2013 原子能科学技术 47 1411 Google Scholar Tian Z N, Ouyang X P, Yin J P, Zhang Y, Yang W J 2013 Atomic Energy Sci. Technol. 47 1411 Google Scholar
[5]	Elanique A, Marzocchi O, Leone D, Hegenbart L, Breustedt B, Oufni L 2012 Appl. Radiat. Isot. 70 538 Google Scholar
[6]	Budjá D, Heisel M, Maneschg W, Simgen H 2009 Appl. Radiat. Isot. 67 706 Google Scholar
[7]	Gasparro J, Hult M, Johnston P N, Tagziria H 2008 Nucl. Instr. Meth. Phys. Res. A 594 196 Google Scholar
[8]	Huya N Q, Binhb D Q, An V X 2007 Nucl. Instr. Meth. Phys. Res. A 573 384 Google Scholar
[9]	Huy N Q 2010 Nucl. Instr. Meth. Phys. Res. A 621 390 Google Scholar
[10]	Luís R, Bento J, Carvalhal G, Nogueira P, Silva L, Teles P, Vaz P 2010 Nucl. Instr. Meth. Phys. Res. A 623 1014 Google Scholar
[11]	Mohammadi M A, Abdi M R, Kamali M, Mostajaboddavati M, Zare M R 2011 Appl. Radiat. Isot. 69 521 Google Scholar
[12]	Presler O, German U, Pelled O, Alfassi Z B 2004 Appl. Radiat. Isot. 60 213 Google Scholar
[13]	Mahling S, Orion I, Alfassi Z B 2006 Nucl. Instr. Meth. Phys. Res. A 557 544 Google Scholar
[14]	熊文彬, 仇春华, 段天英, 刘浩杰, 潘君艳, 陈海涛, 刘进辉 2011 原子能科学技术 45 999 Xiong W B, Qiu C H, Duan T Y, Liu H J, Pan J Y, Chen H T, Liu J H 2011 Atomic Energy Sci. Technol. 45 999
[15]	Noteal A 1971 Nucl. Instr. Meth. 91 513 Google Scholar
[16]	田自宁, 欧阳晓平, 曾鸣, 成智威 2013 62 162902 Google Scholar Tian Z N, Ouyang X P, Zeng M, Cheng Z W 2013 Acta Phys. Sin. 62 162902 Google Scholar
[17]	田自宁, 陈伟, 韩斌, 田言杰, 刘文彪, 冯天成, 欧阳晓平 2016 65 062901 Google Scholar Tian Z N, Chen W, Han B, Tian Y J, Liu W B, Feng T C, Ouyang X P 2016 Acta Phys. Sin. 65 062901 Google Scholar

[1]	Xun Zhi-Peng, Hao Da-Peng. Monte Carlo simulation of bond percolation on square lattice with complex neighborhoods. Acta Physica Sinica, 2022, 71(6): 066401. doi: 10.7498/aps.71.20211757
[2]	Wang Li-Min, Duan Bing-Huang, Xu Xian-Guo, Li Hao, Chen Zhi-Jun, Yang Kun-Jie, Zhang Shuo. Simulation of neutron irradiation damage in lead lanthanum zirconate titanate by Monte Carlo method. Acta Physica Sinica, 2022, 71(7): 076101. doi: 10.7498/aps.71.20212041
[3]	Ren Jie, Ruan Xi-Chao, Chen Yong-Hao, Jiang Wei, Bao Jie, Luan Guang-Yuan, Zhang Qi-Wei, Huang Han-Xiong, Wang Zhao-Hui, An Qi, Bai Huai-Yong, Bao Yu, Cao Ping, Chen Hao-Lei, Chen Qi-Ping, Chen Yu-Kai, Chen Zhen, Cui Zeng-Qi, Fan Rui-Rui, Feng Chang-Qing, Gao Ke-Qing, Gu Min-Hao, Han Chang-Cai, Han Zi-Jie, He Guo-Zhu, He Yong-Cheng, Hong Yang, Huang Wei-Ling, Huang Xi-Ru, Ji Xiao-Lu, Ji Xu-Yang, Jiang Hao-Yu, Jiang Zhi-Jie, Jing Han-Tao, Kang Ling, Kang Ming-Tao, Li Bo, Li Chao, Li Jia-Wen, Li Lun, Li Qiang, Li Xiao, Li Yang, Liu Rong, Liu Shu-Bin, Liu Xing-Yan, Mu Qi-Li, Ning Chang-Jun, Qi Bin-Bin, Ren Zhi-Zhou, Song Ying-Peng, Song Zhao-Hui, Sun Hong, Sun Kang, Sun Xiao-Yang, Sun Zhi-Jia, Tan Zhi-Xin, Tang Hong-Qing, Tang Jing-Yu, Tang Xin-Yi, Tian Bin-Bin, Wang Li-Jiao, Wang Peng-Cheng, Wang Qi, Wang Tao-Feng, Wen Jie, Wen Zhong-Wei, Wu Qing-Biao, Wu Xiao-Guang, Wu Xuan, Xie Li-Kun, Yang Yi-Wei, Yi Han, Yu Li, Yu Tao, Yu Yong-Ji, Zhang Guo-Hui, Zhang Lin-Hao, Zhang Xian-Peng, Zhang Yu-Liang, Zhang Zhi-Yong, Zhao Yu-Bin, Zhou Lu-Ping, Zhou Zu-Ying, Zhu Dan-Yang, Zhu Ke-Jun, Zhu Peng. In-beam γ-rays of back-streaming white neutron source at China Spallation Neutron Source. Acta Physica Sinica, 2020, 69(17): 172901. doi: 10.7498/aps.69.20200718
[4]	Tian Zi-Ning, Chen Wei, Han Bin, Tian Yan-Jie, Liu Wen-Biao, Feng Tian-Cheng, Ouyang Xiao-Ping. Study on the virtual source calibration technology based on the volume of radioactive gas source. Acta Physica Sinica, 2016, 65(6): 062901. doi: 10.7498/aps.65.062901
[5]	Chen Yuan, Wang Xiao-Fang, Shao Guang-Chao. Characteristics and parameter optimization of electron beam radiography. Acta Physica Sinica, 2015, 64(15): 154101. doi: 10.7498/aps.64.154101
[6]	Li Wen-Fang, Du Jin-Jin, Wen Rui-Juan, Yang Peng-Fei, Li Gang, Zhang Tian-Cai. Single-atom transfer in a strongly coupled cavity quantum electrodynamics: experiment and Monte Carlo simulation. Acta Physica Sinica, 2014, 63(24): 244205. doi: 10.7498/aps.63.244205
[7]	Hua Yu-Chao, Dong Yuan, Cao Bing-Yang. Monte Carlo simulation of phonon ballistic diffusive heat conduction in silicon nanofilm. Acta Physica Sinica, 2013, 62(24): 244401. doi: 10.7498/aps.62.244401
[8]	Xiao Yuan, Wang Xiao-Fang, Teng Jian, Chen Xiao-Hu, Chen Yuan, Hong Wei. Simulation study of radiography using laser-produced electron beam. Acta Physica Sinica, 2012, 61(23): 234102. doi: 10.7498/aps.61.234102
[9]	Lan Mu, Xiang Gang, Gu Gang-Xu, Zhang Xi. A Monte Carlo simulation study on growth mechanism of horizontal nanowires on crystal surface. Acta Physica Sinica, 2012, 61(22): 228101. doi: 10.7498/aps.61.228101
[10]	Fan Xiao-Hui, Zhao Xing-Yu, Wang Li-Na, Zhang Li-Li, Zhou Heng-Wei, Zhang Jin-Lu, Huang Yi-Neng. Monte Carlo simulations of the relaxation dynamics of the spatial relaxation modes in the molecule-string model. Acta Physica Sinica, 2011, 60(12): 126401. doi: 10.7498/aps.60.126401
[11]	Chen Shan, Wu Qing-Yun, Chen Zhi-Gao, Xu Gui-Gui, Huang Zhi-Gao. Ferromagnetism of C doped ZnO: first-principles calculation and Monte Carlo simulation. Acta Physica Sinica, 2009, 58(3): 2011-2017. doi: 10.7498/aps.58.2011
[12]	Xiong Kai-Guo, Feng Guo-Lin, Hu Jing-Guo, Wan Shi-Quan, Yang Jie. Monte Carlo simulation of the record-breaking high temperature events of climate changes. Acta Physica Sinica, 2009, 58(4): 2843-2852. doi: 10.7498/aps.58.2843
[13]	Gao Fei, Ryoko Yamada, Mitsuo Watanabe, Liu Hua-Feng. Use of Monte Carlo simulations for the scatter events analysis of PET scanners. Acta Physica Sinica, 2009, 58(5): 3584-3591. doi: 10.7498/aps.58.3584
[14]	Xu Lan-Qing, Li Hui, Xiao Zheng-Ying. Discussion on backscattered photon numbers and their scattering events in a turbid media. Acta Physica Sinica, 2008, 57(9): 6030-6035. doi: 10.7498/aps.57.6030
[15]	Lei Xiao-Wei, Zheng Bo, Ying He-Ping. A numerical study on the aging of two-dimensional spin systems. Acta Physica Sinica, 2007, 56(3): 1713-1718. doi: 10.7498/aps.56.1713
[16]	He Qing-Fang, Xu Zheng, Liu De-Ang, Xu Xu-Rong. Monte Carlo simulation of the effect of impact ionization in thin-film electroluminescent devices. Acta Physica Sinica, 2006, 55(4): 1997-2002. doi: 10.7498/aps.55.1997
[17]	Wang Shi-Qi, Lian Gui-Jun, Xiong Guang-Cheng. Electronic transport properties and simulation of random resistor network in granular mixture system of La0.7Ca0.3MnO3 and CeO 2. Acta Physica Sinica, 2005, 54(8): 3815-3821. doi: 10.7498/aps.54.3815
[18]	Wang Zhi-Jun, Dong Li-Fang, Shang Yong. Monte Carlo simulation of optical emission spectra in electron assisted chemical vapor deposition of diamond. Acta Physica Sinica, 2005, 54(2): 880-885. doi: 10.7498/aps.54.880
[19]	Wang Jian-Hua, Jin Chuan-En. Application of Monte Carlo simulation to the research of ions transport plasma sheaths of glow discharge. Acta Physica Sinica, 2004, 53(4): 1116-1122. doi: 10.7498/aps.53.1116
[20]	Guo Zeng-Bao. . Acta Physica Sinica, 2002, 51(10): 2344-2348. doi: 10.7498/aps.51.2344

Catalog

Vol. 68, Issue 23 - 2019-12-05

Metrics

Abstract views: 7516
PDF Downloads: 47
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板